Nanoparticle-Based Retinal Prostheses: The Effect of Shape and Size on Neuronal Coupling
Abstract
:1. Introduction
2. Materials and Methods
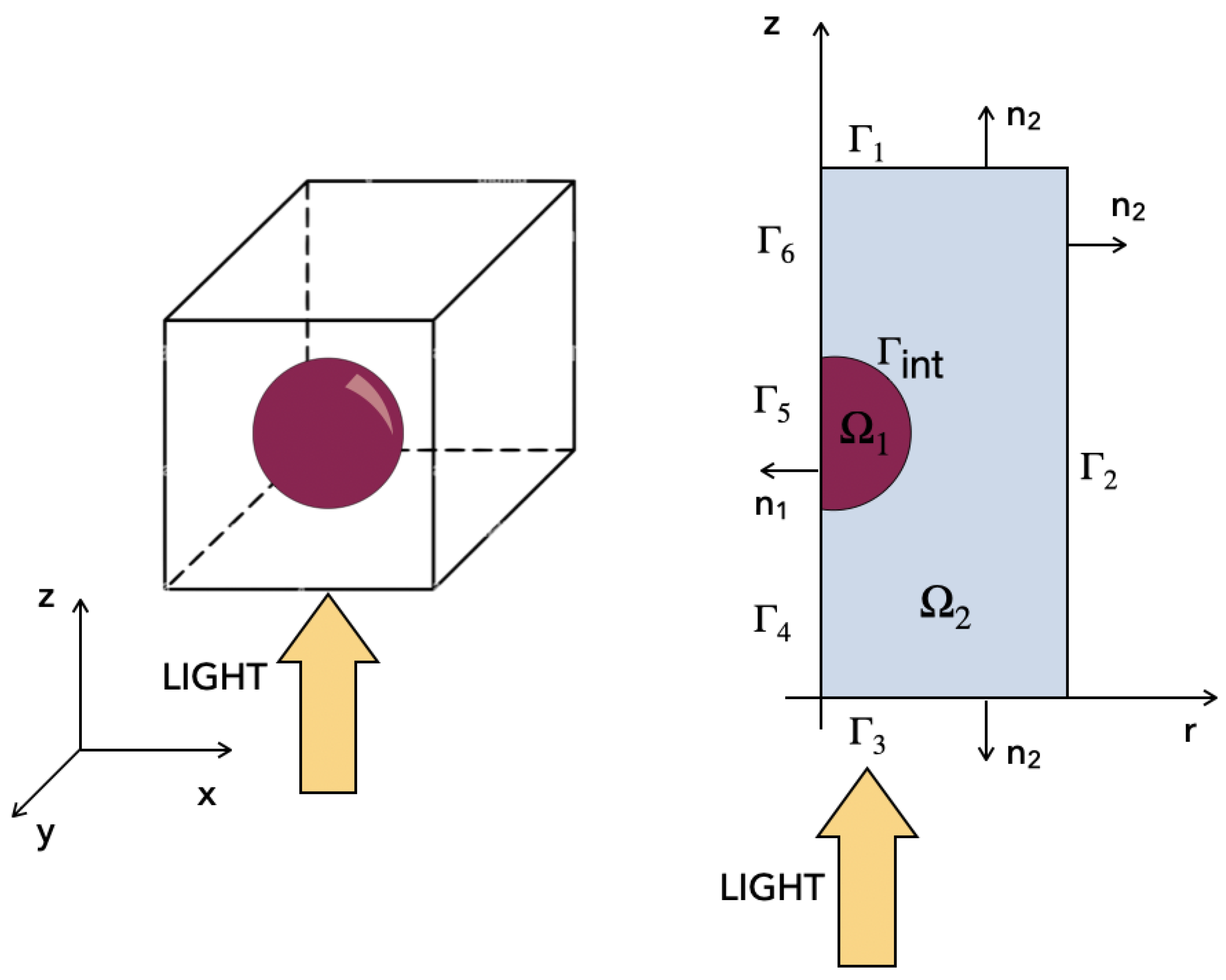
2.1. Geometric Representation of the Bio-Hybrid System
2.2. Model Structure
2.3. Computational Algorithm
- Solve the nonlinear Poisson equation (for given electron, hole, and oxide electrochemical potentials):where is the thermal voltage;
- Solve two linearized continuity equations for n and p (for given electric potential and oxide molar density):
- Solve a linearized continuity equation for (for given electric potential and electron and hole number densities):
- Update the electrochemical potentials for electrons and holes:
- Update the electrochemical potential for the oxide:
- Compute the maximum absolute relative increment:
- Check for convergence:If , then terminate the iteration; otherwise, go back to step 1 and continue.
- Piecewise linear finite elements with mass lumping stabilization ([21], Chapter 22) are used to numerically solve (3a). This prevents the occurrence of unphysical oscillations in the spatial distribution of the electric potential, especially in the neighborhood of material interfaces;
- Piecewise linear finite elements with Scharfetter–Gummel stabilization [22] are used to numerically solve (4a), (4b), and (5). This preserves on the discrete level the strict positivity of the carrier number densities and ion molar density.
3. Results and Discussion
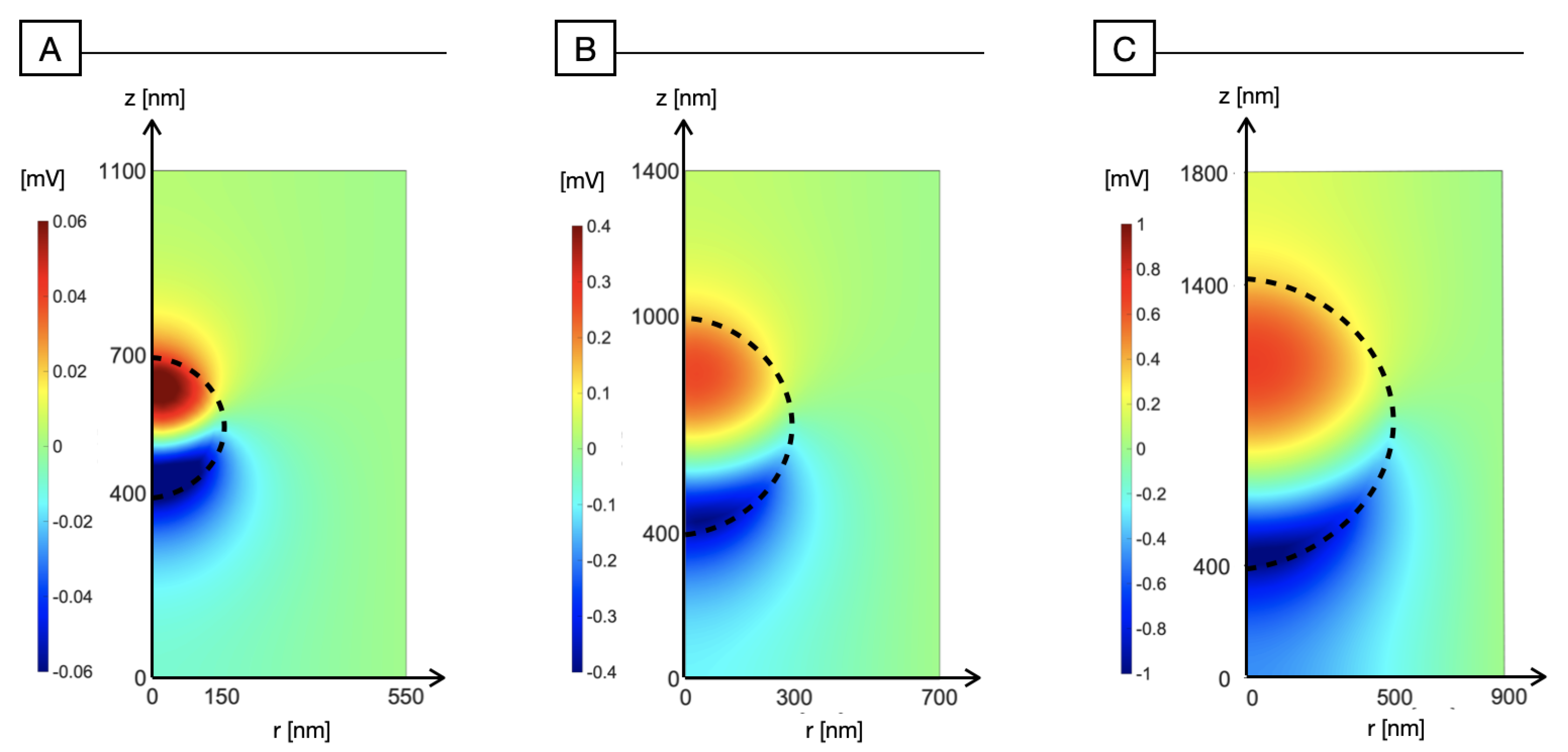
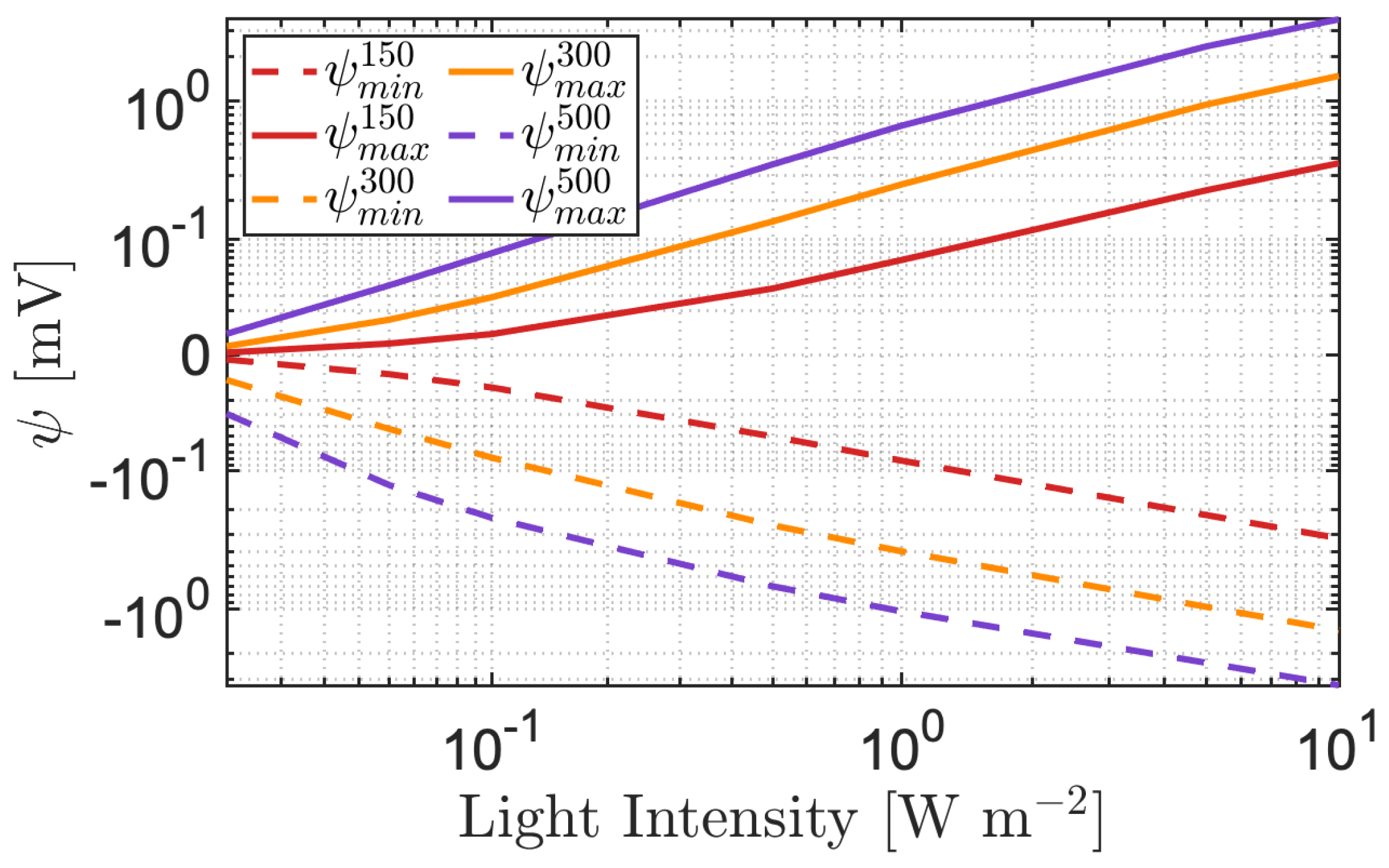
3.1. The Role of Nanoparticle Size
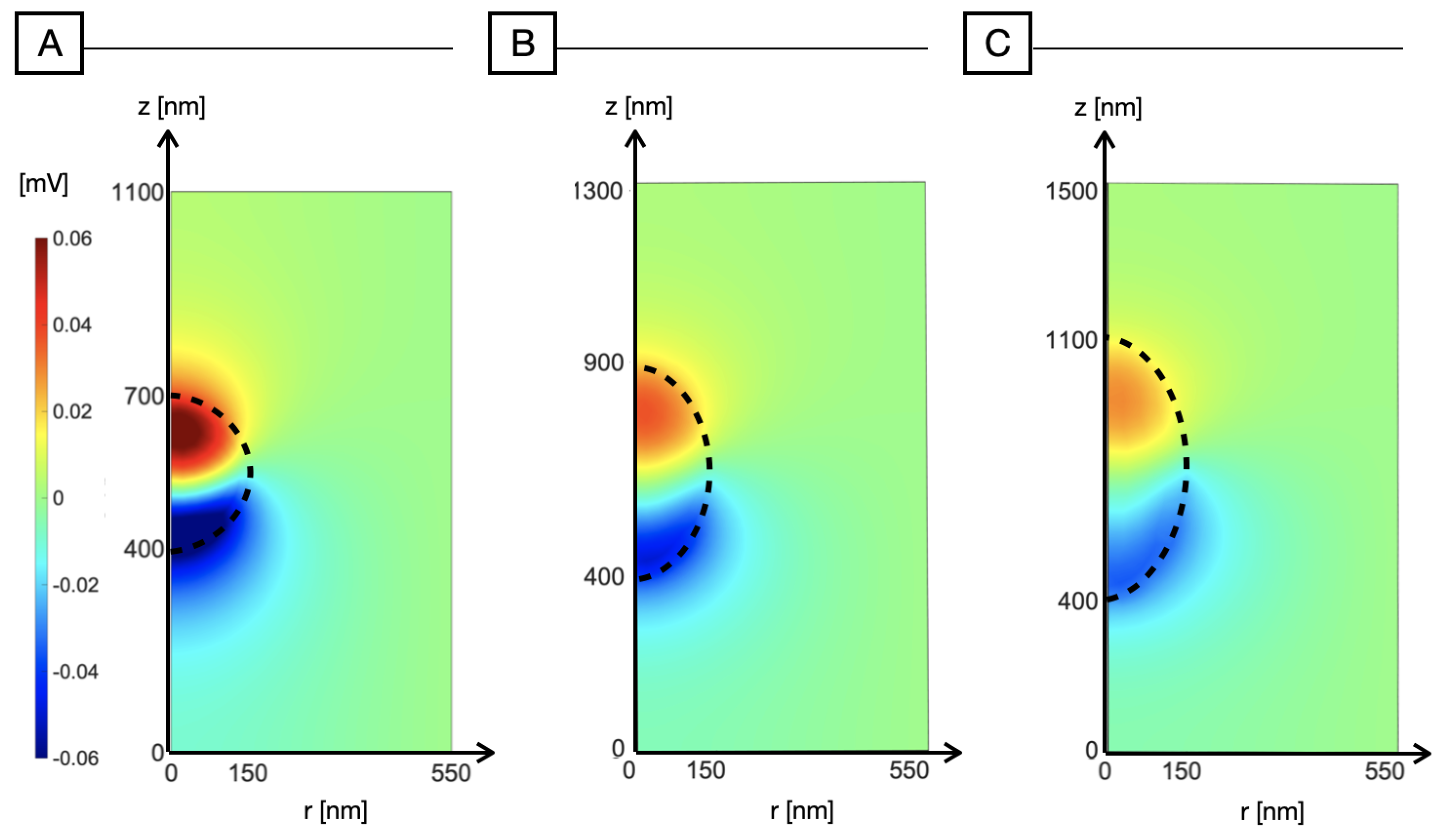
3.2. The Role of NP Ellipticity
3.3. Summary
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Menéndez-Velázquez, A.; Álvarez, C.N.; del Olmo-Aguado, S.; Merayo-Lloves, J.; Fernández-Vega, A.; Osborne, N. Potential application of photoluminescent filters for use in ophthalmology. Opt. Mater. 2018, 86, 505–511. [Google Scholar] [CrossRef]
- Bloch, E.; Luo, Y.; da Cruz, L. Advances in retinal prosthesis systems. Ther. Adv. Ophthalmol. 2019, 11, 251584141881750. [Google Scholar] [CrossRef]
- Luo, Y.H.L.; da Cruz, L. The Argus II Retinal Prosthesis System. Prog. Retin. Eye Res. 2016, 50, 89–107. [Google Scholar] [CrossRef]
- Lorach, H.; Goetz, G.; Smith, R.; Lei, X.; Mandel, Y.; Kamins, T.; Mathieson, K.; Huie, P.; Harris, J.; Sher, A.; et al. Photovoltaic restoration of sight with high visual acuity. Nat. Med. 2015, 21, 476–482. [Google Scholar] [CrossRef] [PubMed]
- Ayton, L.N.; Blamey, P.J.; Guymer, R.H.; Luu, C.D.; Nayagam, D.A.X.; Sinclair, N.C.; Shivdasani, M.N.; Yeoh, J.; McCombe, M.F.; Briggs, R.J.; et al. First-in-human trial of a novel suprachoroidal retinal prosthesis. PLoS ONE 2014, 9, e115239. [Google Scholar] [CrossRef] [PubMed]
- Zrenner, E.; Bartz-Schmidt, K.U.; Benav, H.; Besch, D.; Bruckmann, A.; Gabel, V.P.; Gekeler, F.; Greppmaier, U.; Harscher, A.; Kibbel, S.; et al. Subretinal electronic chips allow blind patients to read letters and combine them to words. Proc. R. Soc. Biol. Sci. 2010, 278, 1489–1497. [Google Scholar] [CrossRef]
- Palanker, D.; Vankov, A.; Huie, P.; Baccus, S. Design of a high-resolution optoelectronic retinal prosthesis. J. Neural. Eng. 2005, 2, S105–S120. [Google Scholar] [CrossRef]
- Manfredi, G.; Colombo, E.; Barsotti, J.; Benfenati, F.; Lanzani, G. Photochemistry of Organic Retinal Prostheses. Annu. Rev. Phys. Chem. 2019, 70, 99–121. [Google Scholar] [CrossRef]
- Maya-Vetencourt, J.F.; Ghezzi, D.; Antognazza, M.R.; Colombo, E.; Mete, M.; Feyen, P.; Desii, A.; Buschiazzo, A.; Di Paolo, M.; Di Marco, S.; et al. A fully organic retinal prosthesis restores vision in a rat model of degenerative blindness. Nat. Mater. 2017, 16, 681–689. [Google Scholar] [CrossRef]
- Maya-Vetencourt, J.F.; Manfredi, G.; Mete, M.; Colombo, E.; Bramini, M.; Di Marco, S.; Shmal, D.; Mantero, G.; Dipalo, M.; Rocchi, A.; et al. Subretinally injected semiconducting polymer nanoparticles rescue vision in a rat model of retinal dystrophy. Nat. Nanotechnol. 2020, 15, 698–708. [Google Scholar] [CrossRef]
- Francia, S.; Schmal, D.; Di Marco, S.; Chiaravalli, G.; Maya-Vetencourt, J.F.; Mantero, G.; Michetti, C.; Cupini, S.; Manfredi, G.; DiFrancesco, M.L.; et al. Light-induced charge generation in polymeric nanoparticles restores vision in advanced-stage retinitis pigmentosa rats. Nat. Commun. 2022, 13, 3677. [Google Scholar] [CrossRef] [PubMed]
- Chiaravalli, G.; Manfredi, G.; Sacco, R.; Lanzani, G. Photoelectrochemistry and Drift-Diffusion Simulations in a Polythiophene Film Interfaced with an Electrolyte. ACS Appl. Mater. Interfaces 2021, 13, 36595–36604. [Google Scholar] [CrossRef] [PubMed]
- Li, L.; Li, C.; Zhang, Z.; Alexov, E. On the Dielectric “Constant” of Proteins: Smooth Dielectric Function for Macromolecular Modeling and Its Implementation in DelPhi. J. Chem. Theory Comput. 2013, 9, 2126–2136. [Google Scholar] [CrossRef] [PubMed]
- Amin, M.; Küpper, J. Variations in Proteins Dielectric Constants. Chem. Open 2020, 9, 691–694. [Google Scholar]
- Roosbroeck, W.V. Theory of flow of electrons and holes in germanium and other semiconductors. Bell Syst. Tech. J. 1950, 29, 560–607. [Google Scholar] [CrossRef]
- Shockley, W.; Read, W.T. Statistics of the Recombinations of Holes and Electrons. Phys. Rev. 1952, 87, 835–842. [Google Scholar] [CrossRef]
- Rubinstein, I. Electrodiffusion of Ions; Society for Industrial and Applied Mathematics (SIAM): Philadelphia, PA, USA, 1990. [Google Scholar]
- Gummel, H. A self-consistent iterative scheme for one-dimensional steady state transistor calculations. IEEE Trans. Electron Devices 1964, 11, 455–465. [Google Scholar] [CrossRef]
- Markowich, P.A. The Stationary Semiconductor Device Equations; Computational Microelectronics; Springer: Vienna, Austria, 1986. [Google Scholar]
- Jerome, J.W. Analysis of Charge Transport; Springer: Berlin/Heidelbrg, Germany, 1996. [Google Scholar]
- Sacco, R.; Guidoboni, G.; Mauri, A.G. A Comprehensive Physically Based Approach to Modeling in Bioengineering and Life Sciences, 1st ed.; Elsevier: Amsterdam, The Netherlands; Academic Press: Cambridge, MA, USA, 2019. [Google Scholar]
- Scharfetter, D.; Gummel, H. Large-signal analysis of a silicon Read diode oscillator. IEEE Trans. Electron Devices 1969, 16, 64–77. [Google Scholar] [CrossRef]
- Goldman, S.R.; Kalikstein, K.; Kramer, B. Dember-effect theory. J. Appl. Phys. 1978, 49, 2849–2854. [Google Scholar] [CrossRef]
- Wu, X.; Park, H.; Zhu, X.-Y. Probing Transient Electric Fields in Photoexcited Organic Semiconductor Thin Films and Interfaces by Time-Resolved Second Harmonic Generation. J. Phys. Chem. C 2014, 118, 10670–10676. [Google Scholar] [CrossRef]
- Helmut, S. Hydrogen peroxide as a central redox signaling molecule in physiological oxidative stress: Oxidative eustress. Redox Biol. 2017, 11, 613–619. [Google Scholar]
- Aziz, I.A.; Malferrari, M.; Roggiani, F.; Tullii, G.; Rapino, S.; Antognazza, M.R. Light-Triggered Electron Transfer between a Conjugated Polymer and Cytochrome C for Optical Modulation of Redox Signaling. iScience 2020, 23, 101091. [Google Scholar] [CrossRef] [PubMed]
- Bossio, C.; Aziz, I.A.; Tullii, G.; Zucchetti, E.; Debellis, D.; Zangoli, M.; Maria, F.D.; Lanzani, G.; Antognazza, M.R. Photocatalytic Activity of Polymer Nanoparticles Modulates Intracellular Calcium Dynamics and Reactive Oxygen Species in HEK-293 Cells. Front. Bioeng. Biotechnol. 2018, 6, 114. [Google Scholar] [CrossRef] [PubMed]
- Abdullaeva, O.S.; Sahalianov, I.; Ejneby, M.S.; Jakešová, M.; Zozoulenko, I.; Liin, S.I.; Głowacki, E.D. Faradaic Pixels for Precise Hydrogen Peroxide Delivery to Control M-Type Voltage-Gated Potassium Channels. Adv. Sci. 2021, 9, 2103132. [Google Scholar] [CrossRef]
- Arnault, E.; Barrau, C.; Nanteau, C.; Gondouin, P.; Bigot, K.; Vienot, F.; Gutman, E.; Fontaine, V.; Villette, T.; Cohen-Tannoudji, D.; et al. Phototoxic Action Spectrum on a Retinal Pigment Epithelium Model of Age-Related Macular Degeneration Exposed to Sunlight Normalized Conditions. PLoS ONE 2013, 8, e71398. [Google Scholar] [CrossRef]


| 150 nm | 300 nm | 500 nm | |
|---|---|---|---|
| [A] | 1.23 · 10 | 7.57 · 10 | 2.96 · 10 |
| 12% | 3% | 1% | |
| 88% | 97% | 99% |
| r = r1 = r2 | r = 150 nm | r = 300 nm | r = 500 nm |
|---|---|---|---|
| −0.082 | −0.398 | −1.04 | |
| 0.067 | 0.260 | 0.676 | |
| r = 150 nm | r = 150 nm | r = 250 nm | r = 350 nm |
| −0.082 | −0.050 | −0.035 | |
| 0.067 | 0.037 | 0.029 | |
| r = 150 nm | r = 150 nm | r = 250 nm | r = 350 nm |
| −0.082 | −0.102 | −0.162 | |
| 0.067 | 0.044 | 0.042 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chiaravalli, G.; Lanzani, G.; Sacco, R. Nanoparticle-Based Retinal Prostheses: The Effect of Shape and Size on Neuronal Coupling. Photonics 2022, 9, 710. https://doi.org/10.3390/photonics9100710
Chiaravalli G, Lanzani G, Sacco R. Nanoparticle-Based Retinal Prostheses: The Effect of Shape and Size on Neuronal Coupling. Photonics. 2022; 9(10):710. https://doi.org/10.3390/photonics9100710
Chicago/Turabian StyleChiaravalli, Greta, Guglielmo Lanzani, and Riccardo Sacco. 2022. "Nanoparticle-Based Retinal Prostheses: The Effect of Shape and Size on Neuronal Coupling" Photonics 9, no. 10: 710. https://doi.org/10.3390/photonics9100710
APA StyleChiaravalli, G., Lanzani, G., & Sacco, R. (2022). Nanoparticle-Based Retinal Prostheses: The Effect of Shape and Size on Neuronal Coupling. Photonics, 9(10), 710. https://doi.org/10.3390/photonics9100710





