Optical Solitons and Vortices in Fractional Media: A Mini-Review of Recent Results
Abstract
:1. Introduction and the Basic Models
2. Basic Soliton Families in the One-Dimensional Fractional Medium
2.1. Stationary States and Analysis of Their Stability
2.2. The Quasi-Local Approximation for Modes Carried by a Rapidly Oscillating Continuous Wave
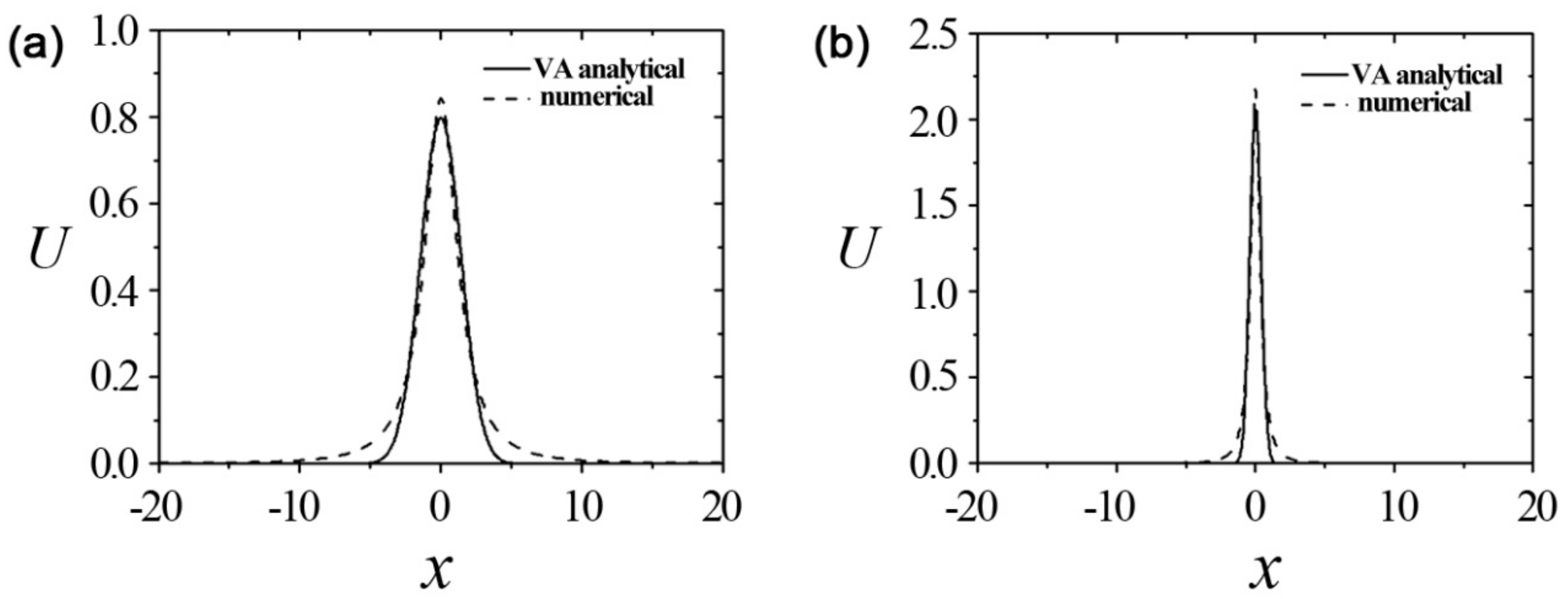
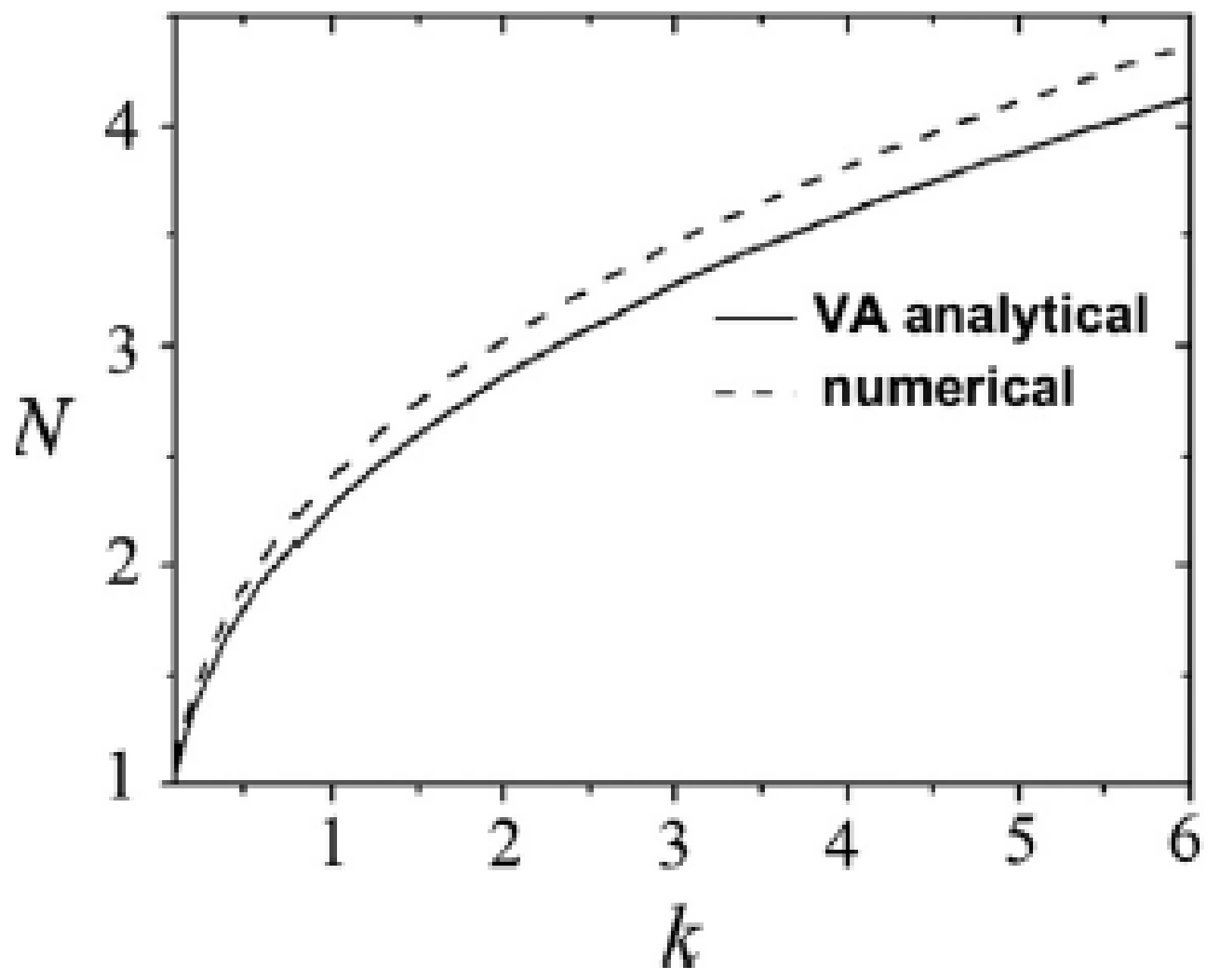
2.3. The Scaling Relation and Variational Approximation (VA) for Soliton Families
2.4. Numerical Findings
3. Further Results for Nonlinear Modes in One-Dimensional Fractional Waveguides
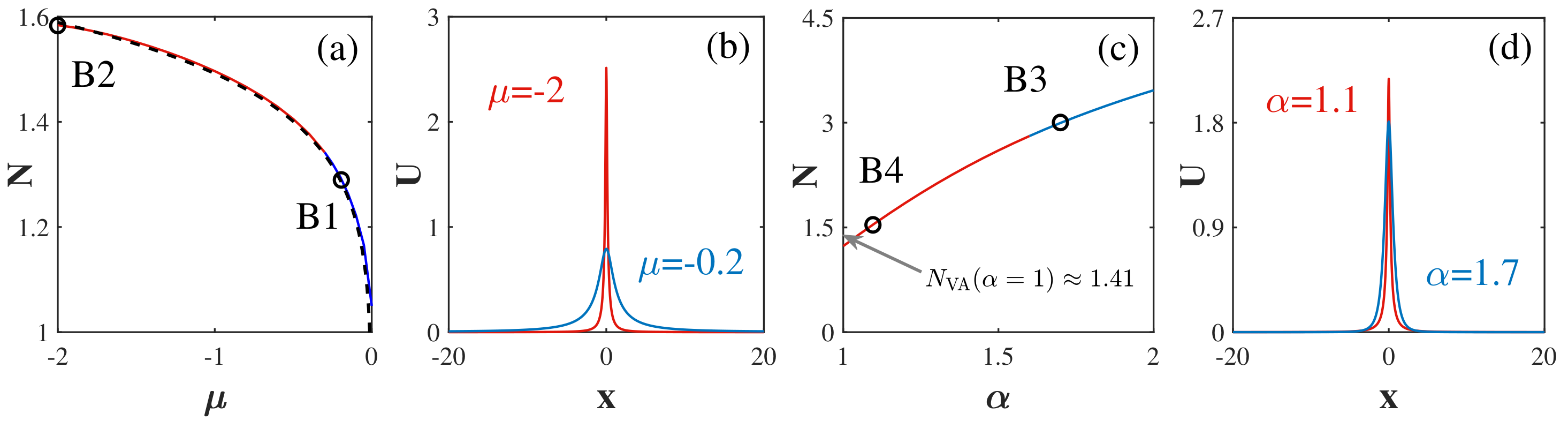
3.1. Systems with Trapping Potentials
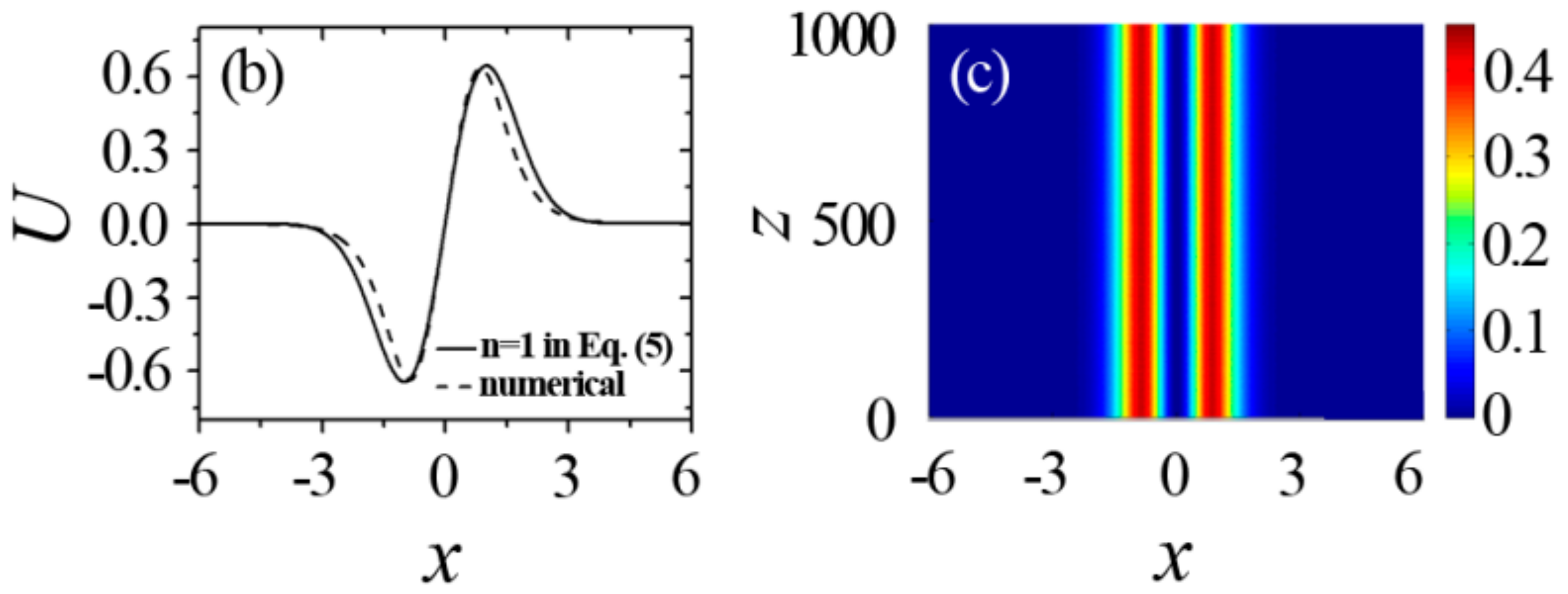
3.2. Dissipative Solitons Produced by the Fractional Complex Ginzburg-Landau Equation (CGLE)
4. Vortex Modes in Two-Dimensional (2D) Fractional-Diffraction Settings
4.1. Stationary Vortex Solitons
4.2. Dynamics of Vortical Clusters
4.3. Stabilization of 2D Solitons by the Trapping Potential
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Kivshar, Y.S.; Malomed, B.A. Dynamics of solitons in nearly integrable systems. Rev. Mod. Phys. 1989, 61, 763–915. [Google Scholar] [CrossRef]
- Malomed, B.A.; Mihalache, D.; Wise, F.; Torner, L. Spatiotemporal optical solitons. J. Opt. B 2005, 7, R53–R72. [Google Scholar] [CrossRef]
- Kartashov, Y.V.; Malomed, B.A.; Torner, L. Solitons in nonlinear lattices. Rev. Mod. Phys. 2011, 83, 247–306. [Google Scholar] [CrossRef]
- Chen, Z.; Segev, M.; Christodoulides, D.N. Optical spatial solitons: Historical overview and recent advances. Rep. Prog. Phys. 2012, 75, 086401. [Google Scholar] [CrossRef] [PubMed]
- Malomed, B.A. Multidimensional solitons: Well-established results and novel findings. Eur. Phys. J. Spec. Top. 2016, 225, 2507–2532. [Google Scholar] [CrossRef] [Green Version]
- Kartashov, Y.V.; Astrakharchik, G.E.; Malomed, B.A.; Torner, L. Frontiers in multidimensional self-trapping of nonlinear fields and matter. Nat. Rev. Phys. 2019, 1, 185–197. [Google Scholar] [CrossRef]
- Malomed, B.A. (INVITED) Vortex solitons: Old results and new perspectives. Physica D 2019, 399, 108–137. [Google Scholar] [CrossRef] [Green Version]
- Mihalache, D. Localized structures in optical and matter-wave media: A selection of recent studies. Rom. Rep. Phys. 2021, 73, 403. [Google Scholar]
- Laskin, N. Fractional quantum mechanics and Lévy path integrals. Phys. Lett. A 2000, 268, 298–305. [Google Scholar] [CrossRef] [Green Version]
- Laskin, N. Fractional Quantum Mechanics; World Scientific: Singapore, 2018. [Google Scholar]
- Stickler, B.A. Potential condensed-matter realization of space-fractional quantum mechanics: The one-dimensional Lévy crystal. Phys. Rev. E 2013, 88, 012120. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pinsker, F.; Bao, W.; Zhang, Y.; Ohadi, H.; Dreismann, A.; Baumberg, J. Fractional quantum mechanics in polariton condensates with velocity-dependent mass. Phys. Rev. B 2015, 92, 195310. [Google Scholar] [CrossRef] [Green Version]
- Longhi, S. Fractional Schrödinger equation in optics. Opt. Lett. 2015, 40, 1117–1120. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Liu, X.; Belić, M.R.; Zhong, W.; Zhang, Y.; Xiao, M. Propagation dynamics of a light beam in a fractional Schrödinger equation. Phys. Rev. Lett. 2015, 115, 180403. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Zhong, H.; Belić, M.R.; Zhu, Y.; Zhong, W.; Zhang, Y.; Christodoulides, D.N.; Xiao, M. PT symmetry in a fractional Schrödinger equation. Laser Photonics Rev. 2016, 10, 526–531. [Google Scholar] [CrossRef] [Green Version]
- Li, P.; Malomed, B.A.; Mihalache, D. Symmetry-breaking bifurcations and ghost states in the fractional nonlinear Schrödinger equation with a PT-symmetric potential. Opt. Lett. 2021, 46, 3267–3270. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; He, Z.; Conti, C.; Wang, Z.; Hu, Y.; Lei, D.; Li, Y.; Fan, A.D. Modulational instability in fractional nonlinear Schrödinger equation. Commun. Nonlinear Sci. Numer. Simul. 2017, 48, 531–540. [Google Scholar] [CrossRef]
- Secchi, S.; Squassina, M. Soliton dynamics for fractional Schrödinger equations. Appl. Anal. 2014, 93, 1702–1729. [Google Scholar] [CrossRef] [Green Version]
- Duo, S.; Zhang, Y. Mass-conservative Fourier spectral methods for solving the fractional nonlinear Schrödinger equation. Comput. Math. Appl. 2016, 71, 2257–2271. [Google Scholar] [CrossRef] [Green Version]
- Zhong, W.P.; Belić, M.R.; Malomed, B.A.; Zhang, Y.; Huang, T. Spatiotemporal accessible solitons in fractional dimensions. Phys. Rev. E 2016, 94, 012216. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhong, W.P.; Belić, M.R.; Zhang, Y. Accessible solitons of fractional dimension. Ann. Phys. 2016, 368, 110–116. [Google Scholar] [CrossRef]
- Hong, Y.; Sire, Y. A new class of traveling solitons for cubic fractional nonlinear Schrödinger equations. Nonlinearity 2017, 30, 1262–1286. [Google Scholar] [CrossRef] [Green Version]
- Chen, M.; Zeng, S.; Lu, D.; Hu, W.; Guo, Q. Optical solitons, self-focusing, and wave collapse in a space-fractional Schrödinger equation with a Kerr-type nonlinearity. Phys. Rev. E 2018, 98, 022211. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.; Li, J.; Zhang, L.; Xie, W. Hermite-Gaussian-like soliton in the nonlocal nonlinear fractional Schrödinger equation. EPL 2018, 122, 64001. [Google Scholar] [CrossRef]
- Wang, Q.; Deng, Z.Z. Elliptic Solitons in (1+2)-dimensional anisotropic nonlocal nonlinear fractional Schrödinger equation. IEEE Photonics J. 2019, 11, 1–8. [Google Scholar] [CrossRef]
- Huang, C.; Dong, L. Gap solitons in the nonlinear fractional Schrödinger equation with an optical lattice. Opt. Lett. 2016, 41, 5636–5639. [Google Scholar] [CrossRef]
- Xiao, J.; Tian, Z.; Huang, C.; Dong, L. Surface gap solitons in a nonlinear fractional Schrödinger equation. Opt. Express 2018, 26, 2650–2658. [Google Scholar] [CrossRef]
- Zhang, L.F.; Zhang, X.; Wu, H.Z.; Li, C.X.; Pierangeli, D.; Gao, Y.X.; Fan, D.Y. Anomalous interaction of Airy beams in the fractional nonlinear Schrödinger equation. Opt. Express 2019, 27, 27936–27945. [Google Scholar] [CrossRef] [PubMed]
- Dong, L.; Tian, Z. Truncated-Bloch-wave solitons in nonlinear fractional periodic systems. Ann. Phys. 2019, 404, 57–64. [Google Scholar] [CrossRef]
- Zeng, L.; Zeng, J. One-dimensional gap solitons in quintic and cubic-quintic fractional nonlinear Schrödinger equations with a periodically modulated linear potential. Nonlinear Dyn. 2019, 98, 985–995. [Google Scholar] [CrossRef] [Green Version]
- Li, P.; Malomed, B.A.; Mihalache, D. Vortex solitons in fractional nonlinear Schrödinger equation with the cubic-quintic nonlinearity. Chaos Solitons Fractals 2020, 137, 109783. [Google Scholar] [CrossRef]
- Wang, Q.; Liang, G. Vortex and cluster solitons in nonlocal nonlinear fractional Schrödinger equation. J. Optics 2020, 22, 055501. [Google Scholar] [CrossRef]
- Zeng, L.; Zeng, J. One-dimensional solitons in fractional Schrödinger equation with a spatially periodical modulated nonlinearity: Nonlinear lattice. Opt. Lett. 2019, 44, 2661–2664. [Google Scholar] [CrossRef]
- Qiu, Y.; Malomed, B.A.; Mihalache, D.; Zhu, X.; Peng, X.; He, Y. Stabilization of single- and multi-peak solitons in the fractional nonlinear Schrödinger equation with a trapping potential. Chaos Solitons Fractals 2020, 140, 110222. [Google Scholar] [CrossRef]
- Li, P.; Malomed, B.A.; Mihalache, D. Metastable soliton necklaces supported by fractional diffraction and competing nonlinearities. Opt. Exp. 2020, 28, 34472–34488. [Google Scholar] [CrossRef]
- Zeng, L.; Mihalache, D.; Malomed, B.A.; Lu, X.; Cai, Y.; Zhu, Q.; Li, J. Families of fundamental and multipole solitons in a cubic-quintic nonlinear lattice in fractional dimension. Chaos Solitons Fractals 2021, 144, 110589. [Google Scholar] [CrossRef]
- Zeng, L.; Zeng, J. Preventing critical collapse of higher-order solitons by tailoring unconventional optical diffraction and nonlinearities. Commun. Phys. 2020, 3, 26. [Google Scholar] [CrossRef]
- Molina, M.I. The fractional discrete nonlinear Schrödinger equation. Phys. Lett. A 2020, 384, 126180. [Google Scholar] [CrossRef] [Green Version]
- Qiu, Y.; Malomed, B.A.; Mihalache, D.; Zhu, X.; Zhang, L.; He, Y. Soliton dynamics in a fractional complex Ginzburg-Landau model. Chaos Solitons Fractals 2020, 131, 109471. [Google Scholar] [CrossRef] [Green Version]
- Li, P.; Li, J.; Han, B.; Ma, H.; Mihalache, D.D. PT-symmetric optical modes and spontaneous symmetry breaking in the space-fractional Schrödinger equation. Rom. Rep. Phys. 2019, 71, 106. [Google Scholar]
- Li, P.; Dai, C. Double Loops and Pitchfork Symmetry Breaking Bifurcations of Optical Solitons in Nonlinear Fractional Schrödinger Equation with Competing Cubic-Quintic Nonlinearities. Ann. Phys. 2020, 532, 2000048. [Google Scholar] [CrossRef]
- Li, P.; Malomed, B.A.; Mihalache, D. Symmetry breaking of spatial Kerr solitons in fractional dimension. Chaos Solitons Fractals 2020, 109602, 132. [Google Scholar] [CrossRef] [Green Version]
- Zeng, L.; Zeng, J. Fractional quantum couplers. Chaos Solitons Fractals 2020, 140, 110271. [Google Scholar] [CrossRef]
- Zeng, L.; Shi, J.; Lu, X.; Cai, Y.; Zhu, Q.; Chen, H.; Long, H.; Li, J. Stable and oscillating solitons of PT-symmetric couplers with gain and loss in fractional dimension. Nonlinear Dyn. 2021, 103, 1831–1840. [Google Scholar] [CrossRef]
- Cai, M.; Li, C.P. On Riesz derivative. Fract. Calc. Appl. Anal. 2019, 22, 287–301. [Google Scholar] [CrossRef]
- Petrov, D.S.; Astrakharchik, G.E. Ultradilute low-dimensional liquids. Phys. Rev. Lett. 2016, 117, 100401. [Google Scholar] [CrossRef] [Green Version]
- Zeng, L.; Zhu, Y.; Malomed, B.A.; Mihalache, D.; Wang, Q.; Long, H.; Cai, Y.; Lu, X.; Li, J. Quadratic fractional solitons. To be published.
- Li, P.; Li, R.; Dai, C. Existence, symmetry breaking bifurcation and stability of two-dimensional optical solitons supported by fractional diffraction. Opt. Exp. 2021, 29, 3193–3210. [Google Scholar] [CrossRef]
- Vakhitov, N.G.; Kolokolov, A.A. Stationary solutions of the wave equation in a medium with nonlinearity saturation. Radiophys. Quantum Electron. 1973, 16, 783–789. [Google Scholar] [CrossRef]
- Bergé, L. Wave collapse in physics: Principles and applications to light and plasma waves. Phys. Rep. 1998, 303, 259–370. [Google Scholar] [CrossRef]
- Zeng, L.; Malomed, B.A.; Mihalache, D.; Cai, Y.; Lu, X.; Zhu, Q.; Li, J. Bubbles and W-shaped solitons in Kerr media with fractional diffraction. Nonlinear Dyn. 2021, 104, 4253–4264. [Google Scholar] [CrossRef]
- Muslih, S.I.; Agrawal, O.P.; Baleanu, D. A Fractional Schrödinger equation and its solution. Int. J. Theor. Phys. 2010, 49, 1746–1752. [Google Scholar] [CrossRef]
- Desaix, M.; Anderson, D.; Lisak, M. Variational approach to collapse of optical pulses. J. Opt. Soc. Am. B 1991, 8, 2082–2086. [Google Scholar] [CrossRef]
- Alexander, T.J.; Bergé, L. Ground states and vortices of matter-wave condensates and optical guided waves. Phys. Rev. E 2001, 65, 026611. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mihalache, D.; Mazilu, D.; Malomed, B.A.; Lederer, F. Vortex stability in nearly two-dimensional Bose-Einstein condensates with attraction. Phys Rev. A 2006, 73, 043615. [Google Scholar] [CrossRef] [Green Version]
- Malomed, B.A. Spontaneous Symmetry Breaking, Self-Trapping, and Josephson Oscillations; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- He, S.; Malomed, B.A.; Mihalache, D.; Peng, X.; Yu, X.; He, Y.; Den, D. Propagation dynamics of abruptly autofocusing circular Airy Gaussian vortex beams in the fractional Schrödinger equation. Chaos Solitons Fractals 2021, 142, 110470. [Google Scholar] [CrossRef]
- He, S.; Malomed, B.A.; Mihalache, D.; Peng, X.; He, Y.; Deng, D. Propagation dynamics of radially polarized symmetric Airy beams in the fractional Schrödinger equation. Phys. Lett. A 2021, 404, 127403. [Google Scholar] [CrossRef]
- Pushkarov, K.I.; Pushkarov, D.I.; Tomov, I.V. Self-action of light beams in nonlinear media: Soliton solutions. Opt. Quant. Electr. 1979, 11, 471–478. [Google Scholar] [CrossRef]
- Mihalache, D.; Mazilu, D.; Crasovan, L.-C.; Towers, I.; Buryak, A.V.; Malomed, B.A.; Torner, L.; Torres, J.P.; Lederer, F. Stable spinning optical solitons in three dimensions. Phys. Rev. Lett. 2002, 88, 073902. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kruglov, V.I.; Vlasov, R.A. Spiral self-trapping propagation of optical beams. Phys. Lett. A 1985, 111, 401–404. [Google Scholar] [CrossRef]
- Kruglov, V.I.; Logvin, Y.A.; Volkov, V.M. The theory of spiral laser beams in nonlinear media. J. Mod. Opt. 1992, 39, 2277–2291. [Google Scholar] [CrossRef]
- Qin, J.; Dong, G.; Malomed, B.A. Stable giant vortex annuli in microwave-coupled atomic condensates. Phys. Rev. A 2016, 94, 053611. [Google Scholar] [CrossRef] [Green Version]
- Soljaćić, M.; Segev, M. Integer and fractional angular momentum borne on self-trapped necklace-ring beams. Phys. Rev. Lett. 2001, 86, 420–423. [Google Scholar] [CrossRef] [Green Version]
- Desyatnikov, A.S.; Kivshar, Y.S. Necklace-ring vector solitons. Phys. Rev. Lett. 2001, 87, 033901. [Google Scholar] [CrossRef]
- Kartashov, Y.V.; Crasovan, L.-C.; Mihalache, D.; Torner, L. Robust propagation of two-color soliton clusters supported by competing nonlinearities. Phys. Rev. Lett. 2002, 89, 273902. [Google Scholar] [CrossRef] [Green Version]
- Mihalache, D.; Mazilu, D.; Crasovan, L.-C.; Malomed, B.A.; Lederer, F.; Torner, L. Robust soliton clusters in media with competing cubic and quintic nonlinearities. Phys. Rev. E 2003, 68, 046612. [Google Scholar] [CrossRef] [Green Version]
- Chen, Z.; Li, Y.; Malomed, B.A.; Salasnich, L. Spontaneous symmetry breaking of fundamental states, vortices, and dipoles in two and one-dimensional linearly coupled traps with cubic self-attraction. Phys. Rev. A 2016, 96, 033621. [Google Scholar] [CrossRef] [Green Version]
- Thirouin, J. On the growth of Sobolev norms of solutions of the fractional defocusing NLS equation on the circle. Ann. Inst. Henri Poincare 2017, AN34, 509–531. [Google Scholar] [CrossRef]
- Zeng, L.; Malomed, B.A.; Mihalache, D.; Cai, Y.; Lu, X.; Zhu, Q.; Li, J. Flat-floor bubbles, dark solitons, and vortices stabilized by inhomogeneous nonlinear media. Nonlinear Dyn. To be published.
- Fujioka, J.; Espinosa, A.; Rodríguez, R.F. Fractional optical solitons. Phys. Lett. A 2010, 374, 1126–1134. [Google Scholar] [CrossRef]
- Fujioka, J.; Espinosa, A.; Rodríguez, R.F.; Malomed, B.A. Radiating subdispersive fractional optical solitons. Chaos 2014, 24, 033121. [Google Scholar] [CrossRef] [PubMed]

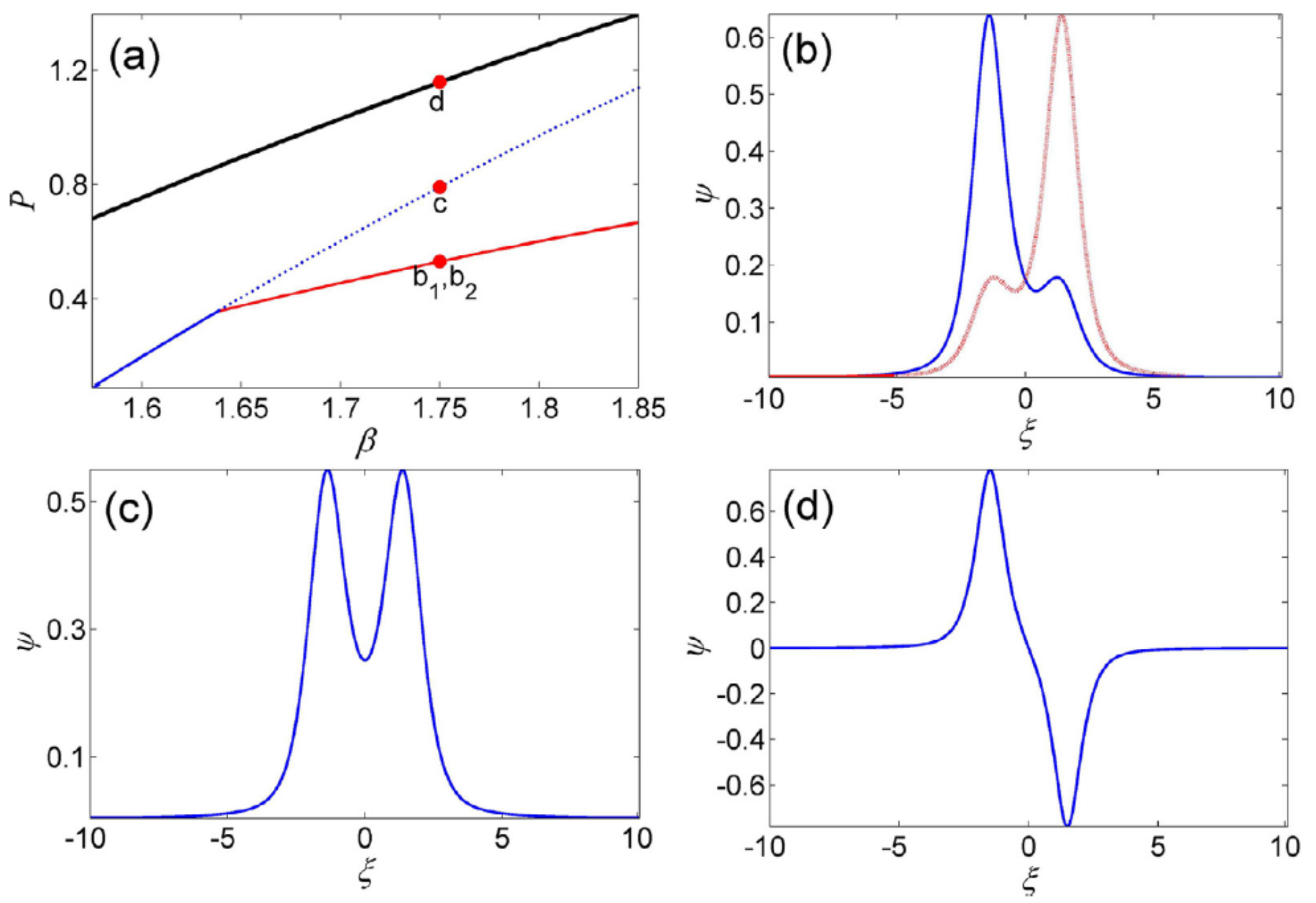
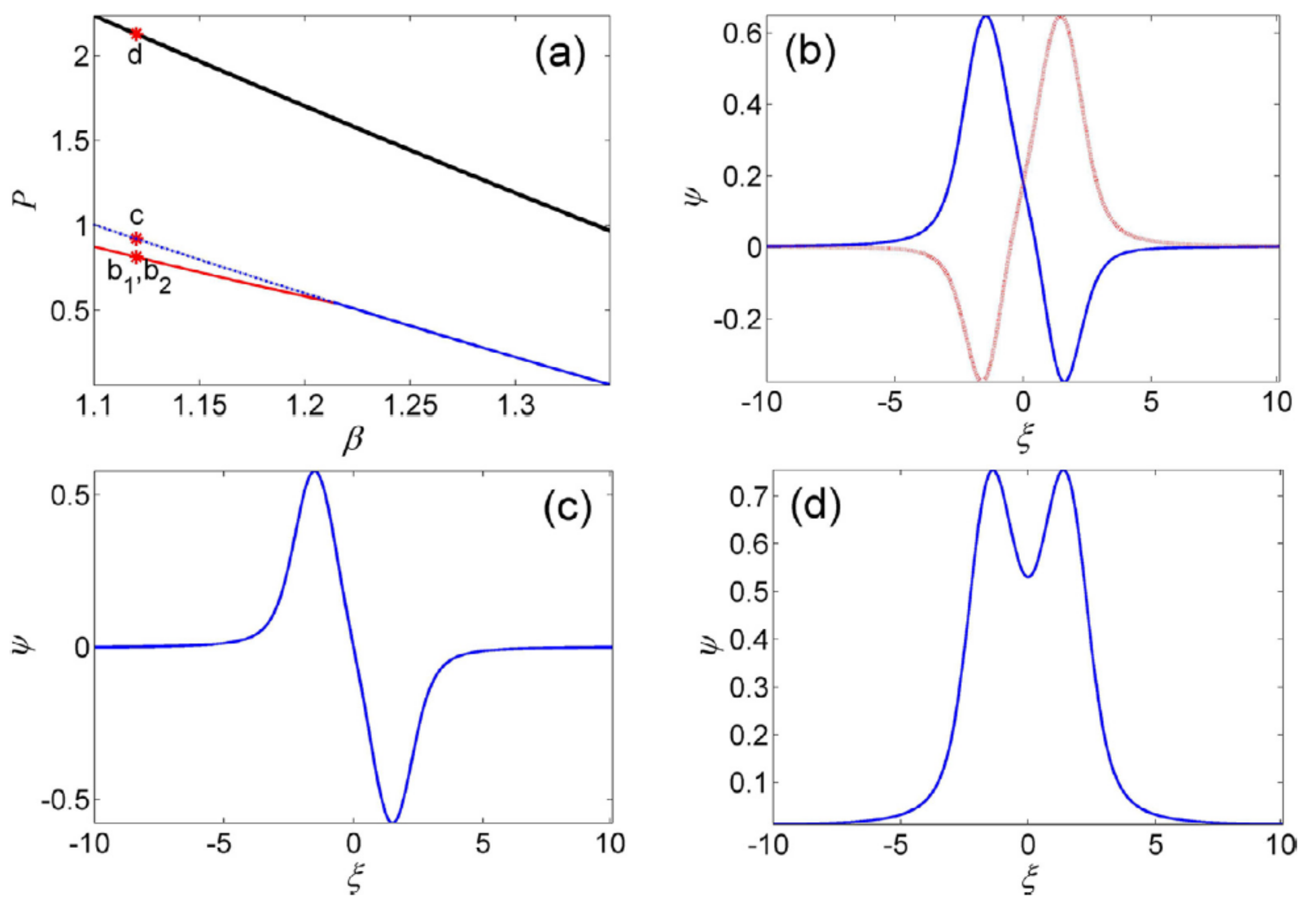


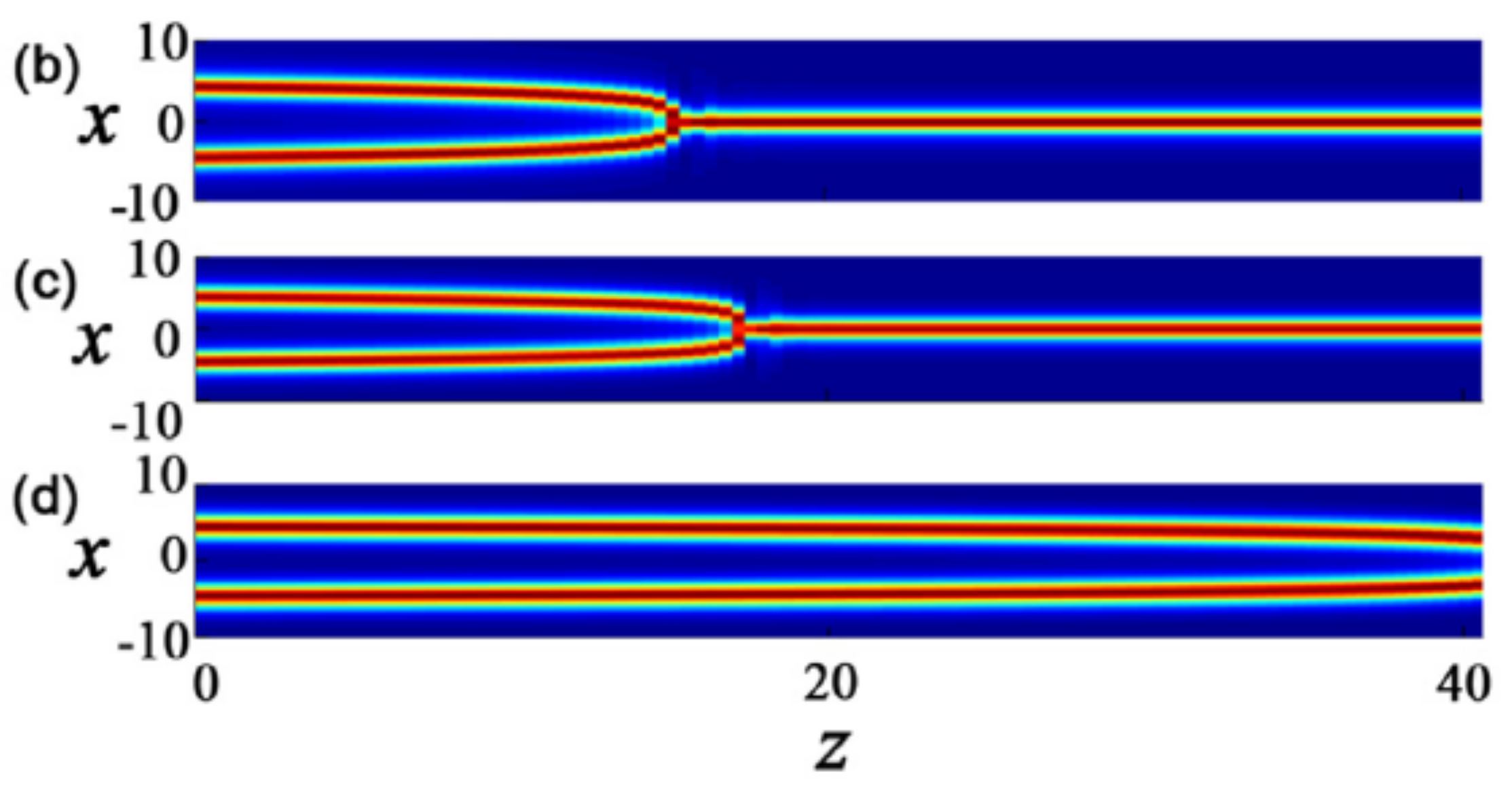
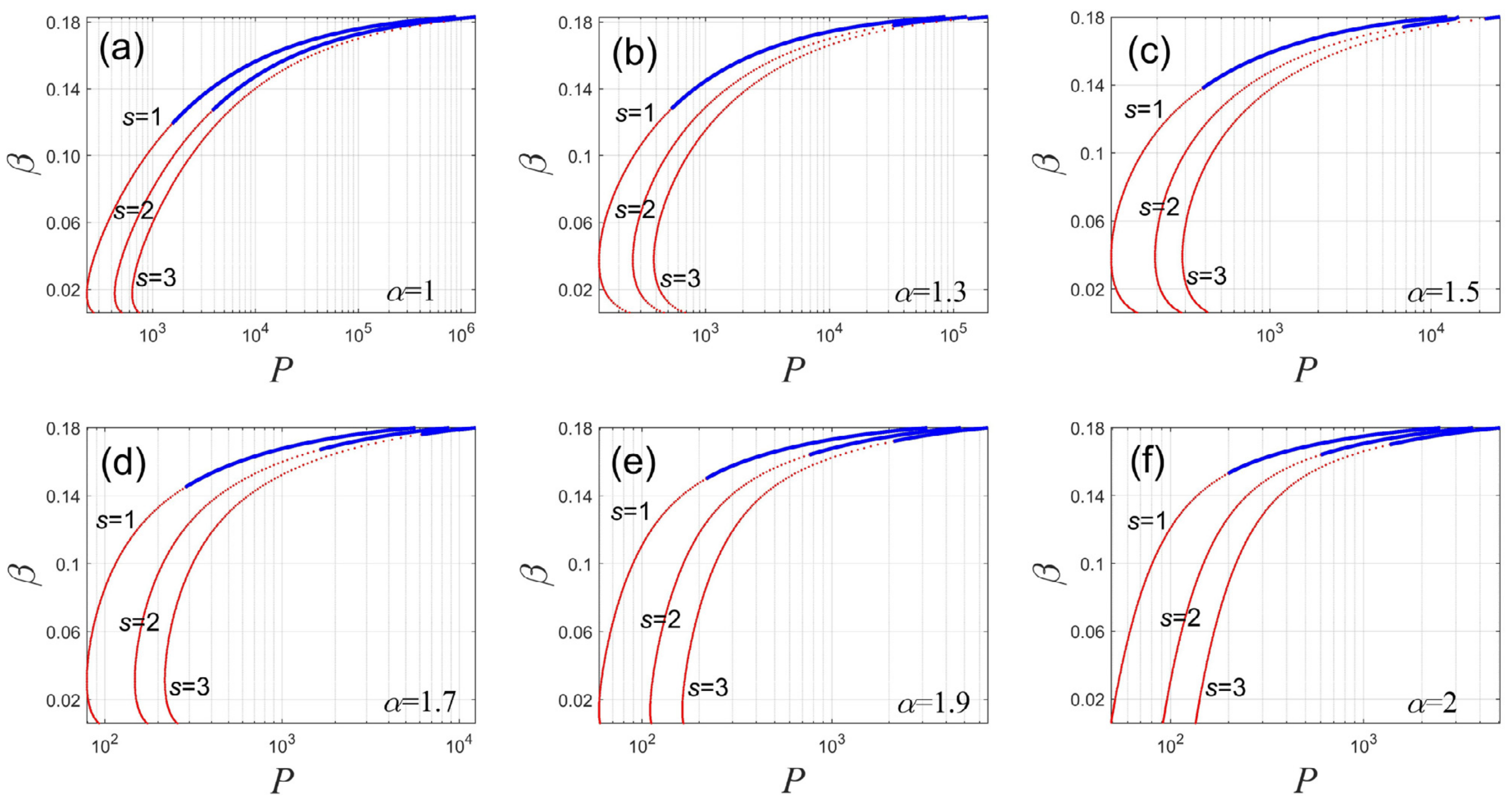

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Malomed, B.A. Optical Solitons and Vortices in Fractional Media: A Mini-Review of Recent Results. Photonics 2021, 8, 353. https://doi.org/10.3390/photonics8090353
Malomed BA. Optical Solitons and Vortices in Fractional Media: A Mini-Review of Recent Results. Photonics. 2021; 8(9):353. https://doi.org/10.3390/photonics8090353
Chicago/Turabian StyleMalomed, Boris A. 2021. "Optical Solitons and Vortices in Fractional Media: A Mini-Review of Recent Results" Photonics 8, no. 9: 353. https://doi.org/10.3390/photonics8090353
APA StyleMalomed, B. A. (2021). Optical Solitons and Vortices in Fractional Media: A Mini-Review of Recent Results. Photonics, 8(9), 353. https://doi.org/10.3390/photonics8090353




