Target Tracking and Ranging Based on Single Photon Detection
Abstract
1. Introduction
2. Experimental Setup Description
3. Single Photon Detection Model and Scanning Model and Target Position
3.1. Single Photon Detection Model and Analysis
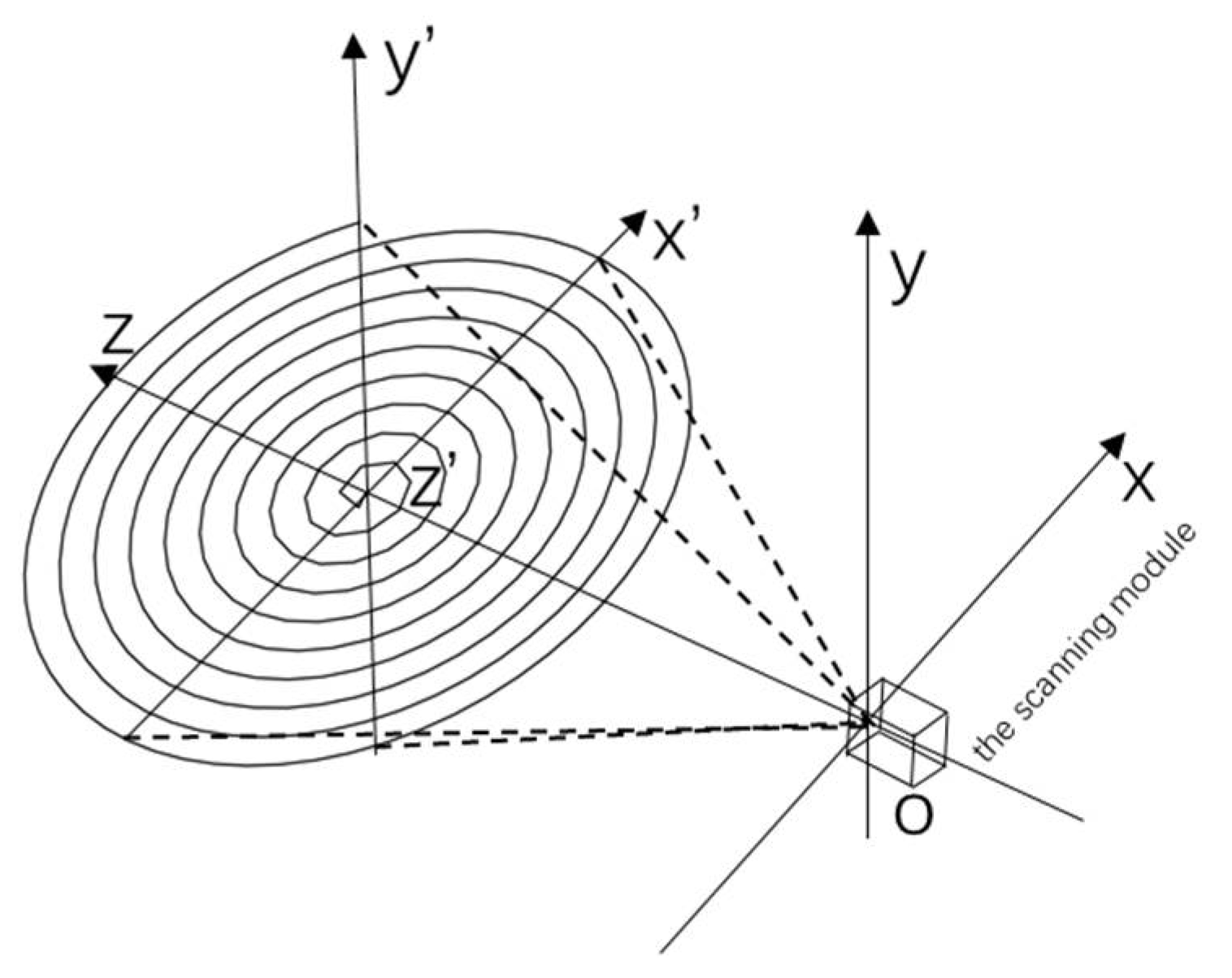
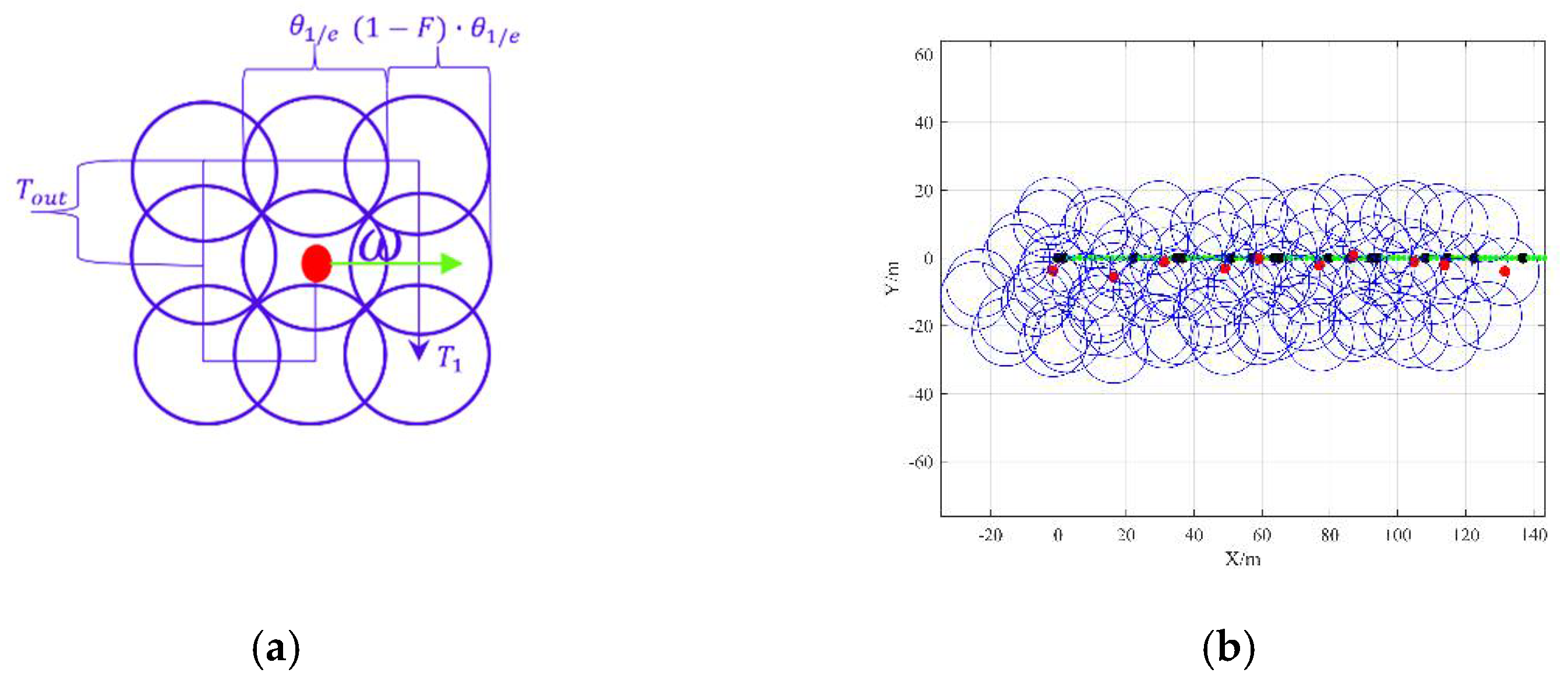
3.2. Scanning Model and Analysis
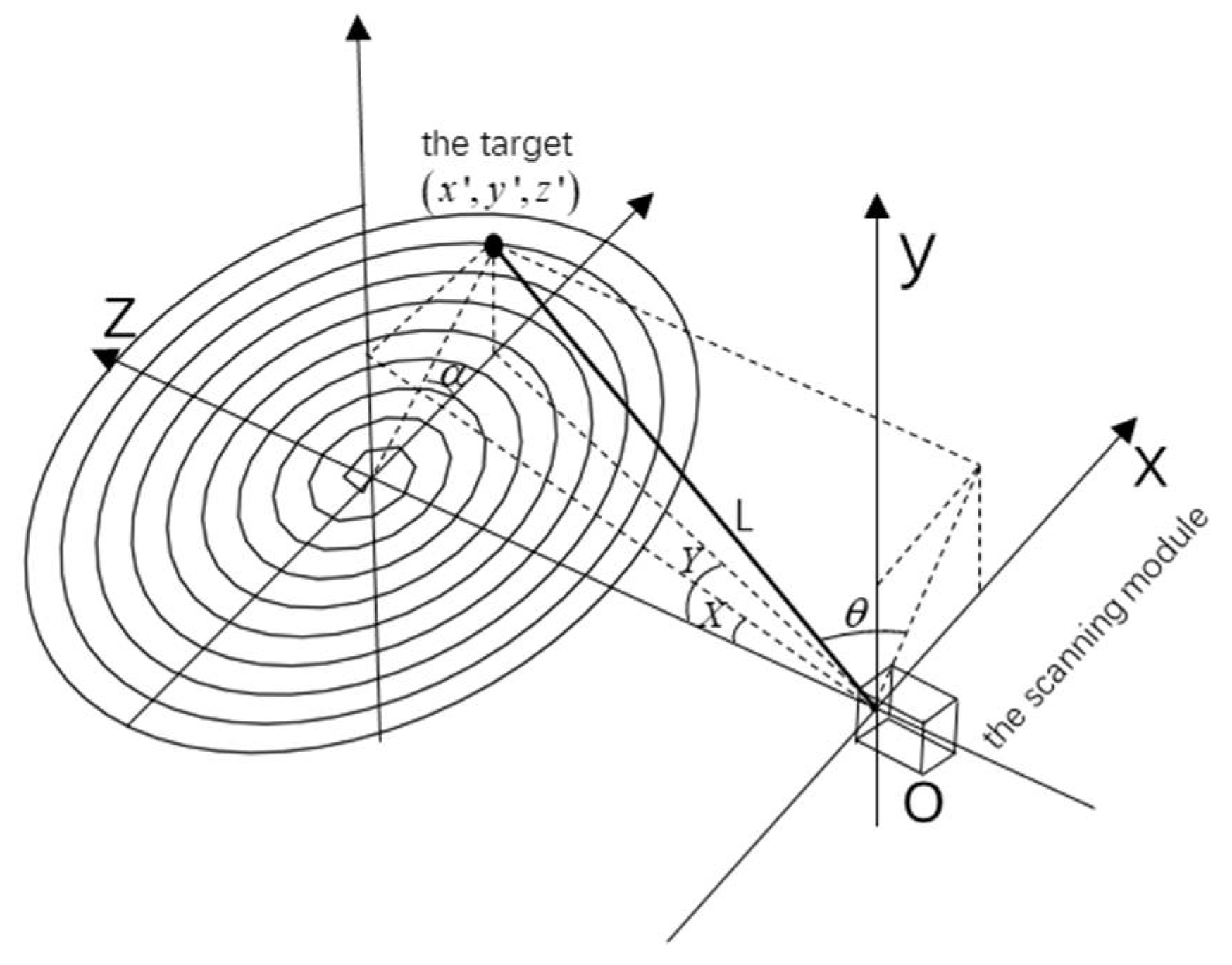
3.3. Derivation and Analysis for the Position of the Target
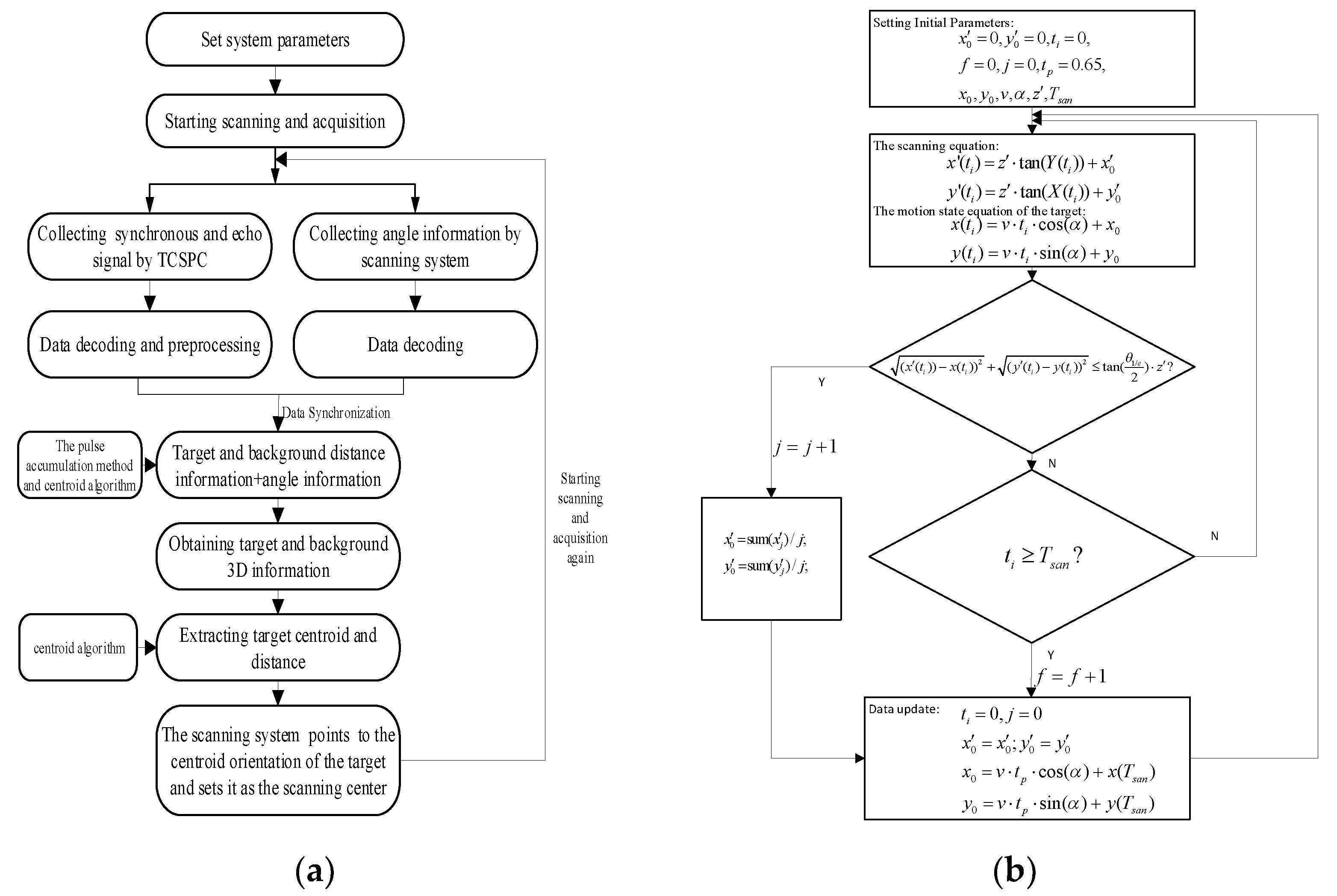
4. Strategy and Simulation Process of Target Tracking and Analysis of Experimental Results, and the Ability of Tracking and Detection
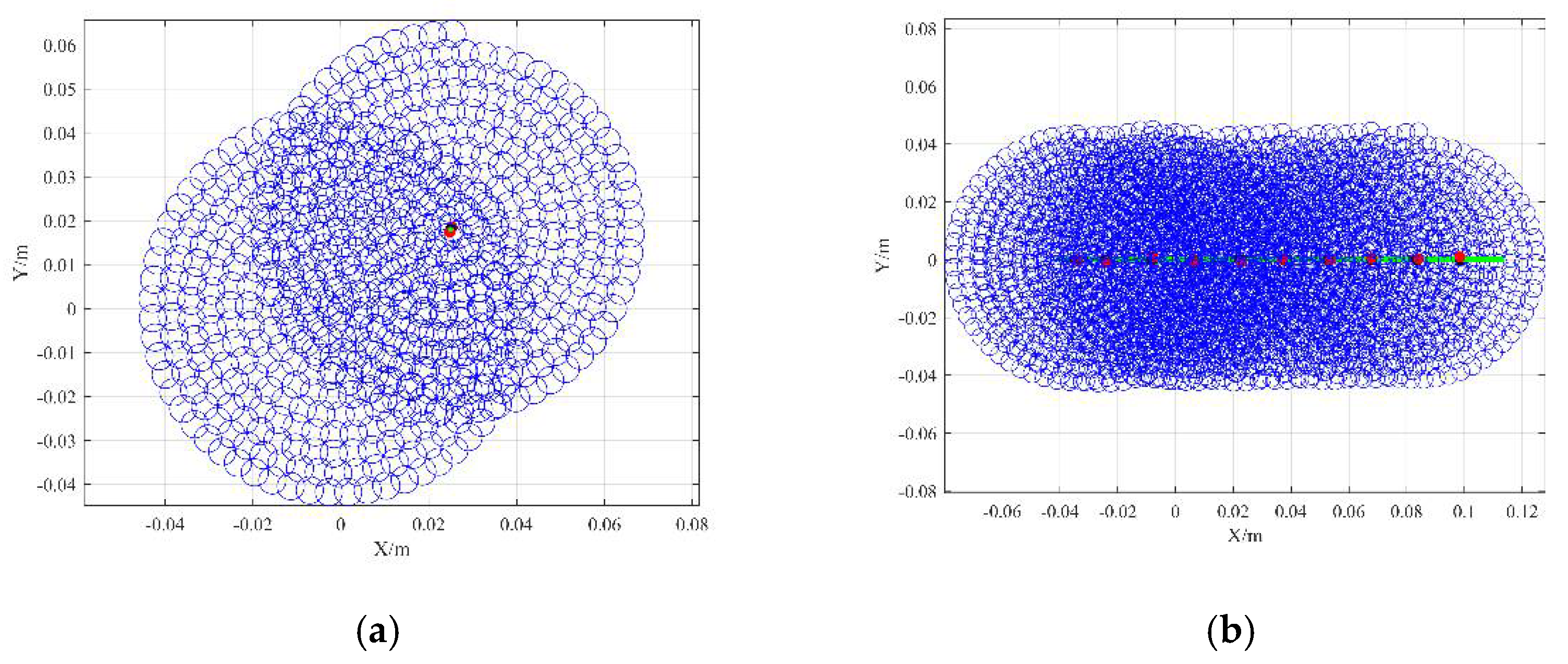
4.1. Tracking and Ranging Strategy of Target and the Simulation Process
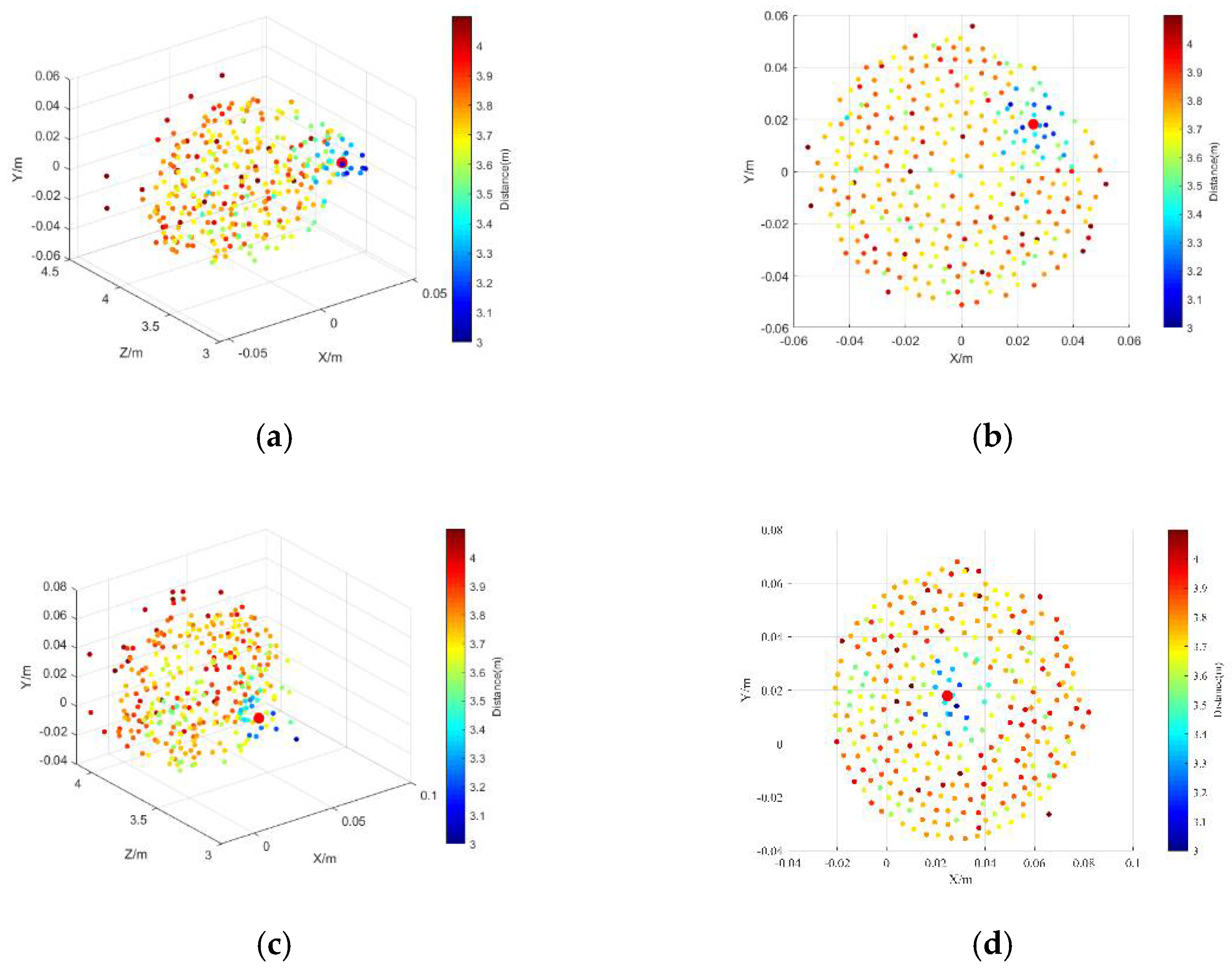
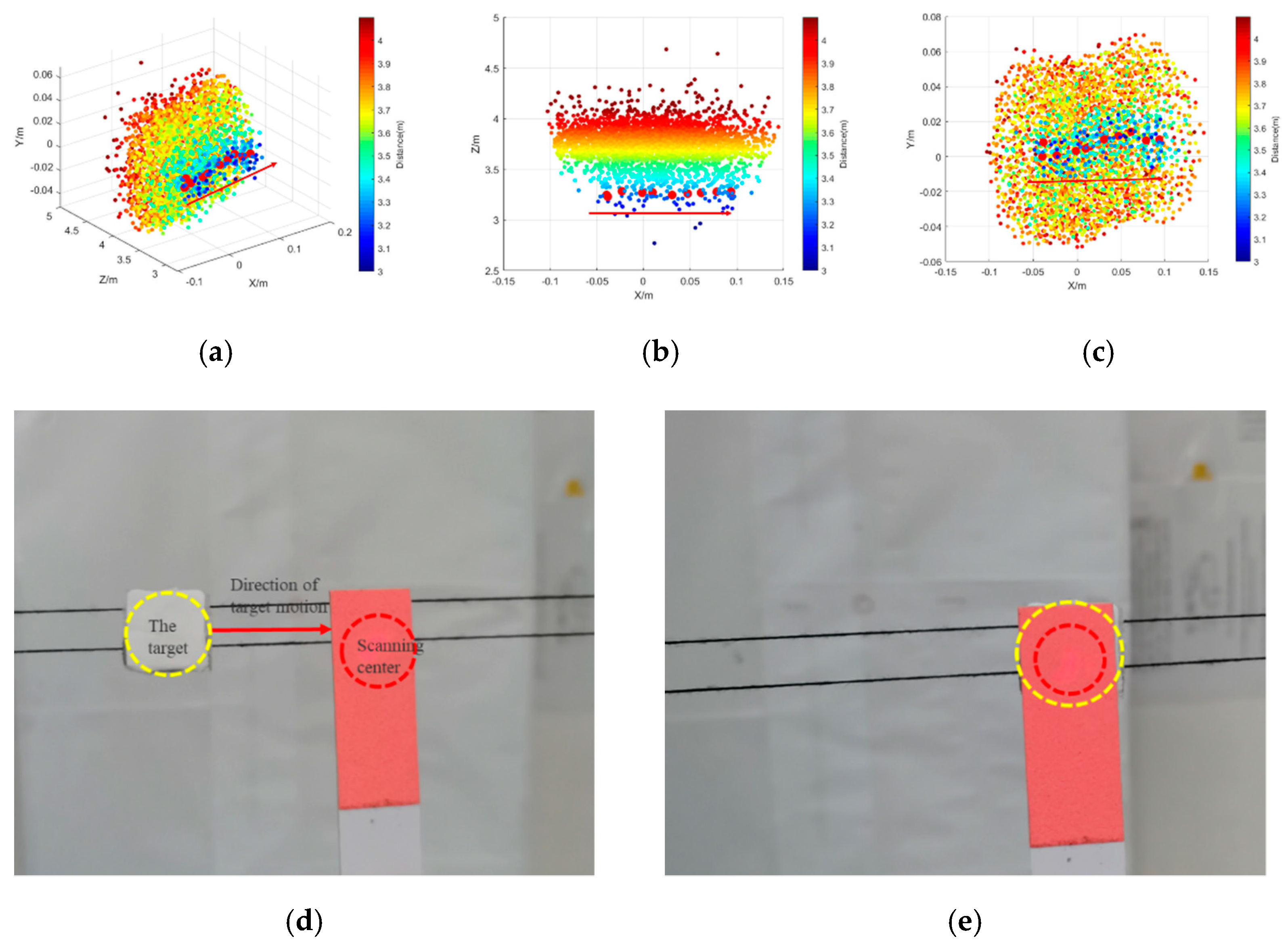
4.2. Simulation and Experimental Results and Analysis
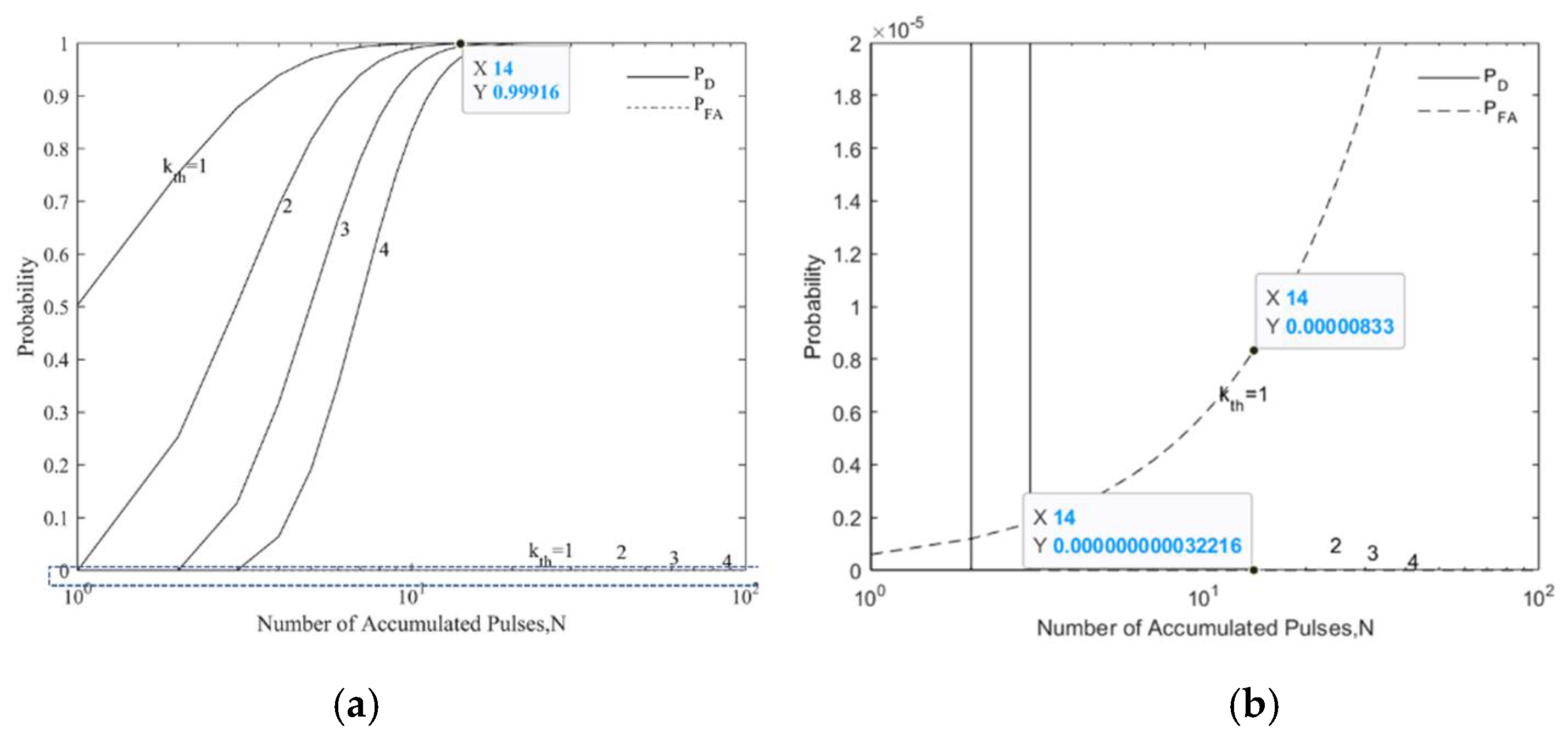
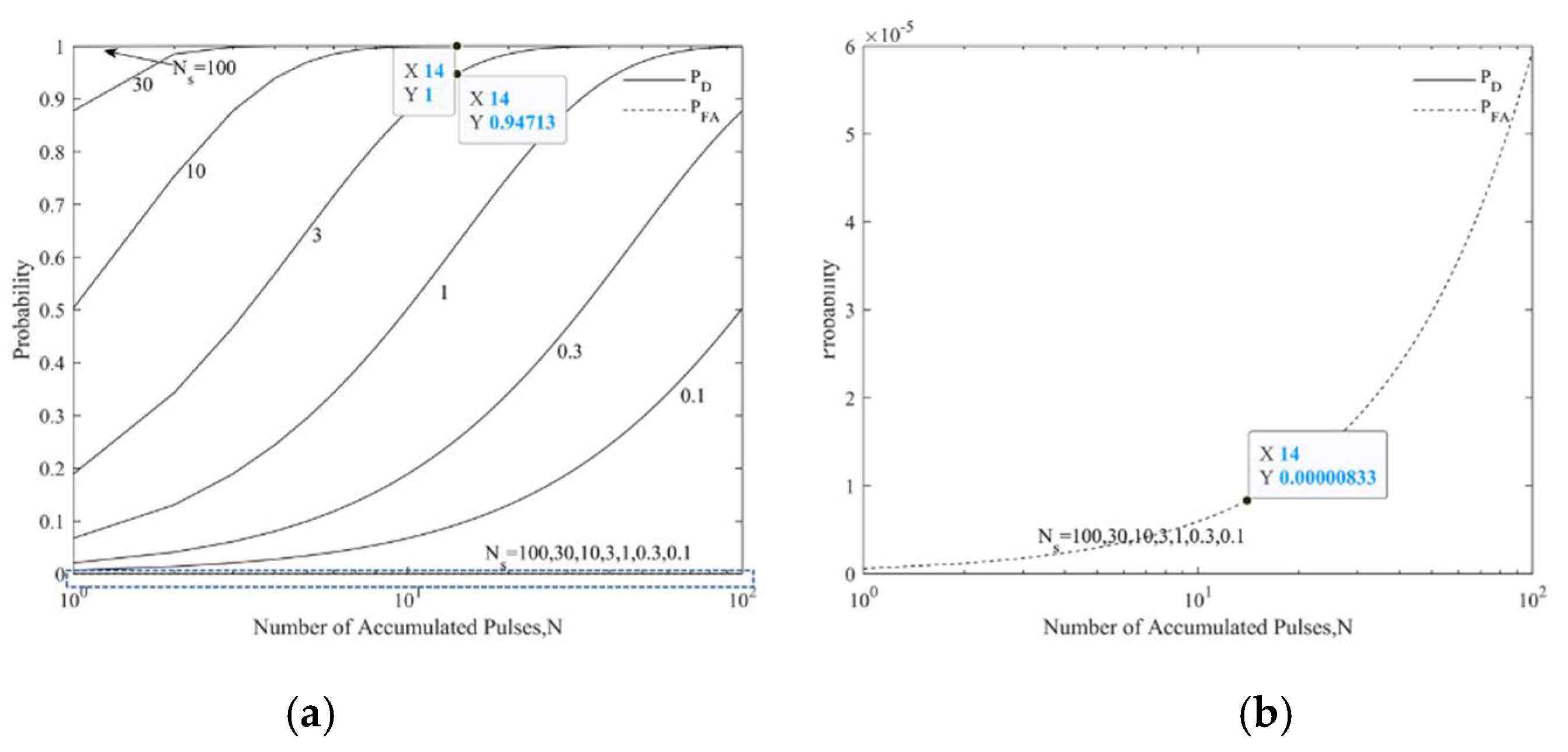
4.3. Analysis of Tracking and Detection Capability
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bachman, C.G. Laser Radar Systems and Techniques; Artech House: Dedham, UK, 1979. [Google Scholar]
- Pfennigbauer, M.; Mbius, B.; Carmo, J.P.D. Echo Digitizing Imaging Lidar for Rendezvous and Docking. In Proceedings of the Laser Radar Technology and Applications XIV, SPIE Defense, Security, and Sensing, Orlando, FL, USA, 30 April 2009. [Google Scholar]
- Ferraro, M.S.; Mahon, R.; Rabinovich, W.S.; Freeman, W.T.; Murphy, J.L.; Goetz, P.G.; Burris, H.R.; Moore, C.I.; Thomas, L.M.; Clark, W.R. InGaAs Avalanche Photodiode Arrays for Simultaneous Communications and Tracking. In Proceedings of the Free-Space and Atmospheric Laser Communications XI, San Diego, CA, USA, 24–25 August 2011; p. 81620D. [Google Scholar]
- Greene, B.; Gao, Y.; Moore, C.; Wang, Y.; Boiko, A.; Ritchie, J.; Sang, J. Laser Tracking of Space Debris. In Proceedings of the 13th International Workshop on Laser Ranging Instrumentation, Washington, DC, USA, 10 October 2002. [Google Scholar]
- Wu, C.; Xing, W.; Feng, Z.; Xia, L. Moving Target Tracking in Marine Aerosol Environment with Single Photon Lidar System. Opt. Lasers Eng. 2020, 127, 105967. [Google Scholar] [CrossRef]
- Jimenez, A.; Ceres, R.; Seco, F. A Laser Range-finder Scanner System for Precise Manoeuver and Obstacle Avoidance in Maritime and Inland Navigation. In Proceedings of the Proceedings Elmar-2004, 46th International Symposium on Electronics in Marine, Zadar, Croatia, 16–18 June 2004; pp. 101–106. [Google Scholar]
- Kim, B.H.; Khan, D.; Choi, W.; Kim, M.Y. Real-time Counter-UAV System for Long Distance Small Drones using Double Pan-tilt Scan Laser Radar. In Proceedings of the Laser Radar Technology and Applications XXIV, SPIE Defense + Commercial Sensing, Baltimore, MD, USA, 2 May 2019; p. 110050C. [Google Scholar]
- Leigh-Lancaster, C.J.; Shirinzadeh, B.; Koh, Y.L. Development of a Laser Tracking System. In Proceedings of the Proceedings Fourth Annual Conference on Mechatronics and Machine Vision in Practice, Toowoomba, Australia, 23–25 September 1997; pp. 163–168. [Google Scholar]
- Buller, G.; Collins, R. Single-photon Generation and Detection. Meas. Sci. Technol. 2009, 21, 012002. [Google Scholar] [CrossRef]
- Du, X.; Xing, J.C.; Huang, H. Method to Simulate the Object Tracking with Photon-counting Laser Ranging System. Opt. Eng. 2015, 54, 114103. [Google Scholar] [CrossRef]
- Castleman, A.W.; Toennies, J.P.; Zinth, W. Advanced Time-Correlated Single Photon Counting Techniques; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Becker, W.; Bergmann, A. Multi-Dimensional Time-Correlated Single Photon Counting. In Reviews in Fluorescence 2005; Geddes, C.D., Lakowicz, J.R., Eds.; Springer: Boston, MA, USA, 2005; pp. 77–108. [Google Scholar]
- Yu, Y.; Liu, B.; Chen, Z.; Li, Z.K. A Macro-Pulse Photon Counting Lidar for Long-Range High-Speed Moving Target Detection. Sensors 2020, 20, 2204. [Google Scholar] [CrossRef] [PubMed]
- Massa, J.S.; Buller, G.S.; Walker, A.C.; Cova, S.; Umasuthan, M.; Wallace, A.M. Time-of-flight Optical Ranging System based on Time-correlated Single-photon Counting. Appl. Opt. 1998, 37, 7298–7304. [Google Scholar] [CrossRef] [PubMed]
- Xue, L.; Zhang, L.; Zhang, S.; Li, M.; Kang, L.; Chen, J.; Wu, P. Satellite Laser Ranging using Superconducting Nanowire Single-photon Detectors at 1064 nm Wavelength. Opt. Lett. 2016, 41, 3848. [Google Scholar] [CrossRef] [PubMed]
- Blazej, J.; Prochazka, I.; Kodet, J. Photon Counting Detector for High-repetition-rate Optical Time Transfer Providing Extremely High Data Yield. Opt. Eng. 2014, 53, 081903. [Google Scholar] [CrossRef][Green Version]
- Kong, H.J.; Kim, T.H.; Jo, S.E.; Oh, M.S. Smart Three-dimensional Imaging Ladar using Two Geiger-mode Avalanche Photodiodes. Opt. Express 2011, 19, 19323. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Yu, Y.; Jiang, S. Review of Advances in LiDAR Detection and 3D Imaging. Guangdian Gongcheng/Opto-Electron. Eng. 2019, 46, 2019–2065. [Google Scholar]
- Yang, Y.; Bo, L.; Zhen, C. Analyzing the Performance of Pseudo-random Single Photon Counting Ranging Lidar. Appl. Opt. 2018, 57, 7733. [Google Scholar]
- Li, C.; Wang, S.; Huo, L.; Wang, Y.; Liang, K.; Yang, R.; Han, D. Position Sensitive Silicon Photomultiplier With Intrinsic Continuous Cap Resistive Layer. IEEE Trans. Electron Devices 2014, 61, 3229–3232. [Google Scholar] [CrossRef]
- Zappa, F.; Lacaita, A.; Cova, S.; Lovati, P. Counting, Timing, and Tracking with a Single-photon Germanium Detector. Opt. Lett. 1996, 21, 59–61. [Google Scholar] [CrossRef] [PubMed]
- De-Bur, V.; Terekhov, A.; Kosolobov, S.; Sheibler, H.; Plokhotnichenko, V. Position-Sensitive Detector with GaAs Photocathode and High Time Resolution; AIP Conference Proceedings 984; American Institute of Physics: College Park, MD, USA, 2008; pp. 186–193. [Google Scholar]
- Fouche, D.G. Detection and False-alarm Probabilities for Laser Radars that Use Geiger-mode Detectors. Appl. Opt. 2003, 42, 5388–5398. [Google Scholar] [CrossRef] [PubMed]
- Gatt, P.; Johnson, S.; Nichols, T. Geiger-mode Avalanche Photodiode Ladar Receiver Performance Characteristics and Detection Statistics. Appl. Opt. 2009, 48, 3261–3276. [Google Scholar] [CrossRef]
- Johnson, S.; Gatt, P.; Nichols, T. Analysis of Geiger-mode APD Laser Radars. In Proceedings of the Laser Radar Technology and Applications VIII, AeroSense 2003, Orlando, FL, USA, 21 August 2003. [Google Scholar]
- Chen, Z.; Liu, B.; Guo, G. Adaptive Single Photon Detection under Fluctuating Background Noise. Opt. Express 2020, 28, 30199. [Google Scholar] [CrossRef] [PubMed]
- O’Connor, D. Time-correlated Single Photon Counting; Academic Press: Cambridge, MA, USA, 1984. [Google Scholar]
- Scheinfeild, M.; Kopeika, N.S.; Melamed, R. Acquisition System for Microsatellites Laser Communication in Space. In Proceedings of the Free-Space Laser Communication Technologies XII, Symposium on High-Power Lasers and Applications, San Jose, CA, USA, 2 May 2000; pp. 166–175. [Google Scholar]
- Hindman, C.; Robertson, L. Beaconless Satellite Laser Acquisition-modeling and Feasability. In Proceedings of the IEEE MILCOM 2004 Military Communications Conference, Monterey, CA, USA, 31 October–3 November 2004. [Google Scholar]
- Teng, Y.; Zhang, M.; Tong, S. The Optimization Design of Sub-regions Scanning and Vibration Analysis for Beaconless Spatial Acquisition in the Inter-satellite Laser Communication System. IEEE Photonics J. 2018, 10, 1–11. [Google Scholar] [CrossRef]
- Zhang, M.; Li, B.; Tong, S. A New Composite Spiral Scanning Approach for Beaconless Spatial Acquisition and Experimental Investigation of Robust Tracking Control for Laser Communication System with Disturbance. IEEE Photonics J. 2020, 12, 1–12. [Google Scholar] [CrossRef]
- Xin, L.; Quanyou, S.; Jing, M.; Siyuan, Y.; Liying, T. Spatial Acquisition Optimization based on Average Acquisition Time for Intersatellite Optical Communications. In Proceedings of the 2010 Academic Symposium on Optoelectronics and Microelectronics Technology and 10th Chinese-Russian Symposium on Laser Physics and Laser TechnologyOptoelectronics Technology (ASOT), Harbin, China, 28 July–1 August 2010; pp. 244–248. [Google Scholar]
- Liu, B.; Jiang, S.; Yu, Y.; Chen, Z. Macro/sub-pulse Coded Photon Counting LIDAR. Opto-Electron. Eng. 2020, 47, 99–109. [Google Scholar]
- Hiskett, P.A.; Parry, C.S.; Mccarthy, A.; Buller, G.S. A Photon-counting Time-of-flight Ranging Technique Developed for the Avoidance of Range Ambiguity at Gigahertz Clock Rates. Opt. Express 2009, 16, 13685–13698. [Google Scholar] [CrossRef]
- Krichel, N.J.; Mccarthy, A.; Buller, G.S. Resolving Range Ambiguity in a Photon Counting Depth Imager Operating at Kilometer Distances. Opt. Express 2010, 18, 9192–9206. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Yu, Y.; Chen, Z.; Han, W. True Random Coded Photon Counting Lidar. Opto-Electron. Adv. 2020, 3, 190044. [Google Scholar] [CrossRef]


| Parameter | Value |
| Pulse repetition frequency () | 5 Khz |
| Divergence angle () | 1.9 mrad |
| Number of accumulated pulses () | 14 |
| Threshold of the recognition target () | 1 |
| Overlap factor () | 0.29 |
| Number of rings () | 10 |
| Time interval of the output angle () | 2.8 ms |
| Total scan time () | 879.6 ms |
| No. | Before Pointing to the Target | After Pointing to the Target | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| x0/m | y0/m | z0/m | L0/m | α/rad | θ/rad | x0/m | y0/m | z0/m | L0/m | α/rad | θ/rad | ||
| 1 | 0.0257 | 0.0181 | 3.2631 | 3.2632 | 0.6134 | 1.5612 | 0.0247 | 0.0179 | 3.2628 | 3.2630 | 0.6271 | 1.5612 | |
| 2 | 0.0239 | 0.0223 | 3.2542 | 3.2544 | 0.7503 | 1.5606 | 0.0153 | 0.0188 | 3.2464 | 3.2465 | 0.8876 | 1.5634 | |
| 3 | 0.0247 | 0.0156 | 3.2797 | 3.2798 | 0.5619 | 1.5619 | 0.0251 | 0.0214 | 3.2709 | 3.2710 | 0.7062 | 1.5609 | |
| 4 | 0.0188 | 0.0215 | 3.2582 | 3.2584 | 0.8536 | 1.5619 | 0.0222 | 0.0234 | 3.2374 | 3.2375 | 0.8106 | 1.5609 | |
| 5 | 0.0229 | 0.0216 | 3.2699 | 3.2701 | 0.7544 | 1.5609 | 0.0300 | 0.0220 | 3.2909 | 3.2911 | 0.6324 | 1.5595 | |
| 6 | 0.0191 | 0.0222 | 3.2760 | 3.2761 | 0.8603 | 1.5619 | 0.0234 | 0.0147 | 3.2643 | 3.2644 | 0.5622 | 1.5622 | |
| 7 | 0.0188 | 0.0233 | 3.2572 | 3.2573 | 0.8915 | 1.5615 | 0.0245 | 0.0189 | 3.2415 | 3.2416 | 0.6578 | 1.5612 | |
| 8 | 0.0188 | 0.0196 | 3.2572 | 3.2573 | 0.8050 | 1.5622 | 0.0276 | 0.0188 | 3.2283 | 3.2285 | 0.5983 | 1.5605 | |
| 9 | 0.0204 | 0.0234 | 3.2467 | 3.2469 | 0.8530 | 1.5612 | 0.0260 | 0.0218 | 3.2646 | 3.2648 | 0.6973 | 1.5606 | |
| 10 | 0.0246 | 0.0174 | 3.2717 | 3.2718 | 0.6157 | 1.5615 | 0.0246 | 0.0205 | 3.3093 | 3.3095 | 0.6937 | 1.5610 | |
| Mean | 0.0218 | 0.0205 | 3.2634 | 3.2635 | 0.7559 | 1.5615 | 0.0243 | 0.0198 | 3.2616 | 3.2618 | 0.6873 | 1.5611 | |
| Standard Deviation | 0.0027 | 0.0026 | 0.0100 | 0.0100 | 0.1133 | 0.0005 | 0.0037 | 0.0024 | 0.0236 | 0.0236 | 0.0932 | 0.0010 | |
| No. | 1 | 2 | |||||||
|---|---|---|---|---|---|---|---|---|---|
| x0/m | y0/m | z0/m | L0/m | x0/m | y0/m | z0/m | L0/m | ||
| 1 | −0.0396 | 0.0000 | 3.2461 | 3.2463 | −0.0399 | −0.0033 | 3.2532 | 3.2534 | |
| 2 | −0.0383 | 0.0082 | 3.2305 | 3.2307 | −0.0298 | 0.0066 | 3.2540 | 3.2541 | |
| 3 | −0.0232 | 0.0007 | 3.2840 | 3.2841 | −0.0136 | 0.0069 | 3.2596 | 3.2597 | |
| 4 | −0.0014 | 0.0032 | 3.2678 | 3.2678 | 0.0068 | 0.0059 | 3.2756 | 3.2756 | |
| 5 | 0.0096 | 0.0047 | 3.2723 | 3.2724 | 0.0180 | 0.0076 | 3.2869 | 3.2869 | |
| 6 | 0.0306 | 0.0097 | 3.2462 | 3.2464 | 0.0389 | 0.0044 | 3.2579 | 3.2581 | |
| 7 | 0.0473 | 0.0118 | 3.2597 | 3.2601 | 0.0491 | 0.0114 | 3.2648 | 3.2652 | |
| 8 | 0.0616 | 0.0142 | 3.2681 | 3.2687 | 0.0693 | 0.0169 | 3.2387 | 3.2395 | |
| 9 | 0.0784 | 0.0093 | 3.2788 | 3.2797 | 0.0861 | 0.0033 | 3.2468 | 3.2480 | |
| 10 | 0.0937 | 0.0098 | 3.2785 | 3.2799 | 0.0961 | 0.0078 | 3.2721 | 3.2735 | |
| Parameter | Value |
|---|---|
| Energy of the laser () | J |
| Laser wavelength () | 1064 nm |
| Laser pulse width () | 2 ns |
| Laser divergence angle () | rad |
| Effective receiving area () | |
| Atmospheric transmittance () | 0.7 |
| Optical efficiency of a lunch system () | 0.9 |
| Optical efficiency of a receiving system () | 0.8 |
| Reflection efficiency of the target () | 0.3 |
| Effective area of the target () | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Liu, B.; Wang, H.; Chen, Z.; Zhang, Q.; Hua, K.; Yang, J. Target Tracking and Ranging Based on Single Photon Detection. Photonics 2021, 8, 278. https://doi.org/10.3390/photonics8070278
Li Z, Liu B, Wang H, Chen Z, Zhang Q, Hua K, Yang J. Target Tracking and Ranging Based on Single Photon Detection. Photonics. 2021; 8(7):278. https://doi.org/10.3390/photonics8070278
Chicago/Turabian StyleLi, Zhikang, Bo Liu, Huachuang Wang, Zhen Chen, Qun Zhang, Kangjian Hua, and Jing Yang. 2021. "Target Tracking and Ranging Based on Single Photon Detection" Photonics 8, no. 7: 278. https://doi.org/10.3390/photonics8070278
APA StyleLi, Z., Liu, B., Wang, H., Chen, Z., Zhang, Q., Hua, K., & Yang, J. (2021). Target Tracking and Ranging Based on Single Photon Detection. Photonics, 8(7), 278. https://doi.org/10.3390/photonics8070278






