Controlled Coherence Plasmonic Light Sources
Abstract
1. Introduction
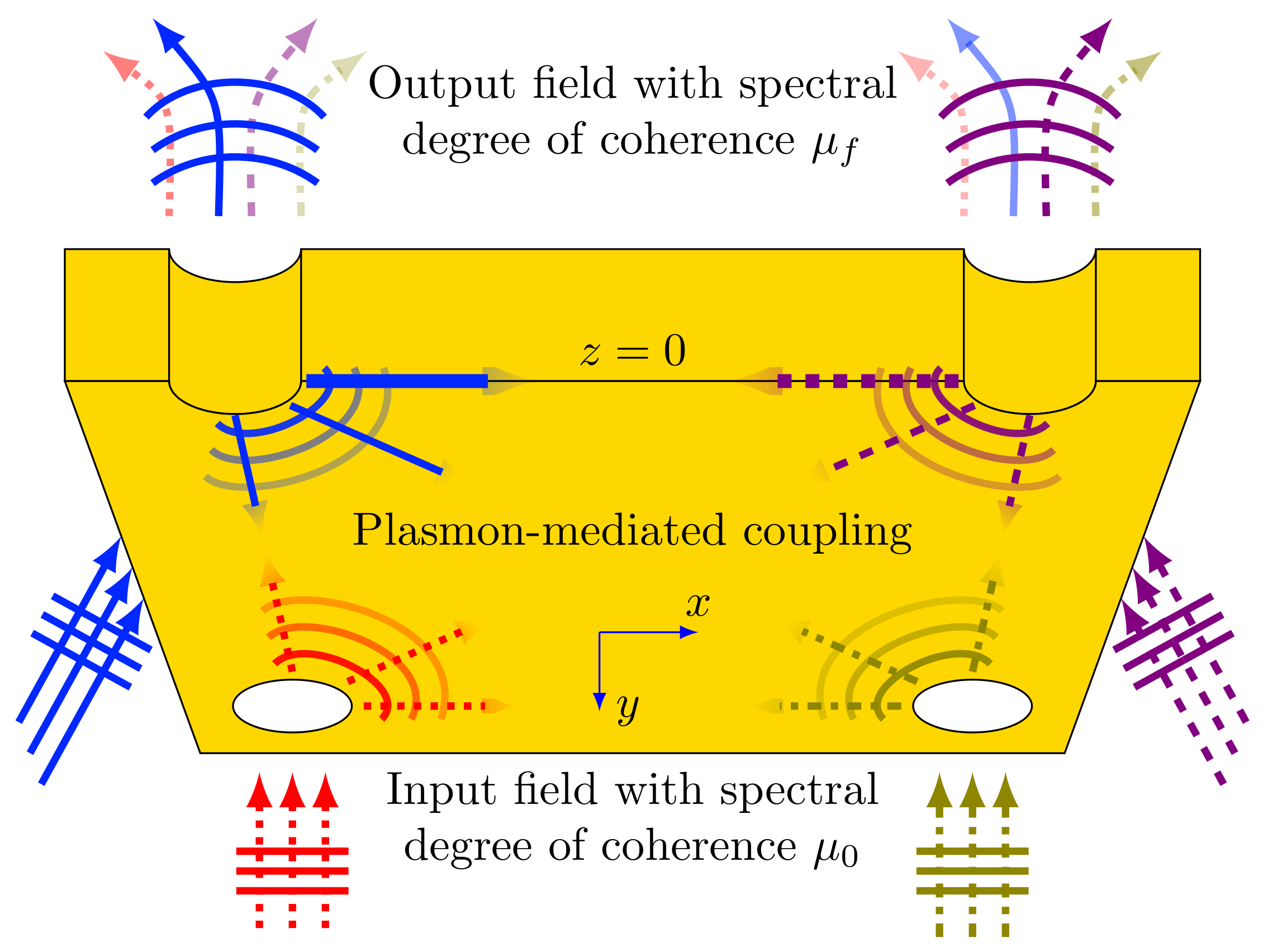
2. Review of the Model
2.1. Coherence Model
2.2. Plasmon Scattering Model
2.3. Average Coherence and Transmittance
3. Results and Discussion
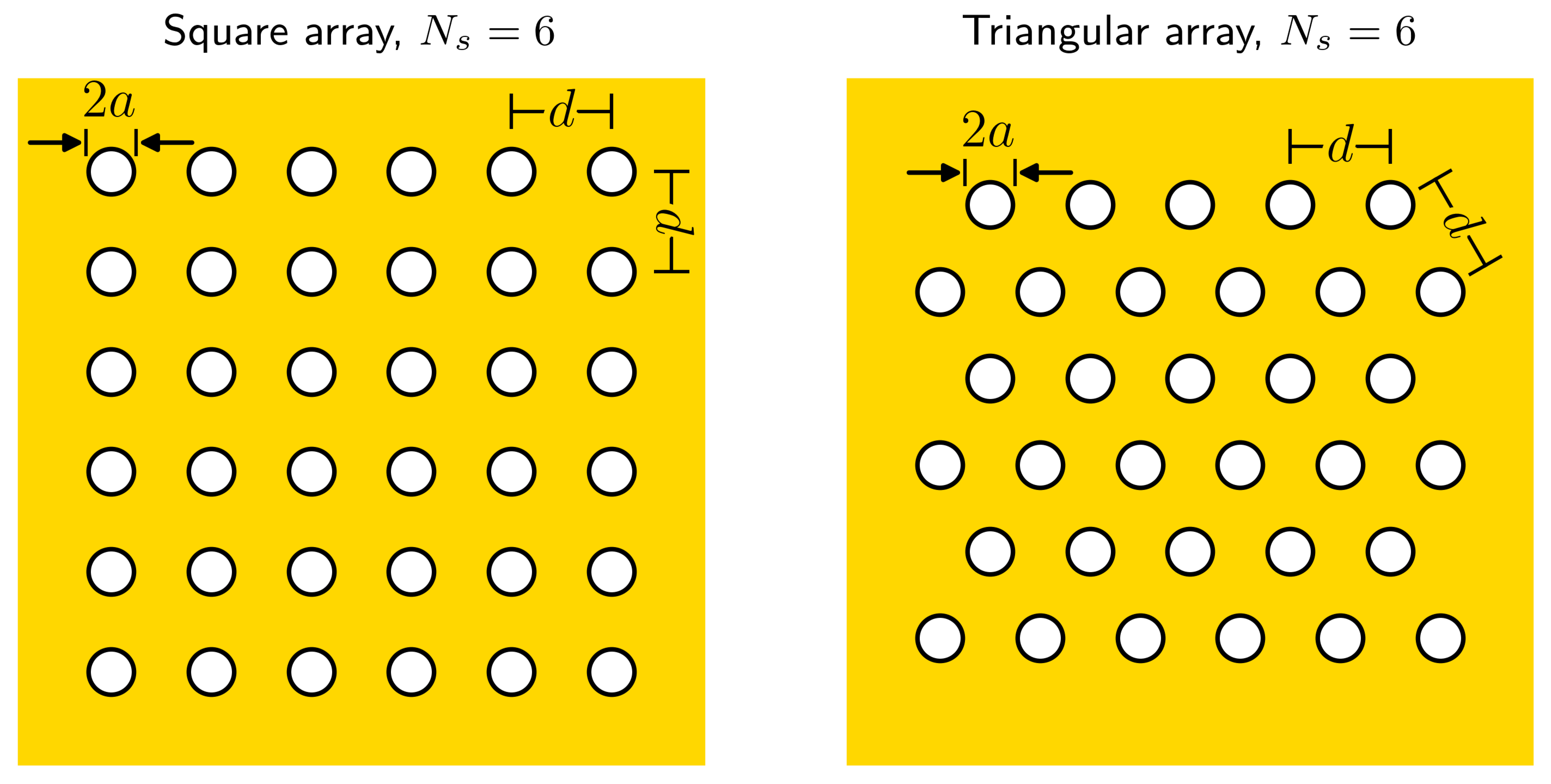
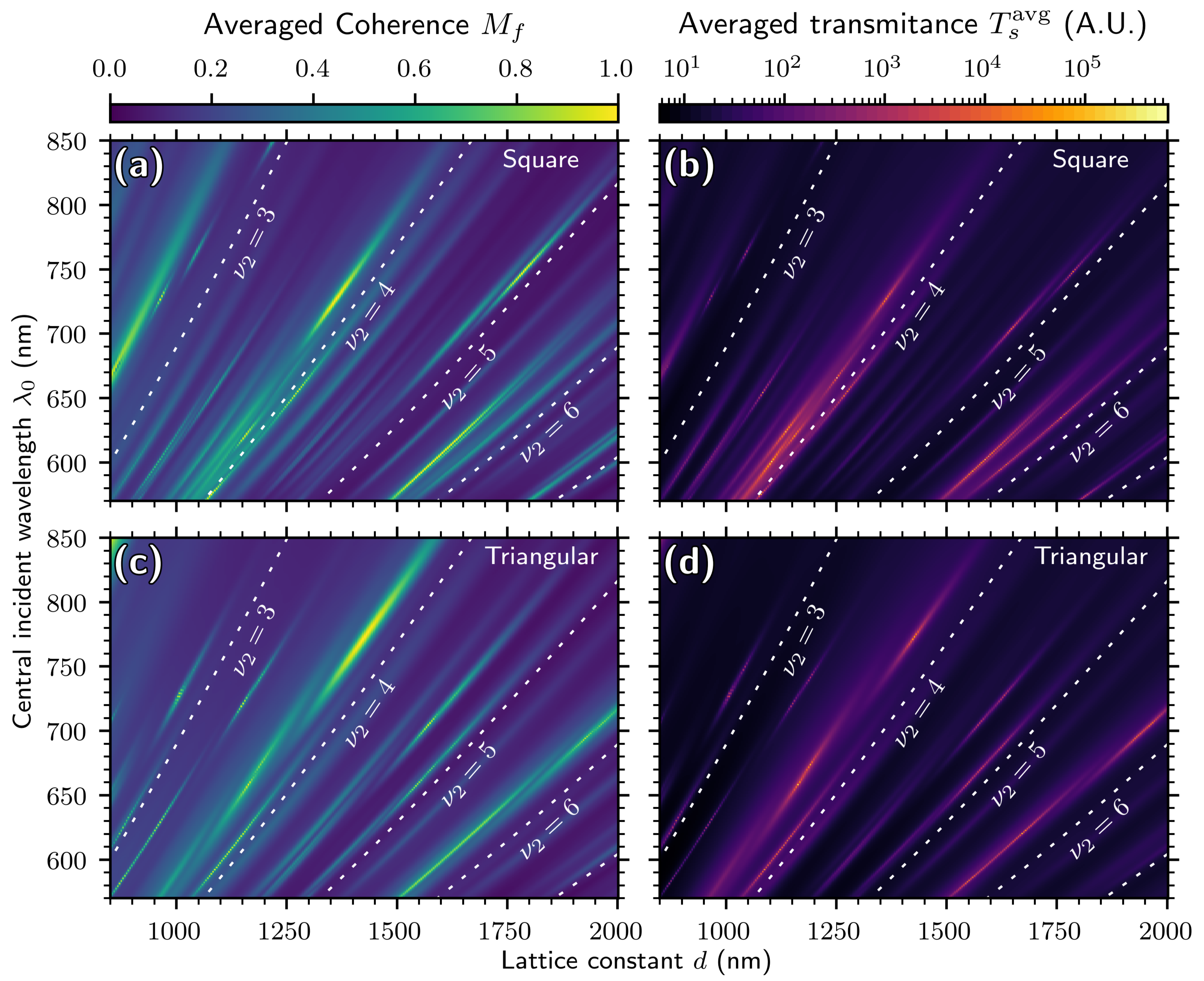
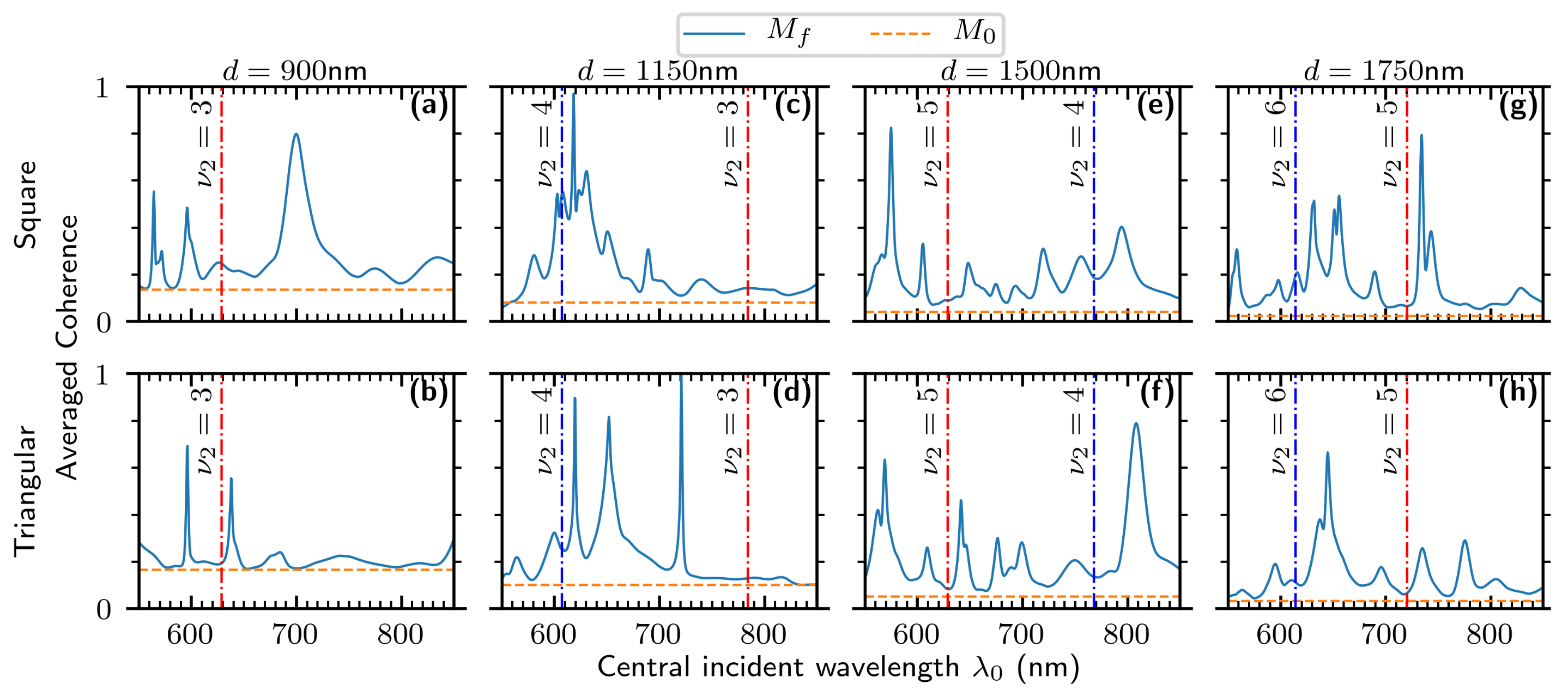
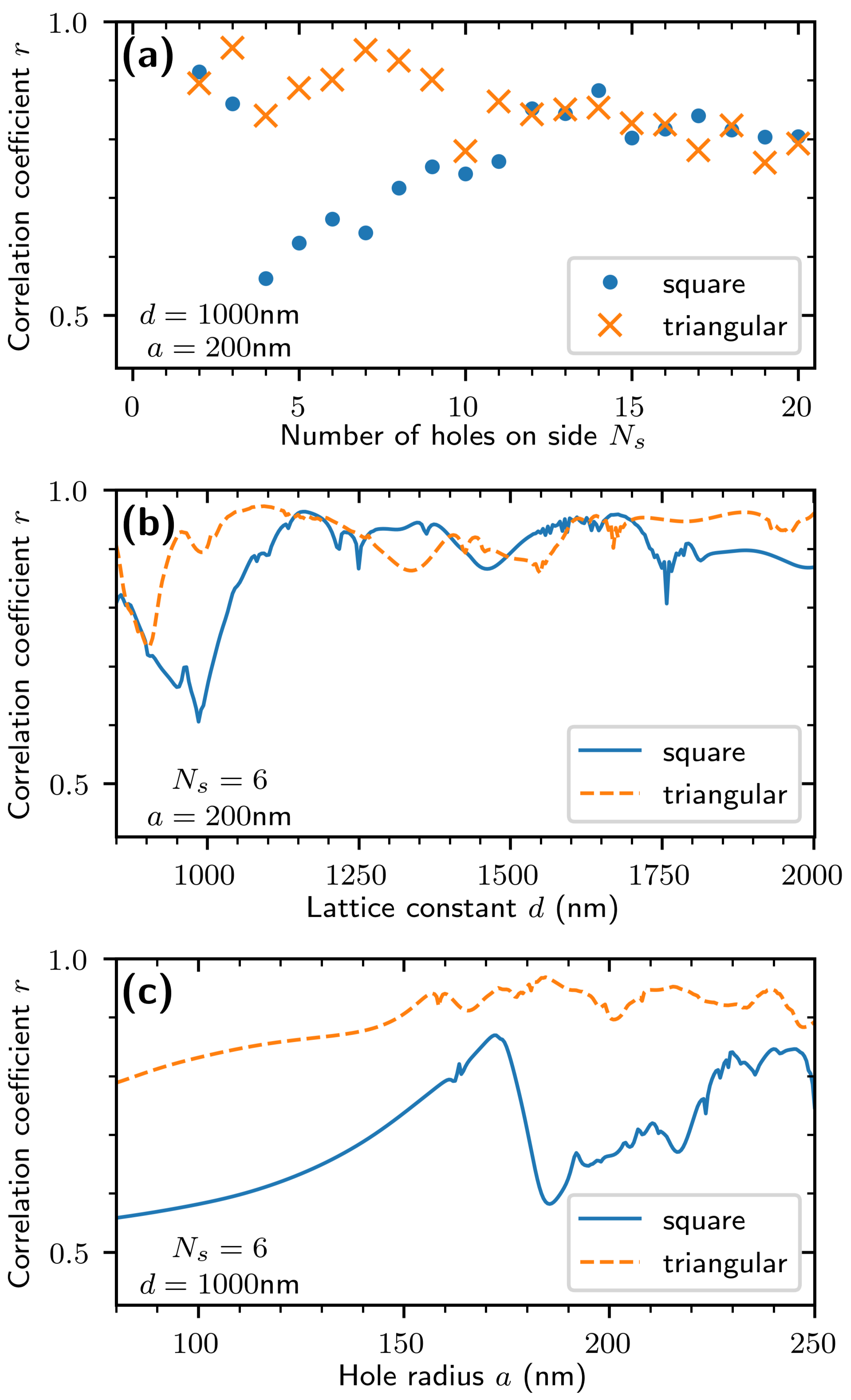
3.1. Changing Lattice Constant d
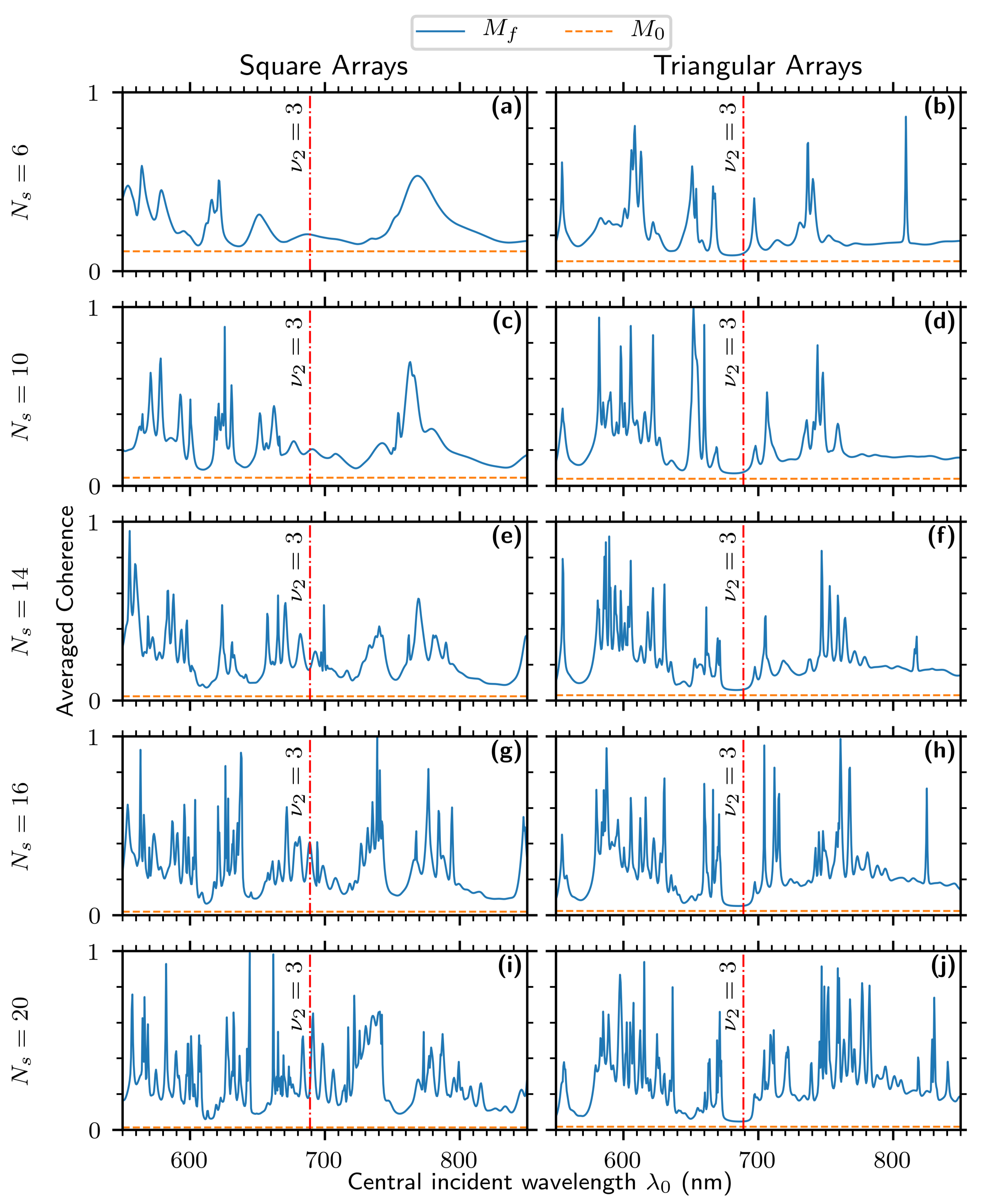
3.2. Changing Array Size
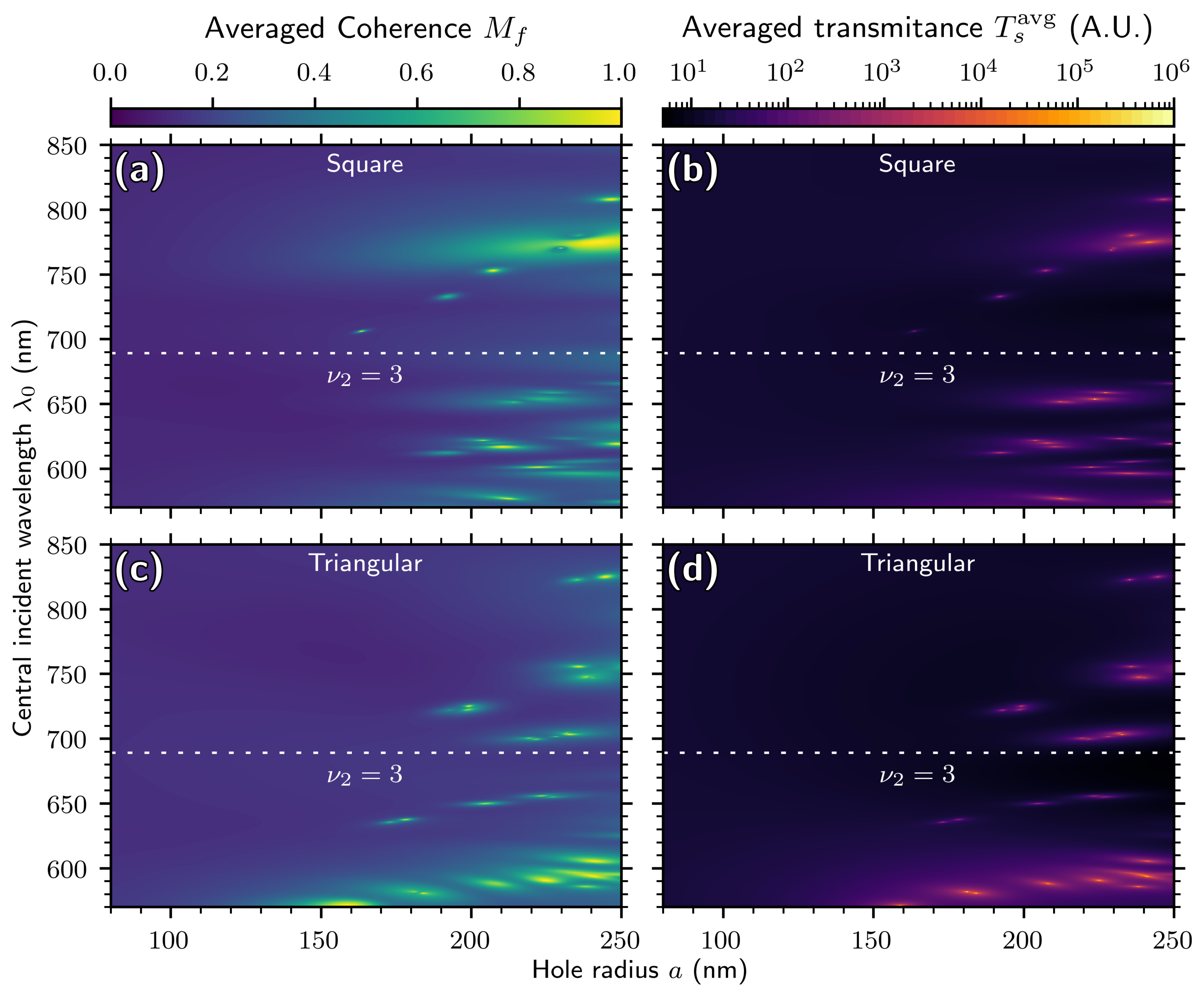
3.3. Changing Hole Radius a
3.4. Correlation between Coherence and Transmittance
4. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Krupin, O.; Wang, C.; Berini, P. Selective capture of human red blood cells based on blood group using long-range surface plasmon waveguides. Biosens. Bioelectron. 2014, 53, 117–122. [Google Scholar] [CrossRef]
- Schwarz, B.; Reininger, P.; Ristanic, D.; Detz, H.; Andrews, A.M.; Schrenk, W.; Strasser, G. Monolithically integrated mid-infrared lab-on-a-chip using plasmonics and quantum cascade structures. Nat. Commun. 2014, 5, 4085. [Google Scholar] [CrossRef]
- Wong, W.R.; Krupin, O.; Sekaran, S.D.; Mahamd Adikan, F.R.; Berini, P. Serological Diagnosis of Dengue Infection in Blood Plasma Using Long-Range Surface Plasmon Waveguides. Anal. Chem. 2014, 86, 1735–1743. [Google Scholar] [CrossRef] [PubMed]
- Gramotnev, D.K.; Bozhevolnyi, S.I. Nanofocusing of electromagnetic radiation. Nat. Photonics 2014, 8, 13–22. [Google Scholar] [CrossRef]
- Xiong, K.; Tordera, D.; Emilsson, G.; Olsson, O.; Linderhed, U.; Jonsson, M.P.; Dahlin, A.B. Switchable Plasmonic Metasurfaces with High Chromaticity Containing Only Abundant Metals. Nano Lett. 2017, 17, 7033–7039. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Cushing, S.K.; Zheng, P.; Meng, F.; Chu, D.; Wu, N. Plasmon-induced photonic and energy-transfer enhancement of solar water splitting by a hematite nanorod array. Nat. Commun. 2013, 4, 2651. [Google Scholar] [CrossRef] [PubMed]
- Ebbesen, T.; Lezec, H.; Ghaemi, H.; Thio, T.; Wolff, P. Extraordinary optical transmission through sub-wavelength hole arrays. Nature 1998, 391, 667–669. [Google Scholar] [CrossRef]
- Schouten, H.; Kuzmin, N.; Dubois, G.; Visser, T.; Gbur, G.; Alkemade, P.F.A.; Blok, H.; Hooft, G.W.; Lenstra, D.; Eliel, E.R. Plasmon-Assisted Two-Slit Transmission: Young’s Experiment Revisited. Phys. Rev. Lett. 2005, 94, 053901. [Google Scholar] [CrossRef]
- Rodrigo, S.G.; de León-Pérez, F.; Martín-Moreno, L. Extraordinary Optical Transmission: Fundamentals and Applications. Proc. IEEE 2016, 104, 2288–2306. [Google Scholar] [CrossRef]
- Gan, C.H.; Gbur, G.; Visser, T.D. Surface Plasmons Modulate the Spatial Coherence of Light in Young’s Interference Experiment. Phys. Rev. Lett. 2007, 98, 043908. [Google Scholar] [CrossRef]
- Kuzmin, N.; ’t Hooft, G.W.; Eliel, E.R.; Gbur, G.; Schouten, H.F.; Visser, T.D. Enhancement of spatial coherence by surface plasmons. Opt. Lett. 2007, 32, 445–447. [Google Scholar] [CrossRef]
- Wolf, E. Coherence and radiometry. J. Opt. Soc. Am. 1978, 68, 6–17. [Google Scholar] [CrossRef]
- Wolf, E.; James, D.F.V. Correlation-induced spectral changes. Rep. Prog. Phys 1996, 59, 771–778. [Google Scholar] [CrossRef]
- James, D.F.V. Change of polarization of light beams on propagation in free space. J. Opt. Soc. Am. A 1994, 11, 1641–1643. [Google Scholar] [CrossRef]
- Wolf, E. Correlation-induced changes in the degree of polarization, the degree of coherence, and the spectrum of random electromagnetic beams on propagation. Opt. Lett. 2003, 28, 1078–1080. [Google Scholar] [CrossRef] [PubMed]
- Korotkova, O.; Wolf, E. Changes in the state of polarization of a random electromagnetic beam on propagation. Opt. Commun. 2005, 246, 35–43. [Google Scholar] [CrossRef]
- Gan, C.H.; Gu, Y.; Visser, T.D.; Gbur, G. Coherence Converting Plasmonic Hole Arrays. Plasmonics 2012, 7, 313–322. [Google Scholar] [CrossRef][Green Version]
- Forbes, A.; de Oliveira, M.; Dennis, M.R. Structured light. Nat. Photonics 2021, 15, 253–262. [Google Scholar] [CrossRef]
- Cox, M.A.; Mphuthi, N.; Nape, I.; Mashaba, N.; Cheng, L.; Forbes, A. Structured Light in Turbulence. IEEE J. Sel. Top. Quantum Electron. 2021, 27, 1–21. [Google Scholar] [CrossRef]
- Gbur, G. Partially coherent beam propagation in atmospheric turbulence (Invited). J. Opt. Soc. Am. A 2014, 31, 2038–2045. [Google Scholar] [CrossRef]
- Smith, M.; Gbur, G. Coherence resonances and band gaps in plasmonic hole arrays. Phys. Rev. A 2019, 99, 023812. [Google Scholar] [CrossRef]
- Smith, M. Optical Vortices and Coherence in Nano-Optics; The University of North Carolina at Charlotte: Charlotte, NC, USA, 2019. [Google Scholar]
- Gan, C.H.; Gbur, G. Spatial Coherence Conversion with Surface Plasmons Using a Three-slit Interferometer. Plasmonics 2008, 3, 111–117. [Google Scholar] [CrossRef][Green Version]
- Wolf, E. New theory of partial coherence in the space–frequency domain. Part I: Spectra and cross spectra of steady-state sources. J. Opt. Soc. Am. 1982, 72, 343–351. [Google Scholar] [CrossRef]
- Foldy, L.L. The Multiple Scattering of Waves I. General Theory of Isotropic Scattering by Randomly Distributed Scatterers. Phys. Rev. 1945, 67, 107–119. [Google Scholar] [CrossRef]
- Lax, M. Multiple Scattering of Waves. II. The Effective Field in Dense Systems. Phys. Rev. 1952, 85, 621–629. [Google Scholar] [CrossRef]
- Etchegoin, P.G.; Le Ru, E.C.; Meyer, M. An analytic model for the optical properties of gold. J. Chem. Phys. 2006, 125, 164705. [Google Scholar] [CrossRef]
- Etchegoin, P.G.; Le Ru, E.C.; Meyer, M. Erratum: “An analytic model for the optical properties of gold” [J. Chem. Phys.125, 164705 (2006)]. J. Chem. Phys. 2007, 127, 189901. [Google Scholar] [CrossRef]
- Norrman, A.; Ponomarenko, S.A.; Friberg, A.T. Partially coherent surface plasmon polaritons. Europhys. Lett. 2016, 116, 64001. [Google Scholar] [CrossRef]
- Chen, Y.; Norrman, A.; Ponomarenko, S.A.; Friberg, A.T. Plasmon coherence determination by nanoscattering. Opt. Lett. 2017, 42, 3279–3282. [Google Scholar] [CrossRef]
- Chen, Y.; Norrman, A.; Ponomarenko, S.A.; Friberg, A.T. Coherence lattices in surface plasmon polariton fields. Opt. Lett. 2018, 43, 3429–3432. [Google Scholar] [CrossRef] [PubMed]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gbur, G.; Smith, M. Controlled Coherence Plasmonic Light Sources. Photonics 2021, 8, 268. https://doi.org/10.3390/photonics8070268
Gbur G, Smith M. Controlled Coherence Plasmonic Light Sources. Photonics. 2021; 8(7):268. https://doi.org/10.3390/photonics8070268
Chicago/Turabian StyleGbur, Greg, and Matt Smith. 2021. "Controlled Coherence Plasmonic Light Sources" Photonics 8, no. 7: 268. https://doi.org/10.3390/photonics8070268
APA StyleGbur, G., & Smith, M. (2021). Controlled Coherence Plasmonic Light Sources. Photonics, 8(7), 268. https://doi.org/10.3390/photonics8070268





