Numerical Simulation of Enhancement of Superficial Tumor Laser Hyperthermia with Silicon Nanoparticles
Abstract
1. Introduction
2. Materials and Methods
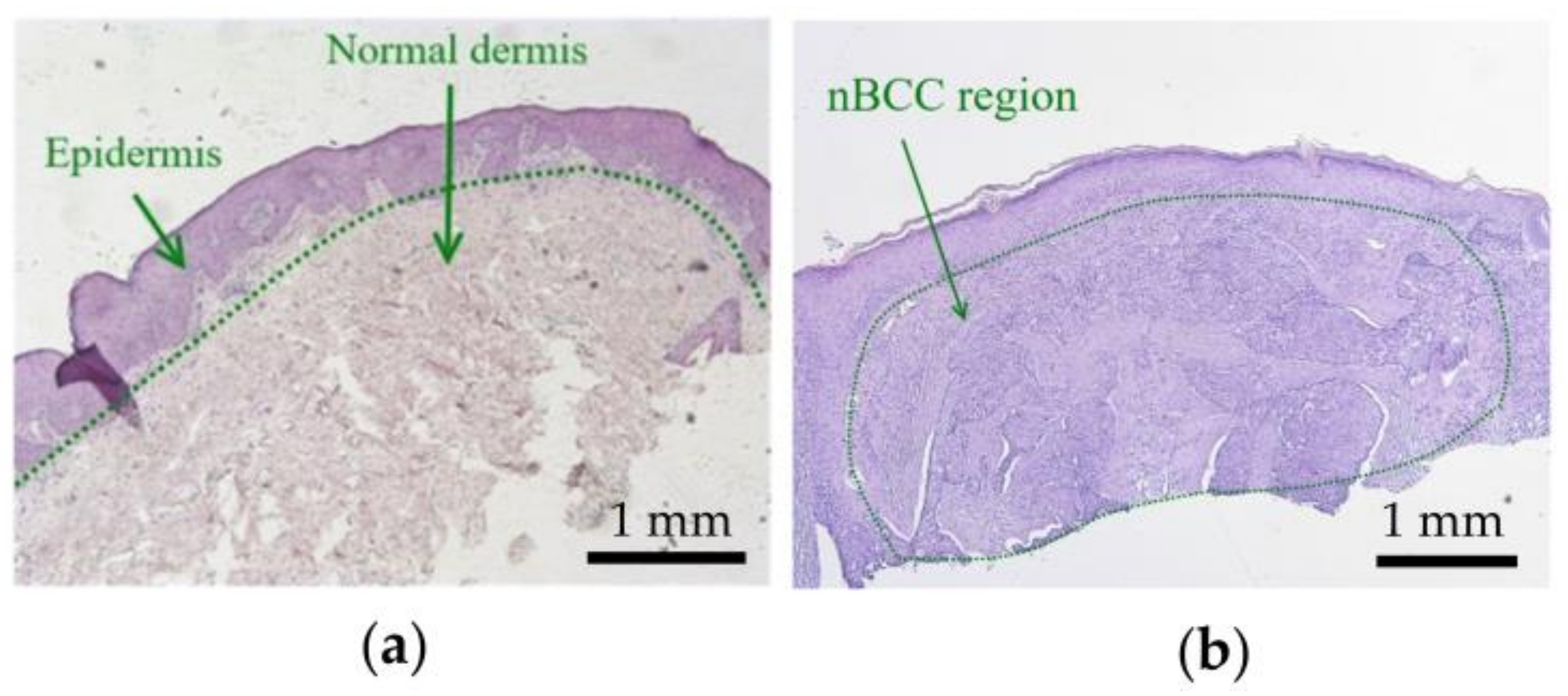
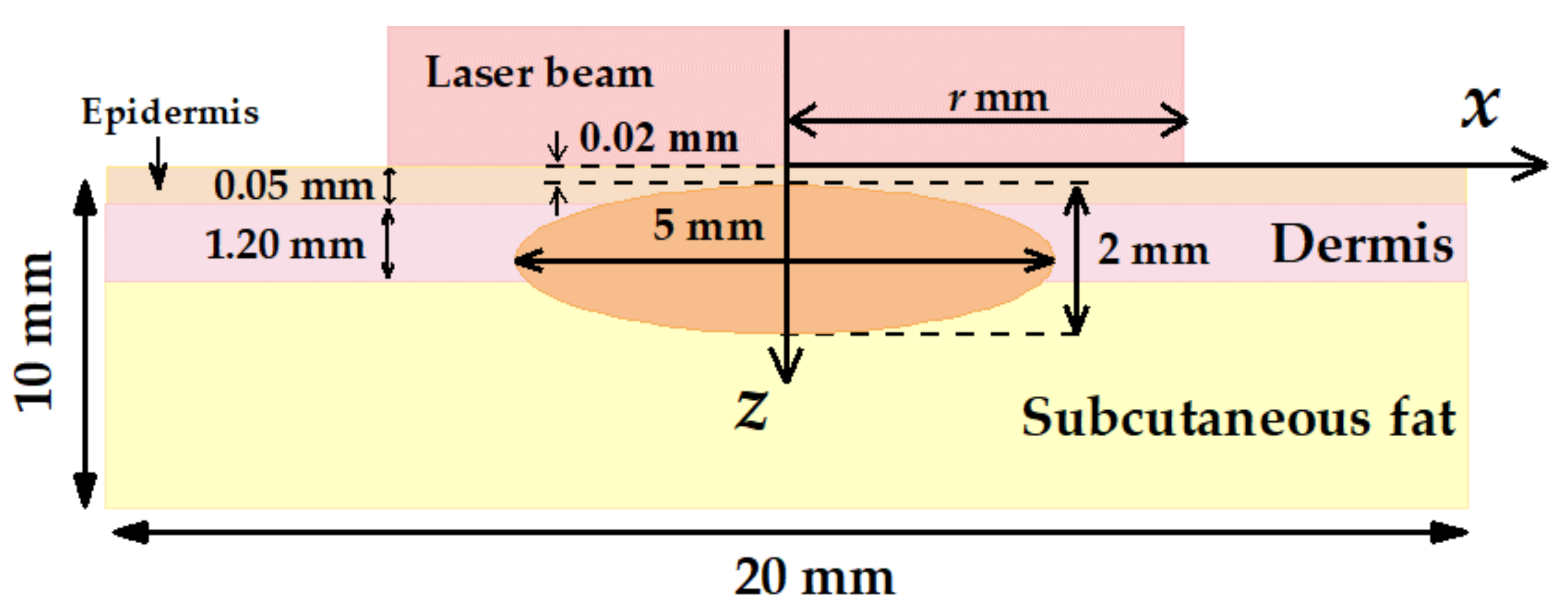
2.1. Geometry and Optical Parameters of the Model
2.2. Optical Characteristics of SiNPs Employed in the Simulations
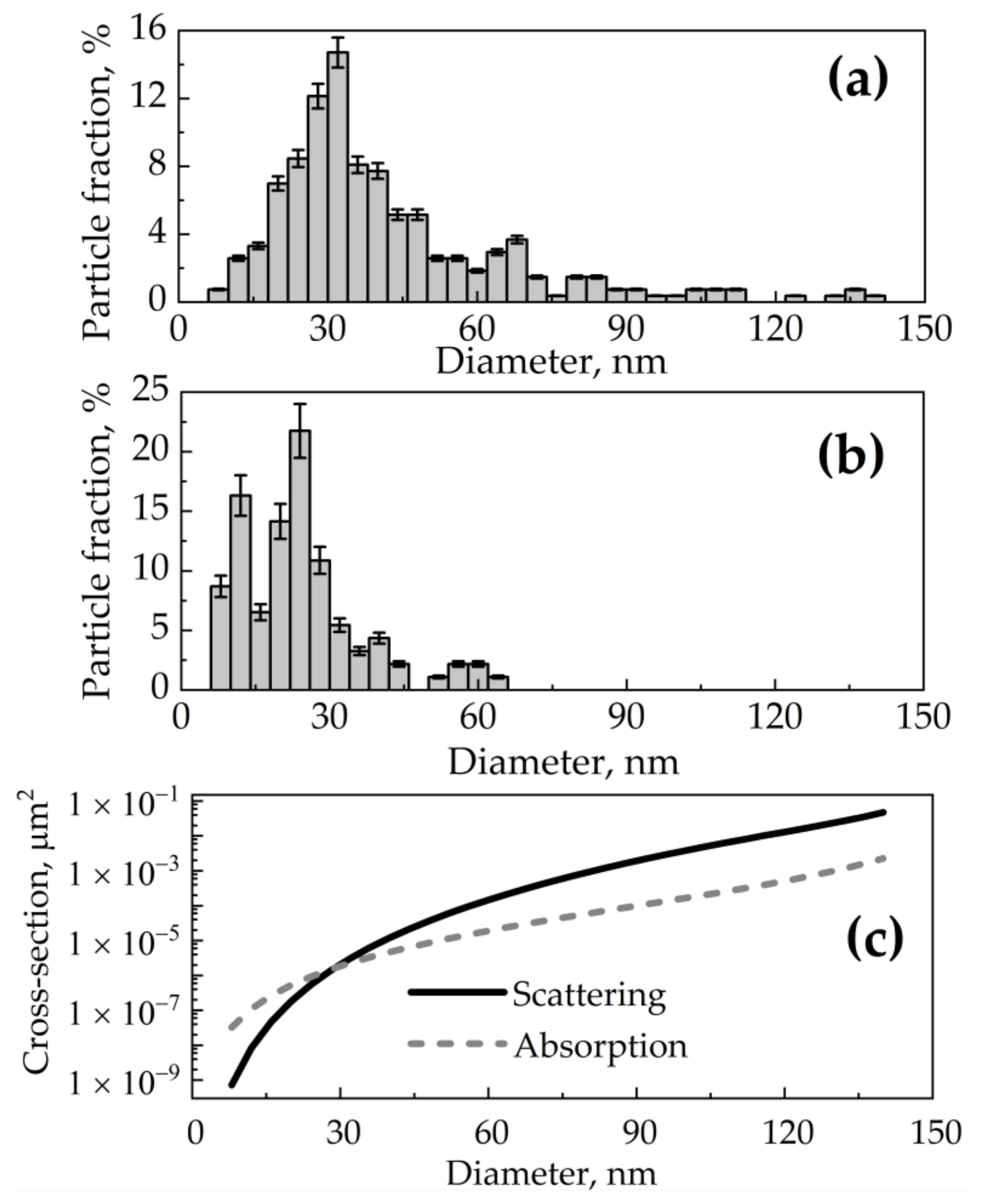
2.3. Simulations of Light Absorption Distribution
2.4. Calculation of Volumetric Temperature Distribution
3. Results and Discussion
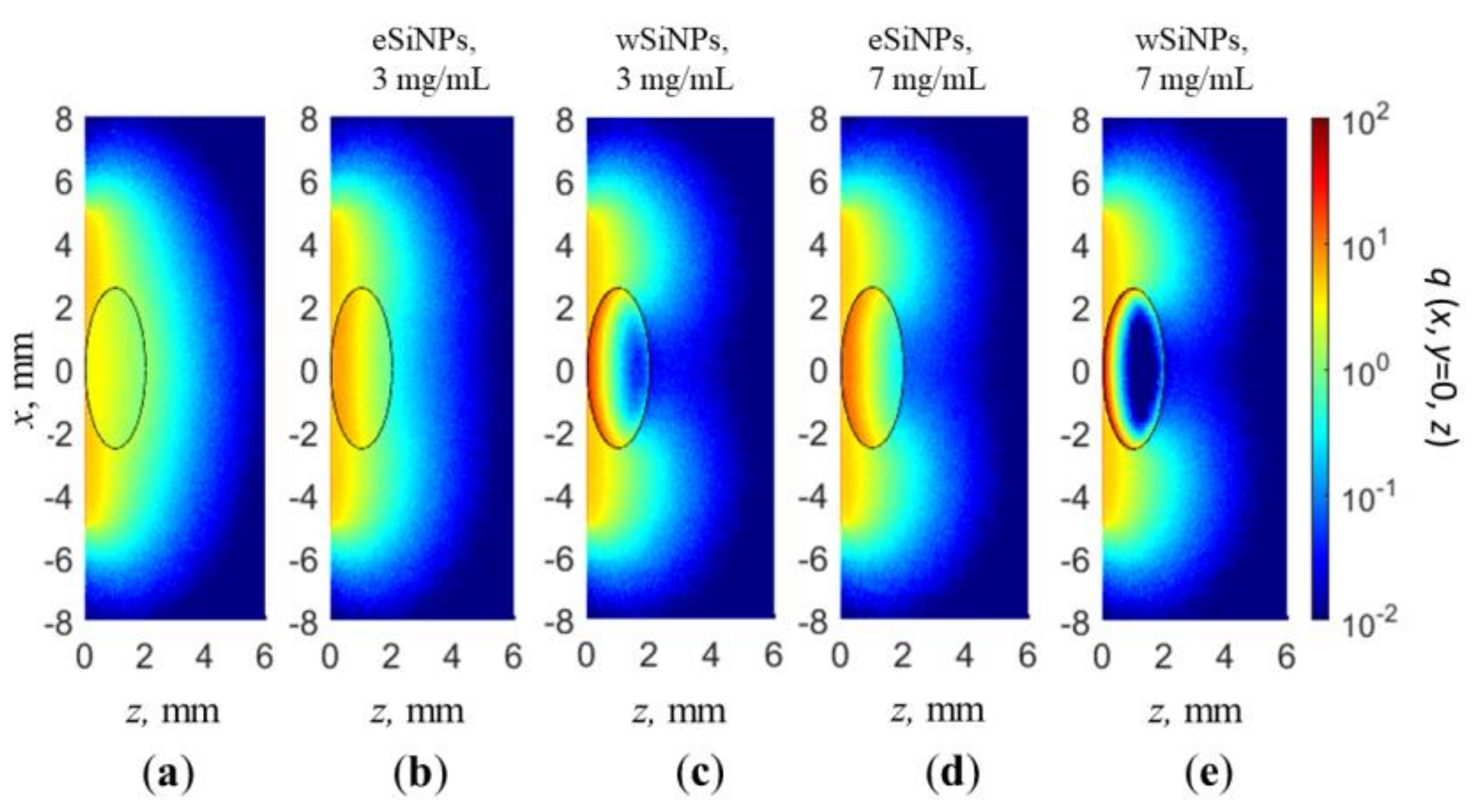
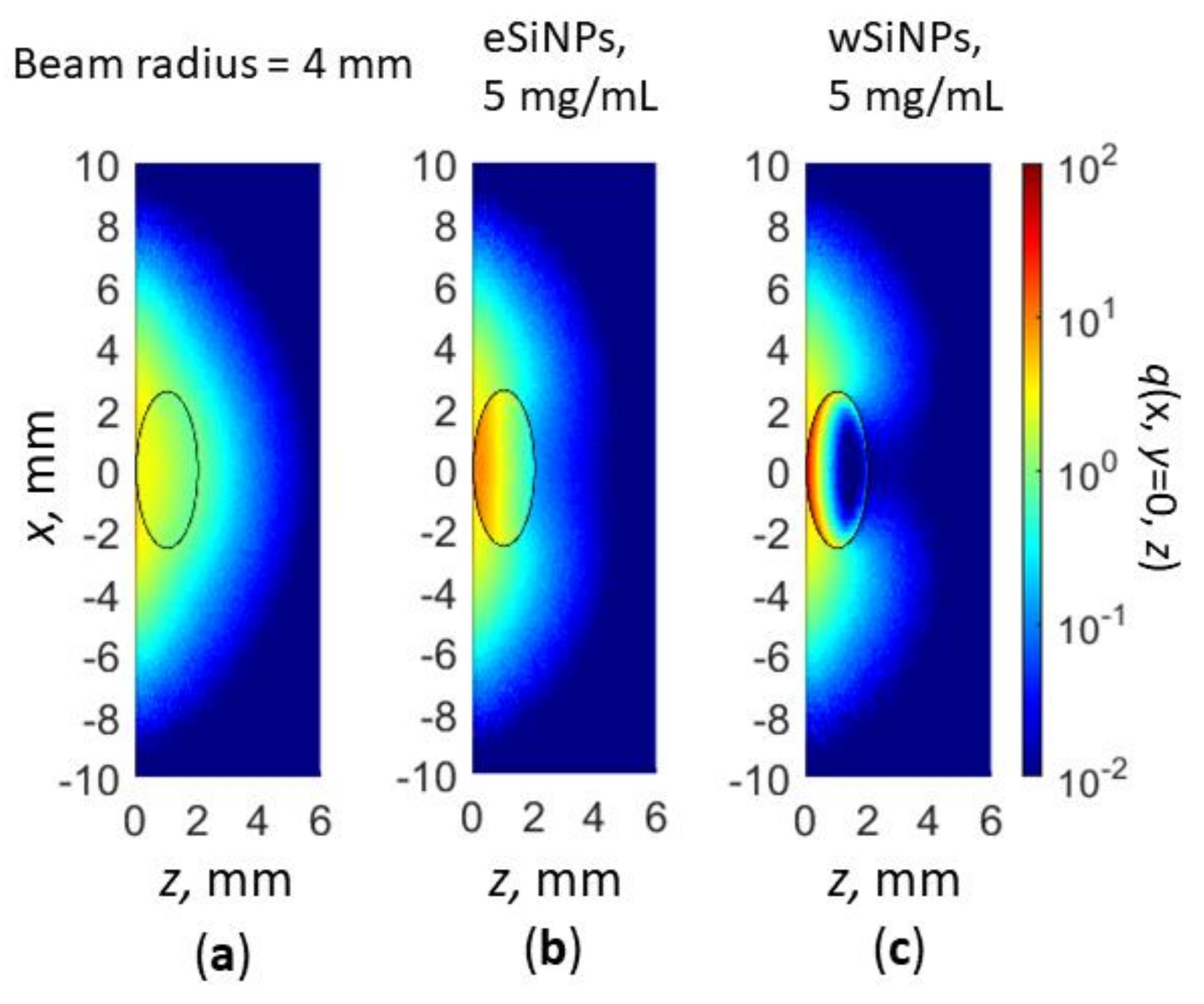
3.1. The Effect of eSiNPs and wSiNPs on Radiation Absorption Efficiency
3.2. The Effect of eSiNPs and wSiNPs on Tumor Heating
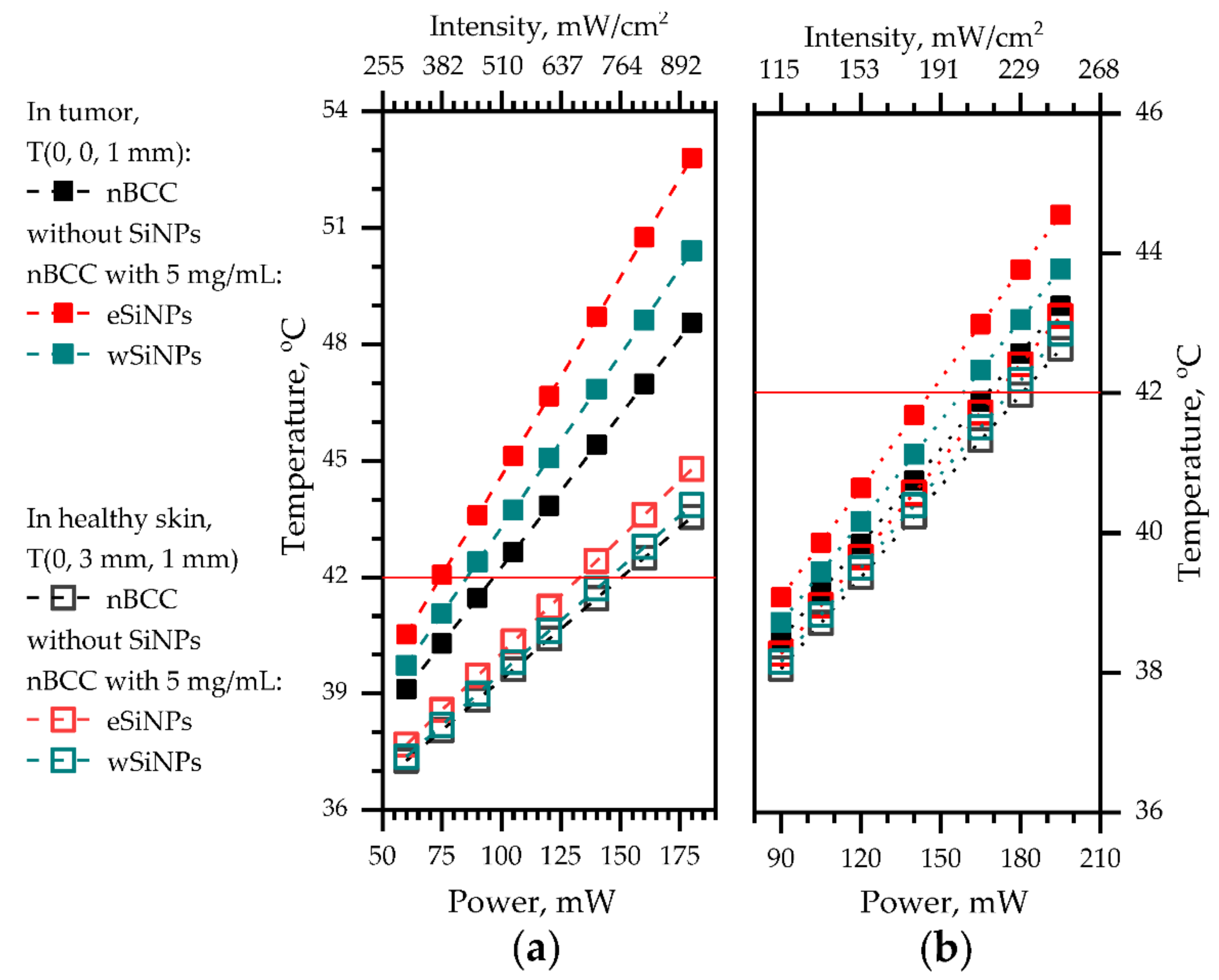
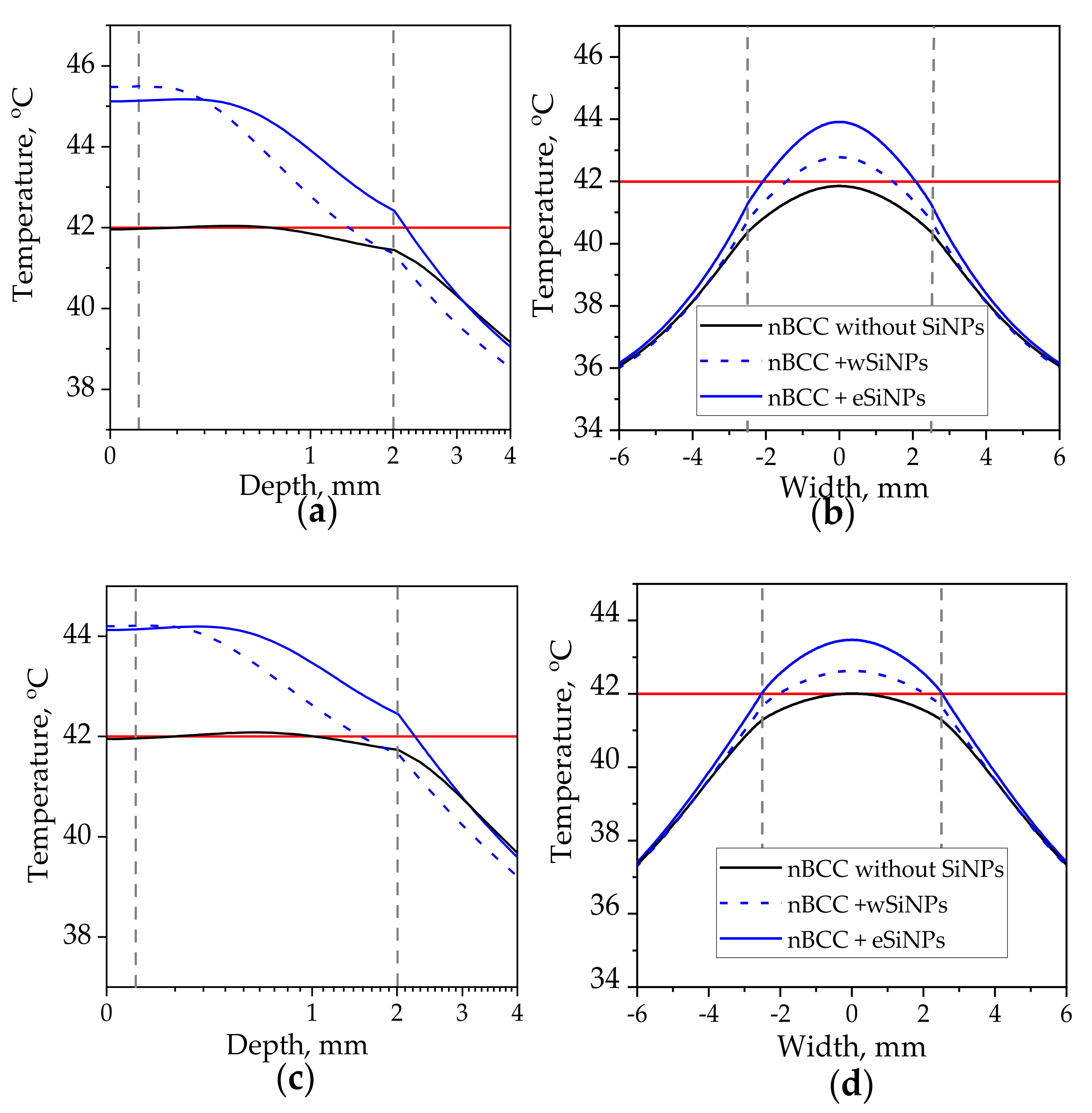
3.2.1. Beam Size Equals the Tumor Transversal Size
3.2.2. Beam Size Exceeds the Tumor Transversal Size
3.2.3. Comparison of Flat Beam and Gaussian Beam Irradiation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kim, J.H.; Hahn, E.W. Clinical and Biological Studies of Localized Hyperthermia. Cancer Res. 1979, 39, 2258–2261. [Google Scholar]
- Song, C.W.; Kang, M.S.; Rhee, J.G.; Levitt, S.H. Vascular Damage and Delayed Cell Death in Tumours after Hyperthermia. Br. J. Cancer 1980, 41, 309–312. [Google Scholar] [CrossRef]
- Szasz, A.; Szasz, N.; Szasz, O. Hyperthermia Results and Challenges. In Oncothermia: Principles and Practices; Springer: Dordrecht, The Netherlands, 2010; pp. 17–88. ISBN 978-90-481-9497-1. [Google Scholar]
- Chatterjee, D.K.; Diagaradjane, P.; Krishnan, S. Nanoparticle-Mediated Hyperthermia in Cancer Therapy. Ther. Deliv. 2011, 2, 1001–1014. [Google Scholar] [CrossRef]
- Tuchin, V.V. Volume 1: Light-Tissue Interaction. In Handbook of Optical Biomedical Diagnostics, 2nd ed.; SPIE: Bellingham, WA, USA, 2016. [Google Scholar]
- Ntziachristos, V.; Ripoll, J.; Wang, L.V.; Weissleder, R. Looking and Listening to Light: The Evolution of Whole-Body Photonic Imaging. Nat. Biotechnol. 2005, 23, 313–320. [Google Scholar] [CrossRef]
- Hu, Q.; Huang, Z.; Duan, Y.; Fu, Z.; Liu, B. Reprogramming Tumor Microenvironment with Photothermal Therapy. Bioconjugate Chem. 2020, 31, 1268–1278. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Lovell, J.F.; Yoon, J.; Chen, X. Clinical Development and Potential of Photothermal and Photodynamic Therapies for Cancer. Nat. Rev. Clin. Oncol. 2020, 17, 657–674. [Google Scholar] [CrossRef] [PubMed]
- Hwang, J.; Jin, J.-O. Attachable Hydrogel Containing Indocyanine Green for Selective Photothermal Therapy against Melanoma. Biomolecules 2020, 10, 1124. [Google Scholar] [CrossRef] [PubMed]
- Singh, S.P.; Alvi, S.B.; Pemmaraju, D.B.; Singh, A.D.; Manda, S.V.; Srivastava, R.; Rengan, A.K. NIR Triggered Liposome Gold Nanoparticles Entrapping Curcumin as in Situ Adjuvant for Photothermal Treatment of Skin Cancer. Int. J. Biol. Macromol. 2018, 110, 375–382. [Google Scholar] [CrossRef] [PubMed]
- Nomura, S.; Morimoto, Y.; Tsujimoto, H.; Arake, M.; Harada, M.; Saitoh, D.; Hara, I.; Ozeki, E.; Satoh, A.; Takayama, E.; et al. Highly Reliable, Targeted Photothermal Cancer Therapy Combined with Thermal Dosimetry Using a Near-Infrared Absorbent. Sci. Rep. 2020, 10, 9765. [Google Scholar] [CrossRef]
- Vervald, A.M.; Burikov, S.A.; Scherbakov, A.M.; Kudryavtsev, O.S.; Kalyagina, N.A.; Vlasov, I.I.; Ekimov, E.A.; Dolenko, T.A. Boron-Doped Nanodiamonds as Anticancer Agents: En Route to Hyperthermia/Thermoablation Therapy. ACS Biomater. Sci. Eng. 2020, 6, 4446–4453. [Google Scholar] [CrossRef] [PubMed]
- Fernandes, N.; Rodrigues, C.F.; Moreira, A.F.; Correia, I.J. Overview of the Application of Inorganic Nanomaterials in Cancer Photothermal Therapy. Biomater. Sci. 2020, 8, 2990–3020. [Google Scholar] [CrossRef]
- Hu, J.-J.; Cheng, Y.-J.; Zhang, X.-Z. Recent Advances in Nanomaterials for Enhanced Photothermal Therapy of Tumors. Nanoscale 2018, 10, 22657–22672. [Google Scholar] [CrossRef]
- Yao, C.; Zhang, L.; Wang, J.; He, Y.; Xin, J.; Wang, S.; Xu, H.; Zhang, Z. Gold Nanoparticle Mediated Phototherapy for Cancer. J. Nanomater. 2016, 2016, 5497136. [Google Scholar] [CrossRef]
- Maksimova, I.L.; Akchurin, G.G.; Khlebtsov, B.N.; Terentyuk, G.S.; Akchurin, G.G.; Ermolaev, I.A.; Skaptsov, A.A.; Soboleva, E.P.; Khlebtsov, N.G.; Tuchin, V.V. Near-Infrared Laser Photothermal Therapy of Cancer by Using Gold Nanoparticles: Computer Simulations and Experiment. Med. Laser Appl. 2007, 22, 199–206. [Google Scholar] [CrossRef]
- Jeynes, J.C.G.; Wordingham, F.; Moran, L.J.; Curnow, A.; Harries, T.J. Monte Carlo Simulations of Heat Deposition During Photothermal Skin Cancer Therapy Using Nanoparticles. Biomolecules 2019, 9, 343. [Google Scholar] [CrossRef]
- Dimitriou, N.M.; Pavlopoulou, A.; Tremi, I.; Kouloulias, V.; Tsigaridas, G.; Georgakilas, A.G. Prediction of Gold Nanoparticle and Microwave-Induced Hyperthermia Effects on Tumor Control via a Simulation Approach. Nanomaterials 2019, 9, 167. [Google Scholar] [CrossRef] [PubMed]
- Kirillin, M.; Shirmanova, M.; Sirotkina, M.; Bugrova, M.; Khlebtsov, B.; Zagaynova, E. Contrasting Properties of Gold Nanoshells and Titanium Dioxide Nanoparticles for Optical Coherence Tomography Imaging of Skin: Monte Carlo Simulations and In Vivo Study. J. Biomed. Opt. 2009, 14, 021017. [Google Scholar] [CrossRef] [PubMed]
- Zagainova, E.V.; Sirotkina, M.A.; Shirmanova, M.V.; Elagin, V.V.; Kirillin, M.; Agrba, P.; Kamensky, V.A.; Nadtochenko, V.A. Plasmon Resonance Gold Nanoparticles for Improving Optical Diagnostics and Photothermal Therapy of Tumor. In Proceedings of the Plasmonics in Biology and Medicine VII, San Francisco, CA, USA, 23–28 January 2010; Vo-Dinh, T., Lakowicz, J.R., Eds.; SPIE: Bellingham, WA, USA, 2010; p. 75770. [Google Scholar] [CrossRef]
- Zagaynova, E.V.; Shirmanova, M.V.; Kirillin, M.Y.; Khlebtsov, B.N.; Orlova, A.G.; Balalaeva, I.V.; Sirotkina, M.A.; Bugrova, M.L.; Agrba, P.D.; Kamensky, V.A. Contrasting Properties of Gold Nanoparticles for Optical Coherence Tomography: Phantom, in Vivo studies and Monte Carlo Simulation. Phys. Med. Biol. 2008, 53, 4995–5009. [Google Scholar] [CrossRef]
- Singh, P.; Pandit, S.; Mokkapati, V.R.S.S.; Garg, A.; Ravikumar, V.; Mijakovic, I. Gold Nanoparticles in Diagnostics and Therapeutics for Human Cancer. Int. J. Mol. Sci. 2018, 19, 1979. [Google Scholar] [CrossRef]
- Sani, A.; Cao, C.; Cui, D. Toxicity of Gold Nanoparticles (AuNPs): A Review. Biochem. Biophys. Rep. 2021, 26, 100991. [Google Scholar] [CrossRef]
- Liu, J.; Peng, Q. Protein-Gold Nanoparticle Interactions and Their Possible Impact on Biomedical Applications. Acta Biomater. 2017, 55, 13–27. [Google Scholar] [CrossRef] [PubMed]
- Zhou, J.; Cao, Z.; Panwar, N.; Hu, R.; Wang, X.; Qu, J.; Tjin, S.C.; Xu, G.; Yong, K.-T. Functionalized Gold Nanorods for Nanomedicine: Past, Present and Future. Coord. Chem. Rev. 2017, 352, 15–66. [Google Scholar] [CrossRef]
- Liu, A.; Wang, G.; Wang, F.; Zhang, Y. Gold Nanostructures with Near-Infrared Plasmonic Resonance: Synthesis and Surface Functionalization. Coord. Chem. Rev. 2017, 336, 28–42. [Google Scholar] [CrossRef]
- Simakin, A.V.; Baimler, I.V.; Smirnova, V.V.; Uvarov, O.V.; Kozlov, V.A.; Gudkov, S.V. Evolution of the Size Distribution of Gold Nanoparticles under Laser Irradiation. Phys. Wave Phenom. 2021, 29, 102–107. [Google Scholar] [CrossRef]
- Simakin, A.V.; Astashev, M.E.; Baimler, I.V.; Uvarov, O.V.; Voronov, V.V.; Vedunova, M.V.; Sevost’yanov, M.A.; Belosludtsev, K.N.; Gudkov, S.V. The Effect of Gold Nanoparticle Concentration and Laser Fluence on the Laser-Induced Water Decomposition. J. Phys. Chem. B 2019, 123, 1869–1880. [Google Scholar] [CrossRef] [PubMed]
- Al-Kattan, A.; Nirwan, V.; Popov, A.; Ryabchikov, Y.; Strelkov, G.; Sentis, M.; Fahmi, A.; Kabashin, A. Recent Advances in Laser-Ablative Synthesis of Bare Au and Si Nanoparticles and Assessment of Their Prospects for Tissue Engineering Applications. Int. J. Mol. Sci. 2018, 19, 1563. [Google Scholar] [CrossRef] [PubMed]
- Braguer, D.; Correard, F.; Maximova, K.; Villard, C.; Roy, M.; Al-Kattan, A.; Sentis, M.; Gingras, M.; Kabashin, A.; Esteve, M.-A. Gold Nanoparticles Prepared by Laser Ablation in Aqueous Biocompatible Solutions: Assessment of Safety and Biological Identity for Nanomedicine Applications. Int. J. Nanomed. 2014, 9, 5415. [Google Scholar] [CrossRef] [PubMed]
- Canham, L. Handbook of Porous Silicon; Springer: New York, NY, USA, 2014; ISBN 978-3-319-05743-9. [Google Scholar]
- Ksenofontova, O.I.; Vasin, A.V.; Egorov, V.V.; Bobyl’, A.V.; Soldatenkov, F.Y.; Terukov, E.I.; Ulin, V.P.; Ulin, N.V.; Kiselev, O.I. Porous Silicon and Its Applications in Biology and Medicine. Tech. Phys. 2014, 59, 66–77. [Google Scholar] [CrossRef]
- Osminkina, L.A.; Tamarov, K.P.; Sviridov, A.P.; Galkin, R.A.; Gongalsky, M.B.; Solovyev, V.V.; Kudryavtsev, A.A.; Timoshenko, V.Y. Photoluminescent Biocompatible Silicon Nanoparticles for Cancer Theranostic Applications. J. Biophotonics 2012, 5, 529–535. [Google Scholar] [CrossRef]
- Zabotnov, S.V.; Skobelkina, A.V.; Kashaev, F.V.; Kolchin, A.V.; Popov, V.V.; Presnov, D.E.; Sergeeva, E.A.; Kirillin, M.Y.; Golovan, L.A. Pulsed Laser Ablation of Silicon Nanowires in Water and Ethanol. SSP 2020, 312, 200–205. [Google Scholar] [CrossRef]
- Skobelkina, A.V.; Kashaev, F.V.; Kolchin, A.V.; Shuleiko, D.V.; Kaminskaya, T.P.; Presnov, D.E.; Golovan, L.V.; Kashkarov, P.K. Silicon Nanoparticles Formed via Pulsed Laser Ablation of Porous Silicon in Liquids. Tech. Phys. Lett. 2020, 46, 687–690. [Google Scholar] [CrossRef]
- Kuzmin, P.G.; Shafeev, G.A.; Bukin, V.V.; Garnov, S.V.; Farcau, C.; Carles, R.; Warot-Fontrose, B.; Guieu, V.; Viau, G. Silicon Nanoparticles Produced by Femtosecond Laser Ablation in Ethanol: Size Control, Structural Characterization, and Optical Properties. J. Phys. Chem. C 2010, 114, 15266–15273. [Google Scholar] [CrossRef]
- Lee, C.; Kim, H.; Hong, C.; Kim, M.; Hong, S.S.; Lee, D.H.; Lee, W.I. Porous Silicon as an Agent for Cancer Thermotherapy Based on Near-Infrared Light Irradiation. J. Mater. Chem. 2008, 18, 4790. [Google Scholar] [CrossRef]
- Hong, C.; Lee, J.; Zheng, H.; Hong, S.-S.; Lee, C. Porous Silicon Nanoparticles for Cancer Photothermotherapy. Nanoscale Res. Lett. 2011, 6, 321. [Google Scholar] [CrossRef] [PubMed]
- Sokolovskaya, O.I.; Zabotnov, S.V.; Golovan, L.A.; Kashkarov, P.K.; Kurakina, D.A.; Sergeeva, E.A.; Kirillin, M.Y. Prospects for Using Silicon Nanoparticles Fabricated by Laser Ablation in Hyperthermia of Tumours. Quantum Electron. 2021, 51, 64–72. [Google Scholar] [CrossRef]
- Martínez-Carmona, M.; Vallet-Regí, M. Advances in Laser Ablation Synthesized Silicon-Based Nanomaterials for the Prevention of Bacterial Infection. Nanomaterials 2020, 10, 1443. [Google Scholar] [CrossRef]
- Serrano-Ruz, J.A.; Quiñones-Galván, J.G.; Santos-Cruz, J.; de Moure-Flores, F.; Campos-González, E.; Chavez Chavez, A.; Gómez-Rosas, G. Synthesis of Silicon Nanoparticles by Laser Ablation at Low Fluences in Water and Ethanol. Mater. Res. Express 2020. [Google Scholar] [CrossRef]
- Al-Kattan, A.; Ryabchikov, Y.V.; Baati, T.; Chirvony, V.; Sánchez-Royo, J.F.; Sentis, M.; Braguer, D.; Timoshenko, V.Y.; Estève, M.-A.; Kabashin, A.V. Ultrapure Laser-Synthesized Si Nanoparticles with Variable Oxidation States for Biomedical Applications. J. Mater. Chem. B 2016, 4, 7852–7858. [Google Scholar] [CrossRef] [PubMed]
- Oleshchenko, V.A.; Kharin, A.Y.; Alykova, A.F.; Karpukhina, O.V.; Karpov, N.V.; Popov, A.A.; Bezotosnyi, V.V.; Klimentov, S.M.; Zavestovskaya, I.N.; Kabashin, A.V.; et al. Localized Infrared Radiation-Induced Hyperthermia Sensitized by Laser-Ablated Silicon Nanoparticles for Phototherapy Applications. Appl. Surf. Sci. 2020, 516, 145661. [Google Scholar] [CrossRef]
- Zabotnov, S.V.; Skobelkina, A.V.; Sergeeva, E.A.; Kurakina, D.A.; Khilov, A.V.; Kashaev, F.V.; Kaminskaya, T.P.; Presnov, D.E.; Agrba, P.D.; Shuleiko, D.V.; et al. Nanoparticles Produced via Laser Ablation of Porous Silicon and Silicon Nanowires for Optical Bioimaging. Sensors 2020, 20, 4874. [Google Scholar] [CrossRef] [PubMed]
- Gongalsky, M.B.; Kharin, A.Y.; Osminkina, L.A.; Timoshenko, V.Y.; Jeong, J.; Lee, H.; Chung, B.H. Enhanced Photoluminescence of Porous Silicon Nanoparticles Coated by Bioresorbable Polymers. Nanoscale Res. Lett. 2012, 7, 446. [Google Scholar] [CrossRef]
- Huang, Z.; Geyer, N.; Werner, P.; de Boor, J.; Gösele, U. Metal-Assisted Chemical Etching of Silicon: A Review. Adv. Mater. 2011, 23, 285–308. [Google Scholar] [CrossRef] [PubMed]
- Osminkina, L.A.; Gonchar, K.A.; Marshov, V.S.; Bunkov, K.V.; Petrov, D.V.; Golovan, L.A.; Talkenberg, F.; Sivakov, V.A.; Timoshenko, V.Y. Optical Properties of Silicon Nanowire Arrays Formed by Metal-Assisted Chemical Etching: Evidences for Light Localization Effect. Nanoscale Res. Lett. 2012, 7, 524. [Google Scholar] [CrossRef]
- Efimova, A.; Eliseev, A.; Georgobiani, V.; Kholodov, M.; Kolchin, A.; Presnov, D.; Tkachenko, N.; Zabotnov, S.; Golovan, L.; Kashkarov, P. Enhanced Photon Lifetime in Silicon Nanowire Arrays and Increased Efficiency of Optical Processes in Them. Opt. Quantum Electron. 2016, 48, 232. [Google Scholar] [CrossRef]
- Cigna, E.; Tarallo, M.; Maruccia, M.; Sorvillo, V.; Pollastrini, A.; Scuderi, N. Basal Cell Carcinoma: 10 Years of Experience. J. Ski. Cancer 2011, 2011, 476362. [Google Scholar] [CrossRef]
- ANSI Z136.1–2014—American National Standard for Safe Use of Lasers; Laser Institute of America: Orlando, FL, USA, 2014.
- Jean, M.; Schulmeister, K. Laser-Induced Injury of the Skin: Validation of a Computer Model to Predict Thresholds. Biomed. Opt. Express 2021, 12, 2586. [Google Scholar] [CrossRef]
- Bristow, A.D.; Rotenberg, N.; van Driel, H.M. Two-Photon Absorption and Kerr Coefficients of Silicon for 850–2200 nm. Appl. Phys. Lett. 2007, 90, 191104. [Google Scholar] [CrossRef]
- Costa-Almeida, R.; Bogas, D.; Fernandes, J.R.; Timochenco, L.; Silva, F.A.L.S.; Meneses, J.; Gonçalves, I.C.; Magalhães, F.D.; Pinto, A.M. Near-Infrared Radiation-Based Mild Photohyperthermia Therapy of Non-Melanoma Skin Cancer with PEGylated Reduced Nanographene Oxide. Polymers 2020, 12, 1840. [Google Scholar] [CrossRef]
- Zheng, Y.; Wang, W.; Zhao, J.; Wu, C.; Ye, C.; Huang, M.; Wang, S. Preparation of injectable temperature-sensitive chitosan-based hydrogel for combined hyperthermia and chemotherapy of colon cancer. Carbohydr. Polym. 2019, 222, 115039. [Google Scholar] [CrossRef] [PubMed]
- Yuan, W.; Xu, C.; Xue, L.; Pang, H.; Cao, A.; Fu, Y.; Deng, Q. Integrated Double-Sided Random Microlens Array Used for Laser Beam Homogenization. Micromachines 2021, 12, 673. [Google Scholar] [CrossRef] [PubMed]
- Green, M.A.; Keevers, M.J. Optical Properties of Intrinsic Silicon at 300 K. Prog. Photovolt. Res. Appl. 1995, 3, 189–192. [Google Scholar] [CrossRef]
- Salomatina, E.; Jiang, B.; Novak, J.; Yaroslavsky, A.N. Optical Properties of Normal and Cancerous Human Skin in the Visible and Near-Infrared Spectral Range. J. Biomed. Opt. 2006, 11, 064026. [Google Scholar] [CrossRef] [PubMed]
- Chopra, K.; Calva, D.; Sosin, M.; Tadisina, K.K.; Banda, A.; De La Cruz, C.; Chaudhry, M.R.; Legesse, T.; Drachenberg, C.B.; Manson, P.N.; et al. A Comprehensive Examination of Topographic Thickness of Skin in the Human Face. Aesthet. Surg. J. 2015, 35, 1007–1013. [Google Scholar] [CrossRef] [PubMed]
- Oltulu, P.; Ince, B.; Kokbudak, N.; Findik, S.; Kilinc, F. Measurement of Epidermis, Dermis, and Total Skin Thicknesses from Six Different Body Regions with a New Ethical Histometric Technique. Turk. J. Plast. Surg. 2018, 26, 56. [Google Scholar] [CrossRef]
- Gongalsky, M.B.; Osminkina, L.A.; Pereira, A.; Manankov, A.A.; Fedorenko, A.A.; Vasiliev, A.N.; Solovyev, V.V.; Kudryavtsev, A.A.; Sentis, M.; Kabashin, A.V.; et al. Laser-Synthesized Oxide-Passivated Bright Si Quantum Dots for Bioimaging. Sci. Rep. 2016, 6, 24732. [Google Scholar] [CrossRef] [PubMed]
- Zabotnov, S.V.; Kolchin, A.V.; Kashaev, F.V.; Skobelkina, A.V.; Nesterov, V.Y.; Presnov, D.E.; Golovan, L.A.; Kashkarov, P.K. Structural Analysis of Nanoparticles Formed via Laser Ablation of Porous Silicon and Silicon Microparticles in Water. Tech. Phys. Lett. 2019, 45, 1085–1088. [Google Scholar] [CrossRef]
- Hull, R. Properties of Crystalline Silicon; INSPEC: London, UK, 1999. [Google Scholar]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Ishimaru, A. Wave Propagation and Scattering in Random Media; Academic Press: New York, NY, USA, 1978. [Google Scholar]
- Barbagiovanni, E.G.; Lockwood, D.J.; Simpson, P.J.; Goncharova, L.V. Quantum Confinement in Si and Ge Nanostructures. J. Appl. Phys. 2012, 111, 034307. [Google Scholar] [CrossRef]
- Aspnes, D.E.; Studna, A.A. Dielectric Functions and Optical Parameters of Si, Ge, GaP, GaAs, GaSb, InP, InAs, and InSb from 1.5 to 6.0 eV. Phys. Rev. B 1983, 27, 985. [Google Scholar] [CrossRef]
- Jellison, G.E.; Modine, F.A. Optical absorption of silicon between 1.6 and 4.7 eV at elevated temperatures. Appl. Phys. Lett. 1982, 41, 180–182. [Google Scholar] [CrossRef]
- Jellison, G.E.; Burke, H.H. The temperature dependence of the refractive index of silicon at elevated temperatures at severed laser wavelengths. J. Appl. Phys. 1986, 60, 841–843. [Google Scholar] [CrossRef]
- Iorizzo, T.W.; Jermain, P.R.; Salomatina, E.; Muzikansky, A.; Yaroslavsky, A.N. Temperature induced changes in the optical properties of skin in vivo. Sci. Rep. 2021, 11, 754. [Google Scholar] [CrossRef] [PubMed]
- Lihong, W. Scattering and Its Biological Origins. Ch. 1.5 Pp 8. Biomedical Optics; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Bolin, F.P.; Preuss, L.E.; Taylor, R.C.; Ference, R.J. Refractive Index of Some Mammalian Tissues. Appl. Opt. 1989, 28, 2297–2303. [Google Scholar] [CrossRef]
- Brocato, T.A.; Coker, E.N.; Durfee, P.N.; Lin, Y.-S.; Townson, J.; Wyckoff, E.F.; Cristini, V.; Brinker, C.J.; Wang, Z. Understanding the Connection between Nanoparticle Uptake and Cancer Treatment Efficacy Using Mathematical Modeling. Sci. Rep. 2018, 8, 7538. [Google Scholar] [CrossRef]
- Wilhelm, S.; Tavares, A.J.; Dai, Q.; Ohta, S.; Audet, J.; Dvorak, H.F.; Chan, W.C.W. Analysis of Nanoparticle Delivery to Tumours. Nat. Rev. Mater. 2016, 1, 16014. [Google Scholar] [CrossRef]
- Krylov, I.V.; Akasov, R.A.; Rocheva, V.V.; Sholina, N.V.; Khochenkov, D.A.; Nechaev, A.V.; Melnikova, N.V.; Dmitriev, A.A.; Ivanov, A.V.; Generalova, A.N.; et al. Local Overheating of Biotissue Labeled with Upconversion Nanoparticles Under Yb3+ Resonance Excitation. Front. Chem. 2020, 8, 295. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Jacques, S.L.; Zheng, L. MCML—Monte Carlo Modeling of Light Transport in Multi-Layered Tissues. Comput. Methods Programs Biomed. 1995, 47, 131–146. [Google Scholar] [CrossRef]
- Loginova, D.; Sergeeva, E.; Fiks, I.; Kirillin, M. Probing Depth in Diffuse Optical Spectroscopy and Structured Illumination Imaging: A Monte Carlo Study. JBPE 2017, 3, 010303. [Google Scholar] [CrossRef]
- Manuchehrabadi, N.; Chen, Y.; Lebrun, A.; Ma, R.; Zhu, L. Computational Simulation of Temperature Elevations in Tumors Using Monte Carlo Method and Comparison to Experimental Measurements in Laser Photothermal Therapy. J. Biomech. Eng. 2013, 135, 121007. [Google Scholar] [CrossRef] [PubMed]
- Kurakina, D.; Khilov, A.; Shakhova, M.; Orlinskaya, N.; Sergeeva, E.; Meller, A.; Turchin, I.; Kirillin, M. Comparative Analysis of Single- and Dual-Wavelength Photodynamic Therapy Regimes with Chlorin-Based Photosensitizers: Animal Study. J. Biomed. Opt. 2019, 25, 063804. [Google Scholar] [CrossRef]
- Mironycheva, A.M.; Kirillin, M.Y.; Khilov, A.V.; Malygina, A.S.; Kurakina, D.A.; Gutakovskaya, V.N.; Turchin, I.V.; Orlinskaya, N.Y.; Shlivko, I.L.; Klemenova, I.A.; et al. Combined Application of Dual-Wavelength Fluorescence Monitoring and Contactless Thermometry during Photodynamic Therapy of Basal Cell Skin Cancer. Sovrem. Tehnol. Med. 2020, 12, 47. [Google Scholar] [CrossRef]
- Pennes, H.H. Analysis of Tissue and Arterial Blood Temperatures in the Resting Human Forearm. J. Appl. Physiol. 1948, 1, 93–122. [Google Scholar] [CrossRef]
- Tunç, M.; Çamdali, Ü.; Parmaksizoğlu, C.; Çikrikçi, S. The Bio-heat Transfer Equation and Its Applications in Hyperthermia Treatments. Eng. Comput. 2006, 23, 451–463. [Google Scholar] [CrossRef]
- Ricketts, P.L.; Mudaliar, A.V.; Ellis, B.E.; Pullins, C.A.; Meyers, L.A.; Lanz, O.I.; Scott, E.P.; Diller, T.E. Non-Invasive Blood Perfusion Measurements Using a Combined Temperature and Heat Flux Surface Probe. Int. J. Heat Mass Transf. 2008, 51, 5740–5748. [Google Scholar] [CrossRef] [PubMed]
- Banerjee, A.; Ogale, A.A.; Das, C.; Mitra, K.; Subramanian, C. Temperature Distribution in Different Materials Due to Short Pulse Laser Irradiation. Heat Transf. Eng. 2005, 26, 41–49. [Google Scholar] [CrossRef]
- Wang, X.; Qi, H.; Yang, X.; Xu, H. Analysis of the Time-Space Fractional Bioheat Transfer Equation for Biological Tissues during Laser Irradiation. Int. J. Heat Mass Transf. 2021, 177, 121555. [Google Scholar] [CrossRef]
- Liu, K.-C.; Chen, T.-M. Comparative Study of Heat Transfer and Thermal Damage Assessment Models for Hyperthermia Treatment. J. Therm. Biol. 2021, 98, 102907. [Google Scholar] [CrossRef]
- Xu, F.; Lu, T.J.; Seffen, K.A.; Ng, E.Y.K. Mathematical Modeling of Skin Bioheat Transfer. Appl. Mech. Rev. 2009, 62, 050801. [Google Scholar] [CrossRef]
- Cheng, T.-Y.; Herman, C. Optimization of Skin Cooling for Thermographic Imaging of Near-Surface Lesions. In Proceedings of the ASME 2011 International Mechanical Engineering Congress and Exposition, Denver, CO, USA, 11–17 November 2011; ASMEDC: New York, NY, USA, 2012; Volume 2, pp. 351–360. [Google Scholar] [CrossRef]
- Carberry, P.A.; Shepherd, A.M.; Johnson, J.M. Resting and Maximal Forearm Skin Blood Flows Are Reduced in Hypertension. Hypertension 1992, 20, 349–355. [Google Scholar] [CrossRef]
- Suleman, M.; Riaz, S. 3D in Silico Study of Magnetic Fluid Hyperthermia of Breast Tumor Using Fe3O4 Magnetic Nanoparticles. J. Therm. Biol. 2020, 91, 102635. [Google Scholar] [CrossRef]
- Patil, H.M.; Maniyeri, R. Finite Difference Method Based Analysis of Bio-Heat Transfer in Human Breast Cyst. Therm. Sci. Eng. Prog. 2019, 10, 42–47. [Google Scholar] [CrossRef]
- Qiu, L.; Zhu, N.; Feng, Y.; Michaelides, E.E.; Żyła, G.; Jing, D.; Zhang, X.; Norris, P.M.; Markides, C.N.; Mahian, O. A review of recent advances in thermophysical properties at the nanoscale: From solid state to colloids. Phys. Rep. 2020, 843, 1–81. [Google Scholar] [CrossRef]
- Priester, M.I.; Curto, S.; van Rhoon, G.C.; ten Hagen, T.L.M. External Basic Hyperthermia Devices for Preclinical Studies in Small Animals. Cancers 2021, 13, 4628. [Google Scholar] [CrossRef]
- Bass, L.; Nikolaeva, O.; Bykov, A.; Kirillin, M. Finite Difference Methods for Solving the Transport Equation in the Problems of Optical Biomedical Diagnostics. JBPE 2017, 3, 010311. [Google Scholar] [CrossRef]
- Deng, Z.-S.; Liu, J. Numerical Simulation of 3-D Freesing and Heating Problems for Combined Cryosurgery and Hyperthermia Therapy. Numer. Heat Transf. Part A Appl. 2004, 46, 587–611. [Google Scholar] [CrossRef]
- Gnyawali, S.C.; Chen, Y.; Wu, F.; Bartels, K.E.; Wicksted, J.P.; Liu, H.; Sen, C.K.; Chen, W.R. Temperature Measurement on Tissue Surface during Laser Irradiation. Med. Biol. Eng. Comput. 2008, 46, 159–168. [Google Scholar] [CrossRef] [PubMed]
- Ng, E.Y.K.; Tan, H.M.; Ooi, E.H. Boundary Element Method with Bioheat Equation for Skin Burn Injury. Burns 2009, 35, 987–997. [Google Scholar] [CrossRef] [PubMed]
- Torvi, D.A.; Dale, J.D. A Finite Element Model of Skin Subjected to a Flash Fire. J. Biomech. Eng. 1994, 116, 250–255. [Google Scholar] [CrossRef] [PubMed]
- Kengne, E.; Mellal, I.; Hamouda, M.B.; Lakhssassi, A. A Mathematical Model to Solve Bio-Heat Transfer Problems through a Bio-Heat Transfer Equation with Quadratic Temperature-Dependent Blood Perfusion under a Constant Spatial Heating on Skin Surface. J. Biomed. Sci. Eng. 2014, 7, 721–730. [Google Scholar] [CrossRef][Green Version]
- Akula, S.C.; Maniyeri, R. Numerical simulation of bioheat transfer: A comparative study on hyperbolic and parabolic heat conduction. J Braz. Soc. Mech. Sci. Eng. 2020, 42, 62. [Google Scholar] [CrossRef]
- Santos, O.; Cancino-Bernardi, J.; Pincela Lins, P.M.; Sampaio, D.; Pavan, T.; Zucolotto, V. Near-Infrared Photoactive Theragnostic Gold Nanoflowers for Photoacoustic Imaging and Hyperthermia. ACS Appl. Bio Mater. 2021, 4, 6780–6790. [Google Scholar] [CrossRef]
- Box, G.E.P.; Muller, M.E. A Note on the Generation of Random Normal Deviates. Ann. Math. Stat. 1958, 29, 610–611. [Google Scholar] [CrossRef]
- Liu, M.; Radu, D.R.; Selopal, G.S.; Bachu, S.; Lai, C.-Y. Stand-Alone CuFeSe2 (Eskebornite) Nanosheets for Photothermal Cancer Therapy. Nanomaterials 2021, 11, 2008. [Google Scholar] [CrossRef] [PubMed]
- Cruz, C.C.R.; da Silva, N.P.; Castilho, A.V.; Favre-Nicolin, V.A.; Cesar, C.L.; Orlande, H.R.B.; Dos Santos, D.S. Synthesis, Characterization and Photothermal Analysis of Nanostructured Hydrides of Pd and PdCeO2. Sci. Rep. 2020, 10, 17561. [Google Scholar] [CrossRef] [PubMed]
- Mulens-Arias, V.; Nicolás-Boluda, A.; Pinto, A.; Balfourier, A.; Carn, F.; Silva, A.K.A.; Pocard, M.; Gazeau, F. Tumor-Selective Immune-Active Mild Hyperthermia Associated with Chemotherapy in Colon Peritoneal Metastasis by Photoactivation of Fluorouracil–Gold Nanoparticle Complexes. ACS Nano 2021, 15, 3330–3348. [Google Scholar] [CrossRef] [PubMed]



| Biotissue | Thickness, mm | Absorption Coefficient μа, cm−1 | Scattering Coefficient μs, cm−1 | Scattering Anisotropy Factor g |
|---|---|---|---|---|
| nBCC | 2 | 1 | 100 | 0.8 |
| Epidermis | 0.05 | 2.5 | 240 | 0.8 |
| Dermis | 1.20 | 1.5 | 150 | 0.8 |
| Subcutaneous fat | 8.75 | 1.5 | 130 | 0.8 |
| Biotissues | SiNP Mass Concentration Cm, mg/mL | Absorption Coefficient μа, cm−1 | Scattering Coefficient μs, cm−1 | Scattering Anisotropy Factor g | Effective Attenuation Coefficient μeff, cm−1 |
|---|---|---|---|---|---|
| nBCC with wSiNPs | 1 | 3.02 | 140.74 | 0.68 | 20.87 |
| 3 | 7.06 | 222.21 | 0.58 | 46.11 | |
| 5 | 11.10 | 303.69 | 0.53 | 71.57 | |
| 7 | 15.15 | 385.13 | 0.51 | 96.26 | |
| nBCC with eSiNPs | 1 | 1.64 | 102.92 | 0.78 | 10.93 |
| 3 | 2.91 | 108.75 | 0.74 | 16.50 | |
| 5 | 4.18 | 114.58 | 0.70 | 21.99 | |
| 7 | 5.46 | 120.41 | 0.68 | 26.84 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sokolovskaya, O.I.; Sergeeva, E.A.; Golovan, L.A.; Kashkarov, P.K.; Khilov, A.V.; Kurakina, D.A.; Orlinskaya, N.Y.; Zabotnov, S.V.; Kirillin, M.Y. Numerical Simulation of Enhancement of Superficial Tumor Laser Hyperthermia with Silicon Nanoparticles. Photonics 2021, 8, 580. https://doi.org/10.3390/photonics8120580
Sokolovskaya OI, Sergeeva EA, Golovan LA, Kashkarov PK, Khilov AV, Kurakina DA, Orlinskaya NY, Zabotnov SV, Kirillin MY. Numerical Simulation of Enhancement of Superficial Tumor Laser Hyperthermia with Silicon Nanoparticles. Photonics. 2021; 8(12):580. https://doi.org/10.3390/photonics8120580
Chicago/Turabian StyleSokolovskaya, Olga I., Ekaterina A. Sergeeva, Leonid A. Golovan, Pavel K. Kashkarov, Aleksandr V. Khilov, Daria A. Kurakina, Natalia Y. Orlinskaya, Stanislav V. Zabotnov, and Mikhail Y. Kirillin. 2021. "Numerical Simulation of Enhancement of Superficial Tumor Laser Hyperthermia with Silicon Nanoparticles" Photonics 8, no. 12: 580. https://doi.org/10.3390/photonics8120580
APA StyleSokolovskaya, O. I., Sergeeva, E. A., Golovan, L. A., Kashkarov, P. K., Khilov, A. V., Kurakina, D. A., Orlinskaya, N. Y., Zabotnov, S. V., & Kirillin, M. Y. (2021). Numerical Simulation of Enhancement of Superficial Tumor Laser Hyperthermia with Silicon Nanoparticles. Photonics, 8(12), 580. https://doi.org/10.3390/photonics8120580







