On the Outage Capacity of Transdermal Optical Wireless Links with Stochastic Spatial Jitter and Skin-Induced Attenuation

Abstract

The tremendous development of both optical wireless communications (OWC) and implantable medical devices (IMDs) has recently enabled the establishment of transdermal optical wireless (TOW) links that utilize light waves to transfer information inside the living body to the outside world and conversely. Indeed, numerous emerging medical applications such as cortical recording and telemetry with cochlear implants require extremely high data rates along with low power consumption that only this new technology could accommodate. Thus, in this paper, a typical TOW link is investigated in terms of outage capacity which is a critical performance metric that has so far not been evaluated for such wireless systems in the open technical literature. More precisely, an outage capacity analysis is performed considering both skin-induced attenuation and stochastic spatial jitter, i.e., pointing error effects. Analytical expressions and results for the outage capacity are derived for a variety of skin channel conditions along with varying stochastic pointing errors which demonstrate the feasibility of this cross-field cooperation. Lastly, the corresponding simulation outcomes further validate our suggestions.

1. Introduction

TOW links are rapidly gaining popularity as a very promising alternative to conventional transdermal radiofrequency (RF) wireless links, which are commonly used todayfor varying medical applications [1,2,3]. Transdermal RF has been shown to support data rates of some Mbps up to 24 Mbps, consuming at the same time at least 30 mW [4]. However, many emerging medical applications such as recording neural signals from in-body devices and actuating these devices from out-of-body signals to guide a prosthesis, require even higher capacities to emulate similar performance to that of human organs, such as the cochlea, along with lower power consumption [5,6,7,8,9,10]. Another representative example ofthe need forhigher-speed bidirectional transdermal communication than RF is when neural signals are recorded, sampled, processed, and used to actuate artificial limbs for rehabilitation human affected by paralysis resulting from stroke, head injury, spinal cord injury, and other neurological disorders. This process can relieve pain, improve neurological disorder, or even regain functionality of damaged limbs [11]. Additionally, in comparison with the achievable data rates via transcutaneous RF, even higher-speed transdermal wireless links may be needed for such brain–machine interface applications [12,13,14,15]. In fact, 50 Mbps would be required when 100 channels are simultaneously recorded [1].

As is the case with the wider free-air optical wireless communication systems [16,17,18], according to TOW modality, the data aretransmitted via a very-high-frequency light carrier and, therefore, with an extremely high data rate which can meet the above growing capacity and energy consumption demands [19]. Nevertheless, owing to the different channel properties of human skin in comparison with atmospheric channels, the utilized wavelength window for TOW is between 600 nm and 1300 nm, while typical TOW link lengths are limited up to a few millimeters due to skin-induced attenuation and transdermal pathloss [20,21,22,23]. Another but equally significant advantage of TOW versus RF transdermal modality is that the former ensures that there is no interference with existing RF networks or electronic equipment, while the latter suffers from electromagnetic interference (EMI) and multipath fading within a highly regulated spectrum [24,25,26].

In view of the above, TOW links have attracted particular research interest in the last few years. The feasibility of establishing TOW links has been experimentally validated by different research organizations around the world [2,5,12,13,27,28,29,30,31,32,33]. In short, the feasibility of establishing modulated retroreflective and direct TOW links was demonstrated in [5,30], while, in [33], a bidirectional TOW link system for artificial hearts was employed. In [28], a 75 Mbps invitro TOW link was presented, while the potential of establishing in-vivo TOW links was verified in [29] by achieving data rates up to 100 Mbps with 2.1 mW of electrical power consumption. Moreover, the feasibility of establishing a bidirectional TOW link for brain–machine interface was validated in [12,13]. Nevertheless, what all these papers have in common is that pointing errors, which refer to unavoidable, random misalignments between transmitter and receiver terminals and may result in significant TOW performance degradation, were either neglected or considered as a deterministic effect. The concept of the stochastic nature of pointing errors was first modeled and reported in [34] and thenin [9,35]. Even more recently, the stochastic impact of pointing errors was reported in [3,10,19,24,36,37,38,39,40]. In these papers, considering the joint impact of transdermal pathloss and stochastic pointing errors, the TOW performance was evaluated in terms of outage probability, average signal-to-noise ratio (SNR), average ergodic capacity, average bit error rate (ABER), or average symbol error probability (ASEP) for various TOW system realizations.

Another critical performance metric, especially for high-speed communication systems is the outage capacity, which denotes the maximum data transmission rate of reliable communications under a specific outage probability [41,42]. However, to the best of our knowledge, although outage capacity has been extensively utilized for the wider free air communication systems [16,41,42,43,44,45], it has not yet been reported in the TOW technical literature. Motivated by the above, in the current contribution, the outage capacity for typical TOW link configurations is estimated, in the presence of skin-induced attenuation and stochastic pointing errors. Under these circumstances, analytical outage capacity expressions are derived with their corresponding results verifying the accuracy of our proposed analysis over a wide average electrical SNR range.

2. System and Channel Model

The TOW under investigation mainly consists of three fundamental parts: the out-of-body transmitter unit, the skin propagation medium which acts as the communication channel, and the in-body receiver unit. The external transmitter emits via a laser source the information-bearing light carrier which is modulated with on off keying (OOK) modulation format. After traversingthe propagation medium, the internal unit collects the light signal via an appropriate photodiode, and then the received signal is demodulated in order to provide the appropriate stimulations, after being processed by the internal digital signal processing unit and the stimulation unit. Under these circumstances, the received signal is expressed as follows [35]:

$$y=\eta hx+n,$$

(1)

where η represents the photo-current conversion ratio at the receiver side, h is the skin channel state, x stands for the modulated binary transmitted signal, and n denotes the additive noise described as a zero mean complex Gaussian process with variance σ² [36].

The channel state is expressed asfollows [10]:

$$h=h_l{h}_p,$$

(2)

where $h_l$ represents the deterministic channel coefficient owing to transdermal propagation loss, and $h_p$ is the stochastic process that describes the misalignment-induced geometric spread at the receiver aperture due to pointing errors [35].

The deterministic pathloss parameter of Equation (2) is given as follows [34]:

$$h_l^{}=\exp \left[-\frac{1}{2}\alpha \left(\lambda \right)\delta \right],$$

(3)

where $\delta$ is the dermal thickness of the skin channel which practically translates into the transdermal link length, and $\alpha \left(\lambda \right)$ is the corresponding attenuation coefficient which depends strongly on the utilized optical wavelength, $\lambda$. Indeed, within the wavelength region between 400 nm and 1800 nm, the latter coefficient is expressed asfollows [9]:

$$\alpha \left(\lambda \right)={\displaystyle \sum _{i=1}^8{a}_i}\exp \left[-{\left(\frac{\lambda -b_i}{c_i}\right)}^2\right],$$

(4)

where wavelength values are expressed in nm, and the remainingparameter values are obtained as described below [24,35].

In fact, for TOW links, we focus on their operation at wavelengths between 600 nm and 1300 nm, since, within this wavelength region, known as the medical or tissue optical window, the photon absorption that stems mainly from water content or hemoglobin and melanin can be minimized [3,23]. Optical signals with smaller or larger wavelengths are practically inappropriate for the establishment of TOW links due to this extremely strong skin-induced photon absorption.

In addition to the selected optical wavelength, the value of the skin attenuation coefficient depends heavily on the optical properties of the skin and can be obtained numerically from experimental results [20]. On the basis of these values and by employing the trust region method, the latter expression along with its parameter values described in

Table 1. The a_i, b_i, and c_i parameter values.

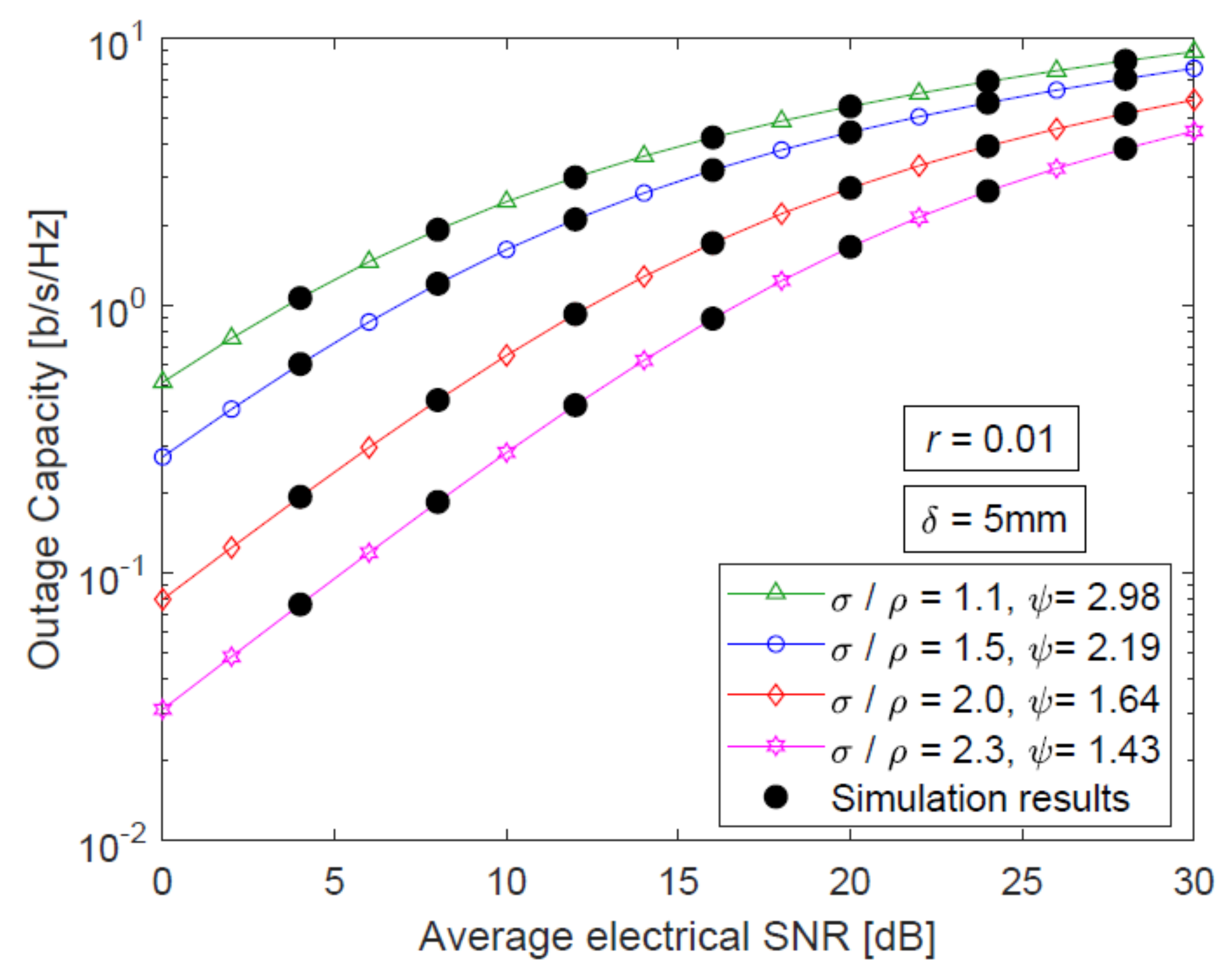
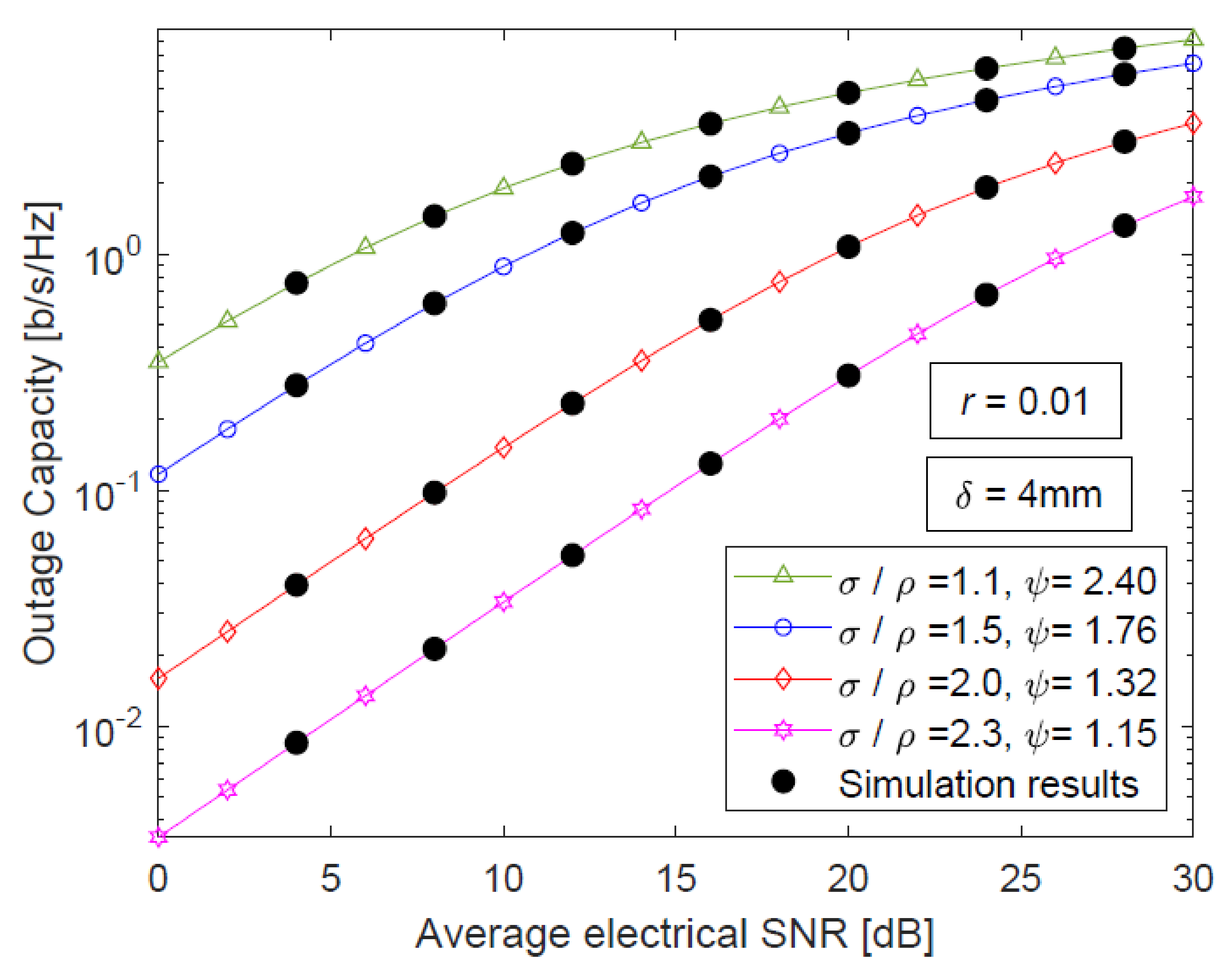
i	ai	bi	ci
1	10	0.35	0.065
2	4.5	0.42	0.25
3	13.48	−1.5	50.12
4	14.7	1442	49.35
5	7.435	1499	75.88
6	48	3322	1033
7	594.1	−183	285.9
8	11.47	−618.5	1054

can be obtained. It is notable that the accuracy of the latter expression was estimated to be higher than 99.7% by using the coefficient of determination (R-squared), which is the square of the correlation between the response values and the predicted response values and can be expressed as the ratio of the sum of squares of the regression and the total sum of squares [9].

Additionally, the random variable that describes stochastic pointing errors obtains the following probability density function (PDF) [16,39,43]:

$$f_{h_p}\left(h_p\right)={\psi }^2{A}_0^{-{\psi }^2}{h}_p^{{\psi }^2-1}, 0\le h_p\le A_0,$$

(5)

where $\psi =w_{eq}/2\sigma$ is proportional to the amount of pointing error strength with $w_{eq}$ denoting the equivalent beam radius in the detector aperture and $\sigma$ representing the spatial jitter, i.e., the pointing error displacement. Specifically, larger $\psi$ parameter values imply stronger pointing errors and, thus, more severe misalignment-induced fading. Indeed, increased $\psi$ values practically refer to smaller spatial jitter values, which means that the beam footprint overlaps the detector aperture to a greater extent, thus describing smaller amounts of pointing mismatch. Consequently, when $\psi \to \infty$ or practically when $\psi$ obtains very large values, pointing errors can be considered as a negligible effect. Furthermore $w_{eq}={\left[\frac{\sqrt{\pi }\mathrm{erf}\left(v\right)w_{\delta }^2}{2vexp(-v^2)}\right]}^{1/2}$, where erf(.) stands for the error function, (Equation (8.250.1) in [46]), and $v=\frac{\sqrt{\pi }\rho }{\sqrt{2}{w}_{\delta }}$, where $\rho$ represents the radius of the circular receiver aperture and $A_0={\mathrm{erf}}^2\left(v\right)$ isthe fraction of the collected power at $\rho$ = 0 [16,24]. Additionally, $w_{\delta }$, which represents the corresponding beam waist on the receiver plane at a propagating transdermal distance $\delta$ along with a transmitter divergence angle $\theta$, is obtained as follows [10,38]:

$$w_{\delta }=\delta \tan \left(\theta /2\right).$$

(6)

By using Equations (2) and (5), the PDF of the random variable hcan be obtained asfollows [16,19]:

$$f_h\left(h\right)={\psi }^2{A}_0^{-{\psi }^2}{h}_l^{-{\psi }^2}{h}^{{\psi }^2-1}, 0\le h\le A_0{h}_l.$$

(7)

Moreover, by using Equations (2) and (3), the instantaneous electrical SNR, $\gamma ={\eta }^2{h}^2{P}_s{N}_0^{-1}$, is expressed asfollows:

$$\gamma ={\eta }^2{h}_l^2{h}_p^2{P}_s{N}_0^{-1}={\eta }^2{h}_p^2\exp \left[-\alpha \left(\lambda \right)\delta \right]P_s{N}_0^{-1},$$

(8)

where $P_s$ and $N_0$ represent the signal and noise optical power spectral density (PSD), respectively [34].

Consequently, the average electrical SNR, $\mu$, is obtained asfollows [35]:

$$\mu =\frac{{\left(\eta {\rm E}\left[h\right]\right)}^2{P}_s}{N_0}=\frac{{\eta }^2{\psi }^2{A}_0^2{P}_s\exp \left[-\alpha \left(\lambda \right)\delta \right]}{\left({\psi }^2+2\right)N_0},$$

(9)

where E[.] denotes expectation.

Next, by using Equations (7) and (8), along with applying the standard technique of transforming random variables $f_{\gamma }\left(\gamma \right)=\frac{f_h\left(h\right)}{\left|\partial \gamma / \partial h\right|}\left|\begin{array}{c}\\ h=\sqrt{\frac{\gamma }{{\eta }^2{P}_s{N}_0^{-1}}}\end{array}\right.$ [47], the PDF of random variable $\gamma$ is obtained asfollows:

$$f_{\gamma }\left(\gamma \right)=\frac{{\psi }^2}{2}{A}_0^{-{\psi }^2}{h}_l^{-{\psi }^2}{\left({\eta }^2{N}_0^{-1}{P}_S\right)}^{\frac{-{\psi }^2}{2}}{\gamma }^{\frac{{\psi }^2-2}{2}}, 0\le \gamma \le A_0^2{h}_l^2{\eta }^2{N}_0^{-1}{P}_S.$$

(10)

By substituting Equation (9) into Equation (10), we get

$$f_{\gamma }\left(\gamma \right)=\frac{{\psi }^2}{2}{\left(\frac{{\psi }^2}{{\psi }^2+2}\right)}^{\frac{{\psi }^2}{2}}{\mu }^{\frac{-{\psi }^2}{2}}{\gamma }^{\frac{{\psi }^2-2}{2}}, 0\le \gamma \le A_0^2{h}_l^2{\eta }^2{N}_0^{-1}{P}_S.$$

(11)

3. Outage Capacity

A very critical performance metric for each OWC system is its outage capacity, $C_{out}$, which stands for the capacity guaranteed for a percentage rate of $\left(1-r\right)$ of the channel realizations [44,45].

$$\mathrm{Pr}\left[C<C_{out}\right]=r,$$

(12)

where Pr[.] denotes probability, and $C$ represents the instantaneous channel capacity which is obtained as follows [43]:

$$C=B{\log }_2\left(1+\gamma \right)=\frac{1}{\ln 2}B\ln \left(1+\gamma \right),$$

(13)

where $B$ is the channel’s bandwidth.

It is appropriate to clarify here the differences between outage probability, outage capacity, and capacity metrics. Capacity denotes the achievable maximum data rate just only for a specific moment, while the outage capacity represents the capacity guaranteed for a specific probability of the channel realizations. Additionally, the outage probability represents the probability that the instantaneous SNR at the receiver side falls below a specific SNR threshold that corresponds to the receiver’s sensitivity, while the outage capacity could represent the capacity guaranteed for a specific outage probability. Moreover, higher outage probability values correspond to a degraded outage performance for the system, while higher outage capacity values correspond to an upgraded outage performance for the system [36,44]. Indeed, considering Equation (12), the outage capacity refers to the minimum capacity which is required for a specificpercentage rate of channel realizations, while outage probability refers to the required instantaneous SNR threshold.

Since, in a real wireless channel, the instantaneous electrical SNR, $\gamma$, is a random variable, it becomes evident from Equation (13) that $C$ is also a random variable. Therefore, the probability of Equation (12) is evaluated asfollows [42,45]:

$$r={\displaystyle \underset{0}{\overset{C_{out}}{\int }}{f}_C\left(C\right)} dC,$$

(14)

where $f_C\left(C\right)$ is the PDF of the random variable $C$.

Using Equations (11) and (13), along with standard technique of transforming random variables, $f_C\left(C\right)=\frac{f_{\gamma }\left(\gamma \right)}{\left|\partial C/\partial \gamma \right|}\left|\begin{array}{c}\\ \gamma =2^{\frac{C}{B}}-1\end{array}\right.$ [47], the latter PDF is obtained asfollows:

$$f_C\left(C\right)=\frac{{\psi }^2\ln 2}{2B}{\left(\frac{{\psi }^2}{{\psi }^2+2}\right)}^{\frac{{\psi }^2}{2}}{\mu }^{\frac{-{\psi }^2}{2}}{2}^{C/B}{\left(2^{C/B}-1\right)}^{\frac{{\psi }^2-2}{2}}.$$

(15)

Thus, by substituting Equation (15) into Equation (14), we get

$$r=\frac{{\psi }^2\ln 2}{2B}{\left(\frac{{\psi }^2}{{\psi }^2+2}\right)}^{\frac{{\psi }^2}{2}}{\mu }^{\frac{-{\psi }^2}{2}}{\displaystyle \underset{0}{\overset{C_{out}}{\int }}{2}^{C/B}{\left(2^{C/B}-1\right)}^{\frac{{\psi }^2-2}{2}}} dC.$$

(16)

In order to calculate the integral of Equation (16), we set $u=\sqrt{2^{C/B}-1}$, $du=\frac{2^{C/B}\ln 2}{2B\sqrt{2^{C/B}-1}}dC$. Thus, the above integral is calculated as

$${\displaystyle \underset{0}{\overset{\sqrt{2^{C_{out}/B}-1}}{\int }}{u}^{{\psi }^2-2}u\left(2B/\ln 2\right)} du=\frac{2B}{{\psi }^2\ln 2}{\left(\sqrt{2^{C_{out}/B}-1}\right)}^{{\psi }^2}.$$

(17)

Therefore, by substituting Equation (17) into Equation (16), we obtain

$$r={\left(\frac{{\psi }^2}{{\psi }^2+2}\right)}^{\frac{{\psi }^2}{2}}{\mu }^{\frac{-{\psi }^2}{2}}{\left(\sqrt{2^{C_{out}/B}-1}\right)}^{{\psi }^2},$$

(18)

which can also be expressed by substituting Equation (9) into Equation (18) asfollows:

$$C_{out}/B={\log }_2\left\{{10}^{\frac{2}{{\psi }^2}\log \left[r{\left(\frac{{\psi }^2}{{\psi }^2+2}\right)}^{-\frac{{\psi }^2}{2}}{\left(\frac{{\eta }^2{\psi }^2{A}_0^2{P}_s\exp \left[-\alpha \left(\lambda \right)\delta \right]}{\left({\psi }^2+2\right)N_0}\right)}^{\frac{{\psi }^2}{2}}\right]}+1\right\}.$$

(19)

The derived analytical expressions in Equations (18) and (19) reveal that, in addition to the available bandwidth, the outage capacity for TOW links depends strongly on the pointing error’s strength, average electrical SNR at the receiver’s side, skin-induced attenuation, and specific link characteristics such as skin channel thickness, power and noise spectral densities, and operational wavelength.

4. Analytical Results

In this section, analytical results are graphically depicted that arise from the derived analytical expressions in Equations (18) and (19). Their validity is further verified by appropriate simulations. The diameter of the receiver’s aperture, $\rho$, wasset to 0.5 mm, while the operational wavelength, $\lambda$, wasfixed at 1100 nm, which can drastically address skin-induced attenuation due to photon absorption, as reported in [9]. Moreover, although the probability $r$ could take numerous values, the values 0.01 and 0.1 werehere utilized, which are very common for any OWC link [42]. Furthermore, $\eta =$ 0.8, $\theta =$ 20°, $P_s=$ 1 μW/MHz, and $N_0$ = ${\left(1.3 pA/\sqrt{Hz}\right)}^2$ [48]. Regarding pointing mismatch, varying weak to strong pointing error effects wereevaluated for two different typical transdermal link lengths, i.e., $\left(\delta , \sigma / \rho , \psi \right)=\left(4 \mathrm{mm}, 1.1, 2.4\right)$, $\left(\delta , \sigma / \rho , \psi \right)=\left(4 \mathrm{mm}, 1.5, 1.76\right)$, $\left(\delta , \sigma / \rho , \psi \right)=\left(4 \mathrm{mm}, 2.0, 1.32\right)$, $\left(\delta , \sigma / \rho , \psi \right)=\left(4 \mathrm{mm}, 2.3, 1.15\right)$, $\left(\delta , \sigma / \rho , \psi \right)=\left(5 \mathrm{mm}, 1.1, 2.98\right)$, $\left(\delta , \sigma / \rho , \psi \right)=\left(5 \mathrm{mm}, 1.5, 2.19\right)$, $\left(\delta , \sigma / \rho , \psi \right)=\left(5 \mathrm{mm}, 2.0, 1.64\right)$, and $\left(\delta , \sigma / \rho , \psi \right)=\left(5 \mathrm{mm}, 2.3, 1.43\right)$. Note that $\psi$ values are getting smaller as $\sigma /\rho$ values are getting larger, especially for smaller $\delta$ values, i.e., pointing errors are getting stronger as spatial jitter increases, as expected, especially for shorter transdermal link lengths, according to Equation (6). Indeed, the latter is valid assuming fixed $\theta$ and $\rho$ parameter values as mentioned above. Under these conditions, different typical TOW link configurations wereinvestigated by means of outage capacity over a wide average SNR range, from 0 dB to 30 dB.

Figure 1 illustrates the outage capacity dependence on the average electrical SNR evolution for a typical TOW link length of 5 mm with different values of normalized jitter standard deviation, provided that $r=$ 0.1. We observe that higher jitter values lead to lower $\psi$ parameter values and, thus, to stronger pointing error effects. In fact, as pointing errors effects are getting stronger, significantly lower outage capacity values are depicted, especially for lower average electrical SNR values.

Figure 2 depicts the corresponding analytical results tothose of Figure 1 but with $r=$ 0.01, i.e., with a smaller value of the probability $r$. Consequently, although similar qualitative results wereobtained for the outage capacity in both cases, the decrease inthe probability value mentioned above broughtabout smaller corresponding outage capacity values. Therefore, the performance comparison between these two figures highlights the outage capacity degradation due to lower $r$ values. This behavior is in good agreement with what happens in the wider OWC field, much less for the same probability values [42]. Additionally, the impact of pointing errors is depicted to become even more severe by decreasing this probability value, especially for lower average electrical SNR values.

Figure 3 presents the corresponding analytical results tothose of Figure 2 but through a shorter transdermal link, i.e., a smaller value of parameter $\delta$. As expected from Equation (6), shorter transdermal link distances led to larger beam waist values for a specific initial divergence angle, which resulted, in turn, in a larger amount of pointing mismatch. Indeed, for the same normalized jitter standard deviation values, we now obtained smaller corresponding $\psi$ parameter values due to the shorter transdermal propagation distance. This translated into more important outage capacity performance degradations due to the presence of stronger pointing errors, especially for lower average electrical SNR values. In this context, Figure 3 highlights the dependence of stochastic pointing error effects on transdermal link length, in terms of TOW outage capacity. Note that this behavior is consistent with what happens with other critical TOW outage performance metrics, such as the outage probability and the average bit error rate [35,37]. It should be noted, however, that, for longer TOW distances, i.e., above some additional millimeters, the impact of skin-induced attenuation dominates the impact of pointing errors. Thus, after some transdermal propagation distance, the corresponding outage performance will be degraded due to the total transdermal pathloss, despite the increase inbeam waist.

5. Discussion and Conclusions

In this work, we first investigated the outage capacity for the performance of TOW links with slow-fading skinchannels along with the presence of varying stochastic spatial jitter. In this respect, an outage capacity analysis was performed, while novel analytical expressions were derived for this crucial performance metric which incorporate the most significant parameters and effects that play a key role in TOW performance and availability. Their analytical results which were validated by proper simulations reveal that the achievable TOW outage capacity largely depends on the average electrical SNR at the receiver’s side, as well as on stochastic pointing errors’ strength, along with transdermal propagating distance for the information-bearing light which penetrates into the skin channel. In this context, the feasibility of establishing high-speed TOW links was demonstrated for typical transdermal link lengths, even in very harsh pointing mismatch conditions. Toward the development of future higher-speed TOW links of enhanced robustness, the analysis proposed can be added to the engineer’s quiver as a useful tool for the design of such effective transdermal wireless systems.

In view of the above, the key contribution of this work was to develop a theoretical framework for the outage capacity estimation of typical TOW links quantifying the impact for weak to strong stochastic pointing errors.It should be noted, however, that, for a real human skin channel, human body temperature, in addition to other specific skin and tissue particularities, should be taken into consideration. Nevertheless, the performance comparison ofour findings in TOW area withcorresponding outage capacity results in traditional free-air communication systems evaluated in [42,43] reveal the feasibility of our findings and suggestions.