LEO Satellites Constellation-to-Ground QKD Links: Greek Quantum Communication Infrastructure Paradigm
Abstract
:1. Introduction
2. QKD Protocol and Architecture Assumptions in the LEO
2.1. System Architecture, Satellite, and Ground Stations
2.2. Weak + Vacuum Decoy-State BB84 Protocol
3. FSO Channel Modeling
3.1. Received Power
3.2. Free-Space Loss
3.3. Transmitter and Receiver Gains
3.4. Atmospheric Attenuation
3.5. Pointing Error Loss
3.6. Scintillation Loss
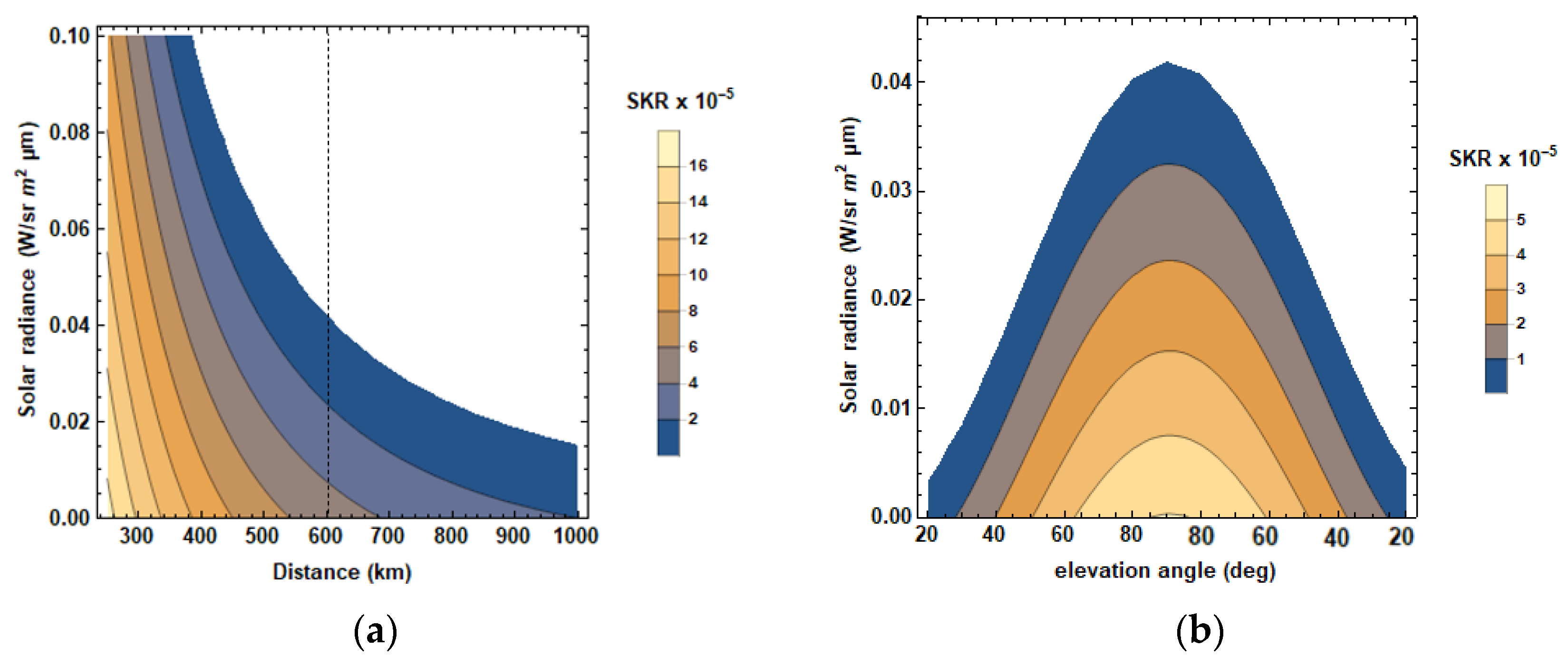
3.7. Background Solar Radiance
4. Simulation Results
4.1. System Parameters
4.2. Feasibility of LEO Satellite-to-Ground QKD in Daytime and Nighttime
4.2.1. Distance Reach
4.2.2. QKD under Different Background Noise Levels
4.3. QKD Link Performance of an LEO Satellite Constellation over Greek QCI
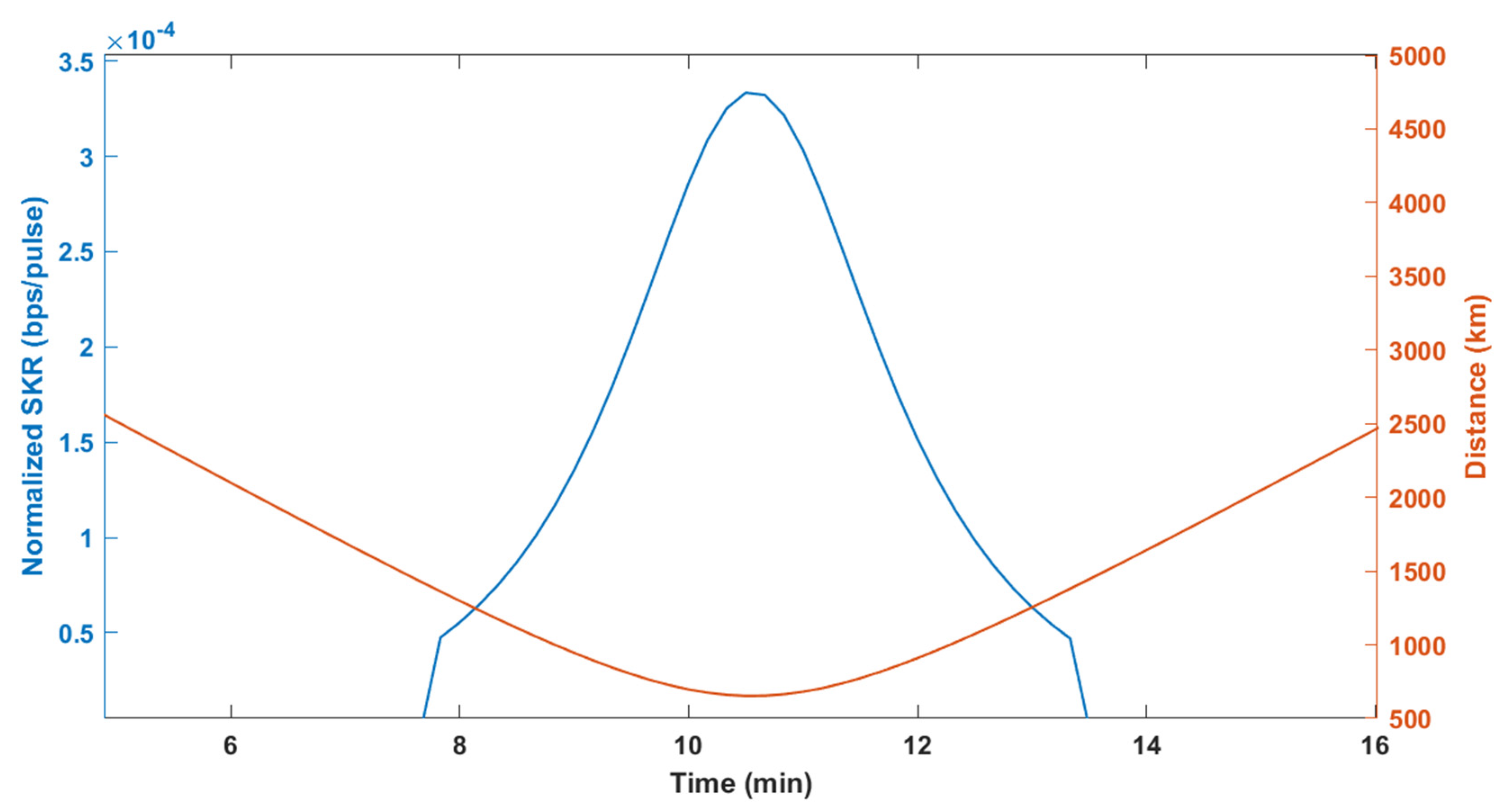
4.3.1. Single Satellite Pass over a Single OGS
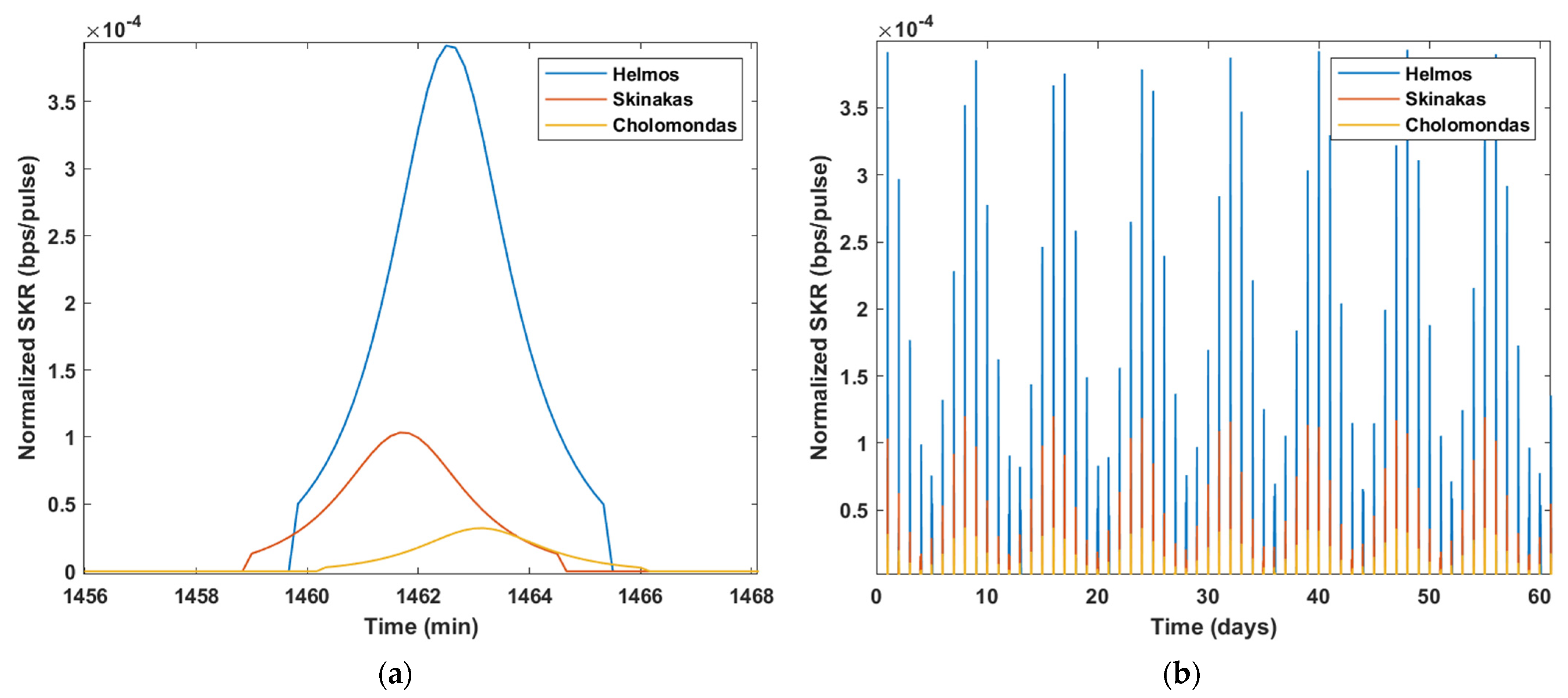
4.3.2. Satellite Full Constellation Pass over a Single OGS
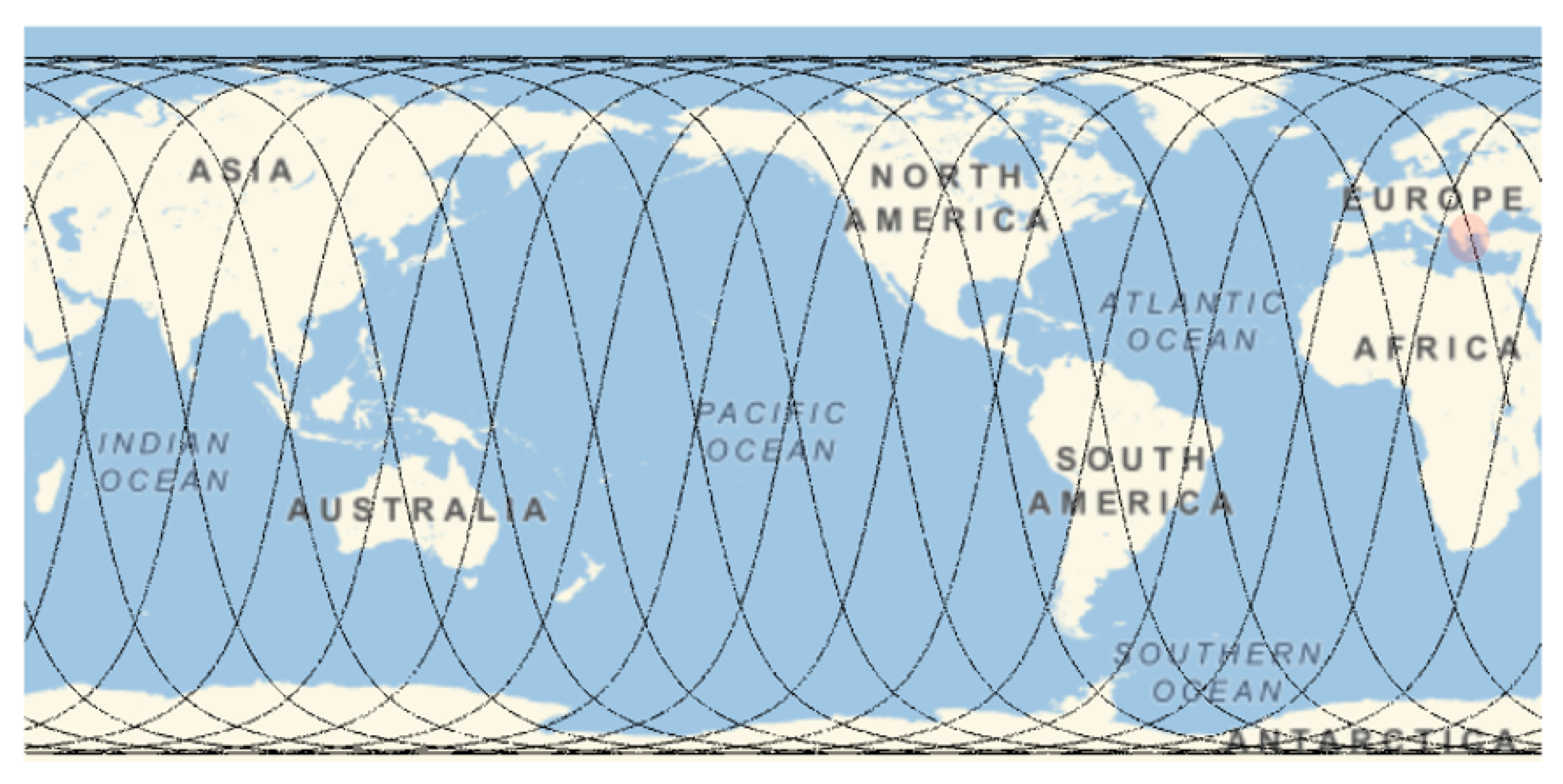
4.3.3. Satellite Full Constellation Pass over Greek QCI
4.4. Practical Exploitation of Distilled Key Bits
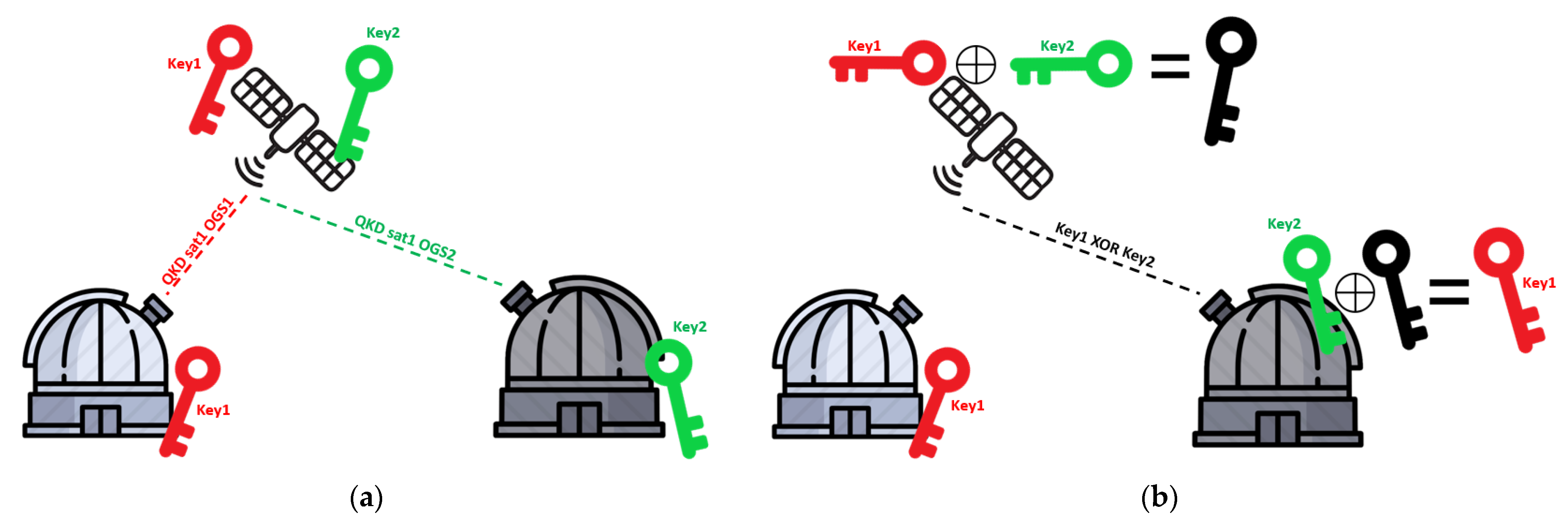
4.4.1. Satellite as a Trusted Node
4.4.2. AES-256 Key Refresh Time
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Appendix A. Decoy-State BB84 Protocol Equation
Appendix B. Maximum Refresh Time Calculation
Appendix C. Minimum Refresh Time Calculation
References
- Wu, Y.; Bao, W.-S.; Cao, S.; Chen, F.; Chen, M.-C.; Chen, X.; Chung, T.-H.; Deng, H.; Du, Y.; Fan, D.; et al. Strong Quantum Computational Advantage Using a Superconducting Quantum Processor. Phys. Rev. Lett. 2021, 127, 180501. [Google Scholar] [CrossRef]
- Shor, P.W. Algorithms for quantum computation: Discrete logarithms and factoring. In Proceedings of the 35th Annual Symposium on Foundations of Computer Science, Santa Fe, NM, USA, 20–22 November 1994; pp. 124–134. [Google Scholar]
- Pirandola, S.; Andersen, U.L.; Banchi, L.; Berta, M.; Bunandar, D.; Colbeck, R.; Englund, D.; Gehring, T.; Lupo, C.; Ottaviani, C.; et al. Advances in quantum cryptography. Adv. Opt. Photonics 2020, 12, 1012. [Google Scholar] [CrossRef] [Green Version]
- Lo, H.-K.; Chau, H.F. Unconditional Security of Quantum Key Distribution over Arbitrarily Long Distances. Science 1999, 283, 2050–2056. [Google Scholar] [CrossRef] [Green Version]
- Shor, P.W.; Preskill, J. Simple Proof of Security of the BB84 Quantum Key Distribution Protocol. Phys. Rev. Lett. 2000, 85, 441–444. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bennett, C.H.; Gilles, B. Proceedings of the IEEE International Conference on Computers, Systems and Signal Processing, Bangalore, India, 9–12 December 1984; IEEE: Piscataway, NJ, USA, 1984. [Google Scholar]
- Peev, M.; Pacher, C.; Alléaume, R.; Barreiro, C.; Bouda, J.; Boxleitner, W.; Debuisschert, T.; Diamanti, E.; Dianati, M.; Dynes, J.F.; et al. The SECOQC quantum key distribution network in Vienna. New J. Phys. 2009, 11, 075001. [Google Scholar] [CrossRef] [Green Version]
- Diamanti, E.; Lo, H.-K.; Qi, B.; Yuan, Z. Practical challenges in quantum key distribution. NPJ Quantum Inf. 2016, 2, 16025. [Google Scholar] [CrossRef] [Green Version]
- Chen, J.-P.; Zhang, C.; Liu, Y.; Jiang, C.; Zhang, W.; Hu, X.-L.; Guan, J.-Y.; Yu, Z.-W.; Xu, H.; Lin, J.; et al. Sending-or-Not-Sending with Independent Lasers: Secure Twin-Field Quantum Key Distribution over 509 km. Phys. Rev. Lett. 2020, 124, 070501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ntanos, A.; Zavitsanos, D.; Lyras, N.K.; Giannoulis, G.; Avramopoulos, H. On the Availability of the Decoy State BB84 QKD over a Terrestrial FSO Link. In Proceedings of the 2021 International Conference on Optical Network Design and Modeling (ONDM), Gothenburg, Sweden, 28 June–1 July 2021. [Google Scholar]
- Ursin, R.; Tiefenbacher, F.; Schmitt-Manderbach, T.; Weier, H.; Scheidl, T.; Lindenthal, M.; Blauensteiner, B.; Jennewein, T.; Perdigues, J.M.; Trojek, P.; et al. Entanglement-based quantum communication over 144 km. Nat. Phys. 2007, 3, 481–486. [Google Scholar] [CrossRef] [Green Version]
- Liao, S.-K.; Cai, W.-Q.; Handsteiner, J.; Liu, B.; Yin, J.; Zhang, L.; Rauch, D.; Fink, M.; Ren, J.-G.; Liu, W.-Y.; et al. Satellite-Relayed Intercontinental Quantum Network. Phys. Rev. Lett. 2018, 120, 030501. [Google Scholar] [CrossRef] [Green Version]
- Polnik, M.; Mazzarella, L.; Di Carlo, M.; Oi, D.; Riccardi, A.; Arulselvan, A. Scheduling of space to ground quantum key distribution. EPJ Quantum Technol. 2020, 7, 3. [Google Scholar] [CrossRef]
- Khan, I.; Heim, B.; Neuzner, A.; Marquardt, C. Satellite-Based QKD. Opt. Photonics News 2018, 29, 26–33. [Google Scholar] [CrossRef]
- Yin, J.; Cao, Y.; Li, Y.-H.; Liao, S.-K.; Zhang, L.; Ren, J.-G.; Cai, W.-Q.; Liu, W.-Y.; Li, B.; Dai, H.; et al. Satellite-based entanglement distribution over 1200 kilometers. Science 2017, 356, 1140–1144. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kaushal, H.; Kaddoum, G. Optical Communication in Space: Challenges and Mitigation Techniques. IEEE Commun. Surv. Tutor. 2016, 19, 57–96. [Google Scholar] [CrossRef] [Green Version]
- Lyras, N.K.; Kourogiorgas, C.I.; Panagopoulos, A.D. Cloud Attenuation Statistics Prediction from Ka-Band to Optical Frequencies: Integrated Liquid Water Content Field Synthesizer. IEEE Trans. Antennas Propag. 2016, 65, 319–328. [Google Scholar] [CrossRef]
- Lyras, N.K.; Efrem, C.N.; Kourogiorgas, C.I.; Panagopoulos, A.D. Optimum Monthly Based Selection of Ground Stations for Optical Satellite Networks. IEEE Commun. Lett. 2018, 22, 1192–1195. [Google Scholar] [CrossRef]
- Liu, J.; Xiao, Y.; Gao, J. Achieving Accountability in Smart Grid. IEEE Syst. J. 2014, 8, 493–508. [Google Scholar] [CrossRef] [Green Version]
- Lyras, N.K.; Kourogiorgas, C.I.; Panagopoulos, A.D. Cloud free line of sight prediction modeling for Low Earth Orbit optical satellite networks. In International Conference on Space Optics—ICSO 2018; SPIE: Bellingham, WA, USA, 2019; Volume 11180, p. 111801G. [Google Scholar]
- Lyras, N.K.; Kourogiorgas, C.I.; Panagopoulos, A.D.; Liolis, K.P.; Sodnik, Z. Long Term Irradiance Statistics for Optical GEO Satellite Feeder Links: Validation Against Experimental Data. Wirel. Pers. Commun. 2020, 114, 749–764. [Google Scholar] [CrossRef]
- Kapsis, T.T.; Lyras, N.K.; Kourogiorgas, C.I.; Panagopoulos, A.D. Time Series Irradiance Synthesizer for Optical GEO Satellite Downlinks in 5G Networks. Futur. Internet 2019, 11, 131. [Google Scholar] [CrossRef] [Green Version]
- Hemmati, H. Near-Earth Laser Communications, 71–73; CRC Press: Boca Raton, FL, USA; New York, NY, USA; London, UK, 2009. [Google Scholar]
- Bonato, C.; Tomaello, A.; Da Deppo, V.; Naletto, G.; Villoresi, P. Feasibility of satellite quantum key distribution. New J. Phys. 2009, 11, 045017. [Google Scholar] [CrossRef] [Green Version]
- Liao, S.-K.; Cai, W.-Q.; Liu, W.-Y.; Zhang, L.; Li, Y.; Ren, J.-G.; Yin, J.; Shen, Q.; Cao, Y.; Li, Z.-P.; et al. Satellite-to-ground quantum key distribution. Nat. Cell Biol. 2017, 549, 43–47. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.-A.; Zhang, Q.; Chen, T.-Y.; Cai, W.-Q.; Liao, S.-K.; Zhang, J.; Chen, K.; Yin, J.; Ren, J.-G.; Chen, Z.; et al. An integrated space-to-ground quantum communication network over 4600 kilometres. Nat. Cell Biol. 2021, 589, 214–219. [Google Scholar]
- Tomaello, A.; Bonato, C.; Da Deppo, V.; Naletto, G.; Villoresi, P. Link budget and background noise for satellite quantum key distribution. Adv. Space Res. 2011, 47, 802–810. [Google Scholar] [CrossRef]
- Liao, S.-K.; Yong, H.-L.; Liu, C.; Shentu, G.-L.; Li, D.-D.; Lin, J.; Dai, H.; Zhao, S.-Q.; Li, B.; Guan, J.-Y.; et al. Long-distance free-space quantum key distribution in daylight towards inter-satellite communication. Nat. Photonics 2017, 11, 509–513. [Google Scholar] [CrossRef]
- Bedington, R.; Arrazola, J.M.; Ling, A. Progress in satellite quantum key distribution. npj Quantum Inf. 2017, 3, 30. [Google Scholar] [CrossRef]
- All Member States Now Committed to Building An EU Quantum Communication Infrastructure. Available online: https://digital-strategy.ec.europa.eu/en/news/all-member-states-now-committed-building-eu-quantum-communication-infrastructure (accessed on 3 November 2021).
- Giannopappa, C. 5G, Fibre in the Sky and beyond. In Proceedings of the ScyLight Workshop on Optical and Quantum Communication, Online Event, 8–9 June 2021. [Google Scholar]
- Andrews, L.C.; Phillips, R.L. Laser Beam Propagation through Random Media; SPIE Optical Engineering Press: Bellingham, WA, USA, 1998; pp. 47–50. [Google Scholar]
- Auer, M.; Freiwang, P.; Baliuka, A.; Schattauer, M.; Knips, L.; Weinfurter, H. A portable and compact decoy-state QKD sender. In Proceedings of the 2021 Conference on Lasers and Electro-Optics Europe & European Quantum Electronics Conference (CLEO/Europe-EQEC), Munich, Germany, 21–25 June 2021; p. 1. [Google Scholar]
- Mazzarella, L.; Lowe, C.; Lowndes, D.; Joshi, S.K.; Greenland, S.; McNeil, D.; Mercury, C.; Macdonald, M.; Rarity, J.; Oi, D.K.L. QUARC: Quantum Research Cubesat—A Constellation for Quantum Communication. Cryptography 2020, 4, 7. [Google Scholar] [CrossRef] [Green Version]
- Lo, H.K.; Ma, X.; Chen, K. Decoy state quantum key distribution. Phys. Rev. Lett. 2005, 94, 230504. [Google Scholar] [CrossRef] [Green Version]
- Mayers, D. Unconditional security in quantum cryptography. JACM 2001, 48, 351–406. [Google Scholar] [CrossRef]
- Huttner, B.; Imoto, N.; Gisin, N.; Mor, T. Quantum cryptography with coherent states. Phys. Rev. A 1995, 51, 1863–1869. [Google Scholar] [CrossRef] [Green Version]
- Hwang, W.-Y. Quantum Key Distribution with High Loss: Toward Global Secure Communication. Phys. Rev. Lett. 2003, 91, 057901. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.-B. Beating the Photon-Number-Splitting Attack in Practical Quantum Cryptography. Phys. Rev. Lett. 2005, 94, 230503. [Google Scholar] [CrossRef] [Green Version]
- Wang, S.; Chen, W.; Yin, Z.-Q.; Li, H.-W.; He, D.-Y.; Li, Y.-H.; Zhou, Z.; Song, X.-T.; Li, F.-Y.; Wang, D.; et al. Field and long-term demonstration of a wide area quantum key distribution network. Opt. Express 2014, 22, 21739–21756. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.; Qi, B.; Ma, X.; Lo, H.-K.; Qian, L. Simulation and Implementation of Decoy State Quantum Key Distribution over 60km Telecom Fiber. In Proceedings of the 2006 IEEE International Symposium on Information Theory, Seattle, WA, USA, 9–14 July 2006; pp. 2094–2098. [Google Scholar]
- Carrasco-Casado, A.; Kunimori, H.; Takenaka, H.; Kubo-Oka, T.; Akioka, M.; Fuse, T.; Koyama, Y.; Kolev, D.; Munemasa, Y.; Toyoshima, M. LEO-to-ground polarization measurements aiming for space QKD using Small Optical TrAnsponder (SOTA). Opt. Express 2016, 24, 12254–12266. [Google Scholar] [CrossRef]
- Takenaka, H.; Carrasco-Casado, A.; Fujiwara, M.; Kitamura, M.; Sasaki, M.; Toyoshima, M. Satellite-to-ground quantum-limited communication using a 50-kg-class microsatellite. Nat. Photon. 2017, 11, 502–508. [Google Scholar] [CrossRef] [Green Version]
- Kolev, D.R.; Toyoshima, M. Satellite-to-ground optical communications using small optical transponder (SOTA)—received-power fluctuations. Opt. Express 2017, 25, 28319–28329. [Google Scholar] [CrossRef]
- Cakaj, S.; Kamo, B.; Lala, A.; Rakipi, A. The Coverage Analysis for Low Earth Orbiting Satellites at Low Elevation. Int. J. Adv. Comput. Sci. Appl. 2014, 5, 8. [Google Scholar] [CrossRef] [Green Version]
- Series, P. Propagation Data and Prediction Methods Required for the Design of Earth-Space Telecommunication Systems. Recommendation ITU-R, 618–12; ITU: Geneva, Switzerland, 2015. [Google Scholar]
- Kiasaleh, K. On the probability density function of signal intensity in free-space optical communications systems im-paired by pointing jitter and turbulence. Opt. Eng. 1994, 33, 3748–3757. [Google Scholar] [CrossRef]
- Yura, H.T.; McKinley, W.G. Aperture averaging of scintillation for space-to-ground optical communication applications. Appl. Opt. 1983, 22, 1608–1609. [Google Scholar] [CrossRef]
- Giggenbach, D.; Moll, F. Scintillation loss in optical Low Earth Orbit data downlinks with avalanche photodiode receivers. In Proceedings of the 2017 IEEE International Conference on Space Optical Systems and Applications (ICSOS), Naha, Japan, 14–16 November 2017; pp. 115–122. [Google Scholar]
- Rollins, D.; Baars, J.; Bajorins, D.P.; Cornish, C.S.; Fischer, K.W.; Wiltsey, T. Background light environment for free-space optical terrestrial communication links. In Optical Wireless Communications; SPIE: Bellingham, WA, USA, 2002; Volume 4873, pp. 99–111. [Google Scholar]
- Carrasco-Casado, A.; Sanchez-Pena, J.M.; Vergaz, R. CTA Telescopes as Deep-Space Lasercom Ground Receivers. IEEE Photonics J. 2015, 7, 1–14. [Google Scholar] [CrossRef]
- Recommendation ITU-R P. 1621, Propagation Data Required for the Design of Earth-Space Systems Operating between 20 THz and 375 THz; ITU: Geneva, Switzerland, 2003.
- Single Quantum Eos. Available online: https://singlequantum.com/products/single-quantum-eos/ (accessed on 3 November 2021).
- Mlejnek, M.; Kaliteevskiy, N.A.; Nolan, D.A. Reducing spontaneous Raman scattering noise in high quantum bit rate QKD systems over optical fiber. arXiv 2017, arXiv:1712.05891. [Google Scholar]
- Eraerds, P.; Walenta, N.; Legré, M.; Gisin, N.; Zbinden, H. Quantum key distribution and 1 Gbps data encryption over a single fibre. New J. Phys. 2010, 12, 063027. [Google Scholar] [CrossRef] [Green Version]
- Dirks, B.P.; Ferrario, I.; Le Pera, A.; Finocchiaro, D.V.; Desmons, M.; de Lange, D.; de Man, H.; Meskers, A.J.H.; Morits, J.; Neumann, N.M.M.; et al. GEOQKD: Quantum key distribution from a geostationary satellite. In Proceedings of the International Conference on Space Optics—ICSO 2020; SPIE: Bellingham, WA, USA, 2021; Volume 11852, p. 118520J. [Google Scholar]
- Er-Long, M.; Zheng-Fu, H.; Shun-Sheng, G.; Tao, Z.; Da-Sheng, D.; Guang-Can, G. Background noise of satellite-to-ground quantum key distribution. New J. Phys. 2005, 7, 215. [Google Scholar] [CrossRef]
- Available online: https://www.agi.com/products/stk (accessed on 3 November 2021).
- Migdall, A.; Polyakov, S.V.; Fan, J.; Bienfang, J.C. Single-Photon Generation and Detection: Physics and Applications; Academic Press: Cambridge, MA, USA, 2013. [Google Scholar]
- Barker, E. Recommendation for Key Management Part 1: General; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2016.
- Tajima, A.; Kondoh, T.; Ochi, T.; Fujiwara, M.; Yoshino, K.; Iizuka, H.; Sakamoto, T.; Tomita, A.; Shimamura, E.; Asami, S.; et al. Quantum key distribution network for multiple applications. Quantum Sci. Technol. 2017, 2, 034003. [Google Scholar] [CrossRef]
- Bonnetain, X.; Naya-Plasencia, M.; Schrottenloher, A. Quantum Security Analysis of AES. IACR Trans. Symmetric Cryptol. 2019, 2, 55–93. [Google Scholar] [CrossRef]
- Luykx, A.; Paterson, K.G. Limits on Authenticated Encryption Use in TLS. 2015. Available online: http://www.isg.rhul.ac.uk/~kp/TLS-AEbounds.pdf (accessed on 2 November 2021).
- Zavitsanos, D.; Ntanos, A.; Giannoulis, G.; Avramopoulos, H. On the QKD Integration in Converged Fiber/Wireless Topologies for Secured, Low-Latency 5G/B5G Fronthaul. Appl. Sci. 2020, 10, 5193. [Google Scholar] [CrossRef]
- Sun, X.; Djordjevic, I.B.; Neifeld, M.A. Secret Key Rates and Optimization of BB84 and Decoy State Protocols Over Time-Varying Free-Space Optical Channels. IEEE Photonics J. 2016, 8, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Sidhu, J.S.; Brougham, T.; McArthur, D.; Pousa, R.G.; Oi, D.K. Finite key effects in satellite quantum key distribution. arXiv 2020, arXiv:2012.07829. [Google Scholar]
- Scarani, V.; Renner, R. Quantum cryptography with finite resources: Unconditional security bound for discrete-variable protocols with one-way postprocessing. Phys. Rev. Lett. 2008, 100, 200501. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.; Jiang, C.; Zhu, H.-T.; Zou, M.; Yu, Z.-W.; Hu, X.-L.; Xu, H.; Ma, S.; Han, Z.; Chen, J.-P.; et al. Field Test of Twin-Field Quantum Key Distribution through Sending-or-Not-Sending over 428 km. Phys. Rev. Lett. 2021, 126, 250502. [Google Scholar] [CrossRef]
- Community Response to the NCSC 2020 Quantum Security Technologies White Paper. Available online: https://www.quantumcommshub.net/news/community-response-to-the-ncsc-2020-quantum-security-technologies-white-paper/?site=industry-government-media (accessed on 3 November 2021).



| Distilled Key Bits (Gbits) | Sat 1 | Sat 2 | Sat 3 | Sat 4 | Sat 5 | Sat 6 | Sat 7 | Sat 8 | Sat 9 | Sat 10 | Total |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Helmos | 0.136 | 0.143 | 0.147 | 0.145 | 0.126 | 1.152 | 0.144 | 0.147 | 0.144 | 0.147 | 1.435 |
| Skinakas | 0.037 | 0.04 | 0.041 | 0.04 | 0.035 | 0.04 | 0.041 | 0.04 | 0.041 | 0.04 | 0.40 |
| Cholomondas | 0.011 | 0.012 | 0.012 | 0.012 | 0.01 | 0.013 | 0.013 | 0.012 | 0.012 | 0.012 | 0.12 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ntanos, A.; Lyras, N.K.; Zavitsanos, D.; Giannoulis, G.; Panagopoulos, A.D.; Avramopoulos, H. LEO Satellites Constellation-to-Ground QKD Links: Greek Quantum Communication Infrastructure Paradigm. Photonics 2021, 8, 544. https://doi.org/10.3390/photonics8120544
Ntanos A, Lyras NK, Zavitsanos D, Giannoulis G, Panagopoulos AD, Avramopoulos H. LEO Satellites Constellation-to-Ground QKD Links: Greek Quantum Communication Infrastructure Paradigm. Photonics. 2021; 8(12):544. https://doi.org/10.3390/photonics8120544
Chicago/Turabian StyleNtanos, Argiris, Nikolaos K. Lyras, Dimitris Zavitsanos, Giannis Giannoulis, Athanasios D. Panagopoulos, and Hercules Avramopoulos. 2021. "LEO Satellites Constellation-to-Ground QKD Links: Greek Quantum Communication Infrastructure Paradigm" Photonics 8, no. 12: 544. https://doi.org/10.3390/photonics8120544
APA StyleNtanos, A., Lyras, N. K., Zavitsanos, D., Giannoulis, G., Panagopoulos, A. D., & Avramopoulos, H. (2021). LEO Satellites Constellation-to-Ground QKD Links: Greek Quantum Communication Infrastructure Paradigm. Photonics, 8(12), 544. https://doi.org/10.3390/photonics8120544








