Simultaneous Enhancement of Contrast and Power of Femtosecond Laser Pulses by Nonlinear Interferometer
Abstract
:1. Introduction
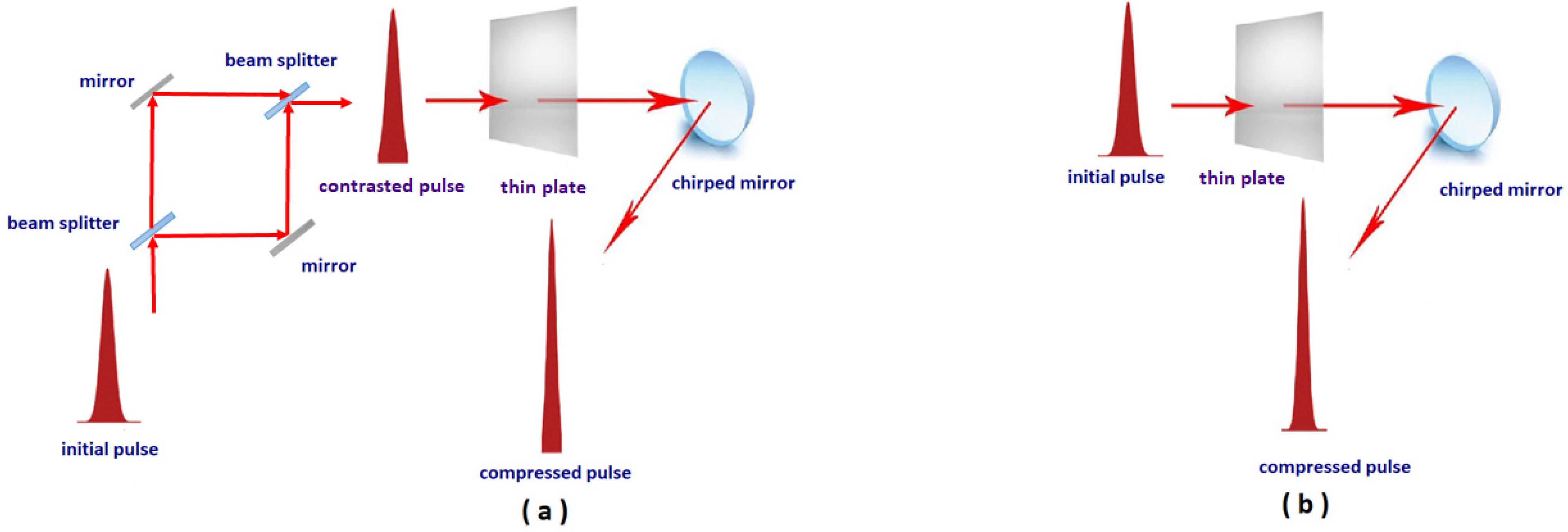
2. Nonlinear Mach–Zehnder Interferometer for Enhancement of Contrast and Pulse Compression
3. Numerical Model
4. Results and Discussion
4.1. Spectral Broadening
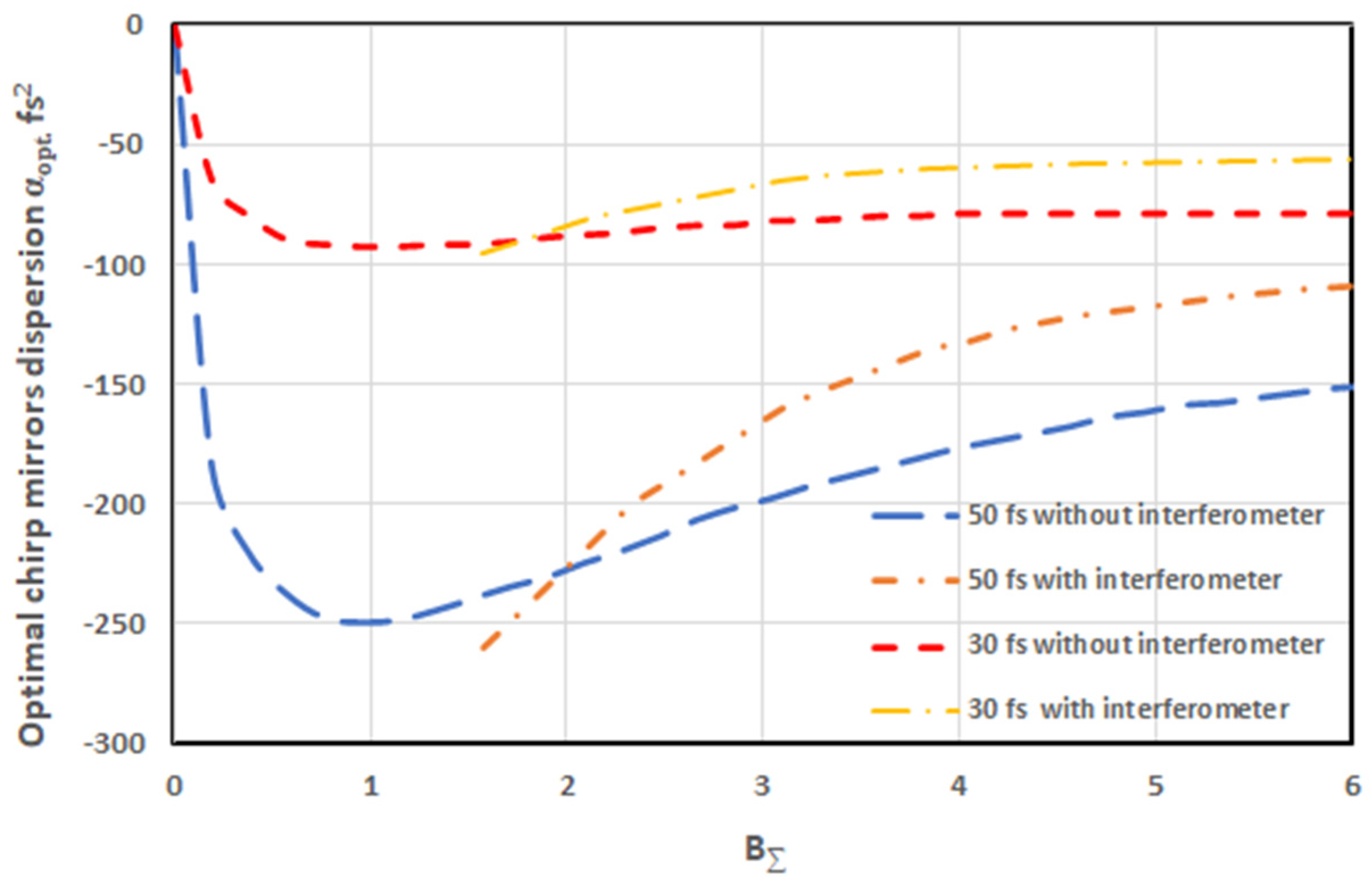
4.2. Optimal Chirped Mirror Dispersion αopt
4.3. Pulse Compression
4.4. Peak Power Increase
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Strickland, D.; Mourou, G. Compression of amplified chirped optical pulses. Opt. Commun. 1985, 56, 219–221. [Google Scholar] [CrossRef]
- Dubietis, A.; Jonusauskas, G.; Piskarskas, A. Powerful femtosecond pulse generation by chirped and stretched pulse parametric amplification in BBO crystal. Opt. Commun. 1992, 88, 437–440. [Google Scholar] [CrossRef]
- Lévy, A.; Ceccotti, T.; D’Oliveira, P.; Réau, F.; Perdrix, M.; Quéré, F.; Monot, P.; Bougeard, M.; Lagadec, H.; Martin, P.; et al. Double plasma mirror for ultrahigh temporal contrast ultraintense laser pulses. Opt. Lett. 2007, 32, 310–312. [Google Scholar] [CrossRef] [PubMed]
- Mironov, S.Y.; Lozhkarev, V.V.; Ginzburg, V.N.; Khazanov, E.A. High-efficiency second-harmonic generation of superintense ultrashort laser pulses. Appl. Opt. 2009, 48, 2051–2057. [Google Scholar] [CrossRef] [PubMed]
- Jullien, A.; Albert, O.; Burgy, F.; Hamoniaux, G.; Rousseau, J.-P.; Chambaret, J.-P.; Augé-Rochereau, F.; Chériaux, G.; Etchepare, J.; Minkovski, N.; et al. 10^-10 temporal contrast for femtosecond ultraintense lasers by cross-polarized wave generation. Opt. Lett. 2005, 30, 920–922. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Buldt, J.; Müller, M.; Klas, R.; Eidam, T.; Limpert, J.; Tünnermann, A. Temporal contrast enhancement of energetic laser pulses by filtered self-phase-modulation-broadened spectra. Opt. Lett. 2017, 42, 3761–3764. [Google Scholar] [CrossRef] [PubMed]
- Mironov, S.Y.; Starodubtsev, M.V.; Khazanov, E.A. Temporal contrast enhancement and compression of output pulses of ultra-high power lasers. Opt. Lett. 2021, 46, 1620–1623. [Google Scholar] [CrossRef] [PubMed]
- Khazanov, E.A. Enhancing the time contrast and power of femtosecond laser pulses by an optical wedge with cubic nonlinearity. Quantum Electron. 2021, 51, 433–436. [Google Scholar] [CrossRef]
- Khazanov, E. Nonlinear polarization interferometer for enhancement of laser pulse contrast and power. Opt. Express 2021, 29, 17277–17285. [Google Scholar] [CrossRef] [PubMed]
- Khazanov, E.A.; Mironov, S.Y. Nonlinear interferometer for increasing the contrast ratio of intense laser pulses. Quantum Electron. 2019, 49, 337–343. [Google Scholar] [CrossRef]
- Mourou, G.; Mironov, S.; Khazanov, E.; Sergeev, A. Single cycle thin film compressor opening the door to Zeptosecond-Exawatt physics. Eur. Phys. J. Spec. Top. 2014, 223, 1181–1188. [Google Scholar] [CrossRef]
- Ginzburg, V.N.; Yakovlev, I.V.; Zuev, A.S.; Korobeinikova, A.A.; Kochetkov, A.A.; Kuz’min, A.A.; Mironov, S.Y.; Shaykin, A.A.; Shaykin, I.A.; Khazanov, A.E. Compression after compressor: Threefold shortening of 200-TW laser pulses. Quantum Electron. 2019, 49, 299–301. [Google Scholar] [CrossRef]
- Balla, P.; Bin Wahid, A.; Sytcevich, I.; Guo, C.; Viotti, A.-L.; Silletti, L.; Cartella, A.; Alisauskas, S.; Tavakol, H.; Grosse-Wortmann, U.; et al. Postcompression of picosecond pulses into the few-cycle regime. Opt. Lett. 2020, 45, 2572–2575. [Google Scholar] [CrossRef] [PubMed]
- Ginzburg, V.; Yakovlev, I.; Zuev, A.; Korobeynikova, A.; Kochetkov, A.; Kuzmin, A.; Mironov, S.; Shaykin, A.; Shaikin, I.; Khazanov, E.; et al. Fivefold compression of 250-TW laser pulses. Phys. Rev. A 2020, 101, 013829. [Google Scholar] [CrossRef]
- Ginzburg, V.N.; Yakovlev, I.V.; Zuev, A.S.; Korobeynikova, A.P.; Kochetkov, A.A.; Kuzmin, A.A.; Mironov, S.Y.; Shaykin, A.A.; Shaikin, I.A.; Khazanov, E.A. Two-stage nonlinear compression of high-power femtosecond laser pulses. Quantum Electron. 2020, 50, 331–334. [Google Scholar] [CrossRef]
- Ginzburg, V.; Yakovlev, I.; Kochetkov, A.; Kuzmin, A.; Mironov, S.; Shaikin, I.; Shaykin, A.; Khazanov, E. 11 fs, 1.5 PW laser with nonlinear pulse compression. Opt. Express 2021, 29, 28297–28306. [Google Scholar] [CrossRef] [PubMed]
- Khazanov, E.A.; Mironov, S.Y.; Mourou, G. Nonlinear compression of high-power laser pulses: Compression after compressor approach. Phys.-Uspekhi 2019, 62, 1096–1124. [Google Scholar] [CrossRef]
- Anderson, D.P.; Lisak, M. Nonlinear asymmetric self-phase modulation and self-steepening of pulses in long optical waveguides. Phys. Rev. A 1983, 27, 1393–1398. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nada, Y.; Khazanov, E. Simultaneous Enhancement of Contrast and Power of Femtosecond Laser Pulses by Nonlinear Interferometer. Photonics 2021, 8, 520. https://doi.org/10.3390/photonics8110520
Nada Y, Khazanov E. Simultaneous Enhancement of Contrast and Power of Femtosecond Laser Pulses by Nonlinear Interferometer. Photonics. 2021; 8(11):520. https://doi.org/10.3390/photonics8110520
Chicago/Turabian StyleNada, Yasser, and Efim Khazanov. 2021. "Simultaneous Enhancement of Contrast and Power of Femtosecond Laser Pulses by Nonlinear Interferometer" Photonics 8, no. 11: 520. https://doi.org/10.3390/photonics8110520
APA StyleNada, Y., & Khazanov, E. (2021). Simultaneous Enhancement of Contrast and Power of Femtosecond Laser Pulses by Nonlinear Interferometer. Photonics, 8(11), 520. https://doi.org/10.3390/photonics8110520




