The Role of Electron Transfer in the Nonlinear Response of Ge2Sb2Te5-Mediated Plasmonic Dimers
Abstract
1. Introduction
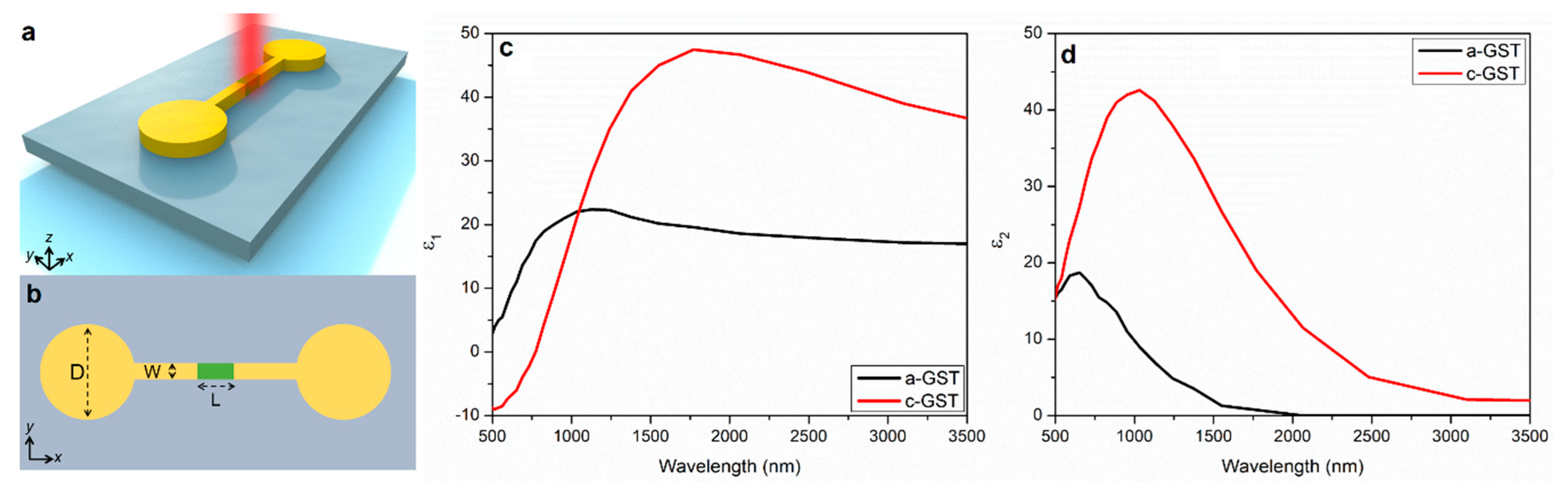
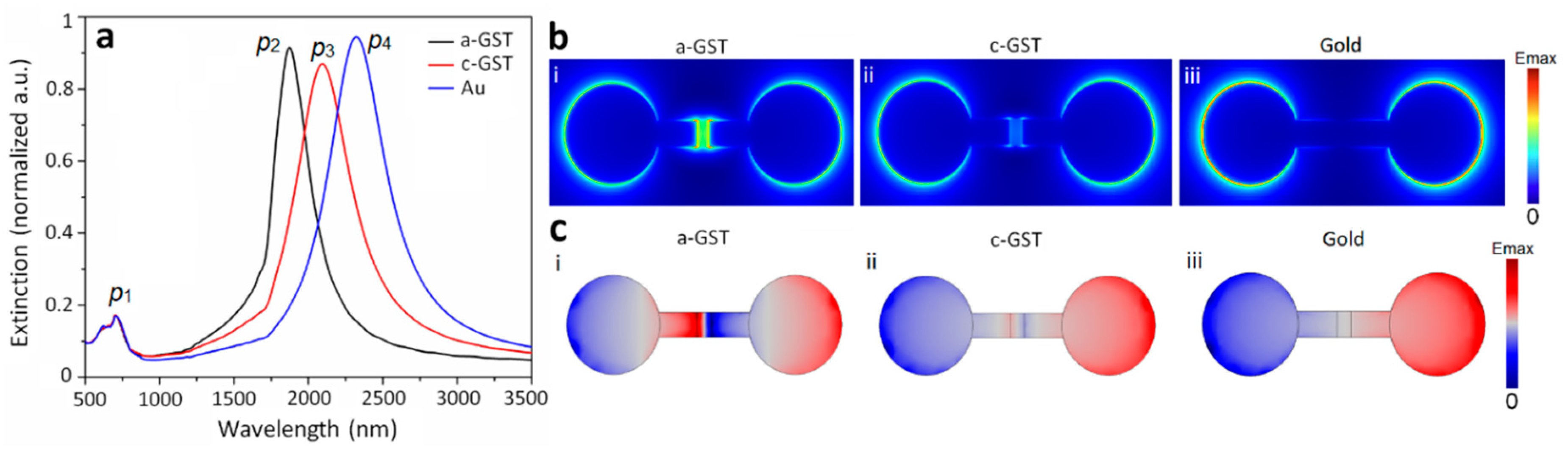
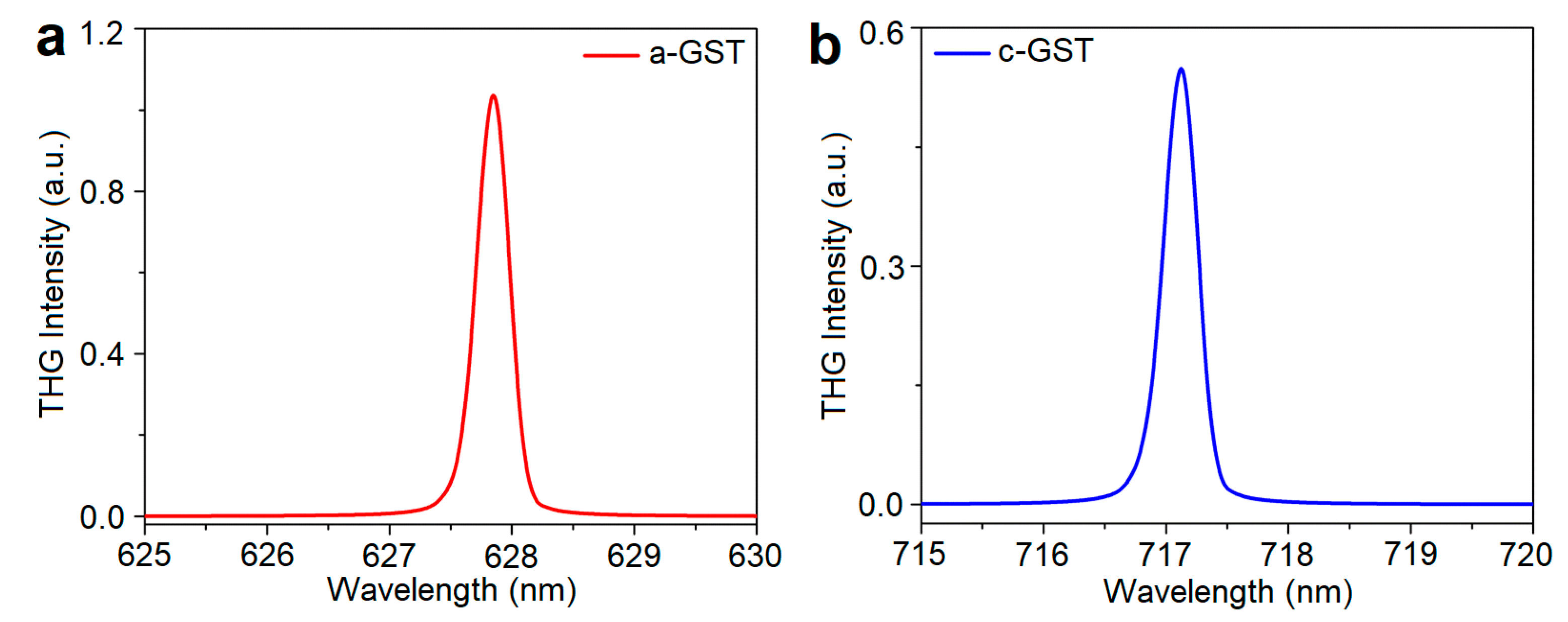
2. Results and Discussion
3. Numerical Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Suh, J.Y.; Kim, C.H.; Zhou, W.; Huntington, M.D.; Co, D.T.; Wasielewski, M.R.; Odom, T.W. Plasmonic bowtie nanolaser arrays. Nano Lett. 2012, 12, 5769–5774. [Google Scholar] [CrossRef] [PubMed]
- Ropers, C.; Neacsu, C.C.; Elsaesser, T.; Albrecht, M.; Raschke, M.B.; Lienau, C. Grating-coupling of surface plasmons onto metallic tips: A nanoconfined light source. Nano Lett. 2007, 7, 2784–2788. [Google Scholar] [CrossRef]
- Neira, A.D.; Olivier, N.; Nasir, M.E.; Dickson, W.; Wurtz, G.A.; Zayats, A.V. Eliminating material constraints for nonlinearity with plasmonic metamaterials. Nat. Commun. 2015, 6, 7757. [Google Scholar] [CrossRef] [PubMed]
- Kauranen, M.; Zayats, A.V. Nonlinear plasmonics. Nat. Photon. 2012, 6, 737–748. [Google Scholar] [CrossRef]
- Ferray, M.; L’Huillier, A.; Li, X.F.; Lompre, L.A.; Mainfray, G.; Manus, C. Multiple-Harmonic Conversion of 1064 nm Radiation in Rare Gases. J. Phys. B At. Mol. Opt. Phys. 1988, 21, L31. [Google Scholar] [CrossRef]
- Gerislioglu, B.; Ahmadivand, A.; Pala, N. Hybridized plasmons in graphene nanorings for extreme nonlinear optics. Opt. Mater. 2017, 73, 729–735. [Google Scholar] [CrossRef]
- Ahmadivand, A.; Gerislioglu, B.; Pala, N. Optothermally controllable multiple high-order harmonics generation by Ge2Sb2Te5-mediated Fano clusters. Opt. Mater. 2018, 84, 301–306. [Google Scholar] [CrossRef]
- Cai, W.; Vasudev, A.P.; Brongersma, M.L. Electrically controlled nonlinear generation of light with plasmonics. Science 2011, 333, 1720–1723. [Google Scholar] [CrossRef]
- Thyagarajan, K.; Butet, J.; Martin, O.J. Augmenting second harmonic generation using Fano resonances in plasmonic systems. Nano Lett. 2013, 13, 1847–1851. [Google Scholar] [CrossRef]
- Shcherbakov, M.R.; Neshev, D.N.; Hopkins, B.; Shorokhov, A.S.; Staude, I.; Melik-Gaykazyan, E.V.; Decker, M.; Ezhov, A.A.; Miroshnichenko, A.E.; Brener, I.; et al. Enhanced third-harmonic generation in silicon nanoparticles driven by magnetic response. Nano Lett. 2014, 14, 6488–6492. [Google Scholar] [CrossRef]
- Cox, J.D.; Marini, A.; de Abajo, F.J.G. Plasmon-assisted high-harmonic generation in graphene. Nat. Commun. 2017, 8, 14380. [Google Scholar] [CrossRef]
- Hong, S.-Y.; Dadap, J.I.; Petrone, N.; Yeh, P.-C.; Hone, J.; Osgood, R.M., Jr. Optical third-harmonic generation in graphene. Phys. Rev. X 2013, 3, 021014. [Google Scholar] [CrossRef]
- Ahmadivand, A.; Gerislioglu, B.; Noe, G.T.; Mishra, Y.K. Gated graphene enabled tunable charge-current configurations in hybrid plasmonic metamaterials. ACS Appl. Electron. Mater. 2019. [Google Scholar] [CrossRef]
- Wang, X.; Chen, C.; Pan, L.; Wang, J. A graphene-based Fabry-Perot spectrometer in mid-infrared region. Sci. Rep. 2016, 6, 32616. [Google Scholar] [CrossRef]
- Romero, I.; Aizpurua, J.; Bryant, G.W.; de Abajo, F.J.G. Plasmons in nearly touching metallic nanoparticles: Singular response in the limit of touching dimers. Opt. Express 2016, 14, 9988–9999. [Google Scholar] [CrossRef]
- Ahmadivand, A.; Gerislioglu, B.; Ramezani, Z. Gated graphene island-enabled tunable charge transfer plasmon terahertz metamodulator. Nanoscale 2019, 11, 8091–8095. [Google Scholar] [CrossRef] [PubMed]
- Gerislioglu, B.; Ahmadivand, A.; Pala, N. Optothermally controlled charge transfer plasmons in Au-Ge2Sb2Te5 core-shell dimers. Plasmonics 2018, 13, 1921–1928. [Google Scholar] [CrossRef]
- Perez-Gonzalez, O.; Zabala, N.; Borisov, A.G.; Halas, N.J.; Nordlander, P.; Aizpurua, J. Optical spectroscopy of conductive junctions in plasmonic cavities. Nano Lett. 2010, 10, 3090–3095. [Google Scholar] [CrossRef]
- Perez-Gonzalez, O.; Zabala, N.; Aizpurua, J. Optical characterization of charge transfer and bonding dimer plasmons in linked interparticle gaps. New J. Phys. 2011, 13, 083013. [Google Scholar] [CrossRef]
- Savage, K.J.; Hawkeye, M.M.; Esteban, R.; Borisov, A.G.; Aizpurua, J.; Baumberg, J.J. Revealing the quantum regime in tunneling plasmons. Nature 2012, 491, 574–577. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Li, Z.; Zhao, K.; Sobhani, A.; Zhu, X.; Fang, Z.; Halas, J.N. Substrate-mediated charge transfer plasmons in simple and complex nanoparticle clusters. Nanoscale 2013, 5, 9897–9901. [Google Scholar] [CrossRef] [PubMed]
- Tserkezis, C.; Herrmann, L.O.; Valev, V.K.; Baumberg, J.J.; Aizpurua, J. Optical response of threaded chain plasmons: From capacitive chains to continuous nanorods. Opt. Express 2014, 22, 23851–23860. [Google Scholar] [CrossRef]
- Tan, S.F.; Wu, L.; Yang, J.K.W.; Bai, P.; Bosman, M.; Nijhuis, C.A. Quantum plasmon resonances controlled by molecular tunnel junctions. Science 2014, 343, 1496–1499. [Google Scholar] [CrossRef]
- Georgiou, G.; Tserkezis, C.; Schaafsma, M.C.; Aizpurua, J.; Rivas, J.G. Active loaded plasmonic antennas at terahertz frequencies: Optical control of their capacitive-inductive coupling. Phys. Rev. B 2015, 91, 125443. [Google Scholar] [CrossRef]
- Gerislioglu, B.; Ahmadivand, A.; Pala, N. Functional quadrumer clusters for switching between Fano and charge transfer plasmons. IEEE Photon. Technol. Lett. 2017, 29, 2226–2229. [Google Scholar] [CrossRef]
- Ahmadivand, A.; Gerislioglu, B.; Pala, N. Azimuthally and radially excited charge transfer plasmon and Fano lineshapes in conductive sublayer-mediated nanoassemblies. J. Opt. Am. A 2017, 34, 2052–2056. [Google Scholar] [CrossRef]
- Ahmadivand, A.; Gerislioglu, B.; Sinha, R.; Karabiyik, M.; Pala, N. Optical switching using transition from dipolar to charge transfer plasmon modes in Ge2Sb2Te5 bridged metallodielectric dimers. Sci. Rep. 2017, 7, 42807. [Google Scholar] [CrossRef]
- Ahmadivand, A.; Gerislioglu, B.; Pala, N. Graphene optical switch based on charge transfer plasmons. Phys. Status Solidi-R 2017, 11, 1700285. [Google Scholar] [CrossRef]
- Kim, J.-H. Correlation between microstructure and optical properties of Ge2Sb2Te5 thin films. J. Appl. Phys. 1999, 86, 6770–6772. [Google Scholar] [CrossRef]
- Bakan, G.; Gerislioglu, B.; Dirisaglik, F.; Jurado, Z.; Sullivan, L.; Dana, A.; Lam, C.; Gokirmak, A.; Silva, H. Extracting the temperature distribution on a phase-change memory cell during crystallization. J. Appl. Phys. 2016, 120, 164504. [Google Scholar] [CrossRef]
- Palik, E.D. Handbook of Optical Constants of Solids; Academic Press: Orlando, FL, USA, 1985. [Google Scholar]
- Jackson, J.D. Classical Electrodynamics; Wiley: Hoboken, NJ, USA, 1999. [Google Scholar]
- Shportko, K.; Kremers, S.; Woda, M.; Lencer, D.; Robertson, J.; Wuttig, M. Resonant bonding in crystalline phase-change materials. Nat. Mater. 2008, 7, 653–658. [Google Scholar] [CrossRef] [PubMed]
- Zheng, F.; Chen, Z. Numerical dispersion analysis of the unconditionally stable 3-D ADI-FDTD method. IEEE Trans. Microwave Theory Tech. 2001, 49, 1006–1009. [Google Scholar] [CrossRef]
- Lassiter, J.B.; Chen, X.; Liu, X.; Ciracì, C.; Hoang, T.B.; Larouche, S.; Oh, S.; Mikkelsen, M.H.; Smith, D.R. Third-harmonic generation enhancement by film-coupled plasmonic stripe resonators. ACS Photonics 2014, 1, 1212–1217. [Google Scholar] [CrossRef]
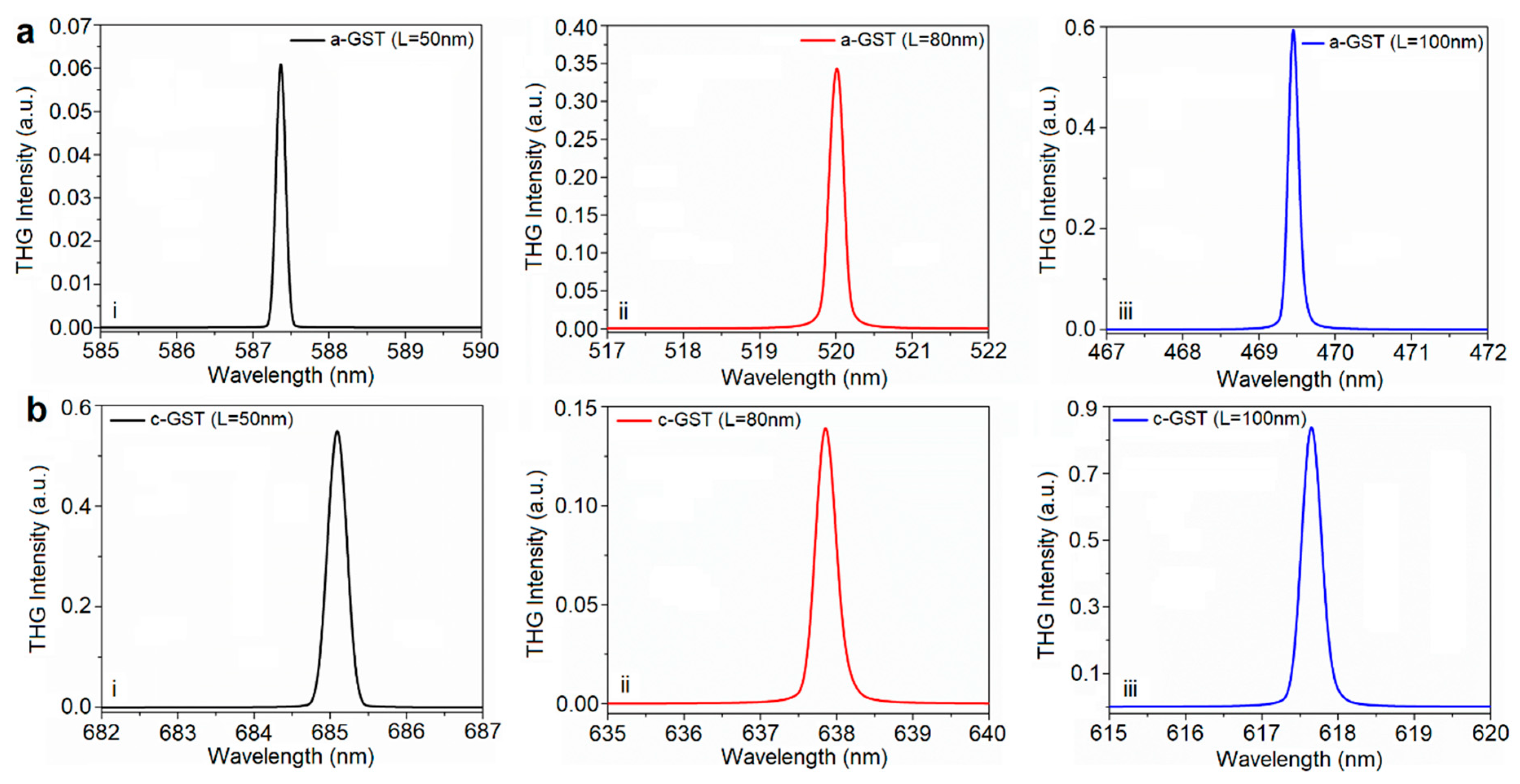

| Length (L) | Width (W) | |||||
|---|---|---|---|---|---|---|
| 50 nm | 80 nm | 100 nm | 40 nm | 60 nm | 80 nm | |
| Third harmonic signal intensity | 0.0625 | 0.347 | 0.59 | 2.23 | 0.92 | 10.8 |
| Third harmonic signal position | 587.4 nm | 520 nm | 469.5 nm | 630.55 nm | 624.4 nm | 620.4 nm |
| Length (L) | Width (W) | |||||
|---|---|---|---|---|---|---|
| 50 nm | 80 nm | 100 nm | 40 nm | 60 nm | 80 nm | |
| Third harmonic signal intensity | 0.55 | 0.14 | 0.83 | 1.75 | 0.3 | 0.22 |
| Third harmonic signal position | 685.1 nm | 637.9 nm | 617.6 nm | 735.6 nm | 701.9 nm | 678.4 nm |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gerislioglu, B.; Ahmadivand, A. The Role of Electron Transfer in the Nonlinear Response of Ge2Sb2Te5-Mediated Plasmonic Dimers. Photonics 2019, 6, 52. https://doi.org/10.3390/photonics6020052
Gerislioglu B, Ahmadivand A. The Role of Electron Transfer in the Nonlinear Response of Ge2Sb2Te5-Mediated Plasmonic Dimers. Photonics. 2019; 6(2):52. https://doi.org/10.3390/photonics6020052
Chicago/Turabian StyleGerislioglu, Burak, and Arash Ahmadivand. 2019. "The Role of Electron Transfer in the Nonlinear Response of Ge2Sb2Te5-Mediated Plasmonic Dimers" Photonics 6, no. 2: 52. https://doi.org/10.3390/photonics6020052
APA StyleGerislioglu, B., & Ahmadivand, A. (2019). The Role of Electron Transfer in the Nonlinear Response of Ge2Sb2Te5-Mediated Plasmonic Dimers. Photonics, 6(2), 52. https://doi.org/10.3390/photonics6020052






