Noise Suppression in Quadrature Phase-Shift-Keying-Oriented All-Optical Matching Systems Using Highly Nonlinear Fiber
Abstract
1. Introduction
2. QPSK-Oriented All-Optical Matching System and Noise Analysis
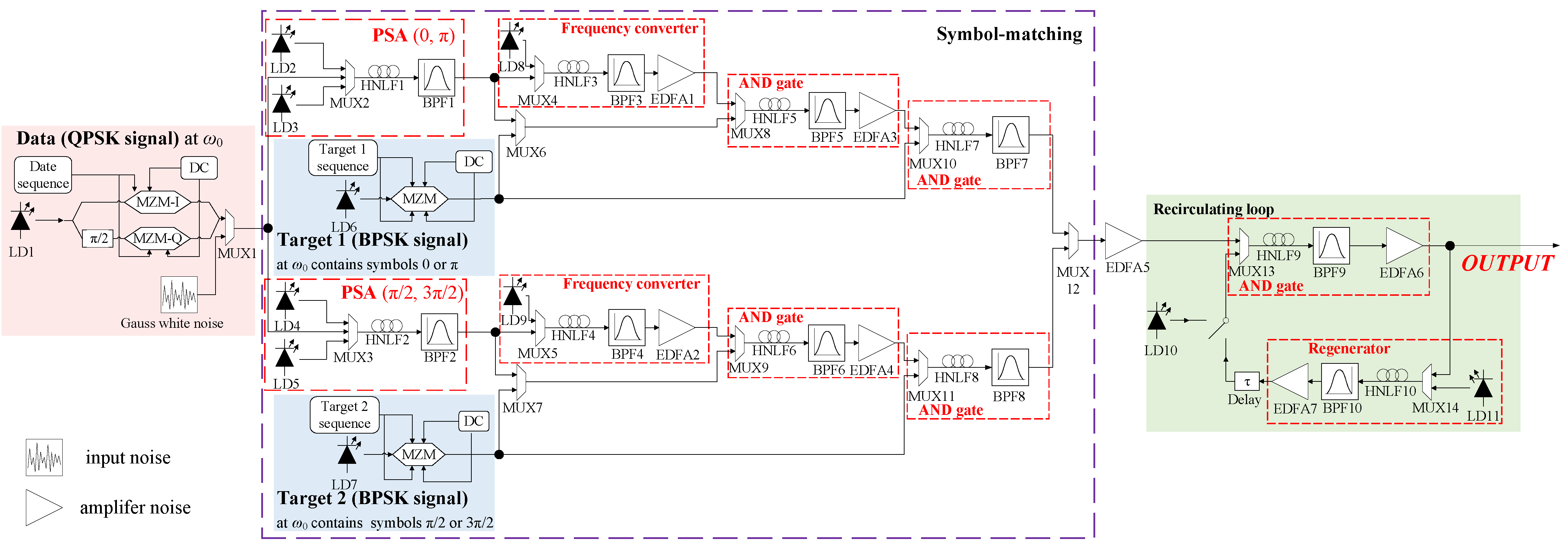
2.1. QPSK-Oriented All-Optical Matching System
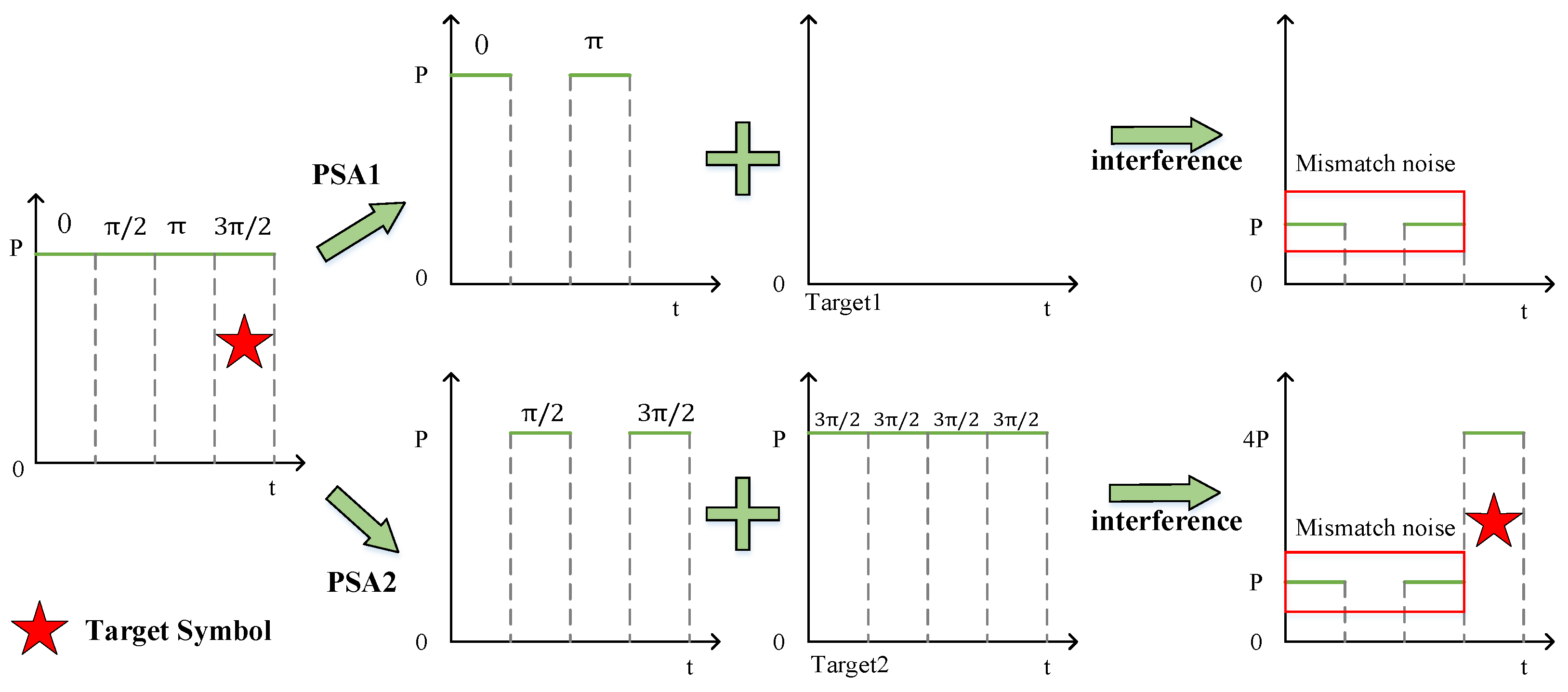
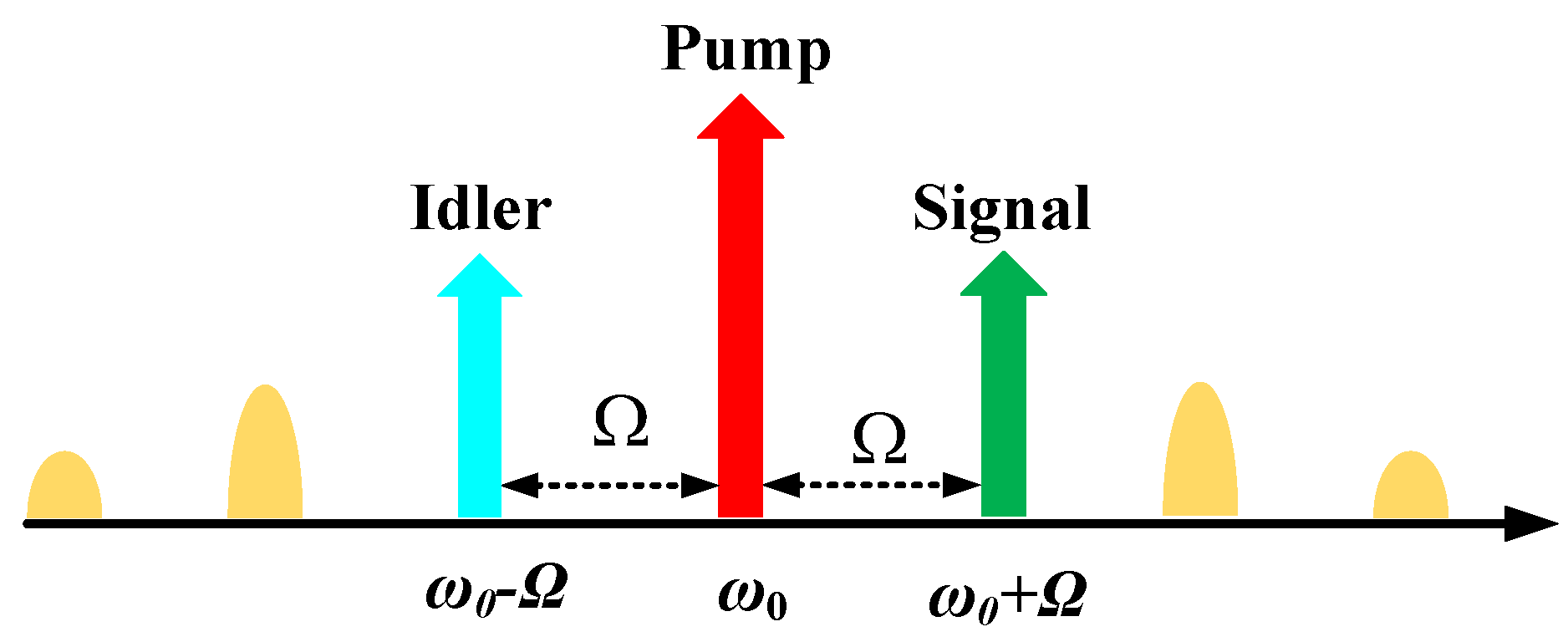
2.2. Noise in the QPSK-Oriented All-Optical Matching System
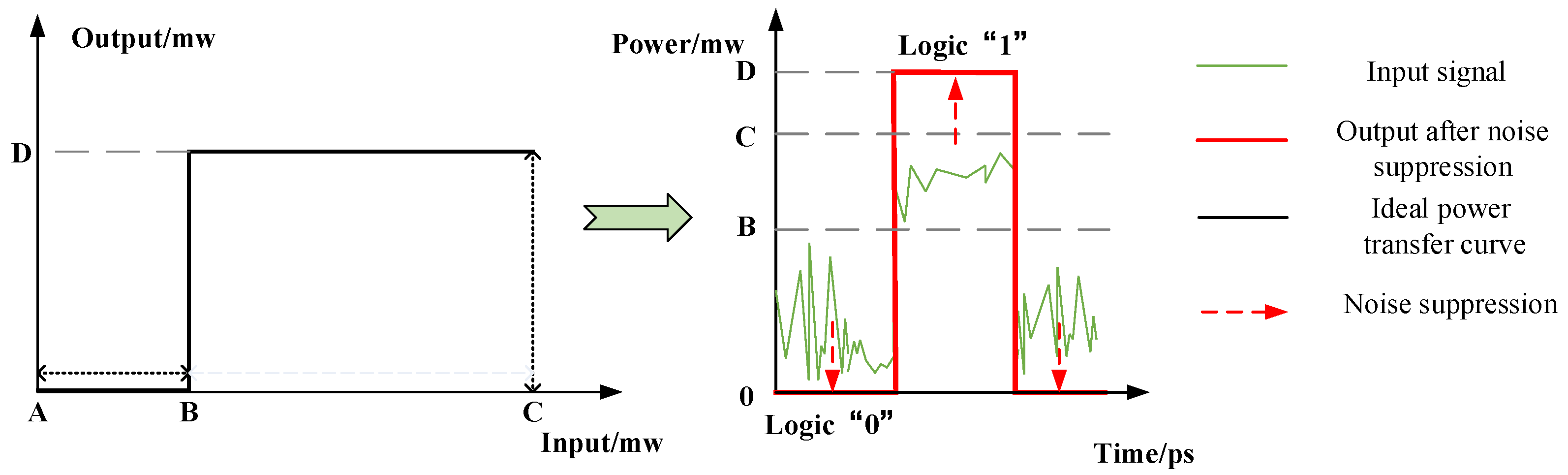
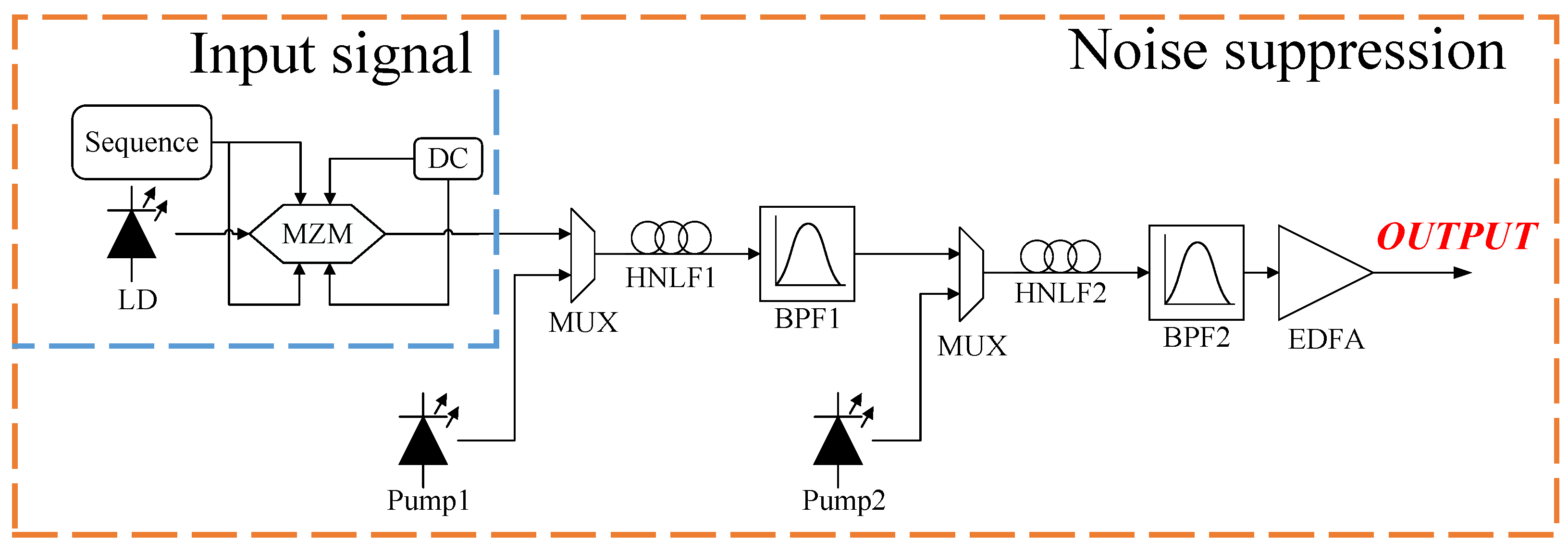
3. Second-Order Noise-Suppression Structure
4. Analysis and Simulation
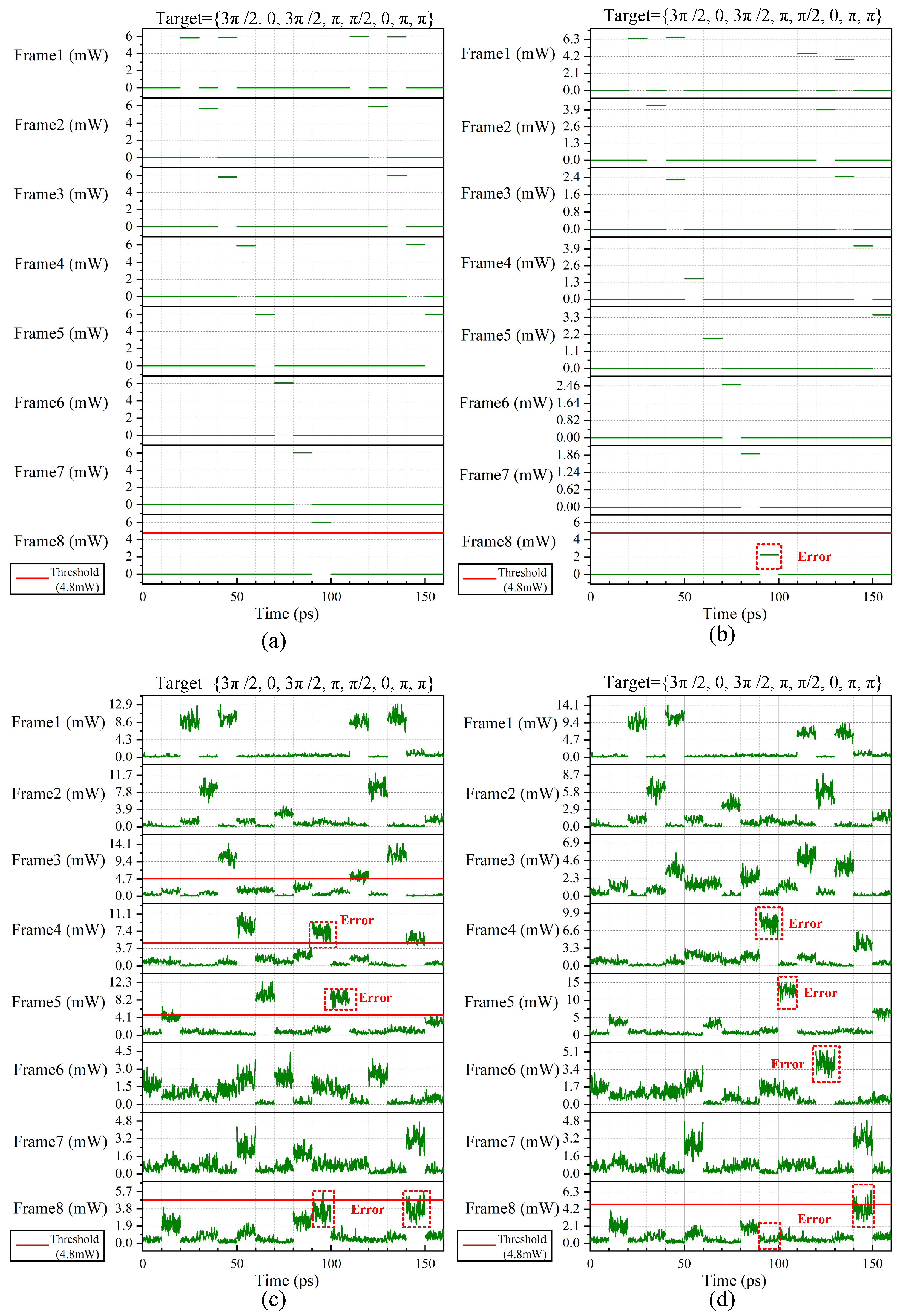
4.1. Noise Analysis
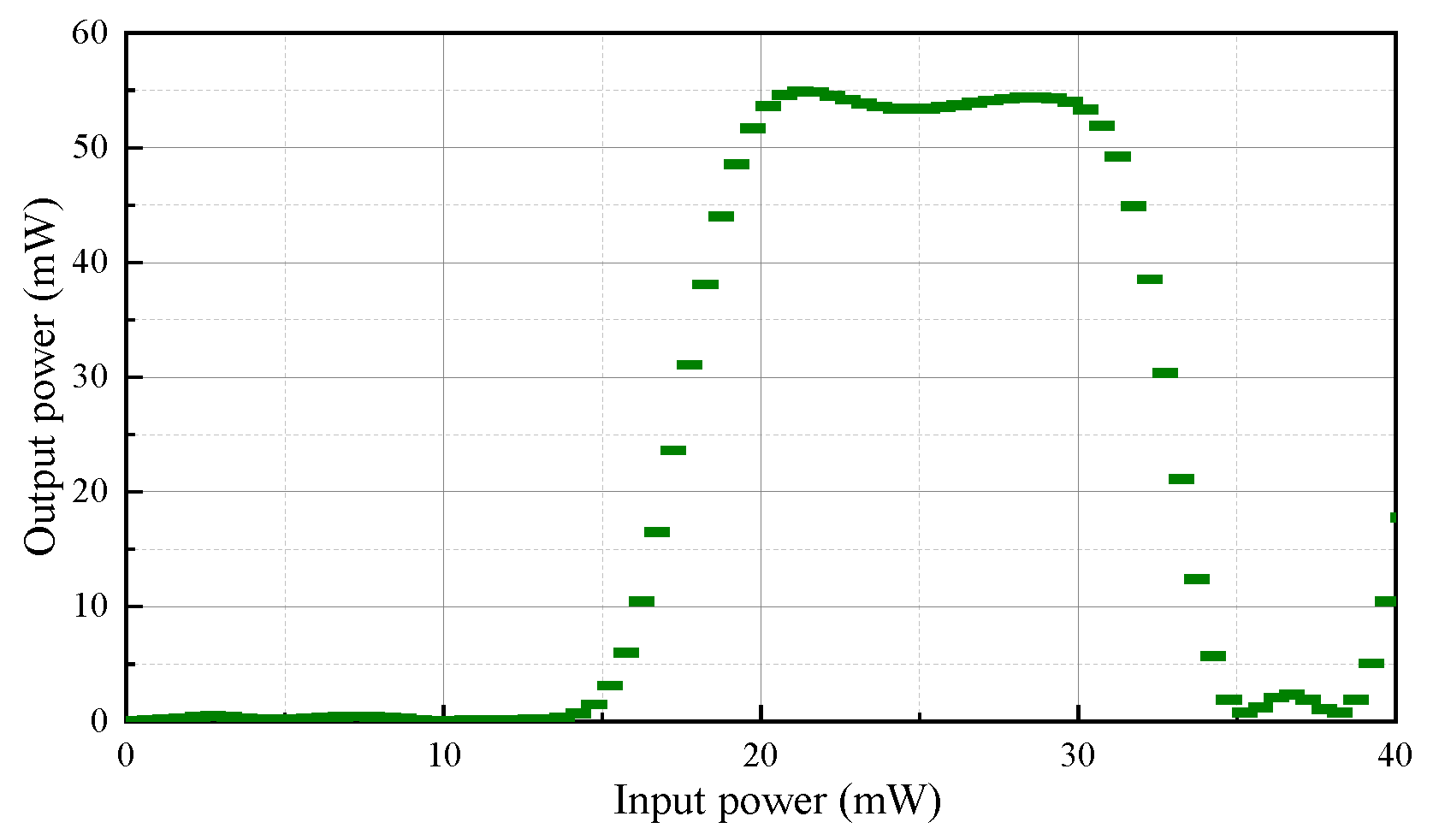
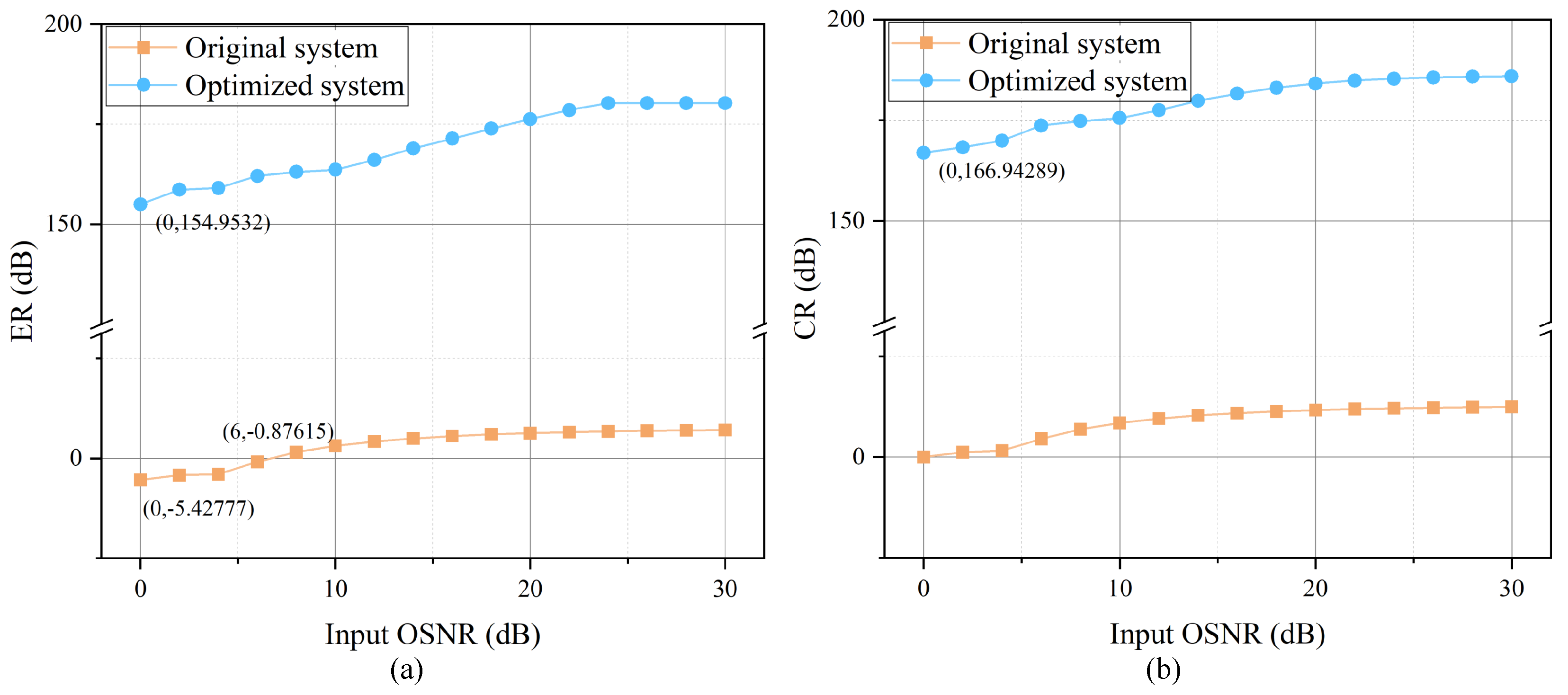
4.2. Noise Suppression
5. Limitations and Challenges
5.1. Limitations in Scalability
5.2. Future Challenges
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dahan, D.; Mahlab, U. Security threats and protection procedures for optical networks. IET Optoelectron. 2017, 11, 186–200. [Google Scholar] [CrossRef]
- Zhao, C.; Li, X.; Xin, J.; Liu, Y.; Li, D.; Huang, S. Intelligent Agent-Based Dynamic Reliability Evaluation for Optical Networks: A Comprehensive Framework and Case Study. IEEE Commun. Mag. 2024, 63, 151–157. [Google Scholar] [CrossRef]
- Du, Y.; Xue, F.; Yoo, S.J.B.; Ding, Z. Security enhancement of SPECTS O-CDMA through concealment against upstream DPSK eavesdropping. J. Light. Technol. 2007, 25, 2799–2806. [Google Scholar] [CrossRef]
- Yin, X.; Pan, D.; Yu, H.; Huo, Y.; Li, F.; Wang, Q. Research of Long-Distance Encrypted Signal Transmission Enhancement Method Based on Quantum Communication Power System. In Proceedings of the 2018 IEEE 9th International Conference on Software Engineering and Service Science (ICSESS), Beijing, China, 23–25 November 2018; pp. 403–405. [Google Scholar] [CrossRef]
- Zhang, W.; Zhang, C.; Jin, W.; Chen, C.; Jiang, N.; Qiu, K. Chaos Coding-Based QAM IQ-Encryption for Improved Security in OFDMA-PON. IEEE Photonics Technol. Lett. 2014, 26, 1964–1967. [Google Scholar] [CrossRef]
- Yang, X.; Webb, R.; Manning, R.; Maxwell, G.; Poustie, A.; Lardenois, S.; Cotter, D. Demonstration of all-optical pattern recognition at 42Gbit/s. In Proceedings of the 2008 34th European Conference on Optical Communication, Brussels, Belgium, 21–25 September 2008; pp. 1–4. [Google Scholar] [CrossRef]
- Webb, R.P.; Yang, X.; Manning, R.J.; Maxwell, G.D.; Poustie, A.J.; Lardenois, S.; Cotter, D. All-Optical Binary Pattern Recognition at 42 Gb/s. J. Light. Technol. 2009, 27, 2240–2245. [Google Scholar] [CrossRef]
- Zhao, Y.; Lombardo, D.; Mathews, J.; Agha, I. Low-power optical logic gate in a silicon waveguide. In Proceedings of the 2017 Conference on Lasers and Electro-Optics (CLEO), San Jose, CA, USA, 14–19 May 2017; pp. 1–2. [Google Scholar]
- Butt, M.; Khonina, S.; Kazanskiy, N. Recent advances in photonic crystal optical devices: A review. Opt. Laser Technol. 2021, 142, 107265. [Google Scholar] [CrossRef]
- Lovkesh; Marwaha, A. Implementation of optical logic gates at 160 Gbps using nonlinear effect of single SOA. Opt. Laser Technol. 2015, 70, 112–118. [Google Scholar] [CrossRef]
- Siarkos, T.; Zoiros, K.E.; Nastou, D. On the feasibility of full pattern-operated all-optical XOR gate with single semiconductor optical amplifier-based ultrafast nonlinear interferometer. Opt. Commun. 2009, 282, 2729–2740. [Google Scholar] [CrossRef]
- Kotb, A.; Zoiros, K.E.; Li, W. Execution of all-optical Boolean OR logic using carrier reservoir semiconductor optical amplifier-assisted delayed interferometer. Opt. Laser Technol. 2021, 142, 107230. [Google Scholar] [CrossRef]
- Liu, Y.; Li, X.; Tang, Y.; Shi, Z.; Huang, S. Binary sequence matching system based on cross-phase modulation and four-wave mixing in highly nonlinear fibers. Opt. Eng. 2020, 59, 105103. [Google Scholar] [CrossRef]
- Zhang, Q.; Gong, X.; Guo, L. All-Optical QPSK Pattern Recognition in High-Speed Optoelectronic Firewalls. IEEE Photonics J. 2023, 15, 1–16. [Google Scholar] [CrossRef]
- Shi, Z.; Li, X.; Shi, H.; Liu, Y.; Guo, K.; Huang, S. All-optical matching structure for multi-order modulation formats. Opt. Eng. 2023, 62, 068106. [Google Scholar] [CrossRef]
- Tang, Y.; Li, X.; Shi, H.; Chang, J.; Tian, Y.; Huang, S. Reconfigurable all-optical pattern-matching system for phase modulation formats based on phase-sensitive amplification in highly nonlinear fiber. Opt. Fiber Technol. 2023, 81, 103548. [Google Scholar] [CrossRef]
- Ruan, F.; Li, X.; Shi, H.; Liu, Y.; Gao, T.; Guo, K.; Huang, S. Phase-locking-free all-optical matching system for 100 Gbaud QPSK optical signal. Opt. Express 2024, 32, 26006–26025. [Google Scholar] [CrossRef]
- Matsumoto, M.; Shimada, Y.; Sakaguchi, H. Two-Stage SPM-Based All-Optical 2R Regeneration by Bidirectional Use of a Highly Nonlinear Fiber. IEEE J. Quantum Electron. 2009, 45, 51–58. [Google Scholar] [CrossRef]
- Suzuki, J.; Tanemura, T.; Kikuchi, K. All-optical regeneration of 40-Gb/s low-Q signal using XPM-induced wavelength shift in highly-nonlinear fiber. In Proceedings of the 2005 31st European Conference on Optical Communication, ECOC 2005, Glasgow, UK, 25–29 September 2005; Volume 2, pp. 199–200. [Google Scholar] [CrossRef]
- Cai, Y.; Wen, F.; Guo, B.; Wu, B.; Qiu, K. All-optical M-PSK Signal Regeneration using a Nonlinear-optical Loop Mirror (NOLM). In Proceedings of the 2020 Asia Communications and Photonics Conference (ACP) and International Conference on Information Photonics and Optical Communications (IPOC), Beijing, China, 24–27 October 2020; pp. 1–3. [Google Scholar]
- Chen, X.; Huo, L.; Jiang, X.; Lou, C. 100-Gb/s 2R regeneration using cross gain compression in semiconductor optical amplifiers. Opt. Express 2015, 23, 23143–23154. [Google Scholar] [CrossRef]
- Shao, L.; Wen, F.; Guo, B.; Krzczanowicz, L.; Yang, F.; Wu, B.; Qiu, K. All-optical Amplitude Noise Suppression in a Nonlinear Semiconductor Optical Amplifier (SOA). In Proceedings of the 2020 Asia Communications and Photonics Conference (ACP) and International Conference on Information Photonics and Optical Communications (IPOC), Beijing, China, 24–27 October 2020; pp. 1–3. [Google Scholar]
- Mohd Shah, N.S.; Matsumoto, M. All-optical regeneration of time-interleaved multi-wavelength signals based on higher-order four-wave mixing. In Proceedings of the 2009 IEEE/LEOS Summer Topical Meeting, Newport Beach, CA, USA, 20–22 July 2009; pp. 103–104. [Google Scholar] [CrossRef]
- Zhou, X.y.; Wu, B.j.; Wen, F.; Zhang, H.c.; Zhou, H.; Qiu, K. Total date rate of multi-wavelength 2R regenerators for time-interleaved RZ-OOK signals. Opt. Express 2014, 22, 22937–22951. [Google Scholar] [CrossRef]
- Li, Q.; Wen, H.; Yang, J.; Xu, Q.; Yang, X.; Li, Y. All-Optical Regeneration and Format Conversion for 4APSK Signals Based on Nonlinear Effects in HNLF. IEEE Photonics J. 2023, 15, 1–9. [Google Scholar] [CrossRef]
- Guo, K.; Li, X.; Shi, H.; Liu, Y.; Shi, Z.; Huang, S. Noise Suppression of All-optical OOK Matching System Based on Cascaded Highly Nonlinear Fiber. In Proceedings of the 2023 21st International Conference on Optical Communications and Networks (ICOCN), Qufu, China, 31 July 2023–3 August 2023; pp. 1–3. [Google Scholar] [CrossRef]
- Poggiolini, P. The GN Model of Non-Linear Propagation in Uncompensated Coherent Optical Systems. J. Light. Technol. 2012, 30, 3857–3879. [Google Scholar] [CrossRef]
- Cho, H.J. Generalized optical signal-to-noise ratio monitoring using a convolutional neural network for digital coherent receivers. Opt. Lett. 2023, 48, 1798–1801. [Google Scholar] [CrossRef]
- Khan, I.; Bilal, M.; Umar Masood, M.; D’Amico, A.; Curri, V. Lightpath QoT computation in optical networks assisted by transfer learning. J. Opt. Commun. Netw. 2021, 13, B72–B82. [Google Scholar] [CrossRef]
- Agrawal, G.P. Loss Management. In Fiber-Optic Communication Systems; John Wiley and Sons, Ltd.: Hoboken, NJ, USA, 2011; pp. 295–344. [Google Scholar] [CrossRef]
- Alnair Labs. LNA-220 Ultra-Low Noise Amplifier. Available online: http://www.alnair-labs.com/product-lineup/product-lna-220/ (accessed on 20 December 2024).
- Hansryd, J.; Andrekson, P.; Westlund, M.; Li, J.; Hedekvist, P.O. Fiber-based optical parametric amplifiers and their applications. IEEE J. Sel. Top. Quantum Electron. 2002, 8, 506–520. [Google Scholar] [CrossRef]
- Kakarla, R.; Venkitesh, D. Demonstration of optical header recognition for BPSK data using novel design of logic gates. Opt. Commun. 2016, 363, 117–122. [Google Scholar] [CrossRef]
- Norimatsu, S.; Maruoka, M. Accurate Q-factor estimation of optically amplified systems in the presence of waveform distortions. J. Light. Technol. 2002, 20, 19–27. [Google Scholar] [CrossRef]
- Lovkesh, S.; Sharma, V.; Singh, S. The design of a reconfigurable all-optical logic device based on cross-phase modulation in a highly nonlinear fiber. J. Comput. Electron. 2021, 20, 1–12. [Google Scholar] [CrossRef]
- Xu, M.; Zhu, Y.; Pittalà, F.; Tang, J.; He, M.; Ng, W.C.; Wang, J.; Ruan, Z.; Tang, X.; Kuschnerov, M.; et al. Dual-polarization thin-film lithium niobate in-phase quadrature modulators for terabit-per-second transmission. Optica 2022, 9, 61–62. [Google Scholar] [CrossRef]
- COHERENT. 100 GHz Balanced Photodetector BPDV412xRv. Available online: https://www.coherent.com/content/dam/coherent/site/en/resources/datasheet/networking/100ghz-balanced-photodetector-ds.pdf (accessed on 20 December 2024).
- Yokogawa. AQ6370 Series Optical Spectrum Analyzer. Available online: https://cdn.tmi.yokogawa.com/1/2706/files/BUAQ6370SR-20EN.pdf (accessed on 1 May 2025).
- Keysight. Infiniium UXR-B Series Oscilloscopes. Available online: https://www.keysight.com.cn/cn/zh/assets/3123-1371/technical-overviews/Infiniium-UXR-Series-Oscilloscopes1.pdf (accessed on 1 May 2025).
- Chul Park, H.; Lu, M.; Bloch, E.; Reed, T.; Griffith, Z.; Johansson, L.; Coldren, L.; Rodwell, M. 40Gbit/s coherent optical receiver using a Costas loop. Opt. Express 2012, 20, B197–B203. [Google Scholar] [CrossRef]
- Zhang, L.; Xie, W.; Feng, Y.; Meng, Y.; Bai, Y.; Yang, J.; Wei, W.; Dong, Y. Modeling and optimization of an unbalanced delay interferometer based OPLL system. Opt. Express 2022, 30, 1994–2005. [Google Scholar] [CrossRef]





| Module | Parameters | Values |
|---|---|---|
| Input signal | Center frequency | 193.1 THz |
| Pump1 | Center frequency | 193.3 THz |
| Power | 300 mW | |
| Pump2 | Center frequency | 193.3 THz |
| Power | 310 mW | |
| HNLF1 | Length | 1200 m |
| Attenuation | dB/m | |
| Nonlinear Index | m2/W | |
| Core Area | m2 | |
| Dispersion | s/m2 | |
| Dispersion slope | s/m3 | |
| HNLF2 | Length | 1000 m |
| Attenuation | dB/m | |
| Nonlinear Index | m2/W | |
| Core Area | m2 | |
| Dispersion | s/m2 | |
| Dispersion slope | s/m3 | |
| BPF1 | Center frequency | 192.9 THz |
| Bandwidth | 100 GHz | |
| BPF2 | Center frequency | 193.7 THz |
| Bandwidth | 100 GHz |
| Input OSNR (dB) | 0 | 2 | 4 | 6 | 8 | 10 | 12 | 14 |
|---|---|---|---|---|---|---|---|---|
| Q factor (dB) | 161.12 | 166.36 | 165.27 | 168.26 | 169.27 | 170.08 | 172.26 | 175.16 |
| Input OSNR (dB) | 16 | 18 | 20 | 22 | 24 | 26 | 28 | 30 |
| Q factor (dB) | 177.66 | 180.15 | 182.45 | 184.25 | 185.31 | 185.76 | 185.91 | 185.94 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Ruan, F.; Tang, Y.; Gao, T.; Huang, S. Noise Suppression in Quadrature Phase-Shift-Keying-Oriented All-Optical Matching Systems Using Highly Nonlinear Fiber. Photonics 2025, 12, 516. https://doi.org/10.3390/photonics12050516
Li X, Ruan F, Tang Y, Gao T, Huang S. Noise Suppression in Quadrature Phase-Shift-Keying-Oriented All-Optical Matching Systems Using Highly Nonlinear Fiber. Photonics. 2025; 12(5):516. https://doi.org/10.3390/photonics12050516
Chicago/Turabian StyleLi, Xin, Feiyang Ruan, Ying Tang, Tenglin Gao, and Shanguo Huang. 2025. "Noise Suppression in Quadrature Phase-Shift-Keying-Oriented All-Optical Matching Systems Using Highly Nonlinear Fiber" Photonics 12, no. 5: 516. https://doi.org/10.3390/photonics12050516
APA StyleLi, X., Ruan, F., Tang, Y., Gao, T., & Huang, S. (2025). Noise Suppression in Quadrature Phase-Shift-Keying-Oriented All-Optical Matching Systems Using Highly Nonlinear Fiber. Photonics, 12(5), 516. https://doi.org/10.3390/photonics12050516





