Nonlinear-Optical-Loop-Mirror-Based Mode-Locked Fiber Laser Sensor for Low-Temperature Measurement
Abstract
:1. Introduction
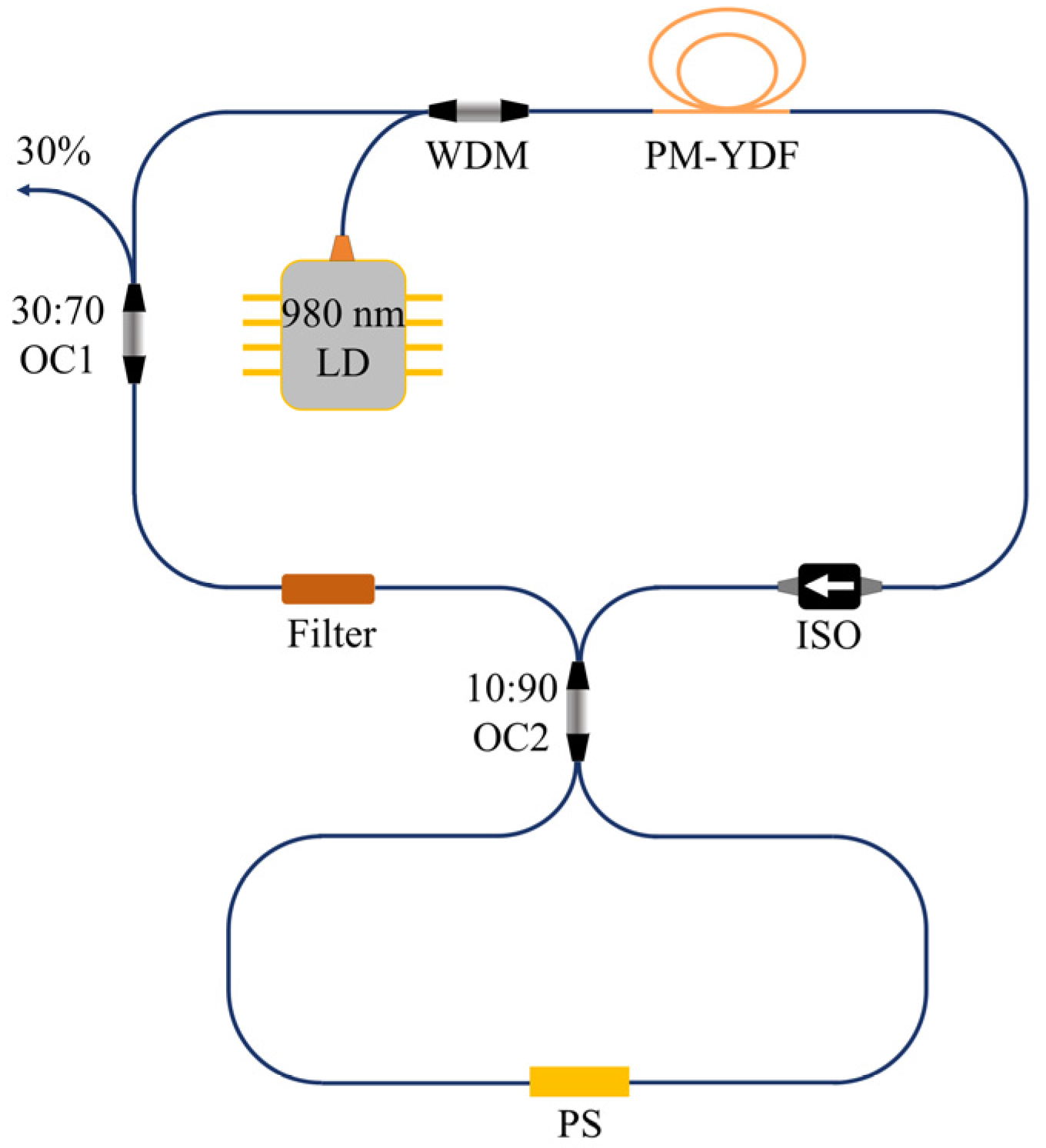
2. Experimental Setup
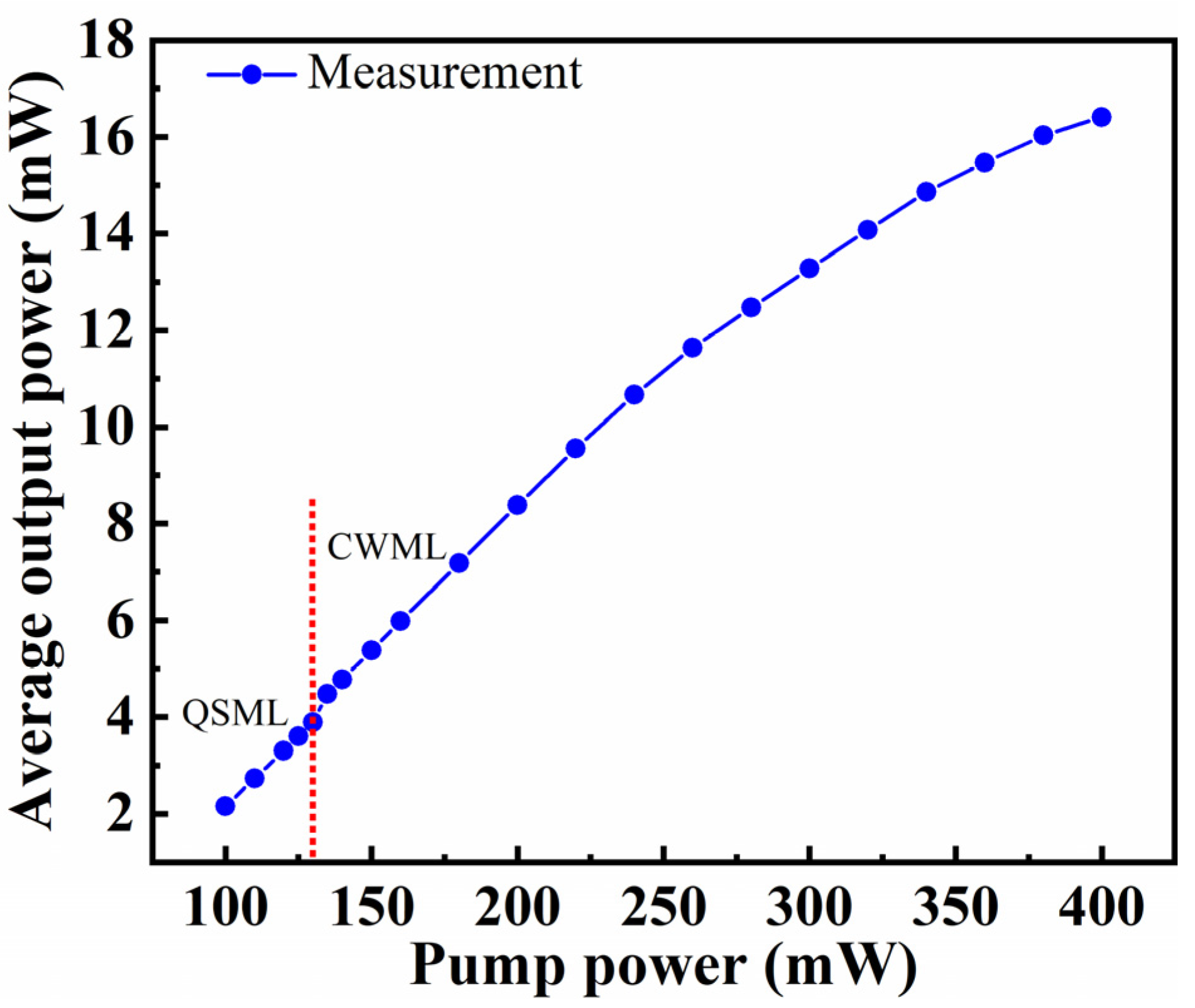
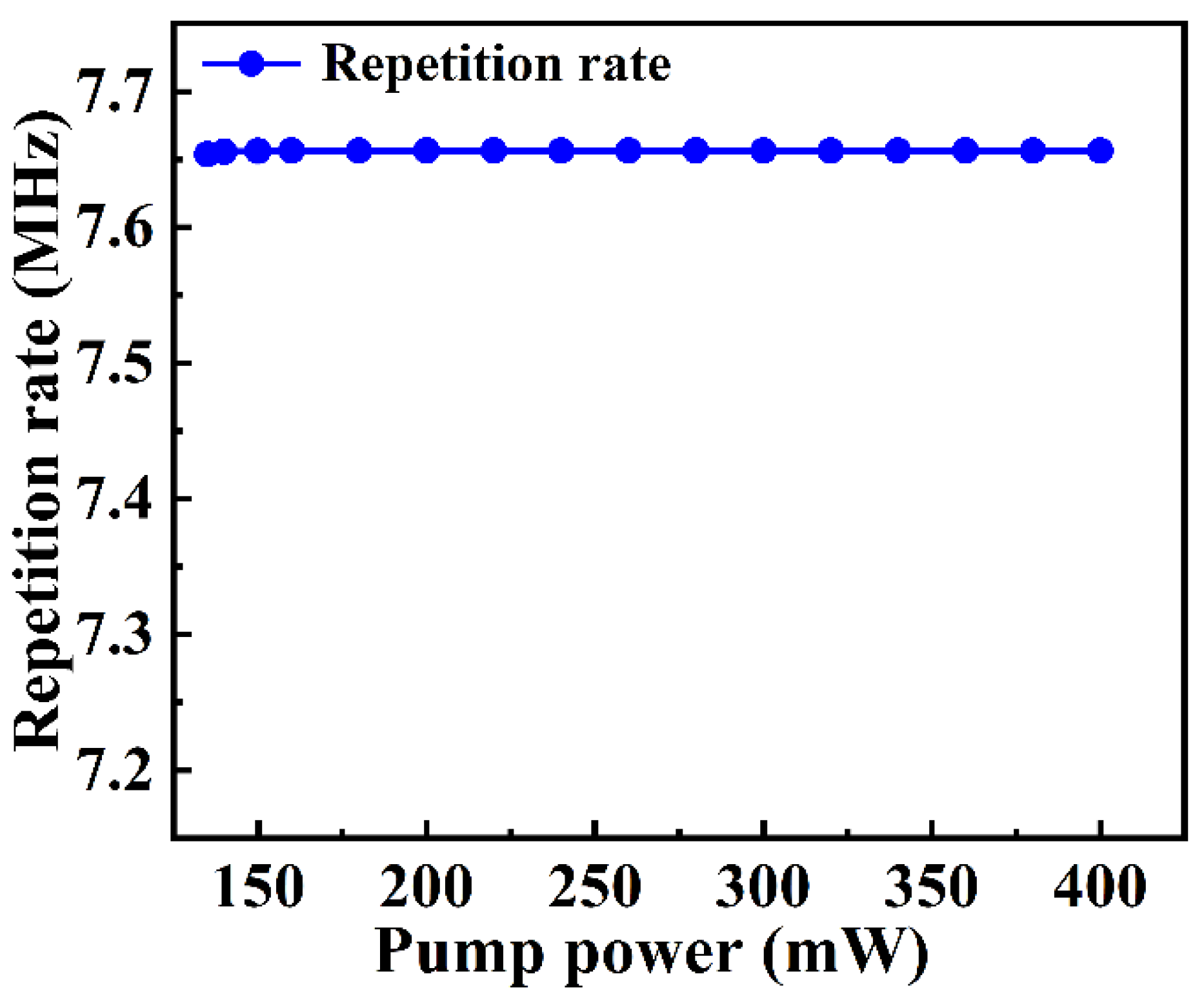
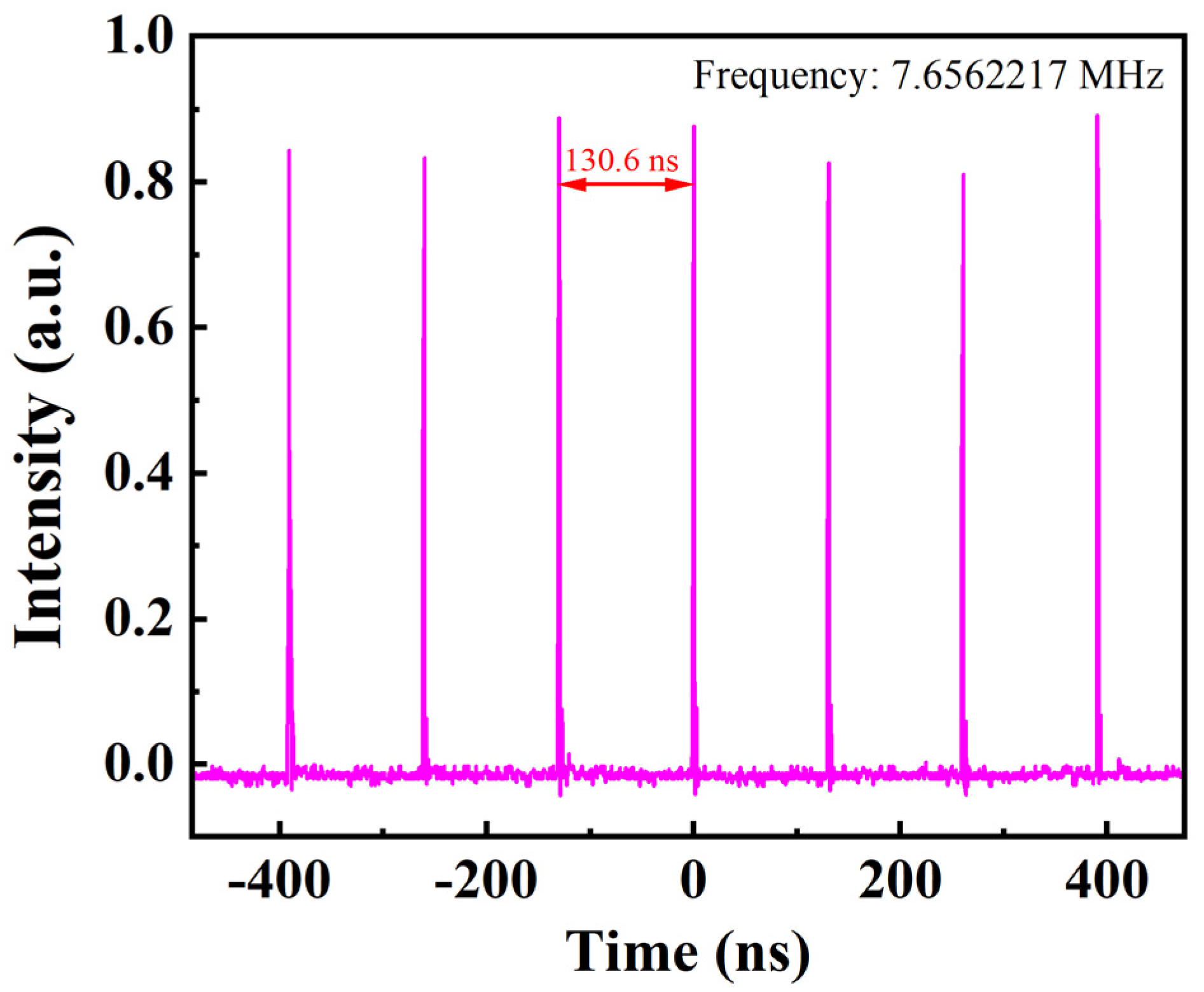
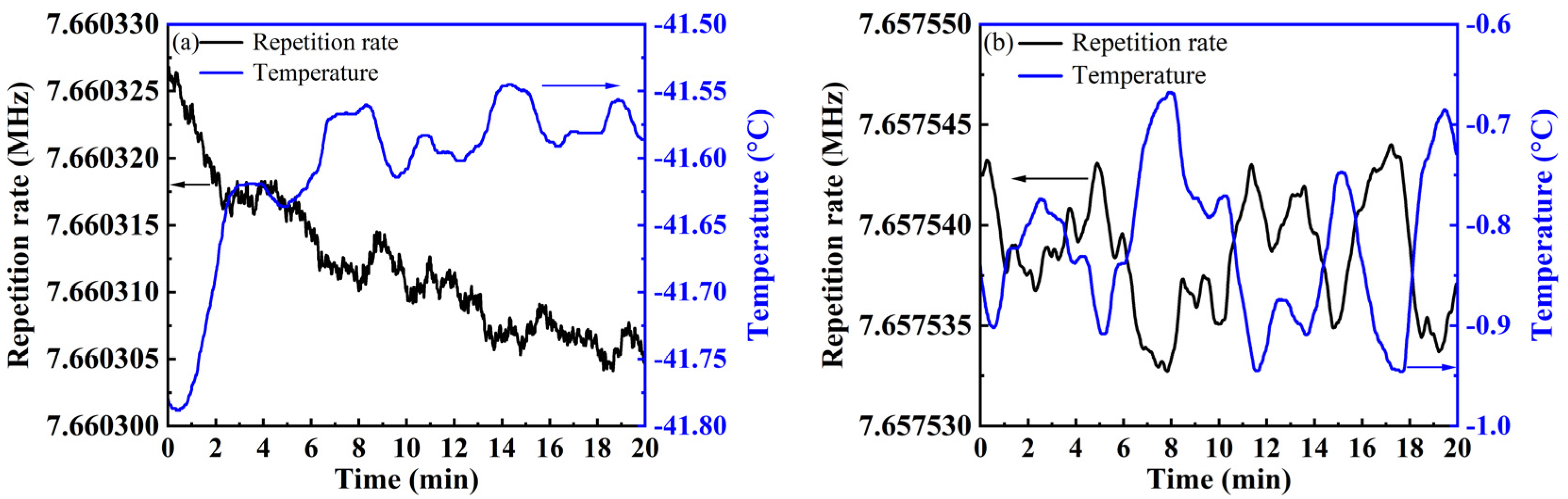
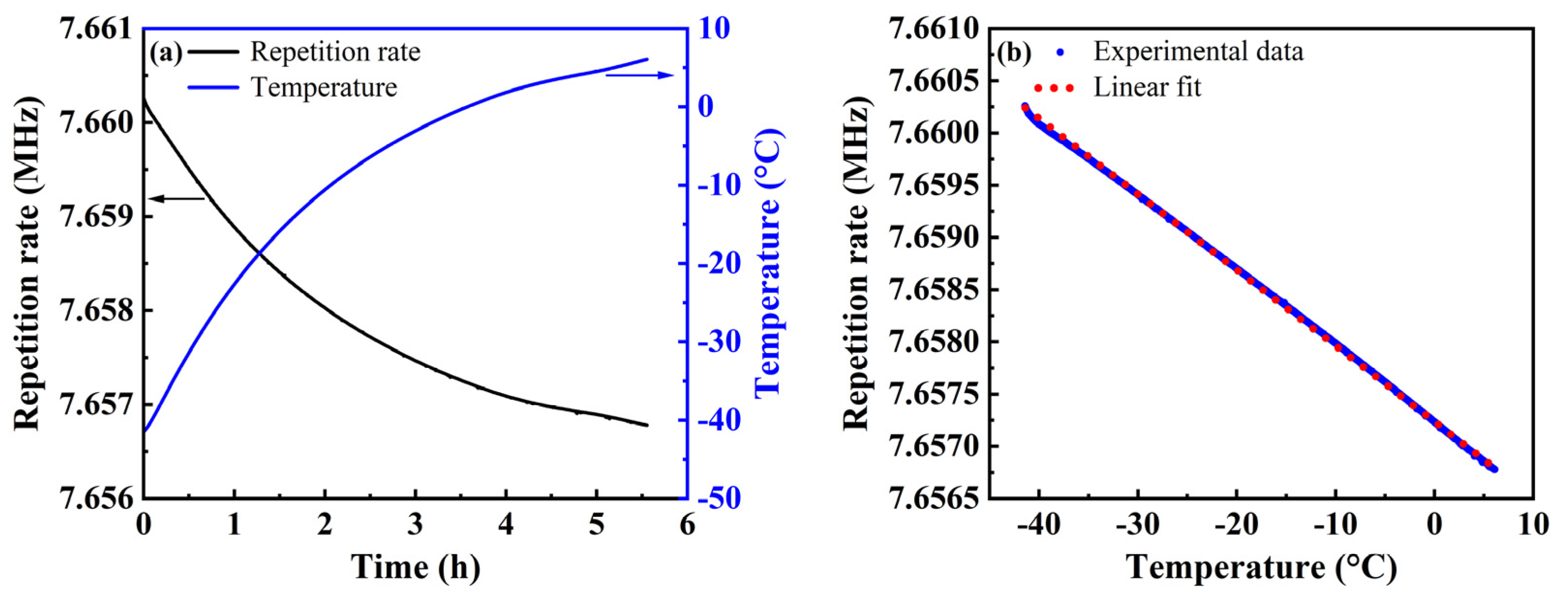
3. Experimental Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shi, Y.T.; Li, X.Y.; Wang, P.; Zhang, Y.; Shi, B.H.; Li, Y.Y. A Review: Salinity and Temperature Measurement Based on Optical Fiber Sensors. Sens. Actuators A Phys. 2024, 380, 116040. [Google Scholar] [CrossRef]
- Tangudu, R.; Sahu, P.K. Review on the Developments and Potential Applications of the Fiber Optic Distributed Temperature Sensing System. IETE Tech. Rev. 2022, 39, 553–567. [Google Scholar] [CrossRef]
- Wang, L.; Wang, Y.J.; Song, S.M.; Li, F. Overview of Fibre Optic Sensing Technology in the Field of Physical Ocean Observation. Front. Phys. 2021, 9, 745487. [Google Scholar] [CrossRef]
- Ma, S.N.; Xu, Y.P.; Pang, Y.X.; Zhao, X.; Li, Y.F.; Qin, Z.G.; Liu, Z.J.; Lu, P.; Bao, X.Y. Optical Fiber Sensors for High-Temperature Monitoring: A Review. Sensors 2022, 22, 5722. [Google Scholar] [CrossRef]
- Paul, K.U.; Dhiman, D.; Muneesh, M.; Yang, Y.W.; Manojit, P. Real-time monitoring of temperature using a pulsed laser-diode-based photoacoustic system. Opt. Lett. 2020, 45, 718–721. [Google Scholar]
- Khan, R.Y.M.; Ullah, R.; Faisal, M. Design and Development of Cost-Effective Fiber Bragg Grating Temperature Sensor Package. Meas. Sci. Technol. 2023, 34, 085122. [Google Scholar] [CrossRef]
- González-Roque, A.A.; Toral-Acosta, D.; Martínez-Ríos, A.; Selvas-Aguilar, R.; Anzueto-Sánchez, G.; Rico-Méndez, M.A.; Guzmán-Ramos, V. Two-Mode Fiber Mach-Zehnder Interferometric Temperature Sensor in the 50 °C–650 °C Range. Opt. Fiber Technol. 2023, 81, 103568. [Google Scholar] [CrossRef]
- Abbas, L.G.; Li, H. Temperature Sensing by Hybrid Interferometer Based on Vernier like Effect. Opt. Fiber Technol. 2021, 64, 102538. [Google Scholar] [CrossRef]
- He, C.; Zhou, C.; Zhou, Q.; Xie, S.Y.; Xiao, M.Z.; Tian, J.J.; Yao, Y. Simultaneous Measurement of Strain and Temperature Using Fabry–Pérot Interferometry and Antiresonant Mechanism in a Hollow-Core Fiber. Chin. Opt. Lett. 2021, 19, 041201. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, M.J.; Wang, P.; Li, L.; Wang, Y.C.; Bao, X.Y. Multiwavelength single-longitudinal-mode Brillouin–erbium fiber laser sensor for temperature measurements with ultrahigh resolution. IEEE Photonics J. 2015, 7, 6802809. [Google Scholar]
- Yin, Z.W.; Gao, L.; Liu, S.C.; Zhang, L.; Wu, F.X.; Chen, L.; Chen, X.F. Fiber Ring Laser Sensor for Temperature Measurement. J. Light. Technol. 2010, 28, 3403–3408. [Google Scholar]
- Huang, L.; Qian, L.; Chen, L.; Gao, L.; Chen, X.F. Multilongitudinal Mode Fiber Laser Sensor for Temperature Measurement. In Proceedings of the 2012 Asia Communications and Photonics Conference (ACP), Guangzhou, China, 7–10 November 2012; p. AF4B.27. [Google Scholar]
- Yu, X.J.; Dong, X.; Chen, X.F.; Zhang, J.T.; Liu, S.C. Polarimetric Multilongitudinal Mode Fiber Laser for Simultaneous Measurement of Strain and Temperature. J. Light. Technol. 2016, 34, 4941–4947. [Google Scholar] [CrossRef]
- Tong, X.X.; Shen, Y.X.; Mao, X.W.; Yu, C.; Guo, Y. Fiber-Optic Temperature Sensor Based on Beat Frequency and Neural Network Algorithm. Opt. Fiber Technol. 2022, 68, 102783. [Google Scholar] [CrossRef]
- Pang, Y.F.; Zhang, X.Q.; Cao, Z.; Xu, L.J. Precise Gas Temperature Measurement Using a Single Dual-Wavelength Mode-Locked Fiber Laser. IEEE Trans. Instrum. Meas. 2024, 73, 7005211. [Google Scholar] [CrossRef]
- Luo, J.; Cai, X.; Wang, H.R.; Fu, H.Y. Temperature Sensing Technique by Using a Microwave Photonics Filter Based on an Actively Mode-locked Fiber Laser. Microw. Opt. Techn. Let. 2021, 63, 2535–2540. [Google Scholar] [CrossRef]
- Kbashi, H.J.; Sheil, B.B.; Perego, A.M. A Mode-Locked Fibre Laser Temperature Independent Strain Sensor Based on Intracavity Pulse Interference. Opt. Laser Eng. 2024, 176, 108040. [Google Scholar] [CrossRef]
- Ma, S.N.; Pang, Y.X.; Ji, Q.; Zhao, X.; Qin, Z.G.; Liu, Z.J.; Xu, Y.P. Relative Humidity Sensing Based on Passively Mode-Locked Fiber Laser with Polyimide-Coated Fiber. IEEE Sens. J. 2024, 24, 6257–6263. [Google Scholar] [CrossRef]
- Luo, J.; Wang, H.R.; Cai, X.; Luo, Z.Q.; Fu, H.Y. Temperature-Sensing Scheme Based on a Passively Mode-Locked Fiber Laser via Beat Frequency Demodulation. Chin. Opt. Lett. 2023, 21, 020603. [Google Scholar] [CrossRef]
- Ma, S.N.; Pang, Y.X.; Ji, Q.; Zhao, X.; Qin, Z.G.; Liu, Z.J.; Lu, P.; Bao, X.Y.; Xu, Y.P. Optomechanically Mode-Locked Laser Sensor for High-Accuracy Temperature Measurement. IEEE Sens. J. 2024, 24, 311–317. [Google Scholar] [CrossRef]
- Liang, X.; Wu, G.; Wang, Q.; Han, Y.; Gao, B.; Tian, X.J.; Guo, S.X.; Liu, L. Dynamics of Solitons in NOLM Based All-Normal Dispersion Yb-Doped Mode-Locked Fiber Laser. Opt. Fiber Technol. 2023, 81, 103507. [Google Scholar] [CrossRef]
- Korobko, D.A.; Fotiadi, A.A.; Zolotovskii, I.O. Mode-Locking Evolution in Ring Fiber Lasers with Tunable Repetition Rate. Opt. Express 2017, 25, 21180. [Google Scholar] [CrossRef] [PubMed]
- Rego, G. Temperature Dependence of the Thermo-Optic Coefficient of SiO2 Glass. Sensors 2023, 23, 6023. [Google Scholar] [CrossRef] [PubMed]
- Rego, G.M. Temperature Dependence of the Thermo-Optic Coefficient of GeO2-Doped Silica Glass Fiber. Sensors 2024, 24, 4857. [Google Scholar] [CrossRef] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dou, X.-A.; Li, L.; Liang, C.; Xu, H.; Ye, Q.; Kong, H.; Bian, J.; Guo, L. Nonlinear-Optical-Loop-Mirror-Based Mode-Locked Fiber Laser Sensor for Low-Temperature Measurement. Photonics 2025, 12, 507. https://doi.org/10.3390/photonics12050507
Dou X-A, Li L, Liang C, Xu H, Ye Q, Kong H, Bian J, Guo L. Nonlinear-Optical-Loop-Mirror-Based Mode-Locked Fiber Laser Sensor for Low-Temperature Measurement. Photonics. 2025; 12(5):507. https://doi.org/10.3390/photonics12050507
Chicago/Turabian StyleDou, Xian-An, Linchan Li, Chang Liang, Haiping Xu, Qing Ye, Hui Kong, Jintian Bian, and Lei Guo. 2025. "Nonlinear-Optical-Loop-Mirror-Based Mode-Locked Fiber Laser Sensor for Low-Temperature Measurement" Photonics 12, no. 5: 507. https://doi.org/10.3390/photonics12050507
APA StyleDou, X.-A., Li, L., Liang, C., Xu, H., Ye, Q., Kong, H., Bian, J., & Guo, L. (2025). Nonlinear-Optical-Loop-Mirror-Based Mode-Locked Fiber Laser Sensor for Low-Temperature Measurement. Photonics, 12(5), 507. https://doi.org/10.3390/photonics12050507




