Near-Field Enhancement in SPASER Nanostructures for High-Efficiency Energy Conversion
Abstract
1. Introduction
2. Methodology
3. Results and Discussion
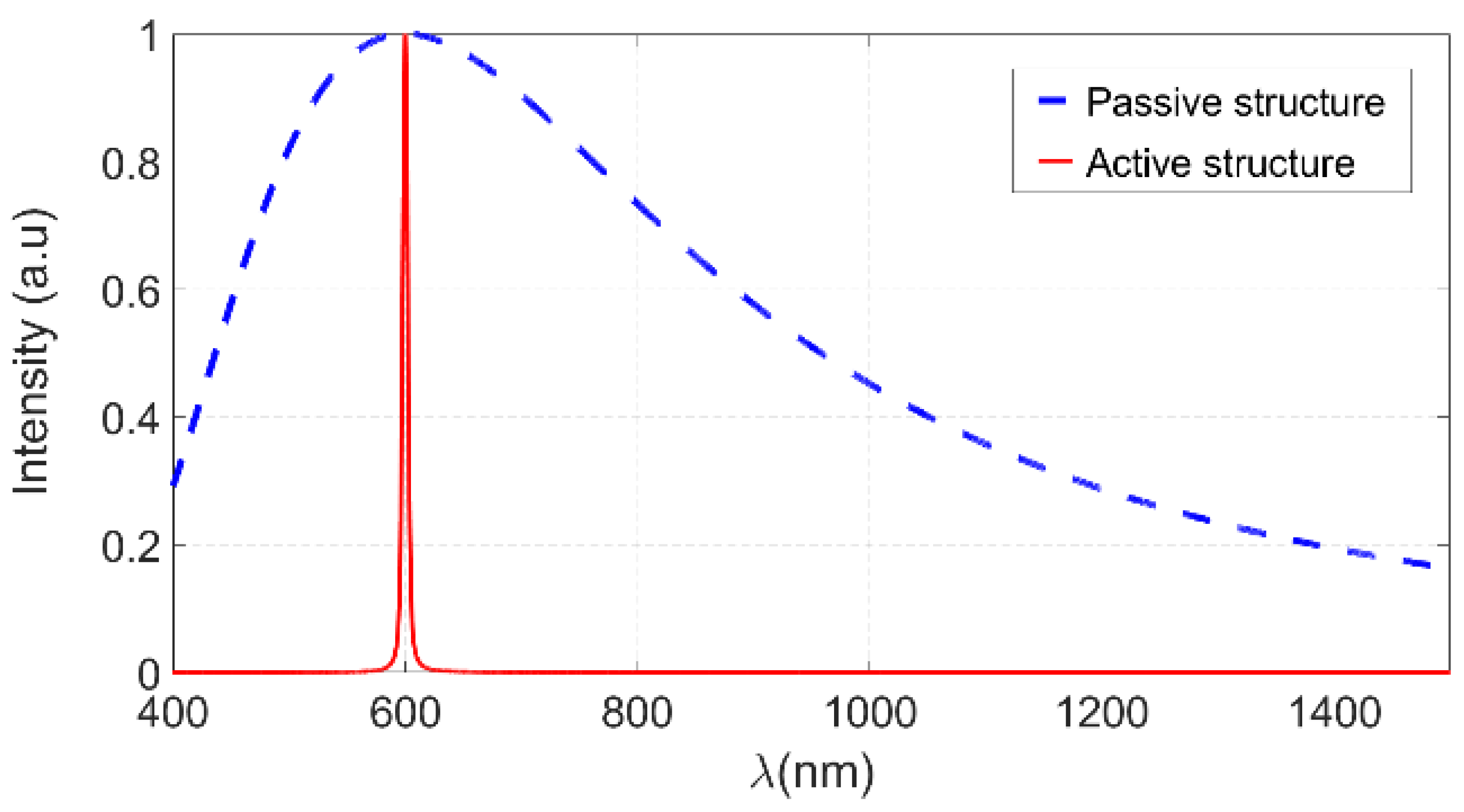
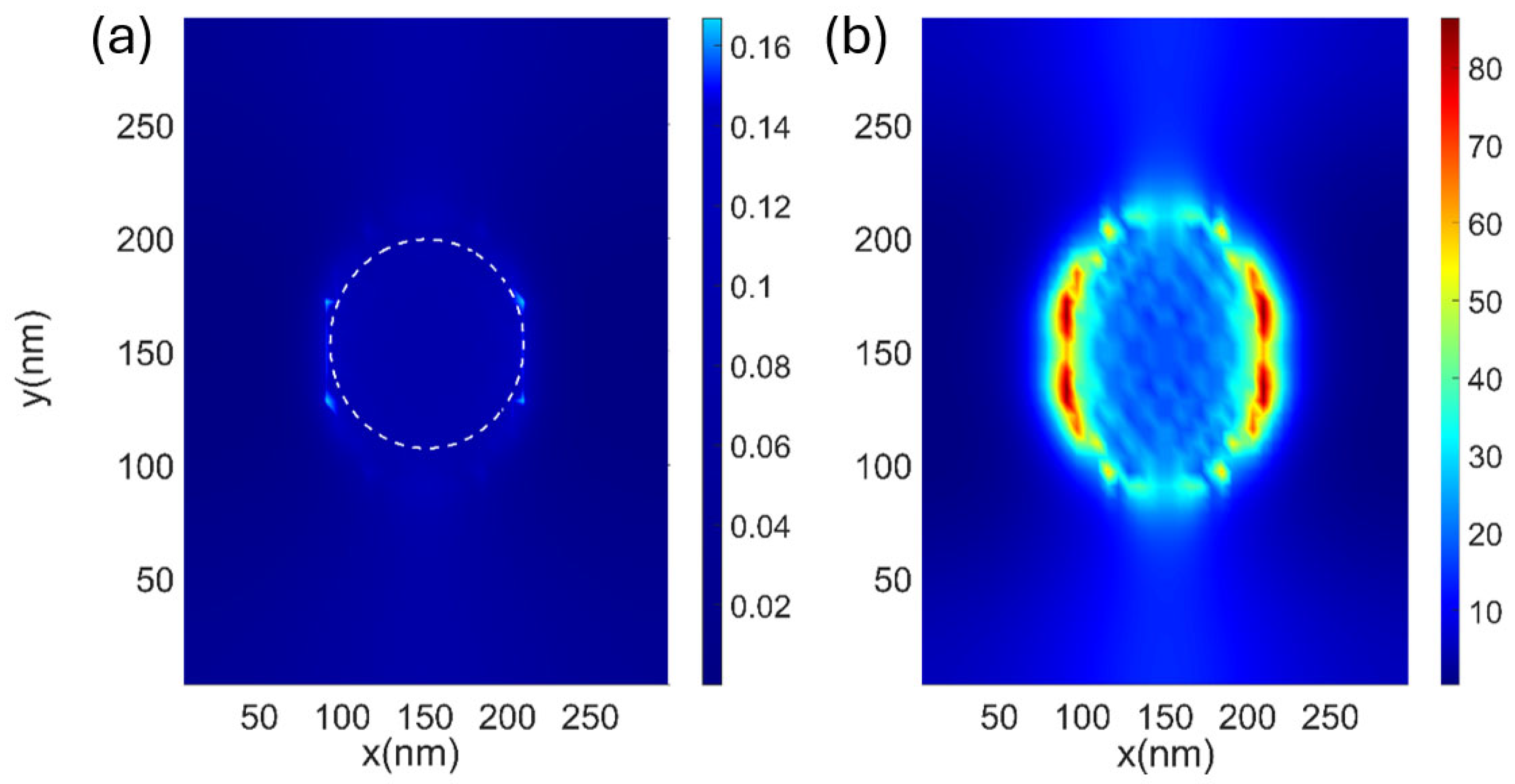
3.1. Enhancement of the Near Field
3.2. Energy Conversion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
References
- Wang, S.; Li, B.; Wang, X.-Y.; Chen, H.-Z.; Wang, Y.-L.; Zhang, X.-W.; Dai, L.; Ma, R.-M. High-Yield Plasmonic Nanolasers with Superior Stability for Sensing in Aqueous Solution. ACS Photonics 2017, 4, 1355–1360. [Google Scholar] [CrossRef]
- Ma, R.-M.; Ota, S.; Li, Y.; Yang, S.; Zhang, X. Explosives Detection in a Lasing Plasmon Nanocavity. Nat. Nanotechnol. 2014, 9, 600–604. [Google Scholar] [CrossRef]
- Galanzha, E.I.; Weingold, R.; Nedosekin, D.A.; Sarimollaoglu, M.; Nolan, J.; Harrington, W.; Kuchyanov, A.S.; Parkhomenko, R.G.; Watanabe, F.; Nima, Z.; et al. Spaser as a Biological Probe. Nat. Commun. 2017, 8, 15528. [Google Scholar] [CrossRef]
- Gao, Z.; Wang, J.; Song, P.; Kang, B.; Xu, J.; Chen, H. Spaser Nanoparticles for Ultranarrow Bandwidth STED Super-Resolution Imaging. Adv. Mater. 2020, 32, 1907233. [Google Scholar] [CrossRef]
- Stockman, M.I. Spasers Explained. Nat. Photonics 2008, 2, 327–329. [Google Scholar] [CrossRef]
- Nezhad, M.P.; Tetz, K.; Fainman, Y. Gain Assisted Propagation of Surface Plasmon Polaritons on Planar Metallic Waveguides. Opt. Express 2004, 12, 4072. [Google Scholar] [CrossRef]
- De Leon, I.; Berini, P. Modeling Surface Plasmon-Polariton Gain in Planar Metallic Structures. Opt. Express 2009, 17, 20191. [Google Scholar] [CrossRef]
- Li, Y.-F.; Feng, J.; Dong, F.-X.; Ding, R.; Zhang, Z.-Y.; Zhang, X.-L.; Chen, Y.; Bi, Y.-G.; Sun, H.-B. Surface Plasmon-Enhanced Amplified Spontaneous Emission from Organic Single Crystals by Integrating Graphene/Copper Nanoparticle Hybrid Nanostructures. Nanoscale 2017, 9, 19353–19359. [Google Scholar] [CrossRef]
- Hill, M.T.; Marell, M.; Leong, E.S.P.; Smalbrugge, B.; Zhu, Y.; Sun, M.; van Veldhoven, P.J.; Geluk, E.J.; Karouta, F.; Oei, Y.-S.; et al. Lasing in Metal-Insulator-Metal Sub-Wavelength Plasmonic Waveguides. Opt. Express 2009, 17, 11107. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, G.; Liu, X.; Qian, F.; Li, Y.; Sum, T.C.; Lieber, C.M.; Xiong, Q. A Room Temperature Low-Threshold Ultraviolet Plasmonic Nanolaser. Nat. Commun. 2014, 5, 4953. [Google Scholar] [CrossRef]
- Wu, Z.; Chen, J.; Mi, Y.; Sui, X.; Zhang, S.; Du, W.; Wang, R.; Shi, J.; Wu, X.; Qiu, X.; et al. All-Inorganic CsPbBr3 Nanowire Based Plasmonic Lasers. Adv. Opt. Mater. 2018, 6, 1800674. [Google Scholar] [CrossRef]
- Wan, M.; Gu, P.; Liu, W.; Chen, Z.; Wang, Z. Low Threshold Spaser Based on Deep-Subwavelength Spherical Hyperbolic Metamaterial Cavities. Appl. Phys. Lett. 2017, 110, 031103. [Google Scholar] [CrossRef]
- Yang, A.; Li, Z.; Knudson, M.P.; Hryn, A.J.; Wang, W.; Aydin, K.; Odom, T.W. Unidirectional Lasing from Template-Stripped Two-Dimensional Plasmonic Crystals. ACS Nano 2015, 9, 11582–11588. [Google Scholar] [CrossRef]
- Dridi, M.; Jaouadi, A.; Colas, F.; Compère, C. Theoretical Study of High Near-Field Enhancement Associated with the Lasing Action in Strongly Coupled Plasmonic Nanocavity Arrays. J. Phys. Chem. C 2021, 125, 749–756. [Google Scholar] [CrossRef]
- Azzam, S.I.; Kildishev, A.V.; Ma, R.-M.; Ning, C.-Z.; Oulton, R.; Shalaev, V.M.; Stockman, M.I.; Xu, J.-L.; Zhang, X. Ten Years of Spasers and Plasmonic Nanolasers. Light Sci. Appl. 2020, 9, 90. [Google Scholar] [CrossRef]
- Siegman, A.E. Lasers; University Science Books: Mill Valley, CA, USA, 1986. [Google Scholar]
- Zhou, W.; Dridi, M.; Suh, J.Y.; Kim, C.H.; Co, D.T.; Wasielewski, M.R.; Schatz, G.C.; Odom, T.W. Lasing Action in Strongly Coupled Plasmonic Nanocavity Arrays. Nat. Nanotechnol. 2013, 8, 506–511. [Google Scholar] [CrossRef]
- Zheludev, N.I.; Prosvirnin, S.L.; Papasimakis, N.; Fedotov, V.A. Lasing Spaser. Nat. Photonics 2008, 2, 351–354. [Google Scholar] [CrossRef]
- Noginov, M.A.; Zhu, G.; Belgrave, A.M.; Bakker, R.; Shalaev, V.M.; Narimanov, E.E.; Stout, S.; Herz, E.; Suteewong, T.; Wiesner, U. Demonstration of a Spaser-Based Nanolaser. Nature 2009, 460, 1110–1112. [Google Scholar] [CrossRef]
- Andrianov, E.S.; Baranov, D.G.; Pukhov, A.A.; Dorofeenko, A.V.; Vinogradov, A.P.; Lisyansky, A.A. Loss Compensation by Spasers in Plasmonic Systems. Opt. Express 2013, 21, 13467. [Google Scholar] [CrossRef]
- Boriskina, S.V.; Cooper, T.A.; Zeng, L.; Ni, G.; Tong, J.K.; Tsurimaki, Y.; Huang, Y.; Meroueh, L.; Mahan, G.; Chen, G. Losses in Plasmonics: From Mitigating Energy Dissipation to Embracing Loss-Enabled Functionalities. Adv. Opt. Photonics 2017, 9, 775. [Google Scholar] [CrossRef]
- Garcia-Blanco, S.M.; Sefunc, M.A.; van Voorden, M.H.; Pollnau, M. Loss Compensation in Metal-Loaded Hybrid Plasmonic Waveguides Using Yb3+ Potassium Double Tungstate Gain Materials. In 2012 14th International Conference on Transparent Optical Networks (ICTON); IEEE: Coventry, UK, 2012; pp. 1–4. [Google Scholar] [CrossRef]
- Gjerding, M.N.; Pandey, M.; Thygesen, K.S. Band Structure Engineered Layered Metals for Low-Loss Plasmonics. Nat. Commun. 2017, 8, 15133. [Google Scholar] [CrossRef] [PubMed]
- Güney, D.Ö.; Koschny, T.; Soukoulis, C.M. Reducing Ohmic Losses in Metamaterials by Geometric Tailoring. Phys. Rev. B 2009, 80, 125129. [Google Scholar] [CrossRef]
- Khurgin, J.B. How to Deal with the Loss in Plasmonics and Metamaterials. Nat. Nanotechnol. 2015, 10, 2–6. [Google Scholar] [CrossRef] [PubMed]
- Izyumskaya, N.; Fomra, D.; Ding, K.; Morkoç, H.; Kinsey, N.; Özgür, Ü.; Avrutin, V. High-Quality Plasmonic Materials TiN and ZnO:Al by Atomic Layer Deposition. Phys. Status Solidi RRL—Rapid Res. Lett. 2021, 15, 2100227. [Google Scholar] [CrossRef]
- Hu, H.; Tian, Y.; Chen, P.; Chu, W. Perspective on Tailored Nanostructure-Dominated SPP Effects for SERS. Adv. Mater. 2023, 36, 2303001. [Google Scholar] [CrossRef]
- Li, X.; Zhu, J.; Wei, B. Hybrid Nanostructures of Metal/Two-Dimensional Nanomaterials for Plasmon-Enhanced Applications. Chem. Soc. Rev. 2016, 45, 3145–3187. [Google Scholar] [CrossRef]
- Dridi, M.; Mahjoub, A.; Jaouadi, A. Plasmonic Lasing in Highly Lossy Nanocylinder Arrays under Optical Pumping. Appl. Phys. B 2023, 129, 171. [Google Scholar] [CrossRef]
- Taflove, A.; Hagness, S.C. Computational Electrodynamics: The Finite-Difference Time-Domain Method, 3rd ed.; Artech House Antennas and Propagation Library; Artech House: Boston, MA, USA, 2005. [Google Scholar]
- Böhringer, K.; Hess, O. A Full-Time-Domain Approach to Spatio-Temporal Dynamics of Semiconductor Lasers. I. Theoretical Formulation. Prog. Quantum Electron. 2008, 32, 159–246. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jaouadi, A.; Mahjoub, A.; Dridi, M. Near-Field Enhancement in SPASER Nanostructures for High-Efficiency Energy Conversion. Photonics 2025, 12, 123. https://doi.org/10.3390/photonics12020123
Jaouadi A, Mahjoub A, Dridi M. Near-Field Enhancement in SPASER Nanostructures for High-Efficiency Energy Conversion. Photonics. 2025; 12(2):123. https://doi.org/10.3390/photonics12020123
Chicago/Turabian StyleJaouadi, Amine, Ahmed Mahjoub, and Montacer Dridi. 2025. "Near-Field Enhancement in SPASER Nanostructures for High-Efficiency Energy Conversion" Photonics 12, no. 2: 123. https://doi.org/10.3390/photonics12020123
APA StyleJaouadi, A., Mahjoub, A., & Dridi, M. (2025). Near-Field Enhancement in SPASER Nanostructures for High-Efficiency Energy Conversion. Photonics, 12(2), 123. https://doi.org/10.3390/photonics12020123




