Equivalent Phase Shift Induced by Longitudinal Temperature Distribution in Pumped DFB Fiber Laser
Abstract
1. Introduction
2. Theoretical Analysis
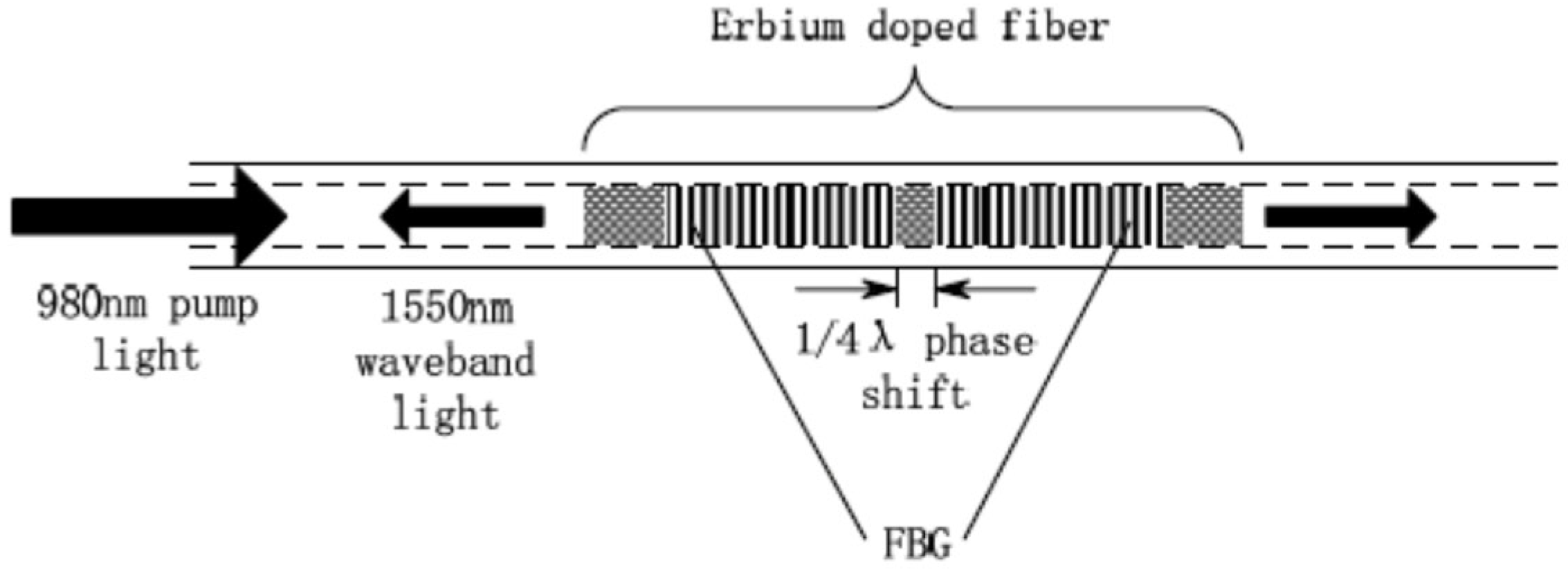
3. Experiment and Results
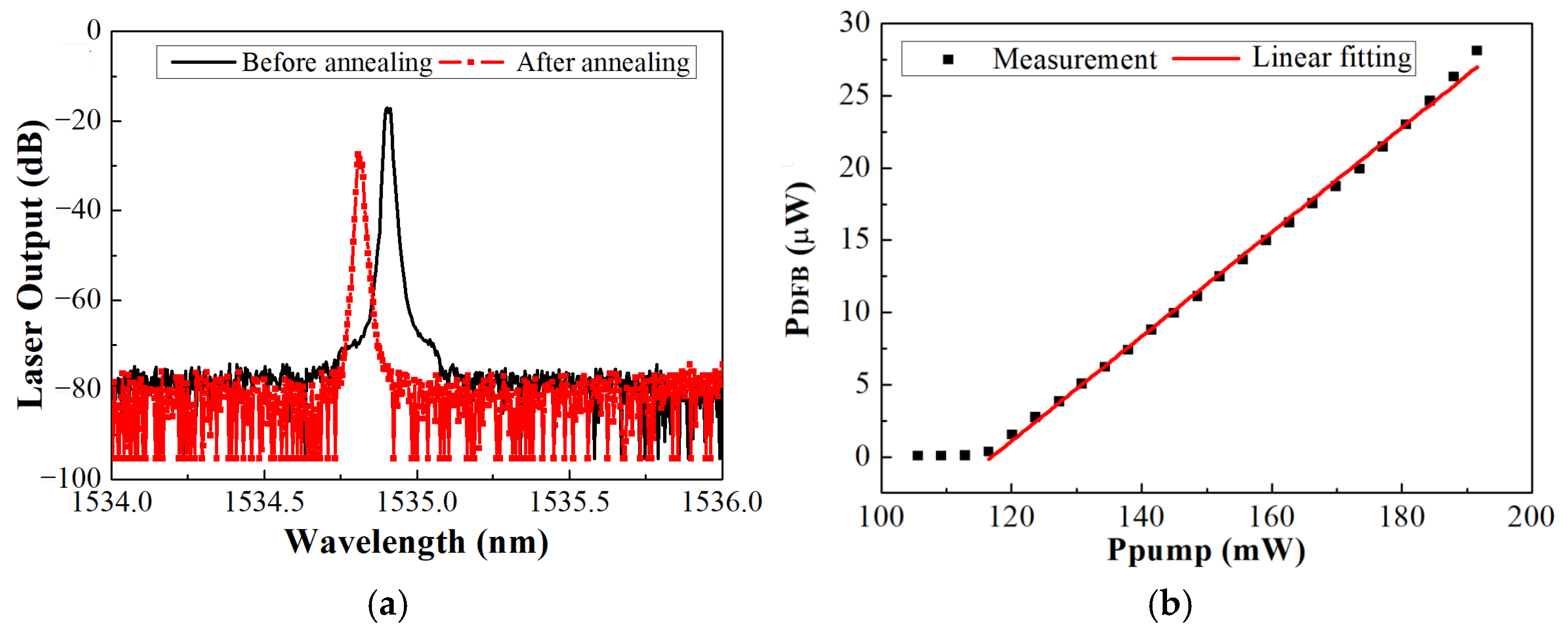
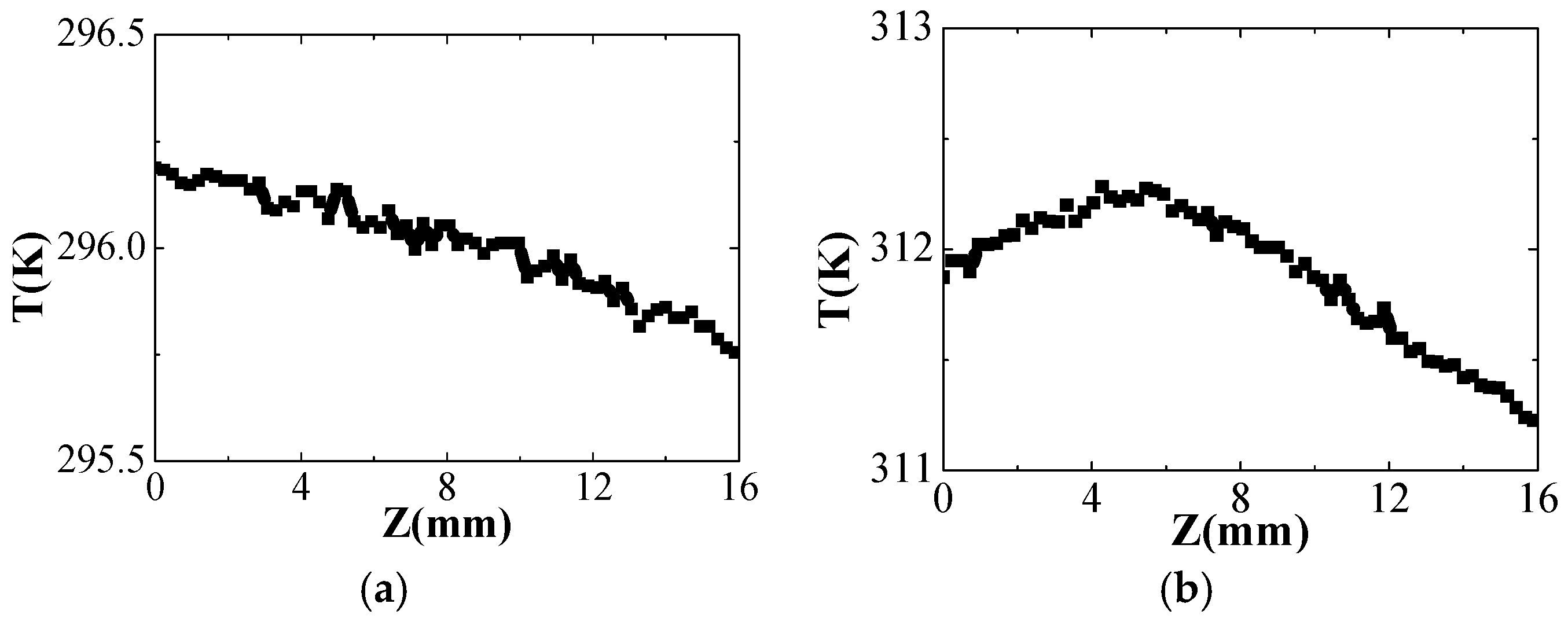
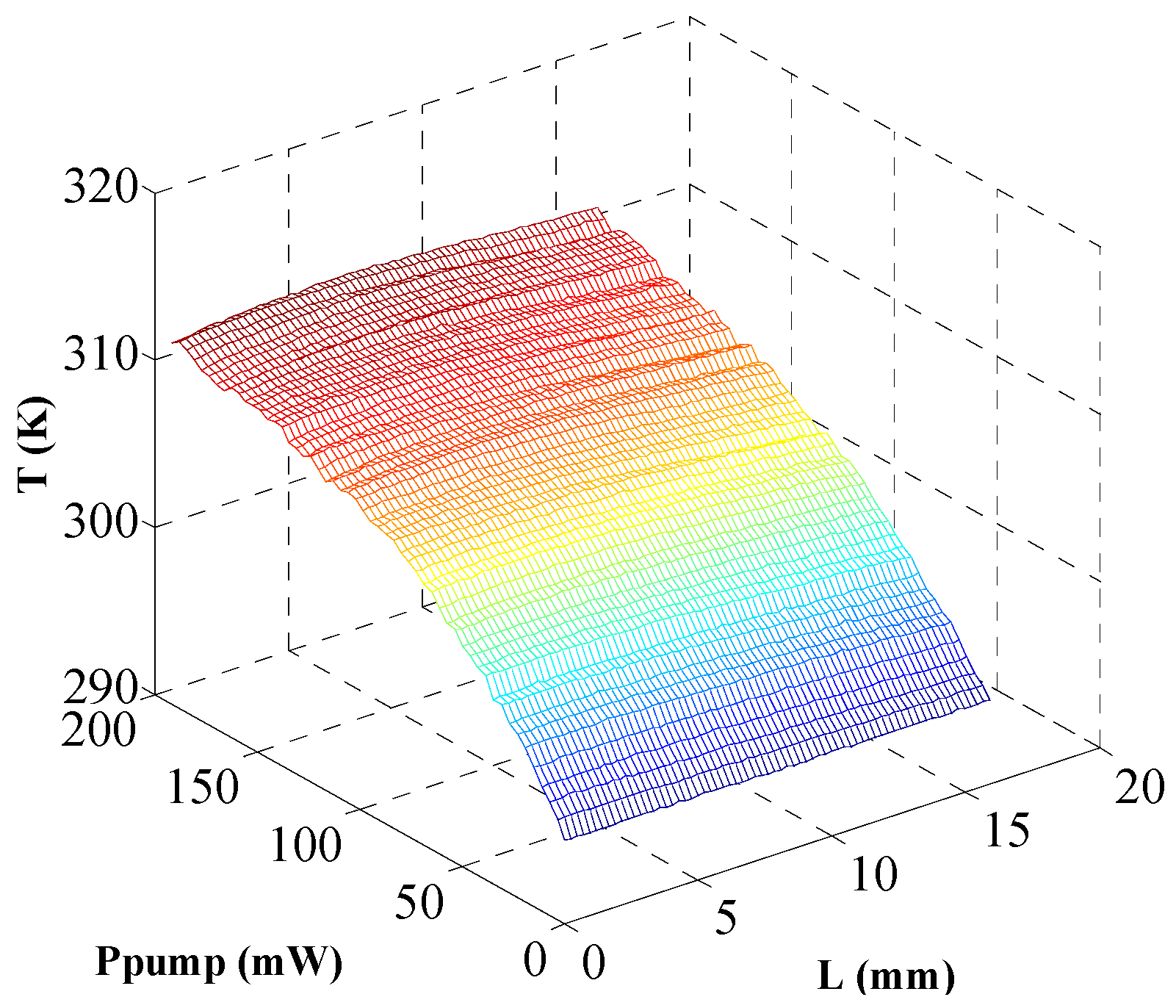
3.1. Pump Characteristics of the DFB Fiber Laser
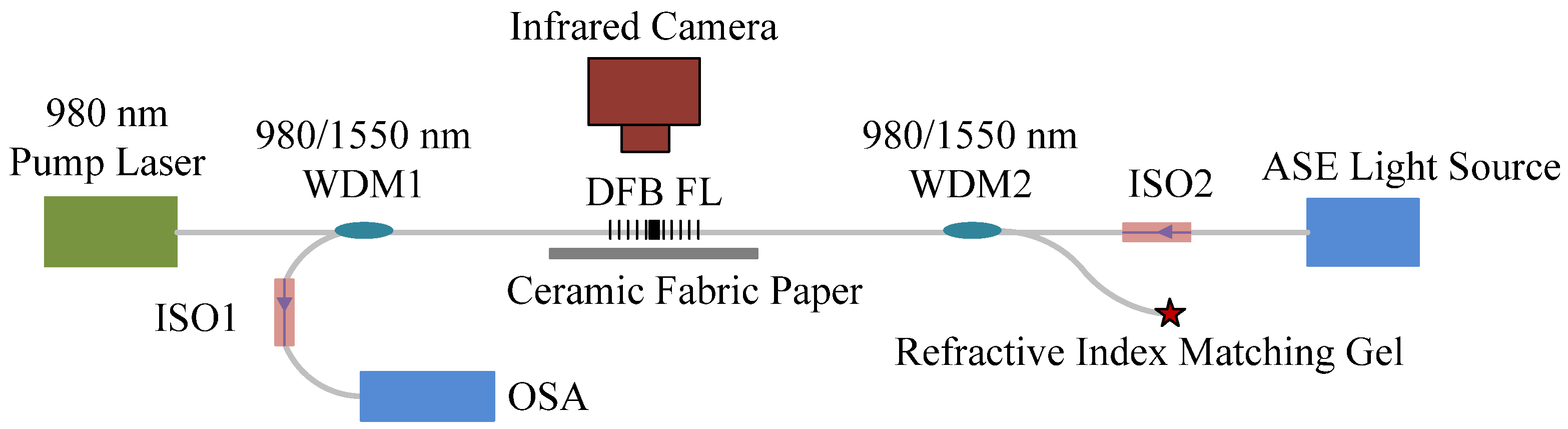
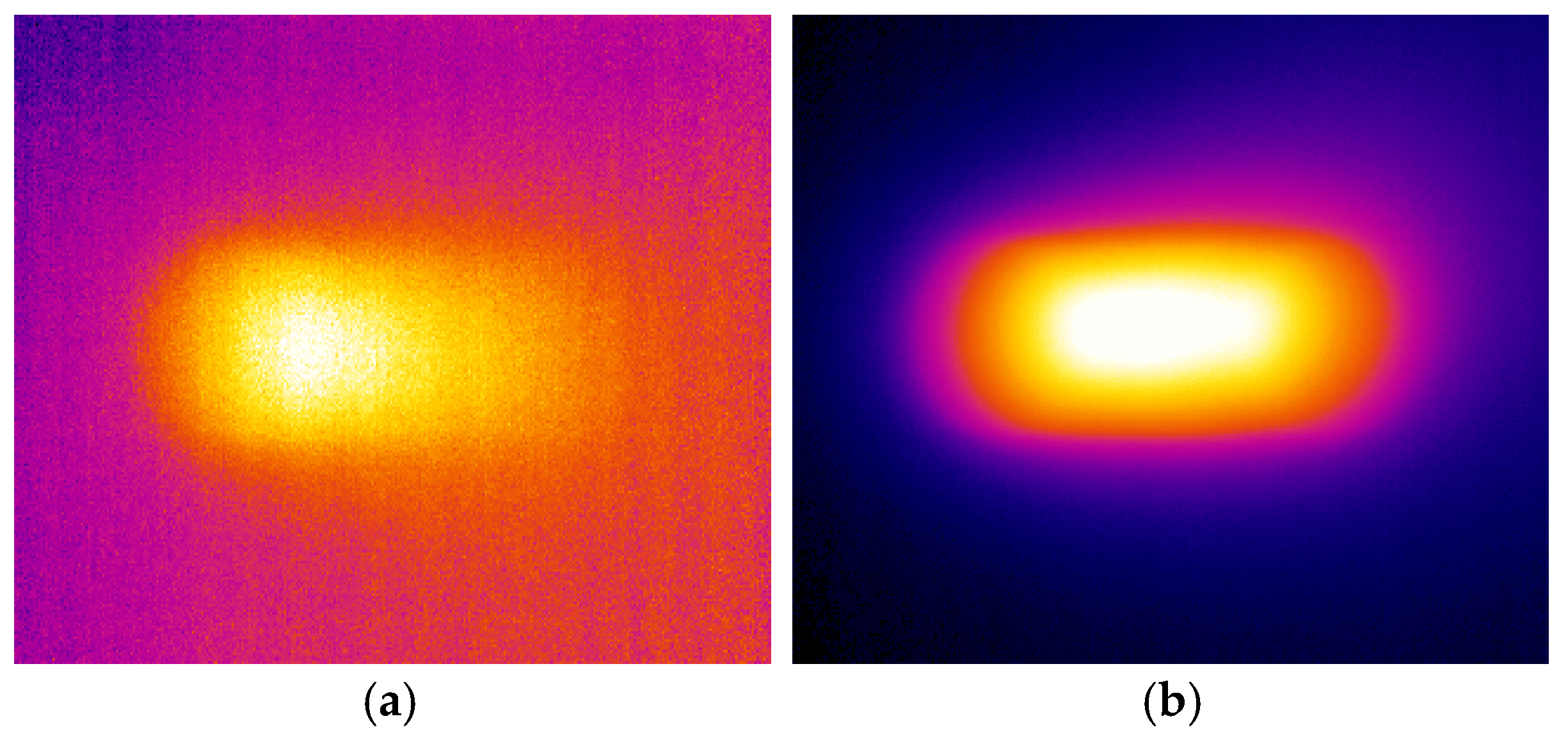
3.2. Temperature Characteristics of the DFB Fiber Laser
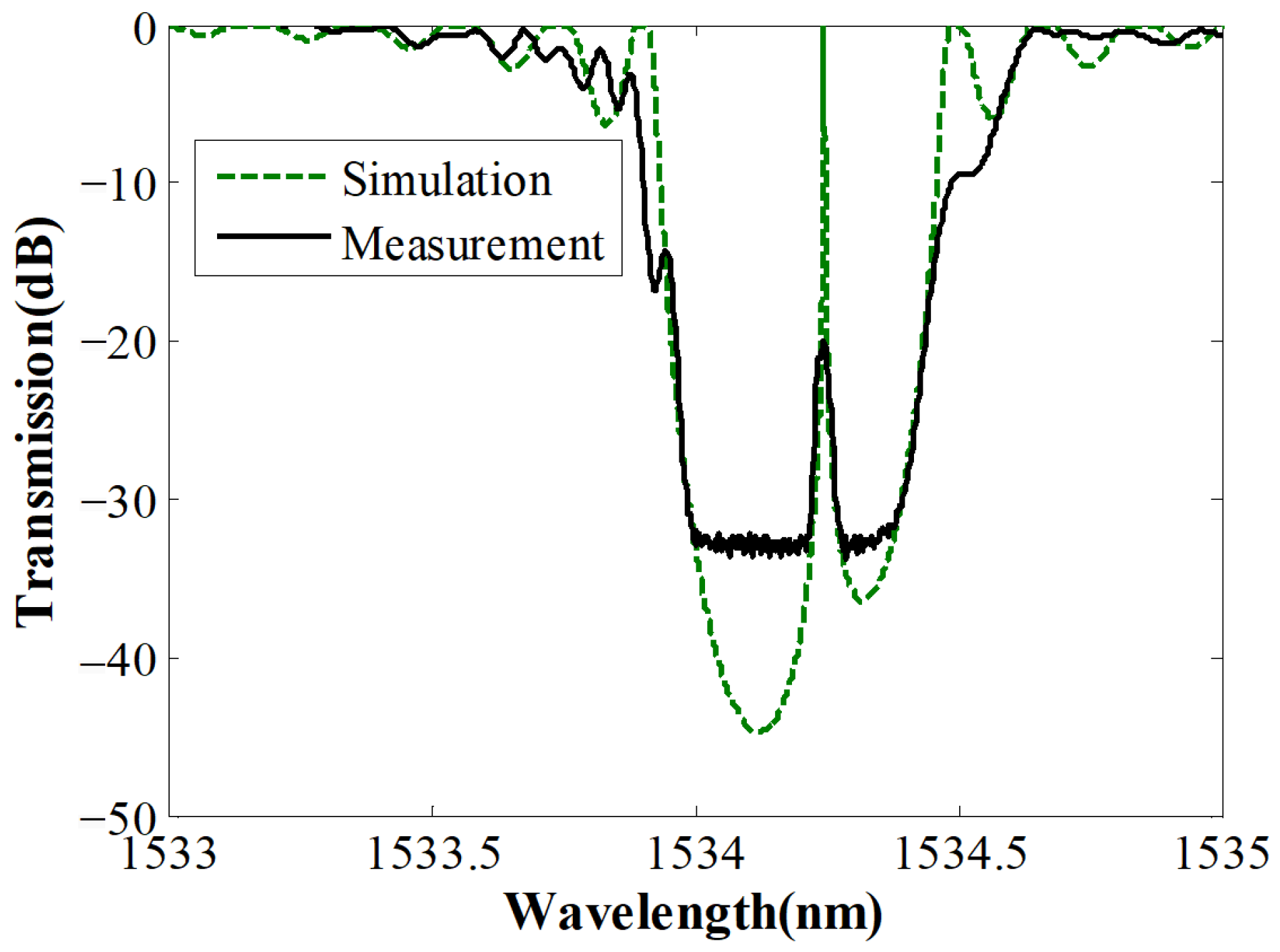
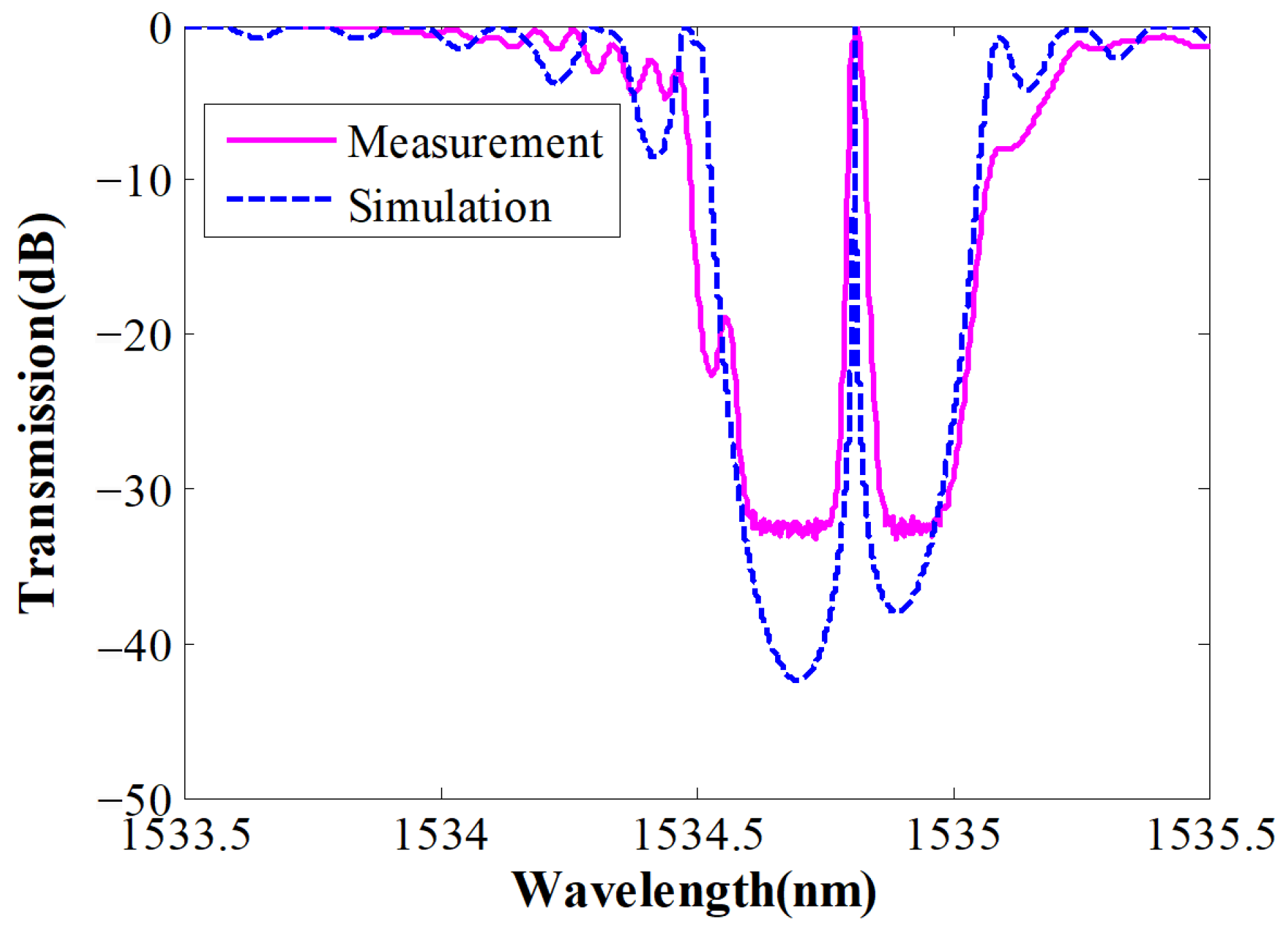
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kringlebotn, J.T.; Archambault, J.-L.; Reekie, L.; Payne, D.N. Er3+:Yb3+-codoped fiber distributed-feedback laser. Opt. Lett. 1994, 19, 2101–2103. [Google Scholar] [CrossRef]
- Hill, D.J.; Nash, P.J.; Jackson, D.A.; Webb, D.J.; O’Neill, S.F.; Bennion, I.; Zhang, L. A fiber laser hydrophone array. In Proceedings of the Fiber Optic Sensor Technology and Applications, Boston, MA, USA, 20–22 September 1999. [Google Scholar]
- Hadeler, O. Application of a DFB fibre laser temperature sensor for characterizing pump induced temperature distributions along another DFB fibre laser. In Proceedings of the Fourteenth International Conference on Optical Fiber Sensors, Venice, Italy, 11–13 October 2000. [Google Scholar]
- Yamada, M.; Sakuda, K. Analysis of almost-periodic distributed feedback slab waveguides via a fundamental matrix approach. Appl. Opt. 1987, 26, 3474–3478. [Google Scholar] [CrossRef]
- Xu, Y.Z.; Tam, H.Y.; Liu, S.Y.; Demokan, M.S. Pump-induced thermal effects in Er-Yb fiber grating DBR lasers. Photon. Technol. Lett. 1998, 10, 1253–1255. [Google Scholar] [CrossRef]
- Qi, L.; Jin, L.; Liang, Y.; Cheng, L.; Guan, B. Efficiency enhancement of optical tuning for Bragg gratings in rare-earth doped fibers. Photon. Technol. Lett. 2014, 26, 1188–1191. [Google Scholar] [CrossRef]
- Man, W.S.; Xu, Y.Z.; Tam, H.Y.; Demokan, M.S. Frequency instability in Er/Yb fiber grating lasers due to heating by nonradiative transitions. Photon. Technol. Lett. 1999, 11, 1390–1392. [Google Scholar] [CrossRef]
- Ying, K.; Liang, H.; Chen, D.; Sun, Y.; Pi, H.Y.; Wei, F.; Yang, F.; Cai, H.W. Ultralow noise DFB fiber laser with self-feedback mechanics utilizing the inherent photothermal effect. Opt. Express 2020, 28, 400083. [Google Scholar] [CrossRef] [PubMed]
- Zhuang, Y.; Zhang, X.L.; Hong, Y.M.; Shang, Y.; Ni, J.S.; Song, Z.Q. Study on the mechanism of fiber-optic hot-wire sensing based on DFB-FL thermally induced chirp effect. Opt. Fiber Technol. 2024, 83, 103678. [Google Scholar] [CrossRef]
- Jiang, S.C.; Wang, J.; Sui, Q.M.; Ye, Q.L.; Wang, L.J. Study of three-component fbg vibration sensor for simultaneous measurement of vibration, temperature, and verticality. J. Sens. 2015, 2015, 382865. [Google Scholar]
- Erdogan, T. Fiber Grating Spectra. J. Lightwave Technol. 1997, 15, 1277–1294. [Google Scholar] [CrossRef]
- Davis, M.K.; Digonnet, M.J.F.; Pantell, R.H. Thermal effects in doped fibers. J. Light. Technol. 1998, 16, 1013–1023. [Google Scholar] [CrossRef]

| Pump Power (mW) | Additional Equivalent Phase Shift | Pump Power (mW) | Additional Equivalent Phase Shift |
|---|---|---|---|
| 0 | 0 | 120.1 | −0.06π |
| 46.13 | −0.01π | 155.6 | −0.08π |
| 83.56 | −0.03π | 191.6 | −0.1π |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, W.; Gu, H.; Zhang, S.; Pang, Y.; Yao, G.; Han, H.; Huang, J. Equivalent Phase Shift Induced by Longitudinal Temperature Distribution in Pumped DFB Fiber Laser. Photonics 2025, 12, 101. https://doi.org/10.3390/photonics12020101
Liu W, Gu H, Zhang S, Pang Y, Yao G, Han H, Huang J. Equivalent Phase Shift Induced by Longitudinal Temperature Distribution in Pumped DFB Fiber Laser. Photonics. 2025; 12(2):101. https://doi.org/10.3390/photonics12020101
Chicago/Turabian StyleLiu, Wen, Hongcan Gu, Su Zhang, Yandong Pang, Gaofei Yao, Hongwei Han, and Junbing Huang. 2025. "Equivalent Phase Shift Induced by Longitudinal Temperature Distribution in Pumped DFB Fiber Laser" Photonics 12, no. 2: 101. https://doi.org/10.3390/photonics12020101
APA StyleLiu, W., Gu, H., Zhang, S., Pang, Y., Yao, G., Han, H., & Huang, J. (2025). Equivalent Phase Shift Induced by Longitudinal Temperature Distribution in Pumped DFB Fiber Laser. Photonics, 12(2), 101. https://doi.org/10.3390/photonics12020101





