Modeling Femtosecond Beam Propagation in Dielectric Hollow-Core Waveguides
Abstract
1. Introduction
2. Model and Methods
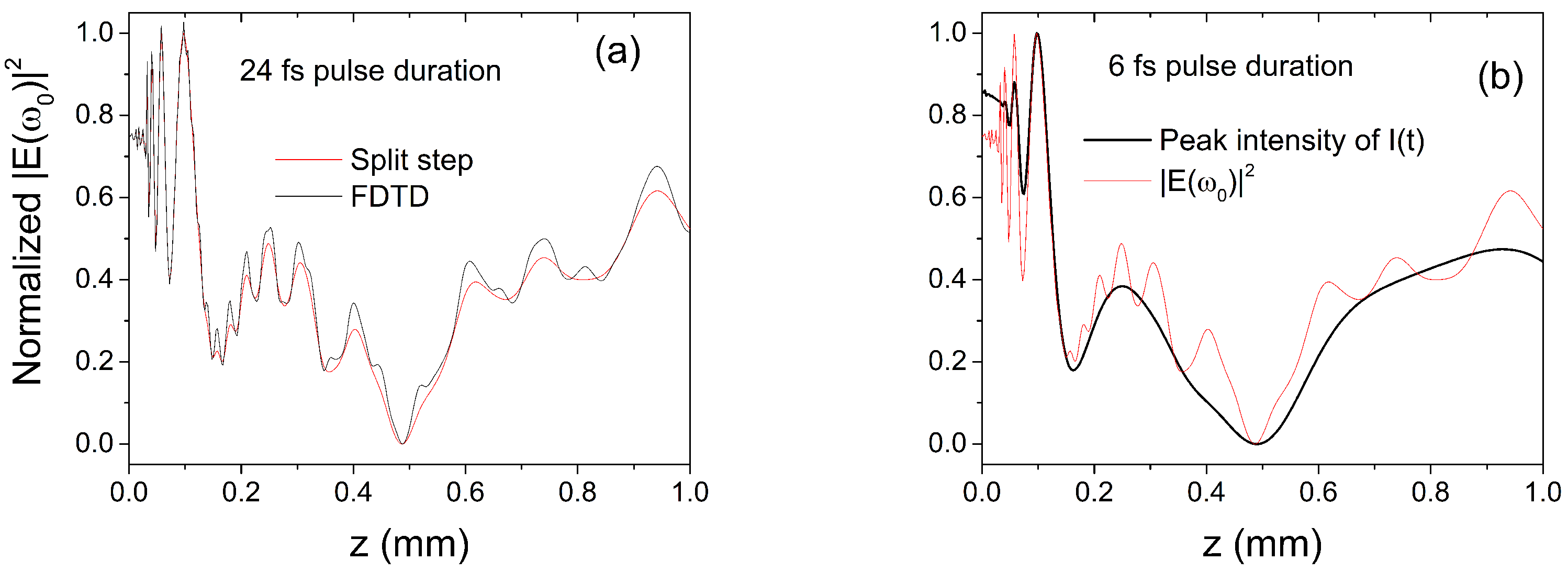
Testing and Tuning the Split-Step Method
3. Results and Discussion
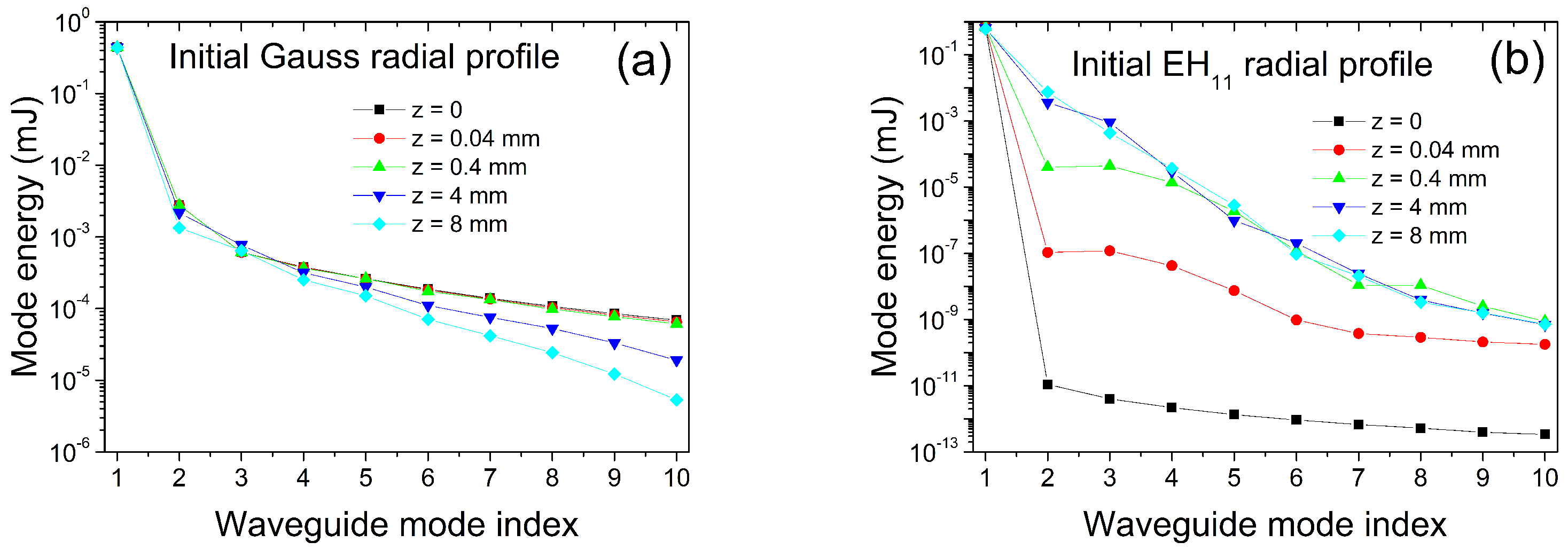
3.1. Influence of Initial Beam Profile in Injection Plane
3.2. Beam Propagation in Modulated HCW
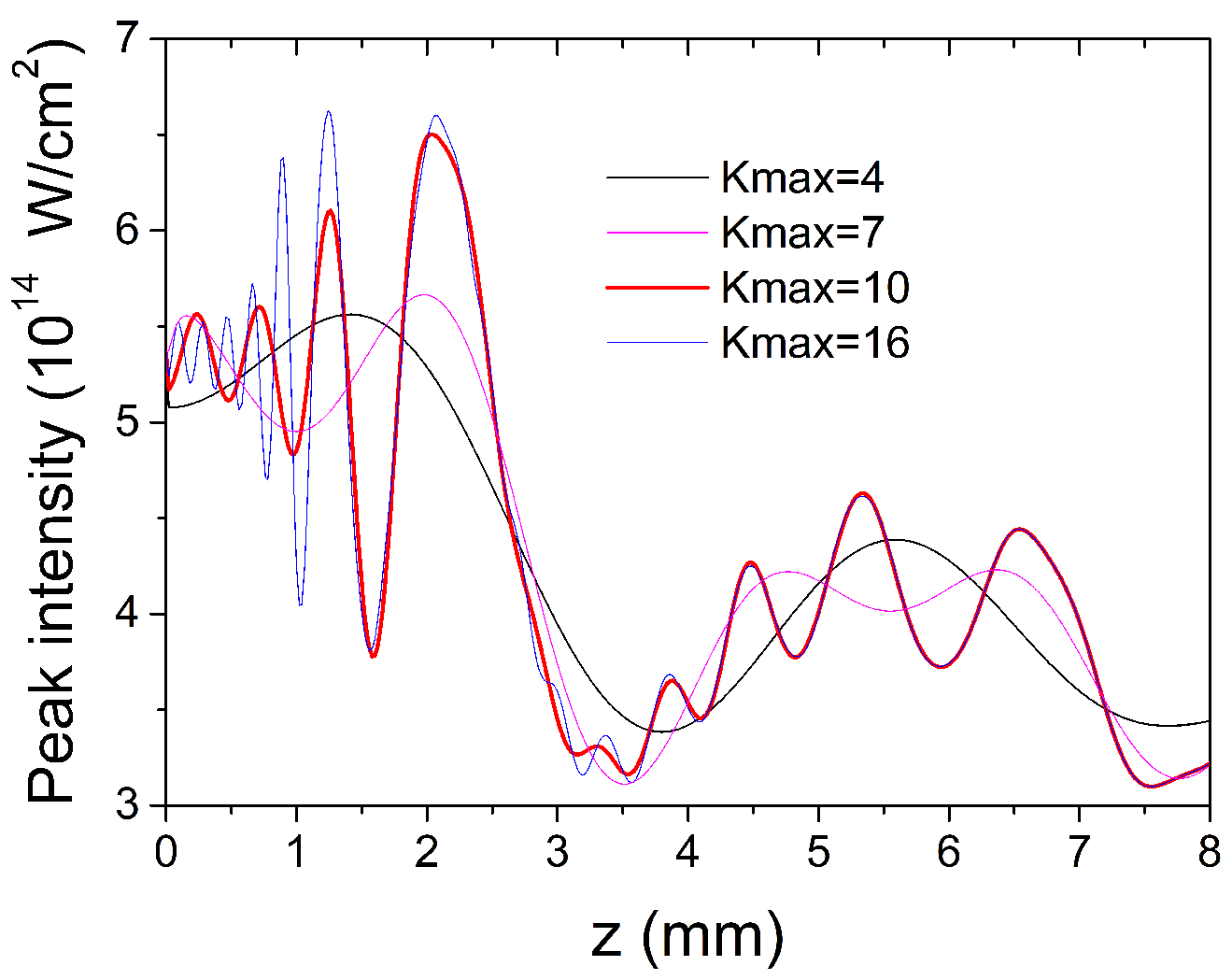
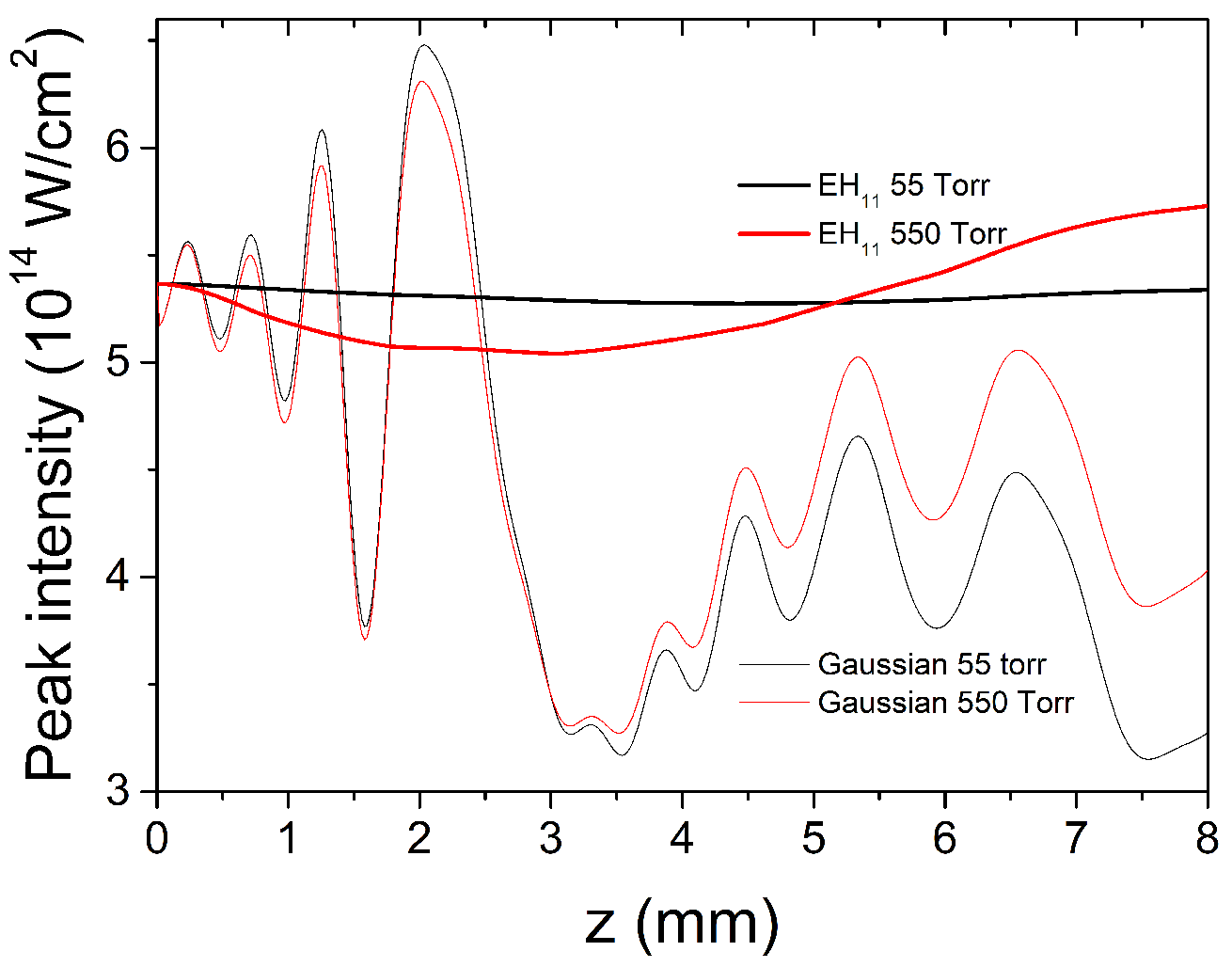
3.3. Scaling Femtosecond Pulse Propagation in HCW
3.4. Scaling High-Order Harmonic Generation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Calegari, F.; Ferrari, F.; Lucchini, M.; Negro, M.; Vozzi, C.; Stagira, S.; Sansone, G.; Nisoli, M. Chapter 8—Principles and Applications of Attosecond Technology. In Advances in Atomic, Molecular, and Optical Physics; Arimondo, E., Berman, P., Lin, C., Eds.; Academic Press: Cambridge, MA, USA, 2011; Volume 60, pp. 371–413. [Google Scholar] [CrossRef]
- The Nobel Prize in Physics 2023. NobelPrize.org. Nobel Prize Outreach. Available online: https://www.nobelprize.org/prizes/physics/2023/summary/ (accessed on 28 February 2024).
- Corkum, P.B. Plasma perspective on strong field multiphoton ionization. Phys. Rev. Lett. 1993, 71, 1994–1997. [Google Scholar] [CrossRef] [PubMed]
- Midorikawa, K. Progress on table-top isolated attosecond light sources. Nat. Photonics 2022, 16, 267–278. [Google Scholar] [CrossRef]
- Horak, P.; Poletti, F. Multimode Nonlinear Fibre Optics: Theory and Applications. In Recent Progress in Optical Fiber Research; Yasin, M., Harun, S.W., Arof, H., Eds.; IntechOpen: Rijeka, Croatia, 2012; Chapter 1. [Google Scholar] [CrossRef]
- Ciriolo, A.G.; Martínez Vázquez, R.; Crippa, G.; Devetta, M.; Faccialà, D.; Barbato, P.; Frassetto, F.; Negro, M.; Bariselli, F.; Poletto, L.; et al. Microfluidic devices for quasi-phase-matching in high-order harmonic generation. APL Photonics 2022, 7, 110801. [Google Scholar] [CrossRef]
- Li, B.; Wang, K.; Tang, X.; Zhang, C.; Wang, B.; Jin, C. Elimination of chromatic aberration in high-order harmonic generation using a plasma-induced flat-top beam in a gas medium. Phys. Rev. A 2024, 110, 043511. [Google Scholar] [CrossRef]
- Major, B.; Kovács, K.; Tosa, V.; Rudawski, P.; L’Huillier, A.; Varjú, K. Effect of plasma-core-induced self-guiding on phase matching of high-order harmonic generation in gases. J. Opt. Soc. Am. B 2019, 36, 1594–1601. [Google Scholar] [CrossRef]
- Popmintchev, T.; Chen, M.C.; Bahabad, A.; Gerrity, M.; Sidorenko, P.; Cohen, O.; Christov, I.P.; Murnane, M.M.; Kapteyn, H.C. Phase matching of high harmonic generation in the soft and hard X-ray regions of the spectrum. Proc. Natl. Acad. Sci. USA 2009, 106, 10516–10521. [Google Scholar] [CrossRef]
- Popmintchev, T.; Chen, M.C.; Popmintchev, D.; Arpin, P.; Brown, S.; Ališauskas, S.; Andriukaitis, G.; Balčiunas, T.; Mücke, O.D.; Pugzlys, A.; et al. Bright Coherent Ultrahigh Harmonics in the keV X-ray Regime from Mid-Infrared Femtosecond Lasers. Science 2012, 336, 1287–1291. [Google Scholar] [CrossRef]
- Ran, Q.; Li, H.; Chang, W.; Wang, Q. Self-Compression of High Energy Ultrashort Laser Pulses. Laser & Photonics Reviews 2024, 18, 2300595. [Google Scholar] [CrossRef]
- Fan, G.; Balčiūnas, T.; Kanai, T.; Flöry, T.; Andriukaitis, G.; Schmidt, B.E.; Légaré, F.; Baltuška, A. Hollow-core-waveguide compression of multi-millijoule CEP-stable 3.2 μm pulses. Optica 2016, 3, 1308–1311. [Google Scholar] [CrossRef]
- Tamas Nagy, P.S.; Veisz, L. High-energy few-cycle pulses: Post-compression techniques. Adv. Phys. X 2021, 6, 1845795. [Google Scholar] [CrossRef]
- Nagar, G.C.; Shim, B. Study of wavelength-dependent pulse self-compression for high intensity pulse propagation in gas-filled capillaries. Opt. Express 2021, 29, 27416–27433. [Google Scholar] [CrossRef]
- Tosa, V.; Kovács, K.; Major, B.; Balogh, E.; Varjú, K. Propagation effects in highly ionised gas media. Quantum Electron. 2016, 46, 321. [Google Scholar] [CrossRef]
- Nurhuda, M.; Suda, A.; Midorikawa, K.; Hatayama, M.; Nagasaka, K. Propagation dynamics of femtosecond laser pulses in a hollow fiber filled with argon: Constant gas pressure versus differential gas pressure. J. Opt. Soc. Am. B 2003, 20, 2002–2011. [Google Scholar] [CrossRef]
- Marcatili, E.A.J.; Schmeltzer, R.A. Hollow metallic and dielectric waveguides for long distance optical transmission and lasers. Bell Syst. Tech. J. 1964, 43, 1783–1809. [Google Scholar] [CrossRef]
- Froud, C.A.; Chapman, R.T.; Rogers, E.T.F.; Praeger, M.; Mills, B.; Grant-Jacob, J.; Butcher, T.J.; Stebbings, S.L.; de Paula, A.M.; Frey, J.G.; et al. Spatially resolved Ar* and Ar+* imaging as a diagnostic for capillary-based high harmonic generation. J. Opt. A Pure Appl. Opt. 2009, 11, 054011. [Google Scholar] [CrossRef]
- Tosa, V.; Ciriolo, A.G.; Vazquez, R.M.; Vozzi, C.; Stagira, S. Modeling femtosecond pulse propagation and high harmonics generation in hollow core fibers. EPJ Web Conf. 2021, 255, 11005. [Google Scholar] [CrossRef]
- Poletti, F.; Horak, P. Description of ultrashort pulse propagation in multimode optical fibers. J. Opt. Soc. Am. B 2008, 25, 1645–1654. [Google Scholar] [CrossRef]
- Ansys Lumerical Inc. 2022. Available online: https://www.ansys.com/products/optics (accessed on 1 November 2024).
- Li, B.; Wang, K.; Tang, X.; Wang, B.; Lin, C.D.; Jin, C. Enhancement of harmonic generation by an intense driving laser with high-order waveguide modes in a high-pressure gas-filled hollow waveguide. Opt. Express 2024, 32, 48972–48986. [Google Scholar] [CrossRef]
- Dromey, B.; Zepf, M.; Landreman, M.; Hooker, S.M. Quasi-phasematching of harmonic generation via multimode beating in waveguides. Opt. Express 2007, 15, 7894–7900. [Google Scholar] [CrossRef]
- Zepf, M.; Dromey, B.; Landreman, M.; Foster, P.; Hooker, S.M. Bright Quasi-Phase-Matched Soft-X-Ray Harmonic Radiation from Argon Ions. Phys. Rev. Lett. 2007, 99, 143901. [Google Scholar] [CrossRef]
- Jin, C.; Hong, K.H.; Lin, C.D. Macroscopic scaling of high-order harmonics generated by two-color optimized waveforms in a hollow waveguide. Phys. Rev. A 2017, 96, 013422. [Google Scholar] [CrossRef]
- Nurhuda, M.; Suda, A.; Hatayama, M.; Nagasaka, K.; Midorikawa, K. Propagation dynamics of femtosecond laser pulses in argon. Phys. Rev. A 2002, 66, 023811. [Google Scholar] [CrossRef]
- Chapman, R.T.; Butcher, T.J.; Horak, P.; Poletti, F.; Frey, J.G.; Brocklesby, W.S. Modal effects on pump-pulse propagation in an Ar-filled capillary. Opt. Express 2010, 18, 13279–13284. [Google Scholar] [CrossRef]
- Gherman, A.M.M.; Tóth, I.; Ciriolo, A.G.; Martínez Vázquez, R.; Nistico, A.; Stagira, S.; Toşa, V. Modeling generation of harmonics in the water window region in hollow core waveguides by mid-infrared femtosecond pulses. J. Appl. Phys. 2024, 136, 043102. [Google Scholar] [CrossRef]
- Heyl, C.M.; Coudert-Alteirac, H.; Miranda, M.; Louisy, M.; Kovacs, K.; Tosa, V.; Balogh, E.; Varjú, K.; L’Huillier, A.; Couairon, A.; et al. Scale-invariant nonlinear optics in gases. Optica 2016, 3, 75–81. [Google Scholar] [CrossRef]
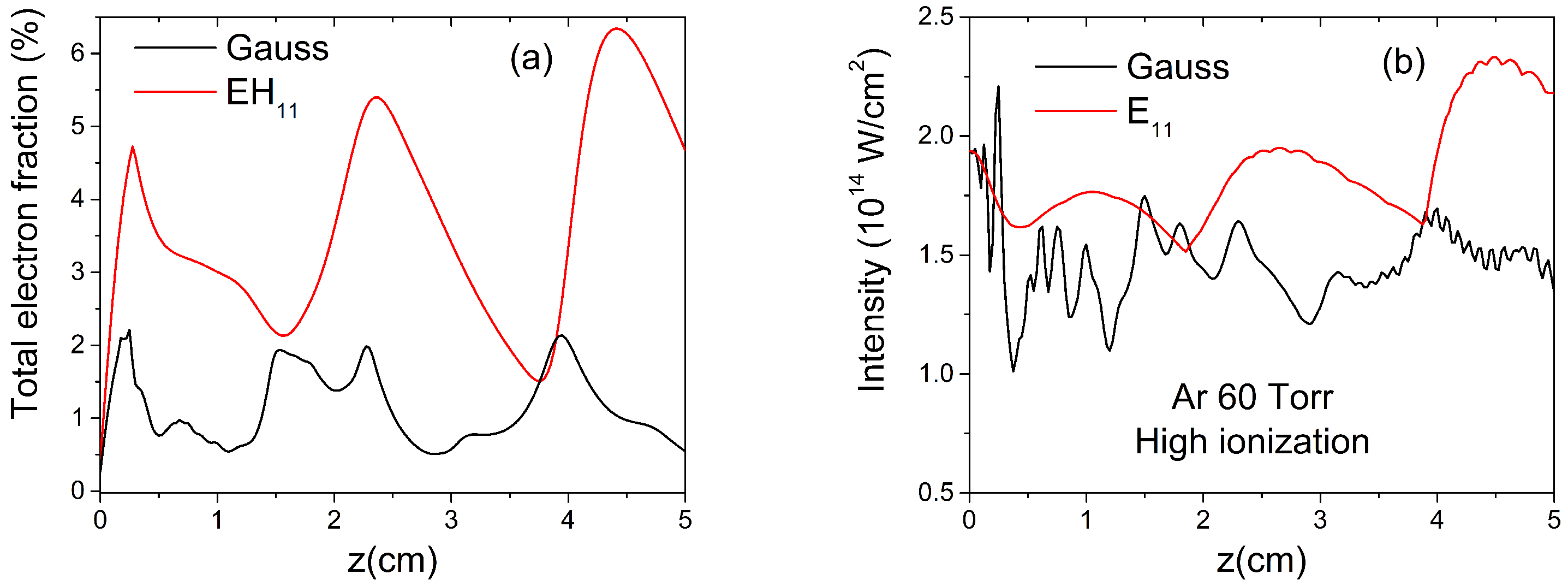
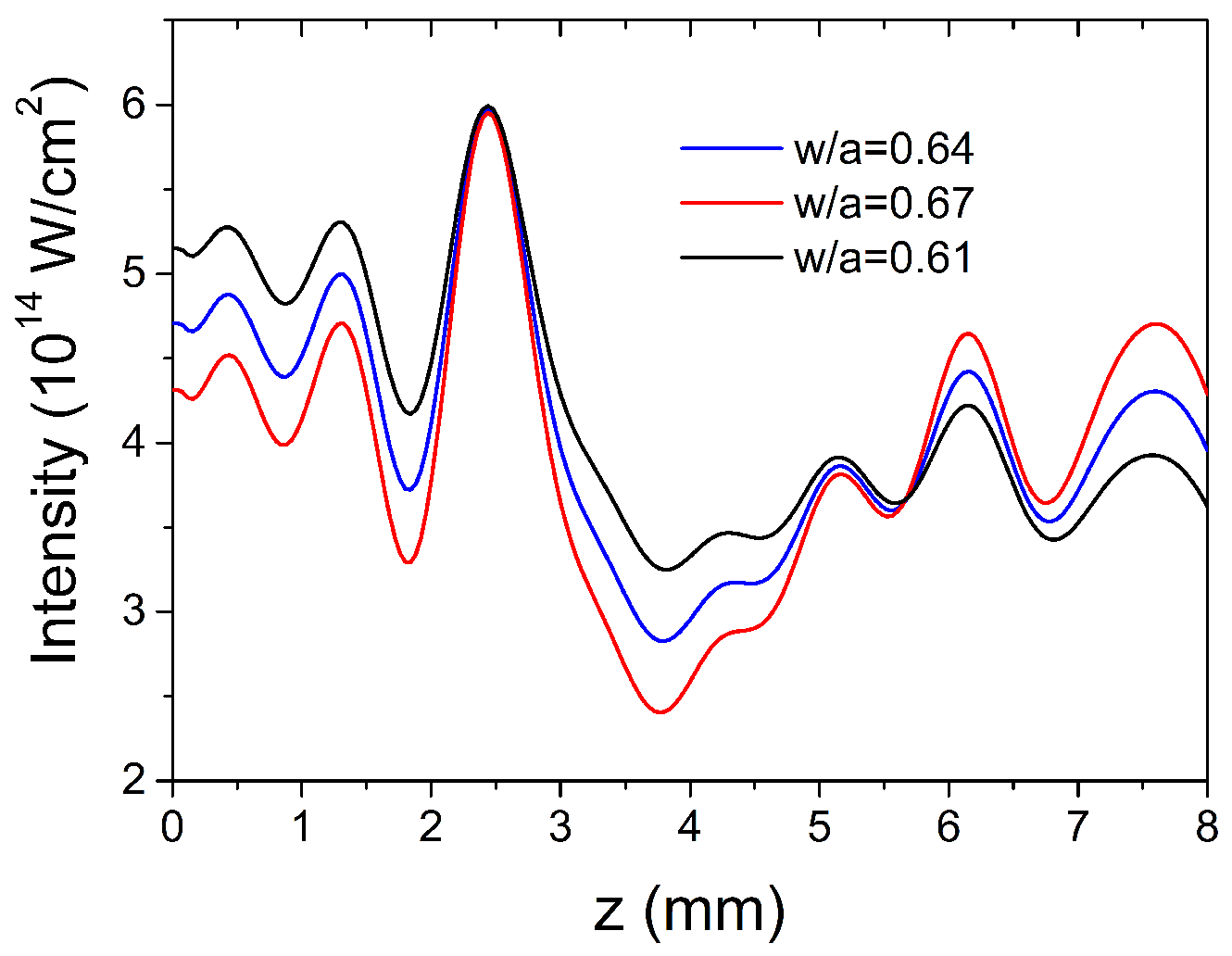


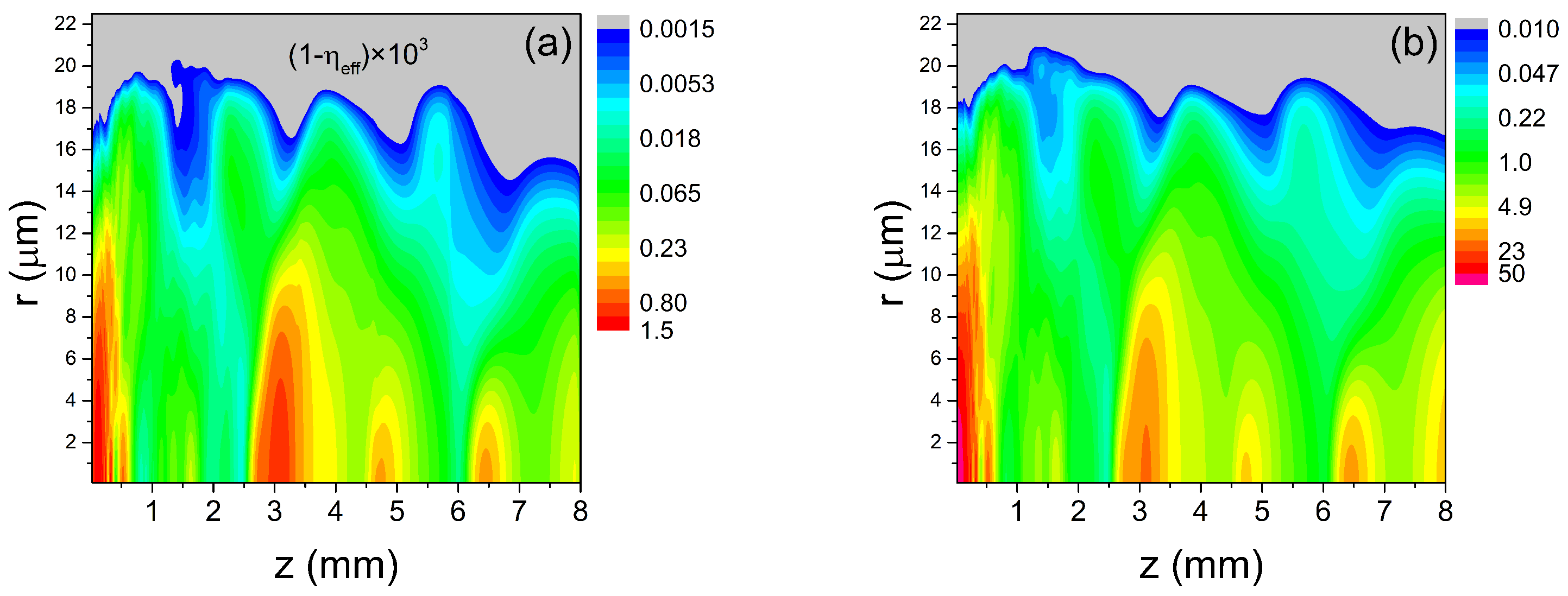
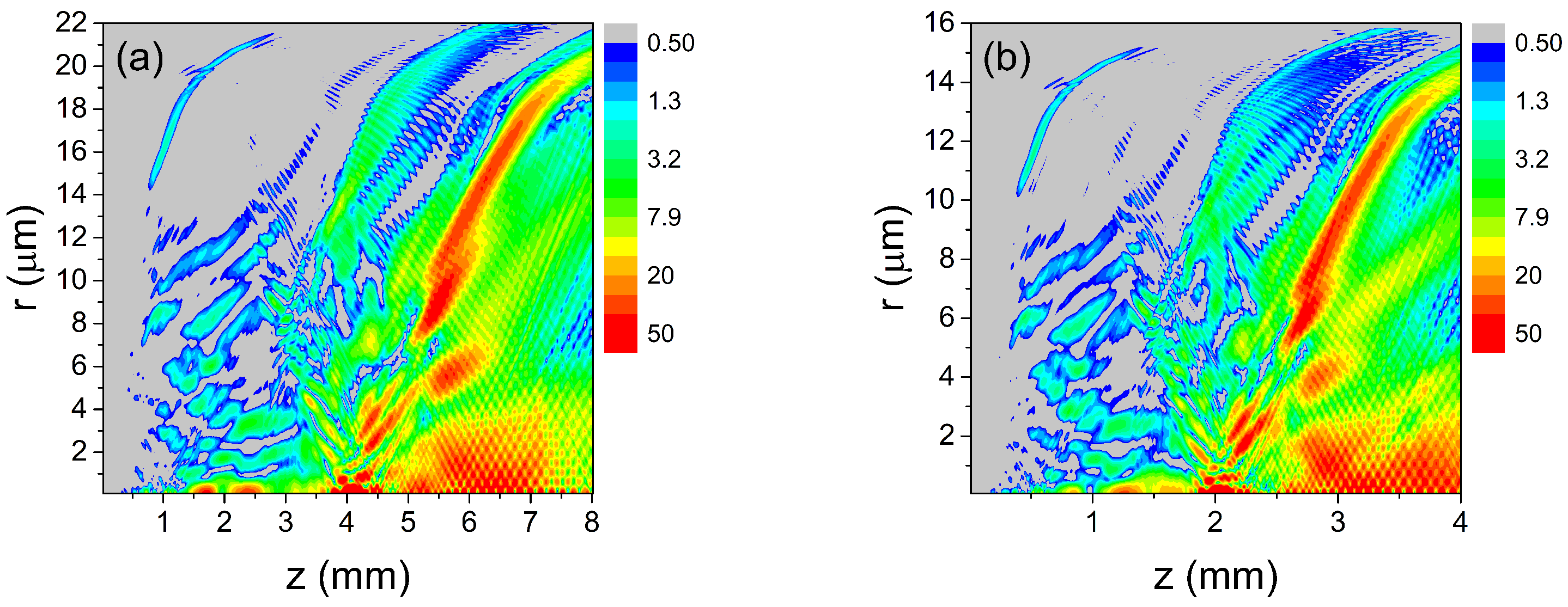
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tosa, V.; Gherman, A.M.M.; Kovacs, K.; Tóth, I. Modeling Femtosecond Beam Propagation in Dielectric Hollow-Core Waveguides. Photonics 2025, 12, 65. https://doi.org/10.3390/photonics12010065
Tosa V, Gherman AMM, Kovacs K, Tóth I. Modeling Femtosecond Beam Propagation in Dielectric Hollow-Core Waveguides. Photonics. 2025; 12(1):65. https://doi.org/10.3390/photonics12010065
Chicago/Turabian StyleTosa, Valer, Ana Maria Mihaela Gherman, Katalin Kovacs, and István Tóth. 2025. "Modeling Femtosecond Beam Propagation in Dielectric Hollow-Core Waveguides" Photonics 12, no. 1: 65. https://doi.org/10.3390/photonics12010065
APA StyleTosa, V., Gherman, A. M. M., Kovacs, K., & Tóth, I. (2025). Modeling Femtosecond Beam Propagation in Dielectric Hollow-Core Waveguides. Photonics, 12(1), 65. https://doi.org/10.3390/photonics12010065




