Study on Echo Characteristics of Debris Laser Ranging Based on Multi-Elevation Method
Abstract
1. Introduction
2. Basic Principle
2.1. Standard Atmospheric Model
2.2. Calculation of Atmospheric Optical Properties Based on Horizontal Visibility
2.3. Atmospheric Optical Properties Are Calculated Based on the Oblique Transmission Atmospheric Stratification Model
2.4. Determination of Atmospheric Optical Characteristics Using the Telescopic Multi-Elevation Approach
3. Numerical Analysis and Experimental Results Are Discussed
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Fried, D.L. Aperture Averaging of Scintillation. Opt. Soc. Am. 1967, 57, 169–175. [Google Scholar] [CrossRef]
- Wriedt, T. Mie Theory: A Review. The Mie Theory: Basics and Applications; Springer: Berlin/Heidelberg, Germany, 2012; pp. 53–71. [Google Scholar]
- Hu, Y.; Hou, A.; Zhang, X.; Han, F.; Zhao, N.; Xu, S.; Ma, Q.; Gu, Y.; Dong, X.; Chen, Y.; et al. Assessment of Lateral Structural Details of Targets Using Principles of Full Waveform Light Detection and Ranging. IEEE Trans. Geosci. Remote Sens. 2023, 61, 5704416. [Google Scholar] [CrossRef]
- Zhang, X.; Hu, Y.; Shen, S.; Fang, J.; Wang, Y.; Liu, Y.; Han, F. Kilometer-level laser reflective tomography experiment and debris barycenter stimation. Acta Phys. Sin. 2022, 71, 114205. [Google Scholar] [CrossRef]
- Lohmann, U.; Feichter, J. Global indirect aerosol effects: A review. Atmos. Chem. Phys. 2005, 5, 715–737. [Google Scholar] [CrossRef]
- LaRocca, A.J. Methods of calculating atmospheric transmittance and radiance in the infrared. Proc. IEEE 1975, 63, 75–94. [Google Scholar] [CrossRef]
- Sato, M.; Hansen, J.E.; McCormick, M.P.; Pollack, J.B. Stratospheric aerosol optical depths, 1850–1990. J. Geophys. Res. Atmos. 1993, 98, 22987–22994. [Google Scholar] [CrossRef]
- Sserunjogi, M.; Ambrose, K. Light extinction coefficient and particle size correlation for real-time prediction and quantitative measurement of suspended dust concentrations. Adv. Powder Technol. 2024, 35, 104354. [Google Scholar] [CrossRef]
- Steinvall, O. Laser system range calculations and the Lambert W function. Appl. Opt. 2009, 48, B1–B7. [Google Scholar] [CrossRef] [PubMed]
- Degnan, J. An upgraded SGSLR link analysis which includes the effects of atmospheric scintillation and target speckle. In Proceedings of the 20th ILRS Workshop, Potsdam, Germany, 9–14 October 2016. [Google Scholar]
- Jiang, D.; Qin, Y.C.; Wang, X.P. Sensitivity Analysis of Atmospheric Delay Correction for Spaceborne Laser Altimeter. Prog. Laser Optoelectron. 2019, 56, 61–68. [Google Scholar]
- Busygin, V.P.; Ginzburg, A.S.; Kuzmina, I.Y. Transfer of Laser Pulses through the Atmosphere into Space in the Presence of Clouds of the Upper and Middle Tiers. Izv. Atmos. Ocean. Phys. 2021, 57, 594–605. [Google Scholar] [CrossRef]
- Li, M.; Xue, L.; Huang, C. Estimation of detection range or space debris laser ranging system based on efficient echo probability. Opt. Precis. Eng. 2016, 24, 260–267. [Google Scholar]
- Du, J.M. Oblique Visibility Estimation Algorithm and Experiment for Double Elevation Optical Thickness of Laser Radar. Master’s Thesis, Xi’an University of Technology, Xi’an, China, 2024. [Google Scholar]
- Wang, A.; Yin, Z.; Mao, S.; Wang, L.; Yi, Y.; Chen, Q.; Müller, D.; Wang, X. Measurements of particle extinction coefficients at 1064 nm with lidar: Temperature dependence of rotational Raman channels. Opt. Express 2024, 32, 4650–4667. [Google Scholar] [CrossRef] [PubMed]
- Angstrom, A. The parameters of atmospheric turbidity. Tellus 1964, 16, 64–75. [Google Scholar] [CrossRef]
- Wiscombe, W.J. Improved Mie scattering algorithms. Appl. Opt. 1980, 19, 1505–1509. [Google Scholar] [CrossRef]
- Li, L.F.; Zhang, J.L.; Li, X.; Wang, Z.B.; Chen, Y.Y. Study on the extinction characteristics of aerosols in the vertical direction of space. J. Light Scatt. 2013, 25, 19–23. [Google Scholar]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Wei, H.M.; Zhao, W.; Dai, X.C. Influence of fog and aerosol particles’ forward-scattering on light extinction. Opt. Precis. Eng. 2018, 26, 1354–1361. [Google Scholar]
- Andrews, E.; Saxena, P.; Musarra, S.; Hildemann, L.; Koutrakis, P.; McMurry, P.; Olmez, I.; White, W. Concentration and composition of atmospheric aerosols from the 1995 SEAVS experiment and a review of the closure between chemical and gravimetric measurements. J. Air Waste Manag. Assoc. 2000, 50, 648–664. [Google Scholar] [CrossRef] [PubMed]
- Meng, W.D.; Zhang, H.F.; Deng, H.R.; Kai, T.; Zhi-Bo, W.; Yu-Rong, W.; Guang, W.; Zhong-Ping, Z.; Xin-Yang, C. 1.06 μm wavelength based high accuracy satellite laser ranging and space debris detection. Acta Phys. Sin. 2020, 69, 019502. [Google Scholar] [CrossRef]
- Ma, J.; Zhou, M.; Yu, Z.; Jiang, X.; Huo, Y.; Zang, K.; Zhang, J.; Harris, J.S.; Jin, G.; Zhang, Q.; et al. Simulation of a high efficiency and low jitter nanostructured silicon single photon avalanche diode. Optica 2015, 2, 974–979. [Google Scholar] [CrossRef]
- Ma, T.M. Research and Realization of Key Technology of Space Debris Laser Ranging. Ph.D. Thesis, Liaoning Technical University, Fuxin, China, 2021. [Google Scholar]


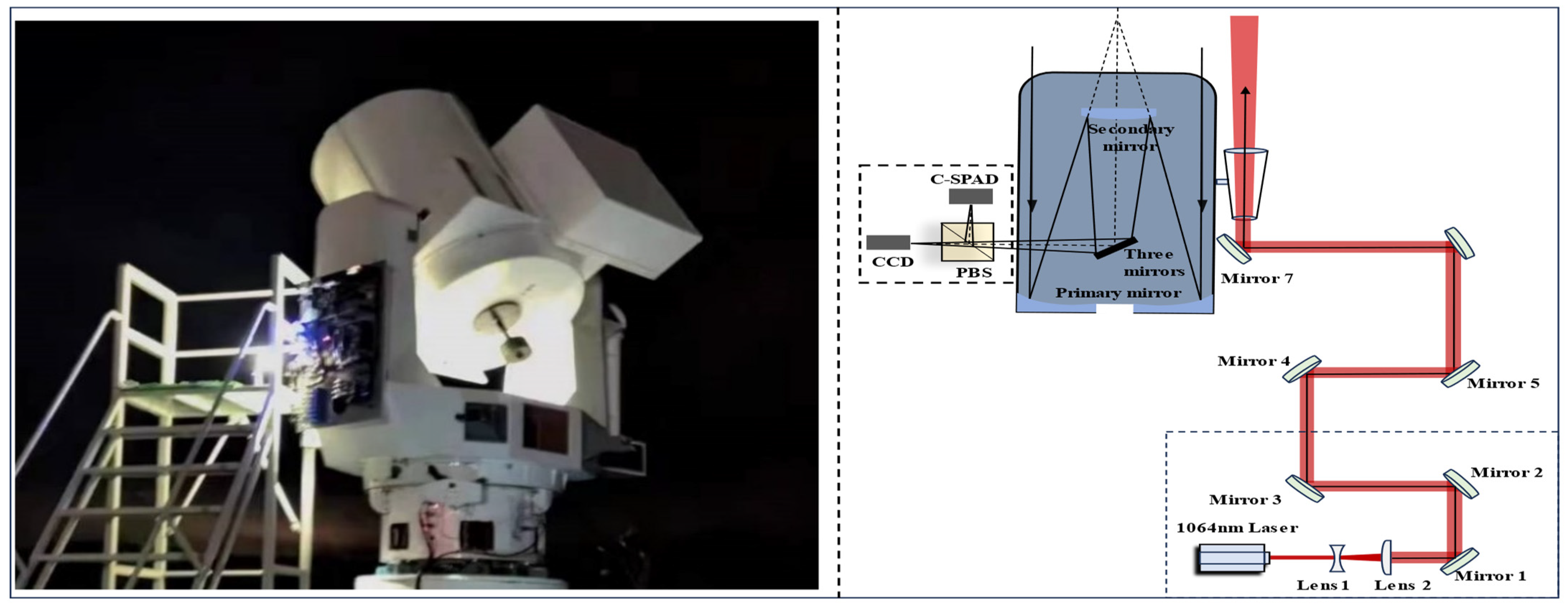
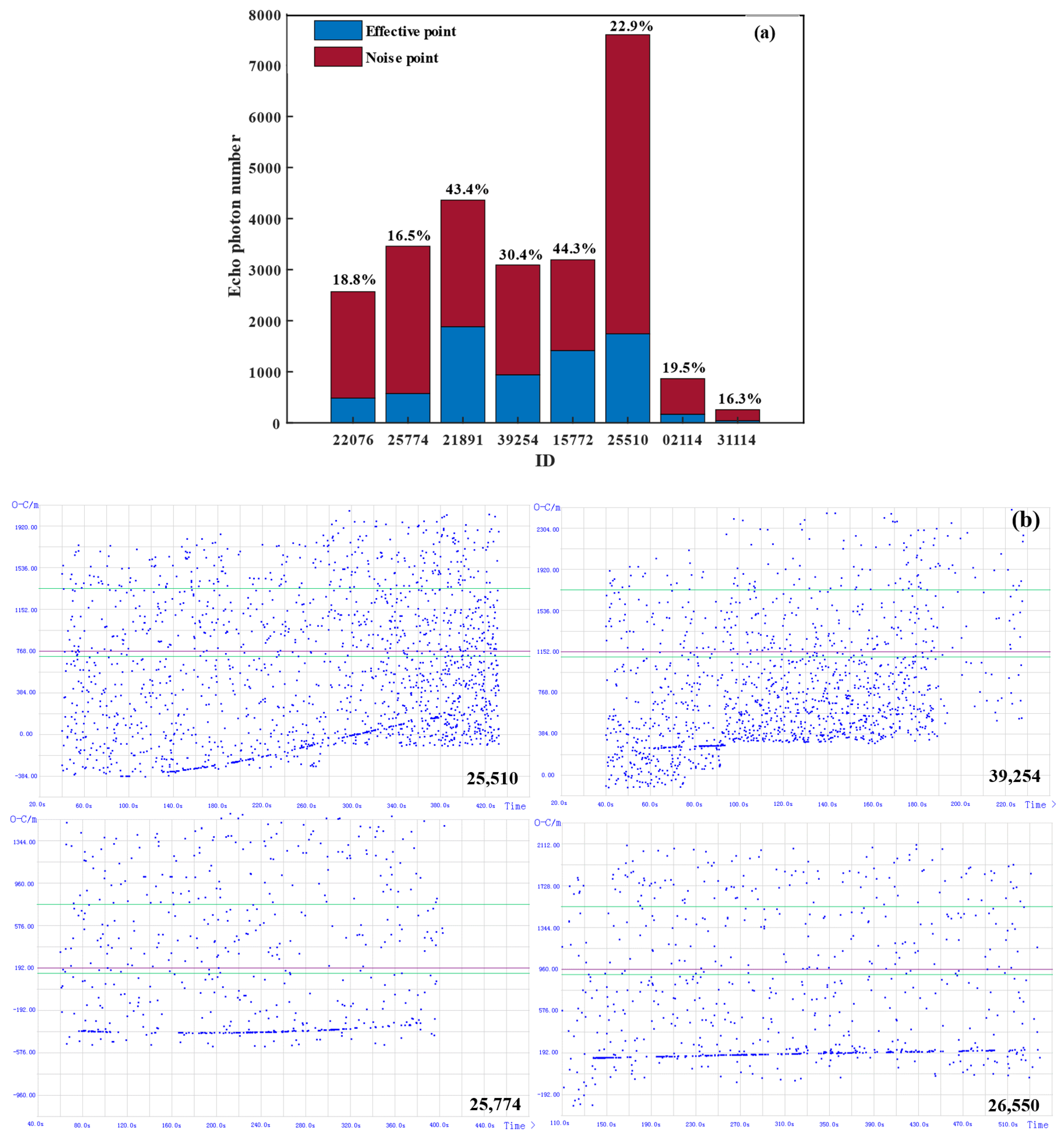
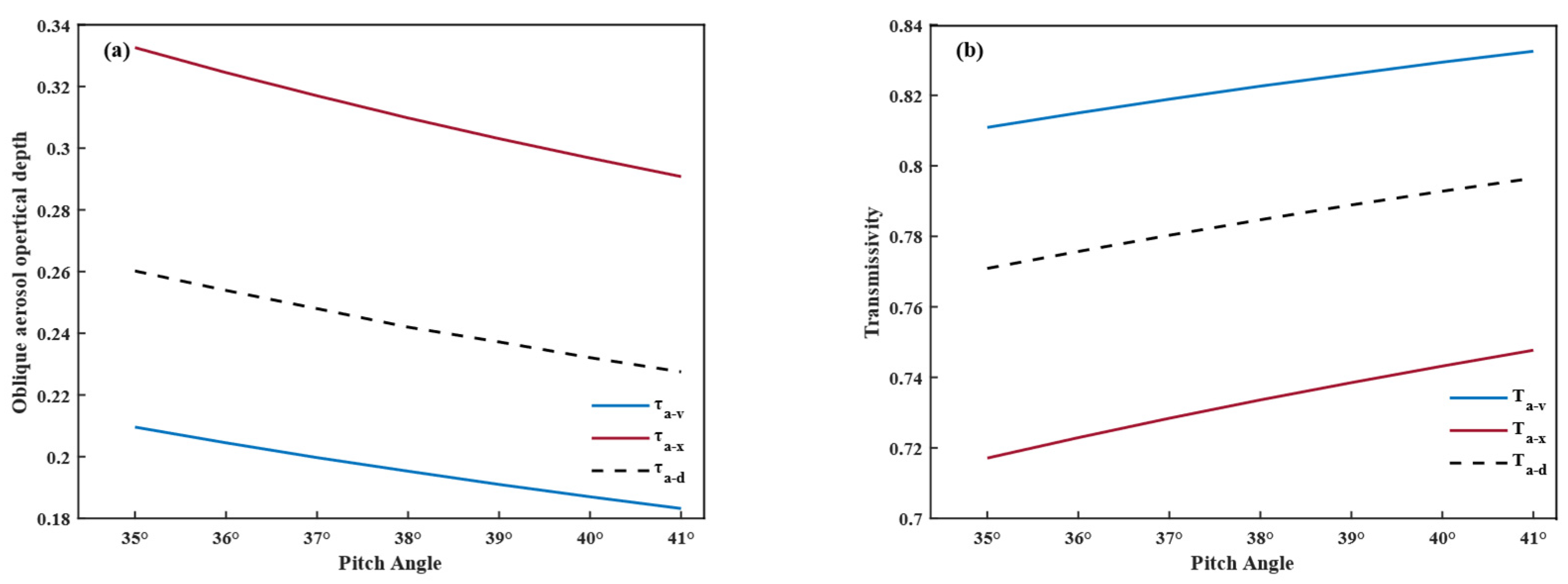
| /km | 2 | 4 | 6 | 8 | 10 | 13 | 25 |
| /km | 0.84 | 0.95 | 1.03 | 1.10 | 1.15 | 1.23 | 1.45 |
| Parameter | Value |
|---|---|
| Laser pulse energy | 2/(J) |
| Planck’s constant | 6.63 × 10−34/(J·s−1) |
| Frequency | 10/(HZ) |
| Aerosol particle radius | 0.001~13 (μm) |
| Effective area | 0.353/(m2) |
| Attenuation factor | 0.05 |
| Photodetector efficiency | 0.20 |
| Emission efficiency | 0.84 |
| Receiving efficiency | 0.60 |
| Angle of divergence | 6.6/(arcsec) |
| Date | Time/UTC | Name | ID | Pitch Angle Range/° | Apogee/km | Perigee/km | RCS/m2 | RMS/cm |
|---|---|---|---|---|---|---|---|---|
| 14 April 2024 | 03:15:09–03:24:34 | TOPEX/POSEIDON | 22076 | 13~35 | 1344 | 1331 | 7.7900 | 103 |
| 14 April 2024 | 21:42:11–21:46:58 | DELTA 2 R/B | 25774 | 24~30 | 1364 | 806 | 8.8586 | 102 |
| 14 April 2024 | 22:07:53–22:14:17 | DNEPR 1 R/B | 26550 | 22~45 | 1356 | 561 | 8.3563 | 123 |
| 14 April 2024 | 22:21:44–22:26:28 | DELTA 2 R/B(1) | 21891 | 20~40 | 635 | 575 | 10.108 | 84 |
| 14 April 2024 | 22:30:58–22:32:55 | EPSILON DEB | 39254 | 16~49 | 1115 | 846 | 2.0335 | 74 |
| 14 April 2024 | 22:55:01–22:57:32 | SL-12 R/B(2) | 15772 | 36~44 | 844 | 796 | 13.142 | 111 |
| 14 April 2024 | 23:00:08–23:04:34 | DELTA 2 R/B(1) | 25510 | 38~59 | 983 | 531 | 9.4862 | 172 |
| 15 April 2024 | 13:47:28–13:49:09 | ATLAS AGENA D R/B | 02144 | 24~28 | 752 | 740 | 6.0394 | 98 |
| 18 April 2024 | 07:33:37–07:34:57 | CZ-2C R/B | 31114 | 13~20 | 869 | 783 | 7.8070 | 139 |
| Pitch Angle | Experimental Data | Visibility Model | Relative Error | Oblique Transmission Model | Relative Error | Multiplicity Elevation Method | Relative Error |
|---|---|---|---|---|---|---|---|
| 35° | 2.15 | 2.51 | 16.74% | 1.960 | 8.837% | 2.266 | 5.395% |
| 36° | 2.38 | 2.65 | 11.34% | 2.090 | 12.18% | 2.238 | 5.966% |
| 37° | 2.50 | 2.77 | 10.80% | 2.189 | 12.44% | 2.512 | 0.480% |
| 38° | 2.71 | 2.86 | 5.540% | 2.275 | 16.05% | 2.603 | 3.948% |
| 39° | 2.85 | 3.02 | 5.965% | 2.413 | 15.33% | 2.754 | 3.368% |
| 40° | 2.60 | 3.10 | 19.23% | 2.484 | 4.462% | 2.803 | 7.808% |
| 41° | 3.00 | 3.61 | 20.33% | 2.913 | 2.900% | 3.166 | 5.533% |
| Mean relative error | 12.850% | 10.316% | 4.643% | ||||
| Pitch Angle | Experimental Data | Visibility Model | Relative Error | Oblique Transmission Model | Relative Error | Multiplicity Elevation Method | Relative Error |
|---|---|---|---|---|---|---|---|
| 22° | 3.60 | 4.436 | 23.22% | 3.044 | 15.44% | 4.084 | 13.456% |
| 23° | 4.25 | 5.027 | 18.28% | 3.503 | 17.58% | 4.509 | 6.0941% |
| 24° | 5.90 | 6.909 | 17.10% | 4.884 | 17.22% | 6.224 | 5.4915% |
| 25° | 6.00 | 7.064 | 17.73% | 5.440 | 9.330% | 6.389 | 6.4833% |
| 26° | 7.50 | 7.772 | 3.627% | 5.620 | 25.07% | 6.809 | 9.2133% |
| 27° | 7.00 | 8.164 | 16.63% | 5.984 | 14.51% | 7.436 | 6.2286% |
| 28° | 8.00 | 9.508 | 18.85% | 7.040 | 12.00% | 8.686 | 8.5750% |
| Mean relative error | 16.492% | 15.879% | 7.934% | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Zhao, Y.; Geng, B. Study on Echo Characteristics of Debris Laser Ranging Based on Multi-Elevation Method. Photonics 2024, 11, 818. https://doi.org/10.3390/photonics11090818
Wang J, Zhao Y, Geng B. Study on Echo Characteristics of Debris Laser Ranging Based on Multi-Elevation Method. Photonics. 2024; 11(9):818. https://doi.org/10.3390/photonics11090818
Chicago/Turabian StyleWang, Jie, Yongzhi Zhao, and Baocun Geng. 2024. "Study on Echo Characteristics of Debris Laser Ranging Based on Multi-Elevation Method" Photonics 11, no. 9: 818. https://doi.org/10.3390/photonics11090818
APA StyleWang, J., Zhao, Y., & Geng, B. (2024). Study on Echo Characteristics of Debris Laser Ranging Based on Multi-Elevation Method. Photonics, 11(9), 818. https://doi.org/10.3390/photonics11090818




