Research and Improvement in Magnetic Field Sensors Using Mach–Zehnder Interferometer with Cobalt Ferrite Nanoparticles
Abstract
1. Introduction
2. Materials and Methods
2.1. Synthesis of CoFe2O4 Nanoparticles
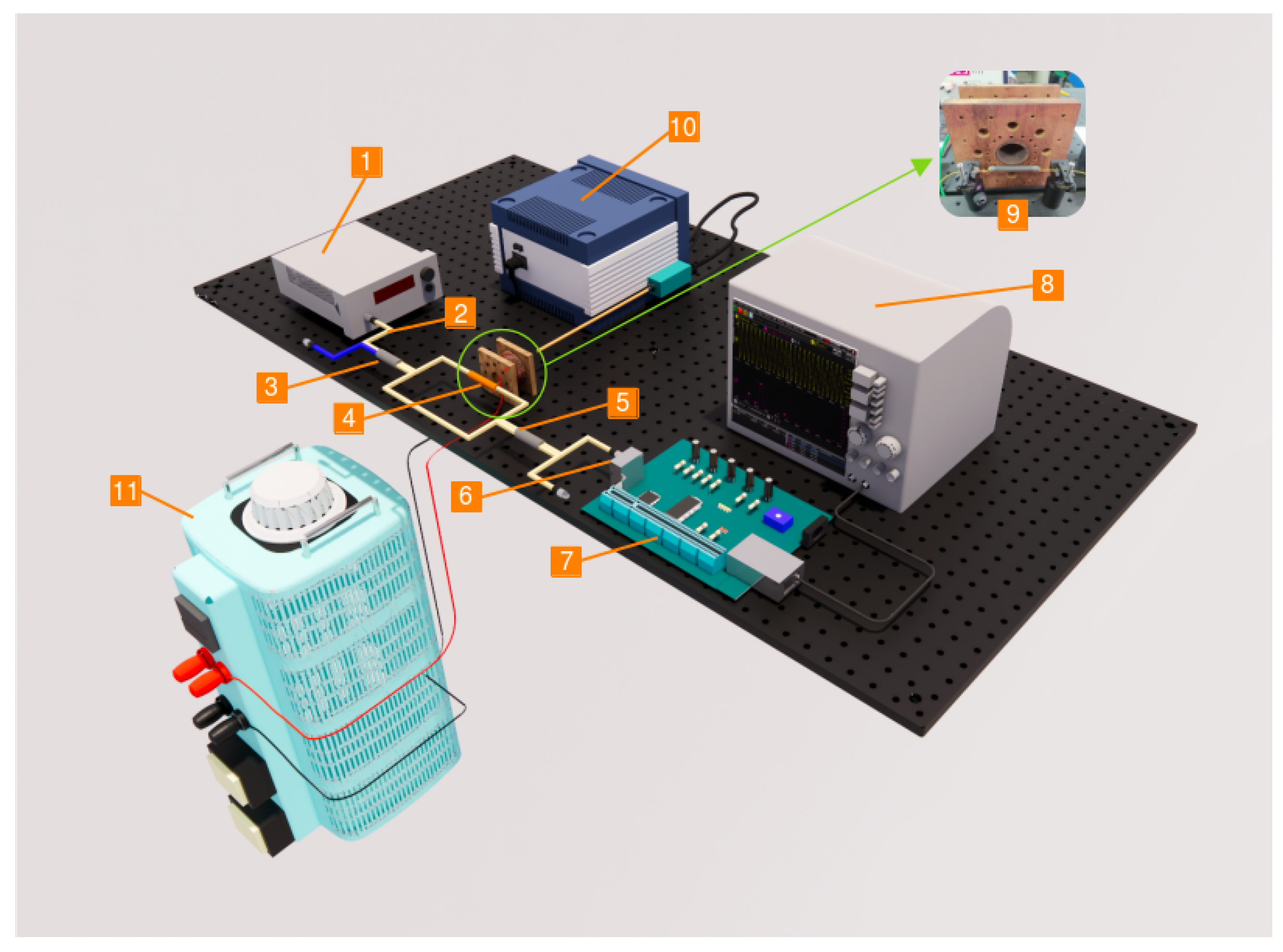
2.2. Description of the MZI Sensor Experimental Setup
3. Results
3.1. Characterization of Nanoparticles
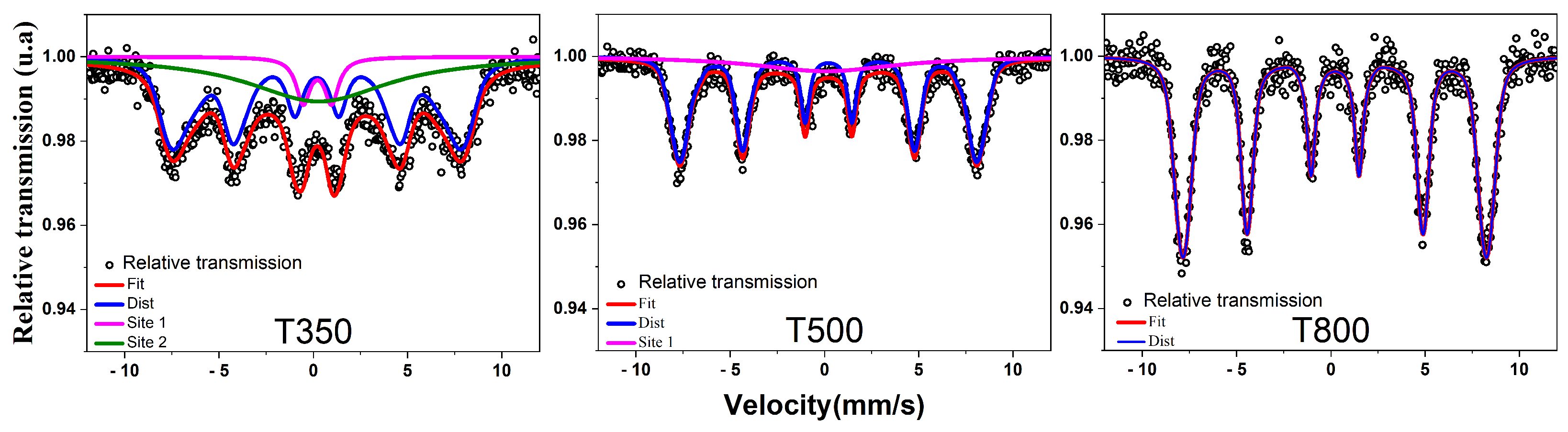
3.2. Mössbauer Spectroscopy
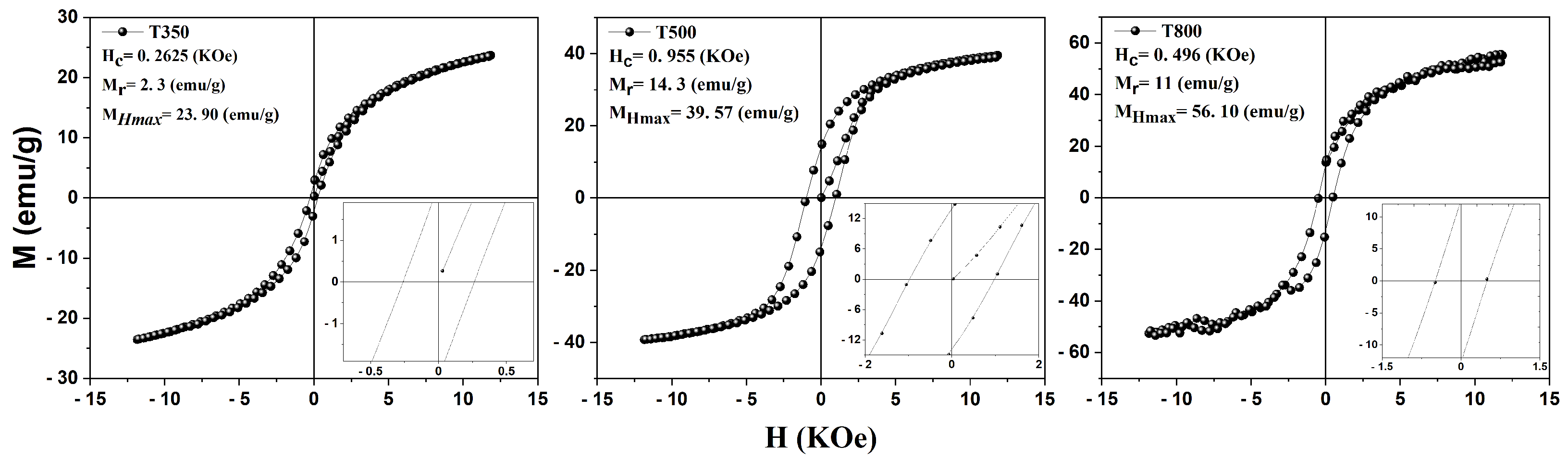
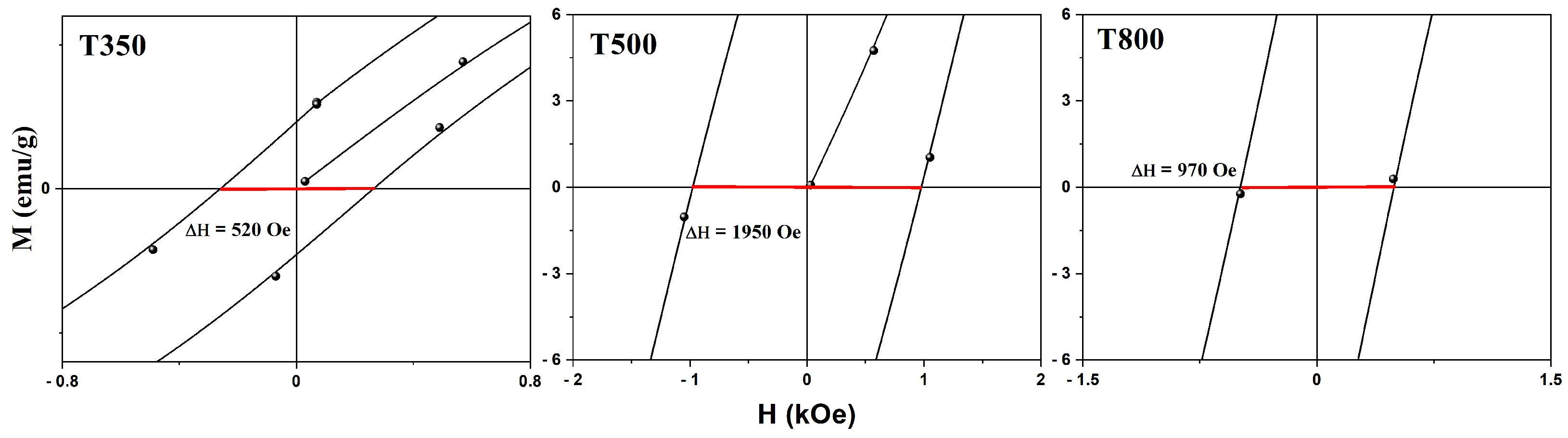
3.3. Vibrating Sample Magnetometer
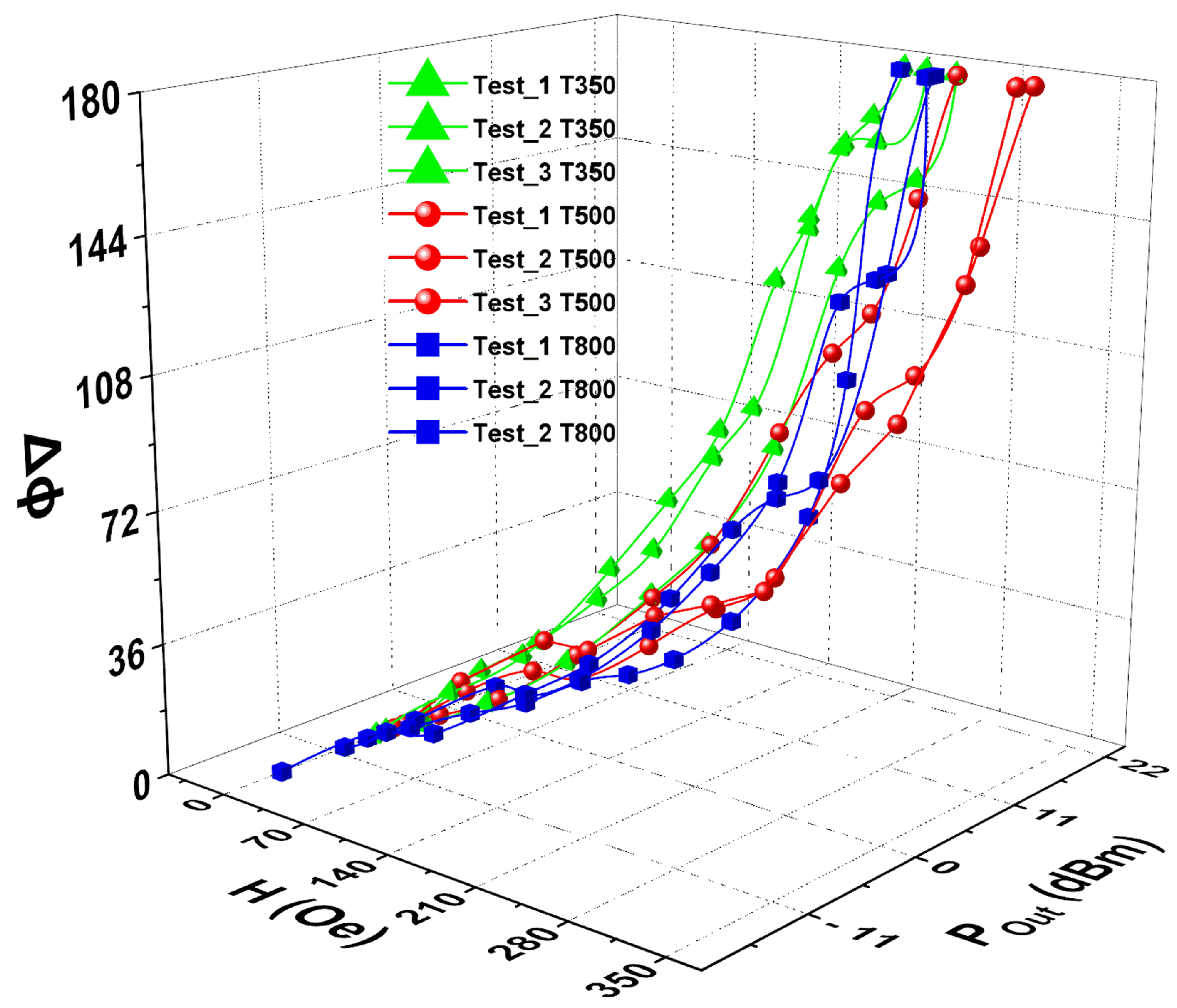
3.4. Experimental Result and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ganapathe, L.S.; Mohamed, M.A.; Mohamad Yunus, R.; Berhanuddin, D.D. Magnetite (Fe3O4) nanoparticles in biomedical application: From synthesis to surface functionalisation. Magnetochemistry 2020, 6, 68. [Google Scholar] [CrossRef]
- Valenzuela, R. Novel applications of ferrites. Phys. Res. Int. 2012, 2012, 591839. [Google Scholar] [CrossRef]
- Duran, N. Nanotecnologia: Introdução, Preparação e Caracterização de Nanomateriais e Exemplos de Aplicação; Artliber: São Paulo, Brazil, 2006. [Google Scholar]
- Fechine, P.B.A. Avanços no Desenvolvimento de Nanomateriais; Imprensa Universitária: Fortaleza, Brazil, 2020. [Google Scholar]
- Souza, F.C.N.; Maia, L.S.P.; Medeiros, G.M.; Miranda, M.A.R.; Sasaki, J.M.; Guimaraes, G.F. Optical Current and Magnetic Field Sensor Using Mach-Zehnder Interferometer with Nanoparticles. IEEE Sens. J. 2018, 18, 7998–8004. [Google Scholar] [CrossRef]
- Maia, L.S.P.; Miranda, M.A.R.; de Souza, I.M.X.; de Assis, B.C.P.; Rocha, D.S.; Sasaki, J.M.; de Alexandria, A.R.; de Freitas Guimarães, G. Optical current and magnetic field sensor using multimodal interference in fiber optics with carbon steel. IEEE Sens. J. 2022, 22, 12877–12885. [Google Scholar] [CrossRef]
- Friebele, E.J. Fiber Bragg grating strain sensors: Present and future applications in smart structures. Opt. Photonics News 1998, 9, 33. [Google Scholar] [CrossRef]
- Frazão, O.; Falate, R.; Fabris, J.; Santos, J.L.; Ferreira, L.A.; Araújo, F. Optical inclinometer based on a single long-period fiber grating combined with a fused taper. Opt. Lett. 2006, 31, 2960–2962. [Google Scholar] [CrossRef] [PubMed]
- Liu, J. Fuzzy modularity and fuzzy community structure in networks. Eur. Phys. J. B 2010, 77, 547–557. [Google Scholar] [CrossRef]
- Grattan, K.T.V.; Sun, T. Fiber optic sensor technology: An overview. Sens. Actuators A Phys. 2000, 82, 40–61. [Google Scholar] [CrossRef]
- Berkovic, G.; Shafir, E. Optical methods for distance and displacement measurements. Adv. Opt. Photonics 2012, 4, 441–471. [Google Scholar] [CrossRef]
- Araújo, M.R.; Maia, L.S.; Miranda, M.A.; Martínez-Camejo, Y.; Sasaki, J.M.; Guimarães, G.F. Optimization of nanoparticles for application in optical sensors. Sens. Actuators A Phys. 2024, 366, 114923. [Google Scholar] [CrossRef]
- Krohn, D.A.; MacDougall, T.; Mendez, A. Fiber Optic Sensors; Spie Press Bellingham: Bellingham, WA, USA, 2014. [Google Scholar]
- Zhou, X.; Li, X.; Li, S.; An, G.W.; Cheng, T. Magnetic field sensing based on SPR optical fiber sensor interacting with magnetic fluid. IEEE Trans. Instrum. Meas. 2018, 68, 234–239. [Google Scholar] [CrossRef]
- Lee, B.H.; Kim, Y.H.; Park, K.S.; Eom, J.B.; Kim, M.J.; Rho, B.S.; Choi, H.Y. Interferometric fiber optic sensors. Sensors 2012, 12, 2467–2486. [Google Scholar] [CrossRef] [PubMed]
- Maia, A.d.O.G.; Oliveira, F.G.S.; Cordeiro, C.H.N.; Teixeira, E.S.; da Silva, E.B.; Soares, J.M.; de Vasconcelos, I.F.; Sasaki, J.M.; Dumelow, T. Synthesis of CoFe2O4 superparamagnetic nanoparticles using a rapid thermal processing furnace with halogen lamps. J. Sol-Gel Sci. Technol. 2021, 99, 527–533. [Google Scholar] [CrossRef]
- Young, R.A. The Rietveld Method; International Union of Crystallography: Chester, UK, 1993; Volume 5. [Google Scholar]
- Chandra, G.; Srivastava, R.; Reddy, V.; Agrawal, H. Effect of sintering temperature on magnetization and Mössbauer parameters of cobalt ferrite nanoparticles. J. Magn. Magn. Mater. 2017, 427, 225–229. [Google Scholar] [CrossRef]
- Gu, Z.; Xiang, X.; Fan, G.; Li, F. Facile synthesis and characterization of cobalt ferrite nanocrystals via a simple reduction-oxidation route. J. Phys. Chem. C 2008, 112, 18459–18466. [Google Scholar] [CrossRef]
- Moumen, N.; Bonville, P.; Pileni, M. Control of the size of cobalt ferrite magnetic fluids: Mössbauer spectroscopy. J. Phys. Chem. 1996, 100, 14410–14416. [Google Scholar] [CrossRef]
- Esharighi, M.; Kameli, P. Magnetic properties of CoFe2O4 nanoparticles prepared by thermal treatment of ball-milled precursors. Curr. Appl. Phys. 2011, 11, 476–481. [Google Scholar] [CrossRef]
- Nogueira, N.A.S.; Utuni, V.H.S.; Silva, Y.C.; Kiyohara, P.K.; Vasconcelos, I.F.; Miranda, M.A.R.; Sasaki, J.M. X-ray diffraction and Mossbauer studies on superparamagnetic nickel ferrite (NiFe2O4) obtained by the proteic sol-gel method. Mater. Chem. Phys. 2015, 163, 402–406. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Zeng, L.; Sun, X.; Zhang, L.; Hu, Y.; Duan, J. High sensitivity magnetic field sensor based on a Mach-Zehnder interferometer and magnetic fluid. Optik 2022, 249, 168234. [Google Scholar] [CrossRef]
- Zhang, J.; Chen, F.; Wang, R.; Qiao, X.; Chen, H.; Zhang, X. Vector magnetic field measurement based on magnetic fluid and high-order cladding-mode Bragg grating. Opt. Laser Technol. 2021, 143, 107264. [Google Scholar] [CrossRef]


| T350 | T500 | T800 | |
|---|---|---|---|
| Element | Mass (%) | Mass (%) | Mass (%) |
| Fe | 62,681 | 62,647 | 59,012 |
| Co | 34,696 | 34,429 | 32,464 |
| Molar (Co) | 1.38 | 1.42 | 1.26 |
| Molar (Fe) | 2.63 | 2.63 | 2.47 |
| Sample | T350 | T500 | T800 |
|---|---|---|---|
| (%) | 19.45 | 20.01 | 21.05 |
| (%) | 14.24 | 14.31 | 16.19 |
| 1.060 | 1.149 | 1.230 | |
| a (Å) | 8.359(6) | 8.382(4) | 8.392(7) |
| V () | 585.47(1) | 588.74(5) | 589.59(3) |
| (g/cm3) | 5.324 | 5.293 | 5.220 |
| (nm) | 8 | 27 | 67 |
| (%) | 0 | 0 | 0.5 |
| (emu/g) | 23.90 | 39.59 | 56.10 |
| (emu/g) | 2.3 | 14.3 | 11 |
| (Oe) | 262.5 | 955 | 496 |
| Sample | T350 | T500 | T800 |
|---|---|---|---|
| (Oe) | 251 | 282 | 254 |
| (dBm) | 23.15 | 21.06 | 28.47 |
| a | |||
| b | |||
| S (dB/Oe) | 1.15 | 0.93 | 1.41 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
de Vasconcelos, F.W.C.; Araújo, M.R.; Maia, L.S.P.; Rodrigues, L.Q.; da Silva, I.K.A.; Miranda, J.I.S.; Sasaki, J.M.; Miranda, M.A.R.; de Andrade, J.S.; Camejo, Y.M.; et al. Research and Improvement in Magnetic Field Sensors Using Mach–Zehnder Interferometer with Cobalt Ferrite Nanoparticles. Photonics 2024, 11, 806. https://doi.org/10.3390/photonics11090806
de Vasconcelos FWC, Araújo MR, Maia LSP, Rodrigues LQ, da Silva IKA, Miranda JIS, Sasaki JM, Miranda MAR, de Andrade JS, Camejo YM, et al. Research and Improvement in Magnetic Field Sensors Using Mach–Zehnder Interferometer with Cobalt Ferrite Nanoparticles. Photonics. 2024; 11(9):806. https://doi.org/10.3390/photonics11090806
Chicago/Turabian Stylede Vasconcelos, Francisco Willame Coelho, Matheus Rodrigues Araújo, Luana Samara Paulino Maia, Lidia Quirino Rodrigues, Ianna Karollayne Alencar da Silva, João Isaac Silva Miranda, José Marcos Sasaki, Marcus Aurélio Ribeiro Miranda, Joacir Soares de Andrade, Yosdan Martinez Camejo, and et al. 2024. "Research and Improvement in Magnetic Field Sensors Using Mach–Zehnder Interferometer with Cobalt Ferrite Nanoparticles" Photonics 11, no. 9: 806. https://doi.org/10.3390/photonics11090806
APA Stylede Vasconcelos, F. W. C., Araújo, M. R., Maia, L. S. P., Rodrigues, L. Q., da Silva, I. K. A., Miranda, J. I. S., Sasaki, J. M., Miranda, M. A. R., de Andrade, J. S., Camejo, Y. M., & Guimarães, G. d. F. (2024). Research and Improvement in Magnetic Field Sensors Using Mach–Zehnder Interferometer with Cobalt Ferrite Nanoparticles. Photonics, 11(9), 806. https://doi.org/10.3390/photonics11090806





