Optical Detection of Underwater Propeller Wake Based on a Position-Sensitive Detector
Abstract
1. Introduction
2. Principle of the Underwater Vehicle Wake Detection
3. Experimental Setup
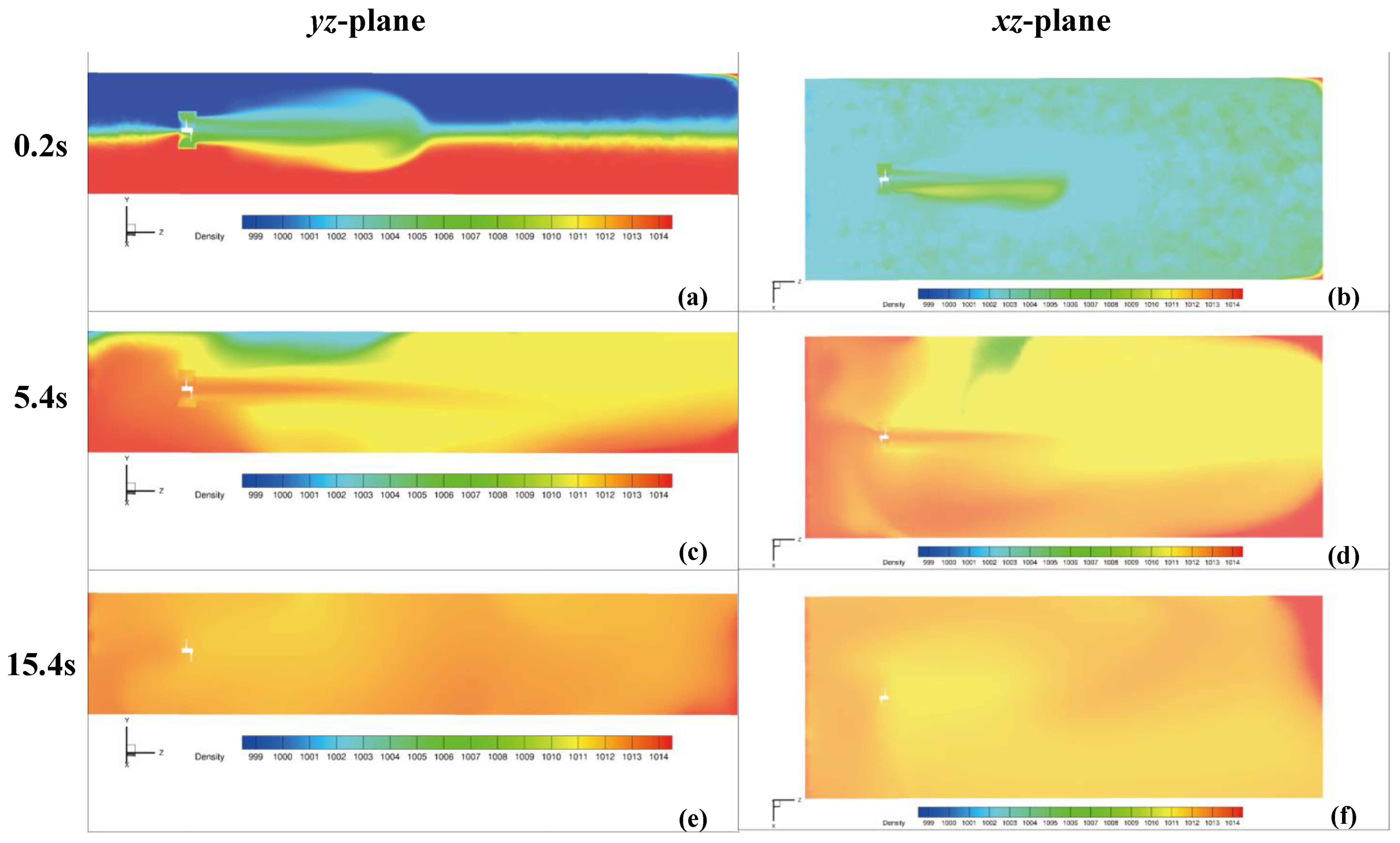
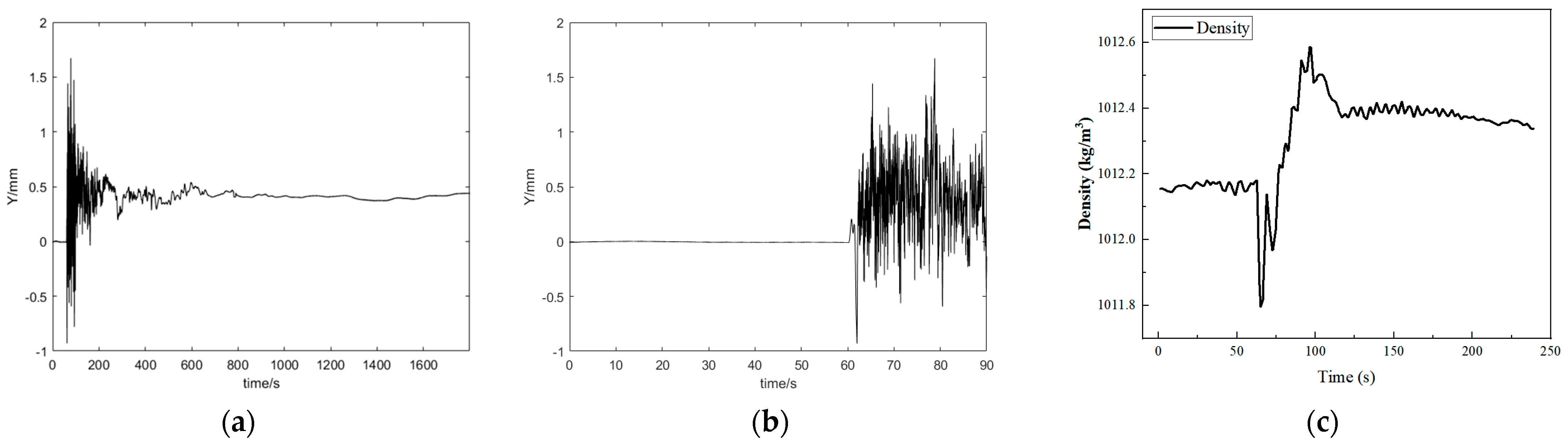
4. Simulation Analysis
5. Results and Discussion
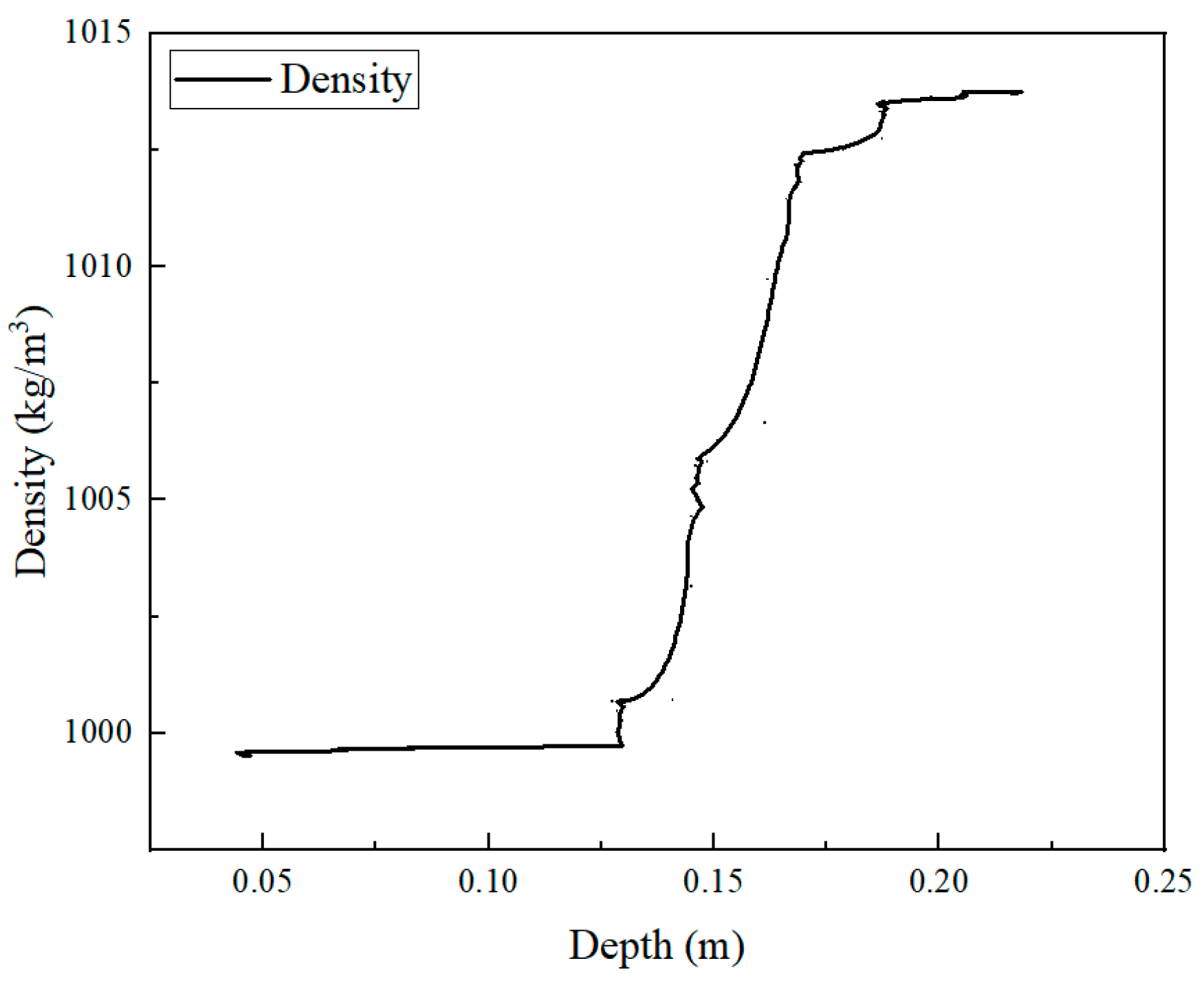
5.1. Results of Propeller Wake Measurements Conducted in a Strong Density Stratification Water Environment
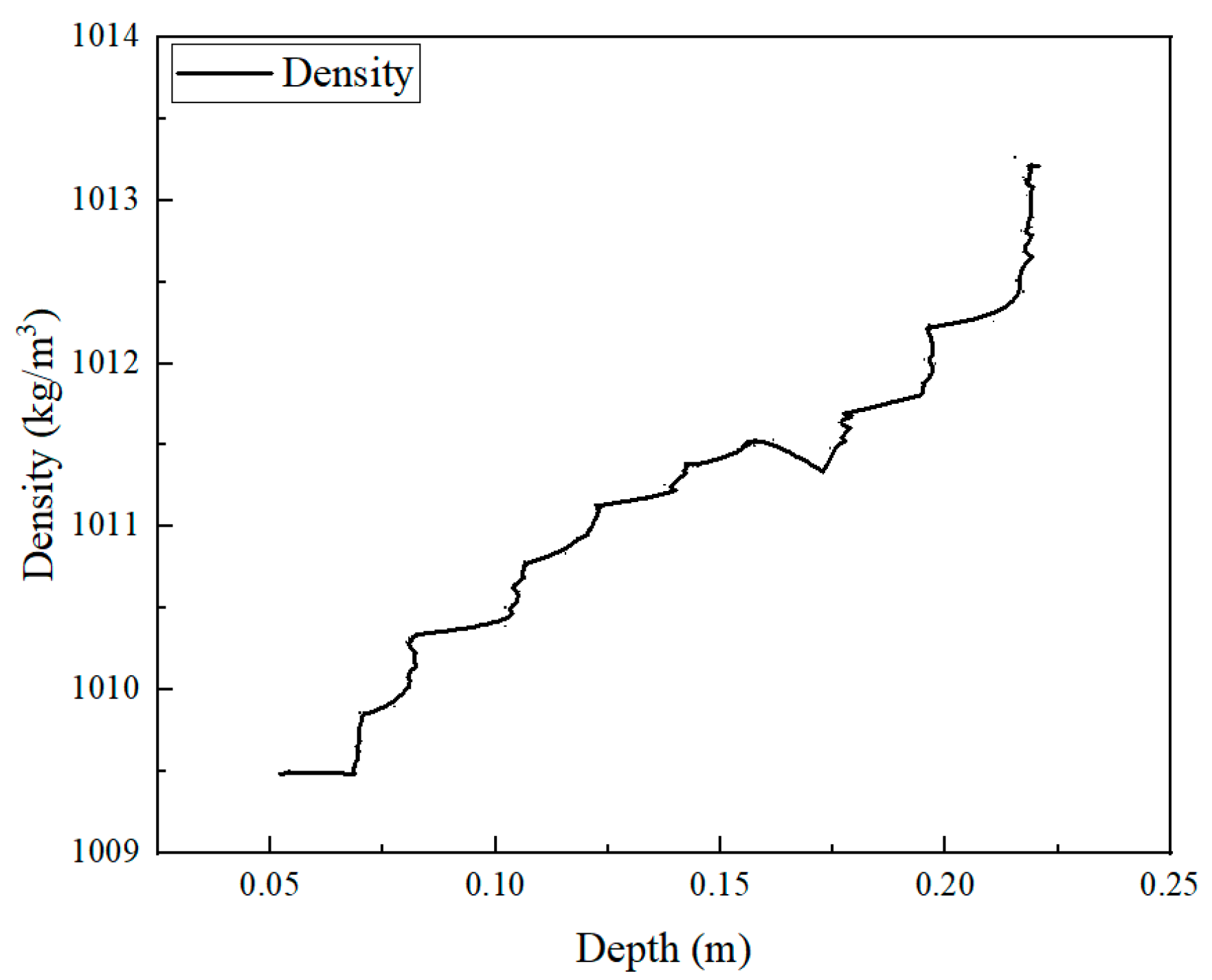
5.2. Results of Propeller Wake Measurements Conducted in a Linear Density Stratification Water Environment
5.3. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zong, S.G.; Zhang, X.; Duan, Z.K.; Yang, S.P.; Chen, B. Research on Laser Dual-Mode Fusion Detection Method of Ship Wake Bubbles. Appl. Sci. 2024, 14, 3695. [Google Scholar] [CrossRef]
- Higley, P.D. A Low-Cost Acoustic Positioning System for Small Manned Submarines. IEEE J. Oceanic. Eng. 1983, 8, 113–115. [Google Scholar] [CrossRef]
- Kumar, S.; Chinthaginjala, R.; Anbazhagan, R.; Nyangaresi, V.O.; Pau, G. Submarine Acoustic Target Strength Modeling at High-Frequency Asymptotic Scattering. IEEE Access 2024, 12, 4859–4870. [Google Scholar] [CrossRef]
- Kim, H.M.; Hong, S.Y.; Kwon, H.W.; Song, J.H.; Jeon, J.J.; Jung, W.J. Numerical simulation of submarines with anechoic coatings for acoustic target strength reduction. Nav. Eng. J. 2012, 124, 49–58. [Google Scholar]
- Zhou, S.L.; Fang, Z. Optimization Design of Acoustic Performance of Underwater Anechoic Coatings. Acoust. Aust. 2022, 50, 297–313. [Google Scholar] [CrossRef]
- Lan, C.F.; Yu, Z.L.; Chen, H.; Zhang, L.; Zhang, M. Research on Underwater Collaborative Detection Method Based on Complex Marine Environment. IEEE Access 2024, 12, 3464–3475. [Google Scholar] [CrossRef]
- Huang, B.; Liu, Z.Y.; Xu, Y.J.; Ding, Q.C.; Pan, M.C.; Hu, J.F.; Zhang, Q. Characteristics of Magnetic Fields Induced by the Wake of an Underwater Vehicle. Appl. Sci. 2022, 12, 7964. [Google Scholar] [CrossRef]
- Sitefanick, T. The Nonacoustic Detection of Submarines. Sci. Am. 1988, 258, 41. [Google Scholar] [CrossRef]
- Jiang, Y.N.; Yang, Z.Y.; Li, K.; Liu, T. Pre-Processing of Simulated Synthetic Aperture Radar Image Scenes Using Polarimetric Enhancement for Improved Ship Wake Detection. Remote Sens. 2024, 16, 658. [Google Scholar] [CrossRef]
- Tatavarti, R.; Ananth, P.N.; Rajasree, A.K.; Vidyalal, V.; Radhakrishnan, P.; Nampoori, V.P.N.; Vallabhan, C.P.G. Internal Waves-a Novel Measurement Technique. Curr. Sci. 1995, 69, 678–684. [Google Scholar]
- Swain, S.K.; Trinath, K. Non-Acoustic Detection of Moving Submerged Bodies in Ocean. Int. J. Innov. Res. Dev. 2012, 1, 361–372. [Google Scholar]
- Voropayev, S.I.; McEachern, G.B.; Fernando, H.J.S.; Boyer, D.L. Large vortex structures behind a maneuvering body in stratified fluids. Phys. Fluids 1999, 11, 1682–1684. [Google Scholar] [CrossRef]
- Voropayev, S.I.; Smirnov, S.A. Vortex streets generated by a moving momentum source in a stratified fluid. Phys. Fluids 2003, 15, 618–624. [Google Scholar] [CrossRef]
- Bentley, J.P.; Mudd, J.W. Vortex shedding mechanisms in single and dual bluff bodies. Flow Meas. Instrum. 2003, 14, 23–31. [Google Scholar] [CrossRef]
- Schulte-Pelkum, N.; Wieskotten, S.; Hanke, W.; Dehnhardt, G.; Mauck, B. Tracking of biogenic hydrodynamic trails in harbour seals (Phoca vitulina). J. Exp. Biol. 2007, 210, 781–787. [Google Scholar] [CrossRef]
- Gläser, N.; Wieskotten, S.; Otter, C.; Dehnhardt, G.; Hanke, W. Hydrodynamic trail following in a California sea lion (Zalophus californianus). J. Comp. Physiol. A 2011, 197, 141–151. [Google Scholar] [CrossRef]
- Hanke, W.; Wieskotten, S.; Marshall, C.; Dehnhardt, G. Hydrodynamic perception in true seals (Phocidae) and eared seals (Otariidae). J. Comp. Physiol. A 2013, 199, 421–440. [Google Scholar] [CrossRef] [PubMed]
- Dehnhardt, G.; Mauck, B.; Bleckmann, H. Seal whiskers detect water movements. Nature 1998, 394, 235–236. [Google Scholar] [CrossRef]
- Eberhardt, W.C.; Wakefield, B.F.; Murphy, C.T.; Casey, C.; Shakhsheer, Y.; Calhoun, B.H.; Reichmuth, C. Development of an artificial sensor for hydrodynamic detection inspired by a seal’s whisker array. Bioinspir. Biomim. 2016, 11, 056011. [Google Scholar] [CrossRef] [PubMed]
- Dalziel, S.B.; Hughes, G.O.; Sutherland, B.R. Whole-field density measurements by ‘synthetic schlieren’. Exp. Fluids 2000, 28, 322–335. [Google Scholar] [CrossRef]
- Elsinga, G.E.; van Oudheusden, B.W.; Scarano, F.; Watt, D.W. Assessment and application of quantitative schlieren methods: Calibrated color schlieren and background oriented schlieren. Exp. Fluids 2004, 36, 309–325. [Google Scholar] [CrossRef]
- Hargather, M.J.; Settles, G.S. A comparison of three quantitative schlieren techniques. Opt. Laser Eng. 2012, 50, 8–17. [Google Scholar] [CrossRef]
- Ben-Gida, H.; Liberzon, A.; Gurka, R. A stratified wake of a hydrofoil accelerating from rest. Exp. Therm. Fluid Sci. 2016, 70, 366–380. [Google Scholar] [CrossRef]
- Stella, A.; Guj, G.; Di Felice, F. Propeller wake flowfield analysis by means of LDV phase sampling techniques. Exp. Fluids 2000, 28, 1–10. [Google Scholar] [CrossRef]
- Paik, B.G.; Ahn, J.W.; Seol, H.S.; Park, Y.H.; Kim, K.; Cheon, H.G. Development of LDV (Laser Doppler Velocimetry) for Measuring Three Dimensional Hull Wake of Ship Model in Large Cavitation Tunnel. J. Soc. Nav. Archit. Korea 2017, 54, 515–521. [Google Scholar] [CrossRef]
- Tatavarti, R.; Sanjaya, K.S.; Trinath, K.; Arulmozhivarman, P. Evolution of Non Acoustic Detection Systems. Inst. Def. Sci. Technol. J. 2013, 12, 1–13. [Google Scholar]
- Zhang, S.W.; Li, L.Y.; Liu, Y.L.; Zhou, Y. Drift Error Compensation Algorithm for Heterodyne Optical Seawater Refractive Index Monitoring of Unstable Signals. Sensors 2023, 23, 8460. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.Z.; Guo, C.Y.; Su, Y.M.; Wu, T.C. A numerical study on the correlation between the evolution of propeller trailing vortex wake and skew of propellers. Int. J. Nav. Arch. Ocean 2018, 10, 212–224. [Google Scholar] [CrossRef]
- Wang, L.Z.; Guo, C.Y.; Xu, P.; Su, Y.M. Analysis of the performance of an oscillating propeller in cavitating flow. Ocean Eng. 2018, 164, 23–39. [Google Scholar] [CrossRef]
- Wang, L.Z.; Guo, C.Y.; Xu, P.; Su, Y.M. Analysis of the wake dynamics of a propeller operating before a rudder. Ocean Eng. 2019, 188, 106250. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, G.; Liu, Q.; Wang, H.; Li, L.; Zhou, Y.; Chen, X. Optical Detection of Underwater Propeller Wake Based on a Position-Sensitive Detector. Photonics 2024, 11, 732. https://doi.org/10.3390/photonics11080732
Zhou G, Liu Q, Wang H, Li L, Zhou Y, Chen X. Optical Detection of Underwater Propeller Wake Based on a Position-Sensitive Detector. Photonics. 2024; 11(8):732. https://doi.org/10.3390/photonics11080732
Chicago/Turabian StyleZhou, Guanlong, Qin Liu, Hu Wang, Liyan Li, Yan Zhou, and Xinyu Chen. 2024. "Optical Detection of Underwater Propeller Wake Based on a Position-Sensitive Detector" Photonics 11, no. 8: 732. https://doi.org/10.3390/photonics11080732
APA StyleZhou, G., Liu, Q., Wang, H., Li, L., Zhou, Y., & Chen, X. (2024). Optical Detection of Underwater Propeller Wake Based on a Position-Sensitive Detector. Photonics, 11(8), 732. https://doi.org/10.3390/photonics11080732





