Broadband Instantaneous Frequency Measurement Using Frequency-to-Time Mapping and Channelization
Abstract
1. Introduction
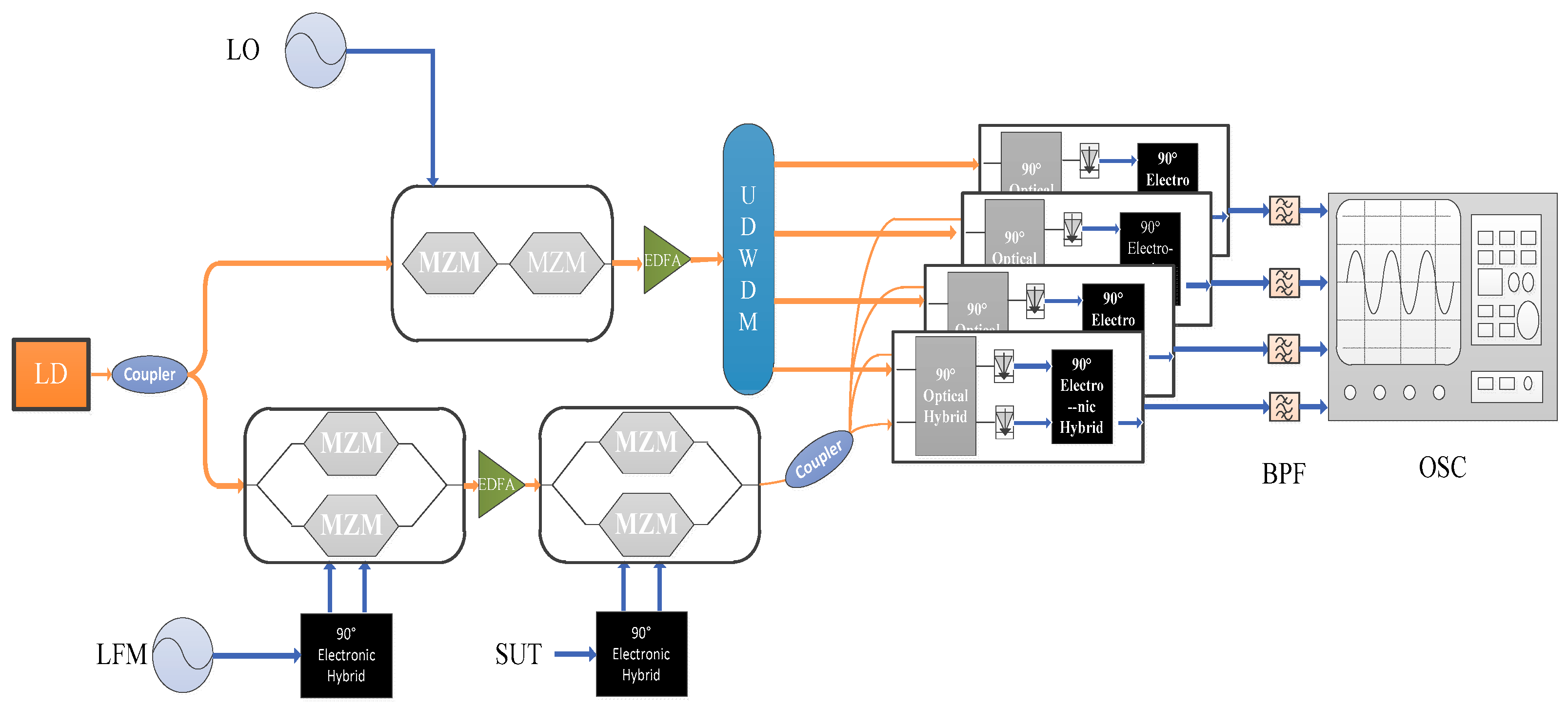
2. Principle
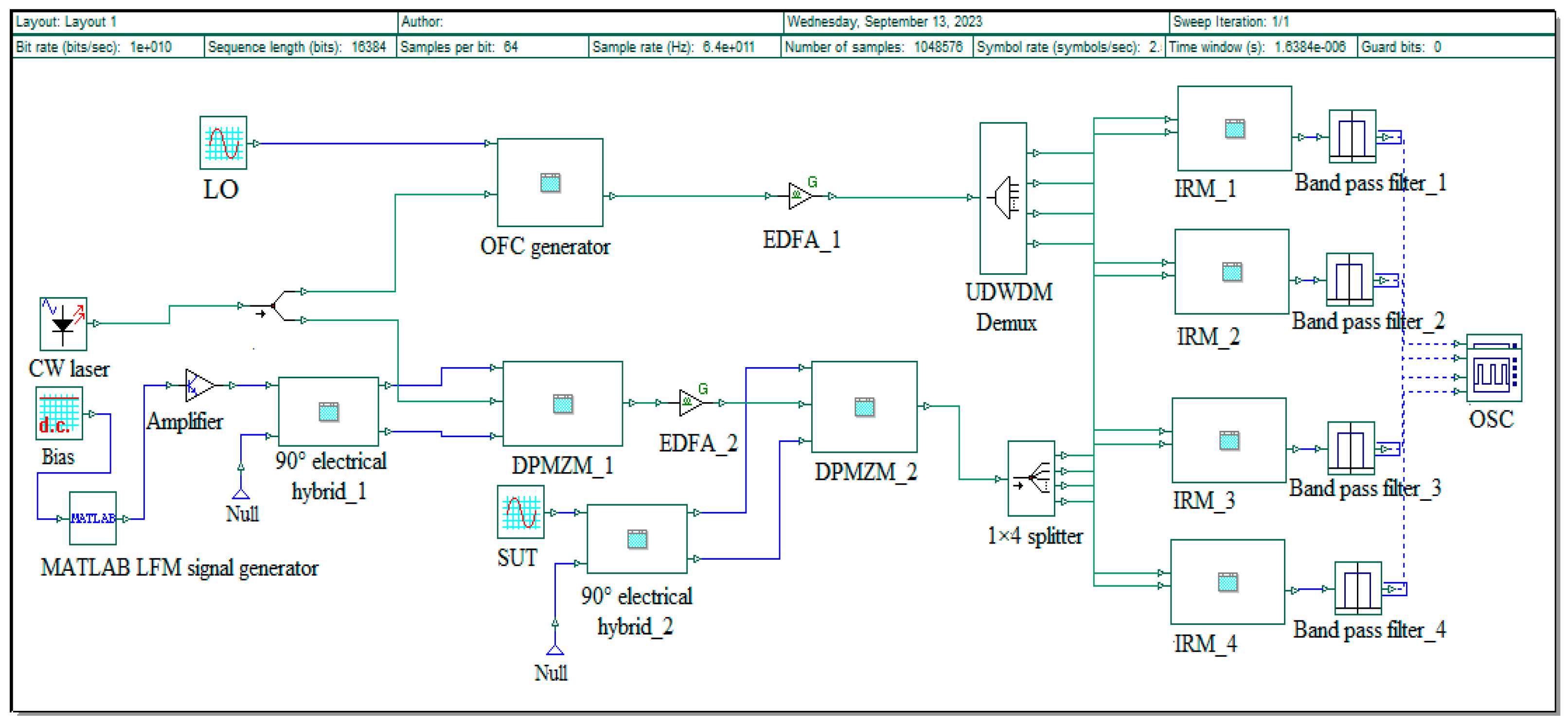
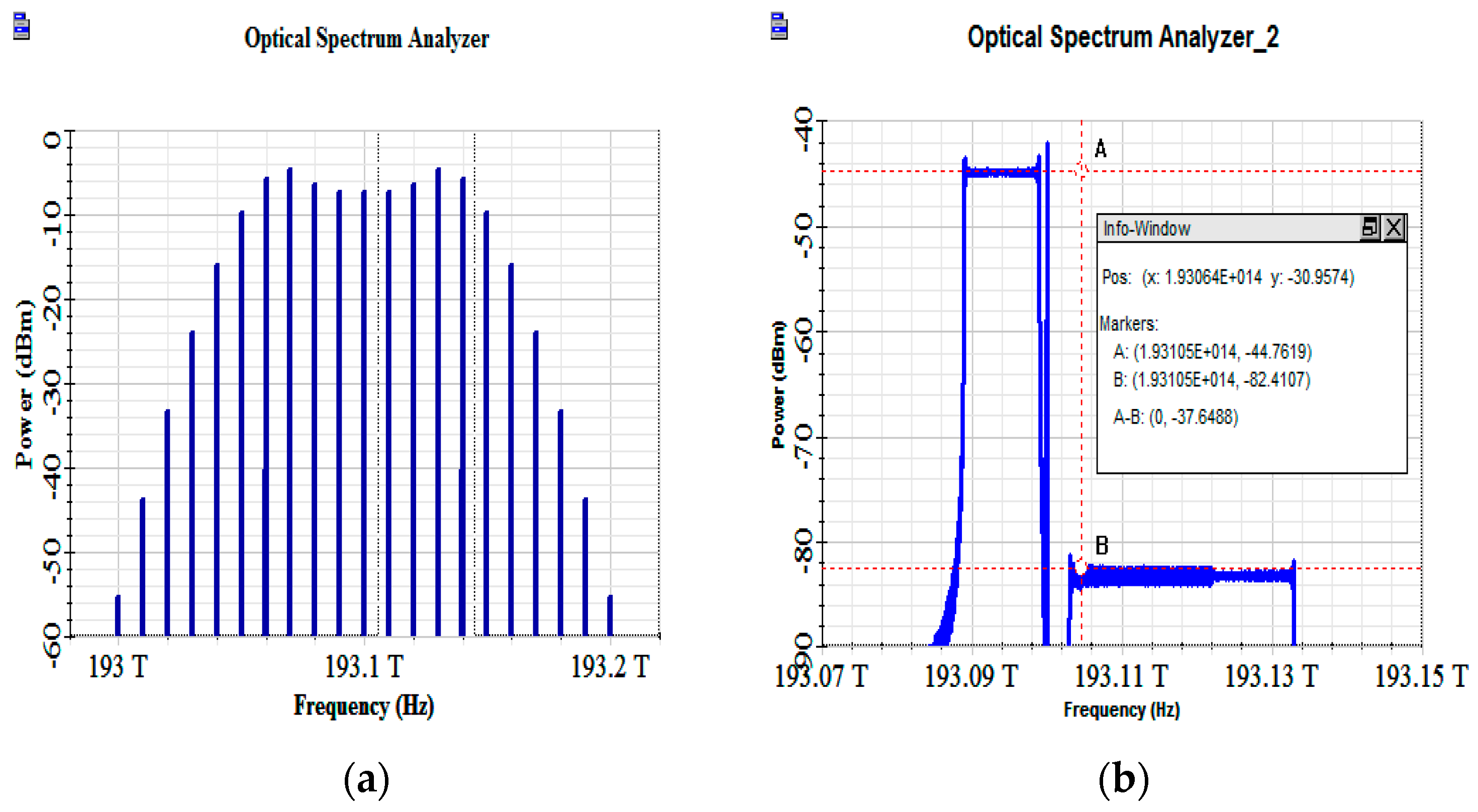
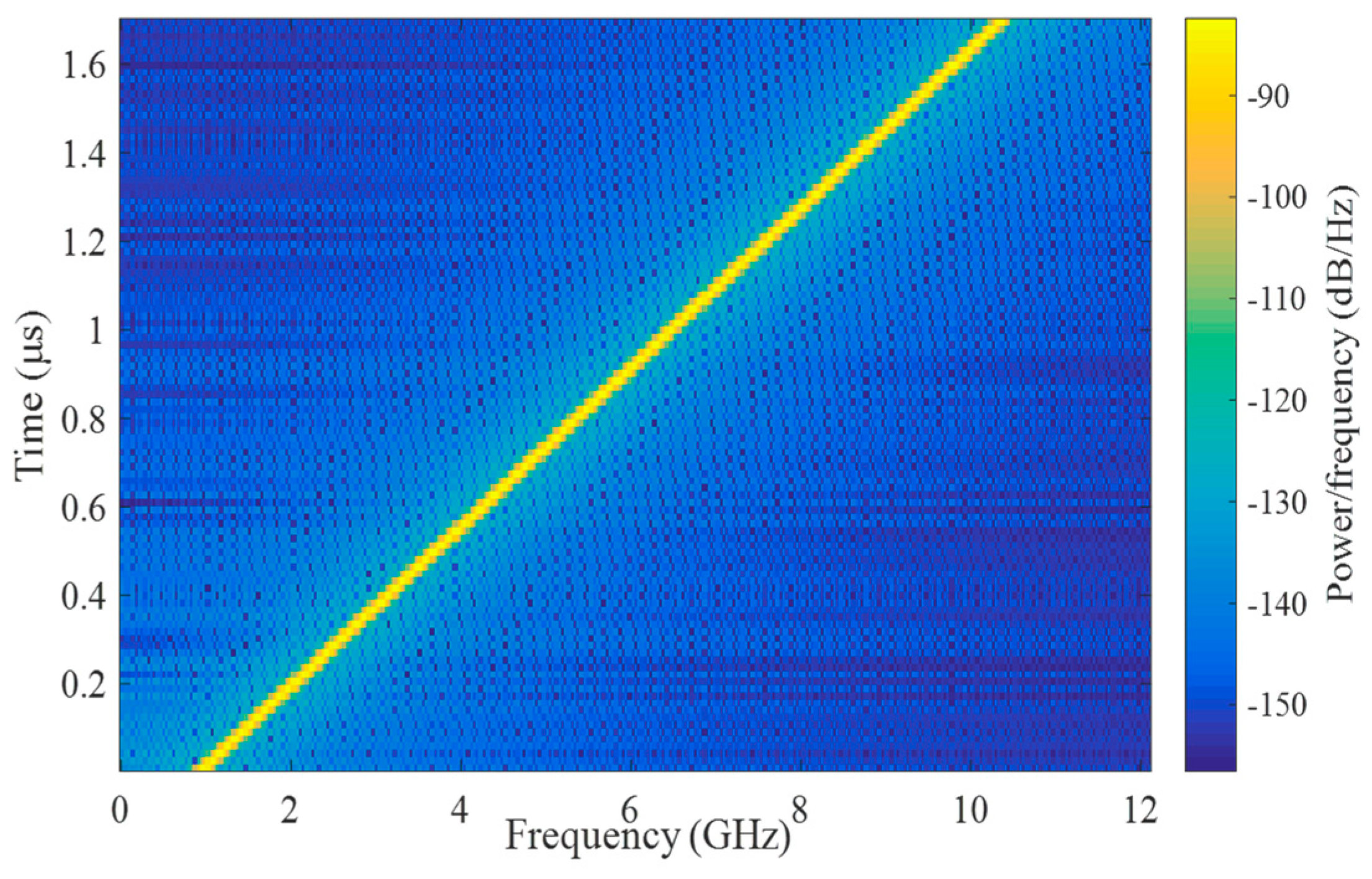
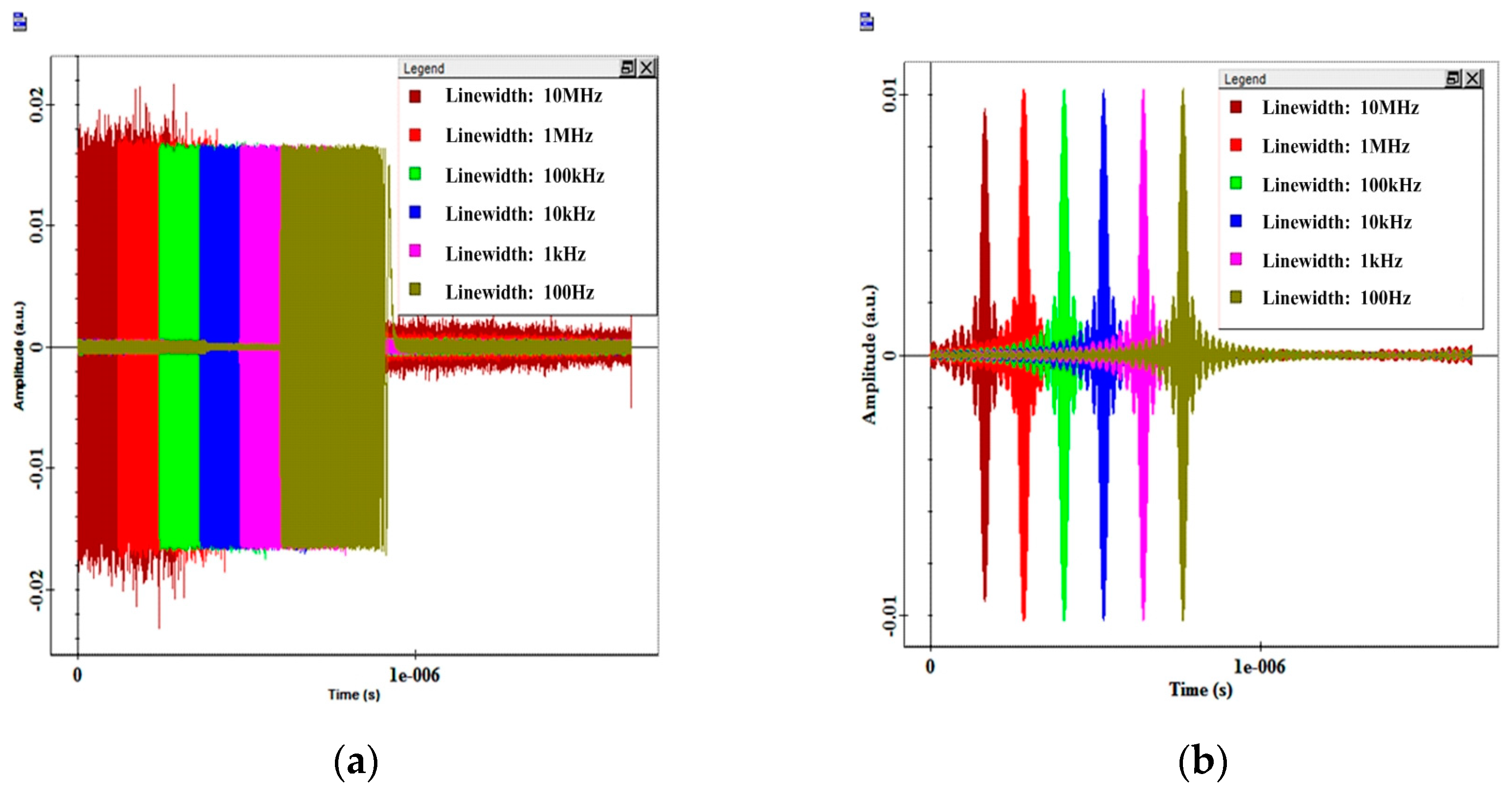
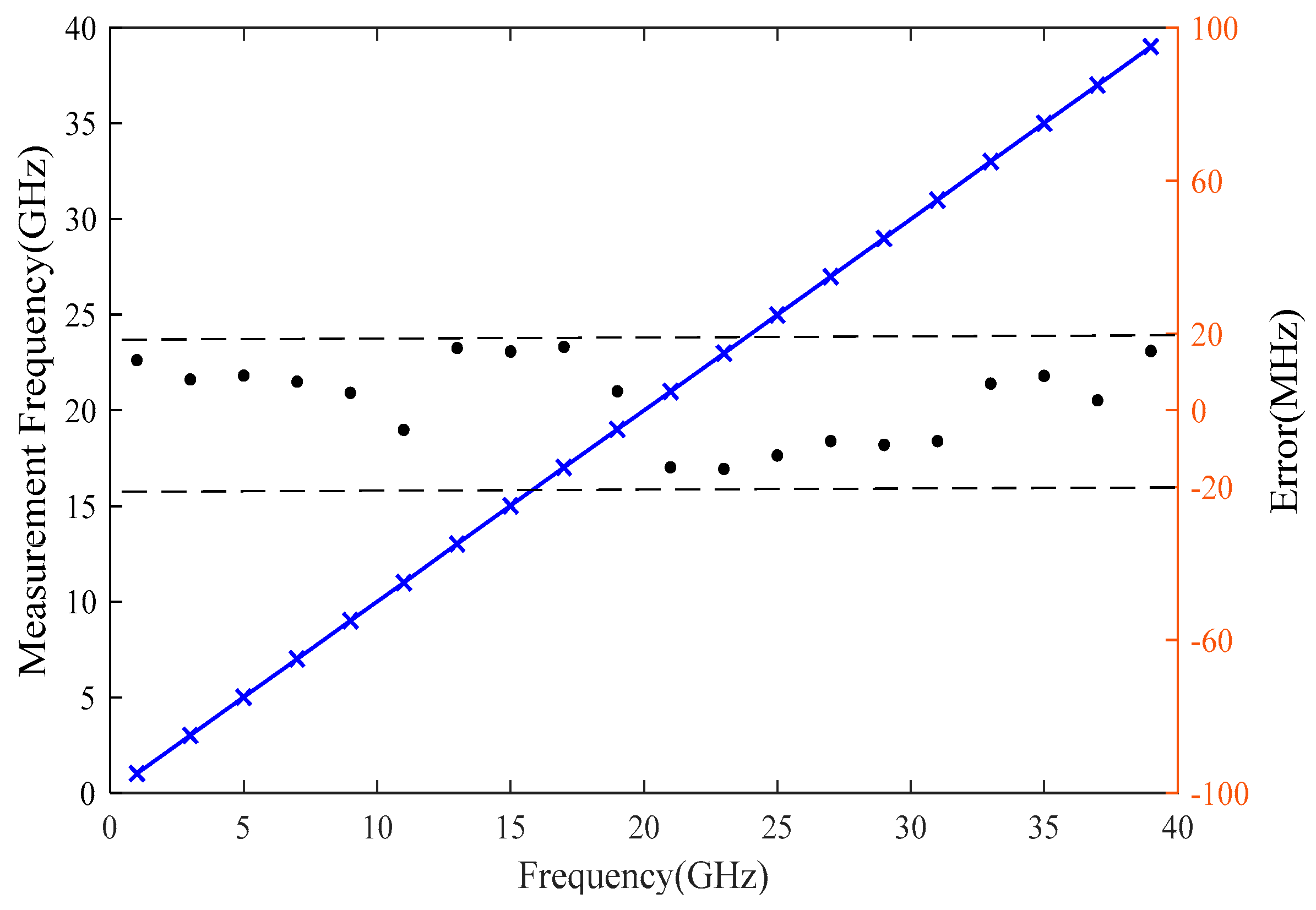
3. Simulation and Experiment
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bui, L.A. Recent Advances in Microwave Photonics Instantaneous Frequency Measurements. Prog. Quantum Electron. 2019, 69, 100237. [Google Scholar] [CrossRef]
- Ivanov, A.; Morozov, O.; Sakhabutdinov, A.; Kuznetsov, A.; Nureev, I. Photonic-Assisted Receivers for Instantaneous Microwave Frequency Measurement Based on Discriminators of Resonance Type. Photonics 2022, 9, 754. [Google Scholar] [CrossRef]
- Yao, J. Microwave Photonic Systems. J. Light. Technol. 2022, 40, 6595–6607. [Google Scholar] [CrossRef]
- Shi, J.; Zhang, F.; Ben, D.; Pan, S. Simultaneous Radar Detection and Frequency Measurement by Broadband Microwave Photonic Processing. J. Light. Technol. 2020, 38, 2171–2179. [Google Scholar] [CrossRef]
- Zhou, P.; Tang, Z.; Zhu, J.; Li, N. Instantaneous Frequency Measurement Using Photonics-Assisted Broadband Signal Generation and Processing. IEEE Microw. Wirel. Technol. Lett. 2023, 33, 619–622. [Google Scholar] [CrossRef]
- Shi, N.; Hao, T.; Li, W.; Li, M. A Compact Multifrequency Measurement System Based on an Integrated Frequency-Scanning Generator. Appl. Sci. 2020, 10, 8571. [Google Scholar] [CrossRef]
- Wang, D.; Xu, K.; Dai, J.; Wu, Z.; Ji, Y.; Lin, J. Photonic-Assisted Approach for Instantaneous Microwave Frequency Measurement with Tunable Range by Using Mach-Zehnder Interferometers. Chin. Opt. Lett. 2013, 11, 020604. [Google Scholar] [CrossRef]
- Zhu, W.; Li, J.; Yan, M.; Pei, L.; Ning, T.; Zheng, J.; Wang, J. Photonic Multiple Microwave Frequency Measurement System with Single-Branch Detection Based on Polarization Interference. Electronics 2023, 12, 455. [Google Scholar] [CrossRef]
- Pan, S.; Fu, J.; Yao, J. Photonic Approach to the Simultaneous Measurement of the Frequency, Amplitude, Pulse Width, and Time of Arrival of a Microwave Signal. Opt. Lett. 2011, 37, 7–9. [Google Scholar] [CrossRef][Green Version]
- Zou, X.; Pan, W.; Luo, B.; Yan, L. Photonic Instantaneous Frequency Measurement Using a Single Laser Source and Two Quadrature Optical Filters. IEEE Photonics Technol. Lett. 2011, 23, 39–41. [Google Scholar] [CrossRef]
- Chi, H.; Zou, X.; Yao, J. An Approach to the Measurement of Microwave Frequency Based on Optical Power Monitoring. IEEE Photonics Technol. Lett. 2008, 20, 1249–1251. [Google Scholar] [CrossRef]
- Zou, X.; Yao, J. An Optical Approach to Microwave Frequency Measurement with Adjustable Measurement Range and Resolution. IEEE Photonics Technol. Lett. 2008, 20, 1989–1991. [Google Scholar] [CrossRef]
- Xu, K.; Dai, J.; Duan, R.; Dai, Y.; Li, Y.; Wu, J.; Lin, J. Instantaneous Microwave Frequency Measurement Based on Phase-Modulated Links with Interferometric Detection. IEEE Photonics Technol. Lett. 2011, 23, 1328–1330. [Google Scholar] [CrossRef]
- Wang, C.; Yao, J. Ultrahigh-Resolution Photonic-Assisted Microwave Frequency Identification Based on Temporal Channelization. IEEE Trans. Microw. Theory Tech. 2013, 61, 4275–4282. [Google Scholar] [CrossRef]
- Huang, C.; Erwin; Hao, P. Frequency-To-Space Mapping Based Instantaneous Frequency Measurement System with Improved Accuracy and Resolution. In Proceedings of the 2023 International Topical Meeting on Microwave Photonics, Nanjing, China, 15–18 October 2023. [Google Scholar] [CrossRef]
- Zhou, F.; Chen, H.; Wang, X.; Zhou, L.; Dong, J.; Zhang, X. Photonic Multiple Microwave Frequency Measurement Based on Frequency-To-Time Mapping. IEEE Photonics J. 2018, 10, 5500807. [Google Scholar] [CrossRef]
- Zhang, B.; Zhu, D.; Lei, Z.; Xu, Z.; Zhou, T.; Zhong, X.; Chen, Z.; Pan, S. Impact of Dispersion Effects on Temporal-Convolution-Based Real-Time Fourier Transformation Systems. J. Light. Technol. 2020, 38, 4664–4676. [Google Scholar] [CrossRef]
- Hugues; Cortés, L.R.; Azaña, J. Optical Real-Time Fourier Transformation with Kilohertz Resolutions. Optica 2015, 3, 1–8. [Google Scholar] [CrossRef]
- Duan, Y.; Chen, L.; Zhou, H.; Zhou, X.; Zhang, C.; Zhang, X. Ultrafast Electrical Spectrum Analyzer Based on All-Optical Fourier Transform and Temporal Magnification. Opt. Express 2017, 25, 7520–7529. [Google Scholar] [CrossRef]
- Yang, H.; Brunel, M.; Vallet, M.; Zhang, H.; Zhao, C. Optical Frequency-To-Time Mapping Using a Phase-Modulated Frequency-Shifting Loop. Opt. Lett. 2021, 46, 2336–2339. [Google Scholar] [CrossRef]
- Shi, J.; Zhang, F.; Ben, D.; Pan, S. Photonics-Based Broadband Microwave Instantaneous Frequency Measurement by Frequency-To-Phase-Slope Mapping. IEEE Trans. Microw. Theory Tech. 2019, 67, 544–552. [Google Scholar] [CrossRef]
- Nguyen, L. Microwave Photonic Technique for Frequency Measurement of Simultaneous Signals. IEEE Photonics Technol. Lett. 2009, 21, 642–644. [Google Scholar] [CrossRef]
- Foster, M.A.; Salem, R.; Geraghty, D.F.; Turner-Foster, A.C.; Lipson, M.; Gaeta, A.L. Silicon-Chip-Based Ultrafast Optical Oscilloscope. Nature 2008, 456, 81–84. [Google Scholar] [CrossRef] [PubMed]
- Xi, K.; Yu, Y.; Tang, H.-T.; Zhang, C. Microwave Photonic Image-Reject Mixer Based on a Tunable Microwave Photonic Filter with High Rejection. IEEE Photonics J. 2018, 10, 5502411. [Google Scholar] [CrossRef]



| Device | Parameter | Title 4 |
|---|---|---|
| Laser | Linewidth | 10 kHz |
| Average power | 10 dBm | |
| Frequency | 193.1 THz | |
| LO | Frequency | 10 GHz |
| Power | 25 dBm | |
| MZM | Half-wave voltage | 4 V |
| Insertion loss | 5 dB | |
| Extinction ratio | 35 dB | |
| UDWDM | FSR | 10 GHz |
| Bandwidth | 4 GHz | |
| Insertion loss | 4 dB | |
| EDFA | Gain | 30 dB |
| NF | 3 dB | |
| PD | Responsivity | 0.65 W/A |
| BPF | Central frequency | 1 GHz |
| Bandwidth | 20 MHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Liu, A.; Ma, X.; Yu, W.; Liu, Y.; Li, Y.; Xing, G. Broadband Instantaneous Frequency Measurement Using Frequency-to-Time Mapping and Channelization. Photonics 2024, 11, 697. https://doi.org/10.3390/photonics11080697
Li S, Liu A, Ma X, Yu W, Liu Y, Li Y, Xing G. Broadband Instantaneous Frequency Measurement Using Frequency-to-Time Mapping and Channelization. Photonics. 2024; 11(8):697. https://doi.org/10.3390/photonics11080697
Chicago/Turabian StyleLi, Shaobo, Anni Liu, Xiang Ma, Wenqi Yu, Yandan Liu, Yihan Li, and Guansu Xing. 2024. "Broadband Instantaneous Frequency Measurement Using Frequency-to-Time Mapping and Channelization" Photonics 11, no. 8: 697. https://doi.org/10.3390/photonics11080697
APA StyleLi, S., Liu, A., Ma, X., Yu, W., Liu, Y., Li, Y., & Xing, G. (2024). Broadband Instantaneous Frequency Measurement Using Frequency-to-Time Mapping and Channelization. Photonics, 11(8), 697. https://doi.org/10.3390/photonics11080697




