Research on Distortion Control in Off-Axis Three-Mirror Astronomical Telescope Systems
Abstract
1. Introduction
2. Methods
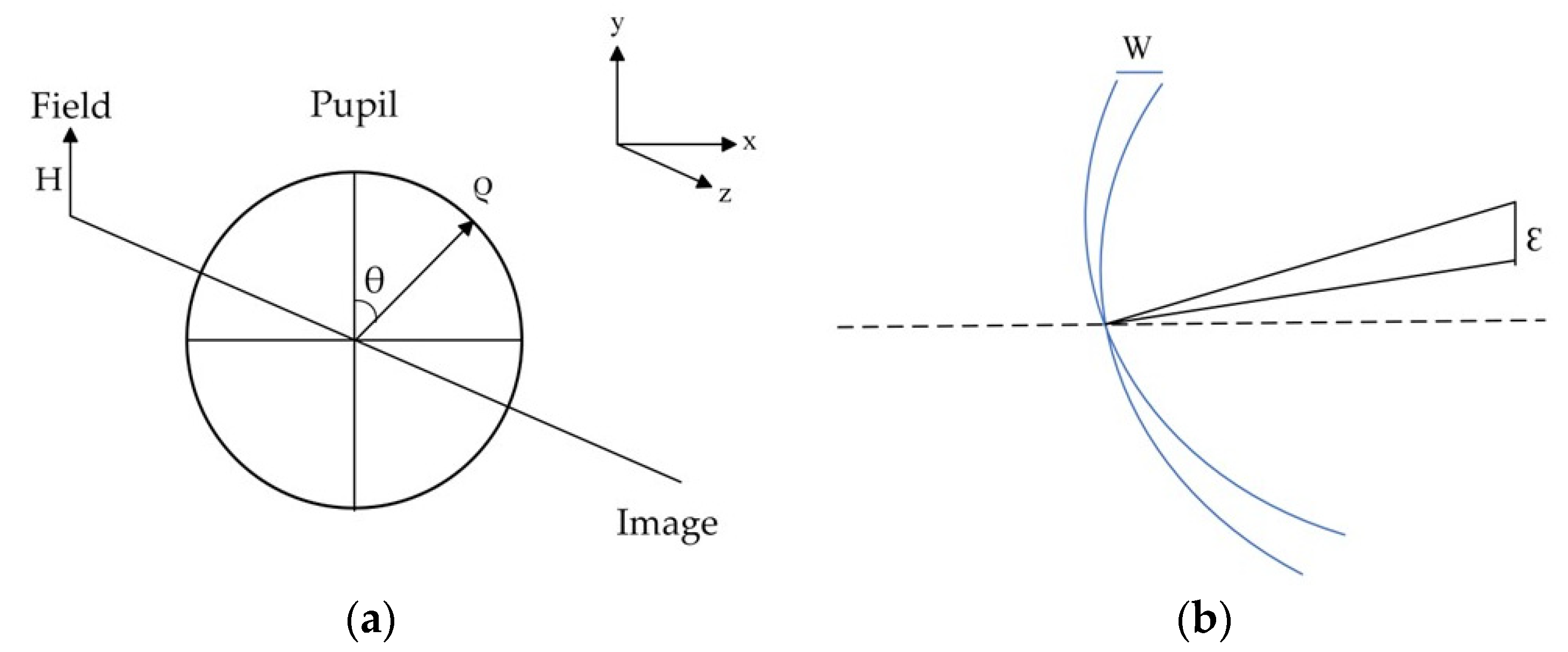
2.1. Analysis of Third-Order Distortion Characteristics
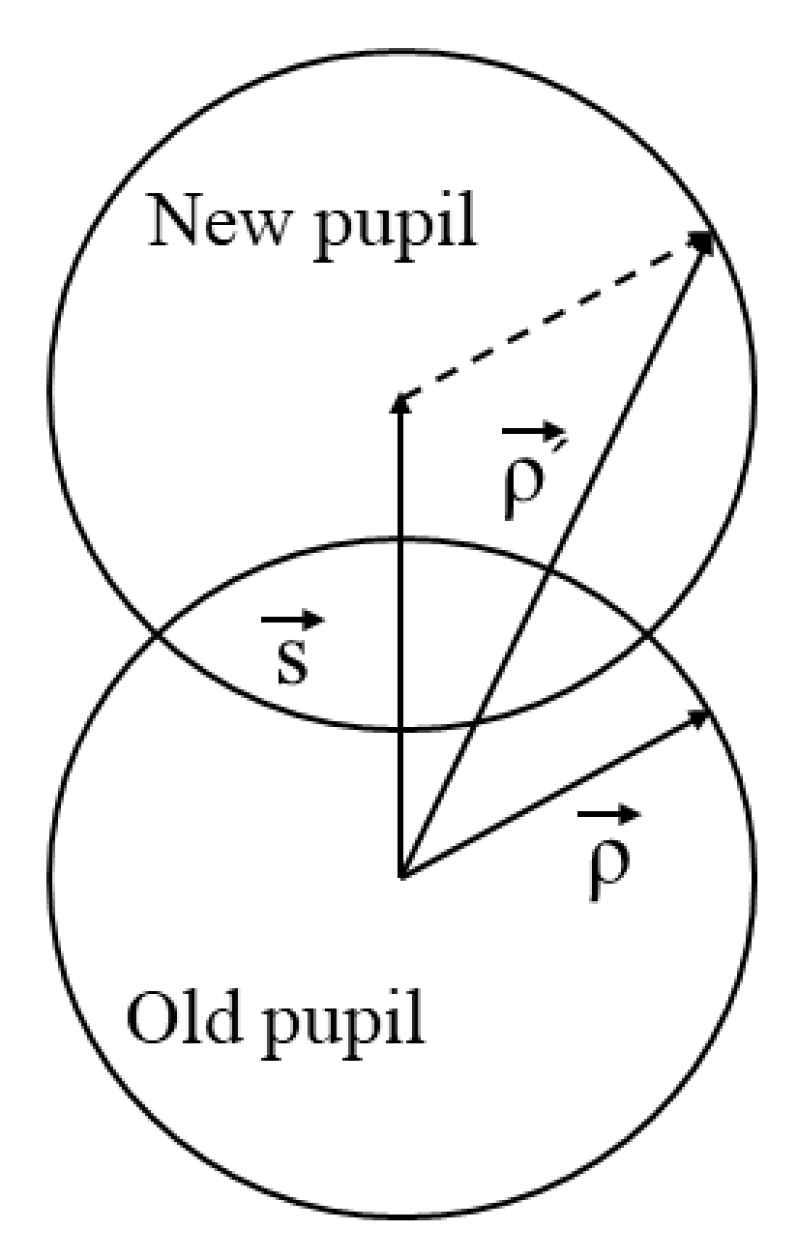
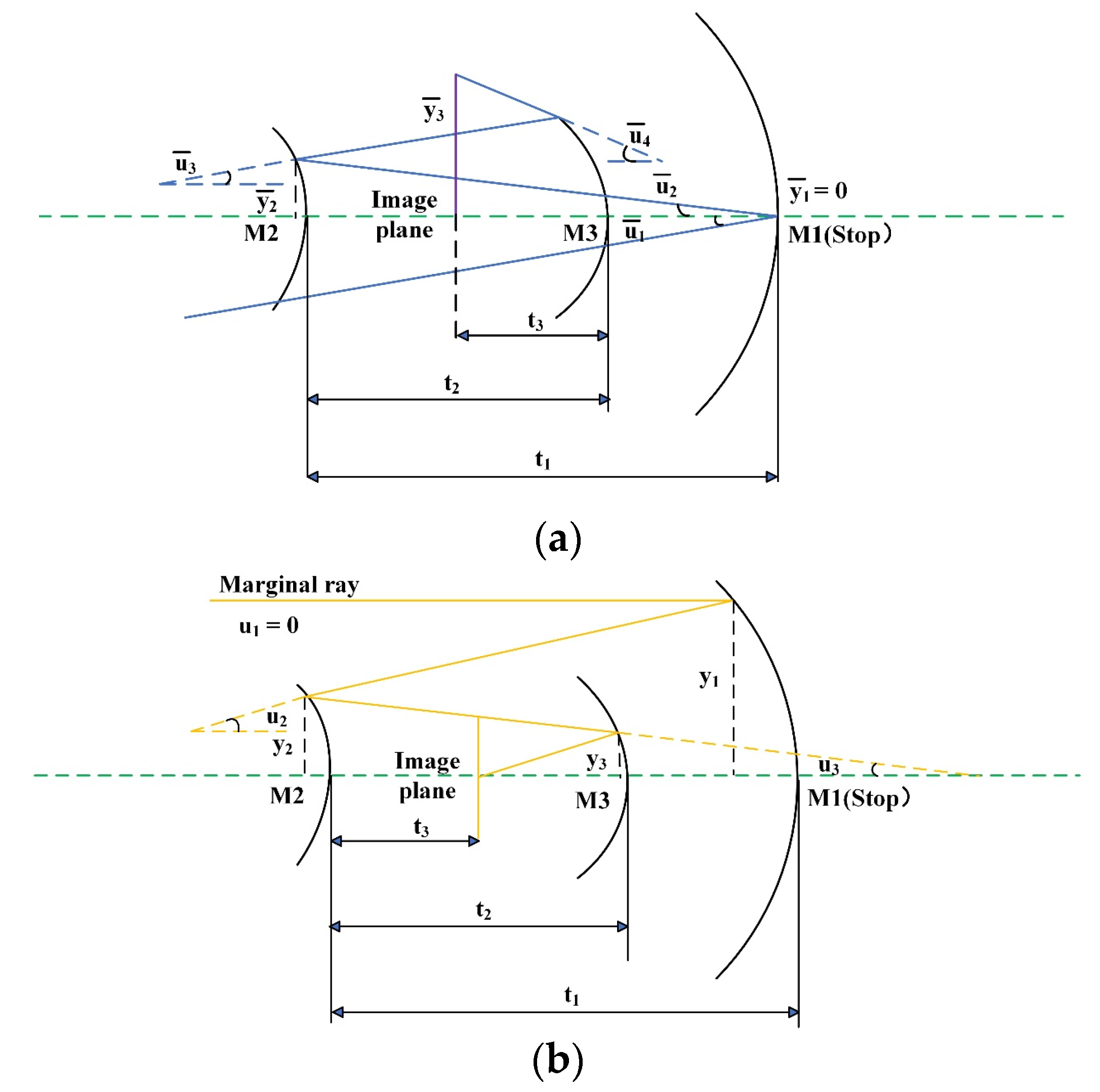
2.2. Calculating Seidel Aberrations
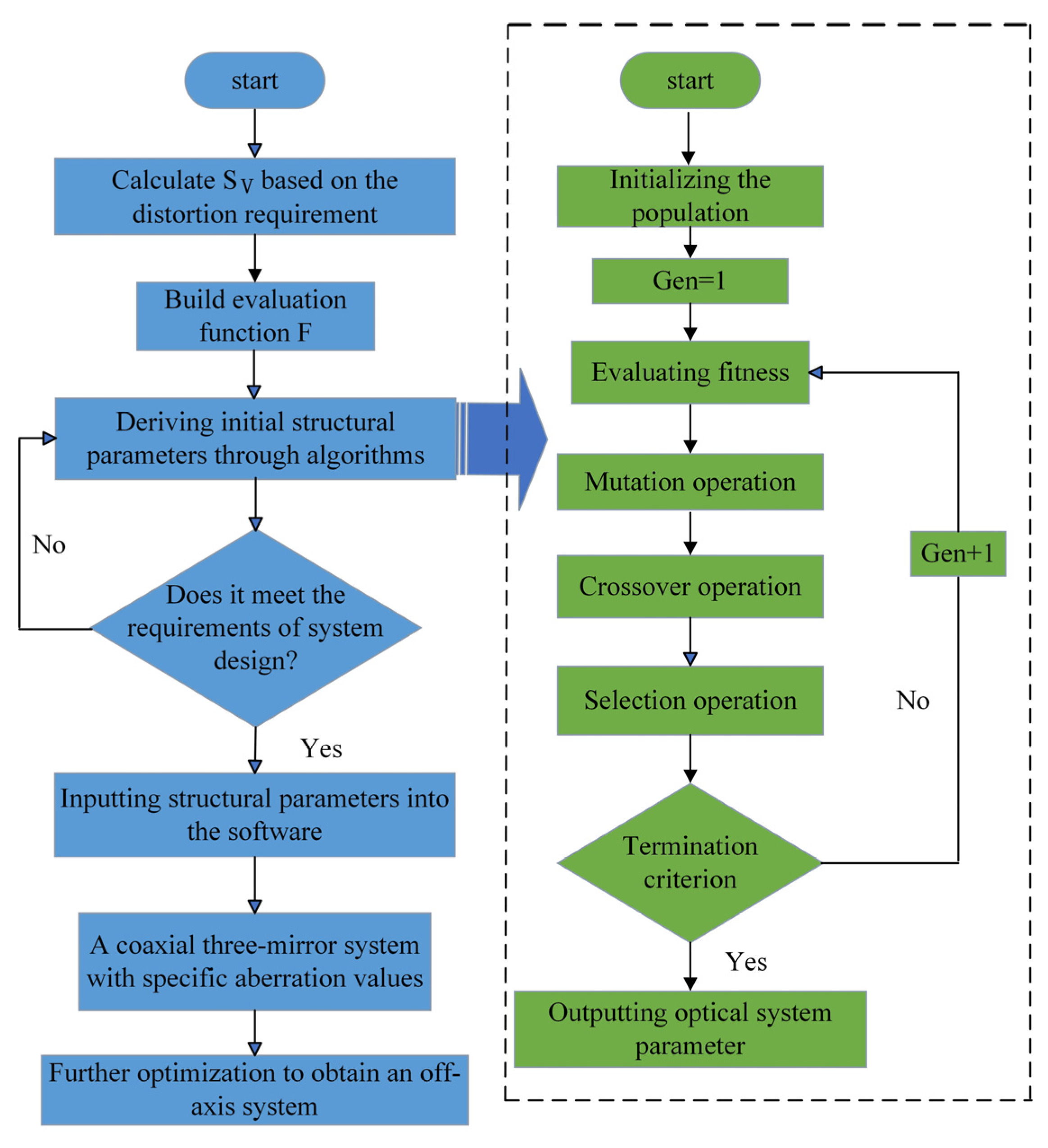
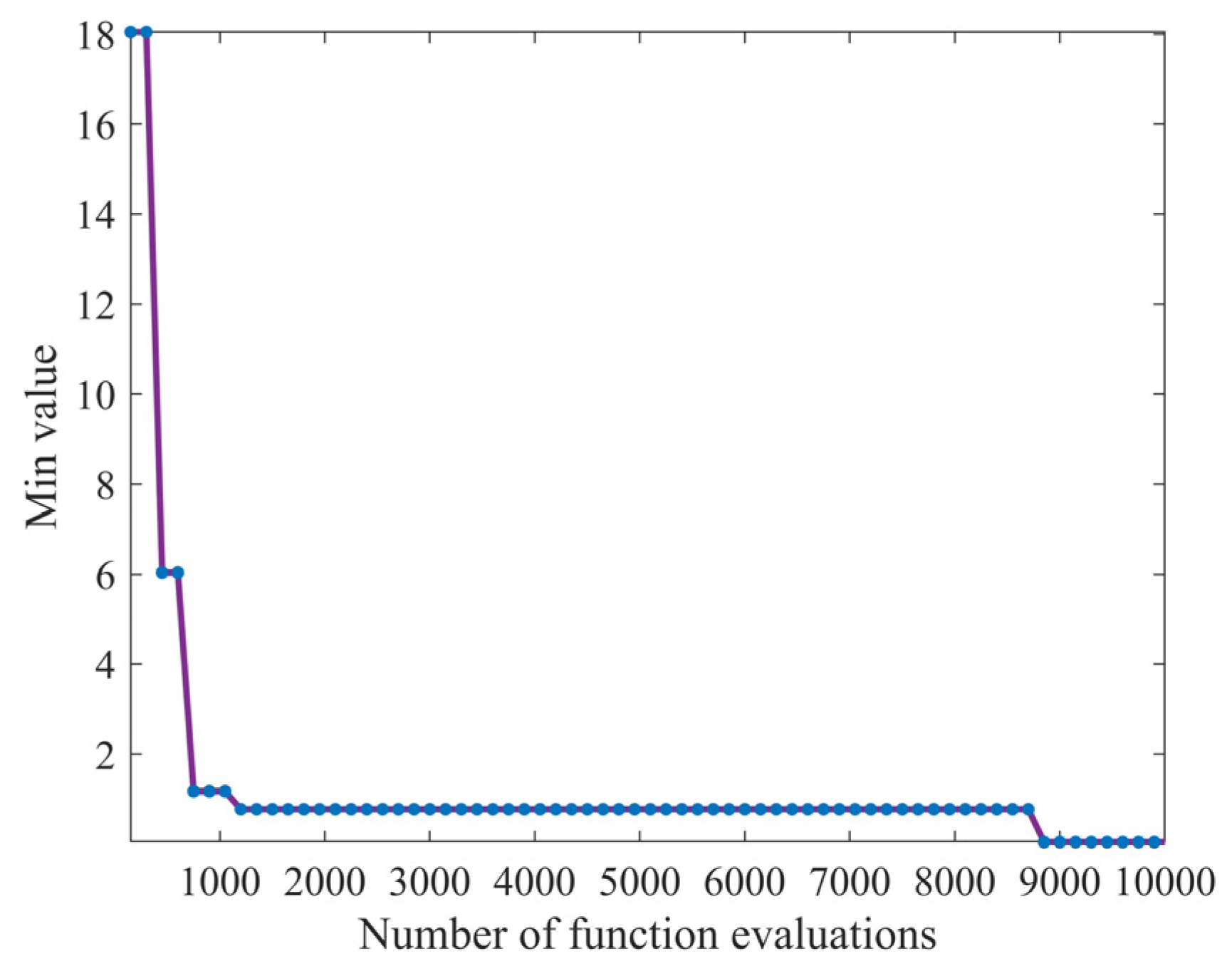
2.3. Using the Differential Evolution Algorithm to Search for the Initial Structure
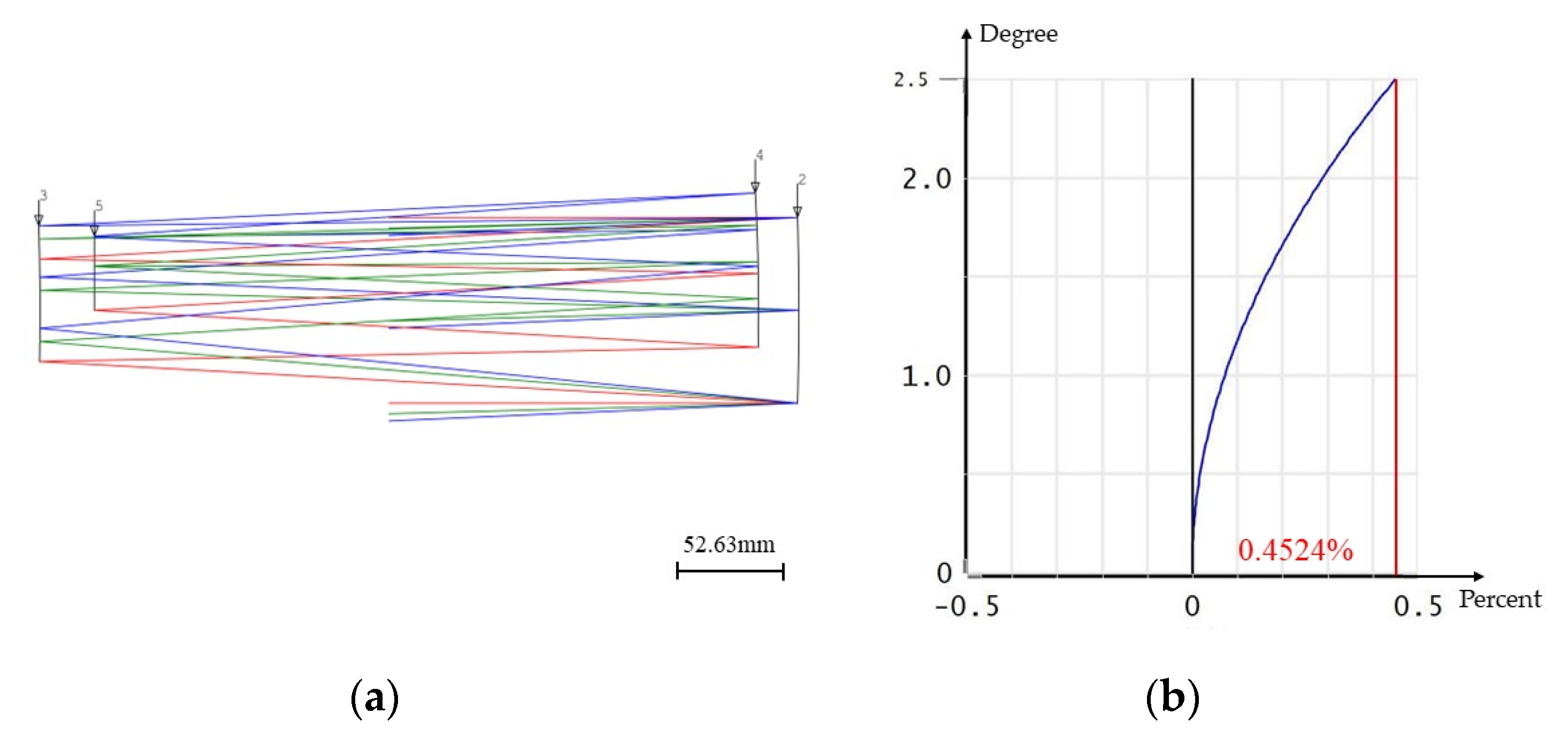
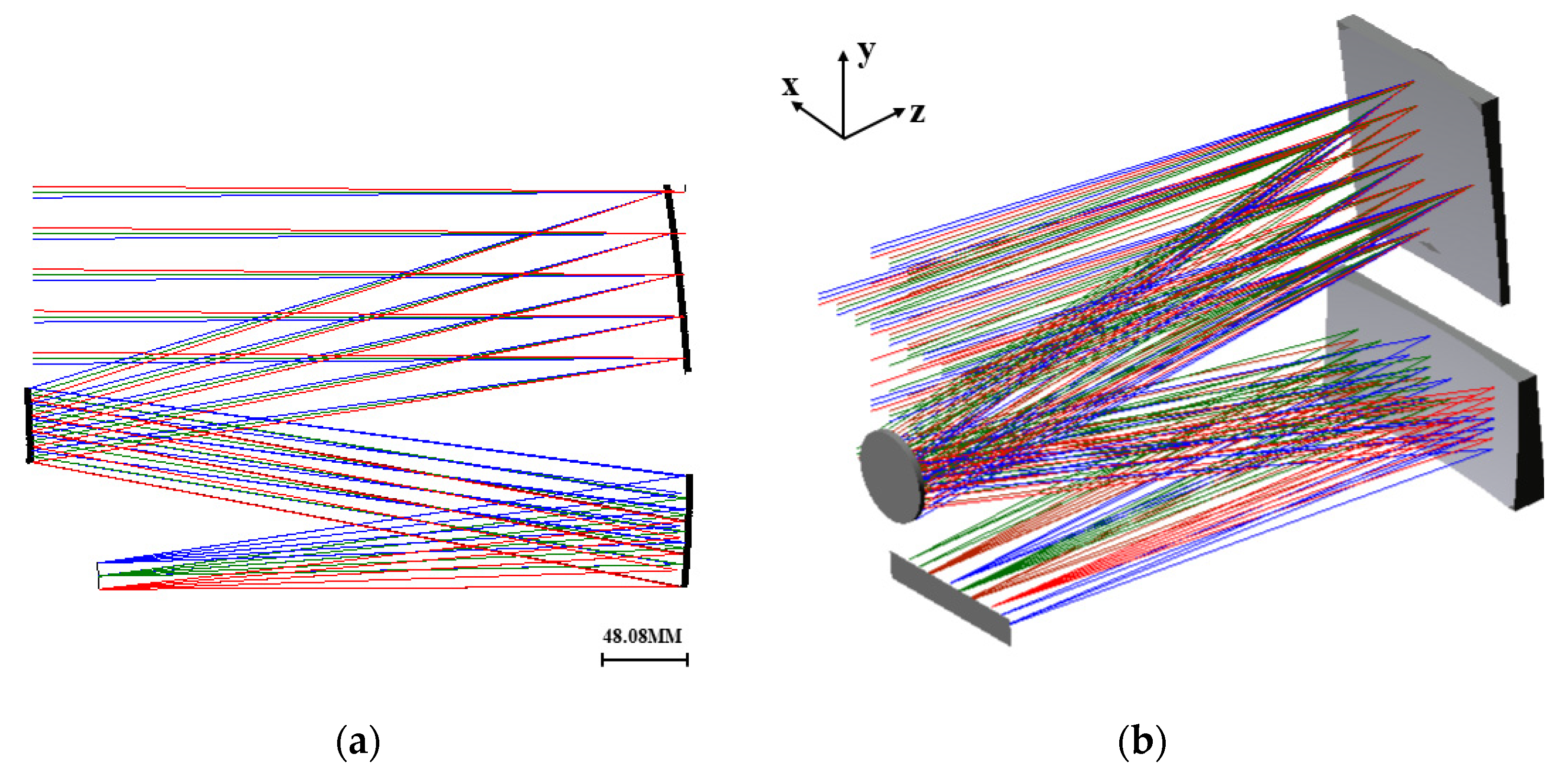
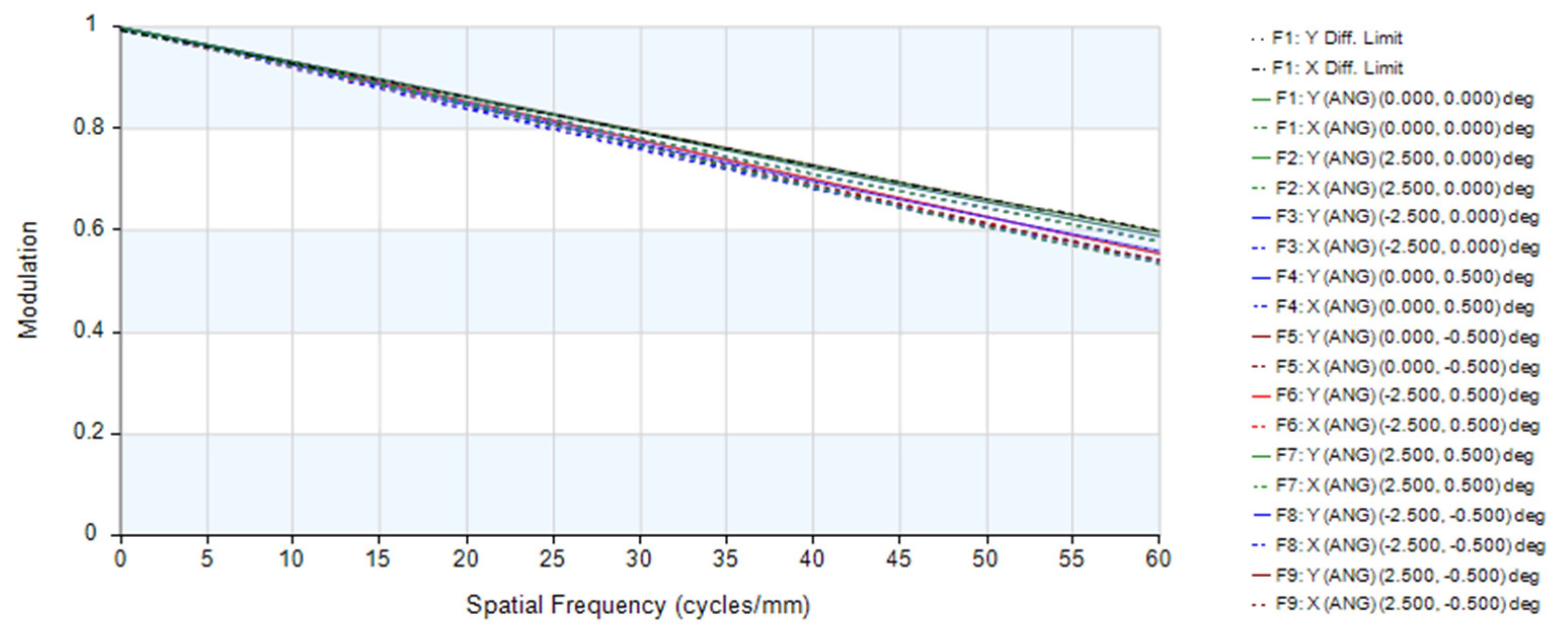
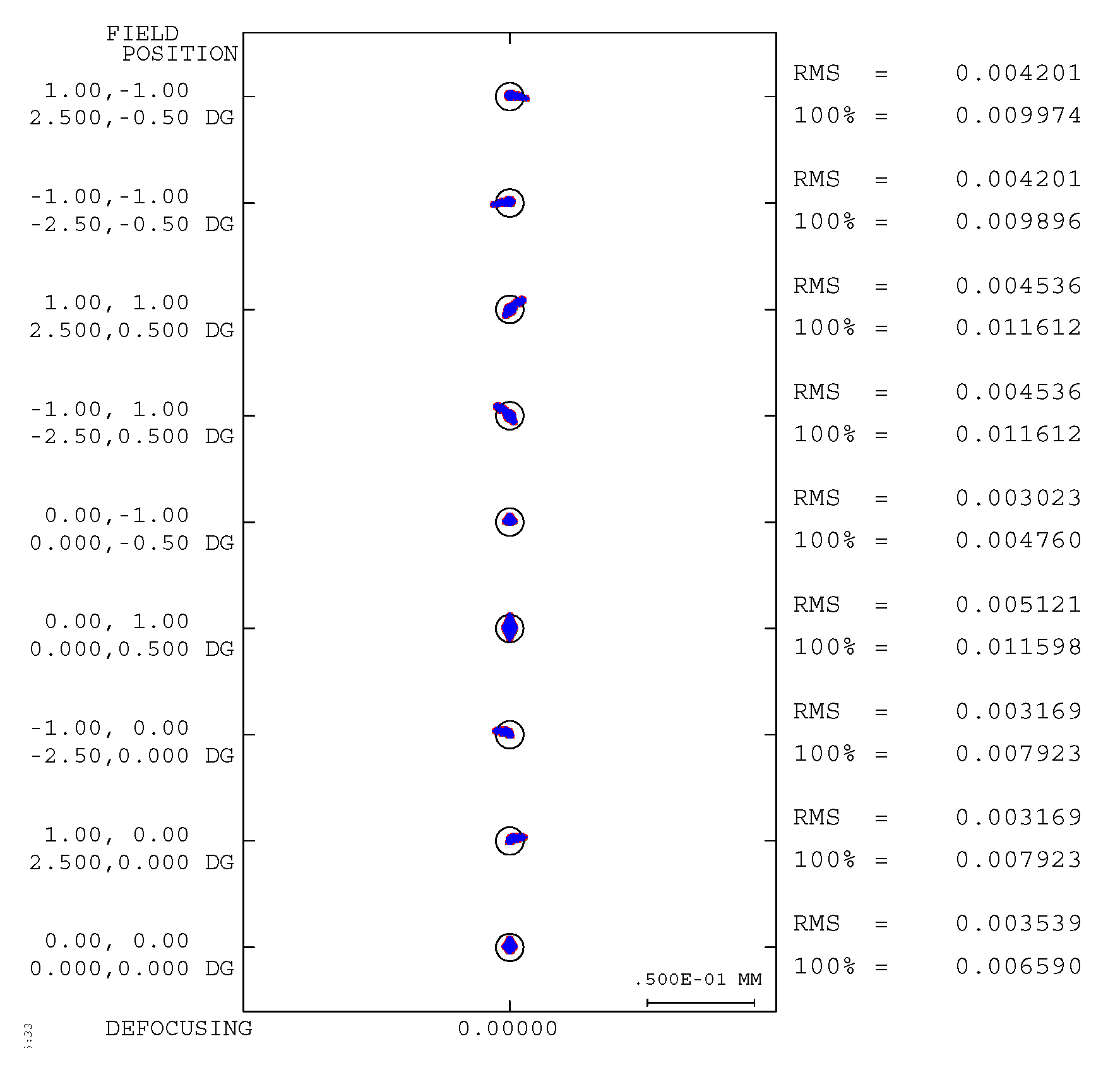
3. Design Example
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, Y.; Bauer, A.; Viard, T.; Rolland, J.P. Freeform hyperspectral imager design in a CubeSat format. Opt. Express 2021, 29, 35915–35928. [Google Scholar] [CrossRef] [PubMed]
- Stuart, M.B.; McGonigle, A.J.S.; Willmott, J.R. Hyperspectral Imaging in Environmental Monitoring: A Review of Recent Developments and Technological Advances in Compact Field Deployable Systems. Sensors 2019, 19, 3071. [Google Scholar] [CrossRef] [PubMed]
- Meng, Q.; Wang, W.; Ma, H.; Dong, J. Easy-aligned off-axis three-mirror system with wide field of view using freeform surface based on integration of primary and tertiary mirror. Appl. Opt. 2014, 53, 3028–3034. [Google Scholar] [CrossRef] [PubMed]
- Qu, Z.; Zhong, X.; Zhang, K.; Wang, Y.; Li, L.; Liu, J.; Zeng, C. Automatic compact-volume design strategy for unobscured reflective optical systems based on conicoid surfaces. Opt. Commun. 2023, 533, 129304. [Google Scholar] [CrossRef]
- Sasian, J.; Reshidko, D. Method for the design of nonaxially symmetric optical systems using free-form surfaces. Opt. Eng. 2018, 57, 101704. [Google Scholar] [CrossRef]
- Shi, H.; Jiang, H.; Zhang, X.; Wang, C.; Liu, T. Analysis of nodal aberration properties in off-axis freeform system design. Appl. Opt. 2016, 55, 6782–6790. [Google Scholar] [CrossRef] [PubMed]
- Xu, C.; Lai, X.; Cheng, D.; Wang, Y.; Wu, K. Automatic optical path configuration variation in off-axis mirror system design. Opt. Express 2019, 27, 15251–15261. [Google Scholar] [CrossRef] [PubMed]
- Zhong, Y.; Gross, H. Initial system design method for non-rotationally symmetric systems based on Gaussian brackets and Nodal aberration theory. Opt. Express 2017, 25, 10016–10030. [Google Scholar] [CrossRef] [PubMed]
- Allain, G.; Thibault, S.; Johnson, R.B.; Mahajan, V.N.; Thibault, S. Distortion controlled optical design using orthogonal surface polynomials. In Proceedings of the Current Developments in Lens Design and Optical Engineering XXI, Online, 24 August–4 September 2020. [Google Scholar]
- Thomas, G.C.; Lacy, G.C. Design concept for a Landsat-class imaging spectrometer with well-corrected spectral fidelity. In Proceedings of the Optical Science and Technology, SPIE’s 48th Annual Meeting, San Diego, CA, USA, 3–8 August 2003; pp. 90–97. [Google Scholar]
- Meng, Q.; Wang, H.; Liang, W.; Yan, Z.; Wang, B. Design of off-axis three-mirror systems with ultrawide field of view based on an expansion process of surface freeform and field of view. Appl. Opt. 2019, 58, 609–615. [Google Scholar] [CrossRef] [PubMed]
- Cao, C.; Liao, S.; Liao, Z.; Bai, Y.; Fan, Z. Initial configuration design method for off-axis reflective optical systems using nodal aberration theory and genetic algorithm. Opt. Eng. 2019, 58, 105101. [Google Scholar] [CrossRef]
- Bakic, S.; Vasiljevic, D. Optimization of the double-Gauss objective with the various evolution strategies and the damped least squares. Phys. Scr. 2014, T162, 014034. [Google Scholar] [CrossRef]
- Cheng, X.; Wang, Y.; Hao, Q.; Isshiki, M. Global and local optimization for optical systems. Optik 2006, 117, 111–117. [Google Scholar] [CrossRef]
- Mouroulis, P.; Green, R.O.; Chrien, T.G. Design of pushbroom imaging spectrometers for optimum recovery of spectroscopic and spatial information. Appl. Opt. 2000, 39, 2210–2220. [Google Scholar] [CrossRef] [PubMed]
- Available online: http://www.opticalres.com (accessed on 21 April 2024).
- Lin, P.D. Seidel primary ray aberration coefficients for objects placed at finite and infinite distances. Opt. Express 2020, 28, 12740–12754. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Guo, B.; Sun, Q.; Lu, Z. Third-order aberration fields of pupil decentered optical systems. Opt. Express 2012, 20, 11652–11658. [Google Scholar] [CrossRef] [PubMed]
- Bilal; Pant, M.; Zaheer, H.; Garcia-Hernandez, L.; Abraham, A. Differential Evolution: A review of more than two decades of research. Eng. Appl. Artif. Intell. 2020, 90, 103479. [Google Scholar] [CrossRef]
- Gui, L.; Xia, X.; Yu, F.; Wu, H.; Wu, R.; Wei, B.; Zhang, Y.; Li, X.; He, G. A multi-role based differential evolution. Swarm Evol. Comput. 2019, 50, 100508. [Google Scholar] [CrossRef]




| Name | Technical Target |
|---|---|
| Focal length/mm | 900 |
| Entrance pupil diameter/mm | 100 |
| Waveband/nm | 486–656 |
| Field of view/° | 5 × 1 |
| Modulation transfer function Distortion/μm | >0.5@50 lp/mm 180/0.46% |
| f/mm | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| −1827.28 | −1590.73 | −1120.78 | −409.14 | 387.45 | −1.91 | −7.16 | −9.41 | −0.02 | 901.80 |
| −2490.75 | 1827.29 | 615.03 | −490.01 | 339.24 | −8.48 | −9.87 | 5.62 | −0.02 | 898.80 |
| −2902.28 | 1762.55 | 619.74 | −459.35 | 390.46 | 2.64 | −7.89 | 5.93 | −0.02 | 905.00 |
| Mirror | r (mm) | d (mm) | Conic |
|---|---|---|---|
| Primary | −1275.00 | −397.00 | −1.17 |
| Secondary | −456.58 | 395.00 | −1.12 |
| Tertiary | −676.73 | −359.98 | −0.005 |
| FOV (Normalization) | Rad Dist% |
|---|---|
| (−1, −1) | 0.46 |
| (−0.8, −1) | 0.38 |
| (−0.6, −1) | 0.32 |
| (−0.4, −1) | 0.28 |
| (−0.2, −1) | 0.25 |
| (0, −1) | 0.22 |
| (0.2, −1) | 0.25 |
| (0.4, −1) | 0.28 |
| (0.6, −1) | 0.32 |
| (0.8, −1) | 0.38 |
| (1, −1) | 0.46 |
| Mirror | R (mm) | D (mm) | Conic |
|---|---|---|---|
| Primary | −1357.63 | −414.10 | −1.34 |
| Secondary | −461.41 | 337.75 | −1.21 |
| Tertiary | −687.63 | −402.00 | −0.08 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, E.; Zheng, Y.; Lin, C.; Zhang, J.; Niu, Y.; Song, L. Research on Distortion Control in Off-Axis Three-Mirror Astronomical Telescope Systems. Photonics 2024, 11, 686. https://doi.org/10.3390/photonics11080686
Liu E, Zheng Y, Lin C, Zhang J, Niu Y, Song L. Research on Distortion Control in Off-Axis Three-Mirror Astronomical Telescope Systems. Photonics. 2024; 11(8):686. https://doi.org/10.3390/photonics11080686
Chicago/Turabian StyleLiu, En, Yuquan Zheng, Chao Lin, Jialun Zhang, Yanlin Niu, and Lei Song. 2024. "Research on Distortion Control in Off-Axis Three-Mirror Astronomical Telescope Systems" Photonics 11, no. 8: 686. https://doi.org/10.3390/photonics11080686
APA StyleLiu, E., Zheng, Y., Lin, C., Zhang, J., Niu, Y., & Song, L. (2024). Research on Distortion Control in Off-Axis Three-Mirror Astronomical Telescope Systems. Photonics, 11(8), 686. https://doi.org/10.3390/photonics11080686