High-Performance Terahertz Coherent Perfect Absorption with Asymmetric Graphene Metasurface
Abstract
1. Introduction
2. CPA Theory and Design of Ultrabroadband CPA with Graphene Metasurfaces

3. Results and Discussion
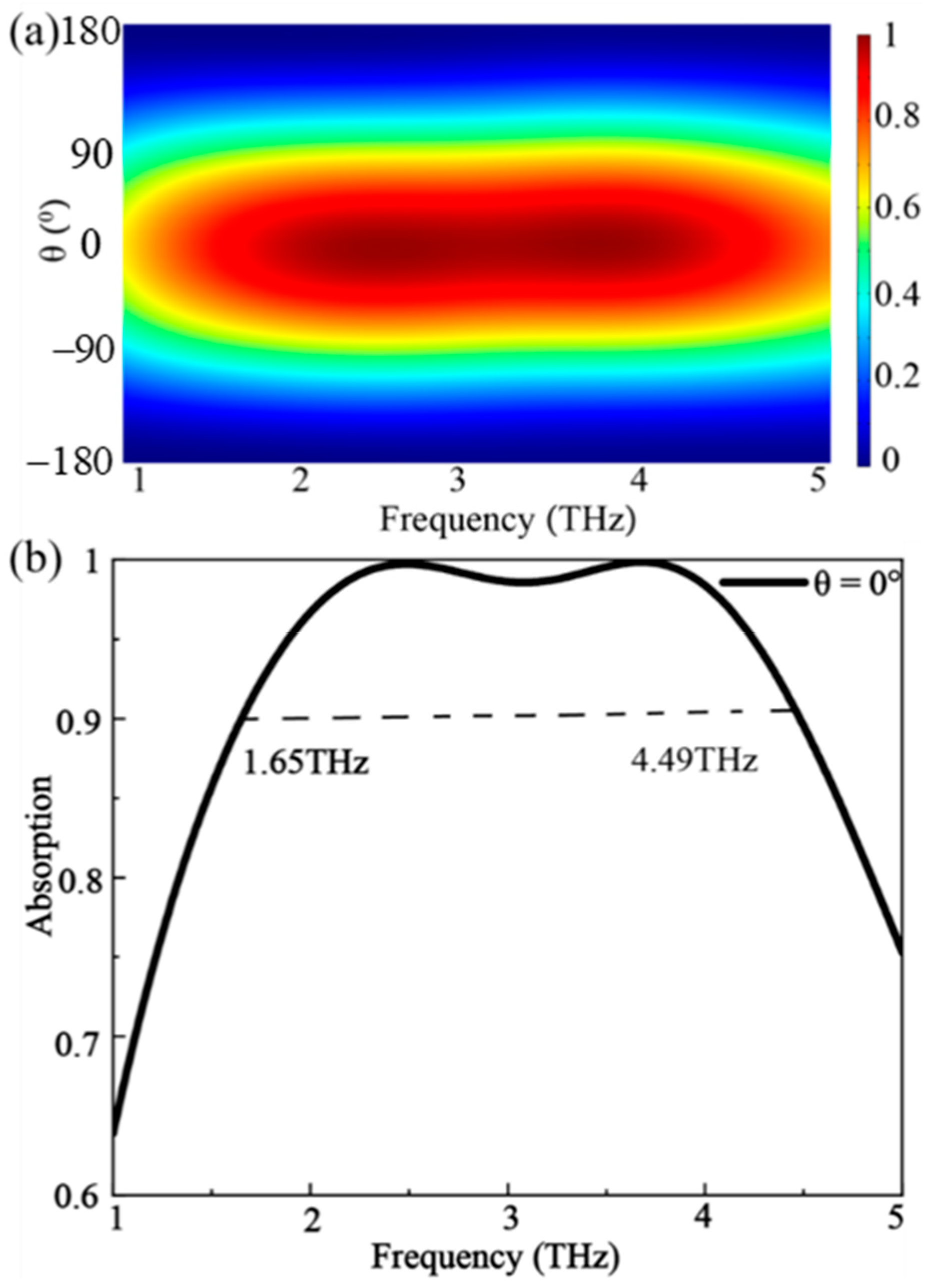
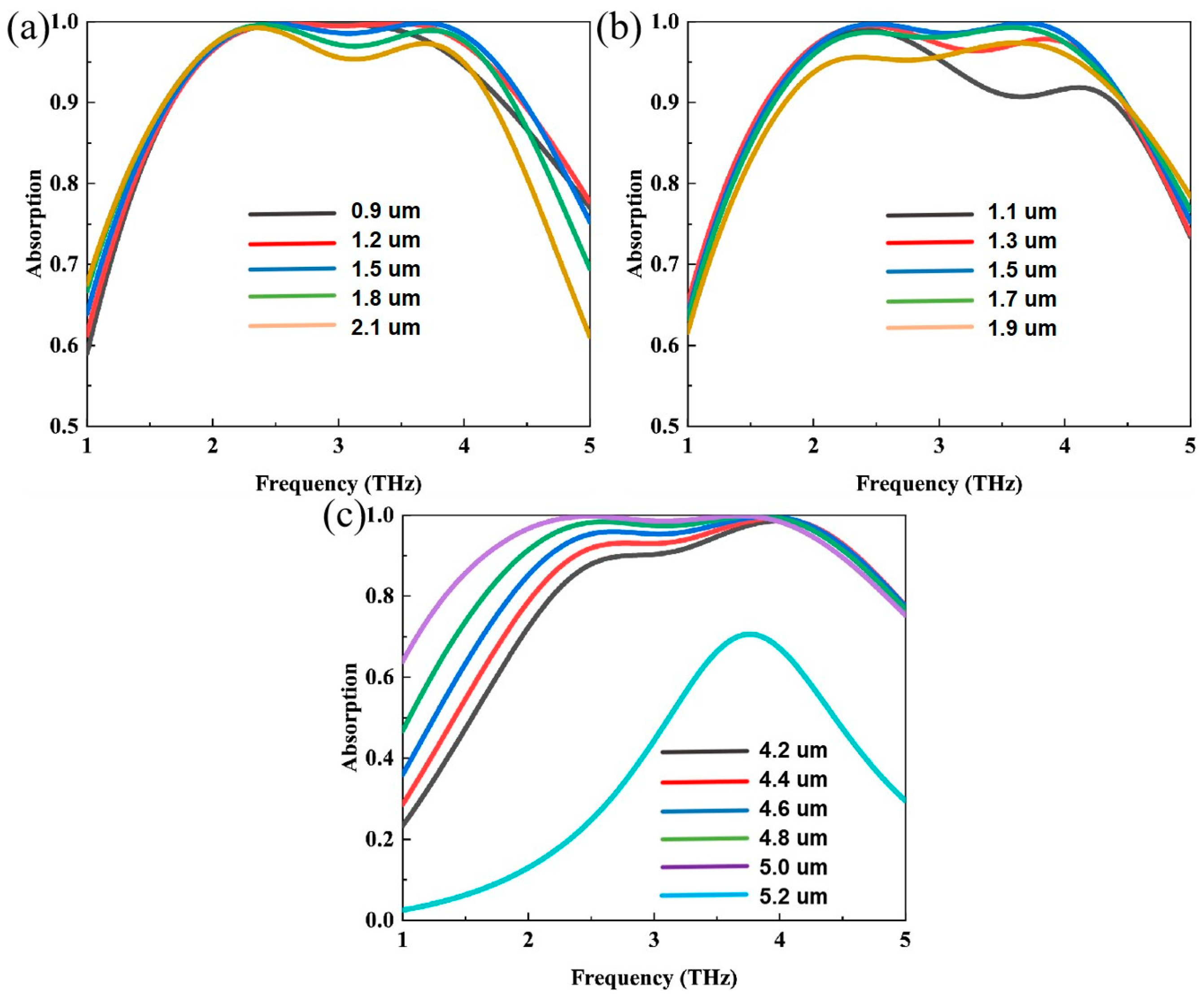
3.1. Results of Broadband Absorber
3.2. Results of Tunable Absorber
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhao, F.; Zhang, C.; Chang, H.; Hu, X. Design of Plasmonic Perfect Absorbers for Quantum-well Infrared Photodetection. Plasmonics 2014, 9, 1397–1400. [Google Scholar] [CrossRef]
- Shrekenhamer, D.; Montoya, J.; Krishna, S.; Padilla, W. Four-Color Metamaterial Absorber THz Spatial Light Modulator. Adv. Opt. Mater. 2013, 1, 905–909. [Google Scholar] [CrossRef]
- Baranov, D.G.; Krasnok, A.; Shegai, T.; Alù, A.; Chong, Y. Coherent perfect absorbers: Linear control of light with light. Nat. Rev. Mater. 2017, 2, 17064. [Google Scholar] [CrossRef]
- Wan, W.; Chong, Y.; Ge, L.; Noh, H.; Stone, A.D.; Cao, H. Time-Reversed Lasing and Interferometric Control of Absorption. Science 2011, 331, 889–892. [Google Scholar] [CrossRef] [PubMed]
- Wong, Z.J.; Xu, Y.L.; Kim, J.; O’Brien, K.; Wang, Y.; Feng, L.; Zhang, X. Lasing and anti-lasing in a single cavity. Nat. Photonics 2016, 10, 796–801. [Google Scholar] [CrossRef]
- Li, M.; Li, W.; Zeng, H. Molecular alignment induced ultraviolet femtosecond pulse modulation. Opt. Express 2013, 21, 27662. [Google Scholar] [CrossRef] [PubMed]
- Kang, M.; Chong, Y.D.; Wang, H.T.; Zhu, W.; Premaratne, M. Critical route for coherent perfect absorption in a Fano resonance plasmonic system. Appl. Phys. Lett. 2014, 105, 131103. [Google Scholar] [CrossRef]
- Wang, B.T.K.A. Wide-angle and polarization-independent chiral metamaterial absorber. Phys. Rev. B Condens. Matter Mater. Phys. 2010, 109, 17–22. [Google Scholar] [CrossRef]
- Alaee, R.; Farhat, M.; Rockstuhl, C.; Lederer, F. A perfect absorber made of a graphene micro-ribbon metamaterial. Opt. Express 2012, 20, 28017–28024. [Google Scholar] [CrossRef]
- Xiong, X.; Jiang, S.C.; Hu, Y.H.; Peng, R.W.; Wang, M. Structured Metal Film as a Perfect Absorber. Adv. Mater. 2013, 25, 3994–4000. [Google Scholar] [CrossRef]
- Hedayati, M.K.; Javaherirahim, M.; Mozooni, B.; Abdelaziz, R.; Tavassolizadeh, A.; Chakravadhanula, V.S.K.; Zaporojtchenko, V.; Strunkus, T.; Faupel, F.; Elbahri, M. Design of a Perfect Black Absorber at Visible Frequencies Using Plasmonic Metamaterials. Adv. Mater. 2011, 23, 5410–5414. [Google Scholar] [CrossRef] [PubMed]
- Huang, S.; Xie, Z.; Chen, W.; Lei, J.; Wang, F.; Liu, K.; Li, L. Metasurface with multi-sized structure for multi-band coherent perfect absorption. Opt. Express 2018, 26, 7066. [Google Scholar] [CrossRef] [PubMed]
- Huang, Y.; Xiao, T.; Chen, S.; Xie, Z.; Zheng, J.; Zhu, J.; Su, Y.; Chen, W.; Liu, K.; Tang, M.; et al. All-optical controlled-NOT logic gate achieving directional asymmetric transmission based on metasurface doublet. Opto-Electron. Adv. 2023, 6, 220073. [Google Scholar] [CrossRef]
- Li, X.; Pu, M.; Wang, Y.; Ma, X.; Li, Y.; Gao, H.; Zhao, Z.; Gao, P.; Wang, C.; Luo, X. Dynamic Control of the Extraordinary Optical Scattering in Semicontinuous 2D Metamaterials. Adv. Opt. Mater. 2016, 4, 659–663. [Google Scholar] [CrossRef]
- Wang, Y.; Huang, W.; Wang, C.; Guo, J.; Zhang, F.; Song, Y.; Ge, Y.; Wu, L.; Liu, J.; Li, J.; et al. An All-Optical, Actively Q-Switched Fiber Laser by an Antimonene-Based Optical Modulator. Laser Photon. Rev. 2019, 13, 1800313. [Google Scholar] [CrossRef]
- Jiang, X.; Liu, S.; Liang, W.; Luo, S.; He, Z.; Ge, Y.; Wang, H.; Cao, R.; Zhang, F.; Wen, Q.; et al. Broadband Nonlinear Photonics in Few-Layer MXene Ti3C2Tx(T = F, O, or OH). Laser Photon. Rev. 2018, 12, 1700229. [Google Scholar] [CrossRef]
- Fan, Y.; Shen, N.H.; Zhang, F.; Zhao, Q.; Wu, H.; Fu, Q.; Wei, Z.; Li, H.; Soukoulis, C.M. Graphene Plasmonics: A Platform for 2D Optics. Adv. Opt. Mater. 2019, 7, 1800537. [Google Scholar] [CrossRef]
- Yan, H.; Li, X.; Chandra, B.; Tulevski, G.; Wu, Y.; Freitag, M.; Zhu, W.; Avouris, P.; Xia, F. Tunable infrared plasmonic devices using graphene/insulator stacks. Nat. Nanotechnol. 2012, 7, 330–334. [Google Scholar] [CrossRef] [PubMed]
- Chen, P.; Argyropoulos, C.; Farhat, M.; Gomez-Diaz, J.S. Flatland plasmonics and nanophotonics based on graphene and beyond. Nanophotonics 2017, 6, 1239–1262. [Google Scholar] [CrossRef]
- Chen, P.; Alù, A. Atomically Thin Surface Cloak Using Graphene Monolayers. ACS Nano 2011, 5, 5855–5863. [Google Scholar] [CrossRef]
- Guo, T.; Argyropoulos, C. Broadband polarizers based on graphene metasurfaces. Opt. Lett. 2016, 41, 5592–5595. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.; Wang, G.; Sang, T. Simple design of novel triple-band terahertz metamaterial absorber for sensing application. J. Phys. D Appl. Phys. 2016, 49, 165307–165313. [Google Scholar] [CrossRef]
- Mak, K.F.; Sfeir, M.Y.; Wu, Y.; Lui, C.H.; Misewich, J.A.; Heinz, T.F.; Brookhaven, N.L.B.N. Measurement of the optical conductivity of graphene. Phys. Rev. Lett. 2008, 101, 196405. [Google Scholar] [CrossRef] [PubMed]
- Piper, J.R.; Fan, S. Total Absorption in a Graphene Monolayer in the Optical Regime by Critical Coupling with a Photonic Crystal Guided Resonance. ACS Photon. 2014, 1, 347–353. [Google Scholar] [CrossRef]
- Thareja, V.; Kang, J.H.; Yuan, H.; Milaninia, K.M.; Hwang, H.Y.; Cui, Y.; Kik, P.G.; Brongersma, M.L. Electrically Tunable Coherent Optical Absorption in Graphene with Ion Gel. Nano Lett. 2015, 15, 1570–1576. [Google Scholar] [CrossRef] [PubMed]
- Li, S.; Duan, Q.; Li, S.; Yin, Q.; Lu, W.; Li, L.; Gu, B.; Hou, B.; Wen, W. Perfect electromagnetic absorption at one-atom-thick scale. Appl. Phys. Lett. 2015, 107, 181112. [Google Scholar] [CrossRef]
- Xu, K.; Li, J.; Zhang, A.; Chen, Q. Tunable multi-band terahertz absorber using a single-layer square graphene ring structure with T-shaped graphene strips. Opt. Express 2020, 28, 11482. [Google Scholar] [CrossRef] [PubMed]
- Pirruccio, G.; Martín Moreno, L.; Lozano, G.; Gómez Rivas, J. Coherent and Broadband Enhanced Optical Absorption in Graphene. ACS Nano 2013, 7, 4810–4817. [Google Scholar] [CrossRef]
- Hu, X.; Wang, J. High-speed gate-tunable terahertz coherent perfect absorption using a split-ring graphene. Opt. Lett. 2015, 40, 5538–5541. [Google Scholar] [CrossRef]
- Guo, T.; Argyropoulos, C. Tunable and broadband coherent perfect absorbers with nonlinear and amplification performance based on asymmetric bifacial graphene metasurfaces. J. Opt. 2020, 22, 84003. [Google Scholar] [CrossRef]
- Emani, N.K.; Chung, T.F.; Ni, X.; Kildishev, A.V.; Chen, Y.P.; Boltasseva, A. Electrically Tunable Damping of Plasmonic Resonances with Graphene. Nano Lett. 2012, 12, 5202–5206. [Google Scholar] [CrossRef] [PubMed]
- Kakenov, N.; Balci, O.; Takan, T.; Ozkan, V.A.; Altan, H.; Kocabas, C. Observation of Gate-Tunable Coherent Perfect Absorption of Terahertz Radiation in Graphene. ACS Photon. 2016, 3, 1531–1535. [Google Scholar] [CrossRef]
- Pu, M.; Feng, Q.; Wang, M.; Hu, C.; Huang, C.; Ma, X.; Zhao, Z.; Wang, C.; Luo, X. Ultrathin broadband nearly perfect absorber with symmetrical coherent illumination. Opt. Express 2012, 20, 2246–2254. [Google Scholar] [CrossRef] [PubMed]
- Zhu, W.; Xiao, F.; Kang, M.; Premaratne, M. Coherent perfect absorption in an all-dielectric metasurface. Appl. Phys. Lett. 2016, 108, 121901. [Google Scholar] [CrossRef]
- Saeed, M.; Alshammari, Y.; Majeed, S.A.; Al-Nasrallah, E. Chemical Vapour Deposition of Graphene—Synthesis, Characterisation, and Applications: A Review. Molecules 2020, 25, 3856. [Google Scholar] [CrossRef] [PubMed]
- Michaud, L.G.; Azrak, E.; Castan, C.; Fournel, F.; Rieutord, F.; Tardif, S.; Montméat, P. Transfer of an ultrathin single-crystal silicon film from a silicon-on-insulator to a polymer. Mater. Today Nano 2021, 13, 100107. [Google Scholar] [CrossRef]
- Fang, Z.; Thongrattanasiri, S.; Schlather, A.; Liu, Z.; Ma, L.; Wang, Y.; Ajayan, P.M.; Nordlander, P.; Halas, N.J.; de Abajo, F.J.G. Gated Tunability and Hybridization of Localized Plasmons in Nanostructured Graphene. ACS Nano 2013, 7, 2388–2395. [Google Scholar] [CrossRef] [PubMed]
- Wang, C.; Liu, W.; Li, Z.; Cheng, H.; Li, Z.; Chen, S.; Tian, J. Dynamically Tunable Deep Subwavelength High-Order Anomalous Reflection Using Graphene Metasurfaces. Adv. Opt. Mater. 2018, 6, 1701047. [Google Scholar] [CrossRef]
- Fang, Z.; Wang, Y.; Schlather, A.E.; Liu, Z.; Ajayan, P.M.; García De Abajo, F.J.; Nordlander, P.; Zhu, X.; Halas, N.J. Active Tunable Absorption Enhancement with Graphene Nanodisk Arrays. Nano Lett. 2014, 14, 299–304. [Google Scholar] [CrossRef]
- Kim, J.; Son, H.; Cho, D.J.; Geng, B.; Regan, W.; Shi, S.; Kim, K.; Zettl, A.; Shen, Y.-R.; Wang, F. Electrical Control of Optical Plasmon Resonance with Graphene. Nano Lett. 2012, 12, 5598–5602. [Google Scholar] [CrossRef]
- Falkovsky, L.A. Optical properties of graphene. J. Phys. Conf. Ser. 2008, 129, 012004. [Google Scholar] [CrossRef]
- Chatzidimitriou, D.; Pitilakis, A.; Kriezis, E.E. Rigorous calculation of nonlinear parameters in graphene-comprising waveguides. J. Appl. Phys. 2015, 118, 023105. [Google Scholar] [CrossRef]
- Gao, E.; Li, H.; Liu, Z.; Xiong, C.; Liu, C.; Ruan, B.; Li, M.; Zhang, B. Terahertz multifunction switch and optical storage based on triple plasmon-induced transparency on a single-layer patterned graphene metasurface. Opt. Express 2020, 28, 40013. [Google Scholar] [CrossRef] [PubMed]
- Liu, M.; Yin, X.; Ulin-Avila, E.; Geng, B.; Zentgraf, T.; Ju, L.; Wang, F.; Zhang, X. A graphene-based broadband optical modulator. Nature 2011, 474, 64–67. [Google Scholar] [CrossRef] [PubMed]
- Morozov, S.V.; Novoselov, K.S.; Katsnelson, M.I.; Schedin, F.; Elias, D.C.; Jaszczak, J.A.; Geim, A.K. Giant intrinsic carrier mobilities in graphene and its bilayer. Phys. Rev. Lett. 2008, 100, 016602. [Google Scholar] [CrossRef] [PubMed]
- Xu, K.; Huang, J.; Wang, W. Broadband perfect optical absorption enabled by quasi-bound states in the continuum in graphene non-concentric rings. Phys. Chem. Chem. Phys. 2022, 25, 604–611. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Xie, Q.; Guo, L.; Su, C.; Wang, M.; Xia, F.; Sun, J.; Li, K.; Feng, H.; Yun, M. Dual-controlled tunable dual-band and ultra-broadband coherent perfect absorber in the THz range. Opt. Express 2022, 30, 30832. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Gao, W.; Guo, L.; Chen, Z.; Li, C.; Zhang, H.; Jiao, J.; An, B. Tunable ultra-broadband terahertz perfect absorber based on vanadium oxide metamaterial. Opt. Express 2021, 29, 41222. [Google Scholar] [CrossRef]
- Huang, J.; Li, J.; Yang, Y.; Li, J.; Li, J.; Zhang, Y.; Yao, J. Broadband terahertz absorber with a flexible, reconfigurable performance based on hybrid-patterned vanadium dioxide metasurfaces. Opt. Express 2020, 28, 17832. [Google Scholar] [CrossRef] [PubMed]
- Zhuo, S.; Liu, Z.; Zhou, F.; Qin, Y.; Luo, X.; Ji, C.; Yang, G.; Yang, R.; Xie, Y. THz broadband and dual-channel perfect absorbers based on patterned graphene and vanadium dioxide metamaterials. Opt. Express 2022, 30, 47647. [Google Scholar] [CrossRef]




| Absorber Bandwidth | Absorptivity | Materials | Ref. |
|---|---|---|---|
| 0.67–1.66 THz (0.99 THz) | 90% | Graphene non-concentric rings, perfectly matched layer | [46] |
| 0.1–10.8 THz (10.7 THz) | 90% | VO2-based metamaterial, transparent dielectric layer | [47] |
| 3.03–8.13 THz (5.1 THz) | 90% | VO2 periodic pattern, dielectric spacer, metallic substrate | [48] |
| 1.85–4.3 THz (2.45 THz) | 90% | VO2 square loops, Au, SiO2 | [49] |
| 5.956–7.639 THz (1.683 THz) | ≈100% | Patterned graphene, VO2, Au, SiO2 | [50] |
| 1.65–4.49 THz (2.84 THz) | 90% | Round and square graphene, silicon | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, J.; Hong, L.; Lei, J.; Shen, Y.; Deng, X.; Chen, J.; Guo, T. High-Performance Terahertz Coherent Perfect Absorption with Asymmetric Graphene Metasurface. Photonics 2024, 11, 544. https://doi.org/10.3390/photonics11060544
Chen J, Hong L, Lei J, Shen Y, Deng X, Chen J, Guo T. High-Performance Terahertz Coherent Perfect Absorption with Asymmetric Graphene Metasurface. Photonics. 2024; 11(6):544. https://doi.org/10.3390/photonics11060544
Chicago/Turabian StyleChen, Jintao, Lujun Hong, Jiangtao Lei, Yun Shen, Xiaohua Deng, Jing Chen, and Tianjing Guo. 2024. "High-Performance Terahertz Coherent Perfect Absorption with Asymmetric Graphene Metasurface" Photonics 11, no. 6: 544. https://doi.org/10.3390/photonics11060544
APA StyleChen, J., Hong, L., Lei, J., Shen, Y., Deng, X., Chen, J., & Guo, T. (2024). High-Performance Terahertz Coherent Perfect Absorption with Asymmetric Graphene Metasurface. Photonics, 11(6), 544. https://doi.org/10.3390/photonics11060544






