1. Introduction
Single longitudinal mode fiber ring lasers, renowned for their high coherence, compact structure, and excellent beam quality, have broad applications in fields such as laser ranging, optical communication, and microwave photon signal generation. In the optical fiber ring cavity, which is several tens of meters long, there exist free spectral ranges (FSRs) on the order of megahertz (MHz), while typical optical filters have bandwidths of the order of gigahertz (GHz). Consequently, by directly using conventional optical filters as wavelength selection elements for laser oscillators, it is challenging to achieve single-mode outputs. To overcome this challenge, common techniques for single-mode selection in fiber ring lasers include compound rings with Vernier effects [
1,
2,
3,
4,
5], saturable absorbers [
6,
7,
8,
9], cascaded microfiber knots [
10], whispering gallery mode resonator [
11], phase-shifted fiber Bragg gratings [
12], and dynamic gratings [
13,
14] to establish narrow linewidth filters. However, these methods often require complex cavity structures and fine parameter tuning.
In recent years, the concept of parity-time (PT) symmetry has emerged as a promising solution for achieving single-mode output in fiber ring lasers [
15,
16,
17,
18,
19,
20,
21,
22,
23,
24]. Typically, PT symmetric fiber ring systems utilize two subsystems with identical geometric length and balanced gain and loss to facilitate mode selection. When the gain coefficient exceeds the coupling coefficient, PT symmetry breaking occurs, significantly enhancing the gain difference between the principal mode and the side modes, thus enabling single-mode oscillation. One approach involves non-reciprocal loop-based PT symmetric fiber lasers [
15,
16,
17,
18] with two loops in the ring cavity. Gain and loss are balanced using optical isolators and polarization controllers (PCs) to establish PT symmetry and attain single-mode outputs. Another technique operates near exceptional points [
19] by leveraging a tunable optical isolator to approach such points in the laser system, thereby achieving narrow linewidth single-mode outputs. Moreover, stimulated Brillouin scattering (SBS)-based PT symmetric schemes [
20,
21] have been investigated, combining adjustable gain and loss to construct PT symmetric cavities for mode selection and narrow linewidth single-mode generation. A dual-loop PT symmetric system [
22] with two loops coupled at a rational length ratio has also been examined to achieve PT symmetry and single-mode outputs. Additionally, in fiber ring cavities, PT symmetry has been realized using polarization-dependent loss [
23,
24], as well as by employing a polarization-maintaining fiber Bragg grating [
25], where adjusting the loss enables single-mode output generation upon PT symmetry breaking. These schemes offer advantages in mode selection, enabling single-mode outputs without high-quality (high-Q) filters, thereby simplifying system design and enhancing stability. However, challenges remain regarding system complexity, cost, and power conversion efficiency. Further optimization is necessary to realize more efficient, stable, and cost-effective single longitudinal mode fiber ring lasers.
In this work, we propose a structurally simple fiber ring laser based on polarization space PT symmetry breaking. By controlling the gain/loss and by coupling coefficients of two polarization states within a single fiber ring, the laser achieves a low-noise, narrow-linewidth, and highly stable single-mode optical output. By adjusting a PC and the pumping current of an erbium-doped fiber amplifier (EDFA) in the cavity, the gain/loss and coupling coefficients of the two polarization loops can be controlled. When the gain balances the loss and the gain coefficient exceeds the coupling coefficient, PT symmetry breaking occurs, significantly enhancing the gain difference between the principal mode and the side modes, thus enabling single-mode laser oscillation. The single-mode laser signal is analyzed using a short-delay self-heterodyne [
26,
27,
28] method to obtain the frequency noise spectral density, fundamental linewidth, integral linewidth, and effective integral linewidth of the proposed PT symmetric fiber ring laser. The experimental results demonstrate that the laser is characterized by extremely low-frequency noise and linewidth, high stability, and a wide tuning range. Specifically, the frequency noise spectral density can be as low as approximately 181 Hz
2/Hz at an offset frequency of 10 kHz, with a fundamental linewidth of approximately 68 Hz. Moreover, the laser exhibits excellent wavelength stability with only 0.64 pm drift over 30 min. By tuning an optical tunable filter (OTF), the single-mode output wavelength can cover the entire C-band. Additionally, the impacts of different fiber lengths on the frequency noise spectral density and the effects of various filter bandwidths on long-term stability are analyzed.
2. Experimental Setup and Operational Principle
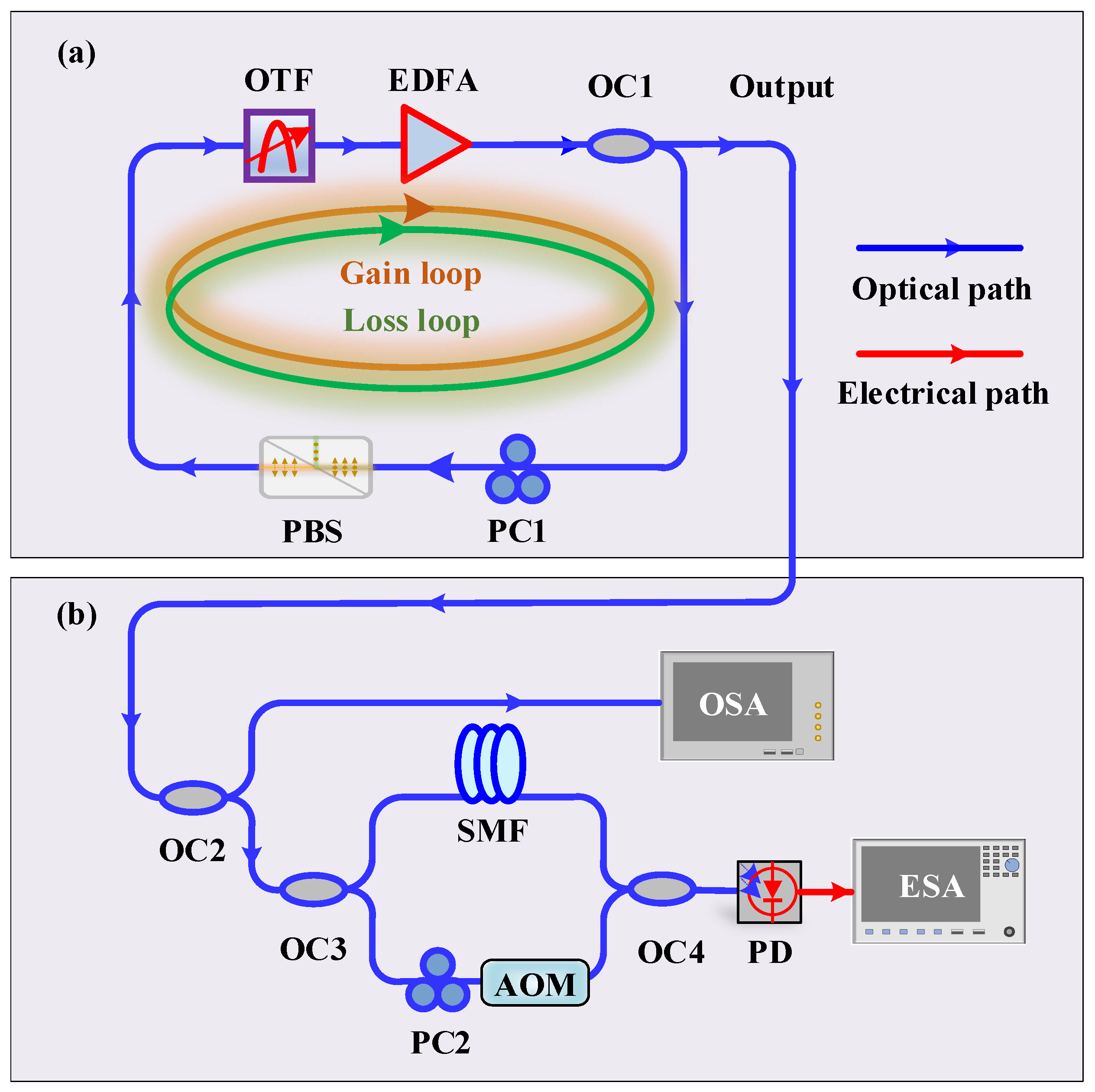
Figure 1 illustrates the schematic diagram of the experimental setup for the proposed polarization space PT symmetric fiber ring laser, along with the setup for evaluating performance of the laser output signal. As shown in
Figure 1a, the fiber ring cavity comprises two polarization loops within a single fiber loop. An erbium-doped fiber amplifier (EDFA) provides gain. An optical tunable filter (OTF) serves for coarse wavelength selection. A 70:30 optical coupler (OC1) serves to close the loop and output port. A polarization controller (PC1) and a polarization beam splitter (PBS) are the key elements realizing the polarization space PT symmetry. Here, the function of PBS is equivalent to that of a polarizer [
23]. The total cavity length is 97.5 m, corresponding to 2.05 MHz of the FSR. The experimental setup for evaluating the laser output signal performance is shown in
Figure 1b. The output is divided into two paths by a 10:90 optical coupler (OC2), where 10% is coupled with a high-precision optical spectrum analyzer (OSA, Aragon Photonics BOSA lite+, 20 MHz resolution bandwidth) for spectral analysis, while the remaining 90% enters a delayed self-heterodyne system for longitudinal mode and frequency noise analysis. In the delayed self-heterodyne system, the signal is split into upper and lower arms by a 3 dB optical coupler (OC3). The upper arm is delayed by a single-mode fiber (SMF), while the lower arm comprises a polarization controller (PC2) and an acousto-optic modulator (AOM, G-1550-80) with an 80 MHz frequency shift. The signals from the two arms are coupled by another 3 dB optical coupler (OC4) and detected by a photodetector (PD, XPDV2120R, 50 GHz bandwidth), generating a beat signal for spectral and phase noise analyzed by an electrical spectrum analyzer (ESA, R&S FSW, 67 GHz bandwidth). Noteworthy is the fact that a 40 km SMF is used to analyze the longitudinal mode and spectral line shape of the laser, while a 9.77 m SMF is employed to measure the frequency noise spectral density and linewidth of the laser in the delayed self-heterodyne system.
In
Figure 1a, the birefringence of PC1 induces two polarization components,
Ex and
Ey. The angle between the electric field vector
Ex and the parallel polarization direction in the PBS, denoted as
θ, can be controlled through PC1. Here, the PBS can be effectively regarded as a linear polarizer. The relations between the electric fields before and after passing through the concatenated combination of PC1 and PBS for one round trip inside the cavity, denoted as
E0 and
E1, can be expressed as [
23,
29]:
where the parameter
G0 represents the round-trip gain when the electric field in the
Ex or
Ey direction is perfectly aligned with the parallel polarization direction of the PBS. Obviously, as the polarization components
Ex and
Ey circulate within the optical fiber loop, their relative angles with respect to the parallel polarization direction of the PBS lead to the round-trip gain coefficients as:
The round-trip coupling coefficient between the two loops can be expressed as:
Based on the definitions of gain and coupling per time unit coefficients, the gain and coupling coefficients can be expressed as [
23,
25]:
where
T is the round-trip time of the light in the cavity.
When gain and loss are balanced, namely
or
, then:
Therefore, by adjusting PC1 to tune the angle
θ or altering the pumping current of the EDFA to modify the gain parameter
G0, the PT symmetric condition can be achieved.
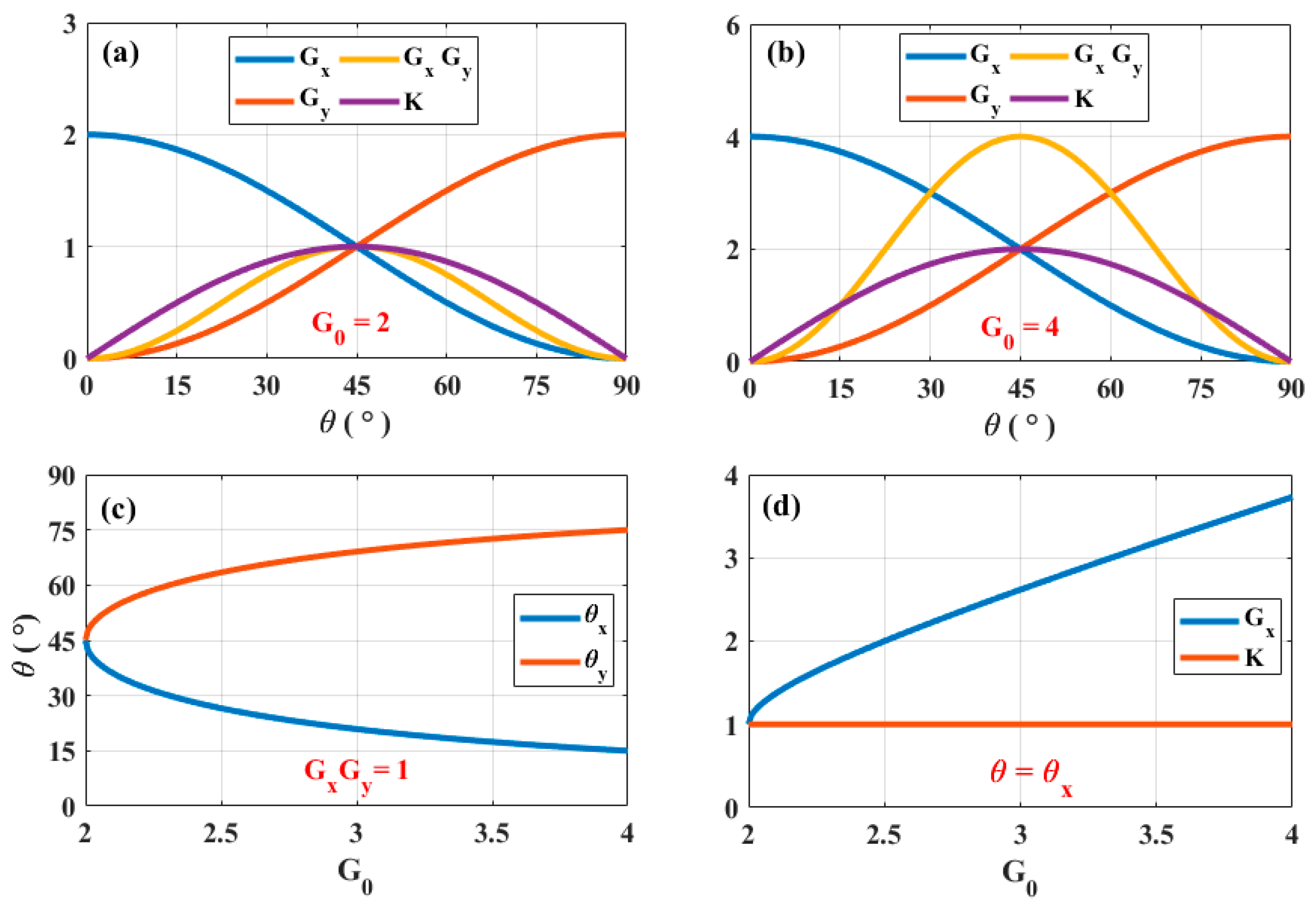
Figure 2 illustrates the interrelationship among the round-trip gain/loss, coupling coefficients, angle
θ, and gain parameter
G0.
Figure 2a,b show the variations in the round-trip gain/loss for both loops, the coupling coefficient, and the
GxGy coefficient as functions of
θ, for
G0 = 2 and
G0 = 4, respectively. As evident from
Figure 2a, when
G0 = 2, the PT symmetric condition (
GxGy = 1) is satisfied only when
θ = 45° and the system lies at the exceptional point (EP) with
K =
Gx. An EP is a branch point singularity in parameter space where two or more eigenvalues and their corresponding eigenvectors of a non-Hermitian system coalesce and become degenerate [
30,
31]. In comparison,
Figure 2b shows that, when
G0 = 4, the PT symmetric condition is satisfied for
θ = 15° or
θ = 75°. However, in this case,
K is smaller than
Gx or
Gy, resulting in PT symmetry breaking.
Figure 2c illustrates the values of θ corresponding to different
G0 values under the fulfillment of the PT symmetric condition. When the value of
θ satisfies Equation (8), it can be observed that, for
θ < 45°,
Ex-mode exhibits gain in the round-trip performance. Under this case,
θ is recorded as
θx. On the other hand, for
θ > 45°,
Ex-mode displays loss, while
Ey-mode exhibits gain in the round-trip performance. Under this case,
θ is recorded as
θy. Finally,
Figure 2d shows the round-trip gain and coupling coefficients for different
G0 values under the fulfillment of the PT symmetric condition. Evidently, when
G0 > 2 and
θ =
θx,
K is smaller than
Gx, leading to PT symmetry breaking.
When converting the round-trip gain/loss and coupling coefficients into per unit time coefficients, the coupling equations of the proposed fiber ring laser can be expressed as [
18,
23]:
where
denotes the frequency of the nth mode of the cavity. When
+
= 0, the PT symmetry condition of the system is satisfied, and the solutions for the eigenfrequencies of the coupled Equation (9) are obtained:
When the gain coefficient equals the coupling coefficient (|
| =
), the eigenmodes become degenerate, reaching the so-called EP [
17]. In contrast, when the gain coefficient is greater than the coupling coefficient (|
| >
), complex solutions exist for the eigenfrequencies, leading to the spontaneous breaking of PT symmetry. In this case, two eigenmodes share the same frequency, yet the two coupled loops provide gain for one eigenmode while imparting an equivalent magnitude of loss to the other. Upon the occurrence of PT symmetry breaking, the eigenfrequencies exhibit a square root dependence on the gain, leading to an enhancement in the gain difference between the principal mode (highest longitudinal mode
) and the secondary mode (second highest gain longitudinal mode
). The enhancement factor for the gain difference is [
16]:
where
and
represent the gain difference between the principal mode and the secondary mode in a system with PT symmetry and another system without PT configuration, respectively. In the PT symmetric non-Hermitian system, the gain difference increases by a factor of F, facilitating the achievement of a single-mode output without the high-Q optical filters.
In
Figure 1b, the single-mode optical signal prior to entering the optical self-heterodyne system is characterized as:
where
denotes the center frequency of the signal,
represents the phase noise of the optical signal, and
P signifies the power of the optical signal. Upon passing through OC3, one path is delayed by a SMF, while the other is frequency-shifted by
with an AOM. Therefore, the optical signals of the two paths can be respectively delineated as:
Then, the beat signal obtained after the mutual superposition of the two via OC4 and detected by a PD with responsivity
R is:
where:
which represents the phase noise in beat signal. The phase noise spectral density
of the beat signal can be measured by the ESA. When the time delay
is significantly smaller than the coherence time of the laser, the relationship between the phase noise spectral density
of the beat signal and the frequency noise spectral density
of the laser can be derived as:
Considering the zero point issue in Equation (17), the laser frequency noise spectral density at zero point needs further restorative measures [
28].
3. Results and Discussion
An experiment was conducted using the setup shown in
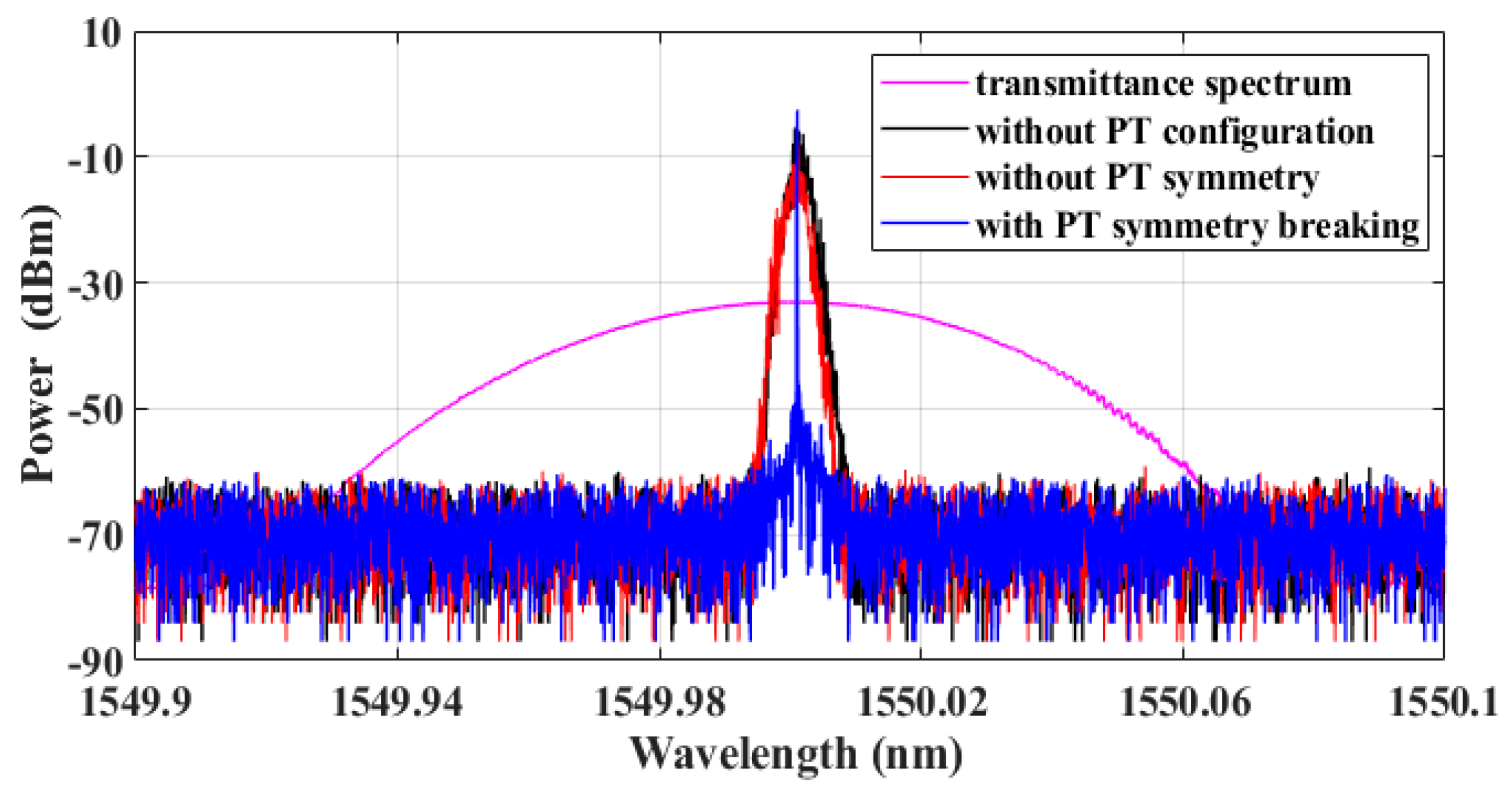
Figure 1. Initially, the transmittance spectrum of the OTF was measured by the ‘Component Analyzer’ module of the OSA, as indicated by the magenta trace in
Figure 3 with a 3 dB bandwidth of 0.0446 nm. Next, the optical spectrum of a fiber ring laser without PT configuration was obtained by removing the PBS and PC1 from the system shown in
Figure 1a, as indicated by the black trace in
Figure 3. Then, the main experiment was performed with the pumping current fixed at 150 mA in the setup shown in
Figure 1a. When PC1 was adjusted without PT symmetry, the optical spectrum of the laser appeared as the red trace in
Figure 3. However, when adjusting PC1 to achieve gain–loss balance, with the gain coefficient exceeding the coupling coefficient, PT symmetry breaking occurred, resulting in the solitary spectral line shown by the blue trace in
Figure 3.
Given the 97.5 m length of the optical fiber ring cavity, the corresponding FSR was 2.051 MHz. However, the minimum resolution bandwidth (RBW) of the OSA was only 0.16 pm (20 MHz), which renders it challenging to definitively determine whether the output laser signal was single-longitudinal-mode. Hence, to assist in analyzing the oscillation modes of the laser signal, the output light was passed through an optical self-heterodyne system with a 40 km SMF length, followed by electric spectrum analysis of the beat signal obtained through the PD. The experimental setup shown in
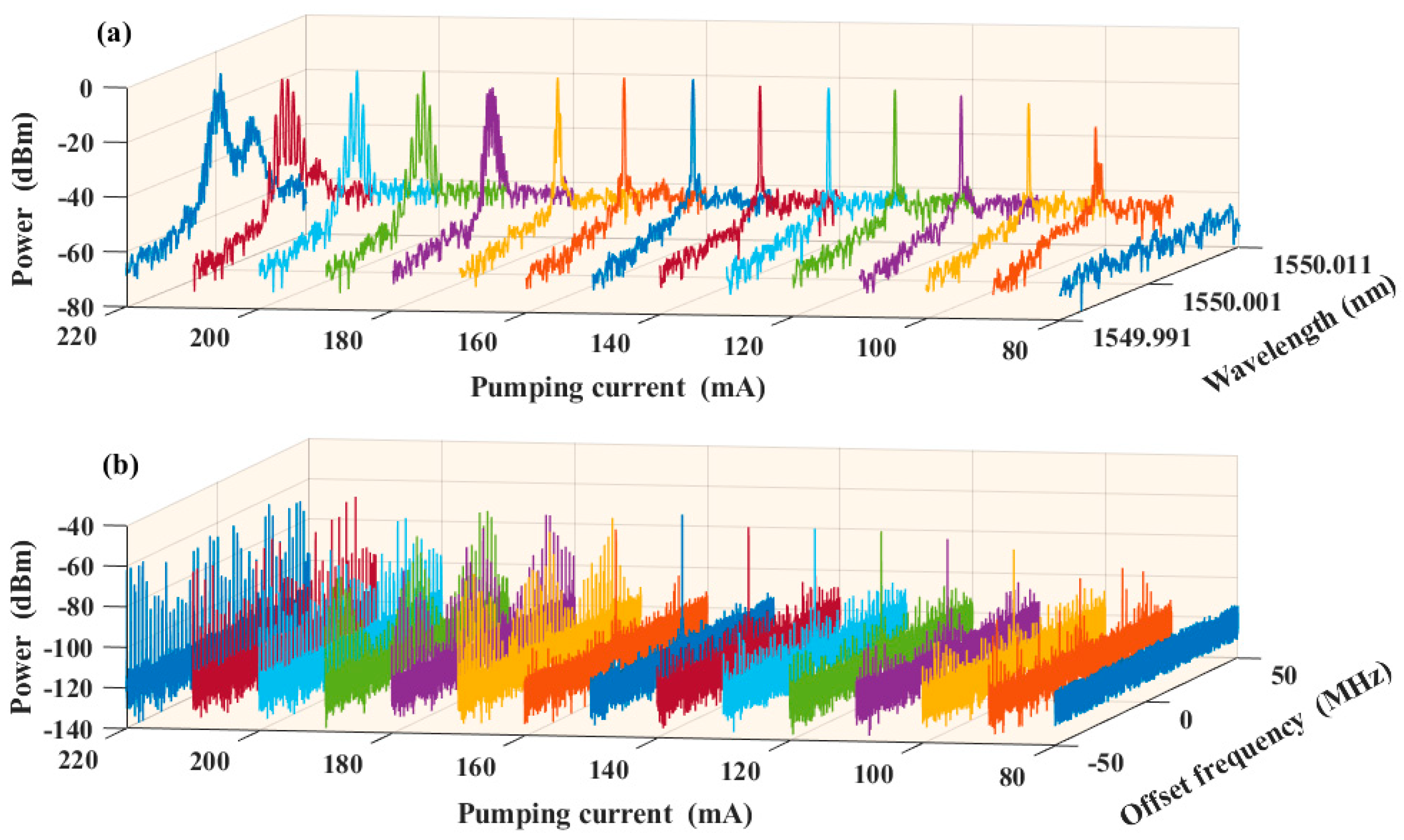
Figure 1b was utilized to observe the evolution of the output optical signal with variations in the EDFA pumping current (in 10 mA increments), while maintaining the state of PC1 fixed, as illustrated in
Figure 4. Under different EDFA pumping currents, the spectral evolution of the output light is depicted in
Figure 4a, with a span of 0.02 nm (25 GHz). Additionally, the spectral evolution of the beat signal is illustrated in
Figure 4b, at a central frequency of 80.0 MHz, with a span of 100 MHz and an RBW of 10 kHz. As it can be seen from
Figure 4 by observing the optical spectrum and electrical spectrum, under an EDFA pumping current of 80 mA, the output was noise. As the pumping current was increased to 90 mA, multiple peaks emerged in both spectra, suggesting a multimode output since the condition of PT symmetry breaking was unsatisfied. For the pumping current located at the range of 100~140 mA, only a peak with strong power was observed in the optical spectrum shown in
Figure 4a, while some extra beating signals with weak power still appeared in the electrical spectrum shown in
Figure 4b. With the increase in pump current, the extra beating signals gradually diminished, indicating that the system gradually approached the PT symmetry breaking state. Especially when the pumping current was increased to 150 mA, the side mode suppression ratio of the beat frequency signal attained its maximum of 55.2 dB. In this case, the PT symmetry breaking condition was well-satisfied, and the output exhibited single-mode behavior, corresponding to the principal mode. By further increasing the pump current to 220 mA, the condition of PT symmetry breaking deviated again, and the system presented the multimode output state. It should be pointed out that the results were obtained under fixed PC1. Our experimental results also show that, by adjusting PC1, the condition of PT symmetry breaking can also be well-satisfied under other pumping current conditions.
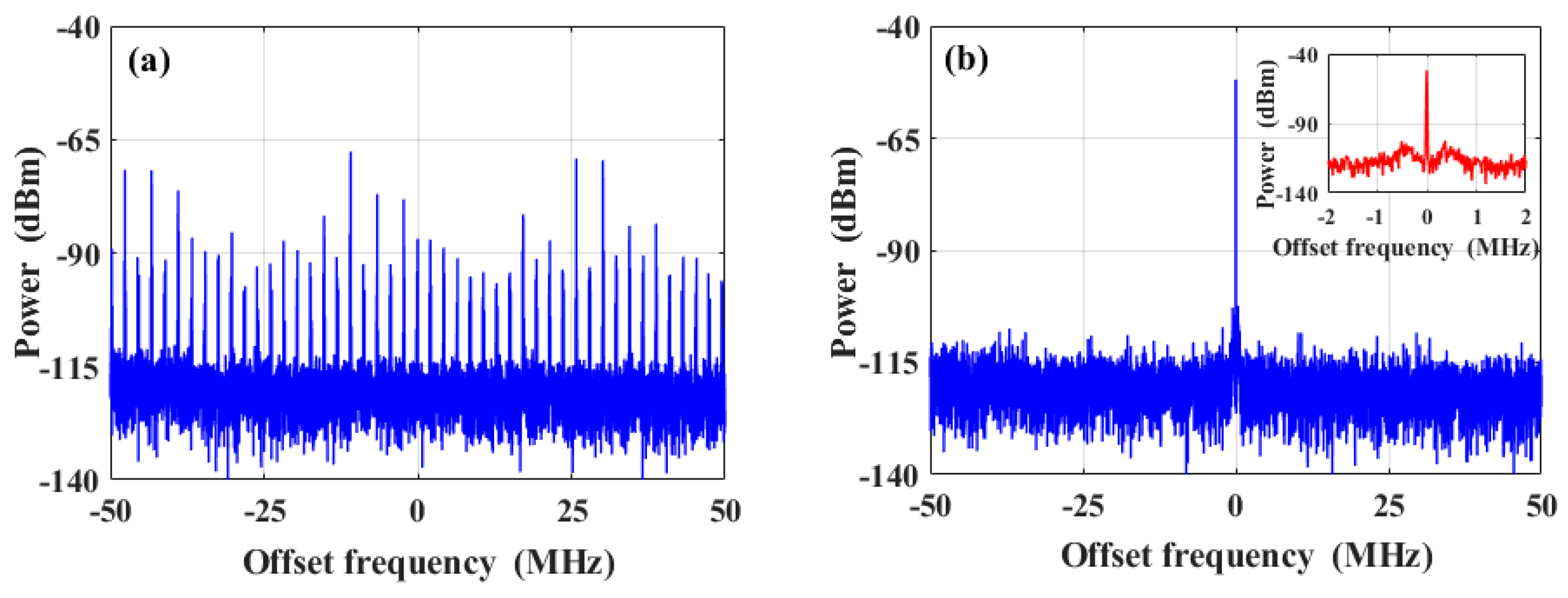
Figure 5 specifically shows the electrical spectrum of the beat signals detected under the conditions of a pumping current equal to 200 mA without PT symmetry, and under the conditions of a pumping current equal to 150 mA with PT symmetry breaking at a central frequency of 80.0 MHz. The resolution bandwidth (RBW) was 10 kHz and the span was 100 MHz. In
Figure 5a, it is evident from the spectrum that there were multimode signals with a mode spacing of 2.051 MHz, corresponding to a cavity length of 97.5 m. By adjusting the pumping current of the EDFA to 150 mA to achieve PT symmetry breaking, a single-mode laser output was realized. In
Figure 5b, it can be observed that all side modes were suppressed, indicating the successful attainment of a single-longitudinal-mode laser operation. The inset in
Figure 5b shows a zoomed-in view with a span of 4 MHz. From the inset, it can be observed that there are two wing-shaped spectral peaks caused by the relaxation oscillation of the laser at the center frequency of the beat signal.
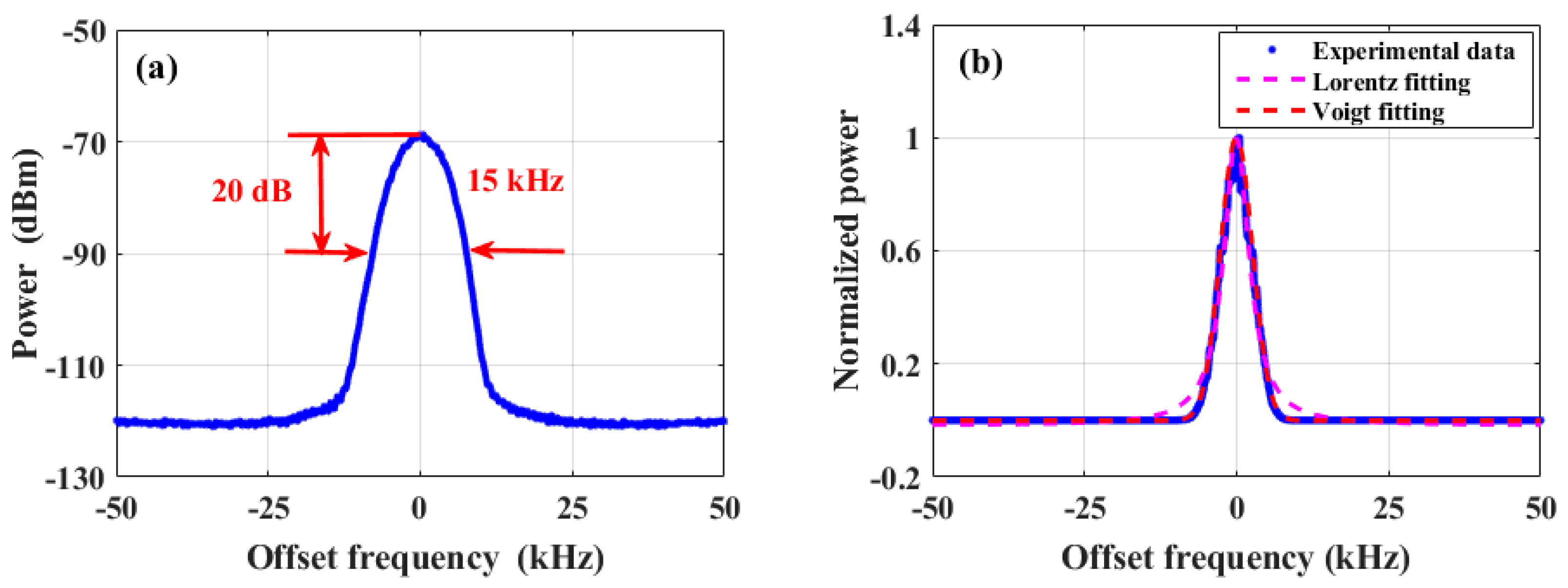
When this fiber ring laser output a single-longitudinal-mode optical signal under the PT symmetry breaking phase, the linewidth and line shape of the laser were analyzed by the conventional long-delayed self-heterodyne method with a 40 km SMF, as shown in
Figure 6.
Figure 6a depicts the electrical spectrum of the beat signal. The measured 20 dB linewidth was determined to be 15.0 kHz, corresponding to a 3 dB Lorentzian linewidth of 750 Hz. Notably, upon power normalization of the measured spectra and subsequent fitting with Lorentzian and Voigt functions, as illustrated in
Figure 6b, the Voigt fitting appeared to better conform to the experimental data. This observation indicates that 1/f noise predominated in the output optical signal. However, this linewidth estimation method has certain limitations. Utilizing the long-delayed self-heterodyne method requires determining the coherent delay length based on the linewidth, and the delay time must be approximately six times the coherence time of the laser [
32]. Yet, excessively long fiber delay lines introduce unnecessary 1/f noise, rendering the long-delayed self-heterodyne method challenging for linewidth estimation. Therefore, the noise of the laser was analyzed using the short-delayed self-heterodyne method.
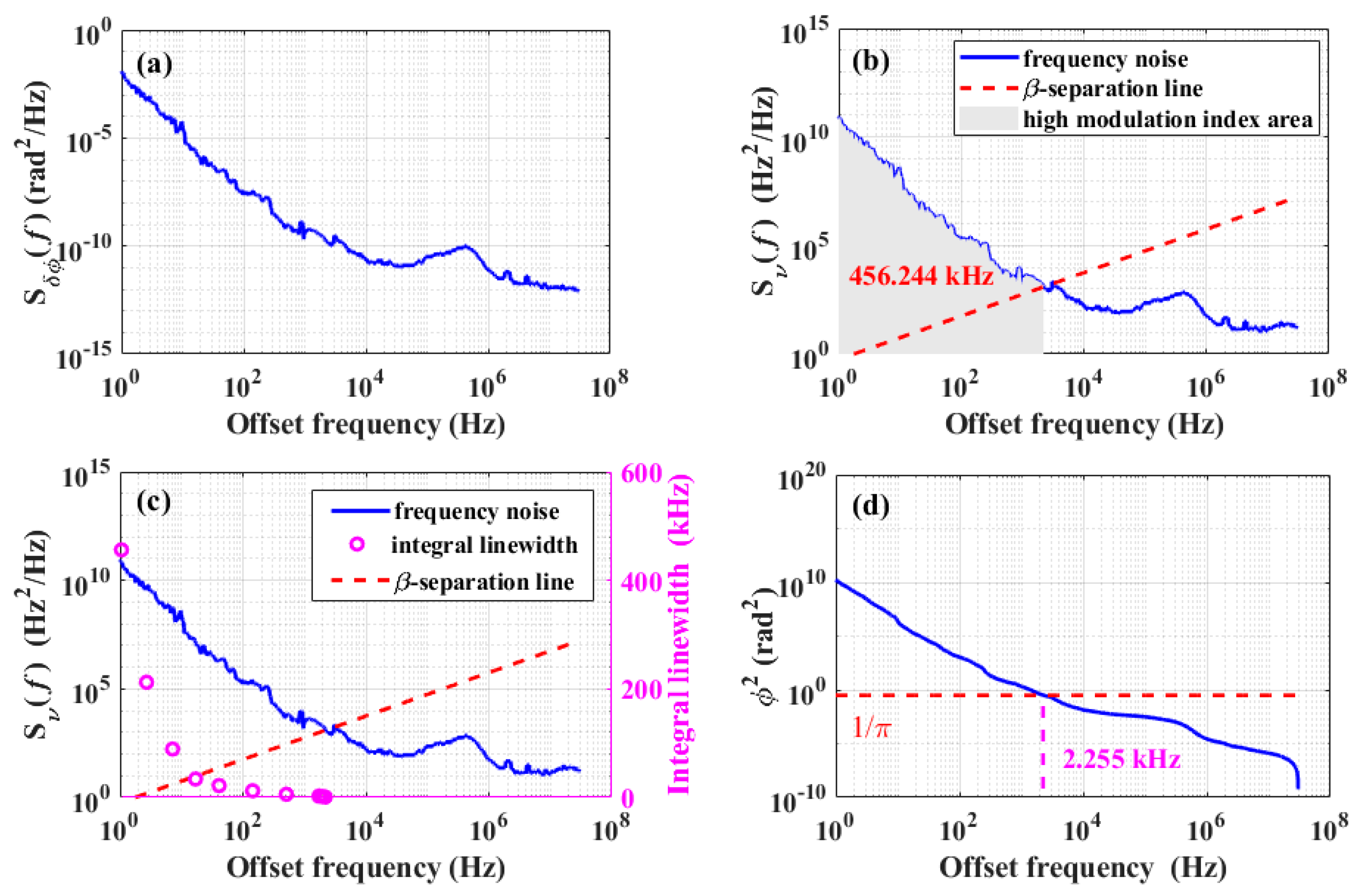
The frequency noise spectral density of the fiber ring laser was measured by a short-delay self-heterodyne system. A 9.77 m SMF was employed to ensure coherence between the optical signals in the two arms. The phase noise spectral density of the coherent beat signal obtained through the short-delay self-heterodyne system is illustrated in
Figure 7a. According to the phase noise spectrum of the beat signal and to Equation (17), the frequency noise spectral density and the integral linewidth [
33] of the laser can be obtained, as shown in
Figure 7b. In
Figure 7b, the blue spectrum line represents the frequency noise spectral density of the PT symmetric fiber ring laser, the red dashed line represents the β-separation line, and the gray area represents the region of high modulation index contributing to the integral linewidth over an observation time of 1 s. As shown in
Figure 7b, the frequency noise decreased by approximately 10
9 times as the frequency offset increased from 1 Hz to 10 kHz, indicating that low-frequency noise dominated the optical signal noise. At an offset frequency of 10 kHz, the frequency noise spectral density could be reduced to approximately 181 Hz
2/Hz. From an offset frequency ranging from 100 kHz to 1 MHz, the frequency noise initially increased and then decreased, possibly due to relaxation oscillations in the fiber laser. The side modes were suppressed below 47 Hz
2/Hz. The fundamental linewidth [
34] can be calculated from the white noise at high frequencies (much greater than 1 MHz) in the frequency noise spectral density, which is approximately 68 Hz. When starting from an offset frequency of 1 Hz (with an observation time of 1 s), the integral linewidth was calculated to be 456.224 kHz. The integral linewidth starting from different offset frequencies is shown in
Figure 7c. From
Figure 7c, it is evident that, as the starting offset frequency increased (corresponding to a short observation timescale), the integral linewidth gradually decreased. When the starting offset frequency was 2.11 kHz (with an observation time of 473.9 μs), the integral linewidth decreased to approximately 718 Hz. Furthermore, by utilizing the frequency noise spectral density of the laser, the effective integral linewidth [
34,
35] of the laser can be obtained, as illustrated in
Figure 7d. By converting the frequency noise into phase noise of the laser and integrating the phase noise from high offset frequencies to a certain offset frequency such that its integrated value equals 1/π rad
2, the corresponding offset frequency is defined as the effective integral linewidth. As depicted in
Figure 7d, the effective integral linewidth of this laser was about 2.255 kHz.
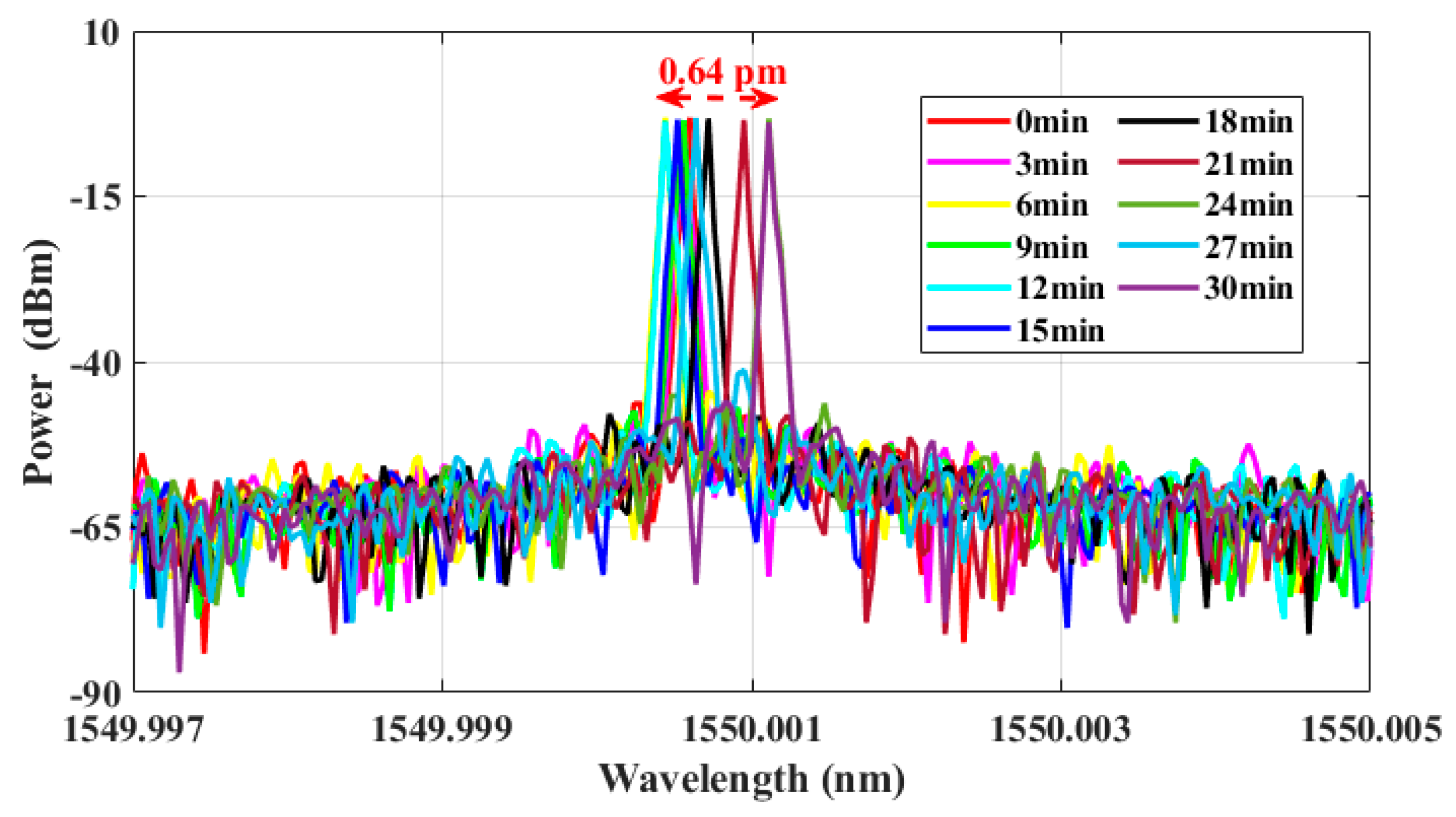
The long-term stability of the laser was monitored during a 30 min period. As depicted in
Figure 8, the optical spectrum of the generated laser signal exhibited a wavelength drift throughout the 30 min interval. The wavelength drift arose from internal noise within the laser device and interference induced by external environmental factors. The wavelength drift range of the PT symmetric fiber ring laser was approximately 0.64 pm.
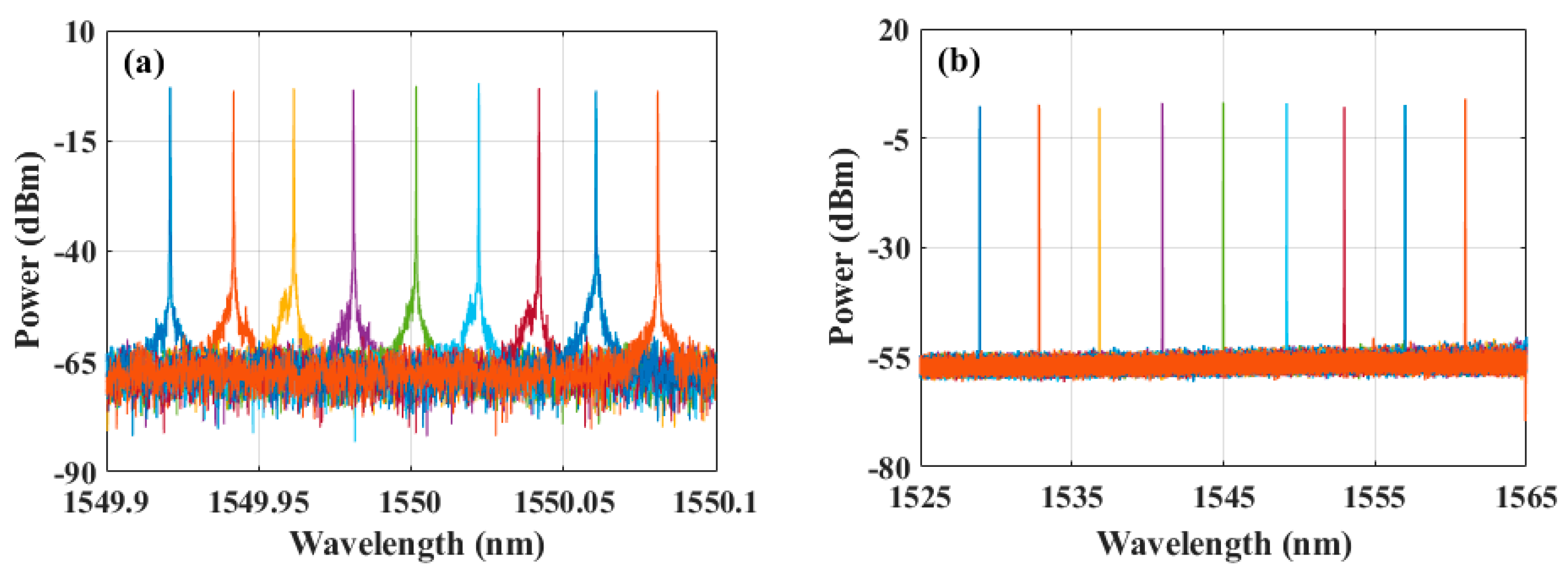
By adjusting the OTF, wavelength tunability of the single-mode laser could be achieved, as illustrated in
Figure 9.
Figure 9a shows the optical spectra of the laser when the wavelength was finely tuned with a step of 20 pm from 1549.92 nm to 1550.08 nm, and
Figure 9b shows the optical spectra of the laser when the wavelength was coarsely tuned with a step of 4 nm from 1529 nm to 1561 nm by tuning the OTF. The spectral range displayed in
Figure 9b spanned from 1525 nm to 1565 nm, a limitation imposed by the measurement range of the OSA. Without the constraints of the measuring range of the instrument, the tuning range of this laser would likely be broader. It can be observed from
Figure 9 that the tuning range of this laser covered the entirety of the C-band. It demonstrates that the polarization space PT symmetric fiber ring laser can effectively realize a wide wavelength tuning range.
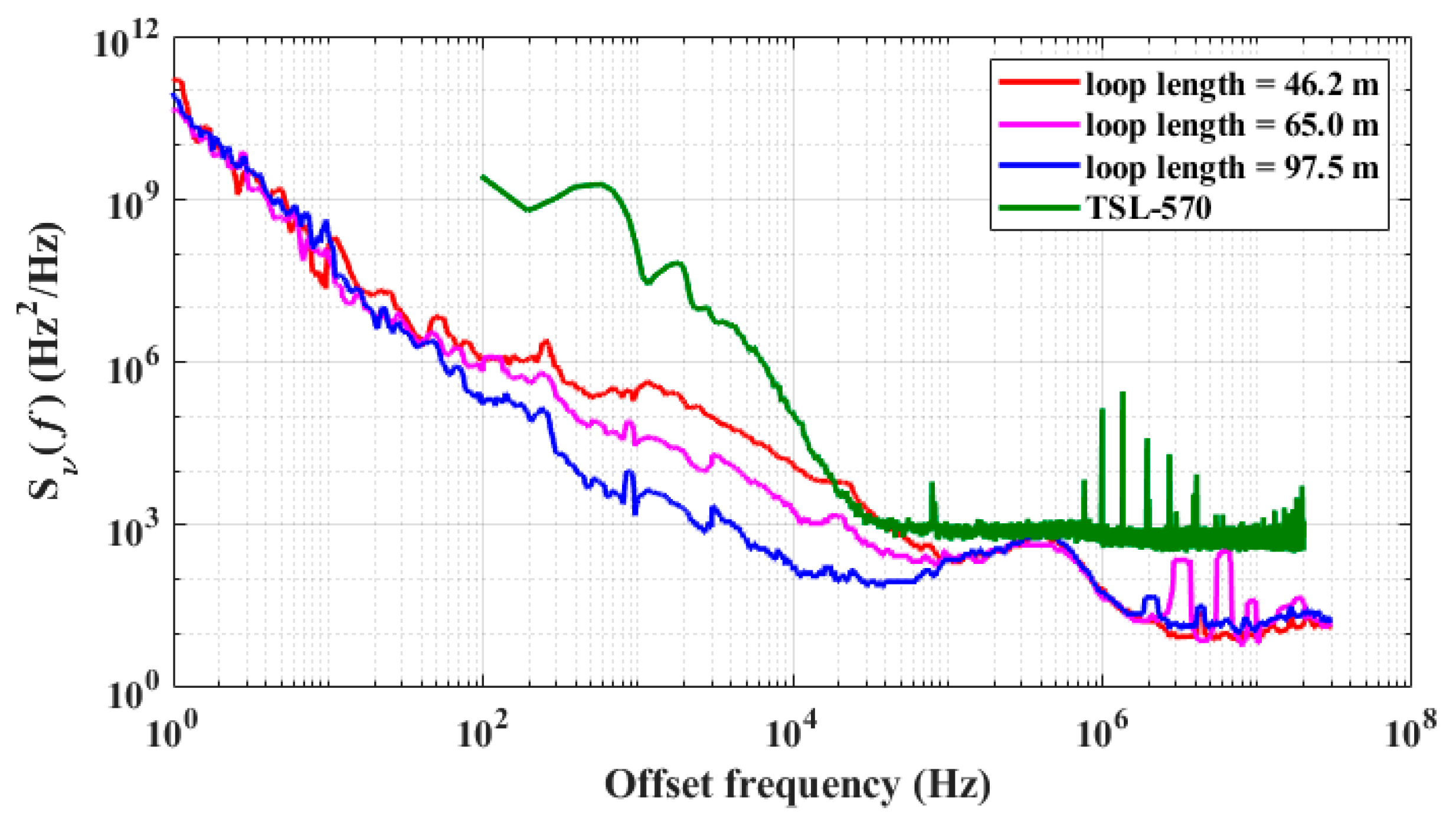
To investigate the influence of cavity length on the frequency noise of polarization space PT symmetric fiber ring lasers, the noise properties of single-mode optical signals generated by PT symmetry breaking in cavities with length of 46.2 m and 65.0 m were also examined, respectively.
Figure 10 depicts the frequency noise spectral density of the PT fiber lasers with different cavity length and a high-performance tunable laser (TSL-570). The red spectrum line represents the frequency noise spectral density for a cavity length of 46.2 m. The magenta spectrum line corresponds to the frequency noise spectral density for a cavity length of 65.0 m. The blue spectrum line corresponds to the frequency noise spectral density for a cavity length of 97.5 m. The green spectrum line represents the frequency noise spectral density of the TSL-570. As it can be observed from
Figure 10, within the offset frequency range of 10
2 Hz to 10
5 Hz, an increase in cavity length resulted in a notable suppression of the frequency noise of the output optical signal. Specifically, at an offset frequency of 10 kHz, the frequency noise spectral density was approximately 1.283 × 10
4 Hz
2/Hz for a cavity length of 46.2 m, 1.657 × 10
3 Hz
2/Hz for a cavity length of 65.0 m, and 181 Hz
2/Hz for a cavity length of 97.5 m. Moreover, the frequency noise of the PT fiber lasers with the three different cavity length was lower than that of the TSL-570. In contrast, the PT symmetric fiber ring lasers demonstrated excellent low noise characteristics.
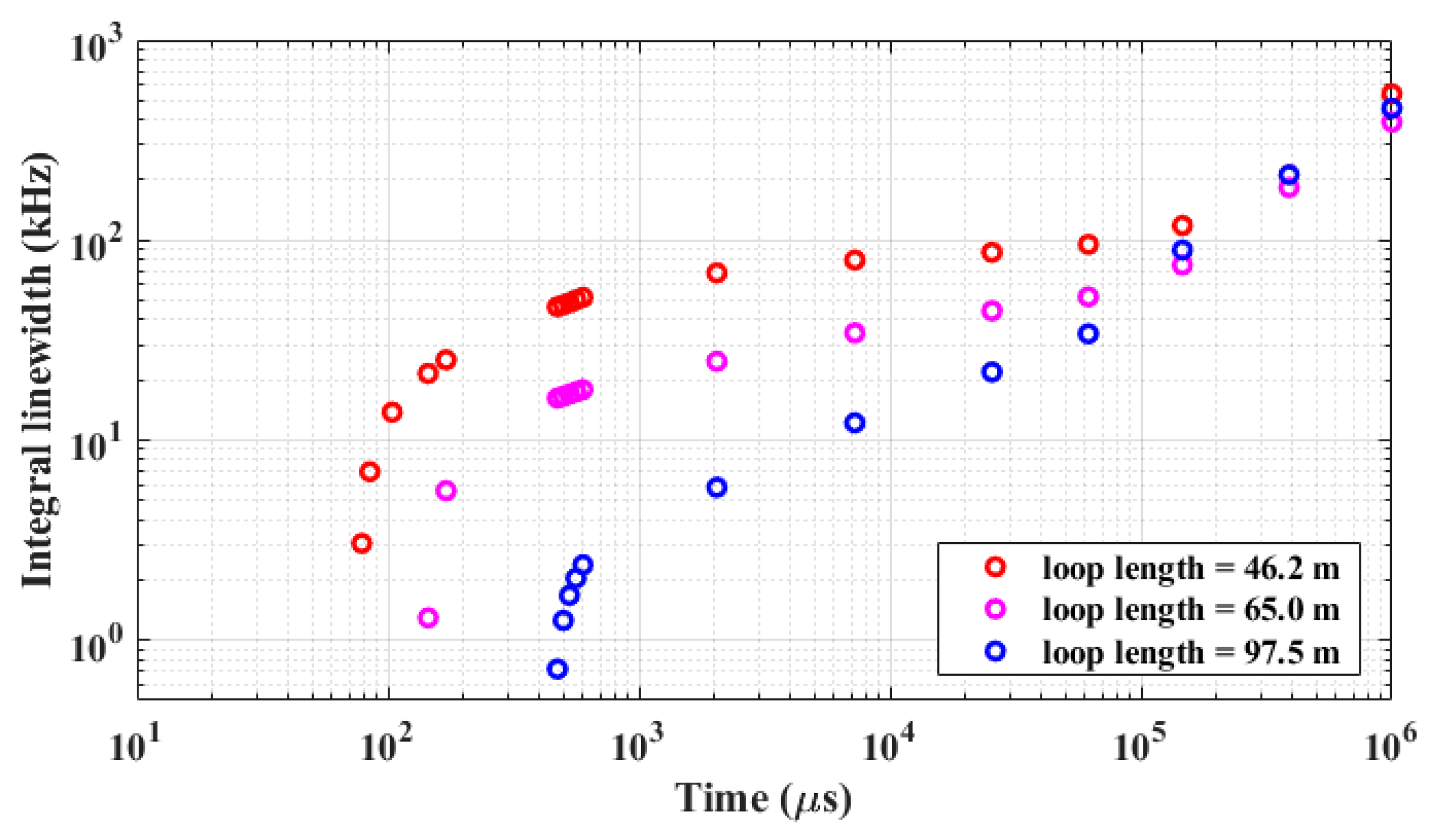
The integral linewidth with various observation timescales of PT symmetric fiber ring lasers with different cavity length can be calculated by the frequency noise spectral density, as illustrated in
Figure 11. The red circle represents the integral linewidth of the laser when the cavity length was 46.2 m, the magenta circle represents it at 65.0 m, and the blue circle represents it at 97.5 m. Specifically, at an observation time of 473.9 μs, the integral linewidth was approximately 46.47 kHz for a cavity length of 46.2 m, 16.25 kHz for a cavity length of 65.0 m, and 0.72 kHz for a cavity length of 97.5 m. When the observation time increased to 2.551 × 10
4 μs, the integral linewidth became approximately 86.97 kHz for a cavity length of 46.2 m, 44.32 kHz for a cavity length of 65.0 m, and 21.93 kHz for a cavity length of 97.5 m. Further increasing the observation time to 3.891 × 10
5 μs resulted in an integral linewidth of approximately 212.75 kHz for a cavity length of 46.2 m, 183.26 kHz for a cavity length of 65.0 m, and 212.27 kHz for a cavity length of 97.5 m. It is observable from
Figure 11 that the integral linewidth widened with an increasing observation time. However, at smaller observation timescales, there was a significant discrepancy among the three different cavity lengths which diminished gradually as the observation time increased.
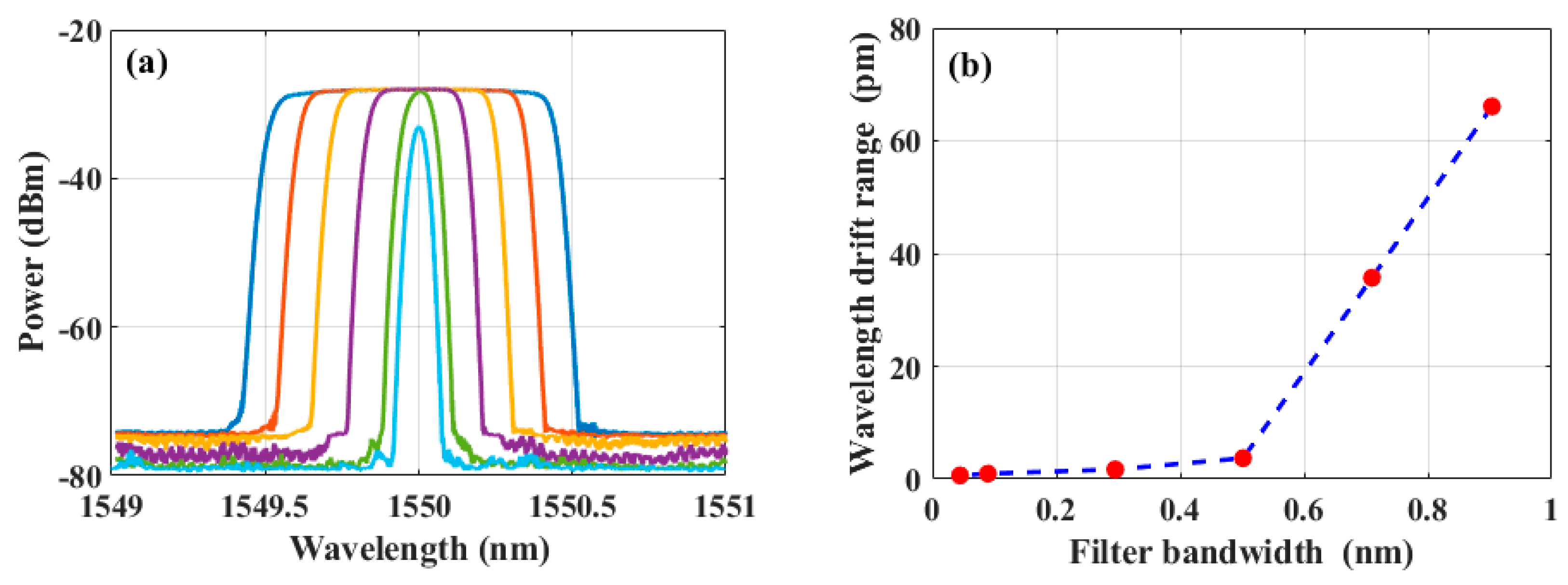
The roll-off characteristics of the OTF can affect the gain difference between the principal mode and the side modes. A larger gain difference leads to a reduced influence of the wavelength drift from internal noise fluctuations and environmental interference within the laser. Therefore, the wavelength drift of the PT symmetric fiber ring laser over a period of 30 min is demonstrated with the different bandwidth of the OTF in
Figure 12.
Figure 12a shows the transmittance spectra of the OTF with different bandwidth measured by the OSA.
Figure 12b illustrates the corresponding relationship between the bandwidth of the OTF and the wavelength drift range of the PT symmetric fiber ring laser during a 30 min period. As it can be observed from
Figure 12b, an increase in the bandwidth of the OTF resulted in a deterioration of the long-term stability of the laser. Particularly, the wavelength drift range began to increase significantly when the bandwidth exceeded 0.5015 nm. The reason why may be due to two factors. One is that the flat-top part in the transmittance spectrum of the OTF significantly expanded, enhancing the variation range of the potential maximum gain position. The other originates from the disturbance of internal noise and the external environment. Consequently, the drift range of the principal mode wavelength selected by the PT symmetry breaking mechanism was much larger than the longitudinal mode spacing. Therefore, the selection of an optical filter with a large roll-off is helpful to improve the stability of the PT symmetric fiber ring laser.