Mueller Matrix Polarizing Power
Abstract
1. Introduction
2. Theoretical Background
3. Polarizing Power
4. Invariance
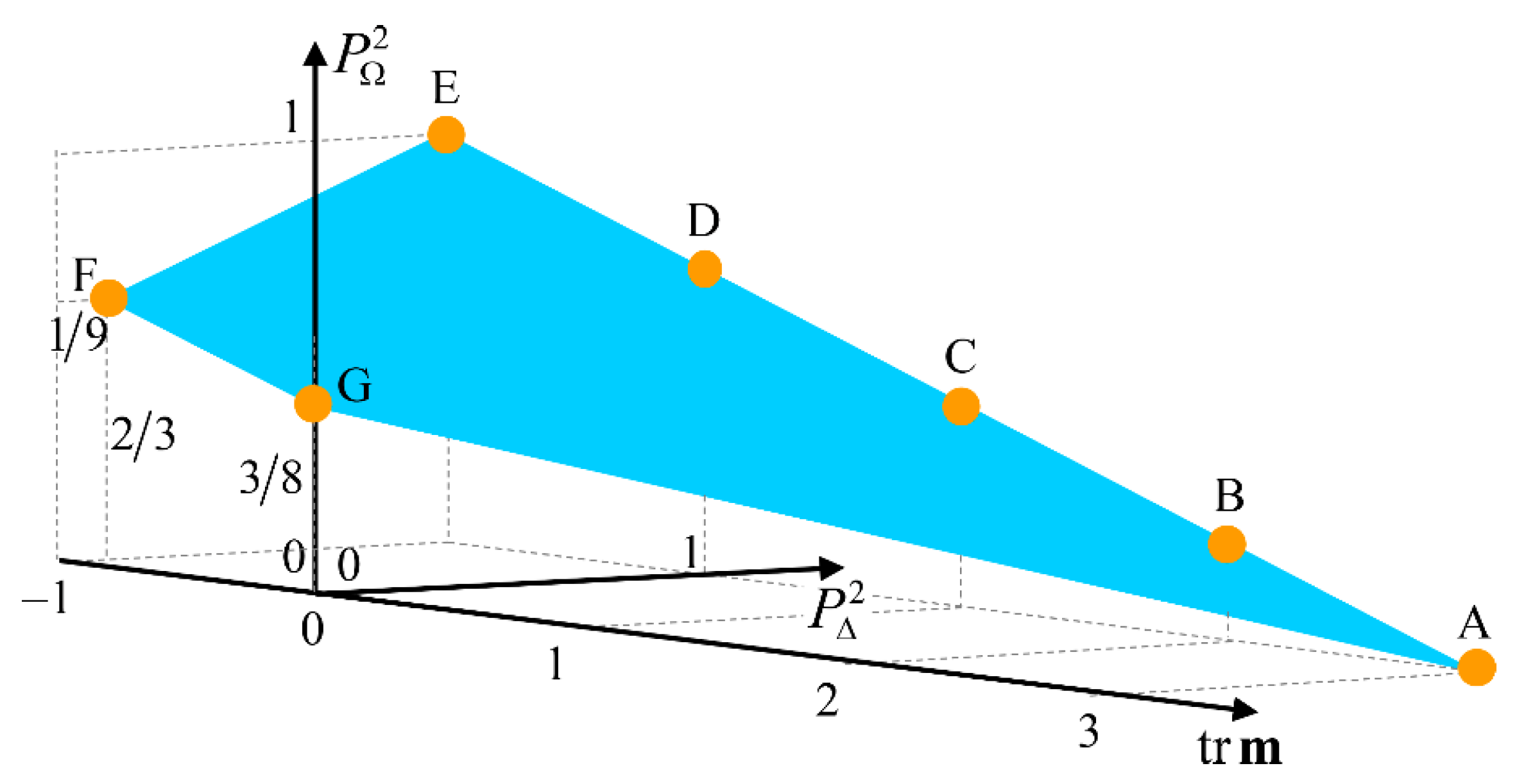
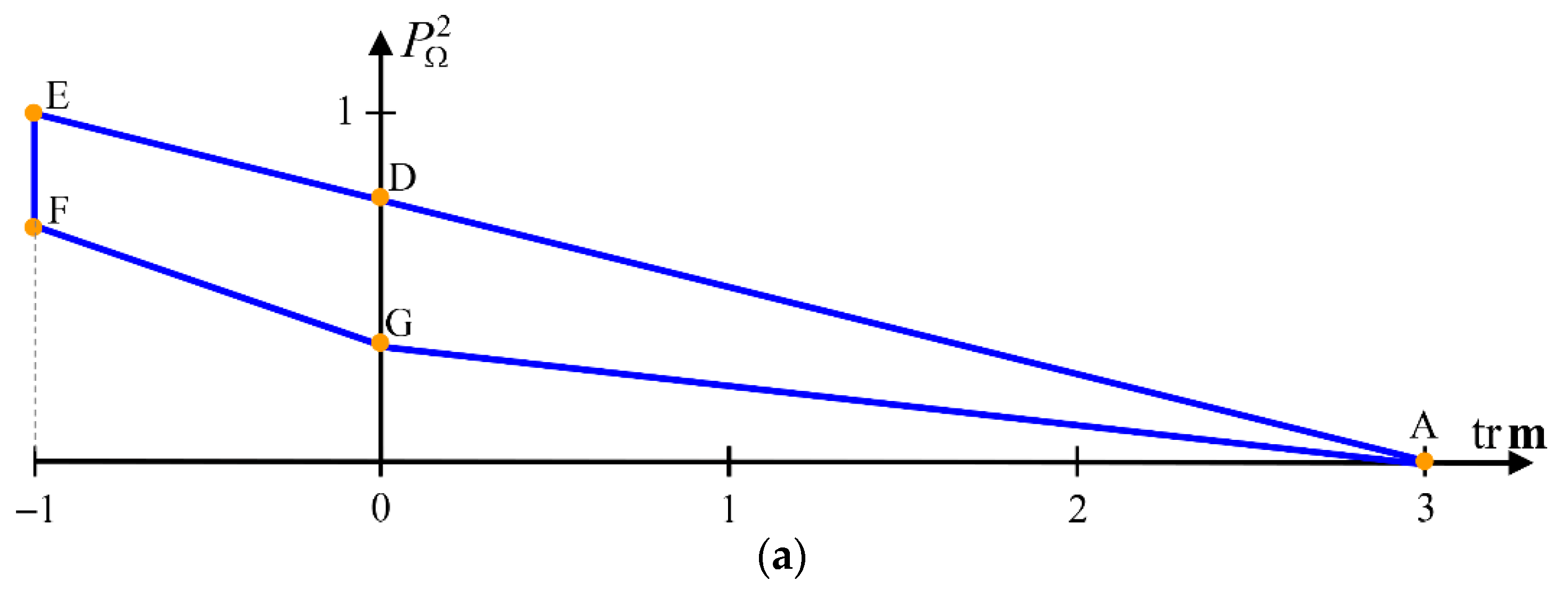
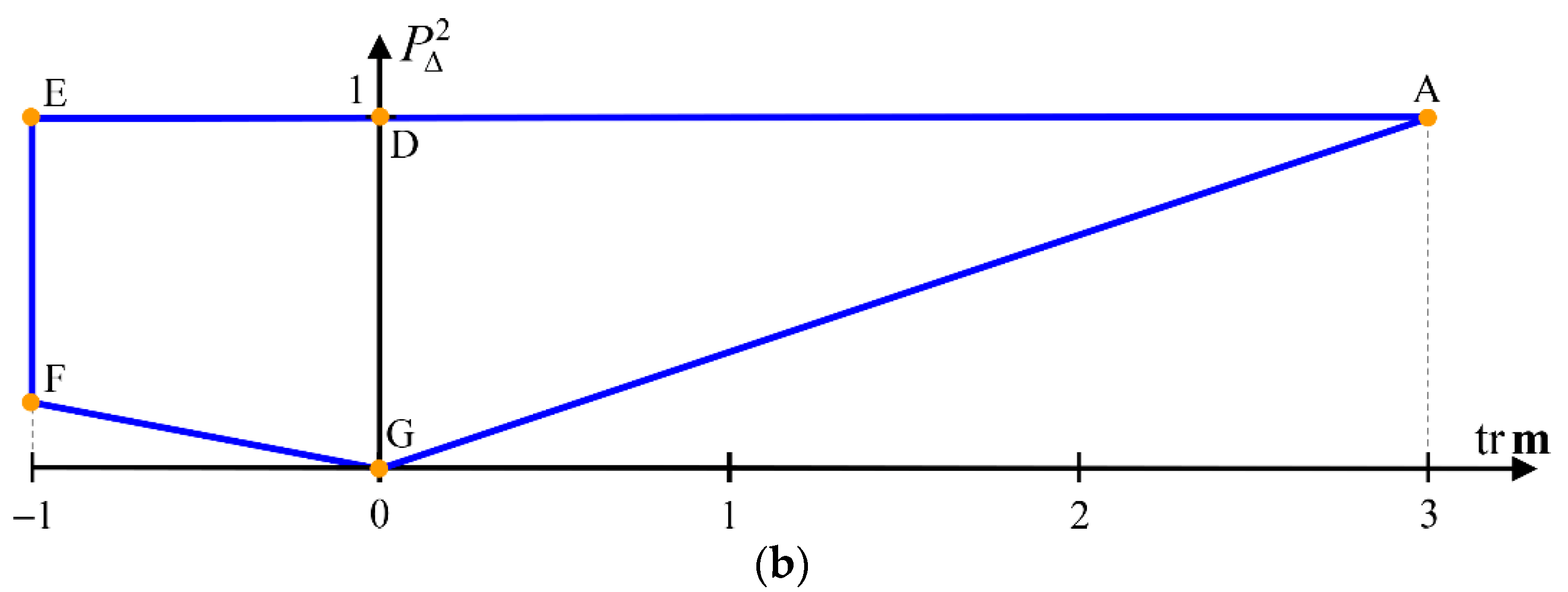
5. Polarizing Power of Typical Devices
5.1. Diattenuators
5.2. Retarders
5.3. Intrinsic Depolarizers
6. Conclusions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lu, S.-Y.; Chipman, R.A. Homogeneous and inhomogeneous Jones matrices. J. Opt. Soc. Am. A 1993, 11, 766–773. [Google Scholar] [CrossRef]
- Barakat, R. Polarization entropy transfer and relative polarization entropy. Opt. Commun. 1996, 123, 443–448. [Google Scholar] [CrossRef]
- Lu, S.-Y.; Chipman, R.A. Mueller matrices and the degree of polarization. Opt. Commun. 1998, 146, 11–14. [Google Scholar] [CrossRef]
- Tudor, T. On the enpolarization/depolarization effects of deterministic devices. Opt. Lett. 2018, 43, 5234–5237. [Google Scholar] [CrossRef]
- Gil, J.J. Polarimetric characterization of light and media. Physical quantities involved in polarimetric phenomena. Eur. Phys. J. Appl. Phys. 2007, 40, 1–47. [Google Scholar] [CrossRef]
- Gil, J.J.; Ossikovski, R. Polarized Light and the Mueller Matrix Approach, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2022. [Google Scholar]
- Gil, J.J.; Bernabéu, E. Depolarization and polarization indices of an optical system. Opt. Acta 1986, 33, 185–189. [Google Scholar] [CrossRef]
- Lu, S.-Y.; Chipman, R.A. Interpretation of Mueller matrices based on polar decomposition. J. Opt. Soc. Am. A 1996, 13, 1106–1113. [Google Scholar] [CrossRef]
- Chipman, R.A. Depolarization index and the average degree of polarization. Appl. Opt. 2005, 44, 2490–2495. [Google Scholar] [CrossRef]
- Espinosa-Luna, R.; Bernabeu, E.; Atondo-Rubio, G. Q(M) and the depolarization index scalar metrics. Appl. Opt. 2008, 47, 1575–1580. [Google Scholar] [CrossRef] [PubMed]
- Ossikovski, R. Alternative depolarization criteria for Mueller matrices. J. Opt. Soc. Am. A 2010, 27, 808–814. [Google Scholar] [CrossRef] [PubMed]
- Espinosa-Luna, R.; Bernabeu, E.; Atondo-Rubio, G.; Hinojosa-Ruíz, S. A simple depolarization criterion for light. Optik 2011, 122, 407–410. [Google Scholar] [CrossRef]
- San José, I.; Gil, J.J. Invariant indices of polarimetric purity: Generalized indices of purity for n×n covariance matrices. Opt. Commun. 2011, 284, 38–47. [Google Scholar] [CrossRef]
- Cloude, S.R. Depolarization synthesis: Understanding the optics of Mueller matrix depolarization. J. Opt. Soc. Am. A 2013, 30, 691–700. [Google Scholar] [CrossRef]
- Gil, J.J. Structure of polarimetric purity of a Mueller matrix and sources of depolarization. Opt. Commun. 2016, 368, 165–173. [Google Scholar] [CrossRef]
- Hilfiker, J.N.; Hale, J.S.; Herzinger, C.M.; Tiwald, T.; Hong, N.; Schöche, S.; Arwin, H. Estimating Depolarization with the Jones Matrix Quality Factor. Appl. Surf. Sci. 2017, 421, 494–499. [Google Scholar] [CrossRef]
- Tariq, A.; Li, P.; Chen, D.; Lv, D.; Ma, H. Physically Realizable Space for the Purity-Depolarization Plane for Polarized Light Scattering Media. Phys. Rev. Lett. 2017, 119, 033202. [Google Scholar] [CrossRef]
- Ossikovski, R.; Vizet, J. Eigenvalue-based depolarization metric spaces for Mueller matrices. J. Opt. Soc. Am. A 2019, 36, 1173–1186. [Google Scholar] [CrossRef]
- Sheppard, C.J.R.; Bendandi, A.; Le Gratiet, A.; Diaspro, A. Characterization of the Mueller Matrix: Purity Space and Reflectance Imaging. Photonics 2022, 9, 88. [Google Scholar] [CrossRef]
- Ossikovski, R. Analysis of depolarizing Mueller matrices through a symmetric decomposition. J. Opt. Soc. Am. A 2009, 26, 1109–1118. [Google Scholar] [CrossRef] [PubMed]
- Gil, J.J. Transmittance constraints in serial decompositions of Mueller matrices. The arrow form of a Mueller matrix. J. Opt. Soc. Am. A 2013, 30, 701–707. [Google Scholar] [CrossRef] [PubMed]
- Gil, J.J.; Ossikovski, R.; San José, I. Physical Significance of the Determinant of a Mueller Matrix. Photonics 2022, 9, 246. [Google Scholar] [CrossRef]
- Gil, J.J. Thermodynamic Reversibility in Polarimetry. Photonics 2022, 9, 650. [Google Scholar] [CrossRef]
- Cloude, S.R. Group theory and polarization algebra. Optik 1986, 75, 26–36. [Google Scholar]
- Kim, K.; Mandel, L.; Wolf, E. Relationship between Jones and Mueller matrices for random media. J. Opt. Soc. Am. A 1987, 4, 433–437. [Google Scholar] [CrossRef]
- Xing, Z.-F. On the Deterministic and Non-deterministic Mueller Matrix. J. Mod. Opt. 1992, 39, 461–484. [Google Scholar] [CrossRef]
- Sridhar, R.; Simon, R. Normal form for Mueller Matrices in Polarization Optics. J. Mod. Opt. 1994, 41, 1903–1915. [Google Scholar] [CrossRef]
- van der Mee, C.V.M. An eigenvalue criterion for matrices. transforming Stokes parameters. J. Math. Phys. 1993, 34, 5072–5088. [Google Scholar] [CrossRef]
- Bolshakov, Y.; van der Mee, C.V.M.; Ran, A.C.M.; Reichstein, B.; Rodman, L. Polar decompositions in finite dimensional indefinite scalar product spaces: Special cases and applications. In Operator Theory: Advances and Applications; Gohberg, I., Ed.; Birkhäuser: Basel, Switzerland, 1996; Volume 87, pp. 61–94. [Google Scholar]
- Bolshakov, Y.; van der Mee, C.V.M.; Ran, A.C.M.; Reichstein, B.; Rodman, L. Errata for: Polar decompositions in finite di-mensional indefinite scalar product spaces: Special cases and applications. Integral Equ. Oper. Theory 1997, 27, 497–501. [Google Scholar] [CrossRef]
- Rao, A.V.G.; Mallesh, K.S.; Sudha. On the algebraic characterization of a Mueller matrix in polarization optics. I. Identifying a Mueller matrix from its N matrix. J. Mod. Opt. 1998, 45, 955–987. [Google Scholar] [CrossRef]
- Rao, A.V.G.; Mallesh, K.S.; Sudha. On the algebraic characterization of a Mueller matrix in polarization optics. II. Necessary and sufficient conditions for Jones derived Mueller matrices. J. Mod. Opt. 1998, 45, 989–999. [Google Scholar]
- Gil, J.J. Characteristic properties of Mueller matrices. J. Opt. Soc. Am. A 2000, 17, 328–334. [Google Scholar] [CrossRef] [PubMed]
- Simon, B.N.; Simon, S.; Mukunda, N.; Gori, F.; Santarsiero, M.; Borghi, R.; Simon, R. A complete characterization of pre-Mueller and Mueller matrices in polarization optics. J. Opt. Soc. Am. A 2010, 27, 188–199. [Google Scholar] [CrossRef] [PubMed]
- Barakat, R. Conditions for the Physical Realizability of Polarization Matrices Characterizing Passive Systems. J. Mod. Opt. 1987, 34, 1535–1544. [Google Scholar] [CrossRef]
- Brosseau, C.; Barakat, R. Jones and Mueller polarization matrices for random media. Opt. Commun. 1991, 84, 127–132. [Google Scholar] [CrossRef]
- Kostinski, A.B.; Givens, R.C. On the gain of a passive linear depolarizing system. J. Mod. Opt. 1992, 39, 1947–1952. [Google Scholar] [CrossRef]
- Devlaminck, V.; Terrier, P. Non-singular Mueller matrices characterizing passive systems. Optik 2010, 121, 1994–1997. [Google Scholar] [CrossRef]
- San José, I.; Gil, J.J. Characterization of passivity in Mueller matrices. J. Opt. Soc. Am. A 2020, 37, 199–208. [Google Scholar] [CrossRef]
- Arnal, P.M. Modelo Matricial para el Estudio de Fenómenos de Polarización de la luz. Ph.D. Thesis, University of Zaragoza, Zaragoza, Spain, 1990. [Google Scholar]
- Savenkov, S.N.; Muttiah, R.S.; Sydoruk, O.I. Conditions for polarization elements to be dichroic and birefringent. J. Opt. Soc. Am. A 2005, 22, 1447–1452. [Google Scholar] [CrossRef]
- Savenkov, S.N.; Sydoruk, O.I.; Muttiah, R.S. Eigenanalysis of dichroic, birefringent, and degenerate polarization elements: A Jones-calculus study. Appl. Opt. 2007, 46, 6700–6709. [Google Scholar] [CrossRef]
- Ossikovski, R. Interpretation of nondepolarizing Mueller matrices based on singular-value decomposition. J. Opt. Soc. Am. A 2008, 25, 473–482. [Google Scholar] [CrossRef]
- Robson, B.A. The Theory of Polarization Phenomena; Clarendon Press: Oxford, UK, 1975. [Google Scholar]
- Gil, J.J. Components of purity of a Mueller matrix. J. Opt. Soc. Am. A 2011, 28, 1578–1585. [Google Scholar] [CrossRef]
- Arteaga, O.; Garcia-Caurel, E.; Ossikovski, R. Anisotropy coefficients of a Mueller matrix. J. Opt. Soc. Am. A 2011, 28, 548–553. [Google Scholar] [CrossRef] [PubMed]
- Sekera, Z. Scattering Matrices and Reciprocity Relationships for Various Representations of the State of Polarization. J. Opt. Soc. Am. 1966, 56, 1732–1740. [Google Scholar] [CrossRef]
- Schönhofer, A.; Kuball, H.-G. Symmetry properties of the Mueller matrix. Chem. Phys. 1987, 115, 159–167. [Google Scholar] [CrossRef]
- Gil, J.J. Invariant quantities of a Mueller matrix under rotation and retarder transformations. J. Opt. Soc. Am. A 2016, 33, 52–58. [Google Scholar] [CrossRef]
- Theocaris, P.S.; Gdoutos, E.E. Matrix Theory of Photoelasticity; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1979; ISBN 9780387215853. [Google Scholar]
- Gil, J.J.; San José, I. Two-vector representation of a nondepolarizing Mueller matrix. Opt. Commun. 2016, 374, 133–141. [Google Scholar] [CrossRef]
- Azzam, R.M.A.; Bashara, N.M. Ellipsometry and Polarized Light; North Holland: Amsterdam, The Netherlands, 1977. [Google Scholar]
- Tudor, T. Operatorial form of the theory of polarization optical devices: I. Spectral theory of the basic devices. Optik 2003, 114, 539–547. [Google Scholar] [CrossRef]
- Tudor, T. Operatorial form of the theory of polarization optical devices: II. Spectral theory of composite devices. Optik 2004, 115, 173–180. [Google Scholar] [CrossRef]
- Tudor, T.; Gheondea, A. Pauli algebraic forms of normal and non-normal operators. J. Opt. Soc. Am. A 2007, 24, 204–210. [Google Scholar] [CrossRef]
- Tudor, T. Interaction of light with the polarization devices: A vectorial Pauli algebraic approach. J. Phys. A Math. Theor. 2008, 41, 415303. [Google Scholar] [CrossRef]
- Ossikovski, R. Canonical forms of depolarizing Mueller matrices. J. Opt. Soc. Am. A 2010, 27, 123–130. [Google Scholar] [CrossRef] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gil, J.J. Mueller Matrix Polarizing Power. Photonics 2024, 11, 411. https://doi.org/10.3390/photonics11050411
Gil JJ. Mueller Matrix Polarizing Power. Photonics. 2024; 11(5):411. https://doi.org/10.3390/photonics11050411
Chicago/Turabian StyleGil, José J. 2024. "Mueller Matrix Polarizing Power" Photonics 11, no. 5: 411. https://doi.org/10.3390/photonics11050411
APA StyleGil, J. J. (2024). Mueller Matrix Polarizing Power. Photonics, 11(5), 411. https://doi.org/10.3390/photonics11050411




