A Novel Inserting Pilot Radio over Fiber System without the Bit Walk-Off Effect for the Generation and Distribution of Frequency 16-Tupling Millimeter Waves by Mach–Zehnder Modulators
Abstract
1. Introduction
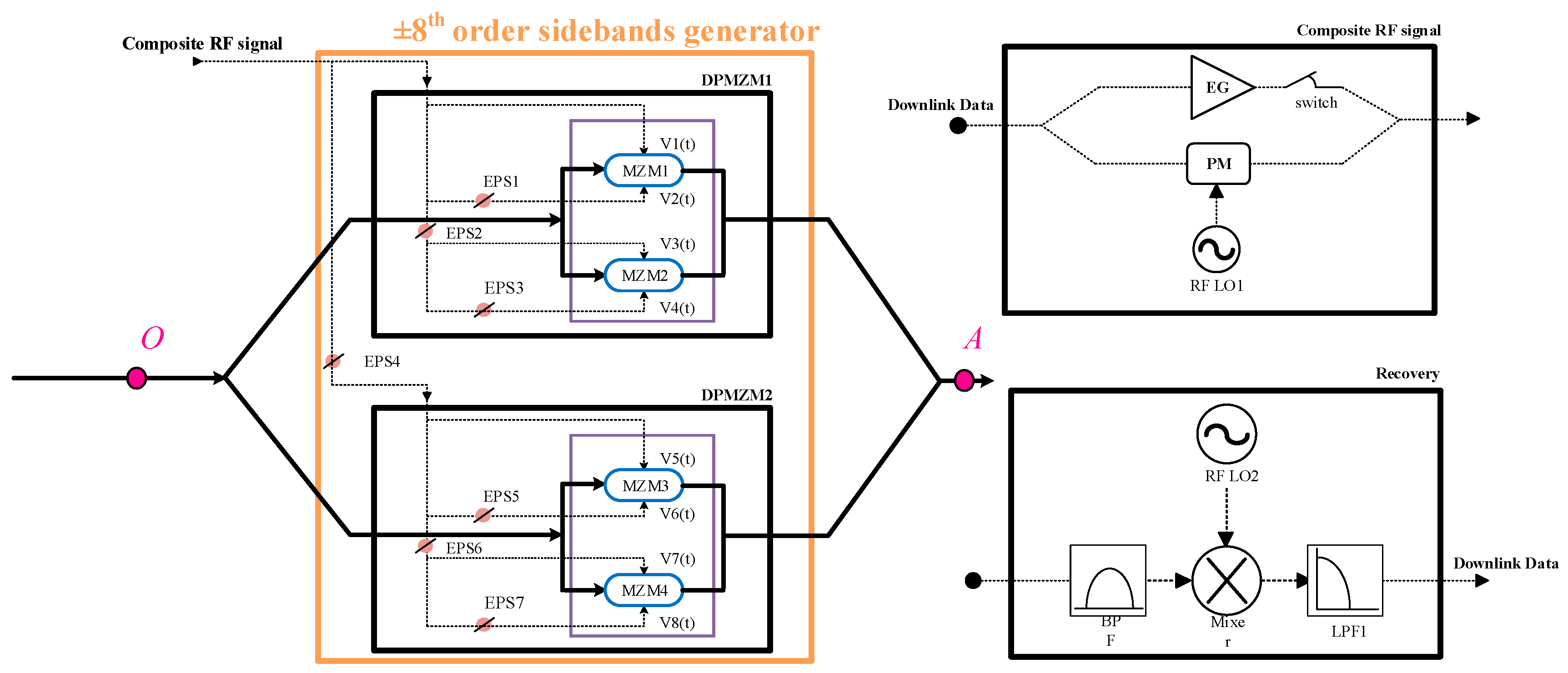
2. System Design
3. Operation Principle
3.1. The Expression of the Output from MZMs
3.2. Selection of the Modulation Index of MZM
3.3. Output of the Central Station
3.4. Effect of Fiber Optic Dispersion on the Generated Frequency 16-Tupling MMW
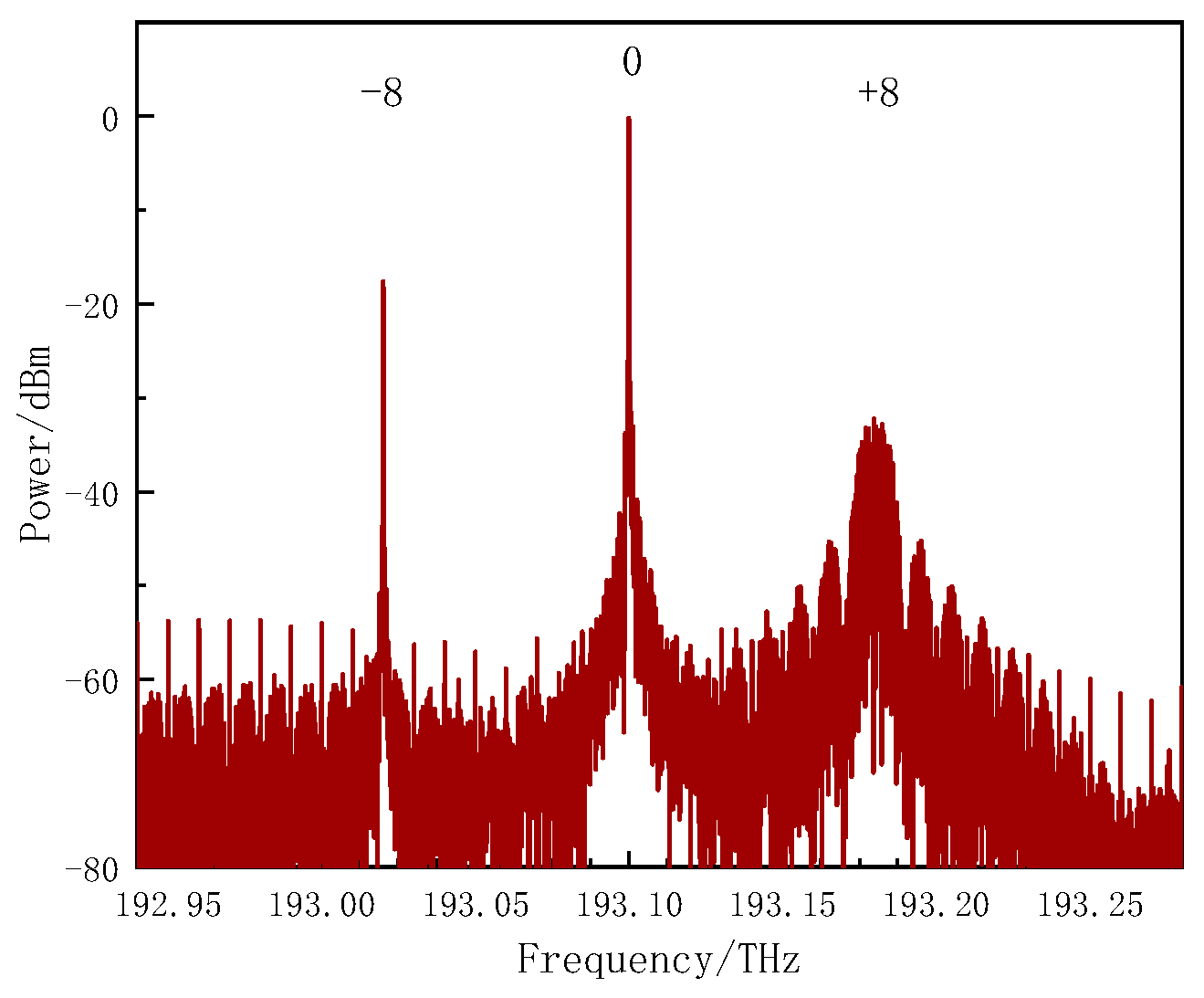
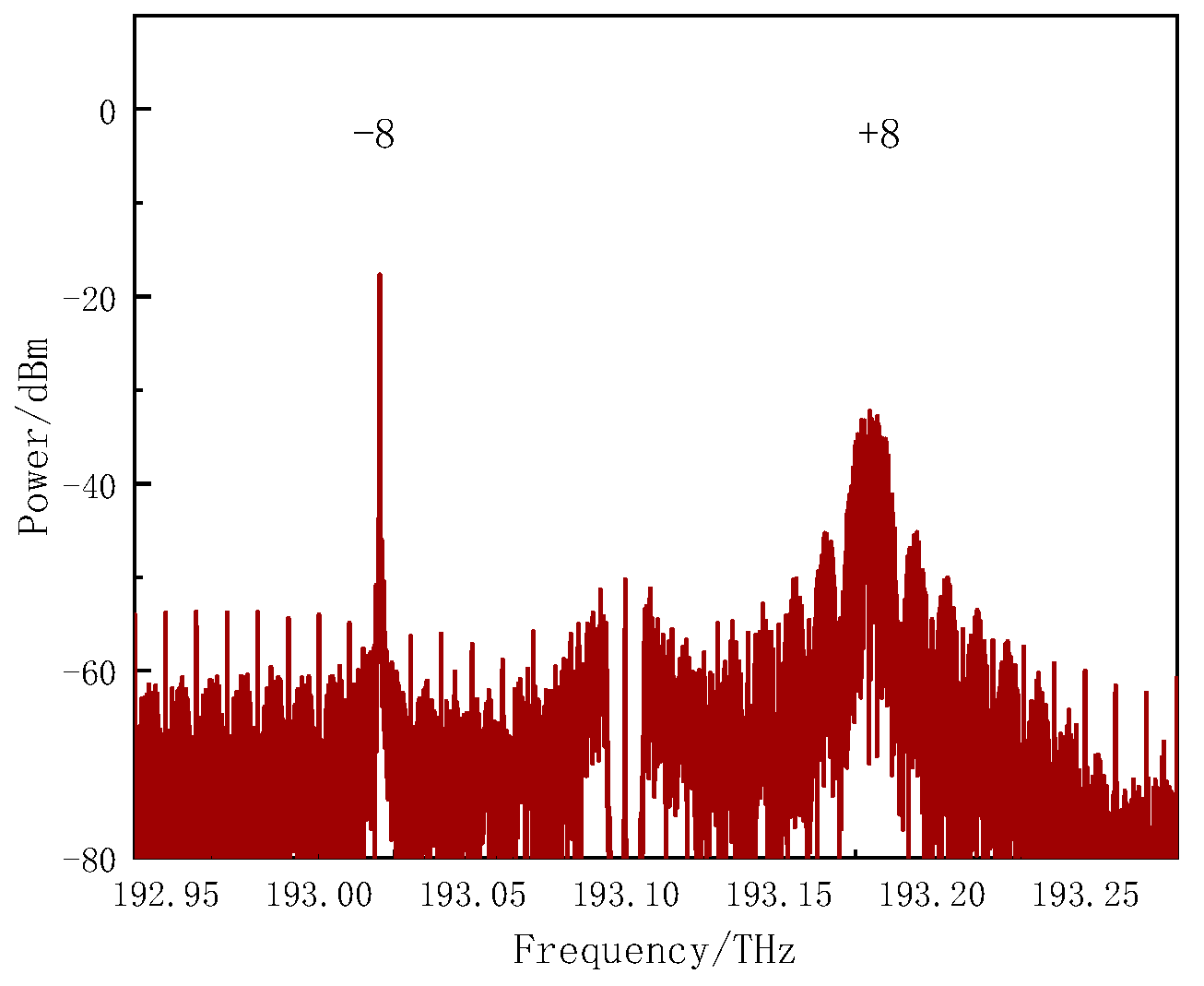
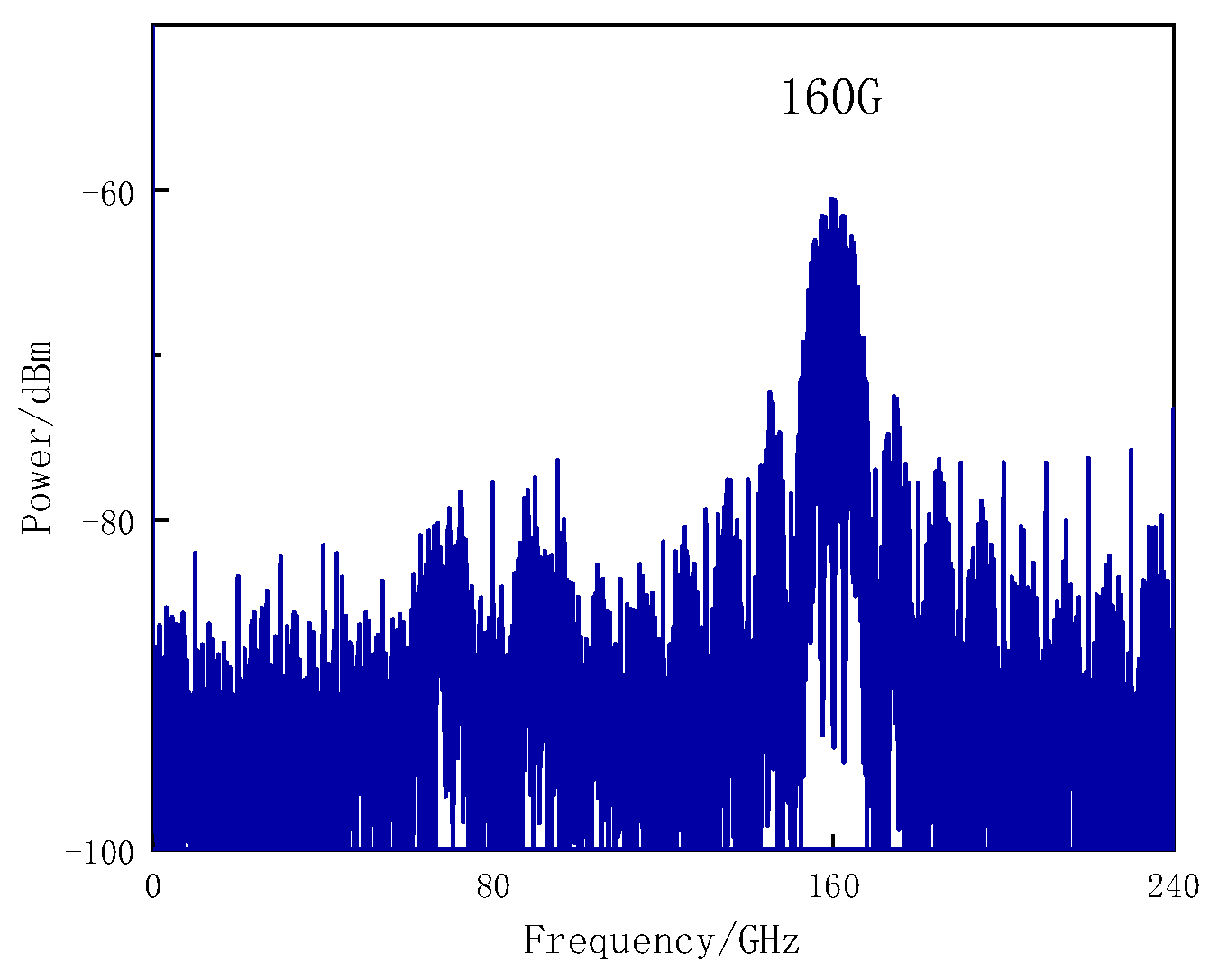
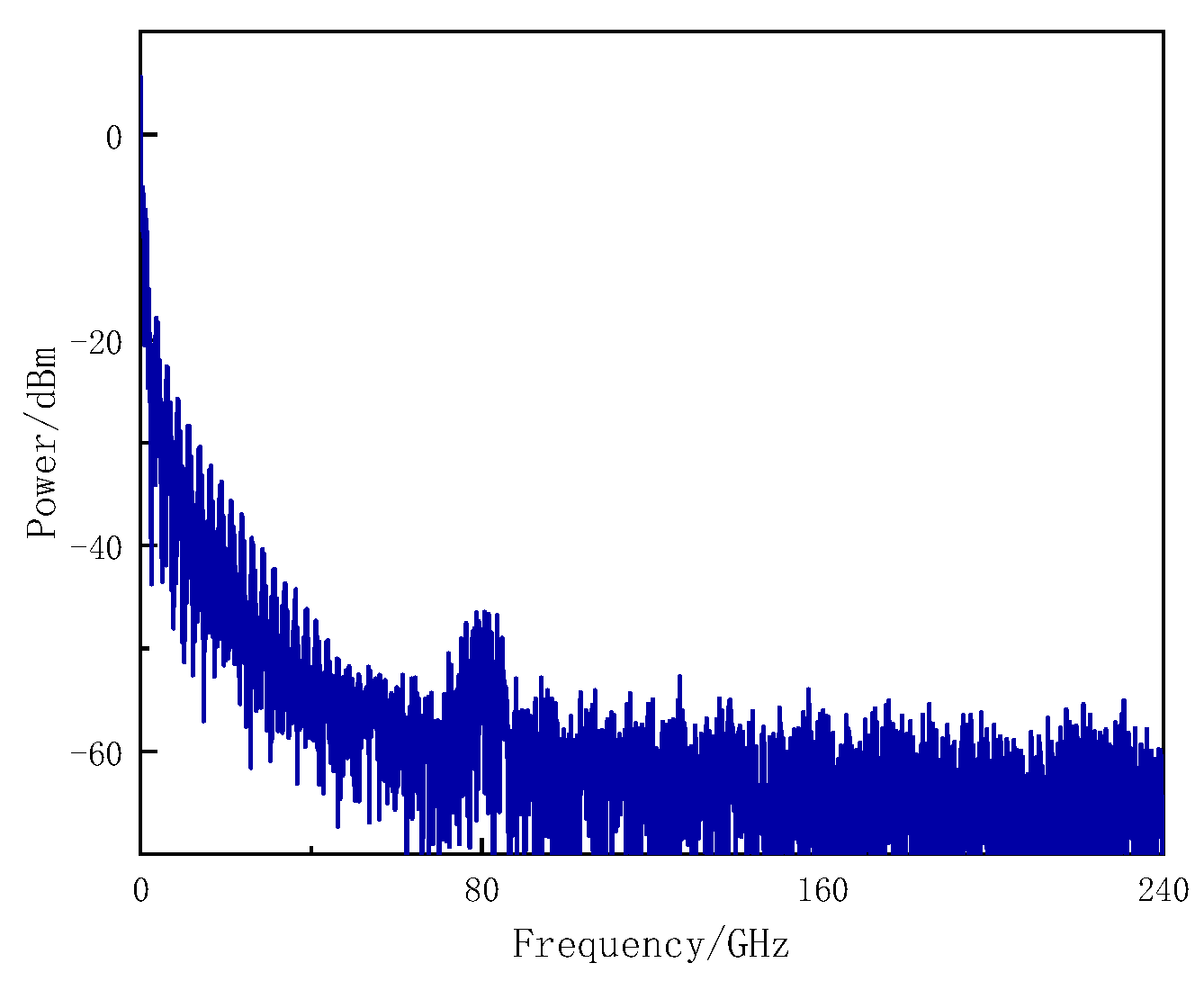
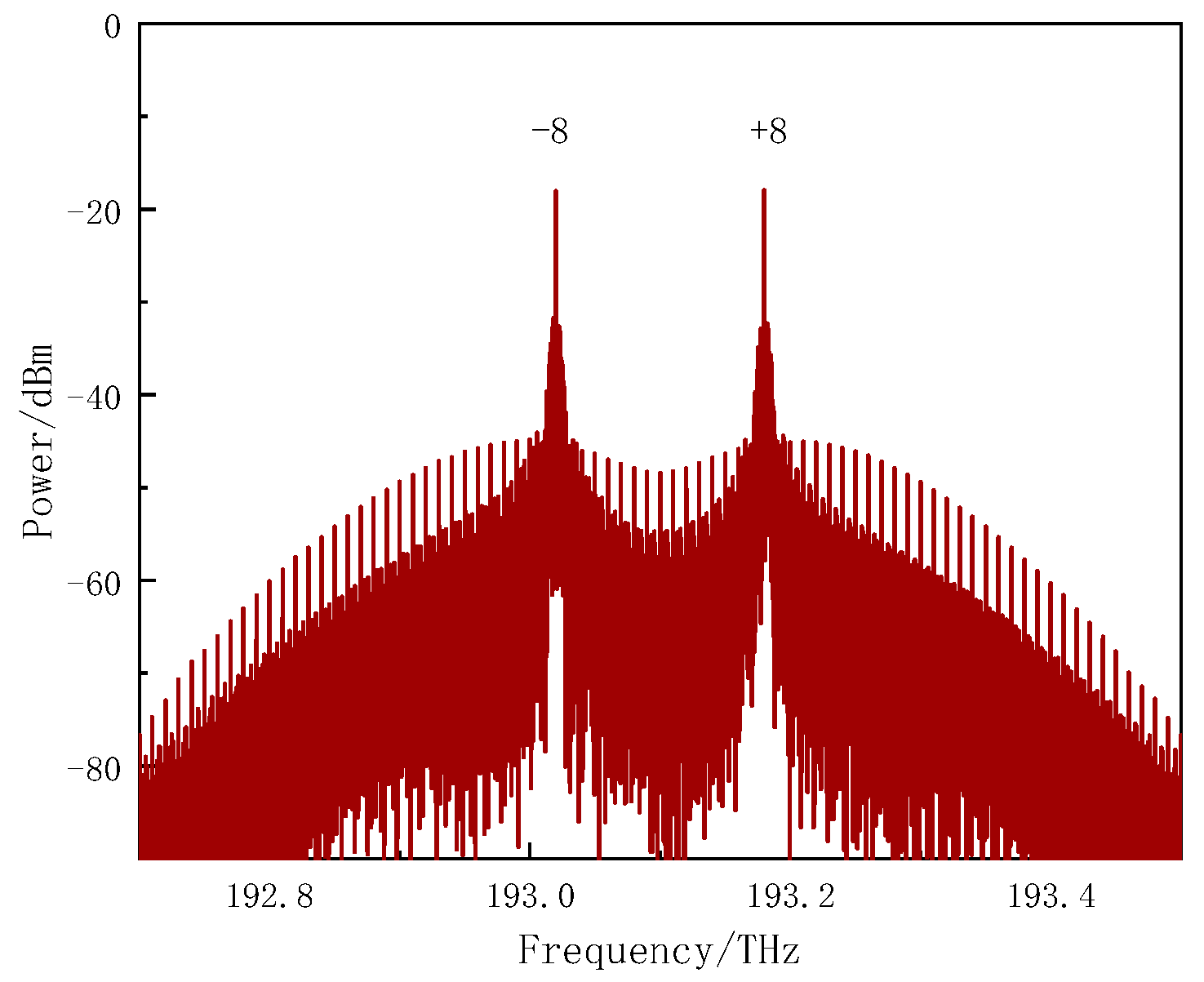
4. Simulation Experiment
4.1. Simulation Parameter
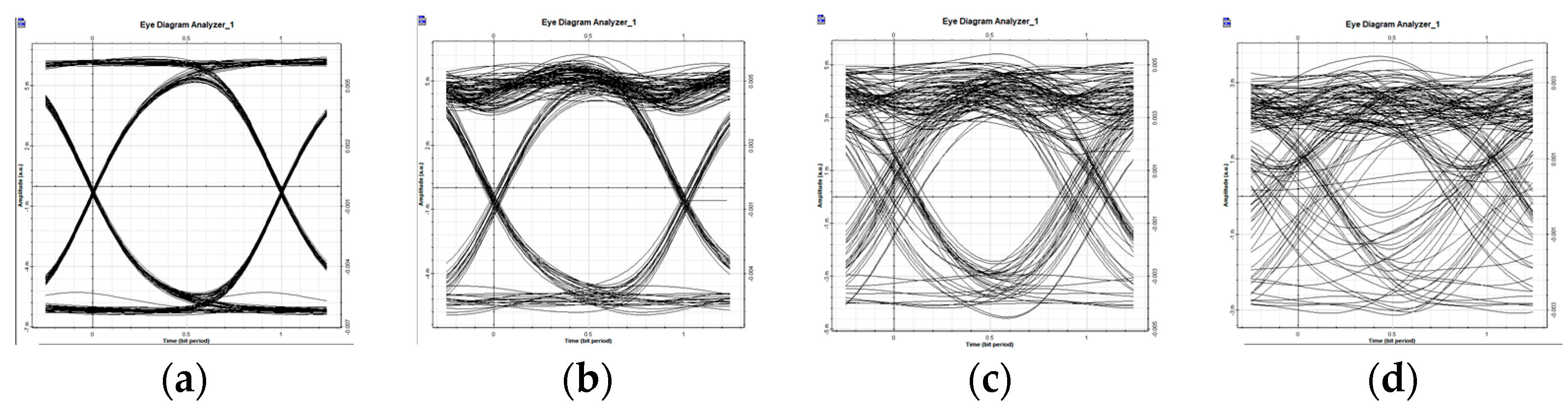
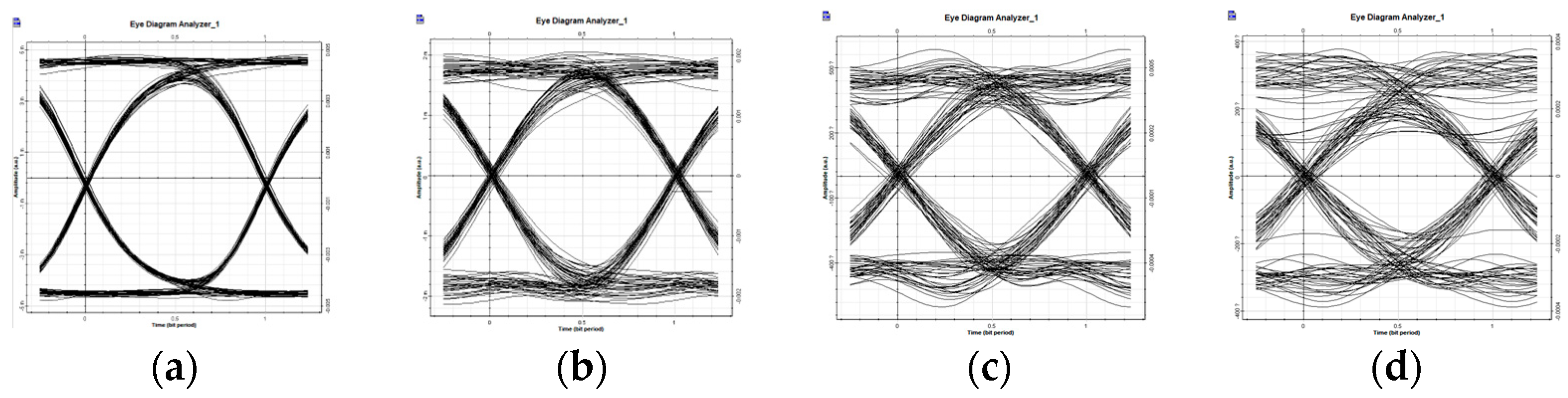
4.2. Results of the Simulation Experiment
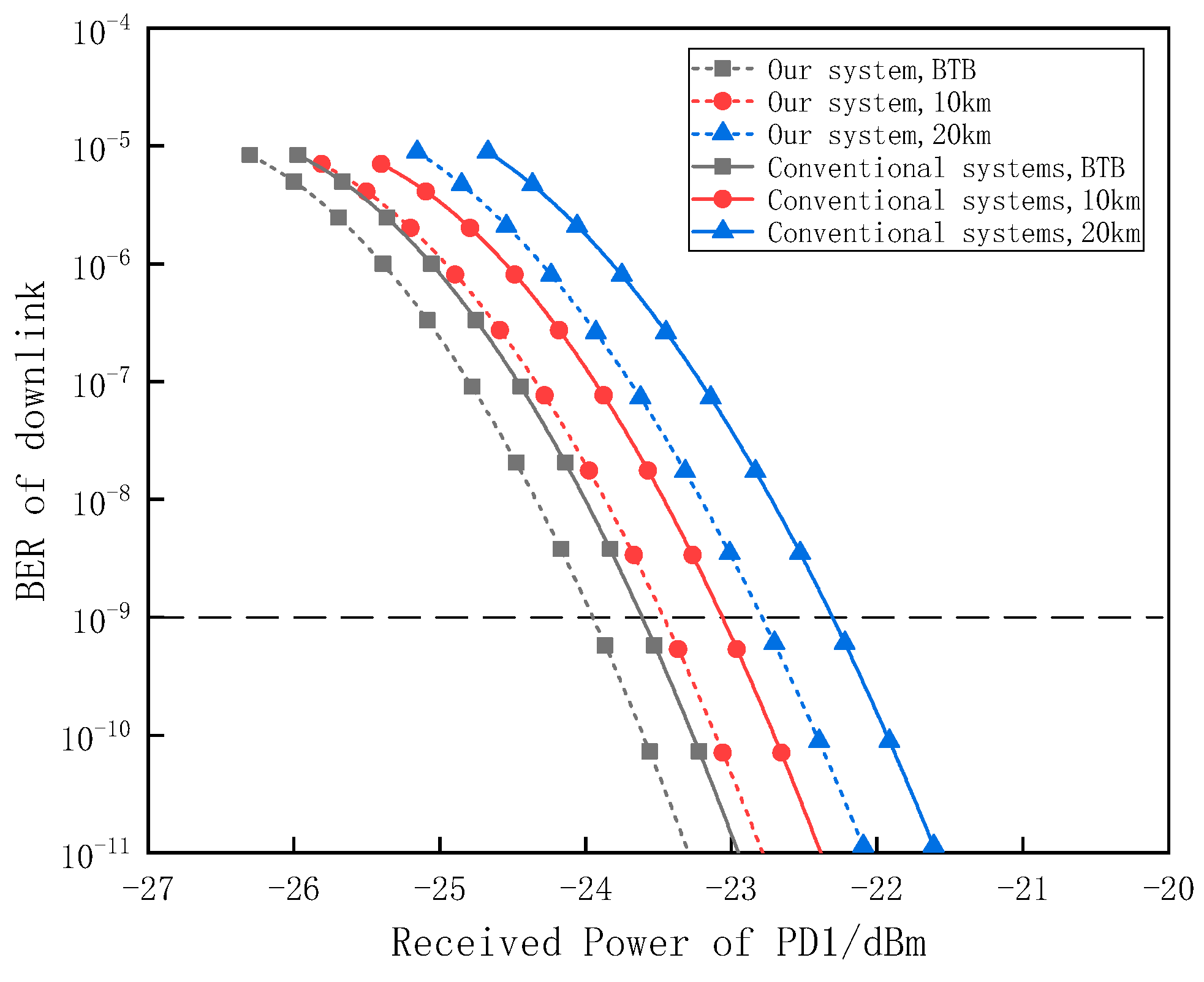
4.3. Compare the Conventional Frequency 16-Tupling ROF System with Our Scheme
4.4. Compare the Conventional Carrier Reuse System with Our Scheme
5. Stability of System
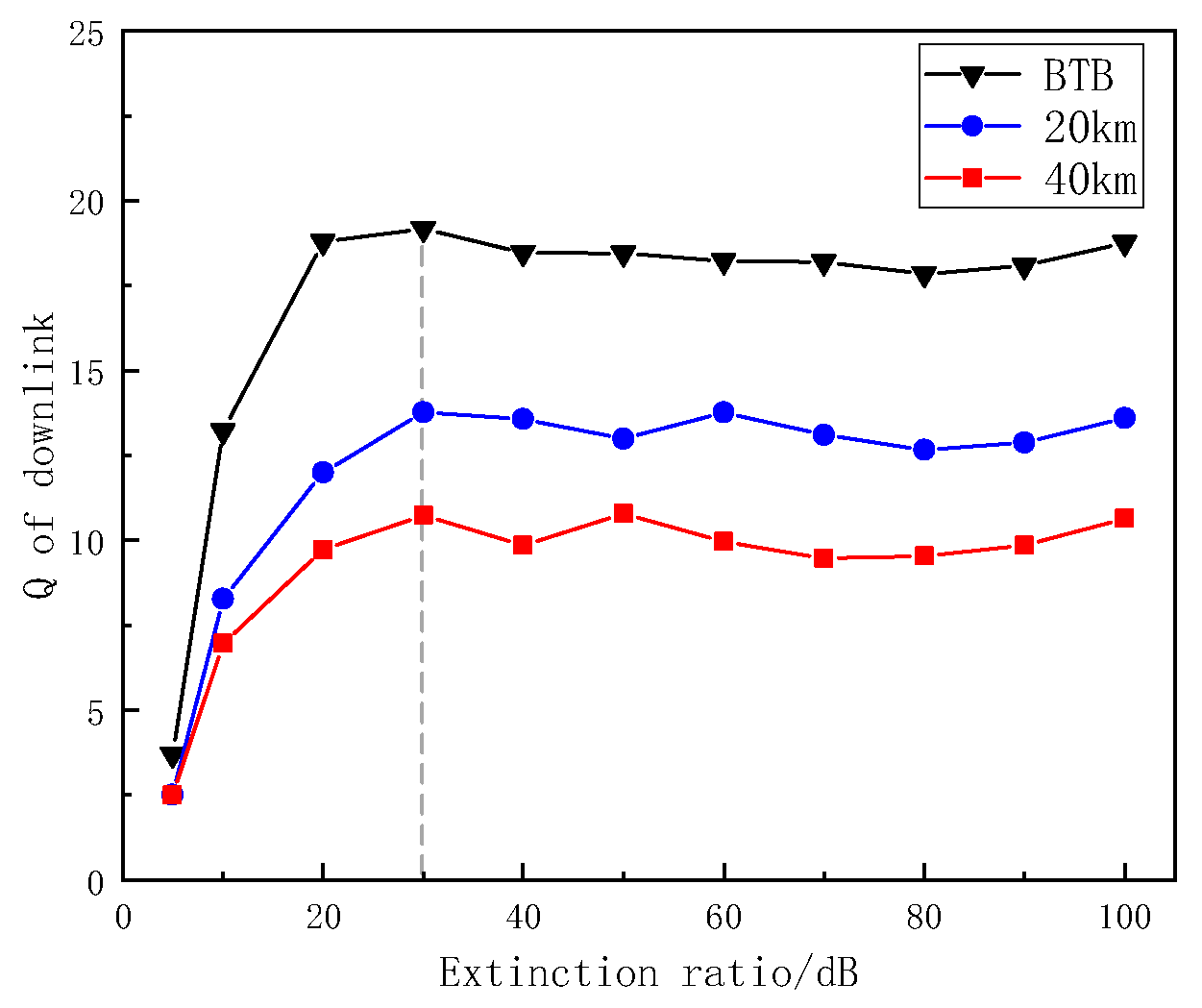
5.1. Effect of Extinction Ratio of MZMs
5.2. Effect of the Phase Modulation Index P of the Phase Modulator PM
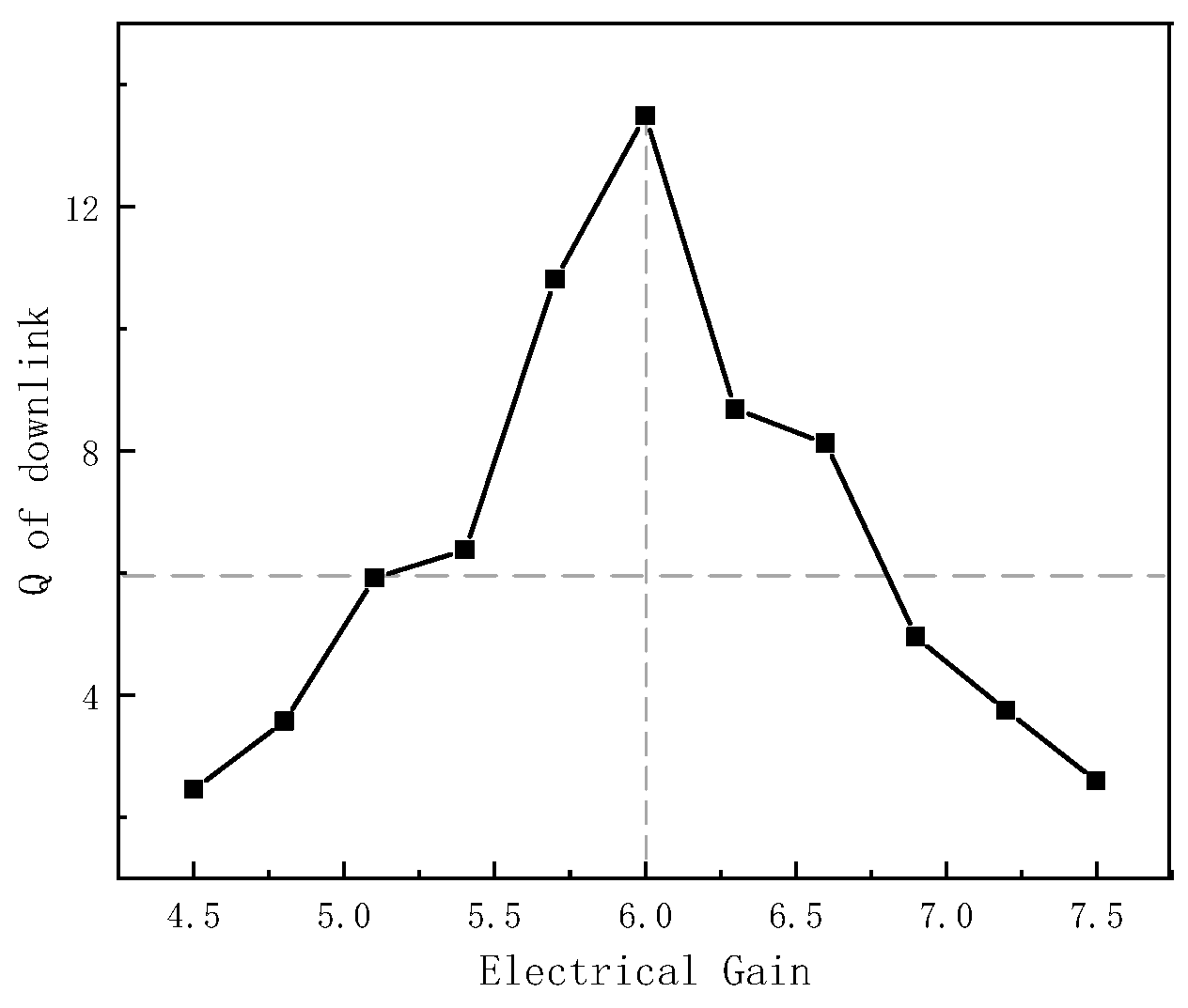
5.3. Effect of the Amplification G of the Electrical EG
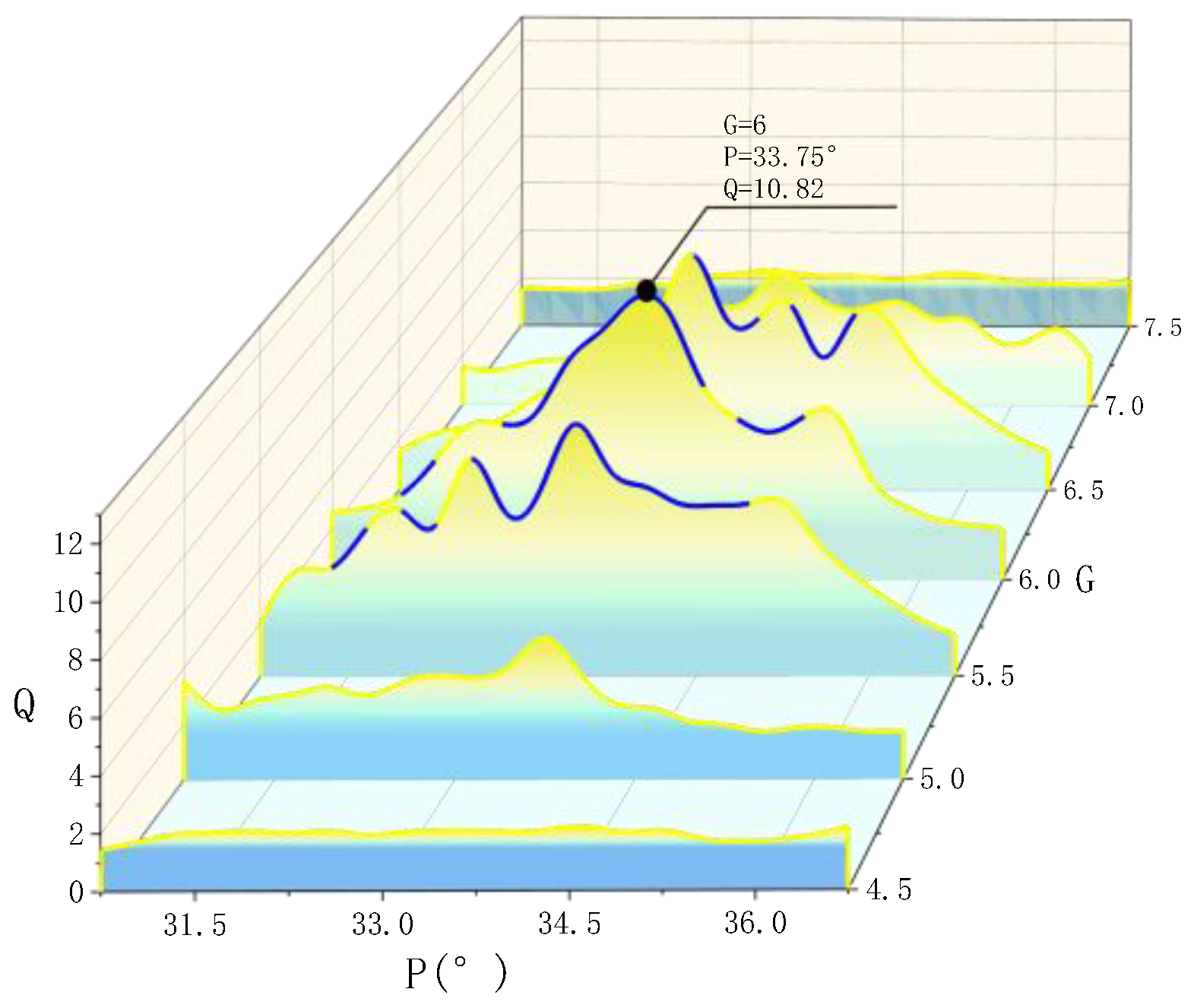
5.4. Effects of P and G
6. Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xavier, N. Fernando, Radio over Fiber for Wireless Communications: From Fundamentals to Advanced Topics; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2014. [Google Scholar]
- Aljumaily, M.; Li, H. A Framework to Optimize the Frame Duration and the Beam Angle for Random Beamforming of mmWave Mobile Networks. In Proceedings of the International Conference of Information and Communication Technology, Baghdad, Iraq, 15–16 April 2019. [Google Scholar]
- Alavi, S.E.; Soltanian, M.R.K.; Amiri, I.S.; Khalily, M.; Supa’at, A.S.M.; Ahmad, H. Towards 5G: A photonic based millimeter wave signal generation for applying in 5G Access Fronthaul. Sci. Rep. 2016, 6, 19891. [Google Scholar] [CrossRef]
- Ranaweera, C.; Wong, E.; Nirmalathas, A.; Jayasundara, C.; Lim, C. 5G C-RAN with optical fronthaul: An analysis from a deployment perspective. J. Light. Technol. 2018, 36, 2059–2068. [Google Scholar] [CrossRef]
- Asha, D.S. A comprehensive review of millimeter wave based radio over fiber for 5G front haul transmissions. Indian J. Sci. Technol. 2020, 14, 86–100. [Google Scholar]
- Dar, A.; Ahmad, F.; Jha, R. Filterless 16 tupled optical millimeter wave generation using cascaded parallel Mach Zehnder modulator with extinction ratio tolerance. Prog. Electromagn. Res. Lett. 2020, 91, 129–135. [Google Scholar] [CrossRef]
- Chen, Y.; Wen, A.; Shang, L. A full-duplex radio-over-fiber link with 12-tupling mm-wave generation and wavelength reuse for upstream signal. Opt. Laser Technol. 2011, 43, 1167–1171. [Google Scholar] [CrossRef]
- Ramos, R.T.; Seeds, A.J. Fast heterodyne optical phase-lock loop using double quantum well laser diodes. Electron. Lett. 1992, 28, 82–83. [Google Scholar] [CrossRef]
- Yao, X.S.; Davis, L.; Maleki, L. Coupled optoelectronic oscillators for generating both RF signal and optical pulses. IEEE/OSA J. Light. Technol. 2000, 18, 73–78. [Google Scholar] [CrossRef]
- Vawter, G.; Mar, A.; Hietala, V.; Zolper, J.; Hohimer, J. All optical millimeter-wave electrical signal generation using an integrated mode-locked semiconductor ring laser and photodiode. IEEE Photonics Technol. Lett. 1997, 9, 1634–1636. [Google Scholar] [CrossRef]
- Zhang, C.; Ning, T.; Li, J.; Pei, L.; Li, C.; Ma, S. A full-duplex WDM-RoF system based on tunable optical frequency comb generator. Opt. Commun. 2015, 344, 65–70. [Google Scholar] [CrossRef]
- Wu, P.; Ma, J. BPSK optical mm-wave signal generation by septupling frequency via a single optical phase modulator. Opt. Commun. 2016, 374, 69–74. [Google Scholar] [CrossRef]
- Zhou, W.; Qin, C. Simultaneous generation of 40, 80 and 120 GHz optical millimeter-wave from one Mach-Zehnder modulator and demonstration of millimeter wave transmission and down-conversion. Opt. Commun. 2017, 398, 101–106. [Google Scholar] [CrossRef]
- Mohamed, M.; Zhang, X.; Hraimel, B.; Wu, K. Frequency sixupler for millimeter-wave over fiber systems. Opt. Express 2008, 16, 10141–10151. [Google Scholar] [CrossRef] [PubMed]
- Baskaran, M.; Madhan, M.G. A novel approach for simultaneous millimeter wave generation and high bit rate data transmission for Radio over Fiber (RoF) systems. Optik 2014, 125, 6347. [Google Scholar] [CrossRef]
- Zheng, H.; Liu, S.; Li, X.; Wang, W.; Tian, Z. Generation and transmission simulation of 60 G millimeter-wave by using semiconductor optical amplifiers for radio-over-fiber systems. Opt. Commun. 2009, 282, 4440. [Google Scholar] [CrossRef]
- O’Reilly, J.J.; Lane, P.M.; Heidemann, R.; Hofstetter, R. Optical generation of very narrow linewidth millimeter wave signals. Electron. Lett. 1992, 28, 2309–2311. [Google Scholar] [CrossRef]
- Shih, P.-T.; Chen, J.; Lin, C.-T.; Jiang, W.-J.; Huang, H.-S.; Peng, P.-C.; Chi, S. Optical millimeter-wave signal generation via frequency 12-tupling. IEEE/OSA J. Light. Technol. 2010, 28, 71–78. [Google Scholar] [CrossRef]
- Li, X.; Xiao, J.; Xu, Y.; Chen, L.; Yu, J. Frequency-doubling photonic vector millimeter-wave signal generation from one DML. IEEE Photon. J. 2015, 7, 5501207. [Google Scholar] [CrossRef]
- Li, X.; Xiao, J.; Yu, J. W-band vector millimeter-wave signal generation based on phase modulator with photonic frequency quadrupling and precoding. IEEE/OSA J. Light. Technol. 2017, 35, 2548–2558. [Google Scholar] [CrossRef]
- Li, X.; Zhang, J.; Xiao, J.; Zhang, Z.; Xu, Y.; Yu, J. W-band 8QAM vector signal generation by MZM-based photonic frequency octupling. IEEE Photonics Technol. Lett. 2015, 27, 1257–1260. [Google Scholar] [CrossRef]
- Wang, D.; Tang, X.; Fan, Y.; Zhang, X.; Xi, L.; Zhang, W. A new approach to generate the optical millimeter-wave signals using frequency 12-tupling without an optical filter. In Proceedings of the International Conference on Information Optics and Photonics, State Key Laboratory of Information Photonics and Optical Communications Beijing University of Posts and Telecommunications, Beijing, China, 8–11 July 2018. [Google Scholar]
- Wun, J.-M.; Liu, H.-Y.; Lai, C.-H.; Chen, Y.-S.; Yang, S.-D.; Pan, C.-L.; Bowers, J.E.; Huang, C.-B.; Shi, J.-W. Photonic high-power 160-GHz signal generation by using ultrafast photodiode and a high-repetition-rate femtosecond optical pulse train generator. IEEE J. Sel. Top. Quantum Electron. 2014, 20, 3803507. [Google Scholar]
- Chen, X.G.; Xia, L.; Huang, D.X. A filterless 24-tupling optical millimeter wave generation and RoF distribution. Optik 2017, 147, 22–26. [Google Scholar] [CrossRef]
- Rani, A.; Kedia, D. Mathematical analysis of 24-tupled mm-wave generation using cascaded MZMs with polarization multiplexing for RoF transmission. Opt. Quantum Electron. 2024, 56, 193. [Google Scholar] [CrossRef]
- Chen, X.; Dai, S.; Li, Z.; Liu, X.; Chen, X.; Xiao, H. Filterless frequency 32-tupling millimeter-wave generation based on two cascaded dual-parallel Mach–Zehnder modulators. Front. Phys. 2023, 11, 1212482. [Google Scholar] [CrossRef]
- Chen, X.; Dai, S.; Li, Z.; Chen, X. Thirty-two-tupling frequency millimeter-wave generation based on eight Mach-Zehnder modulators connected in parallel. ETRI J. 2023, 46, 194–204. [Google Scholar] [CrossRef]
- Wang, S.; Wang, D.; Ren, L.; Zhang, H.; Wu, Z.; Li, W.; Zhang, F.; Wang, X. Photon Generation Scheme of 32-Fold Millimeter-Wave Signal Based on Mach-Zehnder Modulator. Ann. Phys. 2024, 536, 2300360. [Google Scholar] [CrossRef]
- Shang, Y.; Feng, Z.; Cao, C.; Huang, Z.; Wu, Z.; Xu, X.; Geng, J. A using remodulation filterless scheme of generating frequency 32-tupling millimeter-wave based on two DPMZMs. Opt. Laser Technol. 2022, 148, 107793. [Google Scholar] [CrossRef]
- Song, H.J.; Lee, J.S.; Song, J.I. Error-free simultaneous all-optical upconversion of WDM radio-over-fiber signals. IEEE Photonics Technol. Lett. 2005, 17, 1731–1733. [Google Scholar] [CrossRef]
- Yu, J.; Jia, Z.; Yi, L.; Su, Y.; Chang, G.K.; Wang, T. Optical millimeter-wave generation or up-conversion using external modulators. IEEE Photonics Technol. Lett. 2005, 18, 265–267. [Google Scholar]
- Griffin, R.A.; Salgado, H.M.; Lane, P.M.; O’Reilly, J.J. System capacity for millimeter-wave radio-over-fiber distribution employing an optically supported PLL. J. Light. Technol. 1999, 17, 2480. [Google Scholar] [CrossRef]
- Yu, J.; Jia, Z.; Chang, G.K. All-optical mixer based on cross-absorption modulation in electroabsorption modulator. IEEE Photonics Technol. Lett. 2005, 17, 2421–2423. [Google Scholar]
- Corral, J.L.; Marti, J.; Fuster, J.M. General expressions for IM/DD dispersive analog optical links with external modulation or optical up-conversion in a Mach-Zehnder electrooptical modulator. IEEE Trans. Microw. Theory Tech. 2001, 49, 1968–1976. [Google Scholar] [CrossRef]
- Ma, J.X.; Yu, C.X.; Zhou, Z.; Yu, J.J. Optical mm-wave generation by using external modulator based on optical carrier suppression. Opt. Commun. 2006, 268, 51–57. [Google Scholar] [CrossRef]
- Ma, J.X.; Yu, J.J.; Yu, C.; Xin, X.; Zeng, J.; Chen, L. Fiber dispersion influence on transmission of the optical millimeter-waves generated using LN-MZM intensity modulation. J. Light. Technol. 2007, 25, 3244–3256. [Google Scholar] [CrossRef]
- Ma, J.X.; Yu, J.J.; Yu, C.; Jia, Z.S.; Chang, G.K.; Sang, X.Z. The influence of fiber dispersion on the code form of the optical mm-wave signal generated by single sideband intensity-modulation. Opt. Commun. 2007, 271, 396–403. [Google Scholar] [CrossRef]
- Zhou, M.; Ma, J.X. The influence of fiber dispersion on the transmission performance of a quadruple-frequency optical millimeter wave with two signal modulation formats. Opt. Switch. Netw. 2012, 9, 343–350. [Google Scholar] [CrossRef]
- Chen, L.X.; Huang, C.; Chen, L. A modified scheme for optical millimeter-wave generation based on optical carrier suppression modulation. Laser Technol. 2008, 32, 659–662. [Google Scholar]
- Xie, J.L.; Huang, X.G.; Tao, J. A full-duplex radio-over-fiber system based on a novel double-sideband modulation and frequency quadrupling. Opt. Commun. 2010, 283, 874–878. [Google Scholar] [CrossRef]
- Liu, X.L.; Liu, Z.J.; Li, J.D.; Shang, T.; Zhao, J. Generation of optical carrier suppression millimeter-wave signal using one dual-parallel MZM to overcome chromatic dispersion. Opt. Commun. 2010, 283, 3129–3135. [Google Scholar] [CrossRef]
- Guo, D.F.; Zhang, Z.J.; Fang, X.K.; Shang, Y. Schemes to eliminate the time shift of code edges based on the optimal transmission point of DP-MZM. Appl. Opt. 2023, 62, 5652–5659. [Google Scholar] [CrossRef]
- Zhu, Z.H.; Zhao, S.H.; Yao, Z.S.; Tan, Q.G.; Li, Y.J.; Chu, X.C.; Shi, L.; Hou, R. A novel OCS millimeter-wave generation scheme with data carried only by one sideband and wavelength reuse for uplink connection. Opt. Laser Technol. 2012, 44, 2366–2370. [Google Scholar] [CrossRef]
- Zhu, Z.H.; Zhao, S.H.; Yao, Z.S.; Tan, Q.G.; Li, Y.J.; Chu, X.C.; Shi, L.; Zhang, X. Optical millimeter-wave signal generation by frequency quadrupling using one dual-drive Mach-Zehnder modulator to overcome chromatic dispersion. Opt. Commun. 2012, 285, 3021–3026. [Google Scholar] [CrossRef]
- Liang, D.; Jiang, W.; Tan, Q.G.; Zhu, Z.B.; Liu, F. A novel optical millimeter-wave signal generation approach to overcome chromatic dispersion. Opt. Commun. 2014, 320, 94–98. [Google Scholar] [CrossRef]
- Zhu, Z.; Zhao, S.; Li, Y.; Chu, X.; Wang, X.; Zhao, G. A radio-over-fiber system with frequency 12-tupling optical millimeter-wave generation to overcome chromatic dispersion. IEEE J. Quantum Electron. 2013, 49, 919–922. [Google Scholar] [CrossRef]







| Device | Parameter | Value |
|---|---|---|
| CW | frequency | 193.1 THz (default) |
| linewidth | 10 MHz (default) | |
| data | speed | 2.5 Gbit/s (default) |
| RF-LO | frequency | 10 GHz (default) |
| amplitude | 7.0284 V (computed) | |
| phase modulator | P | (computed) |
| electrical gainer | G | 6 (computed) |
| MZM | 4 V (default) |
| Conventional Systems | Our System | Improvement | |
|---|---|---|---|
| BTB | −23.63 dBm | −23.953 dBm | 0.323 dBm |
| 10 km | −23.05 dBm | −23.467 dBm | 0.417 dBm |
| 20 km | −22.31 dBm | −22.793 dBm | 0.483 dBm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Chen, X.; Dai, S.; Li, B.; Wang, L. A Novel Inserting Pilot Radio over Fiber System without the Bit Walk-Off Effect for the Generation and Distribution of Frequency 16-Tupling Millimeter Waves by Mach–Zehnder Modulators. Photonics 2024, 11, 410. https://doi.org/10.3390/photonics11050410
Chen X, Chen X, Dai S, Li B, Wang L. A Novel Inserting Pilot Radio over Fiber System without the Bit Walk-Off Effect for the Generation and Distribution of Frequency 16-Tupling Millimeter Waves by Mach–Zehnder Modulators. Photonics. 2024; 11(5):410. https://doi.org/10.3390/photonics11050410
Chicago/Turabian StyleChen, Xu, Xinqiao Chen, Siyuan Dai, Bin Li, and Ling Wang. 2024. "A Novel Inserting Pilot Radio over Fiber System without the Bit Walk-Off Effect for the Generation and Distribution of Frequency 16-Tupling Millimeter Waves by Mach–Zehnder Modulators" Photonics 11, no. 5: 410. https://doi.org/10.3390/photonics11050410
APA StyleChen, X., Chen, X., Dai, S., Li, B., & Wang, L. (2024). A Novel Inserting Pilot Radio over Fiber System without the Bit Walk-Off Effect for the Generation and Distribution of Frequency 16-Tupling Millimeter Waves by Mach–Zehnder Modulators. Photonics, 11(5), 410. https://doi.org/10.3390/photonics11050410





