Spatially Resolved, Real-Time Polarization Measurement Using Artificial Birefringent Metallic Elements
Abstract
1. Introduction
2. Design
3. Materials and Methods
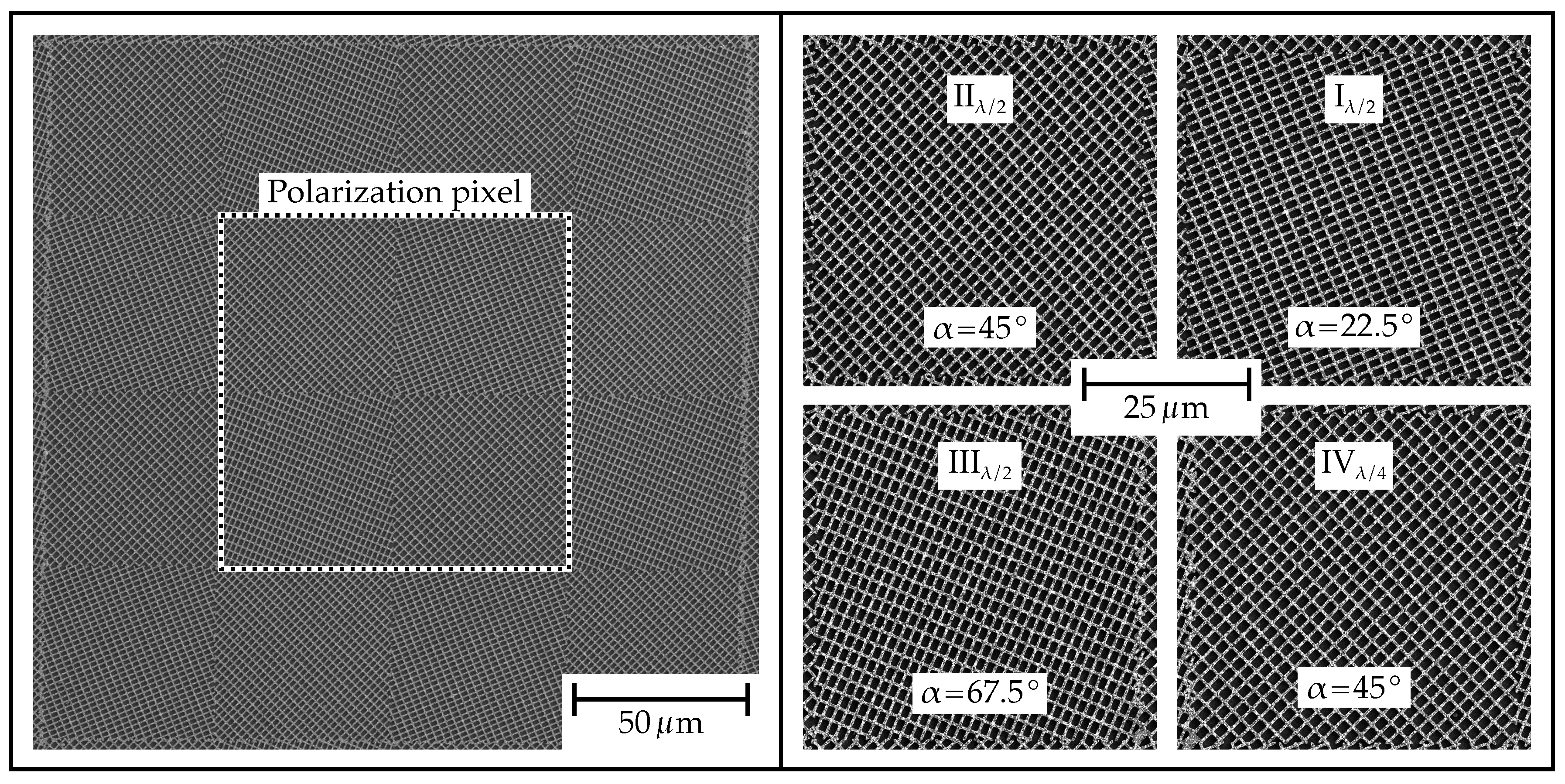
3.1. Fabrication of the Hollow Waveguide Array
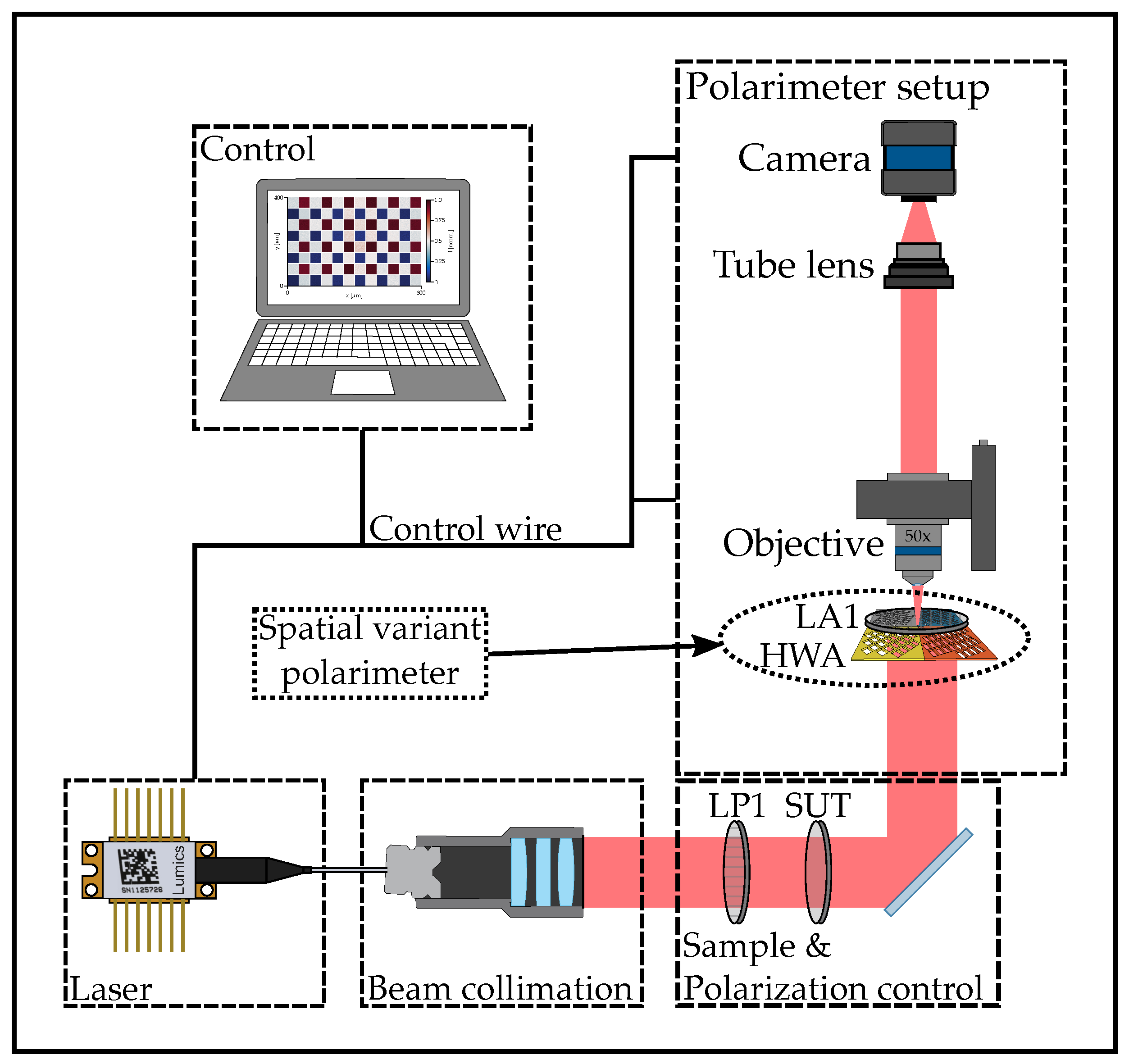
3.2. Experimental Setup
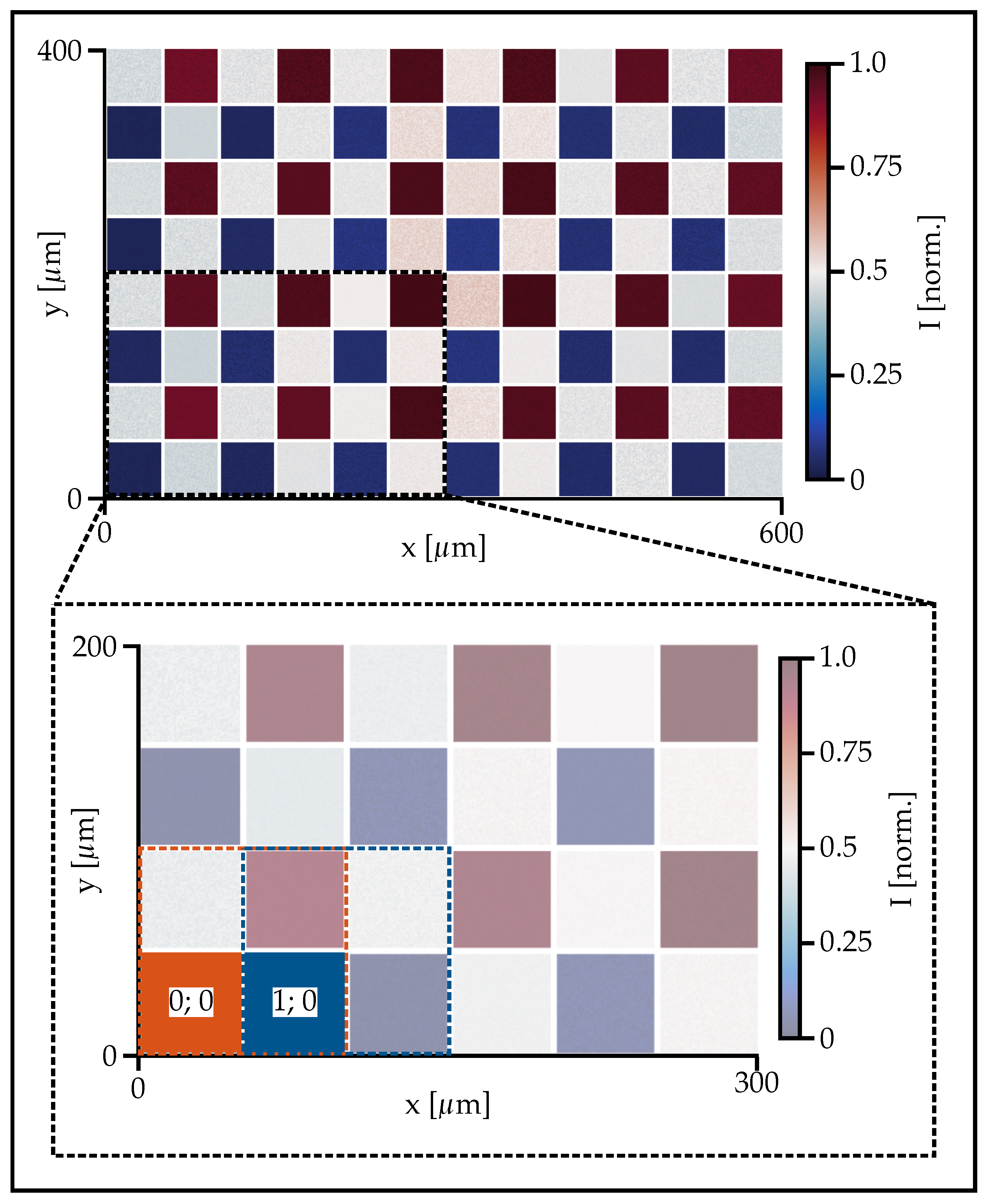
3.3. Evaluation Algorithm
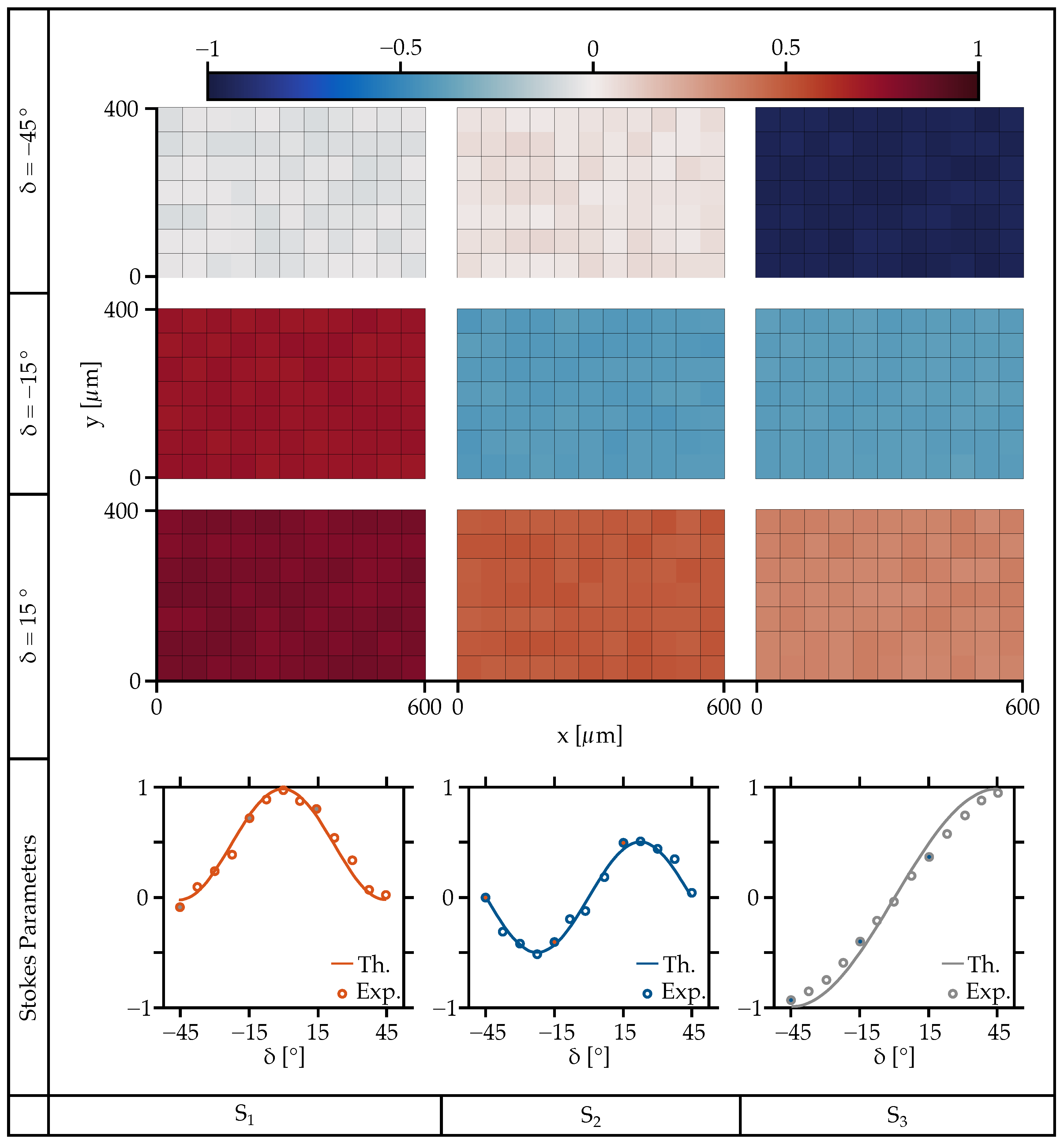
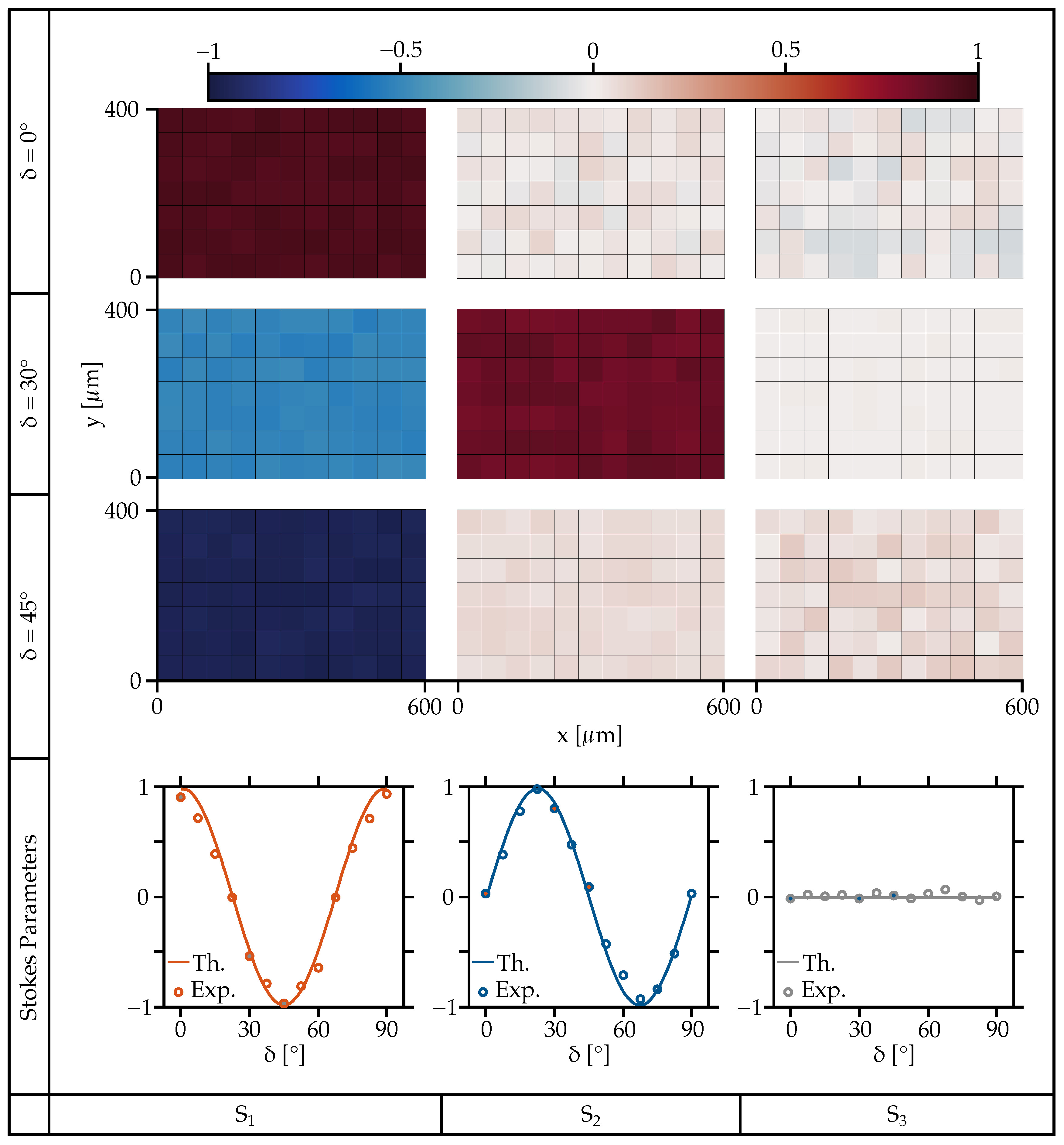
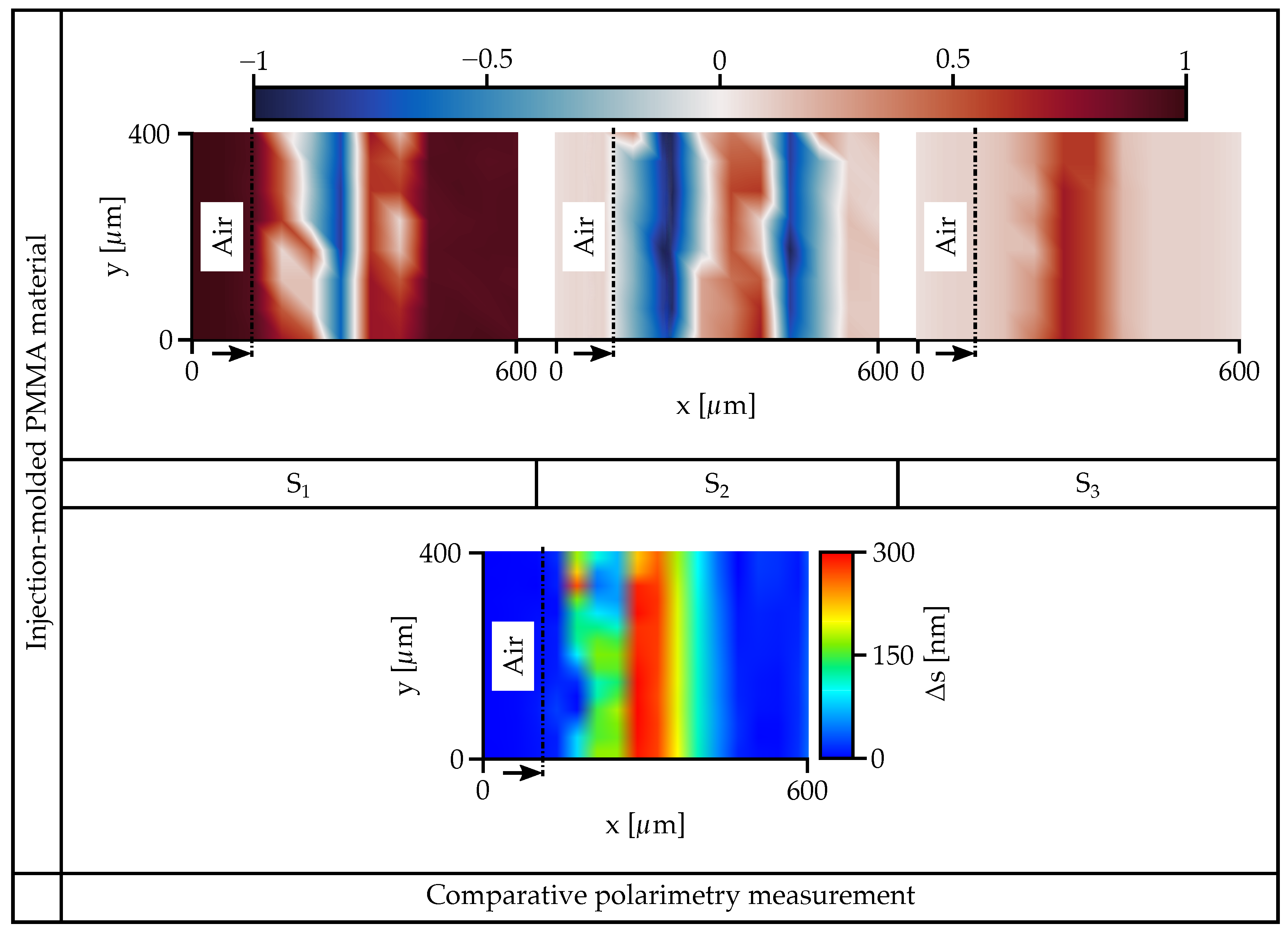
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Huard, S. Polarization of Light; John Wiley and Sons Masson: Chichester, UK; New York, NY, USA; Paris, France, 1997. [Google Scholar]
- Goldstein, D.H. Polarized Light; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar] [CrossRef]
- Meier, M.; Romano, V.; Feurer, T. Material processing with pulsed radially and azimuthally polarized laser radiation. Appl. Phys. A 2007, 86, 329–334. [Google Scholar] [CrossRef]
- Patel, A.; Tikhonchuk, V.T.; Zhang, J.; Kazansky, P.G. Non-paraxial polarization spatio-temporal coupling in ultrafast laser material processing. Laser Photonics Rev. 2017, 11, 1600290. [Google Scholar] [CrossRef]
- Srivastava, A.K.; De Bougrenet De La Tocnaye, J.L.; Dupont, L. Liquid crystal active glasses for 3D cinema. J. Disp. Technol. 2010, 6, 522–530. [Google Scholar] [CrossRef]
- Richtberg, S.; Girwidz, R. Use of linear and circular polarization: The secret LCD screen and 3D cinema. Phys. Teach. 2017, 55, 406–408. [Google Scholar] [CrossRef]
- Oldenbourg, R. A new view on polarization microscopy. Nature 1996, 381, 811–812. [Google Scholar] [CrossRef] [PubMed]
- Ding, C.; Li, C.; Deng, F.; Simpson, G.J. Axially-offset differential interference contrast microscopy via polarization wavefront shaping. Opt. Express 2019, 27, 3837. [Google Scholar] [CrossRef]
- Morizet, J.; Ducourthial, G.; Supatto, W.; Boutillon, A.; Legouis, R.; Schanne-Klein, M.C.; Stringari, C.; Beaurepaire, E. High-speed polarization-resolved third-harmonic microscopy. Optica 2019, 6, 385. [Google Scholar] [CrossRef]
- Juhl, M.; Mendoza, C.; Mueller, J.P.B.; Capasso, F.; Leosson, K. Performance characteristics of 4-port in-plane and out-of-plane in-line metasurface polarimeters. Opt. Express 2017, 25, 28697. [Google Scholar] [CrossRef]
- Gu, N.; Yao, B.; Huang, L.; Rao, C. Design and analysis of a novel compact and simultaneous polarimeter for complete Stokes polarization imaging with a piece of encoded birefringent crystal and a micropolarizer array. IEEE Photonics J. 2018, 10, 1–12. [Google Scholar] [CrossRef]
- Sabatke, D.S.; Descour, M.R.; Dereniak, E.L.; Sweatt, W.C.; Kemme, S.A.; Phipps, G.S. Optimization of retardance for a complete Stokes polarimeter. Opt. Lett. 2000, 25, 802. [Google Scholar] [CrossRef]
- Guan, W.; Cook, P.J.; Jones, G.A.; Shen, T.H. Experimental determination of the Stokes parameters using a dual photoelastic modulator system. Appl. Opt. 2010, 49, 2644–2652. [Google Scholar] [CrossRef]
- Bueno, J.M. Polarimetry using liquid-crystal variable retarders: Theory and calibration. J. Opt. A Pure Appl. Opt. 2000, 2, 216–222. [Google Scholar] [CrossRef]
- Kaneshiro, J.; Watanabe, T.M.; Fujita, H.; Ichimura, T. Full control of polarization state with a pair of electro-optic modulators for polarization-resolved optical microscopy. Appl. Opt. 2016, 55, 1082. [Google Scholar] [CrossRef]
- Balthasar Mueller, J.P.; Leosson, K.; Capasso, F. Ultracompact metasurface in-line polarimeter. Optica 2016, 3, 42. [Google Scholar] [CrossRef]
- Peinado, A.; Lizana, A.; Campos, J. Design of polarimeters based on liquid crystals and biaxial crystals for polarization metrology. Opt. Pura Apl. 2016, 49, 167–177. [Google Scholar] [CrossRef]
- Gruev, V.; Perkins, R.; York, T. CCD polarization imaging sensor with aluminum nanowire optical filters. Opt. Express 2010, 18, 19087. [Google Scholar] [CrossRef]
- Hsu, W.L.; Myhre, G.; Balakrishnan, K.; Brock, N.; Ibn-Elhaj, M.; Pau, S. Full-Stokes imaging polarimeter using an array of elliptical polarizer. Opt. Express 2014, 22, 3063. [Google Scholar] [CrossRef]
- Arbabi, E.; Kamali, S.M.; Arbabi, A.; Faraon, A. Full-Stokes imaging polarimetry using dielectric metasurfaces. ACS Photonics 2018, 5, 3132–3140. [Google Scholar] [CrossRef]
- Intaravanne, Y.; Chen, X. Recent advances in optical metasurfaces for polarization detection and engineered polarization profiles. Nanophotonics 2020, 9, 1003–1014. [Google Scholar] [CrossRef]
- Huang, Z.; Zheng, Y.; Li, J.; Cheng, Y.; Wang, J.; Zhou, Z.K.; Chen, L. High-resolution metalens imaging polarimetry. Nano Lett. 2023, 23, 10991–10997. [Google Scholar] [CrossRef]
- Helfert, S.F.; Edelmann, A.; Jahns, J. Hollow waveguides as polarization converting elements: A theoretical study. J. Eur. Opt. Soc. Rapid Publ. 2015, 10, 15006. [Google Scholar] [CrossRef]
- Edelmann, A.; Moeller, L.; Jahns, J. Coupling of terahertz radiation to metallic wire using end-fire technique. Electron. Lett. 2013, 49, 884–886. [Google Scholar] [CrossRef]
- Helfert, S.F.; Seiler, T.; Jahns, J.; Becker, J.; Jakobs, P.; Bacher, A. Numerical simulation of hollow waveguide arrays as polarization converting elements and experimental verification. Opt. Quantum Electron. 2017, 49, 313. [Google Scholar] [CrossRef]
- Belle, S.; Helfert, S.F.; Hellmann, R.; Jahns, J. Hollow waveguide array with subwavelength dimensions as a space-variant polarization converter. J. Opt. Soc. Am. B 2019, 36, D119–D125. [Google Scholar] [CrossRef]
- Hessler, S.; Rüth, M.; Sauvant, C.; Lemke, H.D.; Schmauss, B.; Hellmann, R. Hemocompatibility of EpoCore/EpoClad photoresists on COC substrate for optofluidic integrated Bragg sensors. Sensors Actuators B Chem. 2017, 239, 916–922. [Google Scholar] [CrossRef]
- Chaturvedi, M.; Bhandare, R.; Kumar, S.; Verma, Y.; Raja, S. A compact full Stokes polarimeter. Opt.-Int. J. Light Electron Opt. 2022, 267, 169645. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, H.; Zhao, C.; Luo, P. Error analysis and optimization for a full-Stokes division-of-space polarimeter. Appl. Opt. 2023, 62, 6816–6825. [Google Scholar] [CrossRef]
- Hemmati, H.; Bootpakdeetam, P.; Lee, K.J.; Magnusson, R. Rapid large-scale fabrication of multipart unit cell metasurfaces. Opt. Express 2020, 28, 19304–19314. [Google Scholar] [CrossRef] [PubMed]
- Leng, B.; Zhang, Y.; Tsai, D.P.; Xiao, S. Meta-device: Advanced manufacturing. Light. Adv. Manuf. 2024, 5, 1–16. [Google Scholar] [CrossRef]
- Chen, W.T.; Capasso, F. Will flat optics appear in everyday life anytime soon? Appl. Phys. Lett. 2021, 118, 100503. [Google Scholar] [CrossRef]
- Zeitner, U.D.; Banasch, M.; Trost, M. Potential of e-beam lithography for micro- and nano-optics fabrication on large areas. J. Micro/Nanopatterning Mater. Metrol. 2023, 22, 041405. [Google Scholar] [CrossRef]
- Belle, S.; Goetzendorfer, B.; Hellmann, R. Challenges in a hybrid fabrication process to generate metallic polarization elements with sub-wavelength dimensions. Materials 2020, 13, 5279. [Google Scholar] [CrossRef] [PubMed]
- Isayev, A.I.; Hieber, C.A. Toward a viscoelastic modelling of the injection molding of polymers. Rheol. Acta 1980, 19, 168–182. [Google Scholar] [CrossRef]
- Rosenberger, M.; Kefer, S.; Girschikofsky, M.; Roth, G.L.; Belle, S.; Schmauss, B.; Hellmann, R. High-temperature stable and sterilizable waveguide Bragg grating in planar cyclo-olefin copolymer. Opt. Lett. 2018, 43, 3321–3324. [Google Scholar] [CrossRef] [PubMed]
- Roth, G.L.; Hessler, S.; Kefer, S.; Girschikofsky, M.; Esen, C.; Hellmann, R. Femtosecond laser inscription of waveguides and Bragg gratings in transparent cyclo-olefin copolymers. Opt. Express 2020, 28, 18077–18084. [Google Scholar] [CrossRef] [PubMed]
- Kefer, S.; Limbach, T.; Pape, N.; Klamt, K.; Schmauss, B.; Hellmann, R. Birefringence in injection-molded cyclic olefin copolymer substrates and its impact on integrated photonic structures. Polymers 2024, 16, 168. [Google Scholar] [CrossRef] [PubMed]
- Park, K.; Kim, B.; Yao, D. Numerical simulation for injection molding with a rapidly heated mold, part II: Birefringence prediciton. Polym.-Plast. Technol. Eng. 2006, 45, 903–909. [Google Scholar] [CrossRef]
- Min, I.; Yoon, K. An experimental study on the effects of injection-molding types for the birefringence distribution in polycarbonate discs. Korea-Aust. Rheol. J. 2011, 23, 155–162. [Google Scholar] [CrossRef]

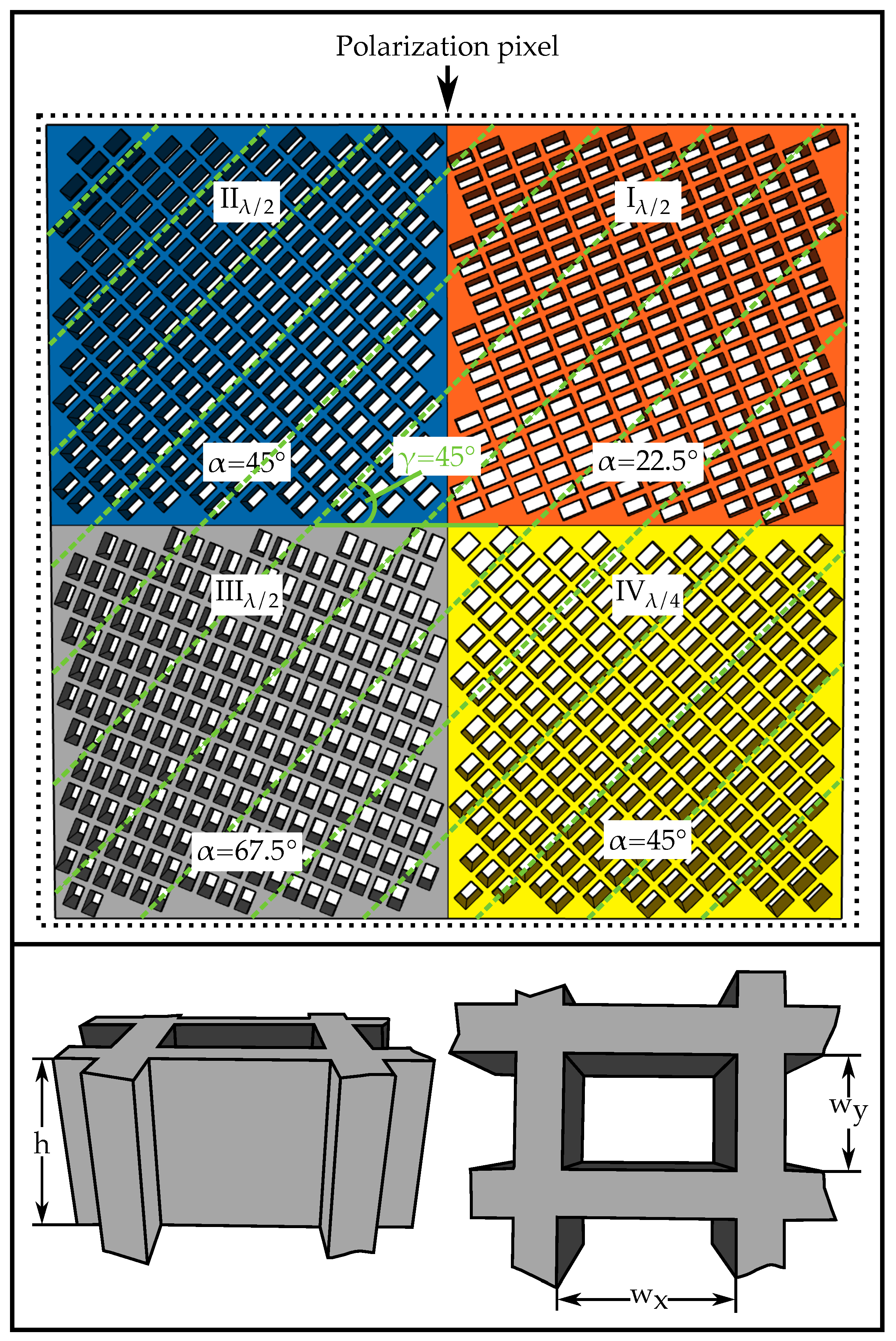
| I | 22.5° | 45° | 1550 nm | 872 nm | 45° | |
| II | 45° | 45° | 1550 nm | 872 nm | 90° | |
| III | 67.5° | 45° | 1550 nm | 872 nm | 135° | |
| IV | 45° | 45° | 1550 nm | 1034 nm | 45° |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Belle, S.; Kefer, S.; Hellmann, R. Spatially Resolved, Real-Time Polarization Measurement Using Artificial Birefringent Metallic Elements. Photonics 2024, 11, 397. https://doi.org/10.3390/photonics11050397
Belle S, Kefer S, Hellmann R. Spatially Resolved, Real-Time Polarization Measurement Using Artificial Birefringent Metallic Elements. Photonics. 2024; 11(5):397. https://doi.org/10.3390/photonics11050397
Chicago/Turabian StyleBelle, Stefan, Stefan Kefer, and Ralf Hellmann. 2024. "Spatially Resolved, Real-Time Polarization Measurement Using Artificial Birefringent Metallic Elements" Photonics 11, no. 5: 397. https://doi.org/10.3390/photonics11050397
APA StyleBelle, S., Kefer, S., & Hellmann, R. (2024). Spatially Resolved, Real-Time Polarization Measurement Using Artificial Birefringent Metallic Elements. Photonics, 11(5), 397. https://doi.org/10.3390/photonics11050397






