Flexible Modulation of Perfect Vortex Beams by Combining Coherent Beams
Abstract
1. Introduction
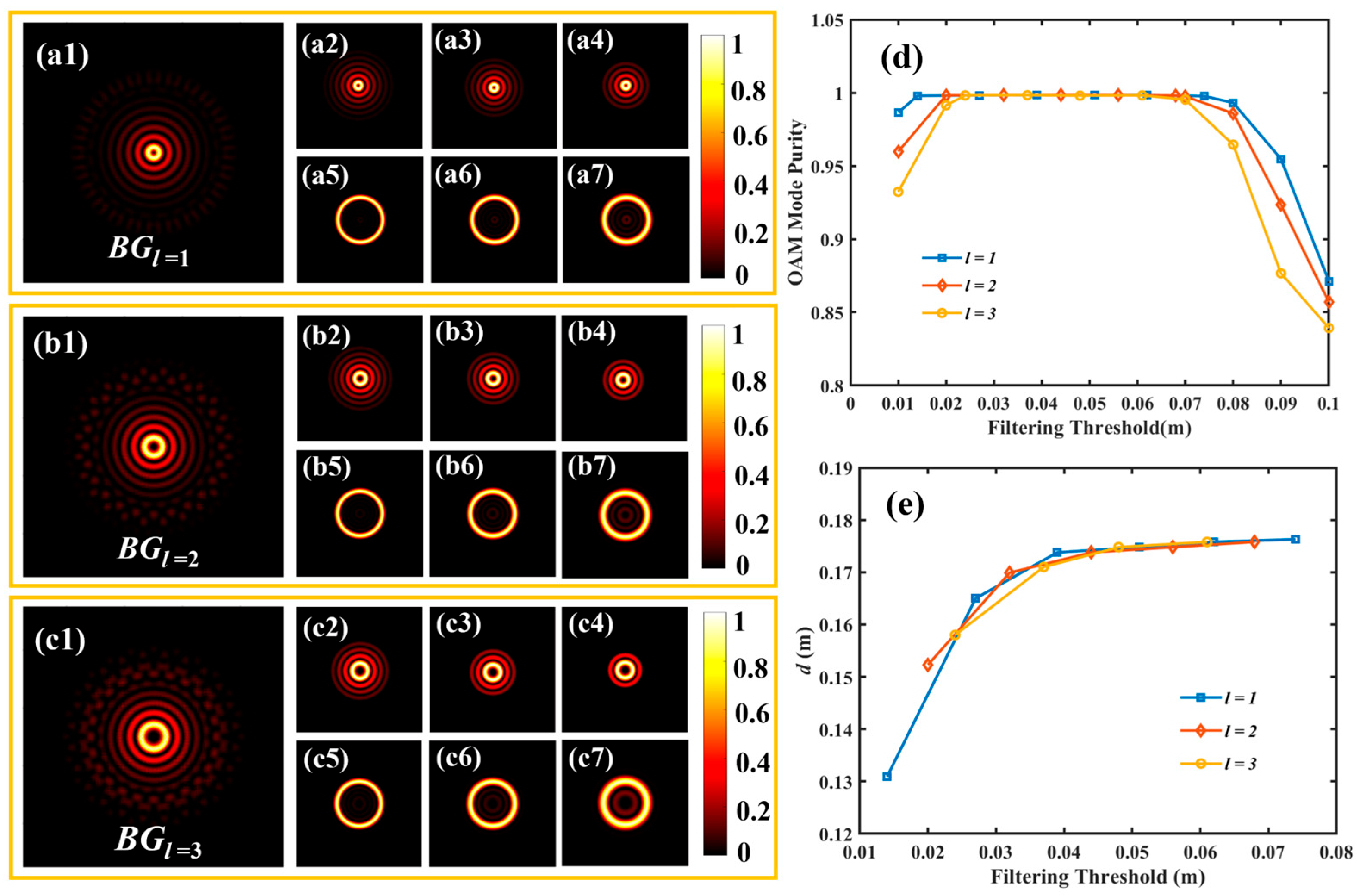
2. Simulation Analysis Methods
3. Results and Discussion
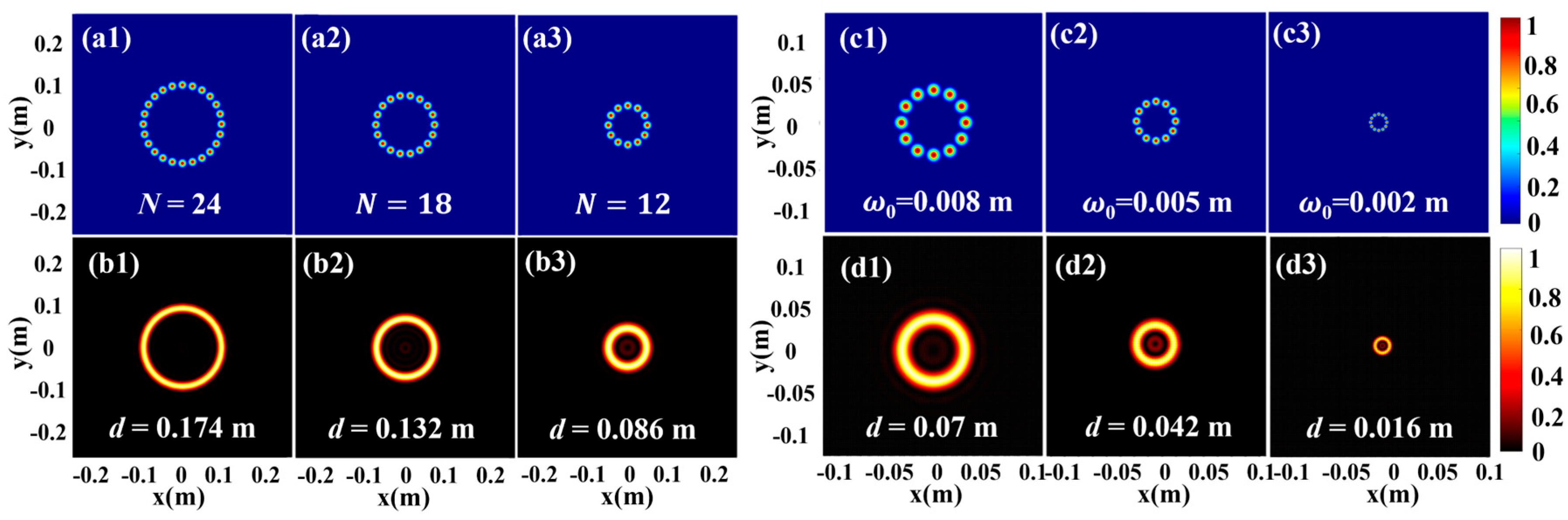
3.1. Generation of PVBs by Employing a Piston Phase in a CBC System
3.2. Generation and Modulation of FPVBs via a Piston Phase
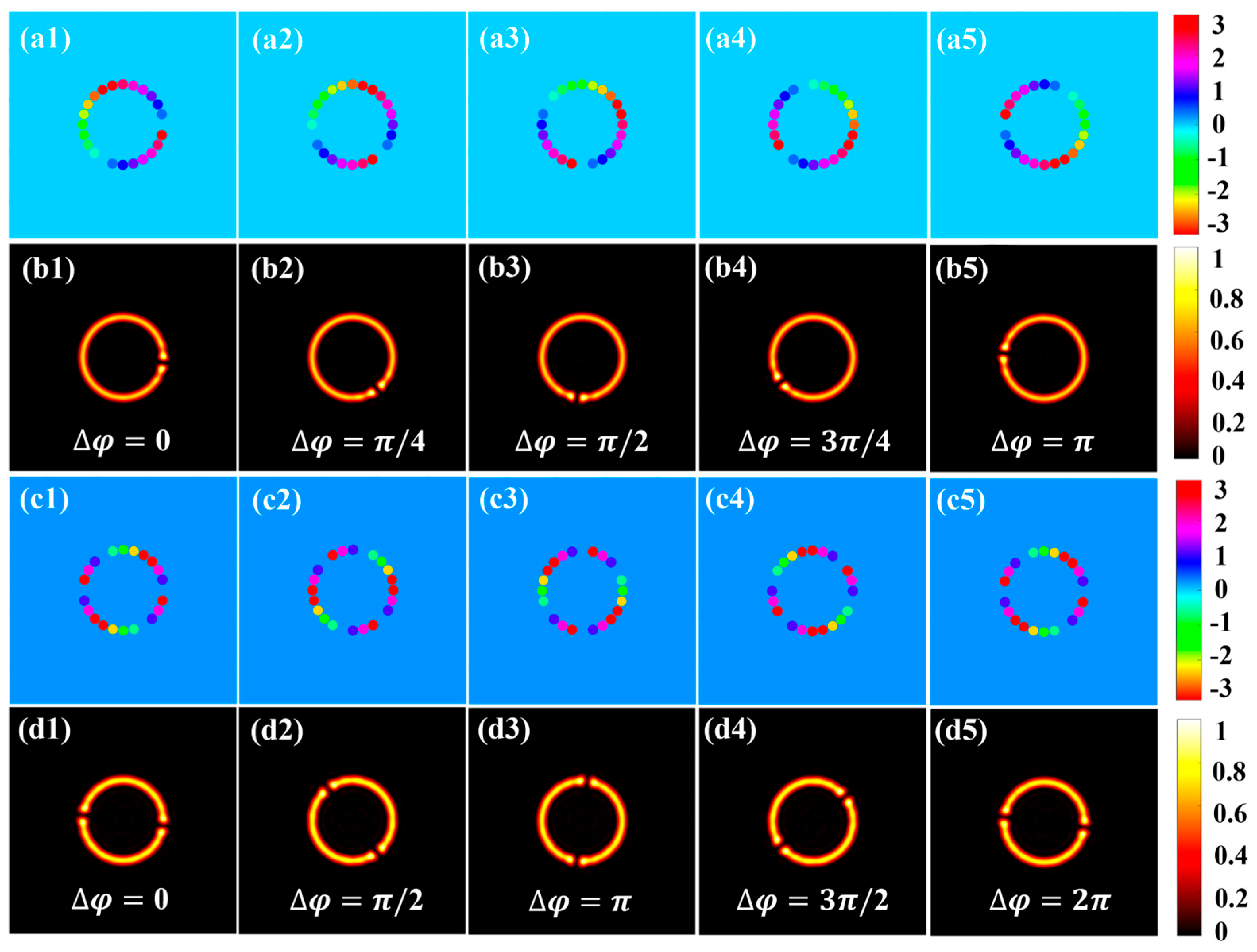
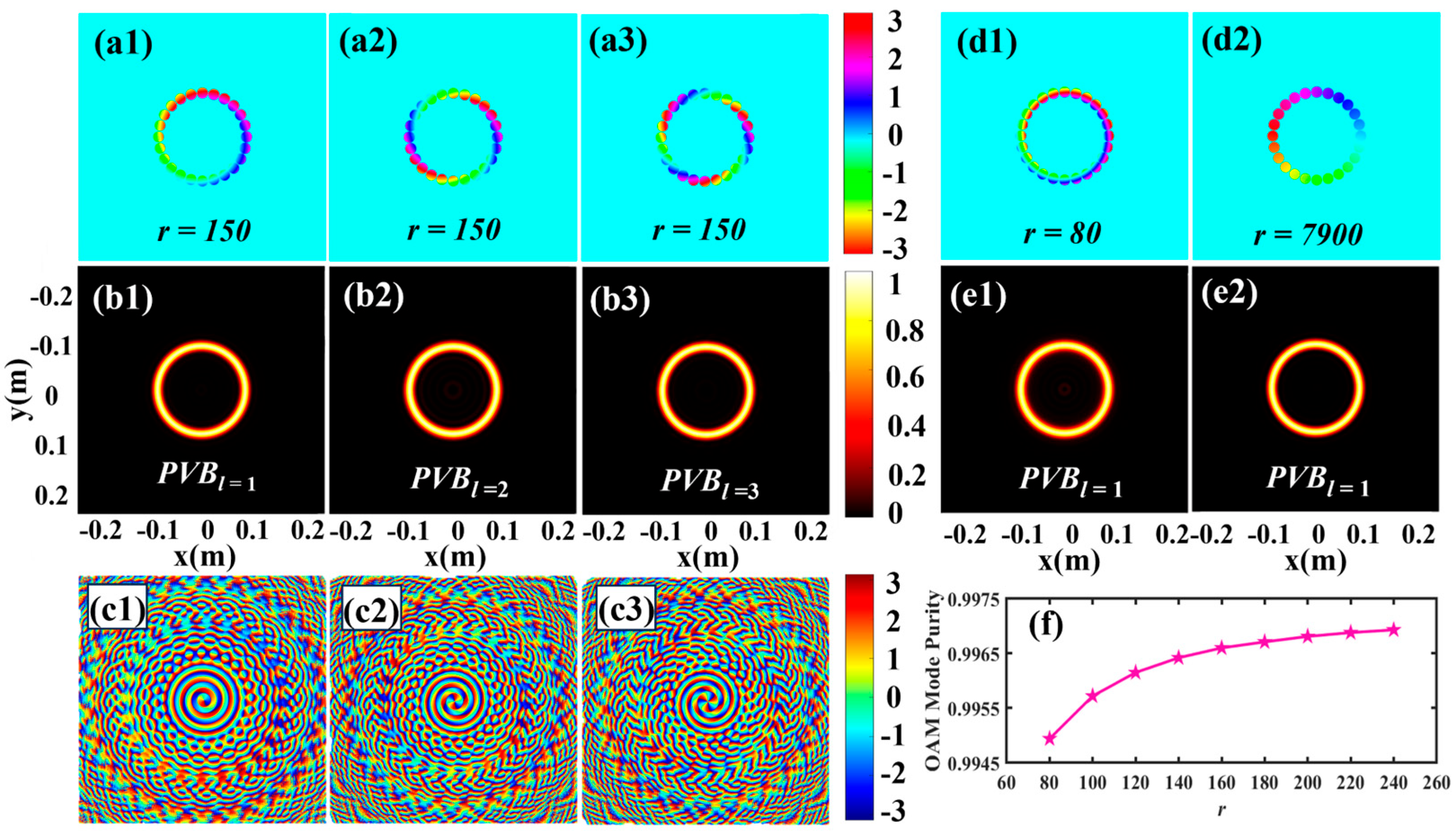
3.3. Generation of PVBs by Employing a Tilting Phase in a CBC System
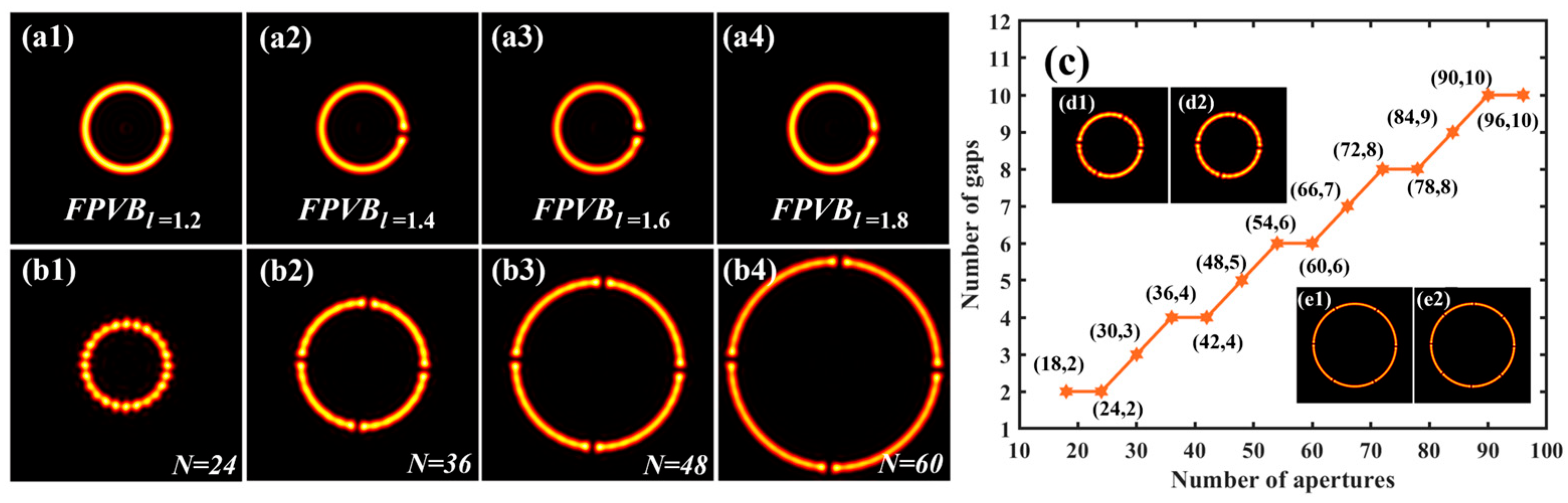
3.4. Generation and Modulation of FPVBs via a Tilting Phase
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ostrovsky, A.S.; Rickenstorff-Parrao, C.; Arrizón, V. Generation of the “perfect” optical vortex using a liquid-crystal spatial light modulator. Opt. Lett. 2013, 38, 534–536. [Google Scholar] [CrossRef] [PubMed]
- García-García, J.; Rickenstorff-Parrao, C.; Ramos-García, R.; Arrizón, V.; Ostrovsky, A.S. Simple technique for generating the perfect optical vortex. Opt. Lett. 2014, 39, 5305–5308. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Ma, H.; Yin, C.; Tang, J.; Li, H.; Tang, M.; Wang, J.; Tai, Y.; Li, X.; Wang, Y. Controllable mode transformation in perfect optical vortices. Opt. Express 2018, 26, 651–662. [Google Scholar] [CrossRef]
- Zhu, F.; Huang, S.; Shao, W.; Zhang, J.; Chen, M.; Zhang, W.; Zeng, J. Free-space optical communication link using perfect vortex beams carrying orbital angular momentum (OAM). Opt. Commun. 2017, 396, 50–57. [Google Scholar] [CrossRef]
- Villalba, N.; Melo, C.; Ayala, S.; Mancilla, C.; Valenzuela, W.; Figueroa, M.; Baradit, E.; Lin, R.; Tang, M.; Walborn, S.P.; et al. Transmission of optical communication signals through ring core fiber using perfect vortex beams. Opt. Express 2023, 31, 40113–40123. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Xu, J.; Yang, Y.; Cheng, M. Optimization of wireless optical communication using perfect vortex beam. Opt. Commun. 2024, 556, 130258. [Google Scholar] [CrossRef]
- Wang, G.; Weng, X.; Kang, X.; Li, Z.; Chen, K.; Gao, X.; Zhuang, S. Free-space creation of a perfect vortex beam with fractional topological charge. Opt. Express 2023, 31, 5757–5766. [Google Scholar] [CrossRef] [PubMed]
- Chen, M.; Mazilu, M.; Arita, Y.; Wright, E.M.; Dholakia, K. Dynamics of microparticles trapped in a perfect vortex beam. Opt. Lett. 2013, 38, 4919–4922. [Google Scholar] [CrossRef] [PubMed]
- Diouf, M.; Harling, M.; Yessenov, M.; Hall, L.A.; Abouraddy, A.F.; Toussaint, K.C. Space-time vector light sheets. Opt. Express 2021, 29, 37225–37233. [Google Scholar] [CrossRef] [PubMed]
- Diouf, M.; Lin, Z.; Harling, M.; Toussaint, K.C. Demonstration of speckle resistance using space–time light sheets. Sci. Rep. 2022, 12, 14064. [Google Scholar] [CrossRef]
- Vaity, P.; Rusch, L. Perfect vortex beam: Fourier transformation of a Bessel beam. Opt. Lett. 2015, 40, 597–600. [Google Scholar] [CrossRef] [PubMed]
- Kotlyar, V.V.; Kovalev, A.A.; Porfirev, A.P. Optimal phase element for generating a perfect optical vortex. J. Opt. Soc. Am. A 2016, 33, 2376–2384. [Google Scholar] [CrossRef] [PubMed]
- Arrizón, V.; Ruiz, U.; Sánchez-de-la-Llave, D.; Mellado-Villaseñor, G.; Ostrovsky, A.S. Optimum generation of annular vortices using phase diffractive optical elements. Opt. Lett. 2015, 40, 1173–1176. [Google Scholar] [CrossRef] [PubMed]
- Tkachenko, G.; Chen, M.; Dholakia, K.; Mazilu, M. Is it possible to create a perfect fractional vortex beam? Optica 2017, 4, 330–333. [Google Scholar] [CrossRef]
- Li, L.; Chang, C.; Yuan, X.; Yuan, C.; Feng, S.; Nie, S.; Ding, J. Generation of optical vortex array along arbitrary curvilinear arrangement. Opt. Express 2018, 26, 9798–9812. [Google Scholar] [CrossRef] [PubMed]
- Long, J.; Chen, X.; Chang, Q.; Hou, T.; Deng, Y.; Zhang, J.; Su, R.; Ma, Y.; Ma, P.; Zhou, P. Controllable customization of optical vortex lattices with coherent laser array. Opt. Laser Technol. 2023, 160, 109045. [Google Scholar] [CrossRef]
- Kurti, R.; Halterman, K.; Shori, R.; Wardlaw, M. Discrete cylindrical vector beam generation from an array of optical fibers. Opt. Express 2009, 17, 13982–13988. [Google Scholar] [CrossRef] [PubMed]
- Adamov, E.V.; Aksenov, V.P.; Atuchin, V.V.; Dudorov, V.V.; Kolosov, V.V.; Levitsky, M.E. Laser beam shaping based on amplitude-phase control of a fiber laser array. OSA Contin. 2021, 4, 182–192. [Google Scholar] [CrossRef]
- Long, J.; Jin, K.; Chen, Q.; Chang, H.; Chang, Q.; Ma, Y.; Wu, J.; Su, R.; Ma, P.; Zhou, P. Generating the 1.5 kW mode-tunable fractional vortex beam by a coherent beam combining system. Opt. Lett. 2023, 48, 5021–5024. [Google Scholar] [CrossRef] [PubMed]
- Veinhard, M.; Bellanger, S.; Daniault, L.; Fsaifes, I.; Bourderionnet, J.; Larat, C.; Lallier, E.; Brignon, A.; Chanteloup, J.-C. Orbital angular momentum beams generation from 61 channels coherent beam combining femtosecond digital laser. Opt. Lett. 2021, 46, 25–28. [Google Scholar] [CrossRef] [PubMed]
- Adamov, E.V.; Aksenov, V.P.; Dudorov, V.V.; Kolosov, V.V.; Levitskii, M.E. Controlling the spatial structure of vector beams synthesized by a fiber laser array. Opt. Laser Technol. 2022, 154, 108351. [Google Scholar] [CrossRef]
- Lin, D.; Carpenter, J.; Feng, Y.; Jain, S.; Jung, Y.; Feng, Y.; Zervas, M.N.; Richardson, D.J. Reconfigurable structured light generation in a multicore fibre amplifier. Nat. Commun. 2020, 11, 3986. [Google Scholar] [CrossRef] [PubMed]
- Chu, X.; Liu, Z.; Zhou, P. Generation of a high-power Airy beam by coherent combining technology. Laser Phys. Lett. 2013, 10, 125102. [Google Scholar] [CrossRef]
- Chu, X. Analytical study on the self-healing property of Bessel beam. Eur. Phys. J. D 2012, 66, 259. [Google Scholar] [CrossRef]
- Yu, T.; Xia, H.; Xie, W.; Xiao, G.; Li, H. The generation and verification of Bessel-Gaussian beam based on coherent beam combining. Results Phys. 2020, 16, 102872. [Google Scholar] [CrossRef]
- Chu, X.; Sun, Q.; Wang, J.; Lü, P.; Xie, W.; Xu, X. Generating a Bessel-Gaussian beam for the application in optical engineering. Sci. Rep. 2015, 5, 18665. [Google Scholar] [CrossRef] [PubMed]
- Ju, P.; Fan, W.; Gao, W.; Li, Z.; Gao, Q.; Jiang, X.; Zhang, T. Phase control scheme of the coherent beam combining system for generating perfect vectorial vortex beams assisted by a Dammann vortex grating. Opt. Express 2023, 31, 22372–22384. [Google Scholar] [CrossRef] [PubMed]
- Ju, P.; Fan, W.; Gao, W.; Li, Z.; Gao, Q.; Li, G.; Jiang, X.; Zhang, T. Generation of perfect vectorial vortex beams by employing coherent beam combining. Opt. Express 2023, 31, 11885–11898. [Google Scholar] [CrossRef]
- Woerdemann, M.; Alpmann, C.; Esseling, M.; Denz, C. Advanced optical trapping by complex beam shaping. Laser Photonics Rev. 2013, 7, 839–854. [Google Scholar] [CrossRef]
- Kovalev, A.A.; Kotlyar, V.V.; Porfirev, A.P. A highly efficient element for generating elliptic perfect optical vortices. Appl. Phys. Lett. 2017, 110, 261102. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shu, B.; Zhang, Y.; Chang, H.; Tang, S.; Leng, J.; Xu, J.; Zhou, P. Flexible Modulation of Perfect Vortex Beams by Combining Coherent Beams. Photonics 2024, 11, 385. https://doi.org/10.3390/photonics11040385
Shu B, Zhang Y, Chang H, Tang S, Leng J, Xu J, Zhou P. Flexible Modulation of Perfect Vortex Beams by Combining Coherent Beams. Photonics. 2024; 11(4):385. https://doi.org/10.3390/photonics11040385
Chicago/Turabian StyleShu, Bowang, Yuqiu Zhang, Hongxiang Chang, Shiqing Tang, Jinyong Leng, Jiangming Xu, and Pu Zhou. 2024. "Flexible Modulation of Perfect Vortex Beams by Combining Coherent Beams" Photonics 11, no. 4: 385. https://doi.org/10.3390/photonics11040385
APA StyleShu, B., Zhang, Y., Chang, H., Tang, S., Leng, J., Xu, J., & Zhou, P. (2024). Flexible Modulation of Perfect Vortex Beams by Combining Coherent Beams. Photonics, 11(4), 385. https://doi.org/10.3390/photonics11040385



