Optical Design of a Wavelength Selective Switch Utilizing a Waveguide Frontend with Beamsteering Capability
Abstract
1. Introduction
2. Principle and Design
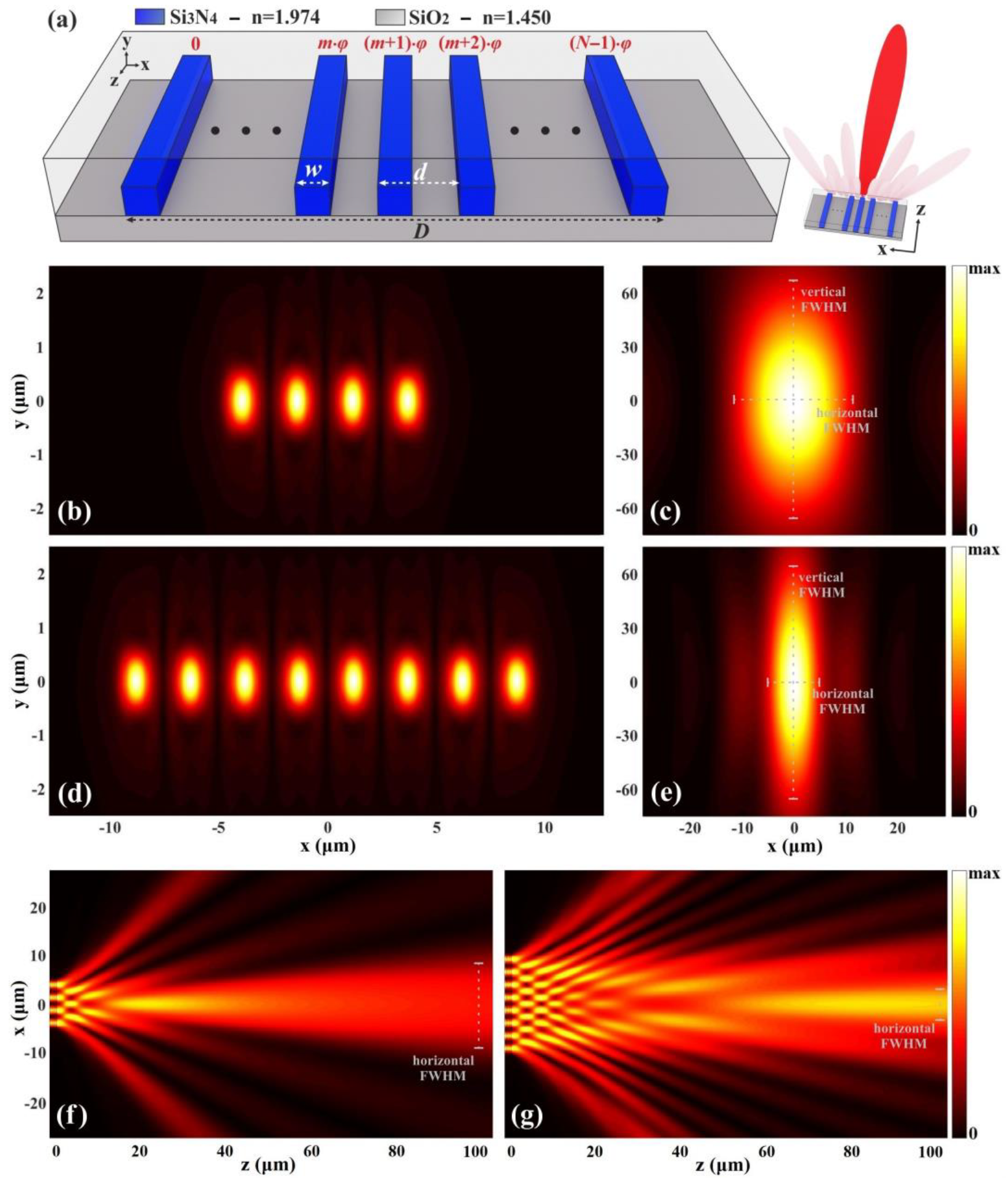
2.1. Main Beam Calculations for the SiN OPA
2.2. Incorporation of an OPA-Based WFE in a Hybrid WSS
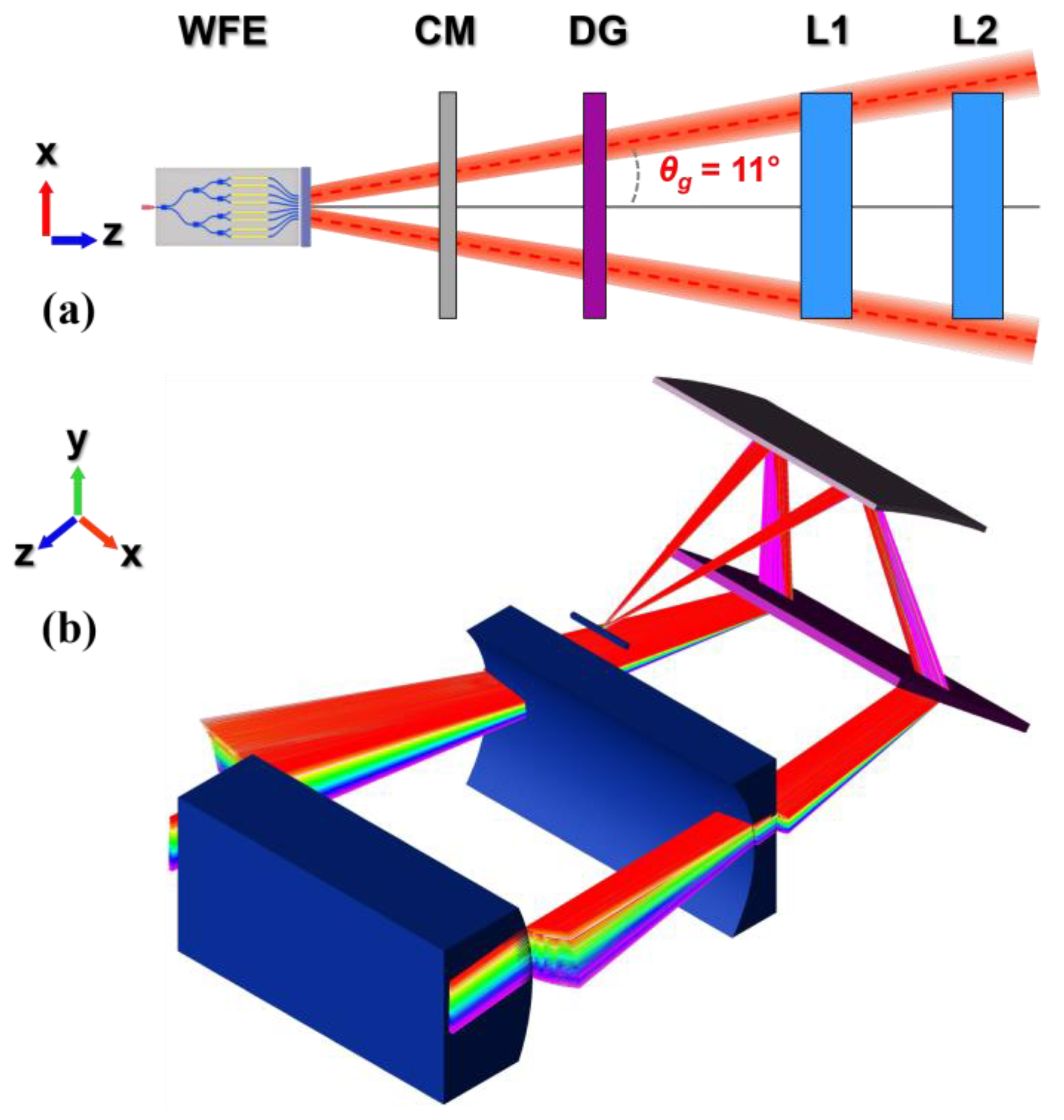
3. Optical Design of the OPA-Based WSS System with Beamsteering Capability
3.1. Beamsteering Calculations for SiN-Based OPAs
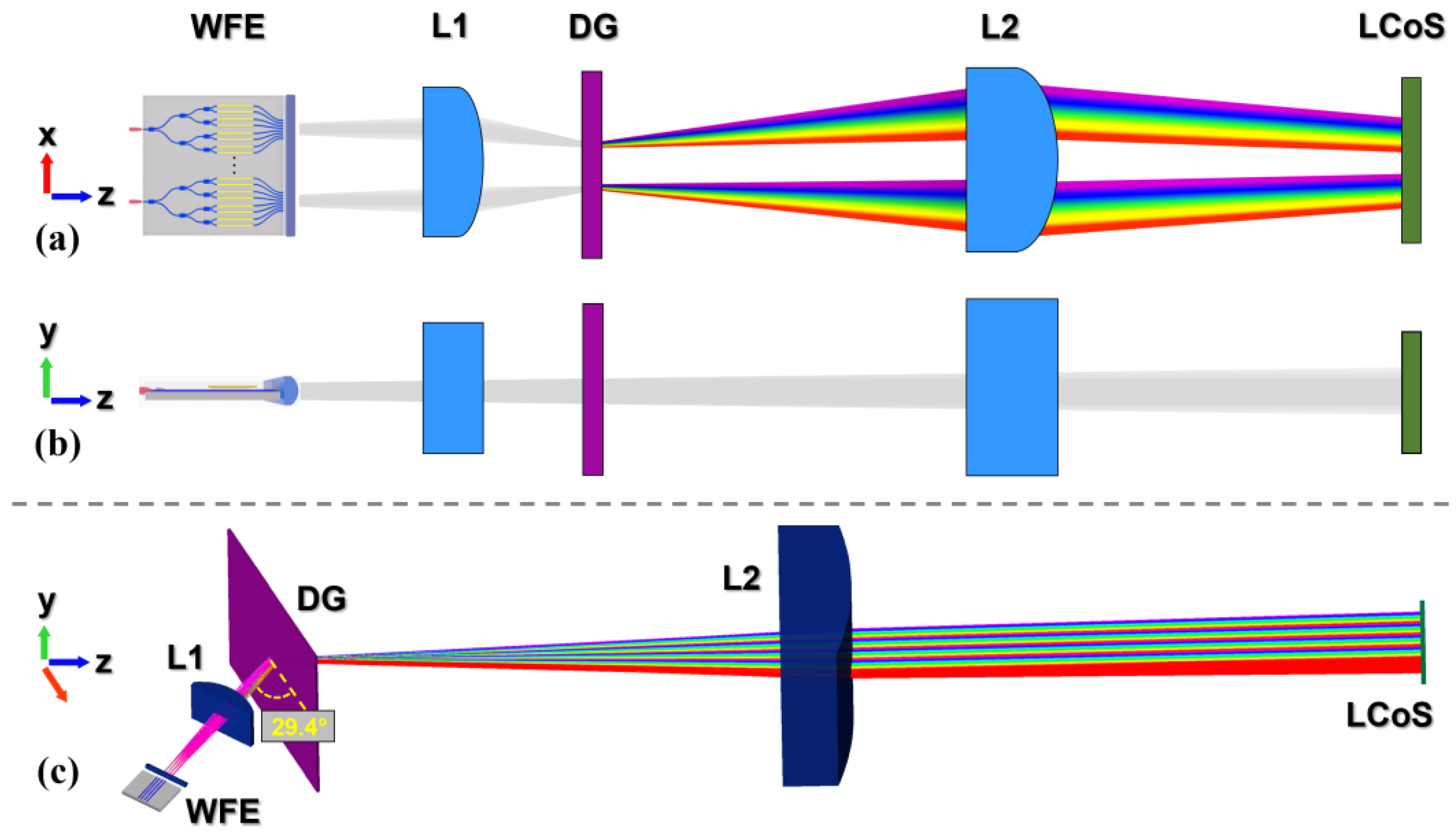
3.2. Hybrid WSS Enabling Beamsteering Functionality of the Frontend
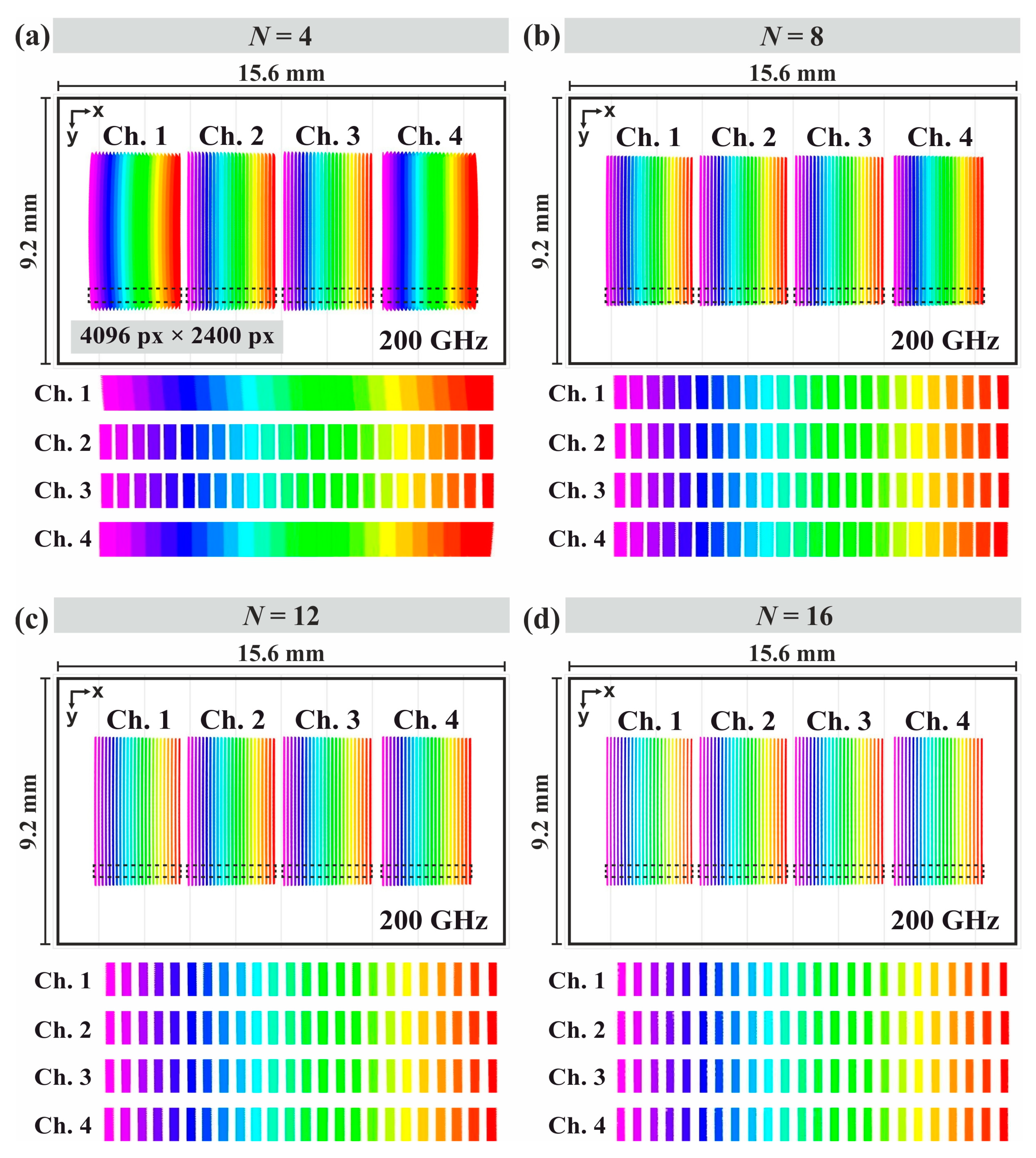
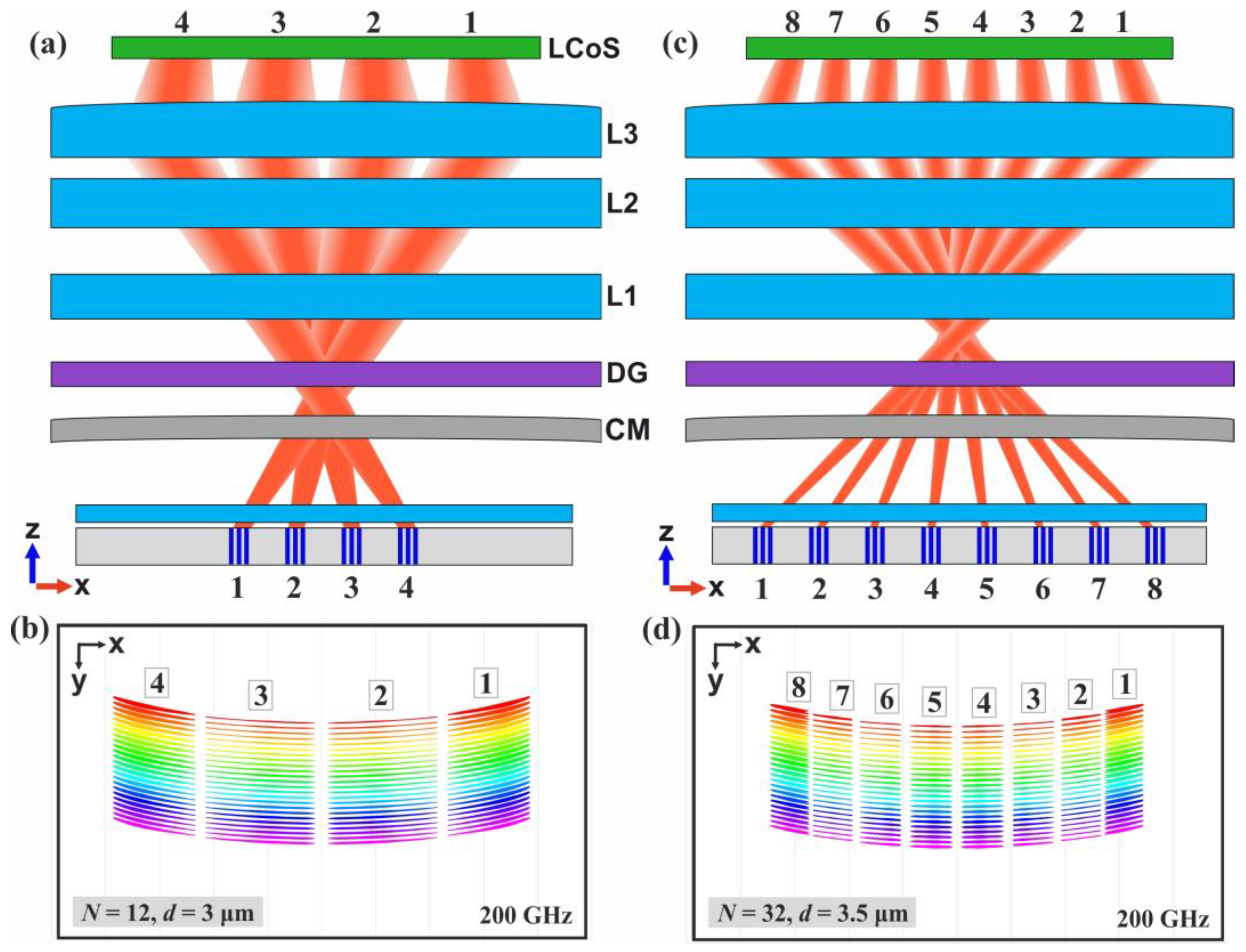
3.3. OPA Beamwidth and WSS Channels
3.4. Simulation of Undesired Grating-Lobes in the Hybrid WSS
3.5. Channel Routing by Varying Input Beam Angles
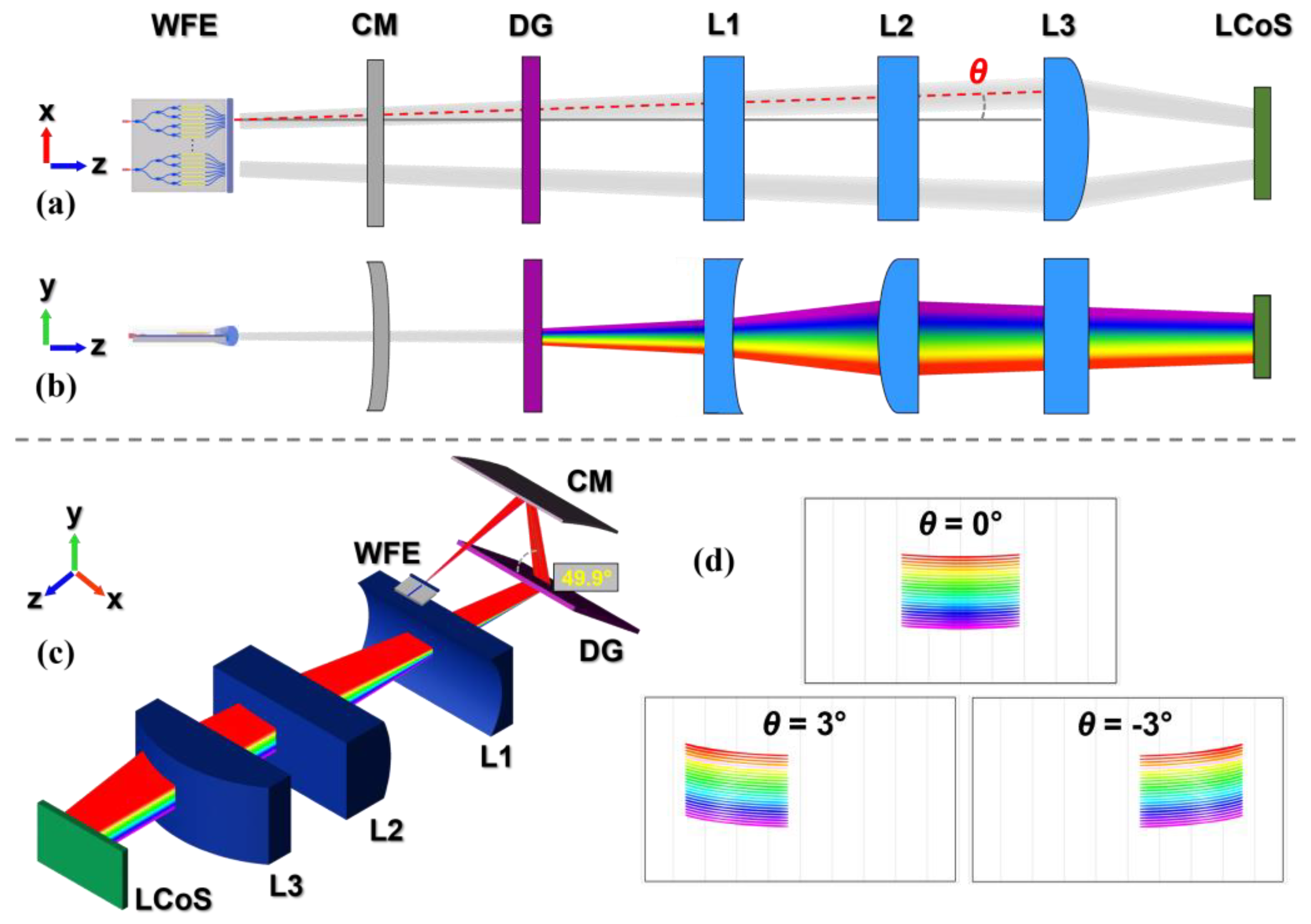
4. Misalignment Tolerance Study
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kozdrowski, S.; Żotkiewicz, M.; Sujecki, S. Optimization of Optical Networks Based on CDC-ROADM Technology. Appl. Sci. 2019, 9, 399. [Google Scholar] [CrossRef]
- Li, Y.; Gao, L.; Shen, G.; Peng, L. Impact of ROADM colorless, directionless, and contentionless (CDC) features on optical network performance [Invited]. J. Opt. Commun. Netw. 2012, 4, B58–B67. [Google Scholar] [CrossRef]
- Yamamoto, S.; Taniguchi, H.; Kisaka, Y.; Camatel, S.; Ma, Y.; Ogawa, D.; Hadama, K.; Fukutoku, M.; Goh, T.; Suzuki, K. First demonstration of a C + L band CDC-ROADM with a simple node configuration using multiband switching devices. Opt. Express 2021, 29, 36353–36365. [Google Scholar] [CrossRef] [PubMed]
- Xie, D.; Wang, D.; Zhang, M.; Liu, Z.; You, Q.; Yang, Q.; Yu, S. LCoS-Based Wavelength-Selective Switch for Future Finer-Grid Elastic Optical Networks Capable of All-Optical Wavelength Conversion. IEEE Photonics J. 2017, 9, 7101212. [Google Scholar] [CrossRef]
- Robertson, B.; Yang, H.; Redmond, M.M.; Collings, N.; Moore, J.R.; Liu, J.; Jeziorska Chapman, A.M.; Pivnenko, M.; Lee, S.; Wonfor, A.; et al. Demonstration of Multi-Casting in a 1 × 9 LCOS Wavelength Selective Switch. J. Light. Technol. 2014, 32, 402–410. [Google Scholar] [CrossRef]
- Huang, Z.; Yang, S.; Zheng, Z.; Pan, X.; Li, H.; Yang, H. Highly Compact Twin 1 × 35 Wavelength Selective Switch. J. Light. Technol. 2023, 41, 233–239. [Google Scholar] [CrossRef]
- Yang, H.; Wilkinson, P.; Robertson, B.; Giltrap, S.; Snowdon, O.; Prudden, H.; Chu, D. 24 [1 × 12] Wavelength Selective Switches Integrated on a Single 4k LCoS Device. J. Light. Technol. 2021, 39, 1033–1039. [Google Scholar] [CrossRef]
- Suzuki, K.; Seno, K.; Ikuma, Y. Application of Waveguide/Free-Space Optics Hybrid to ROADM Device. J. Light. Technol. 2017, 35, 596–606. [Google Scholar] [CrossRef]
- Sakamaki, Y.; Shikama, K.; Ikuma, Y.; Suzuki, K. Wavelength selective switch array employing silica-based waveguide frontend with integrated polarization diversity optics. Opt. Express 2017, 25, 19946–19954. [Google Scholar] [CrossRef]
- Kim, S.M.; Lee, E.S.; Chun, K.W.; Jin, J.; Oh, M.C. Compact solid-state optical phased array beam scanners based on polymeric photonic integrated circuits. Sci. Rep. 2021, 11, 10576. [Google Scholar] [CrossRef]
- Li, Y.; Chen, B.; Na, Q.; Tao, M.; Liu, X.; Zhi, Z.; Peng, T.; Li, X.; Luo, X.; Lo, G.; et al. High-Data-Rate and Wide-Steering-Range Optical Wireless Communication Via Nonuniform-Space Optical Phased Array. J. Light. Technol. 2023, 41, 4933–4940. [Google Scholar] [CrossRef]
- Di, Y.; Sun, C.; Chen, S.; Liu, W.; Dai, Y.; Li, B.; Shi, W.; Lin, J.; Shao, Y.; Xu, J.; et al. Capacity Enhancement of VLC by Blue-green Wavelength Division Multiplexing Using Optical Phased Array. In Proceedings of the 2023 Optical Fiber Communications Conference and Exhibition (OFC), San Diego, CA, USA, 5–9 March 2023; p. M4F.2. [Google Scholar]
- Bauters, J.F.; Heck MJ, R.; John, D.; Dai, D.; Tien, M.-C.; Barton, J.S.; Leinse, A.; Heideman, R.G.; Blumenthal, D.J.; Bowers, J.E. Ultra-low-loss high-aspect-ratio Si3N4 waveguides. Opt. Express 2011, 19, 3163–3174. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Fan, Z.; Zhou, J.; Cong, Q.; Zeng, X.; Zhang, Y.; Jia, L. Process Development of Low-Loss LPCVD Silicon Nitride Waveguides on 8-Inch Wafer. Appl. Sci. 2023, 13, 3660. [Google Scholar] [CrossRef]
- Liu, J.; Huang, G.; Wang, R.N.; He, J.; Raja, A.S.; Liu, T.; Engelsen, N.J.; Kippenberg, T.J. High-yield, wafer-scale fabrication of ultralow-loss, dispersion-engineered silicon nitride photonic circuits. Nat. Commun. 2021, 12, 2236. [Google Scholar] [CrossRef]
- Tien, M.-C.; Bauters, J.F.; Heck MJ, R.; Blumenthal, D.J.; Bowers, J.E. Ultra-low loss Si3N4 waveguides with low nonlinearity and high power handling capability. Opt. Express 2010, 18, 23562–23568. [Google Scholar] [CrossRef]
- Volakis, J.L. Antenna Engineering Handbook, 4th ed.; McGraw-Hill Education: New York, NY, USA, 2007; Chapter One; p. 21. [Google Scholar]
- Balanis, C.A. Antenna Theory: Analysis and Design, 4th ed.; Wiley: Hoboken, NJ, USA, 2016; pp. 293–297. [Google Scholar]
- Ji, H.; Shan, Y.; Mo, Y.; Chen, Z.; Ma, D. Optical Design of an LCoS-Based 1 × 10 WSS with High Coupling Efficiency and Compact Light Paths. Photonics 2023, 10, 1107. [Google Scholar] [CrossRef]
- Schifano, L.; Berghmans, F.; Dewitte, S.; Smeesters, L. Optical Design of a Novel Wide-Field-of-View Space-Based Spectrometer for Climate Monitoring. Sensors 2022, 22, 5841. [Google Scholar] [CrossRef]
- Zeng, C.; Han, Y.; Liu, B.; Sun, P.; Li, X.; Chen, P. Optical design of a high-resolution spectrometer with a wide field of view. Opt. Lasers Eng. 2021, 140, 106547. [Google Scholar] [CrossRef]
- Hu, Y.; Chang, J.; Ji, Z.; Chen, W.; Li, Y.; Li, D. Construction method for designing a spectrometer with variable spectral resolution and wide bandwidth using multiple off-axis convex gratings. Opt. Express 2022, 30, 2472–2486. [Google Scholar] [CrossRef]
- Peatross, J.; Ware, M. Physics of Light and Optics; Brigham Young University: Provo, UT, USA, 2015; pp. 236–247. [Google Scholar]
- Website of the Commercial LCoS SLM. Available online: https://holoeye.com/products/spatial-light-modulators/gaea-2-phase-only/ (accessed on 1 June 2023).
- Website of the Commercial Diffraction Grating. Available online: https://ibsen.com/products/transmission-gratings/ping-telecom-gratings/ (accessed on 1 June 2023).
- Yunshu, G.; Xiao, C.; Genxiang, C.; Ying, C.; Qiao, C.; Feng, X.; Yiquan, W. 1 × 25 LCOS-based wavelength selective switch with flexible passbands and channel selection. Opt. Fiber Technol. 2018, 45, 29–34. [Google Scholar]
- Muttikulangara, S.S.; Baranski, M.; Rehman, S.; Hu, L.; Miao, J. Diffraction grating integrated on micromachined stepper motor for diversity implementation in imaging spectroscopy. In Proceedings of the 2018 IEEE Micro Electro Mechanical Systems (MEMS), Belfast, UK, 21–25 January 2018; pp. 696–699. [Google Scholar]
- Poulton, C.V.; Byrd, M.J.; Russo, P.; Moss, B.; Shatrovoy, O.; Khandaker, M.; Watts, M.R. Coherent LiDAR With an 8192-Element Optical Phased Array and Driving Laser. IEEE J. Sel. Top. Quantum Electron. 2022, 28, 6100508. [Google Scholar] [CrossRef]
- Zhang, X.; Kwon, K.; Henriksson, J.; Luo, J.; Wu, M.C. A large-scale microelectromechanical-systems-based silicon photonics LiDAR. Nature 2022, 603, 253–258. [Google Scholar] [CrossRef] [PubMed]
- Das, A.N.; Sin, J.; Popa, D.O.; Stephanou, H.E. On the precision alignment and hybrid assembly aspects in manufacturing of a microspectrometer. In Proceedings of the 2008 IEEE International Conference on Automation Science and Engineering, Arlington, VA, USA, 23–26 August 2008; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA; pp. 959–966. [Google Scholar]
- Mohaupt, M.; Beckert, E.; Eberhardt, R.; Tünnermann, A. Alignment Procedures for Micro-optics. In IFIP Advances in Information and Communication Technology; Springer: Berlin/Heidelberg, Germany, 2010; Volume 315, pp. 143–150. [Google Scholar]
- Poulton, C.V.; Byrd, M.J.; Raval, M.; Su, Z.; Li, N.; Timurdogan, E.; Coolbaugh, D.; Vermeulen, D.; Watts, M.R. Large-scale silicon nitride nanophotonic phased arrays at infrared and visible wavelength. Opt. Lett. 2017, 42, 21–24. [Google Scholar] [CrossRef] [PubMed]
- Yao, W.; Gilardi, G.; Calabretta, N.; Smit, M.K.; Wale, M.J. Experimental and Numerical Study of Electrical Crosstalk in Photonic-Integrated Circuits. J. Light. Technol. 2015, 33, 934–9425. [Google Scholar] [CrossRef]
- Jacques, M.; Samani, A.; El-Fiky, E.; Patel, D.; Xing, Z.; Plant, D.V. Optimization of thermo-optic phase-shifter design and mitigation of thermal crosstalk on the SOI platform. Opt. Express 2019, 27, 10456–10471. [Google Scholar] [CrossRef] [PubMed]
- Brunetti, G.; Heuvink, R.; Schreuder, E.; Armenise, M.N.; Ciminelli, C. Silicon Nitride Spot Size Converter With Very Low-Loss Over the C-Band. IEEE Photonics Technol. Lett. 2023, 22, 1215–1218. [Google Scholar] [CrossRef]
- Bhandari, B.; Wang, C.; Gwon, J.Y.; Heo, J.M.; Ko, S.Y.; Oj, M.C.; Lee, S.S. Dispersive silicon–nitride optical phased array incorporating arrayed waveguide delay lines for passive line beam scanning. Sci. Rep. 2022, 12, 18759. [Google Scholar] [CrossRef] [PubMed]
- Idres, S.; Hashemi, H. Low-Power SiN Thermo-Optic Phase Modulator Operating in Red Visible Wavelength Range. In Proceedings of the Conference on Lasers and Electro-Optics: Applications and Technology 2020, Washington, DC, USA, 10–15 May 2020; pp. 1–2. [Google Scholar]
- Yong, Z.; Chen, H.; Luo, X.; Govdeli, A.; Chua, H.; Azadeh, S.S.; Stalmashonak, A.; Lo, G.Q.; Poon, J.K.S.; Sacher, W.D. Power-efficient silicon nitride thermo-optic phase shifters for visible light. Opt. Express 2022, 30, 7225. [Google Scholar] [CrossRef]
- Wu, Z.; Lin, S.; Yu, S.; Zhang, Y. Submilliwatt Silicon Nitride Thermo-Optic Modulator Operating at 532 nm. Photonics 2024, 11, 213. [Google Scholar] [CrossRef]
- Alemany, R.; Muñoz, P.; Pastor, D.; Domínguez, C. Thermo-Optic Phase Tuners Analysis and Design for Process Modules on a Silicon Nitride Platform. Photonics 2021, 8, 496. [Google Scholar] [CrossRef]
- Ortmann, J.E.; Eltes, F.; Caimi, D.; Meimer, N.; Demkov, A.A.; Czornomaz, L.; Fompeyrine, J.; Abel, S. Ultra-Low-Power Tuning in Hybrid Barium Titanate–Silicon Nitride Electro-optic Devices on Silicon. ACS Photonics 2019, 6, 2677–2684. [Google Scholar] [CrossRef]
- Kohli, M.; Chelladurai, D.; Messner, A.; Horst, Y.; Moor, D.; Winiger, J.; Blatter, T.; Buriakova, T.; Convertino, C.; Eltes, F.; et al. Plasmonic Ferroelectric Modulator Monolithically Integrated on SiN for 216 GBd Data Transmission. J. Light. Technol. 2023, 41, 3825–3831. [Google Scholar] [CrossRef]
- Ahmed AN, R.; Nelan, S.; Shi, S.; Yao, P.; Mercante, A.; Prather, D.W. Subvolt electro-optical modulator on thin-film lithium niobate and silicon nitride hybrid platform. Opt. Lett. 2020, 45, 1112–1115. [Google Scholar] [CrossRef] [PubMed]
- Lee, W.B.; Kwon, Y.J.; Kim, D.H.; Sunwoo, Y.H.; Lee, S.S. Hybrid integrated thin-film lithium niobate–silicon nitride electro-optical phased array incorporating silicon nitride grating antenna for two-dimensional beam steering. Opt. Express 2024, 32, 9171–9183. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Li, X.; Ji, J.; Sun, Z.; Sun, J.; Fang, B.; Lu, J.; Li, S.; Ma, X.; Chen, X.; et al. Fast-speed and low-power-consumption optical phased array based on lithium niobate waveguides. Nanophotonics 2024. [Google Scholar] [CrossRef]
- Qiu, C.; Yang, Y.; Li, C.; Wang, Y.; Wu, K.; Chen, J. All-optical control of light on a graphene-on-silicon nitride chip using thermo-optic effect. Sci. Rep. 2017, 7, 17046. [Google Scholar] [CrossRef]
- Ji, L.; Chen, W.; Gao, Y.; Xu, Y.; Wu, C.; Wang, X.; Yi, Y.; Li, B.; Sun, X.; Zhang, D. Low-power electro–optic phase modulator based on multilayer graphene/silicon nitride waveguide. Chin. Phys. B 2020, 29, 084207. [Google Scholar] [CrossRef]
- Brunetti, G.; Conteduca, D.; Dell’Olio, F.; Ciminelli, C.; Armenise, M.N. Design of an ultra-compact graphene-based integrated microphotonic tunable delay line. Opt. Express 2018, 26, 4593–4604. [Google Scholar] [CrossRef]
- Capmany, J.; Domenech, D.; Muñoz, P. Silicon Graphene Reconfigurable CROWS and SCISSORS. IEEE Photonics J. 2015, 7, 2700609. [Google Scholar] [CrossRef]
- Sacher, W.D.; Mikkelsen, J.C.; Huang, Y.; Mak JC, C.; Yong, Z.; Luo, X.; Li, Y.; Dumais, P.; Jiang, J.; Goodwill, D.; et al. Monolithically Integrated Multilayer Silicon Nitride-on-Silicon Waveguide Platforms for 3-D Photonic Circuits and Devices. Proc. IEEE 2018, 106, 2232–2245. [Google Scholar] [CrossRef]
- Hulme, J.C.; Doylend, J.K.; Heck MJ, R.; Peters, J.D.; Davenport, M.L.; Bovington, J.T.; Coldren, L.A.; Bowers, J.E. Fully integrated hybrid silicon two dimensional beam scanner. Opt. Express 2015, 23, 5861–5874. [Google Scholar] [CrossRef] [PubMed]
- He, X.; Dong, T.; He, J.; Xu, Y. A Design Approach of Optical Phased Array with Low Side Lobe Level and Wide Angle Steering Range. Photonics 2021, 8, 63. [Google Scholar] [CrossRef]
- Kim, Y.; Yoon, H.; You, J.-B.; Kim, M.; Park, H.-H. Wide-Angle Beam-Steering Using an Optical Phased Array with Non-Uniform-Width Waveguide Radiators. Photonics 2020, 7, 56. [Google Scholar] [CrossRef]




| Grating-Lobe Angle (for φ = π/2) | Steering Angle θ (for φ = π/2) | Main Beam FWHM | Total Length D (μm) | Spacing d (μm) | N |
|---|---|---|---|---|---|
| 26.88° 23.54° | 9.32° 7.82° | 4.16° 3.47° | 18.5 22 | 2.5 3 | 8 |
| 28.46° 23.61° | 9.41° 7.82° | 2.77° 2.31° | 28.5 34 | 2.5 3 | 12 |
| 23.56° 20.19° | 7.81° 6.82° | 1.75° 1.46° | 46 53.5 | 3 3.5 | 16 |
| 23.60° 20.17° | 7.85 6.73° | 1.22° 1.12° | 70 81.5 | 3 3.5 | 24 |
| 20.23° 17.57° | 6.70° 5.87° | 0.84° 0.72° | 109.5 125 | 3.5 4 | 32 |
| 4 Channels (N = 12, d = 3 μm) | ||||||||
|---|---|---|---|---|---|---|---|---|
| Ch. number | 1 | 2 | 3 | 4 | ||||
| OPA Position (mm) | −0.75 | −0.25 | 0.25 | 0.75 | ||||
| Angle θ | 4.6° | 1.55° | −1.55° | −4.6° | ||||
| Hologram X size (px) | 634 | 845 | 845 | 634 | ||||
| Hologram Y size (px) | 28 ± 3 | 9 ± 4 | 28 ± 3 | 9 ± 4 | ||||
| Hologram Y spacing (px) | 12 ± 2 | 29 ± 6 | 29 ± 6 | 12 ± 2 | ||||
| 8 Channels (N = 32, d = 3.5 μm) | ||||||||
| Ch. number | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| OPA Position (mm) | −1.75 | −1.25 | −0.75 | −0.25 | 0.25 | 0.75 | 1.25 | 1.75 |
| Angle θ | 4.9° | 3.5° | 2.1° | 0.7° | −0.7° | −2.1° | −3.5° | −4.9° |
| Hologram X size (px) | 296 | 303 | 312 | 320 | 320 | 312 | 303 | 296 |
| Hologram Y size (px) | 25 ± 4 | 9 ± 4 | 7 ± 3 | 11 ± 4 | 11 ± 4 | 7 ± 3 | 9 ± 4 | 25 ± 4 |
| Hologram Y spacing (px) | 14 ± 3 | 32 ± 5 | 33 ± 5 | 28 ± 5 | 28 ± 5 | 33 ± 5 | 32 ± 5 | 14 ± 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Patsamanis, G.; Ketzaki, D.; Chatzitheocharis, D.; Vyrsokinos, K. Optical Design of a Wavelength Selective Switch Utilizing a Waveguide Frontend with Beamsteering Capability. Photonics 2024, 11, 381. https://doi.org/10.3390/photonics11040381
Patsamanis G, Ketzaki D, Chatzitheocharis D, Vyrsokinos K. Optical Design of a Wavelength Selective Switch Utilizing a Waveguide Frontend with Beamsteering Capability. Photonics. 2024; 11(4):381. https://doi.org/10.3390/photonics11040381
Chicago/Turabian StylePatsamanis, Georgios, Dimitra Ketzaki, Dimitrios Chatzitheocharis, and Konstantinos Vyrsokinos. 2024. "Optical Design of a Wavelength Selective Switch Utilizing a Waveguide Frontend with Beamsteering Capability" Photonics 11, no. 4: 381. https://doi.org/10.3390/photonics11040381
APA StylePatsamanis, G., Ketzaki, D., Chatzitheocharis, D., & Vyrsokinos, K. (2024). Optical Design of a Wavelength Selective Switch Utilizing a Waveguide Frontend with Beamsteering Capability. Photonics, 11(4), 381. https://doi.org/10.3390/photonics11040381







