Damascene Process Development for Low-Loss Photonics Devices with Applications in Frequency Comb
Abstract
1. Introduction
| Research Team | Wafer Size (inch) | Deposition Method | Cross-Sectional Size (μm2) |
|---|---|---|---|
| Ying Huang [31] | 8 | PECVD | 0.4 × 1.0 |
| Sheng Chun Mao [32] | 8 | PECVD | 0.4 × 0.7 |
| Zhendong Zhu [27] | 6 | LPCVD | 0.4 × 1.8 |
| Ling Hua Wang [33] | 4 | PECVD | 0.6 × 1.5 |
| Clemens J. Krückel [34] | 4 | LPCVD | 0.7 × 1.65 |
| Jörn P. Epping [26] | 4 | LPCVD | 0.9 × 1.2 |
| Tobias Kippenberg [4] | 4 | LPCVD | 0.9 × 2.1 |
| Zhichao Ye [35] | 3 | LPCVD | 0.74 × 1.5 |
| Houssein El Dirani [25] | 8 | LPCVD | 0.8 × 1.5 |
| This paper | 8 | LPCVD | 0.8 × 0.8 |
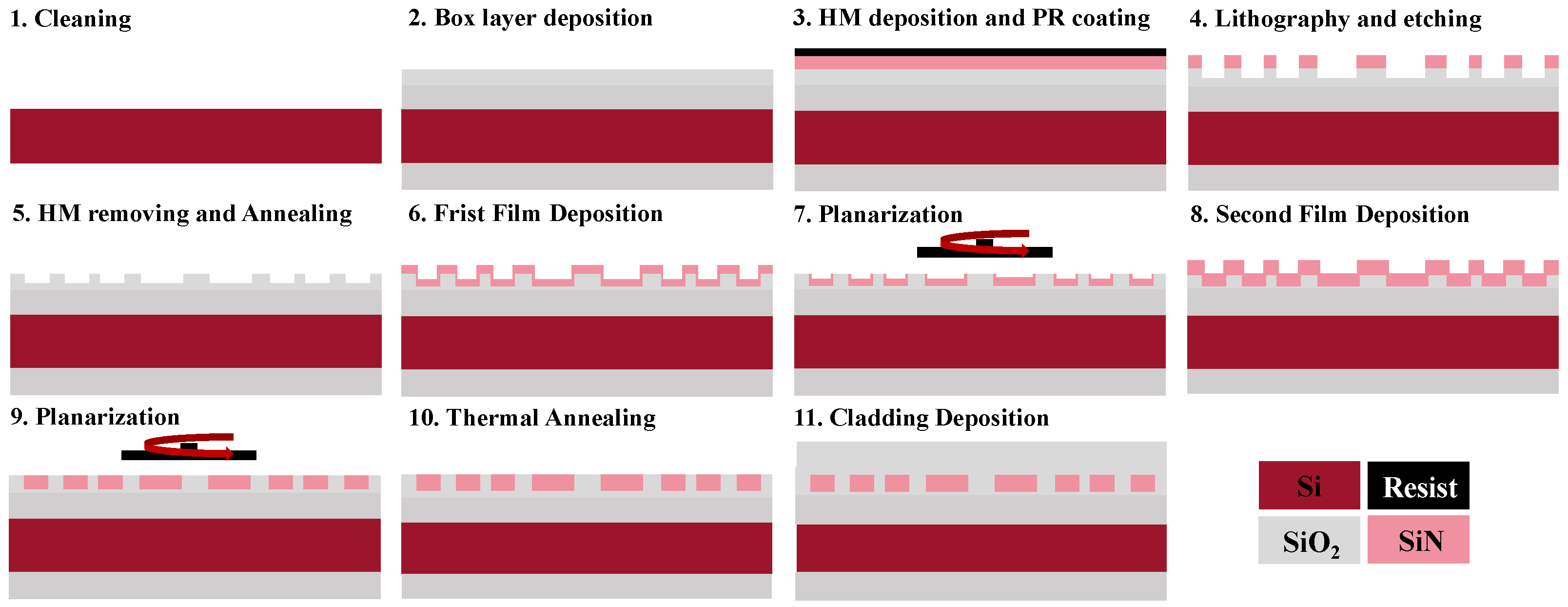
2. Fabrication Process
3. Silicon Nitride Waveguide
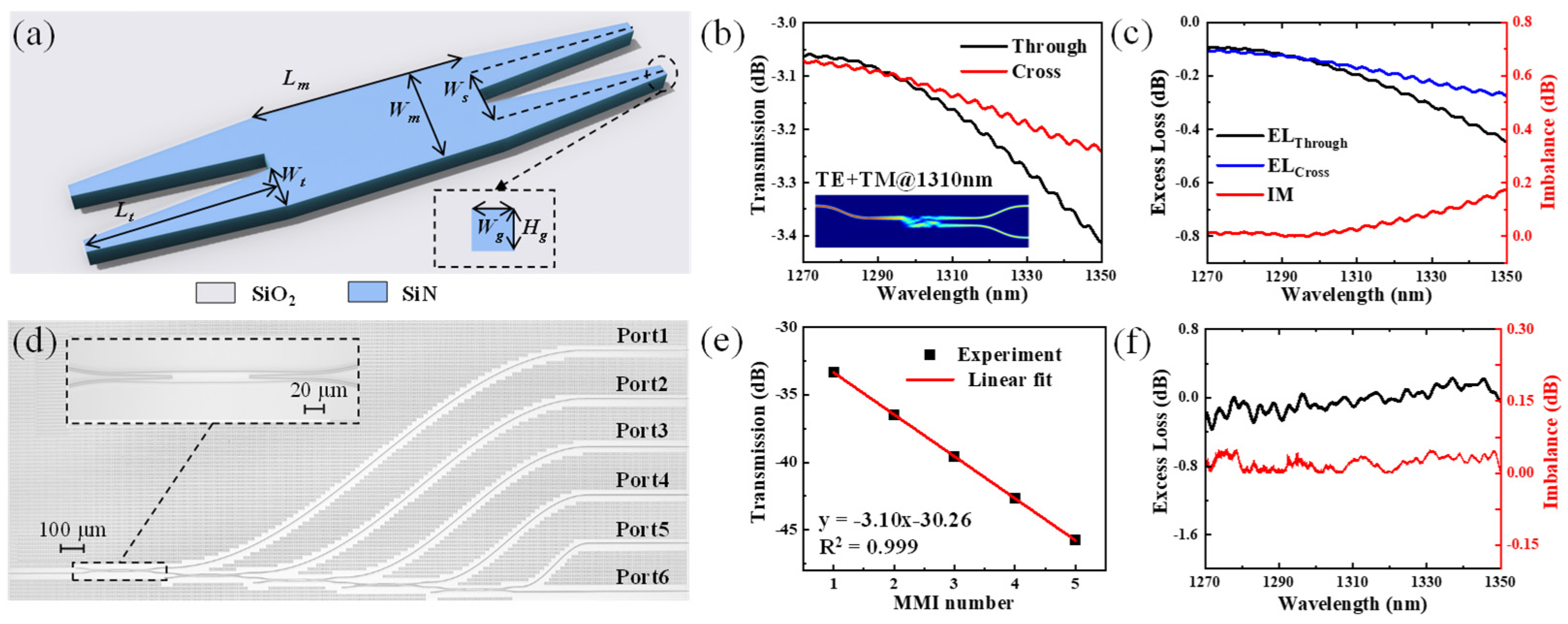
4. 2 × 2 Multimode Interference (MMI) Coupler
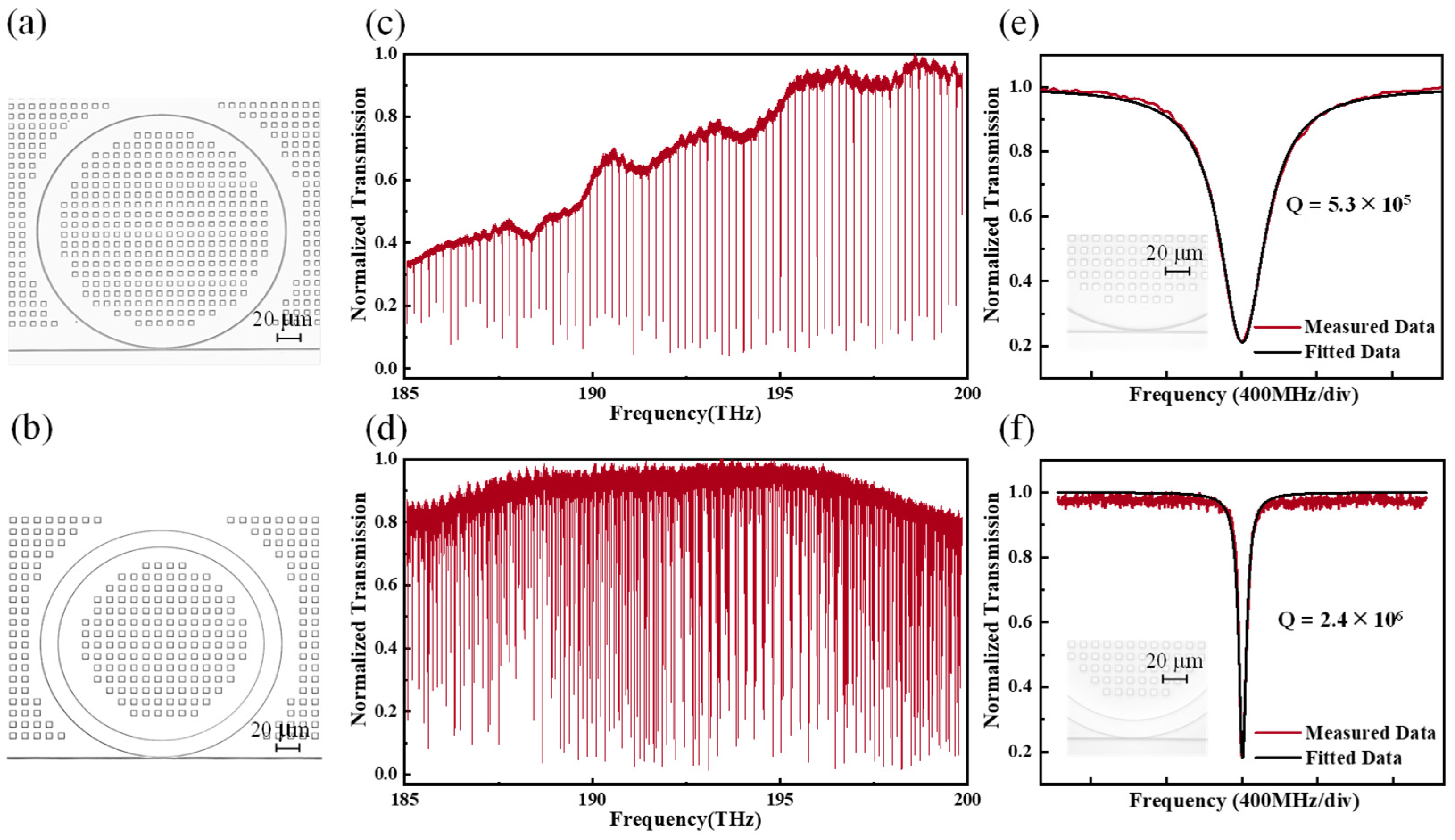
5. Micro-Ring Resonator
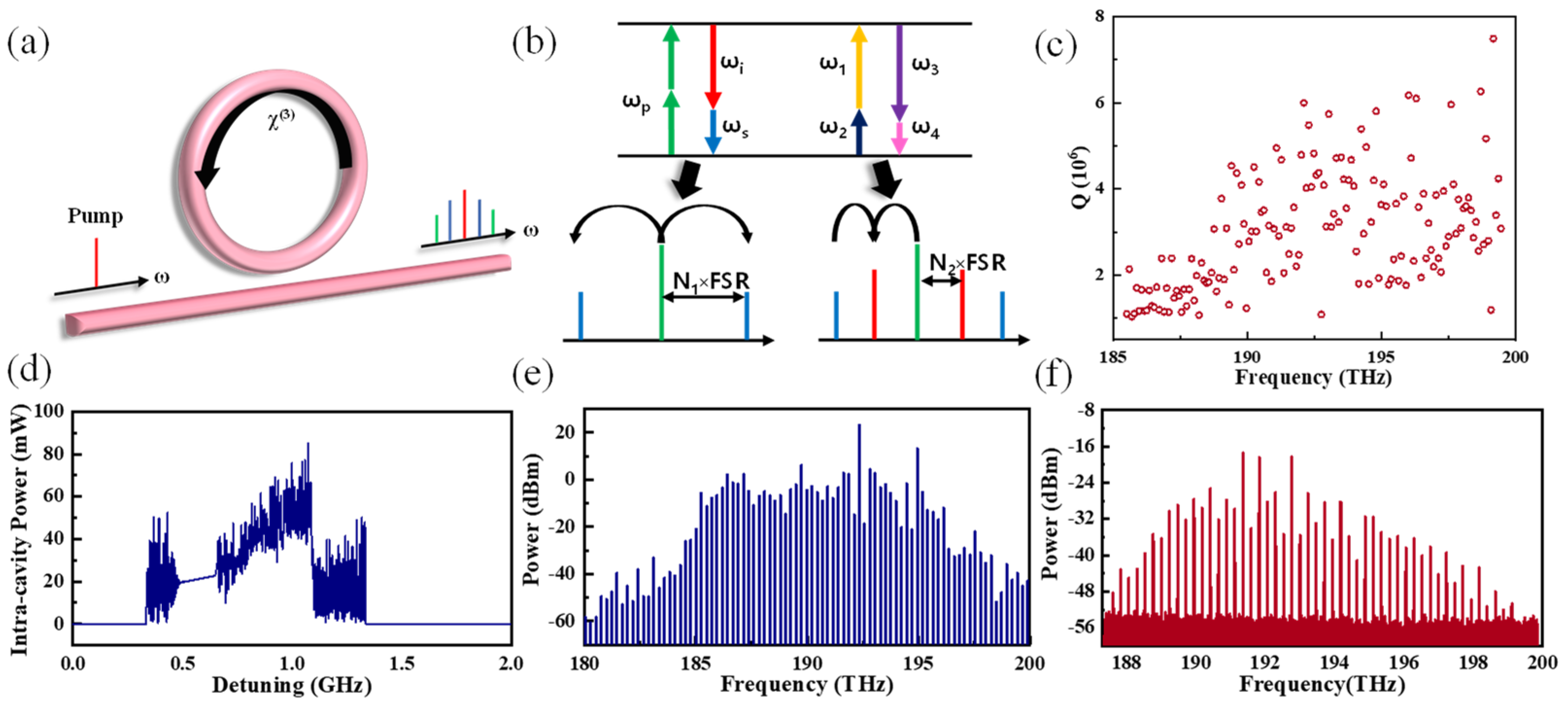
6. Optical Frequency Comb
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cummins, C.; Pan, B.; Roelkens, G.; Dahlem, M.; Saseendran, S.S.; Helin, P. Material Characterisation of LPCVD SiN and Understanding Loss Behavior. In Proceedings of the 2023 Conference on Lasers and Electro-Optics Europe & European Quantum Electronics Conference (CLEO/Europe-EQEC), Munich, Germany, 26–30 June 2023; p. 1. [Google Scholar]
- Chembo, Y.K. Kerr optical frequency combs: Theory, applications and perspectives. Nanophoton 2016, 5, 214–230. [Google Scholar] [CrossRef]
- Xiang, C.; Guo, J.; Jin, W.; Wu, L.; Peters, J.; Xie, W.; Chang, L.; Shen, B.; Wang, H.; Yang, Q.-F.; et al. High-performance lasers for fully integrated silicon nitride photonics. Nat. Commun. 2021, 12, 6650. [Google Scholar] [CrossRef]
- Liu, J.; Huang, G.; Wang, R.N.; He, J.; Raja, A.S.; Liu, T.; Engelsen, N.J.; Kippenberg, T.J. High-yield, wafer-scale fabrication of ultralow-loss, dispersion-engineered silicon nitride photonic circuits. Nat. Commun. 2021, 12, 2236. [Google Scholar] [CrossRef]
- Lu, J.; Shao, Z.; Wang, Y.; Zhang, Y.; Liu, L.; Yang, C.; Chen, Y.; Yu, S. Tunable Optical True Time Delay Lines Based on SiNx Arrayed Waveguide Grating and Spirals. In Proceedings of the 2017 Asia Communications and Photonics Conference (ACP), Guangzhou, China, 10–13 November 2017; pp. 1–3. [Google Scholar]
- Brasch, V.; Geiselmann, M.; Herr, T.; Lihachev, G.; Pfeiffer, M.H.P.; Gorodetsky, M.L.; Kippenberg, T.J. Photonic chip–based optical frequency comb using soliton Cherenkov radiation. Science 2016, 351, 357–360. [Google Scholar] [CrossRef]
- Chang, L.; Liu, S.; Bowers, J.E. Integrated optical frequency comb technologies. Nat. Photonics 2022, 16, 95–108. [Google Scholar] [CrossRef]
- Ye, Z.; Twayana, K.; Torres-Company, V. High-Q Si3N4 microresonators based on a subtractive processing for Kerr nonlinear optics. Opt. Express 2019, 27, 35719. [Google Scholar] [CrossRef] [PubMed]
- Xiang, C.; Jin, W.; Guo, J.; Peters, J.D.; Kennedy, M.J.; Selvidge, J.; Morton, P.A.; Bowers, J.E. Narrow-linewidth III-V/Si/Si3N4 laser using multilayer heterogeneous integration. Optica 2020, 7, 20–21. [Google Scholar] [CrossRef]
- Gaeta, A.L.; Lipson, M.; Kippenberg, T.J. Photonic-chip-based frequency combs. Nat. Photonics 2019, 13, 158–169. [Google Scholar] [CrossRef]
- Trocha, P.; Karpov, M.; Ganin, D.; Pfeiffer, M.H.P.; Kordts, A.; Wolf, S.; Krockenberger, J.; Marin-Palomo, P.; Weimann, C.; Randel, S.; et al. Ultrafast optical ranging using microresonator soliton frequency combs. Science 2019, 359, 887–891. [Google Scholar] [CrossRef]
- Bao, C.; Zhang, L.; Matsko, A.; Yan, Y.; Zhao, Z.; Xie, G.; Agarwal, A.M.; Kimerling, L.C.; Michel, J.; Maleki, L.; et al. Nonlinear conversion efficiency in Kerr frequency comb generation. Opt. Lett. 2014, 39, 6126–6129. [Google Scholar] [CrossRef]
- Xue, X.; Zheng, X.; Zhou, B. Super-efhcient temporal solitons in mutuacoupled optical cavities. Nat. Photonics 2019, 13, 616–622. [Google Scholar] [CrossRef]
- Helgason, Ó.B.; Girardi, M.; Ye, Z.; Lei, F.; Schröder, J.; Victor Torres Company. Power-efcient soliton microcombs. arXiv 2022, arXiv:2202.09410. [Google Scholar]
- Liu, J.; Lucas, E.; Raja, A.S.; He, J.; Riemensberger, J.; Wang, R.N.; Karpov, M.; Guo, H.; Bouchand, R.; Kippenberg, T.J. Photonic microwave generation in the x- and k-bandusing integrated soliton microcombs. Nat. Photonics 2020, 14, 486–491. [Google Scholar] [CrossRef]
- Liu, Y.; Xuan, Y.; Xue, X.; Wang, P.H.; Chen, S.; Metcalf, A.J.; Wang, J.; Leaird, D.E.; Qi, M.; Weiner, A.M. Lnvestigation of mode coupling in normal-dispersionsilicon nitride microresonators for Kerr frequency comb generation. Optica 2014, 1, 137. [Google Scholar] [CrossRef]
- Lucas, E. Physics Ofdissipative Kerr Solitons in Optical Microresonators and Applicationto Frequency Synthesis; Swiss Federal Institute of Technology in Lausanne: Lausanne, Switzerland, 2019. [Google Scholar]
- Yu, M.; Jang, J.K.; Okawachi, Y.; Griffith, A.G.; Luke, K.; Miller, S.A.; Ji, X.; Lipson, M.; Gaeta, A.L. Breather soliton dynamics in microresonators. Nat. Commun. 2017, 8, 14569. [Google Scholar] [CrossRef]
- Qi, Z.; Wang, S.; Jaramillo-Villegas, J.; Qi, M.; Weiner, A.M.; D’aguanno, G.; Carruthers, T.F.; Menyuk, C.R. Dissipative cnoidal waves (Turing rolls) and the soliton limit in microring resonators. Optica 2019, 6, 1220. [Google Scholar] [CrossRef]
- Liu, J.; Raja, A.S.; Karpov, M.; Ghadiani, B.; Pfeiffer, M.H.P.; Du, B.; Engelsen, N.J.; Guo, H.; Zervas, M.; Kippenberg, T.J. Ultralow-power chip-based soliton microcombs for photonic integration. Optica 2018, 5, 1347. [Google Scholar] [CrossRef]
- Pfeiffer, M.H.P.; Kordts, A.; Kippenberg, T.J. Photonic Damascene process for integrated high-Q microresonator based nonlinear photonics. Optica 2016, 3, 20–25. [Google Scholar] [CrossRef]
- Li, D.; Li, B.; Tang, B.; Zhang, P.; Yang, Y.; Liu, R.; Xie, L.; Li, Z. Broadband Silicon Nitride Power Splitter Based on Bent Directional Couplers with Low Thermal Sensitivity. Micromachines 2022, 13, 559. [Google Scholar] [CrossRef]
- Jiang, W.; Xu, D. Effect of Hyperthermal Annealing on Lpcvd Silicon Nitride. Mater. Sci. Semicond. Process. 2016, 43, 222–229. [Google Scholar] [CrossRef]
- Park, T.; Son, C.; Kim, T.; Lim, S. Understanding of Si3N4-H3PO4 Reaction Chemistry for the Control of Si3N4 Dissolution Kinetics. J. Ind. Eng. Chem. 2021, 102, 146–154. [Google Scholar] [CrossRef]
- El Dirani, H.; Youssef, L.; Petit-Etienne, C.; Kerdiles, S.; Grosse, P.; Monat, C.; Pargon, E.; Sciancalepore, C. Ultralow-loss tightly confining Si3N4 waveguides and high-Q microresonators. Opt. Express 2019, 27, 30726–30740. [Google Scholar] [CrossRef] [PubMed]
- Li, B.; Li, D.; Tang, B.; Li, Z. Towards monolithic low-loss silicon nitride waveguides on a mature 200 mm CMOS platform. Optic 2021, 250, 168309. [Google Scholar] [CrossRef]
- Zhu, Z.; Lin, P.; Wang, X.; Sun, Z.; Bai, B. Fabrication of silicon nitride-based integrated microcavity optical frequency comb devices (Invited). Infrared Laser Eng. 2022, 51, 20220214. [Google Scholar]
- Roeloffzen, C.G.H.; Hoekman, M.; Klein, E.J.; Wevers, L.S.; Timens, R.B.; Marchenko, D.; Geskus, D.; Dekker, R.; Alippi, A.; Grootjans, R.; et al. Low-Loss Si3N4 Triplex Optical Waveguides: Technology and Applications Overview. IEEE J. Sel. Top. Quantum Electron. 2018, 24, 4400321. [Google Scholar] [CrossRef]
- Shang, K.; Pathak, S.; Guan, B.; Liu, G.; Yoo, S.J.B. Low-Loss Compact Multilayer Silicon Nitride Platform for 3d Photonic Integrated Circuits. Opt. Express 2015, 23, 21334–21342. [Google Scholar] [CrossRef] [PubMed]
- De Oliveira, E.C.; Gerosa, R.M.; Phelan, C.; de Matos, C.J. Fabrication and Characterization of Silicon Nitride Waveguides for Mid-Infrared Applications. In Proceedings of the 2019 SBFoton International Optics and Photonics Conference (SBFoton IOPC), Sao Paulo, Brazil, 7–9 October 2019. [Google Scholar]
- Huang, Y.; Song, J.; Luo, X.; Liow, T.-Y.; Lo, G.-Q. CMOS compatible monolithic multi-layer Si3N4-on-SOI platform for low-loss high performance silicon photonics dense integration. Opt. Express 2014, 22, 21859–21865. [Google Scholar] [CrossRef] [PubMed]
- Mao, S.C.; Tao, S.H.; Xu, Y.L.; Sun, X.W.; Yu, M.B.; Lo, G.Q.; Kwong, D.L. Low propagation loss SiN optical waveguide prepared by optimal low-hydrogen module. Opt. Express 2008, 16, 20809–20816. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Xie, W.; Van Thourhout, D.; Zhang, Y.; Yu, H.; Wang, S. Nonlinear Silicon Nitride Waveguides Based on a Pecvd Deposition Platform. Opt. Express 2018, 26, 9645–9654. [Google Scholar] [CrossRef]
- Krückel, C.J.; Fülöp, A.; Klintberg, T.; Bengtsson, J.; Andrekson, P.A.; Torres-Company, V. Linear and nonlinear characterization of low-stress high-confinement silicon-rich nitride waveguides. Opt. Express 2015, 23, 25827–25837. [Google Scholar] [CrossRef]
- YiKai, H.; PeiHsun, W. CMOS-compatible 6-inch wafer integration of photonic waveguides and uniformity analysis. Opt. Express 2024, 32, 7197–7206. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Q.; Jin, Y.; Zheng, S.; Zhao, X.; Qiu, Y.; Jia, L.; Dong, Y.; Zhong, Q.; Hu, T. Damascene Process Development for Low-Loss Photonics Devices with Applications in Frequency Comb. Photonics 2024, 11, 375. https://doi.org/10.3390/photonics11040375
Zhou Q, Jin Y, Zheng S, Zhao X, Qiu Y, Jia L, Dong Y, Zhong Q, Hu T. Damascene Process Development for Low-Loss Photonics Devices with Applications in Frequency Comb. Photonics. 2024; 11(4):375. https://doi.org/10.3390/photonics11040375
Chicago/Turabian StyleZhou, Qiaoling, Yejia Jin, Shaonan Zheng, Xingyan Zhao, Yang Qiu, Lianxi Jia, Yuan Dong, Qize Zhong, and Ting Hu. 2024. "Damascene Process Development for Low-Loss Photonics Devices with Applications in Frequency Comb" Photonics 11, no. 4: 375. https://doi.org/10.3390/photonics11040375
APA StyleZhou, Q., Jin, Y., Zheng, S., Zhao, X., Qiu, Y., Jia, L., Dong, Y., Zhong, Q., & Hu, T. (2024). Damascene Process Development for Low-Loss Photonics Devices with Applications in Frequency Comb. Photonics, 11(4), 375. https://doi.org/10.3390/photonics11040375





