Excitation and Tuning of Optical Tamm States in a Hybrid Structure with a Metal Film Adjacent to a Four-Layer Polymer–Liquid Crystal Stack
Abstract
1. Introduction
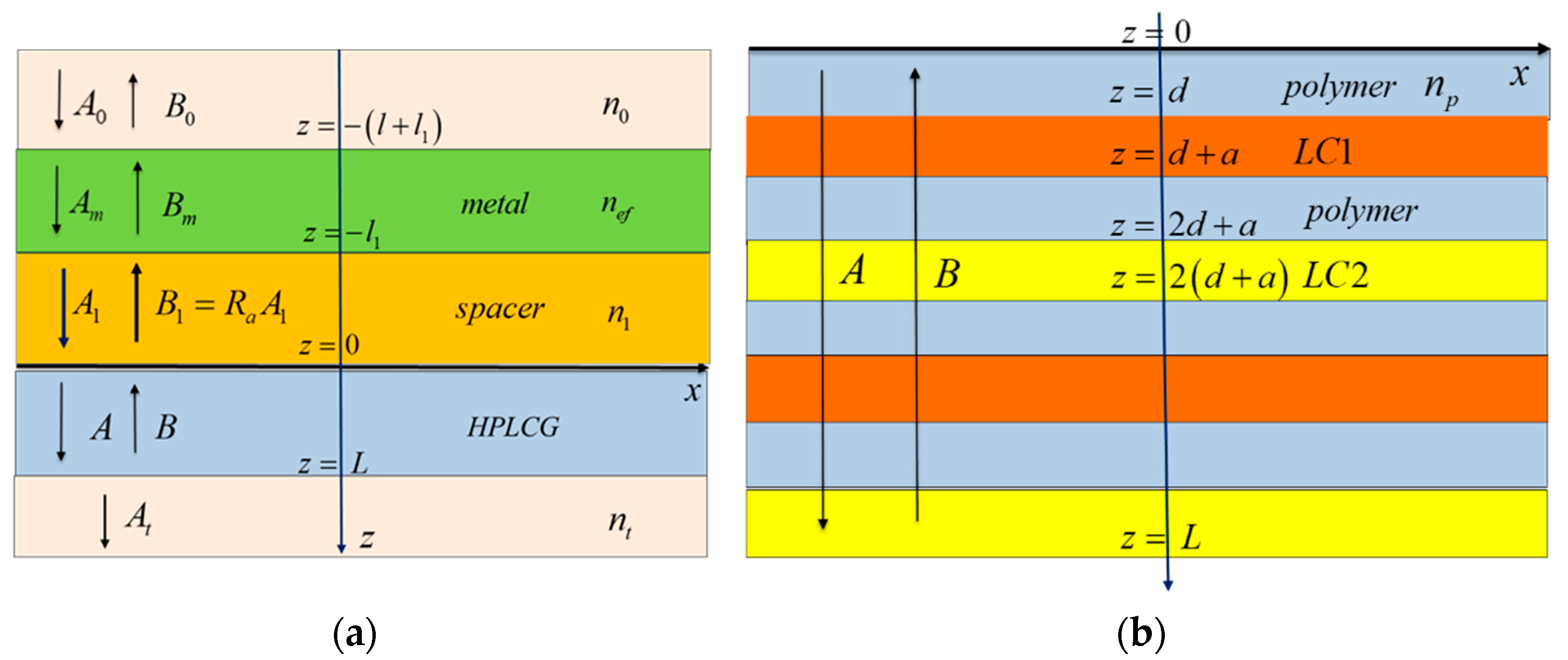
2. Materials and Methods
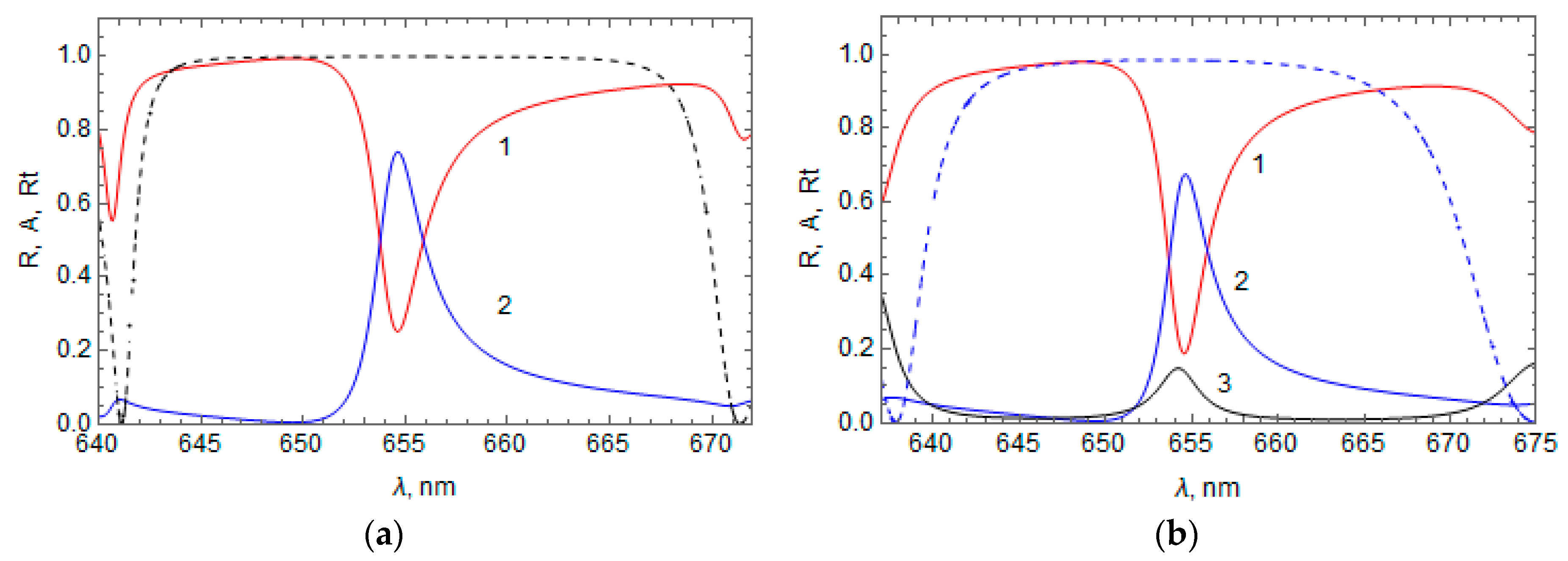
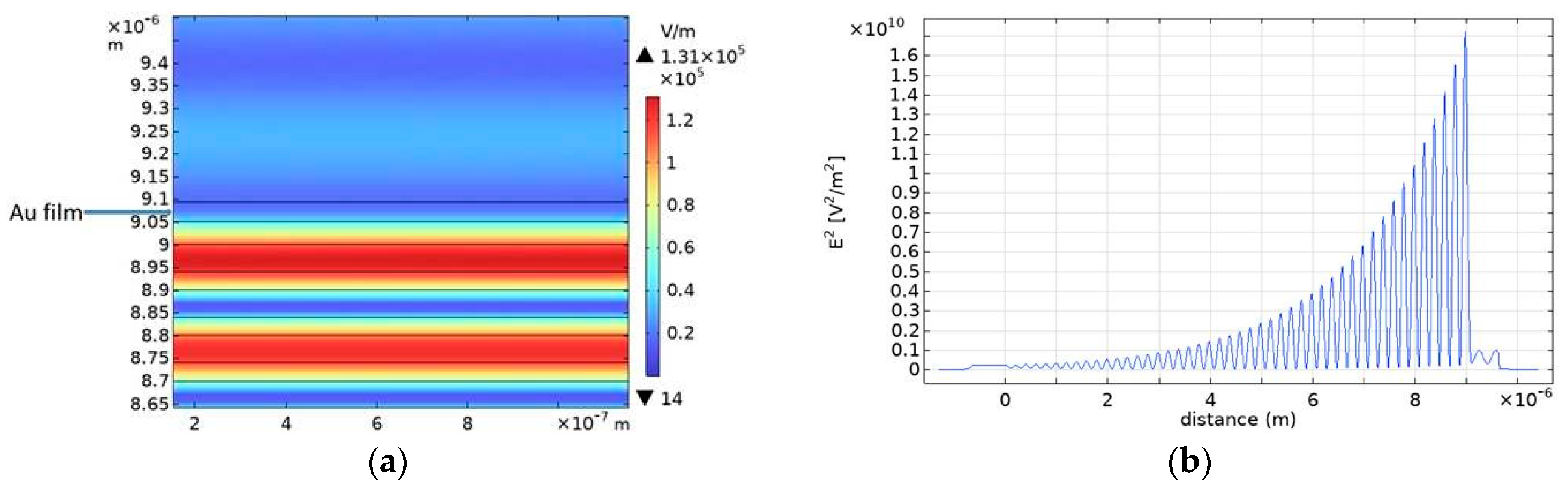
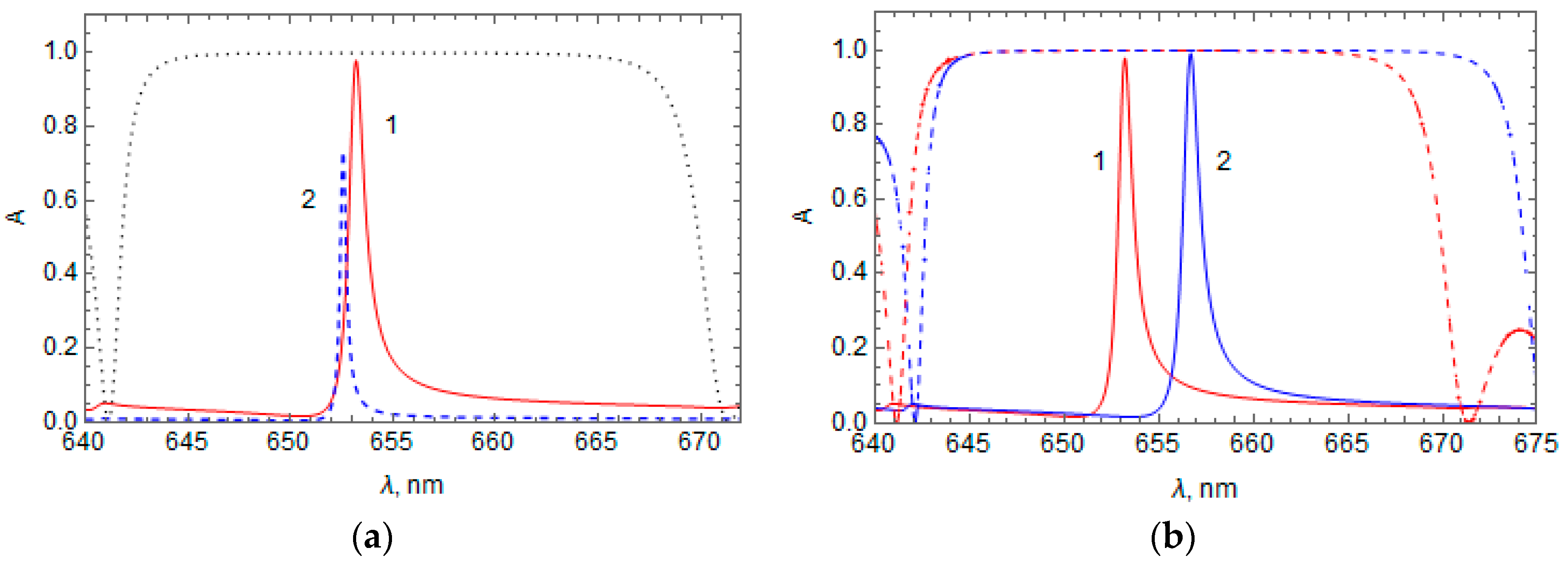
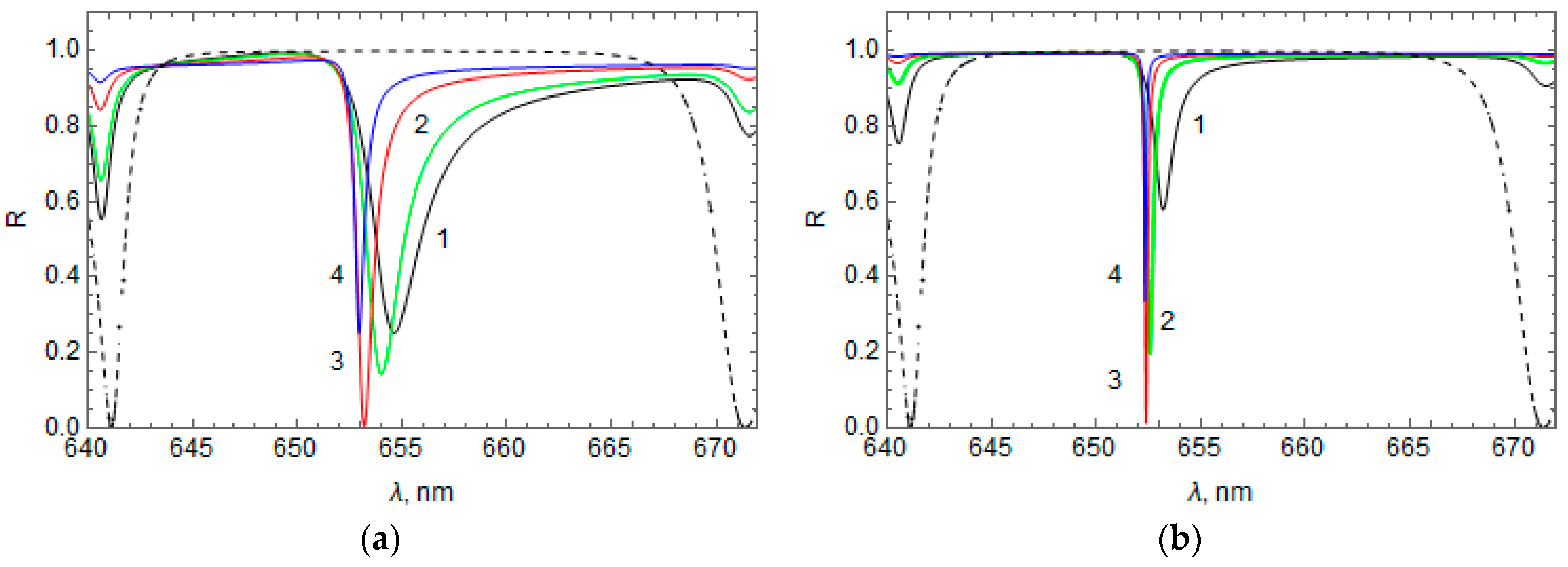
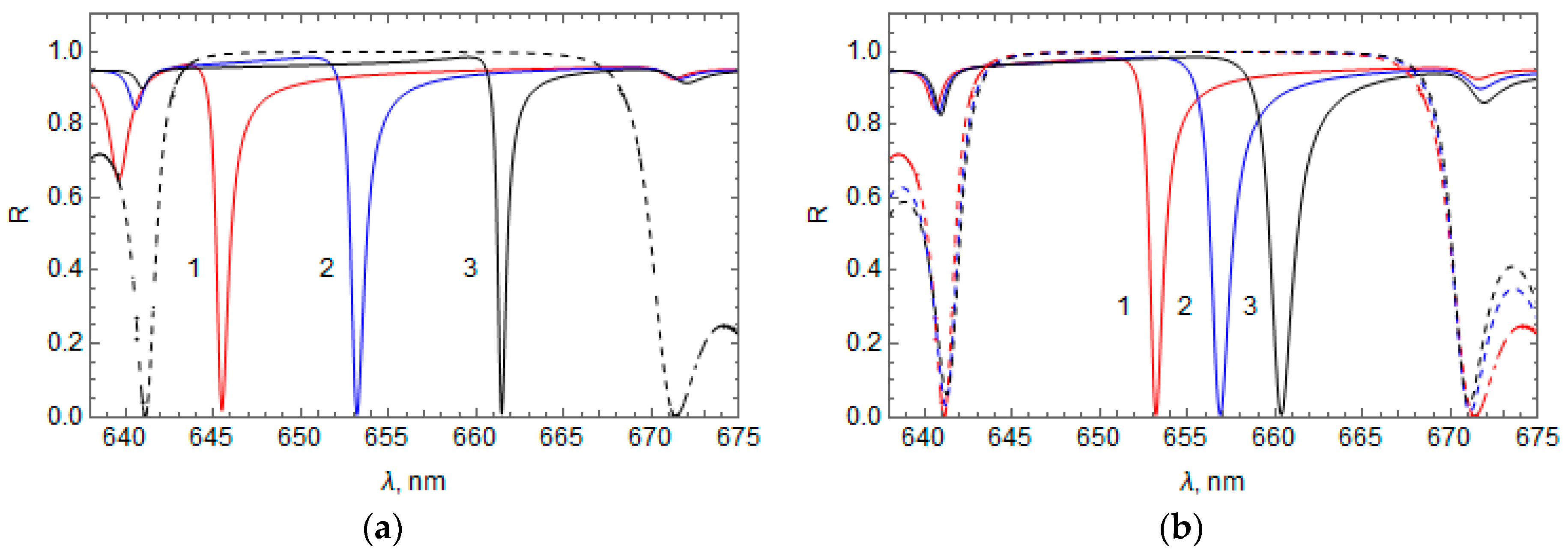
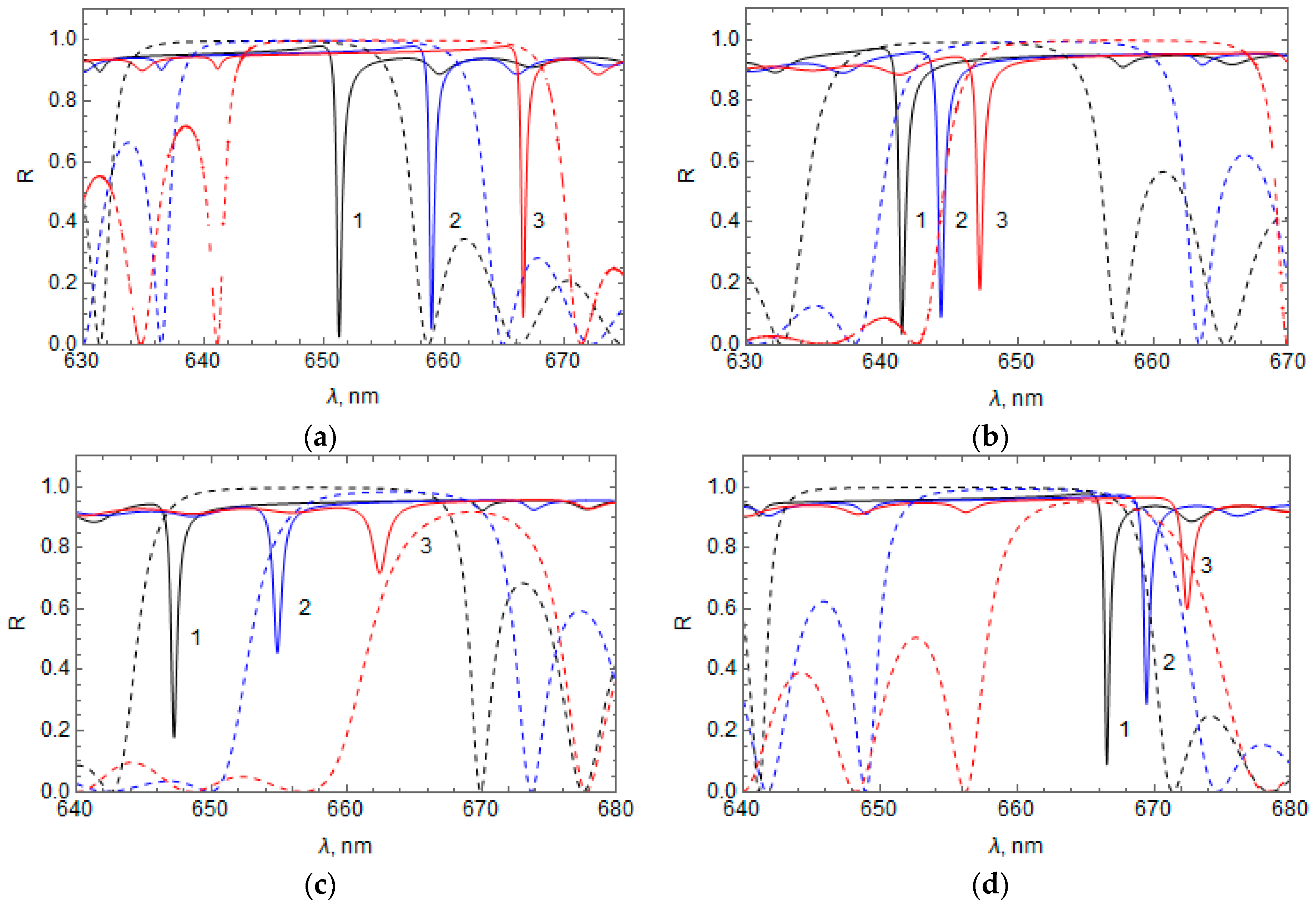
3. Results of Numerical Calculations and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kavokin, A.V.; Shelykh, I.A.; Malpuech, G. Lossless interface modes at the boundary between two periodic dielectric structures. Phys. Rev. B 2005, 72, 233102. [Google Scholar] [CrossRef]
- Vinogradov, A.P.; Dorofeenko, A.V.; Erokhin, S.G.; Inoue, M.; Lisyansky, A.A.; Merzlikin, A.M.; Granovsky, A.B. Surface state peculiarities in one-dimensional photonic crystal interfaces. Phys. Rev. B 2006, 74, 045128. [Google Scholar] [CrossRef]
- Goto, T.; Dorofeenko, A.V.; Merzlikin, A.M.; Baryshev, A.V.; Vinogradov, A.P.; Inoue, M.; Lisyanskii, A.A.; Granovsky, A.B. Optical Tamm states in one-dimensional magnetophotonic structures. Phys. Rev. Lett. 2008, 101, 113902. [Google Scholar] [CrossRef] [PubMed]
- Vetrov, S.Y.; Bikbaev, R.G.; Timofeev, I.V. Optical Tamm states at the interface between a photonic crystal and a nanocomposite with resonance dispersion. J. Exp. Theor. Phys. 2013, 117, 988–998. [Google Scholar] [CrossRef]
- Gaspar-Armenta, A.; Villa, F. Photonic surface-wave excitation: Photonic crystal-metal interface. J. Opt. Soc. Am. B 2003, 20, 2349–2354. [Google Scholar] [CrossRef]
- Kaliteevski, M.; Iorsh, I.; Brand, S.; Abram, R.A.; Chamberlain, J.M.; Kavokin, A.V.; Shelykh, I.A. Tamm plasmon-polaritons: Possible electromagnetic states at the interface of a metal and a dielectric Bragg mirror. Phys. Rev. B 2007, 76, 165415. [Google Scholar] [CrossRef]
- Sasin, M.E.; Seisyan, R.P.; Kalitteevski, M.A.; Brand, S.; Abram, R.A.; Chamberlain, J.M.; Egorov, A.Y.; Vasil’ev, A.P.; Mikhrin, V.S.; Kavokin, A.V. Tamm plasmon polaritons: Slow and spatially compact light. Appl. Phys. Lett. 2008, 92, 251112. [Google Scholar] [CrossRef]
- Afinogenov, B.I.; Bessonov, V.O.; Nikulin, A.A.; Fedyanin, A.A. Observation of hybrid state of Tamm and surface plasmon-polaritons in one-dimensional photonic crystals. Appl. Phys. Lett. 2013, 103, 061112. [Google Scholar] [CrossRef]
- Vyunishev, A.M.; Bikbaev, R.G.; Svyakhovskiy, S.E.; Timofeev, I.V.; Pankin, P.S.; Evlashin, S.A.; Vetrov, S.A.; Myslivets, S.A.; Arkhipkin, V.G. Broadband Tamm plasmon polariton. J. Opt. Soc. Am. B 2019, 36, 2299–2305. [Google Scholar] [CrossRef]
- Reshetnyak, V.Y.; Pinkevych, I.P.; Bunning, T.J.; Evans, D.R. Influence of rugate filters on the spectral manifestation of Tamm plasmon-polaritons. Materials 2021, 14, 1282. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.L.; Wang, F.; Rao, Y.J.; Jiang, Y. Novel sensing concept based on optical Tamm plasmon. Opt. Exp. 2014, 22, 14524. [Google Scholar] [CrossRef]
- Auguié, B.; Fuertes, M.C.; Angelomé, P.C.; Abdala, N.L.; Soler Illia, G.J.A.A.; Fainstein, A. Tamm Plasmon Resonance in Mesoporous Multilayers: Toward a Sensing Application. ACS Photonics 2014, 1, 775–780. [Google Scholar] [CrossRef]
- Kumar, S.; Maji, P.S.; Das, R. Tamm-plasmon resonance based temperature sensor in a Ta2O5/SiO2 based distributed Bragg reflector. Sens. Actuators A 2017, 260, 10–15. [Google Scholar] [CrossRef]
- Balevicius, Z. Strong coupling between Tamm and surface plasmons for advanced optical bio-sensing. Coatings 2020, 10, 1187. [Google Scholar] [CrossRef]
- Zhang, W.L.; Yu, S.F. Bistable switching using an optical Tamm cavity with a Kerr medium. Opt. Commun. 2010, 283, 2622–2626. [Google Scholar] [CrossRef]
- Yang, Z.-Y.; Ishii, S.; Yokoyama, T.; Dao, T.D.; Sun, M.G.; Nagao, T.; Chen, K.-P. Tamm plasmon selective thermal emitters. Opt. Lett. 2016, 41, 4453–4456. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.-Y.; Ishii, S.; Yokoyama, T.; Dao, T.D.; Sun, M.G.; Pankin, P.S.; Timofeev, I.V.; Nagao, T.; Chen, K.-P. Narrowband wavelength selective thermal emitters by confined Tamm plasmon polaritons. ACS Photonics 2017, 4, 2212–2219. [Google Scholar] [CrossRef]
- Cao, T.; Lian, M.; Chen, X.; Mao, L.; Liu, K.; Jia, J.; Su, Y.; Ren, H.; Zhang, S.; Xu, Y.; et al. Multi-cycle reconfigurable THz extraordinary optical transmission using chalcogenide metamaterials. Opto-Electron. Sci. 2022, 1, 210010. Available online: https://www.oejournal.org/article/doi/10.29026/oes.2022.210010 (accessed on 24 August 2021). [CrossRef]
- Lee, B.J.; Fu, C.J.; Zhang, Z.M. Coherent thermal emission from one-dimensional photonic crystals. Appl. Phys. Lett. 2005, 87, 071904. [Google Scholar] [CrossRef]
- Gazzano, O.; Vasconcellos, S.M.; Gauthron, K.; Symonds, C. Single photon source using confined Tamm plasmon modes. Appl. Phys. Lett. 2012, 100, 232111. [Google Scholar] [CrossRef]
- Jiménez-Solano, A.; Galisteo-López, J.F.; Míguez, H. Flexible and adaptable light-emitting coatings for arbitrary metal surfaces based on optical Tamm mode coupling. Adv. Opt. Mater. 2018, 6, 1700560. [Google Scholar] [CrossRef]
- Symonds, G.; Lemaître, A.; Senellart, P.; Jomaa, M.H.; Aberra Guebrou, S.; Homeyer, E.; Brucoli, G.; Bellessa, J. Lasing in a hybrid GaAs/silver Tamm structure. Appl. Phys. Lett. 2012, 100, 121122. [Google Scholar] [CrossRef]
- Symonds, C.; Lheureux, G.; Hugonin, J.P.; Greffet, J.J.; Laverdant, J.; Brucoli, G.; Lemaitre, A.; Senellart, P.; Bellessa, J. Confined Tamm plasmon lasers. Nano Lett. 2013, 13, 3179–3184. [Google Scholar] [CrossRef]
- Cheng, H.-C.; Kuo, C.-Y.; Hung, Y.-J.; Chen, K.-P.; Jeng, S.-C. Liquid-crystal active Tamm-plasmon devices. Phys. Rev. Appl. 2018, 9, 064034. [Google Scholar] [CrossRef]
- Buchnev, O.; Belosludtsev, A.; Reshetnyak, V.; Evans, D.R.; Fedotov, V.A. Observing and controlling a Tamm plasmon at the interface with a metasurface. Nanophotonics 2020, 9, 897–903. [Google Scholar] [CrossRef]
- Park, J.; Won, K.; Kim, J. Liquid crystal between two distributed Bragg reflectors enables multispectral small-pitch spatial light modulator. Light Sci. Appl. 2022, 11, 210. [Google Scholar] [CrossRef] [PubMed]
- De Gennes, P.G.; Prost, J. The Physics of Liquid Crystals; Clarendon: Oxford, UK, 1993; Chapter 6. [Google Scholar]
- Vetrov, S.Y.; Pyatnov, M.V.; Timofeev, I.V. Spectral and polarization properties of a ‘cholesteric liquid crystal-phase film-metal’ structure. J. Opt. 2016, 18, 015103. [Google Scholar] [CrossRef]
- Pyatnov, M.V.; Vetrov, S.Y.; Timofeev, I.V. Localised optical states in a structure formed by two oppositely handed cholesteric liquid crystal layers and a metal. Liq. Cryst. 2017, 44, 674–678. [Google Scholar] [CrossRef]
- Reshetnyak, V.Y.; Pinkevych, I.P.; Bunning, T.J.; McConney, M.E.; Evans, D.R. Spectral manifestation of optical Tamm states in a metal-cholesteric liquid crystals stack. Phys. Rev. E 2023, 107, 014702. [Google Scholar] [CrossRef] [PubMed]
- Bunning, T.; Natarajan, L.; Tondiglia, V.; Sutherland, R. Holographic polymer dispersed liquid crystals (H-PDLCs). Annu. Rev. Mater. Sci. 2000, 30, 83–115. [Google Scholar] [CrossRef]
- Caputo, R.; De Sio, L.; Veltri, A.; Umeton, C.P. Development of a new kind of switchable holographic grating made of liquid-crystal films separated by slices of polymeric material. Opt. Lett. 2004, 29, 1261–1263. [Google Scholar] [CrossRef]
- Holmstrom, S.A.; Natarajan, L.V.; Tondiglia, V.P.; Sutherland, R.L. Mechanical tuning of holographic polymer-dispersed liquid crystal reflection gratings. Appl. Phys. Lett. 2004, 85, 1949–1951. [Google Scholar] [CrossRef]
- Lucchetta, D.E.; Criante, L.; Simoni, F. Optical characterization of polymer dispersed liquid crystals for holographic recording. J. Appl. Phys. 2003, 93, 9669–9674. [Google Scholar] [CrossRef]
- Kato, K.; Hisaki, T.; Date, M. Alignment-controlled holographic polymer dispersed liquid crystal for reflective display devices. Jpn. J. Appl. Phys. 1999, 38, 805. [Google Scholar] [CrossRef]
- Urbas, A.M.; Tondiglia, V.P.; Natarajan, L.V. Sutherland, Optically switchable liquid crystal photonic structures. J. Am. Chem. Soc. 2004, 126, 13580–13581. [Google Scholar] [CrossRef]
- Lee, K.M.; Tondiglia, V.P.; Godman, N.P.; White, T.J.; Bunning, T.J.; McConney, M.E. Reconfigurable reflective colors in holographically patterned liquid crystal gels. ACS Photonics 2020, 7, 1978–1982. [Google Scholar] [CrossRef]
- Lee, K.M.; Reshetnyak, V.Y.; Ambuloab, C.P.; Marshab, Z.M.; McConney, M.E.; Godman, N.P. Anti-counterfeiting holographic liquid crystal gels with color and pattern control. Mater. Adv. 2023, 4, 2418–2424. [Google Scholar] [CrossRef]
- Reshetnyak, V.Y.; Pinkevych, I.P.; Godman, N.P.; Bunning, T.J.; Evans, D.R. Optical Tamm states in a hybrid structure with a holographic polymer-liquid crystal grating. Liq. Cryst. 2023, 50, 45–53. [Google Scholar] [CrossRef]
- Zel’dovich, B.Y.; Tabiryan, N.V.; Chilingaryan, Y.S. Fredericks transitions induced by light fields. Sov. Phys. JETP 1981, 54, 32–37. [Google Scholar]
- Yariv, A.; Yeh, P. Optical Waves in Crystals. Propagation and Control of Laser Radiation; Wiley: New York, NY, USA, 1984; pp. 155–219. [Google Scholar]
- Kogelnik, H. Coupled wave theory for thick hologram gratings. Bell Syst. Tech. J. 1969, 48, 2909–2947. [Google Scholar] [CrossRef]
- Rioux, D.; Vallières, S.; Besner, S.; Muñoz, P.; Mazur, E.; Meunier, M. An analytic model for the dielectric function of Au, Ag, and their alloys. Adv. Opt. Mater. 2014, 2, 176–182. [Google Scholar] [CrossRef]
- Tkachenko, V.; Abbate, G.; Marino, A.; Vita, F. Nematic liquid crystal optical dispersion in the visible-near infrared range. Mol. Cryst. Liq. Cryst. 2006, 454, 263–271. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reshetnyak, V.Y.; Pinkevych, I.P.; Bunning, T.J.; Evans, D.R. Excitation and Tuning of Optical Tamm States in a Hybrid Structure with a Metal Film Adjacent to a Four-Layer Polymer–Liquid Crystal Stack. Photonics 2024, 11, 278. https://doi.org/10.3390/photonics11030278
Reshetnyak VY, Pinkevych IP, Bunning TJ, Evans DR. Excitation and Tuning of Optical Tamm States in a Hybrid Structure with a Metal Film Adjacent to a Four-Layer Polymer–Liquid Crystal Stack. Photonics. 2024; 11(3):278. https://doi.org/10.3390/photonics11030278
Chicago/Turabian StyleReshetnyak, Victor Y., Igor P. Pinkevych, Timothy J. Bunning, and Dean R. Evans. 2024. "Excitation and Tuning of Optical Tamm States in a Hybrid Structure with a Metal Film Adjacent to a Four-Layer Polymer–Liquid Crystal Stack" Photonics 11, no. 3: 278. https://doi.org/10.3390/photonics11030278
APA StyleReshetnyak, V. Y., Pinkevych, I. P., Bunning, T. J., & Evans, D. R. (2024). Excitation and Tuning of Optical Tamm States in a Hybrid Structure with a Metal Film Adjacent to a Four-Layer Polymer–Liquid Crystal Stack. Photonics, 11(3), 278. https://doi.org/10.3390/photonics11030278





