Crosstalk-Free Multiplane Full-Color Holography Based on the Space Division Method
Abstract
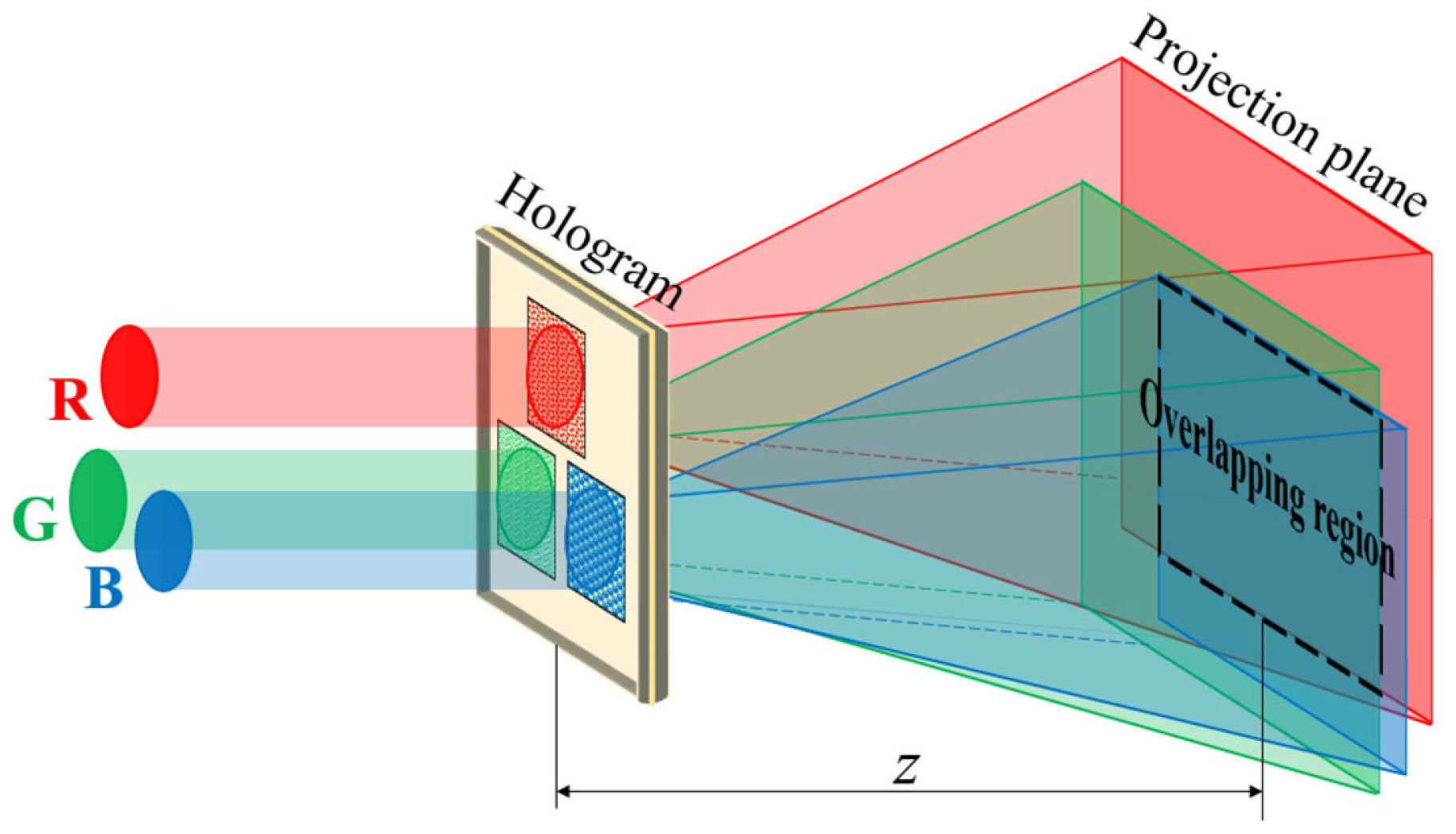
1. Introduction
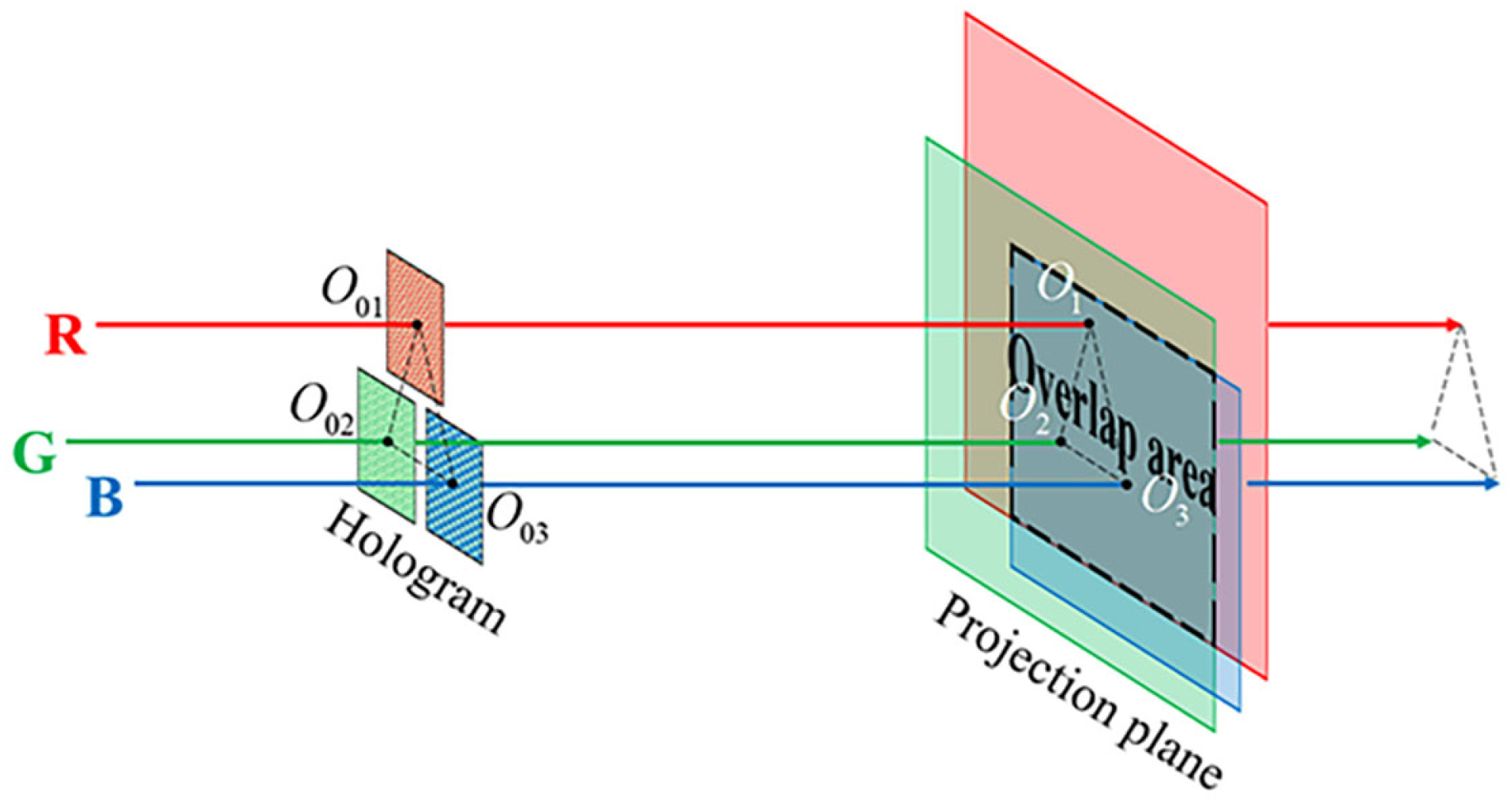
2. Principle of Color Image Reproduction
3. Design Method
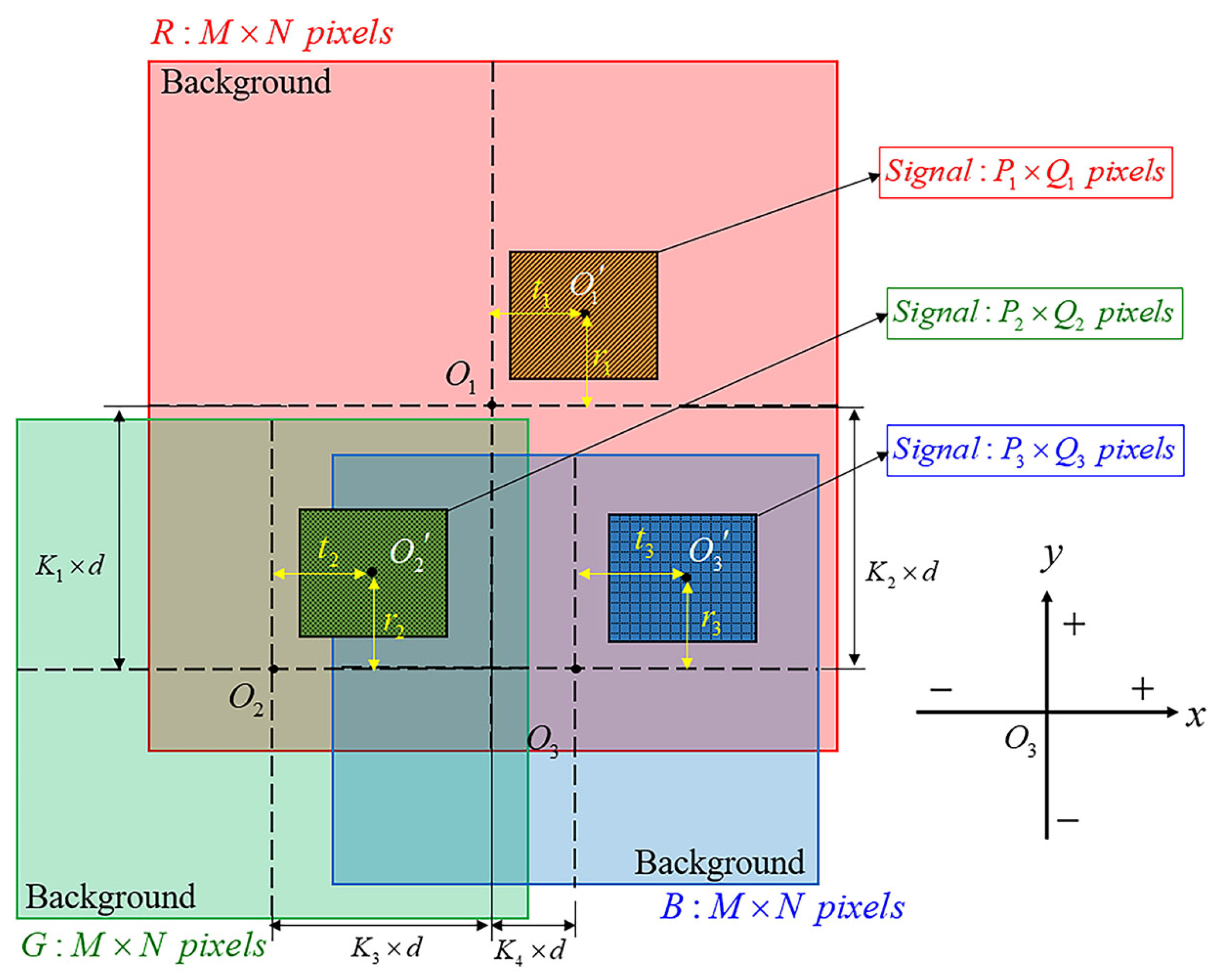
3.1. Image Dimension Matching
3.2. Image Position Matching
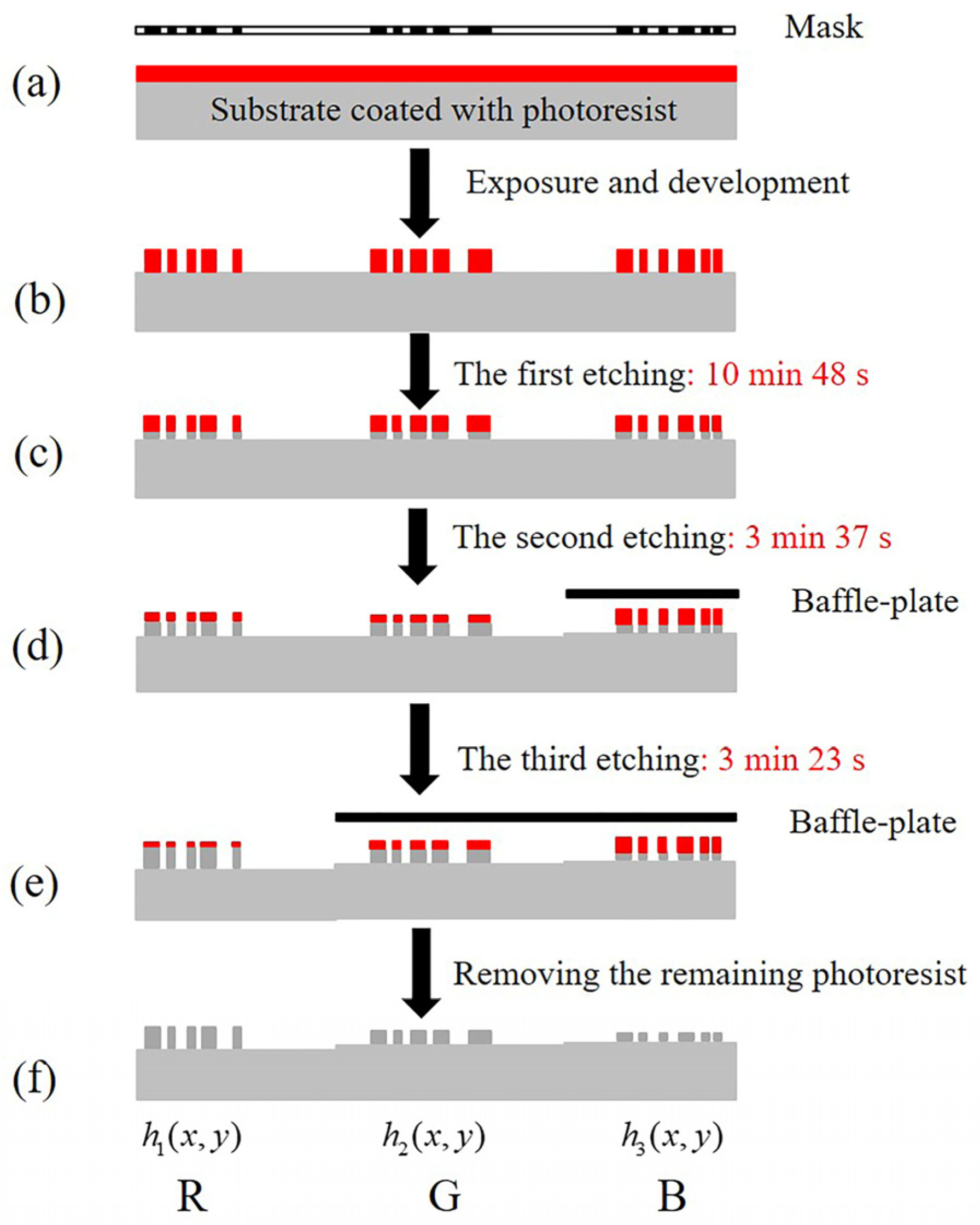
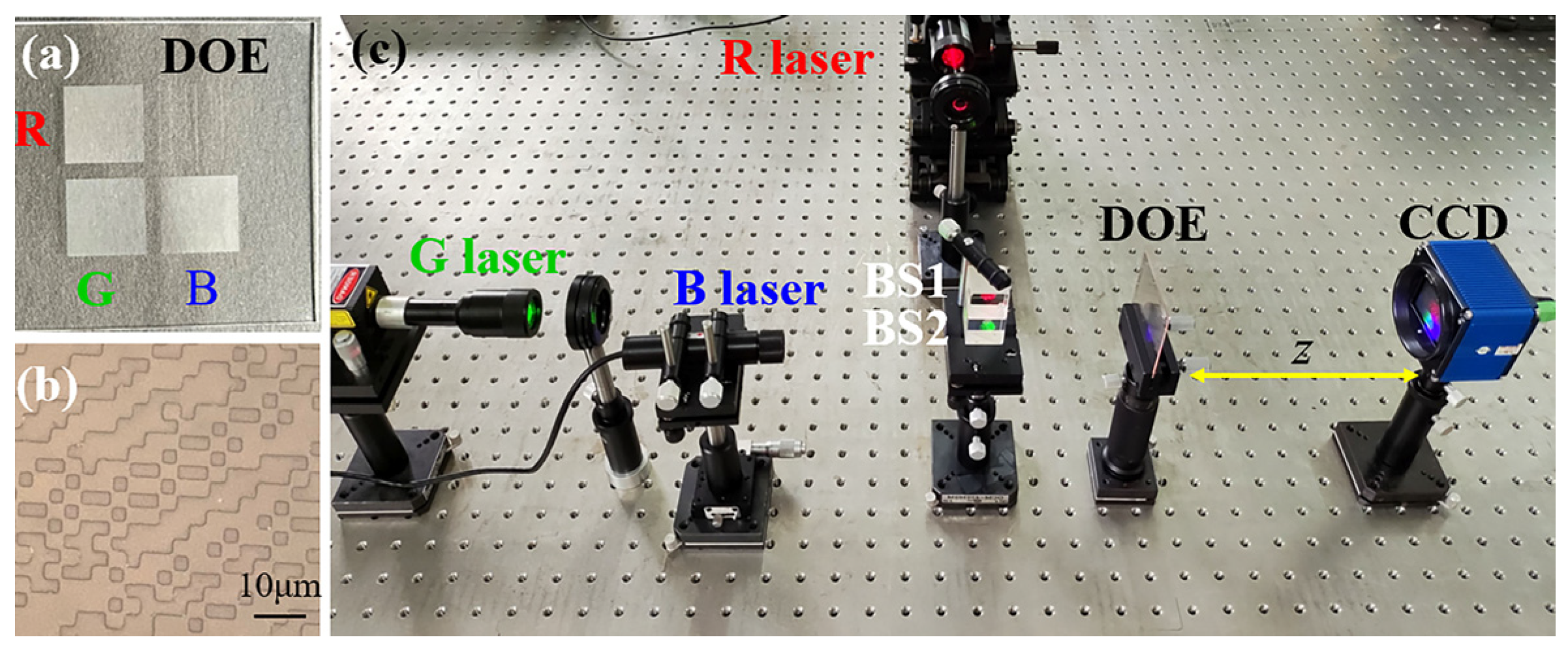
4. Simulation and Experimental Verification
4.1. Single Plane Color Image Reconstruction
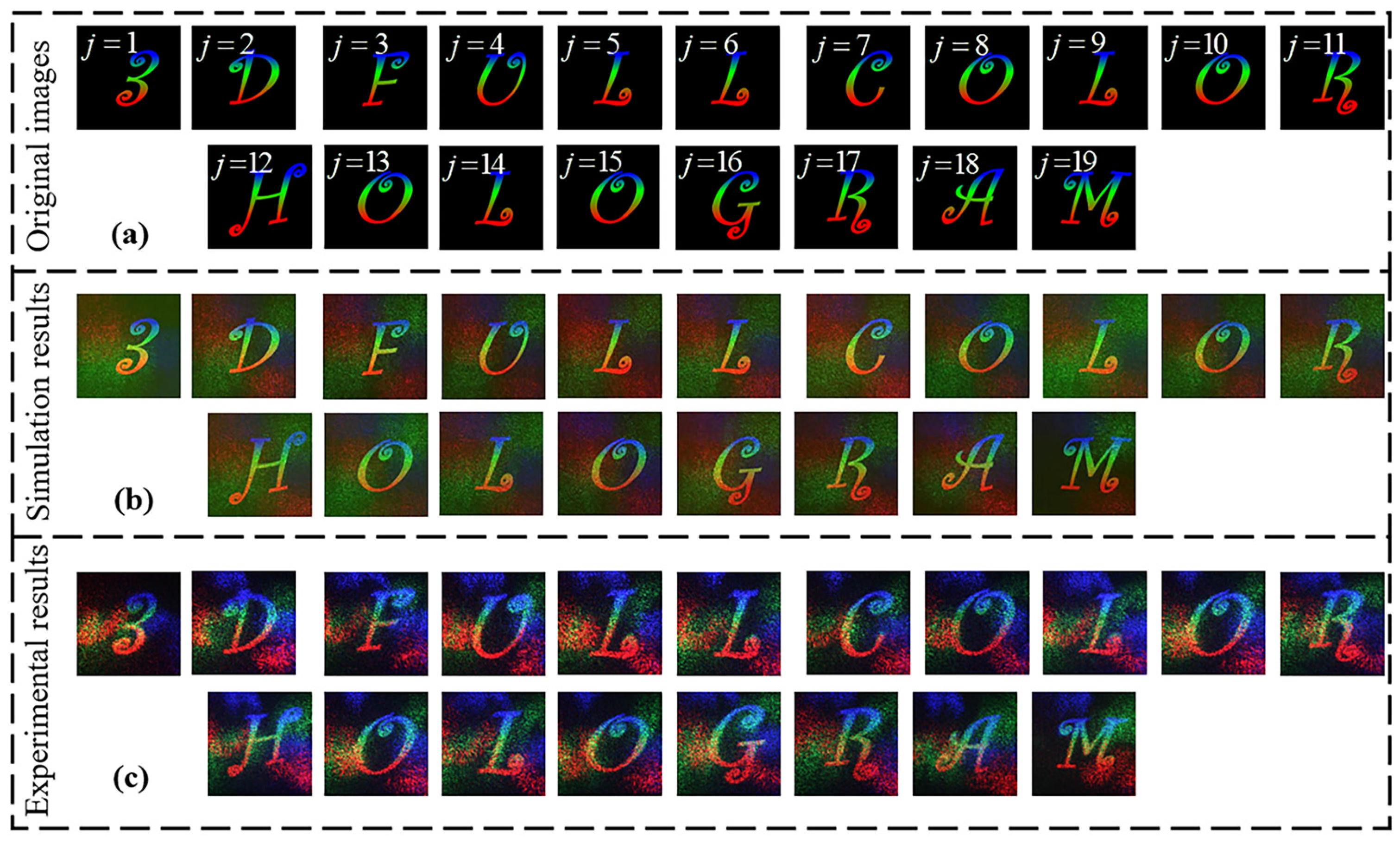
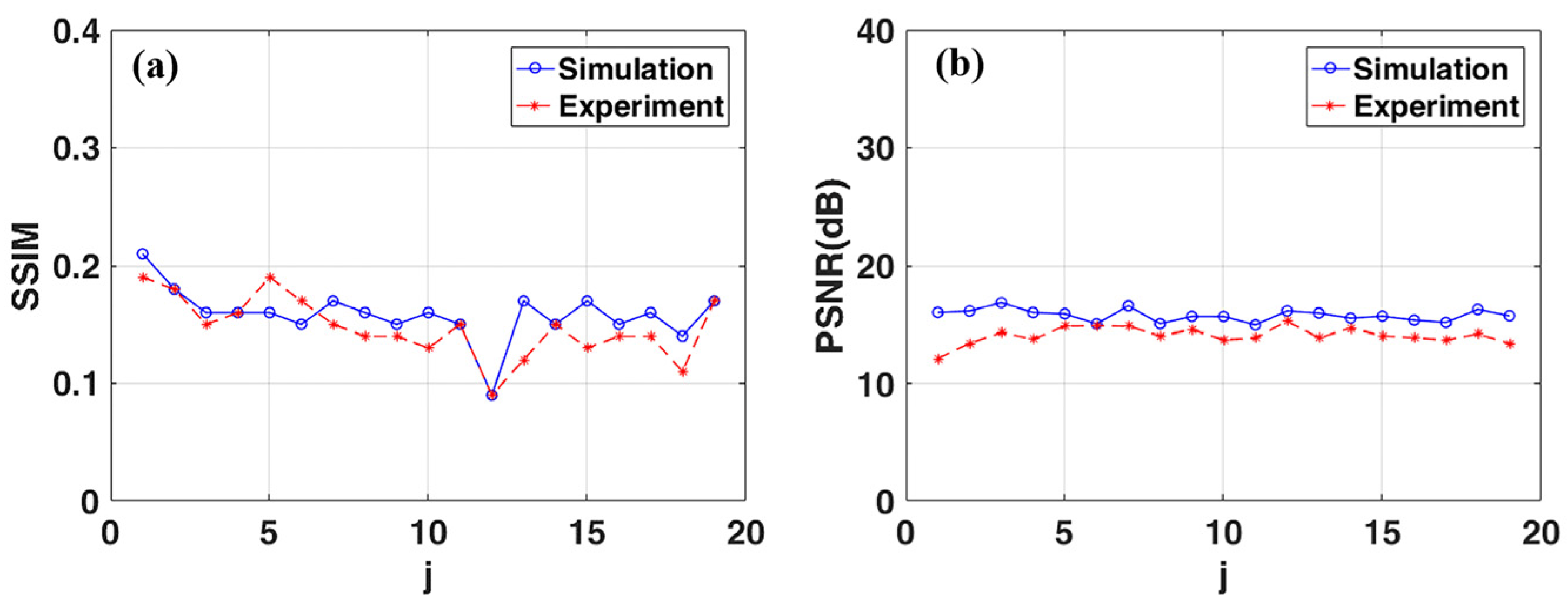
4.2. Multiplane Color Image Reconstruction
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bruder, F.-K.; Fäcke, T.; Rölle, T. Thermal Fringe Formation during a Hologram Recording Using a Dry Photopolymer. Photonics 2021, 8, 589. [Google Scholar] [CrossRef]
- Shin, C.-W.; Wu, H.-Y.; Kwon, K.-C.; Piao, Y.-L.; Lee, K.-Y.; Gil, S.-K.; Kim, N. Diffraction Efficiency Enhancement and Optimization in Full-Color HOE Using the Inhibition Characteristics of the Photopolymer. Opt. Express 2021, 29, 1175. [Google Scholar] [CrossRef]
- Wang, W.; Wang, X.; Xu, B.; Chen, J. Optical Image Encryption and Authentication Using Phase-Only Computer-Generated Hologram. Opt. Lasers Eng. 2021, 146, 106722. [Google Scholar] [CrossRef]
- Mu, C.-T.; Chen, C.-H. Diffractive Distortion of a Pixelated Computer-Generated Hologram with Oblique Illumination. Appl. Opt. 2020, 59, 7153. [Google Scholar] [CrossRef]
- Bai, Y.; Zhang, Z.; Chen, T.; Wang, R.; Wei, H.; Zeng, X.; Zhang, X. Evaluation of Wavefront Aberrations Induced by Overlay Errors in Stitching Computer-Generated Holograms. Opt. Lasers Eng. 2022, 152, 106944. [Google Scholar] [CrossRef]
- Sifatul Islam, M.; Piao, Y.-L.; Zhao, Y.; Kwon, K.-C.; Cho, E.; Kim, N. Max-Depth-Range Technique for Faster Full-Color Hologram Generation. Appl. Opt. 2020, 59, 3156. [Google Scholar] [CrossRef] [PubMed]
- Yamada, S.; Shimobaba, T.; Kakue, T.; Ito, T. Full-Color Computer-Generated Hologram Using Wavelet Transform and Color Space Conversion. Opt. Express 2019, 27, 8153. [Google Scholar] [CrossRef] [PubMed]
- Park, S.; Lee, J.; Lim, S.; Kim, M.; Ahn, S.; Hwang, S.; Jeon, S.; Jeong, J.; Hahn, J.; Kim, H. Wide-Viewing Full-Color Depthmap Computer-Generated Holograms. Opt. Express 2021, 29, 26793. [Google Scholar] [CrossRef] [PubMed]
- Martinez-Carranza, J.; Kozacki, T.; Kukołowicz, R.; Chlipala, M.; Idicula, M.S. Occlusion Culling for Wide-Angle Computer-Generated Holograms Using Phase Added Stereogram Technique. Photonics 2021, 8, 298. [Google Scholar] [CrossRef]
- Zheng, H.; Zhou, C.; Shui, X.; Yu, Y. Computer-Generated Full-Color Phase-Only Hologram Using a Multiplane Iterative Algorithm with Dynamic Compensation. Appl. Opt. 2022, 61, B262. [Google Scholar] [CrossRef]
- Sakamoto, Y.; Oshinomi, T.; Matsuno, K. Method of Calculating Speckle-Reduced Hologram Data Using a Convergence Light Wave for a Computer-Generated Hologram. Appl. Opt. 2023, 62, 1970. [Google Scholar] [CrossRef] [PubMed]
- Sando, Y.; Itoh, M.; Yatagai, T. Full-Color Computer-Generated Holograms Using 3-D Fourier Spectra. Opt. Express 2004, 12, 6246. [Google Scholar] [CrossRef]
- Nakayama, H.; Takada, N.; Ichihashi, Y.; Awazu, S.; Shimobaba, T.; Masuda, N.; Ito, T. Real-Time Color Electroholography Using Multiple Graphics Processing Units and Multiple High-Definition Liquid-Crystal Display Panels. Appl. Opt. 2010, 49, 5993. [Google Scholar] [CrossRef]
- Shimobaba, T.; Ito, T. A Color Holographic Reconstruction System by Time Division Multiplexing with Reference Lights of Laser. Opt. Rev. 2003, 10, 339–341. [Google Scholar] [CrossRef]
- Zhang, Z.; Chen, S.; Zheng, H.; Zeng, Z.; Gao, H.; Yu, Y.; Asundi, A.K. Full-Color Holographic 3D Display Using Slice-Based Fractional Fourier Transform Combined with Free-Space Fresnel Diffraction. Appl. Opt. 2017, 56, 5668. [Google Scholar] [CrossRef]
- Makowski, M.; Sypek, M.; Kolodziejczyk, A. Colorful Reconstructions from a Thin Multi-Plane Phase Hologram. Opt. Express 2008, 16, 11618. [Google Scholar] [CrossRef] [PubMed]
- Deng, Z.; Jin, M.; Ye, X.; Wang, S.; Shi, T.; Deng, J.; Mao, N.; Cao, Y.; Guan, B.; Alù, A.; et al. Full-Color Complex-Amplitude Vectorial Holograms Based on Multi-Freedom Metasurfaces. Adv. Funct. Mater. 2020, 30, 1910610. [Google Scholar] [CrossRef]
- Pang, Y.; Cao, A.; Wang, J.; Pang, H.; Deng, Q. Simple Encoding Method of Phase-Only Hologram for Low Crosstalk Full-Color Multi-Plane Holographic Projection. Opt. Lasers Eng. 2021, 147, 106748. [Google Scholar] [CrossRef]
- Shimobaba, T.; Takahashi, T.; Masuda, N.; Ito, T. Numerical Study of Color Holographic Projection Using Space-Division Method. Opt. Express 2011, 19, 10287. [Google Scholar] [CrossRef]
- Ito, T.; Okano, K. Color Electroholography by Three Colored Reference Lights Simultaneously Incident upon One Hologram Panel. Opt. Express 2004, 12, 4320. [Google Scholar] [CrossRef]
- Makowski, M.; Ducin, I.; Kakarenko, K.; Suszek, J.; Sypek, M.; Kolodziejczyk, A. Simple Holographic Projection in Color. Opt. Express 2012, 20, 25130. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Liu, L.; Cao, A.; Pang, H.; Xu, C.; Mu, Q.; Chen, J.; Shi, L.; Deng, Q. Generation of Color Images by Utilizing a Single Composite Diffractive Optical Element. Micromachines 2018, 9, 508. [Google Scholar] [CrossRef] [PubMed]
- Makowski, M.; Ducin, I.; Sypek, M.; Siemion, A.; Siemion, A.; Suszek, J.; Kolodziejczyk, A. Color Image Projection Based on Fourier Holograms. Opt. Lett. 2010, 35, 1227. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.; Seong, J.; Yang, Y.; Moon, S.-W.; Badloe, T.; Rho, J. Tunable Metasurfaces towards Versatile Metalenses and Metaholograms: A Review. Adv. Photonics 2022, 4, 024001. [Google Scholar] [CrossRef]
- Su, D.; Wang, X.; Shang, G.; Ding, X.; Burokur, S.N.; Liu, J.; Li, H. Amplitude-Phase Modulation Metasurface Hologram with Inverse Angular Spectrum Diffraction Theory. J. Phys. Appl. Phys. 2022, 55, 235102. [Google Scholar] [CrossRef]
- Kim, N.; Ee, H.-S. Multi-Color Metasurface Hologram Based on Depth-Division Multiplexing Method. J. Korean Phys. Soc. 2023, 82, 166–172. [Google Scholar] [CrossRef]
- Li, X.; Chen, Q.; Zhang, X.; Zhao, R.; Xiao, S.; Wang, Y.; Huang, L. Time-Sequential Color Code Division Multiplexing Holographic Display with Metasurface. Opto-Electron. Adv. 2023, 6, 220060. [Google Scholar] [CrossRef]
- Wan, W.; Yang, W.; Ye, S.; Jiang, X.; Han, Y.; Feng, H.; Liu, Y.; Gong, Q.; Xiao, S.; Li, Y. Tunable Full-Color Vectorial Meta-Holography. Adv. Opt. Mater. 2022, 10, 2201478. [Google Scholar] [CrossRef]
- Pang, Y.; Wu, X.; Pang, H.; Liu, L.; Xue, L.; Liu, W.; Shi, L.; Cao, A.; Deng, Q. Error Tracking-Control-Reduction Algorithm for Designing Diffractive Optical Element with High Image Reconstruction Quality. Opt. Express 2020, 28, 10090. [Google Scholar] [CrossRef]
- Makey, G.; Yavuz, Ö.; Kesim, D.K.; Turnalı, A.; Elahi, P.; Ilday, S.; Tokel, O.; Ilday, F.Ö. Breaking Crosstalk Limits to Dynamic Holography Using Orthogonality of High-Dimensional Random Vectors. Nat. Photonics 2019, 13, 251–256. [Google Scholar] [CrossRef]


| Plane | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 140 | 147.1 | 154.9 | 163.6 | 173.4 | 184.3 | 196.8 | 211.1 | 227.6 | 246.9 | |
| −2250 | −2141 | −2033 | −1925 | −1817 | −1708 | −1600 | −1492 | −1384 | −1275 | |
| 2250 | 2141 | 2033 | 1925 | 1817 | 1708 | 1600 | 1492 | 1384 | 1275 | |
| 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | ||
| 269.8 | 297.3 | 331.2 | 373.7 | 428.7 | 502.8 | 607.8 | 768.2 | 1043.8 | ||
| −1167 | −1059 | −951 | −842 | −734 | −626 | −518 | −410 | −301 | ||
| 1167 | 1059 | 951 | 842 | 734 | 626 | 518 | 410 | 301 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, A.; Pang, Y.; Deng, Q. Crosstalk-Free Multiplane Full-Color Holography Based on the Space Division Method. Photonics 2024, 11, 230. https://doi.org/10.3390/photonics11030230
Cao A, Pang Y, Deng Q. Crosstalk-Free Multiplane Full-Color Holography Based on the Space Division Method. Photonics. 2024; 11(3):230. https://doi.org/10.3390/photonics11030230
Chicago/Turabian StyleCao, Axiu, Yingfei Pang, and Qiling Deng. 2024. "Crosstalk-Free Multiplane Full-Color Holography Based on the Space Division Method" Photonics 11, no. 3: 230. https://doi.org/10.3390/photonics11030230
APA StyleCao, A., Pang, Y., & Deng, Q. (2024). Crosstalk-Free Multiplane Full-Color Holography Based on the Space Division Method. Photonics, 11(3), 230. https://doi.org/10.3390/photonics11030230





