Bandwidth-Tunable Optical Amplifier with Narrowband Filtering Function Enabled by Parity-Time Symmetry at Exceptional Points
Abstract
1. Introduction
2. Model and Principle
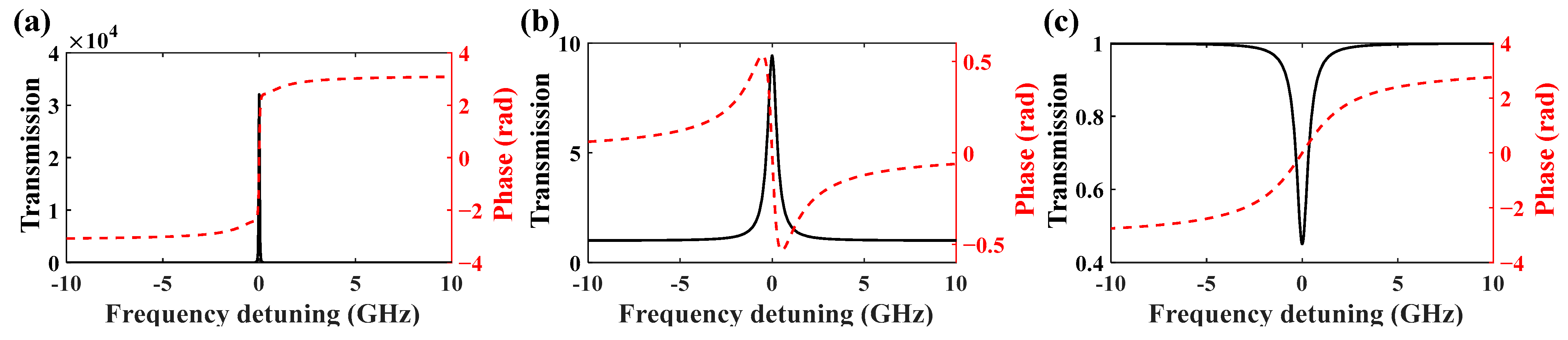
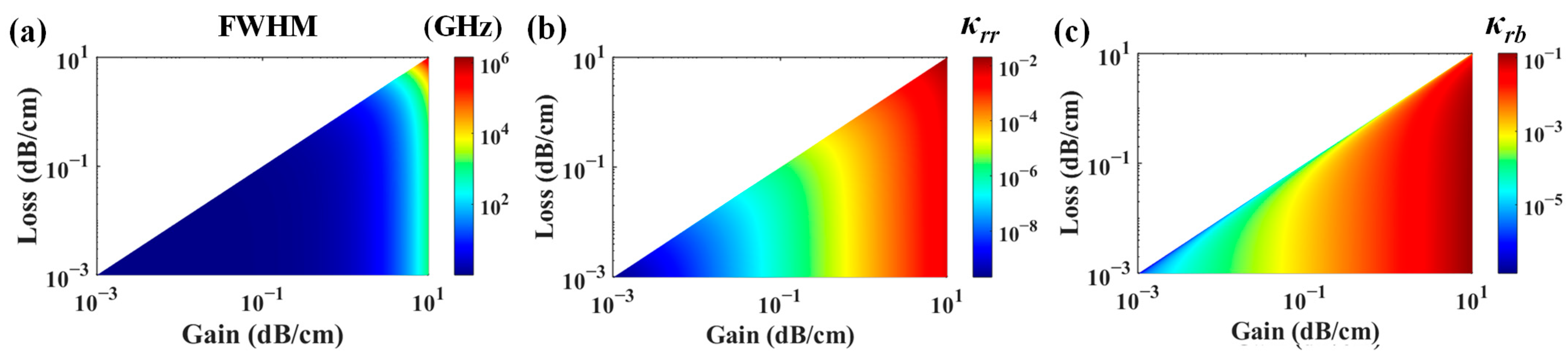
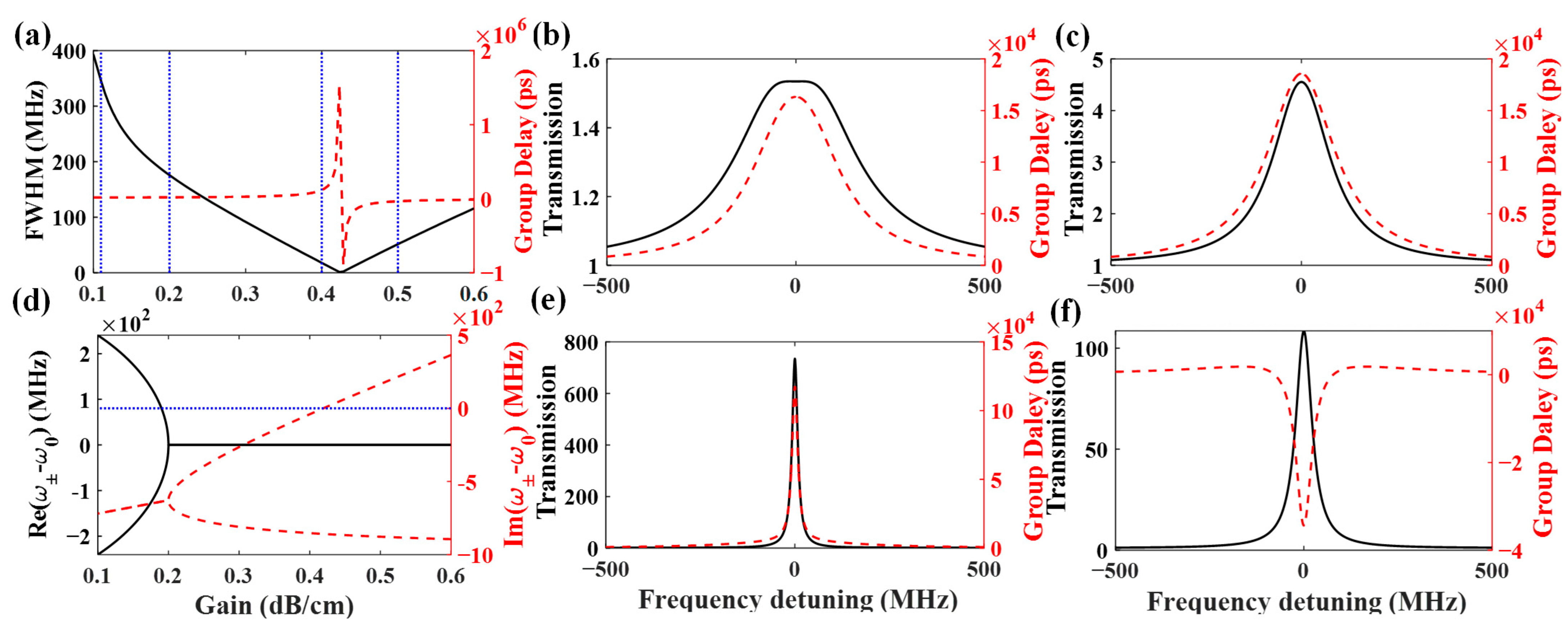
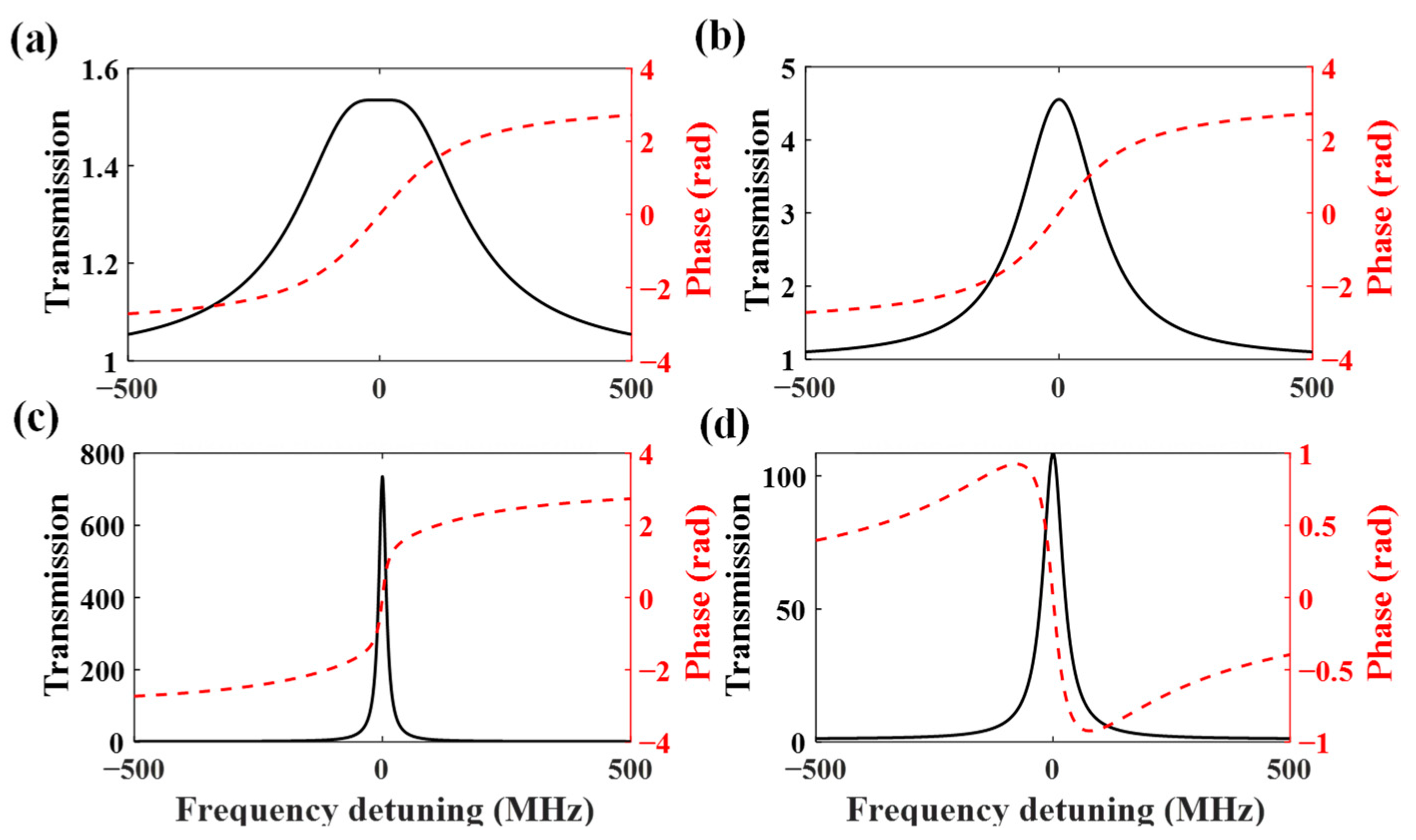
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bender, C.M.; Boettcher, S. Real Spectra in Non-Hermitian Hamiltonians Having P T Symmetry. Phys. Rev. Lett. 1998, 80, 5243–5246. [Google Scholar] [CrossRef]
- Özdemir, Ş.K.; Rotter, S.; Nori, F.; Yang, L. Parity–Time Symmetry and Exceptional Points in Photonics. Nat. Mater. 2019, 18, 783–798. [Google Scholar] [CrossRef] [PubMed]
- Feng, L.; El-Ganainy, R.; Ge, L. Non-Hermitian Photonics Based on Parity–Time Symmetry. Nat. Photonics 2017, 11, 752–762. [Google Scholar] [CrossRef]
- Moiseyev, N. Non-Hermitian Quantum Mechanics; Cambridge University Press: Cambridge, UK, 2011; ISBN 978-0-521-88972-8. [Google Scholar]
- El-Ganainy, R.; Makris, K.G.; Khajavikhan, M.; Musslimani, Z.H.; Rotter, S.; Christodoulides, D.N. Non-Hermitian Physics and PT Symmetry. Nat. Phys. 2018, 14, 11–19. [Google Scholar] [CrossRef]
- Hodaei, H.; Miri, M.-A.; Heinrich, M.; Christodoulides, D.N.; Khajavikhan, M. Parity-Time–Symmetric Microring Lasers. Science 2014, 346, 975–978. [Google Scholar] [CrossRef]
- Peng, B.; Özdemir, Ş.K.; Lei, F.; Monifi, F.; Gianfreda, M.; Long, G.L.; Fan, S.; Nori, F.; Bender, C.M.; Yang, L. Parity–Time-Symmetric Whispering-Gallery Microcavities. Nat. Phys. 2014, 10, 394–398. [Google Scholar] [CrossRef]
- Chang, L.; Jiang, X.; Hua, S.; Yang, C.; Wen, J.; Jiang, L.; Li, G.; Wang, G.; Xiao, M. Parity–Time Symmetry and Variable Optical Isolation in Active–Passive-Coupled Microresonators. Nat. Photonics 2014, 8, 524–529. [Google Scholar] [CrossRef]
- Feng, L.; Wong, Z.J.; Ma, R.-M.; Wang, Y.; Zhang, X. Single-Mode Laser by Parity-Time Symmetry Breaking. Science 2014, 346, 972–975. [Google Scholar] [CrossRef]
- Hodaei, H.; Miri, M.; Hassan, A.U.; Hayenga, W.E.; Heinrich, M.; Christodoulides, D.N.; Khajavikhan, M. Single Mode Lasing in Transversely Multi-moded PT-symmetric Microring Resonators. Laser Photonics Rev. 2016, 10, 494–499. [Google Scholar] [CrossRef]
- Hodaei, H.; Hassan, A.U.; Wittek, S.; Garcia-Gracia, H.; El-Ganainy, R.; Christodoulides, D.N.; Khajavikhan, M. Enhanced Sensitivity at Higher-Order Exceptional Points. Nature 2017, 548, 187–191. [Google Scholar] [CrossRef] [PubMed]
- Ren, J.; Hodaei, H.; Harari, G.; Hassan, A.U.; Chow, W.; Soltani, M.; Christodoulides, D.; Khajavikhan, M. Ultrasensitive Micro-Scale Parity-Time-Symmetric Ring Laser Gyroscope. Opt. Lett. 2017, 42, 1556–1559. [Google Scholar] [CrossRef]
- Lai, Y.-H.; Lu, Y.-K.; Suh, M.-G.; Yuan, Z.; Vahala, K. Observation of the Exceptional-Point-Enhanced Sagnac Effect. Nature 2019, 576, 65–69. [Google Scholar] [CrossRef] [PubMed]
- Hokmabadi, M.P.; Schumer, A.; Christodoulides, D.N.; Khajavikhan, M. Non-Hermitian Ring Laser Gyroscopes with Enhanced Sagnac Sensitivity. Nature 2019, 576, 70–74. [Google Scholar] [CrossRef] [PubMed]
- Chen, W.; Kaya Özdemir, Ş.; Zhao, G.; Wiersig, J.; Yang, L. Exceptional Points Enhance Sensing in an Optical Microcavity. Nature 2017, 548, 192–196. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, X.; Zhang, Q.; Chen, Y.; Wang, Y.; Yu, Y.; Yang, Y.; Wang, T. Dominated Mode Switching and Nanoparticle Detection at Exceptional Points. J. Opt. Soc. Am. B 2023, 40, 108. [Google Scholar] [CrossRef]
- Li, J.; Tang, M.; Duan, J.; Xu, X.; Xu, K.; Ma, L.; Wang, J. Exceptional Points in a Spiral Ring Cavity for Enhanced Biosensing. J. Light. Technol. 2023, 41, 2870–2878. [Google Scholar] [CrossRef]
- Wang, C.; Sweeney, W.R.; Stone, A.D.; Yang, L. Coherent Perfect Absorption at an Exceptional Point. Science 2021, 373, 1261–1265. [Google Scholar] [CrossRef] [PubMed]
- Zhang, B.; Chen, N.; Lu, X.; Hu, Y.; Yang, Z.; Zhang, X.; Xu, J. Bandwidth Tunable Optical Bandpass Filter Based on Parity-Time Symmetry. Micromachines 2022, 13, 89. [Google Scholar] [CrossRef] [PubMed]
- Lu, X.; Chen, N.; Zhang, B.; Yang, H.; Chen, Y.; Zhang, X.; Xu, J. Parity-Time Symmetry Enabled Band-Pass Filter Featuring High Bandwidth-Tunable Contrast Ratio. Photonics 2022, 9, 380. [Google Scholar] [CrossRef]
- Vahala, K. Optical Microcavities; Advanced Series in Applied Physics; World Scientific: Hackensack, NJ, USA; Singapore, 2007; ISBN 978-981-238-775-2. [Google Scholar]
- Yu, B.; Chen, Y.; Pan, J.; Zhang, B.; Li, F.; Wan, L.; Guo, X.; Li, J.; Li, Z. Silica-Microsphere-Cavity-Based Microwave Photonic Notch Filter with Ultra-Narrow Bandwidth and High Peak Rejection. Opt. Lett. 2019, 44, 1411. [Google Scholar] [CrossRef]
- Liu, L.; Liao, S. Si3 N4-Based Narrowband and High Peak Rejection Microwave Photonic Filter with Adjustable Bandwidth. J. Light. Technol. 2024, 42, 1580–1585. [Google Scholar] [CrossRef]
- Little, B.E.; Chu, S.T.; Absil, P.P.; Hryniewicz, J.V.; Johnson, F.G.; Seiferth, F.; Gill, D.; Van, V.; King, O.; Trakalo, M. Very High-Order Microring Resonator Filters for WDM Applications. IEEE Photon. Technol. Lett. 2004, 16, 2263–2265. [Google Scholar] [CrossRef]
- Popovíc, M.A.; Barwicz, T.; Watts, M.R.; Rakich, P.T.; Socci, L.; Ippen, E.P.; Kärtner, F.X.; Smith, H.I. Multistage High-Order Microring-Resonator Add-Drop Filters. Opt. Lett. 2006, 31, 2571. [Google Scholar] [CrossRef] [PubMed]
- Liu, D.; Xu, H.; Tan, Y.; Shi, Y.; Dai, D. Silicon Photonic Filters. Micro Opt. Tech. Lett. 2021, 63, 2252–2268. [Google Scholar] [CrossRef]
- Shi, W.; Wang, X.; Zhang, W.; Yun, H.; Lin, C.; Chrostowski, L.; Jaeger, N.A.F. Grating-Coupled Silicon Microring Resonators. Appl. Phys. Lett. 2012, 100, 121118. [Google Scholar] [CrossRef]
- Liu, D.; Zhang, C.; Liang, D.; Dai, D. Submicron-Resonator-Based Add-Drop Optical Filter with an Ultra-Large Free Spectral Range. Opt. Express 2019, 27, 416. [Google Scholar] [CrossRef] [PubMed]
- Ong, J.R.; Kumar, R.; Mookherjea, S. Ultra-High-Contrast and Tunable-Bandwidth Filter Using Cascaded High-Order Silicon Microring Filters. IEEE Photon. Technol. Lett. 2013, 25, 1543–1546. [Google Scholar] [CrossRef]
- Haus, H.A. Waves and Fields in Optoelectronics; Prentice-Hall Series in Solid State Physical Electronics; Prentice-Hall: Englewood Cliffs, NJ, USA, 1984; ISBN 978-0-13-946053-1. [Google Scholar]
- Little, B.E.; Chu, S.T.; Haus, H.A.; Foresi, J.; Laine, J.-P. Microring Resonator Channel Dropping Filters. J. Light. Technol. 1997, 15, 998–1005. [Google Scholar] [CrossRef]
- Yariv, A. Universal Relations for Coupling of Optical Power between Microresonators and Dielectric Waveguides. Electron. Lett. 2000, 36, 321–322. [Google Scholar] [CrossRef]
- Van, V. Optical Microring Resonators: Theory, Techniques, and Applications; CRC Press: Boca Raton, FL, USA, 2016; ISBN 978-1-315-30351-2. [Google Scholar]
- Zhu, K.; Zhou, X.; Zhang, Y.; Huang, Z.; Zhang, L. A New Exceptional Point Condition for Coupled Microresonators with Coupled Mode Theory in Space. arXiv 2024, arXiv:2410.17955. [Google Scholar]
- Vázquez-Córdova, S.A.; Dijkstra, M.; Bernhardi, E.H.; Ay, F.; Wörhoff, K.; Herek, J.L.; García-Blanco, S.M.; Pollnau, M. Erbium-Doped Spiral Amplifiers with 20 dB of Net Gain on Silicon. Opt. Express 2014, 22, 25993. [Google Scholar] [CrossRef] [PubMed]
- Rönn, J.; Zhang, W.; Autere, A.; Leroux, X.; Pakarinen, L.; Alonso-Ramos, C.; Säynätjoki, A.; Lipsanen, H.; Vivien, L.; Cassan, E.; et al. Ultra-High on-Chip Optical Gain in Erbium-Based Hybrid Slot Waveguides. Nat. Commun. 2019, 10, 432. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Qiu, Z.; Ji, X.; Lukashchuk, A.; He, J.; Riemensberger, J.; Hafermann, M.; Wang, R.N.; Liu, J.; Ronning, C.; et al. A Photonic Integrated Circuit–Based Erbium-Doped Amplifier. Science 2022, 376, 1309–1313. [Google Scholar] [CrossRef] [PubMed]
- Frankis, H.C.; Mbonde, H.M.; Bonneville, D.B.; Zhang, C.; Mateman, R.; Leinse, A.; Bradley, J.D.B. Erbium-Doped TeO2-Coated Si3 N4 Waveguide Amplifiers with 5 dB Net Gain. Photon. Res. 2020, 8, 127. [Google Scholar] [CrossRef]
- Ma, Z.; Zhou, X.; Zhang, L. Phase Regimes of Parity-Time-Symmetric Coupled-Ring Systems at Exceptional Points. Photon. Res. 2022, 10, 2374. [Google Scholar] [CrossRef]
- Yu, C.; Luo, T.; Zhang, L.; Willner, A.E. Data Pulse Distortion Induced by a Slow-Light Tunable Delay Line in Optical Fiber. Opt. Lett. 2007, 32, 20. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, K.; Zhou, X.; Zhang, Y.; Huang, Z.; Zhang, L. Bandwidth-Tunable Optical Amplifier with Narrowband Filtering Function Enabled by Parity-Time Symmetry at Exceptional Points. Photonics 2024, 11, 1188. https://doi.org/10.3390/photonics11121188
Zhu K, Zhou X, Zhang Y, Huang Z, Zhang L. Bandwidth-Tunable Optical Amplifier with Narrowband Filtering Function Enabled by Parity-Time Symmetry at Exceptional Points. Photonics. 2024; 11(12):1188. https://doi.org/10.3390/photonics11121188
Chicago/Turabian StyleZhu, Kunpeng, Xiaoyan Zhou, Yinxin Zhang, Zhanhua Huang, and Lin Zhang. 2024. "Bandwidth-Tunable Optical Amplifier with Narrowband Filtering Function Enabled by Parity-Time Symmetry at Exceptional Points" Photonics 11, no. 12: 1188. https://doi.org/10.3390/photonics11121188
APA StyleZhu, K., Zhou, X., Zhang, Y., Huang, Z., & Zhang, L. (2024). Bandwidth-Tunable Optical Amplifier with Narrowband Filtering Function Enabled by Parity-Time Symmetry at Exceptional Points. Photonics, 11(12), 1188. https://doi.org/10.3390/photonics11121188






