Selective Ablation and Laser-Induced Periodical Surface Structures (LIPSS) Produced on (Ni/Ti) Nano Layer Thin Film with Ultra-Short Laser Pulses
Abstract
1. Introduction
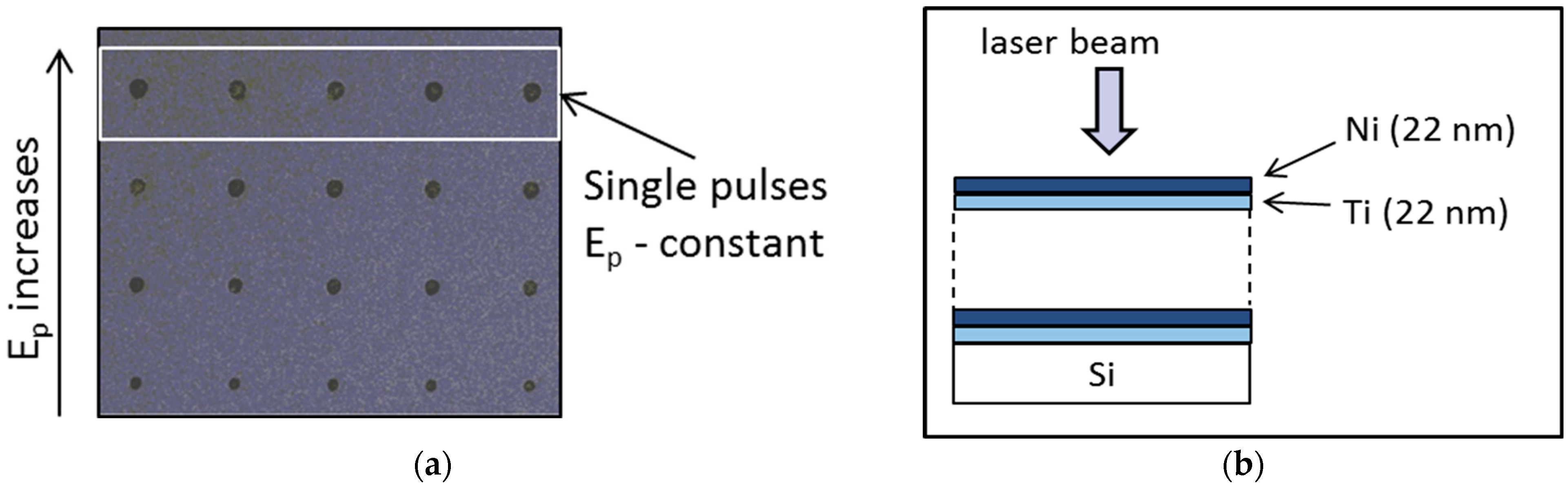
2. Experimental
2.1. Thin Film Preparation and Characterization
2.2. Laser Irradiation Parameters
3. Results and Discussion
3.1. Single Pulse Irradiation—Selective Ablation of 10 × (Ni/Ti)
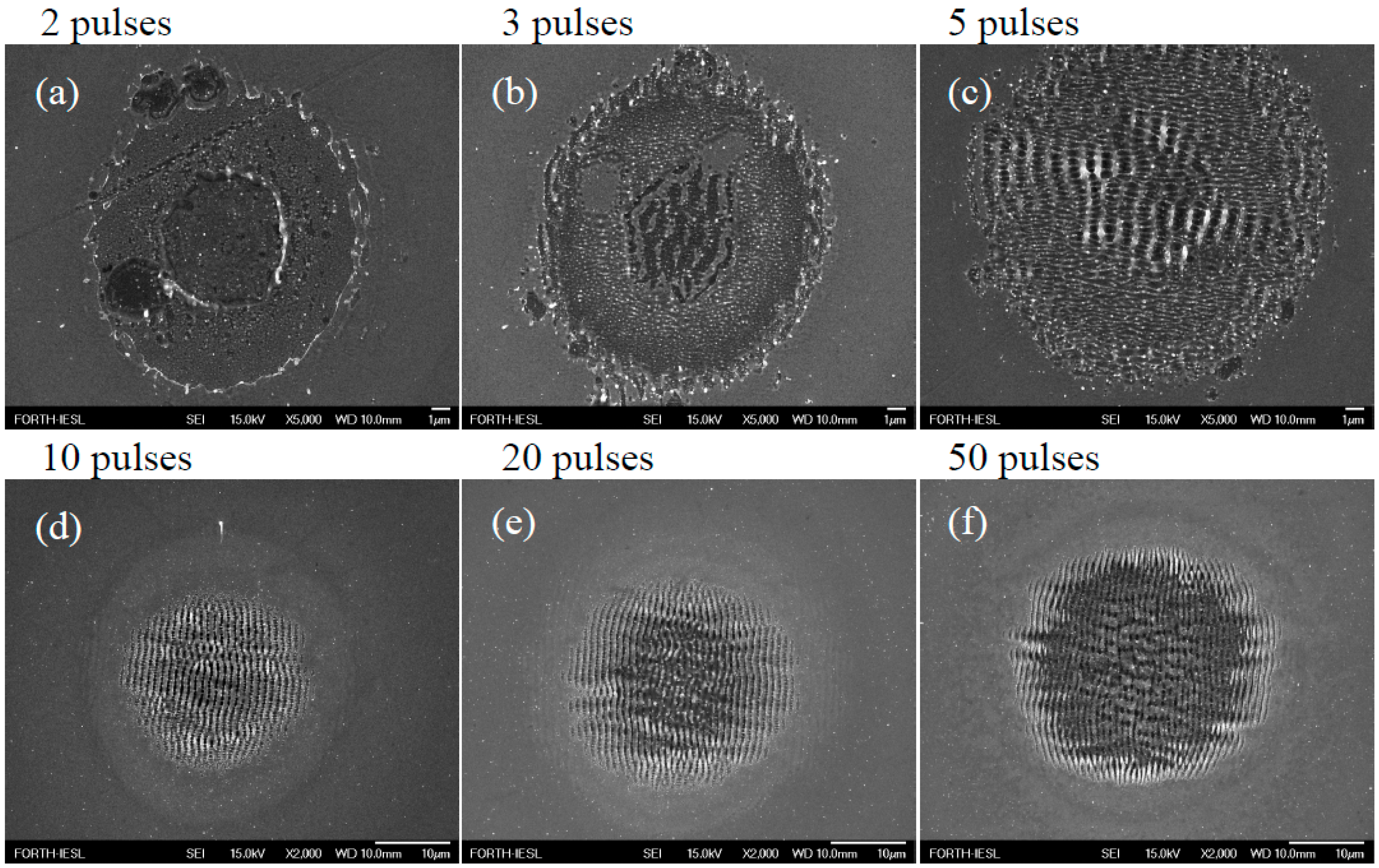
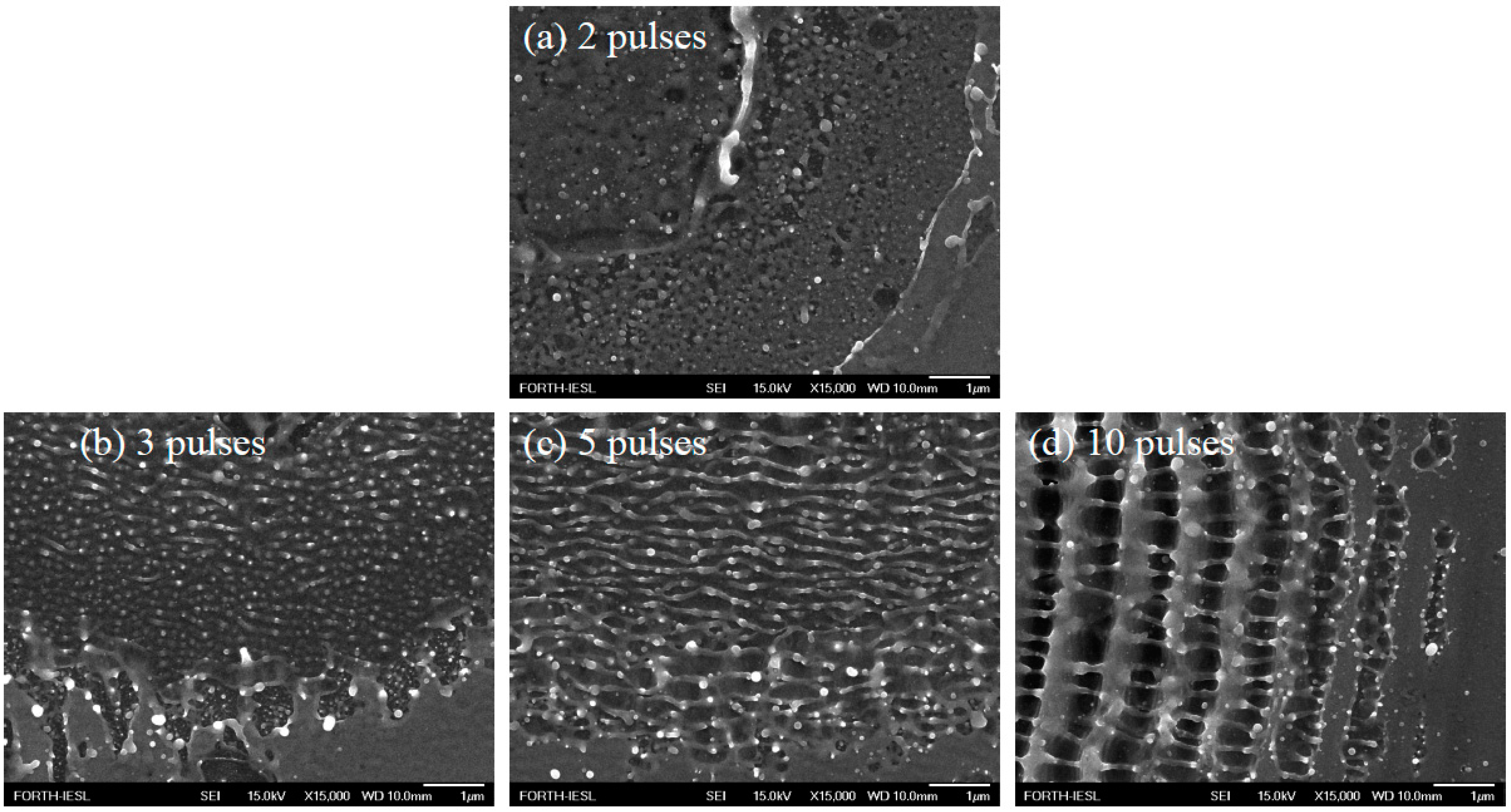
3.2. Multi-Pulse Irradiation—LIPSS Formation on 10 × (Ni/Ti)
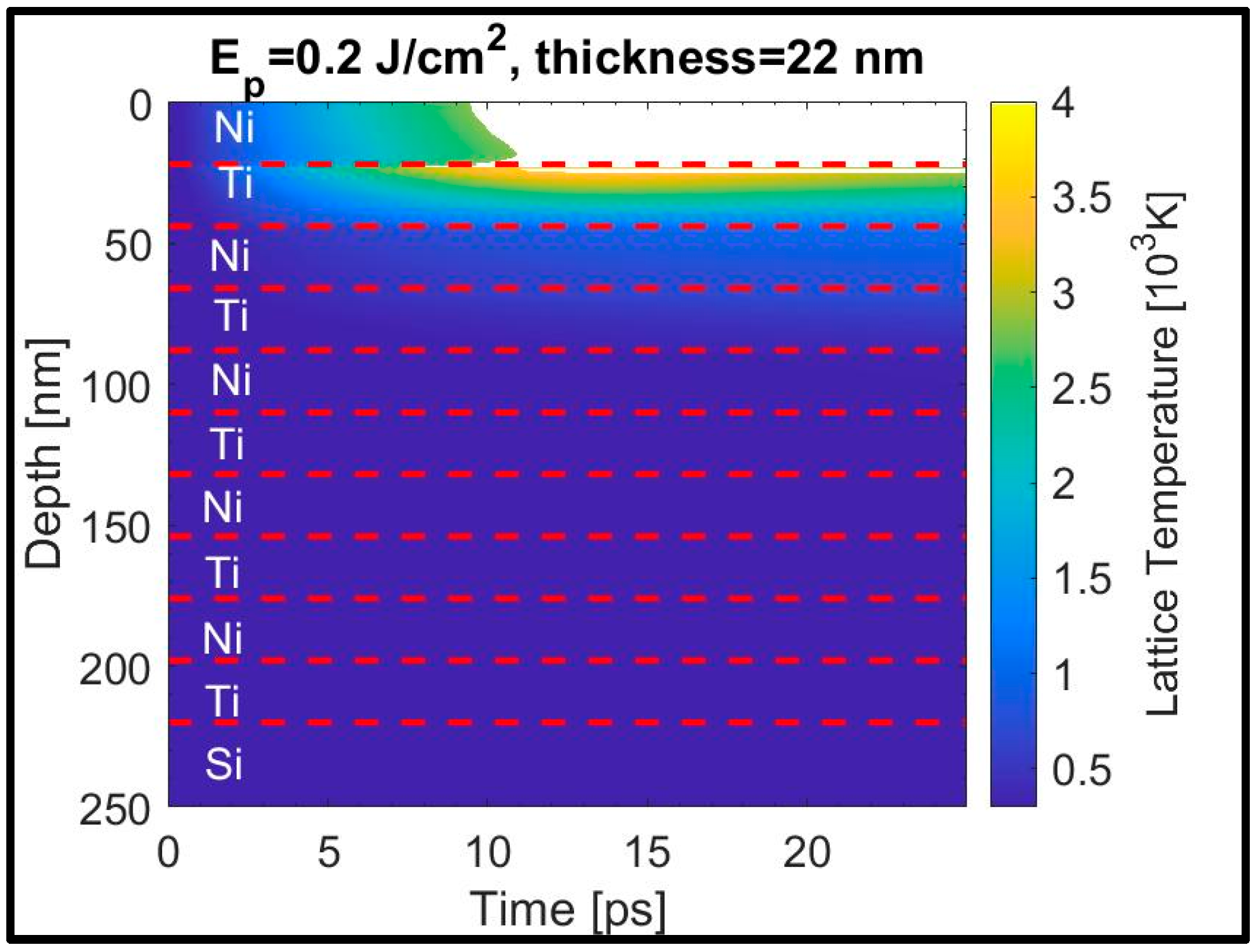
4. Temperature Evolution After Single-Pulse Irradiation
| Parameter | Ti | Ni |
|---|---|---|
| GeL [Wm−3K−1] | Fitting [29,33,34] | Fitting [35] |
| Ce [Jm−3K−1] | Fitting [29,33,34] | Fitting [35] |
| CL [Jm−3K−2] | 2.3521 × 106 [29,33,34] | Fitting [35] |
| ke0 [Jm−1s−1K−1] | 21.9 [29,33,34] | 90 [35] |
| Tmelting [K] | 1941 [29,34] | 1728 [35] |
| Tcritical [K] | 15,500 [29,34] | 8500 [35,36] |
| A [s−1K−2] | Fitting [29] | 0.59 × 107 [35] |
| B [s−1K−1] | Fitting [29] | 1.4 × 1011 [35] |
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shi, J.; Cao, Z.H.; Liu, Y.; Zhao, Z.P. Size dependent alloying and plastic deformation behaviors of Ti/Ni nano-multilayers. J. Alloys Compd. 2017, 727, 691–695. [Google Scholar] [CrossRef]
- Gupta, R.; Gupta, M.; Kulkarni, S.K.; Kharrazi, S.; Gupta, A.; Chaudhari, S.M. Thermal stability of nanometer range Ti/Ni multilayers. Thin Solid Film. 2006, 515, 2213–2219. [Google Scholar] [CrossRef]
- Pietropaolo, A.; Angelone, M.; Bedogni, R.; Colonna, N.; Hurd, A.; Khaplanov, A.; Murtas, F.; Pillon, M.; Piscitelli, F.; Schooneveld, E.; et al. Neutron detection techniques from µeV to GeV. Phys. Rep. 2020, 875, 1–65. [Google Scholar] [CrossRef]
- Adams, D.P. Reactive multilayers fabricated by vapor deposition: A critical review. Thin Solid Film. 2015, 576, 98–128. [Google Scholar] [CrossRef]
- Perez, D.; Lewis, L.J.; Lorazo, P.; Meunier, M. Ablation of molecular solids under nanosecond laser pulses: The role of inertial confinement. Appl. Phys. Lett. 2006, 89, 141907. [Google Scholar] [CrossRef]
- Koji, S.; Cheng, Y. Ultrafast lasers—Reliable tools for advanced materials processing. Light Sci. Appl. 2014, 3, e149. [Google Scholar] [CrossRef]
- Wang, X.; Yu, H.; Li, P.; Zhang, Y.; Wen, Y.; Qiu, Y.; Liu, Z.; Li, Y.; Liu, L. Femtosecond laser-based processing methods and their applications in optical device manufacturing: A review. Opt. Laser Technol. 2021, 135, 106687. [Google Scholar] [CrossRef]
- Gamaly, E.G.; Rode, A.V. Physics of ultra-short laser interaction with matter: From phonon excitation to ultimate transformations. Prog. Quantum Electron. 2013, 37, 215–323. [Google Scholar] [CrossRef]
- Li, X.; Guan, Y. Theoretical fundamentals of short pulse laser–metal interaction: A review. Nanotechnol. Precis. Eng. 2020, 3, 105–125. [Google Scholar] [CrossRef]
- Paltauf, G.; Dyer, P.E. Photomechanical Processes and Effects in Ablation. Chem. Rev. 2003, 103, 487–518. [Google Scholar] [CrossRef]
- Wu, C.; Zhigilei, L.V. Microscopic mechanisms of laser spallation and ablation of metal targets from large-scale molecular dynamics simulations. Appl. Phys. A 2014, 114, 11–32. [Google Scholar] [CrossRef]
- Kim, B.; Nam, H.K.; Watanabe, S.; Park, S.; Kim, Y.; Kim, Y.-J.; Fushinobu, K.; Kim, S.-W. Selective Laser Ablation of Metal Thin Films Using Ultrashort Pulses. Int. J. Precis. Eng. Manuf.-Green Technol. 2020, 8, 771–782. [Google Scholar] [CrossRef]
- Kudryashov, S.I.; Gakovic, B.; Danilov, P.A.; Petrovic, S.M.; Milovanovic, D.; Rudenko, A.A.; Ionin, A.A. Single-shot selective femtosecond laser ablation of multi-layered Ti/Al and Ni/Ti films: “Cascaded” heat conduction and interfacial thermal effects. Appl. Phys. Lett. 2018, 112, 023103. [Google Scholar] [CrossRef]
- Romashevskiy, S.A.; Tsygankov, P.A.; Ashitkov, S.I.; Agranat, M.B. Layer-by-layer modification of thin-film metal–semiconductor multilayers with ultrashort laser pulses. Appl. Phys. A 2018, 124, 375–383. [Google Scholar] [CrossRef]
- Ionin, A.A.; Kudryashov, S.I.; Samokhin, A.A. Reviews of topical problems: Material surface ablation produced by ultrashort laser pulses. Phys. Uspekhi 2017, 60, 149–160. [Google Scholar] [CrossRef]
- Shugaev, M.V.; Zhigilei, L.V. Thermodynamic analysis and atomistic modeling of subsurface cavitation in photomechanical spallation. Comput. Mater. Sci. 2019, 166, 311–317. [Google Scholar] [CrossRef]
- Bonse, J.; Gräf, S. Ten Open Questions about Laser-Induced Periodic Surface Structures. Nanomaterials 2021, 11, 3326. [Google Scholar] [CrossRef]
- Bonse, J.; Höhm, S.; Kirner, S.V.; Rosenfeld, A.; Krüger, J. Laser-induced periodic surface structures-A scientific evergreen. IEEE J. Sel. Top. Quantum Electron. 2017, 23, 9000615. [Google Scholar] [CrossRef]
- De Palo, R.; Mazzarone, A.E.; Volpe, A.; Gaudiuso, C.; Mezzapesa, F.P.; Spagnolo, V.; Ancona, A. Investigation of Laser-Induced Surface Structures (LIPSS) on quartz and evaluation of their influence on material wettability. Opt. Laser Technol. 2024, 169, 110097. [Google Scholar] [CrossRef]
- Rudenko, A.; Mauclair, C.; Garrelie, F.; Stoian, R.; Colombier, J.-P. Amplification and regulation of periodic nanostructures in multi pulse ultrashort laser-induced surface evolution by electromagnetic-hydrodynamic simulations. Phys. Rev. B 2019, 99, 235412. [Google Scholar] [CrossRef]
- Tsibidis, G.D.; Fotakis, C.; Stratakis, E. From ripples to spikes: A hydrodynamical mechanism to interpret femtosecond laser-induced self-assembled structures. Phys. Rev. B 2015, 92, 041405(R). [Google Scholar] [CrossRef]
- Cangueiro, L.T.; Cavaleiro, A.J.; Morgiel, J.; Vilar, R. Mechanisms of formation of low spatial frequency LIPSS on Ni/Ti reactive multilayers. J. Phys. D Appl. Phys. 2016, 49, 365103. [Google Scholar] [CrossRef]
- Lewis, L.J.; Perez, D. Laser ablation with short and ultrashort laser pulses: Basic mechanisms from molecular-dynamics simulations. Appl. Surf. Sci. 2009, 255, 5101–5106. [Google Scholar] [CrossRef]
- Shugaev, M.V.; Wu, C.; Armbruster, O.; Naghilou, A.; Brouwer, N.; Ivanov, D.S.; Derrien, T.J.-Y.; Bulgakova, N.M.; Kautek, W.; Rethfeld, B.; et al. Fundamentals of ultrafast laser–material Interaction. MRS Bull. 2016, 41, 960–968. [Google Scholar] [CrossRef]
- Bulgakova, N.M.; Bourakov, I.M. Phase explosion under ultrashort pulsed laser ablation: Modeling with analysis of metastable state of melt. Appl. Surf. Sci. 2002, 197–198, 41–44. [Google Scholar] [CrossRef]
- Gnilitskyi, I.; Derrien, T.J.Y.; Levy, Y.; Bulgakova, N.M.; Mocek, T.; Orazi, L. High-speed manufacturing of highly regular femtosecond laser-induced periodic surface structures: Physical origin of regularity. Sci. Rep. 2017, 7, 8485. [Google Scholar] [CrossRef]
- Skoulas, E.; Tasolamprou, A.C.; Kenanakis, G.; Stratakis, E. Laser induced periodic surface structures as polarizing optical elements. Appl. Surf. Sci. 2021, 541, 148470. [Google Scholar] [CrossRef]
- Gaković, B.; Tsibidis, G.D.; Skoulas, E.; Petrović, S.M.; Vasić, B.; Stratakis, E. Partial ablation of Ti/Al nano-layer thin film by single femtosecond laser pulse. J. Appl. Phys. 2017, 122, 223106. [Google Scholar] [CrossRef]
- Kuznietsov, O.V.; Tsibidis, G.D.; Demchishin, A.V.; Demchishin, A.A.; Babizhetskyy, V.; Saldan, I.; Bellucci, S.; Gnilitskyi, I. Femtosecond Laser-Induced Periodic Surface Structures on 2D Ti-Fe Multilayer Condensates. Nanomaterials 2021, 11, 316. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light, 7th expanded ed.; Cambridge University Press: Cambridge, UK; New York, NY, USA, 1999. [Google Scholar]
- Tsibidis, G.D.; Barberoglou, M.; Loukakos, P.A.; Stratakis, E.; Fotakis, C. Dynamics of ripple formation on silicon surfaces by ultrashort laser pulses in subablation conditions. Phys. Rev. B 2012, 86, 115316. [Google Scholar] [CrossRef]
- Kelly, R.; Miotello, A. Comments on explosive mechanisms of laser sputtering. Appl. Surf. Sci. 1996, 96–98, 205–215. [Google Scholar] [CrossRef]
- Lin, Z.; Zhigilei, L.V.; Celli, V. Electron-phonon coupling and electron heat capacity of metals under conditions of strong electron-phonon non equilibrium. Phys. Rev. B 2008, 77, 075133. [Google Scholar] [CrossRef]
- Lide, D.R. CRC Handbook of Chemistry and Physics, 84th ed.; CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Horvath, A.L. Critical temperature of elements and the periodic system. J. Chem. Educ. 1973, 50, 335. [Google Scholar] [CrossRef]
- Tsibidis, G.D. Ultrafast dynamics of non-equilibrium electrons and strain generation under femtosecond laser irradiation of Nickel. Appl. Phys. A 2018, 124, 311. [Google Scholar] [CrossRef]

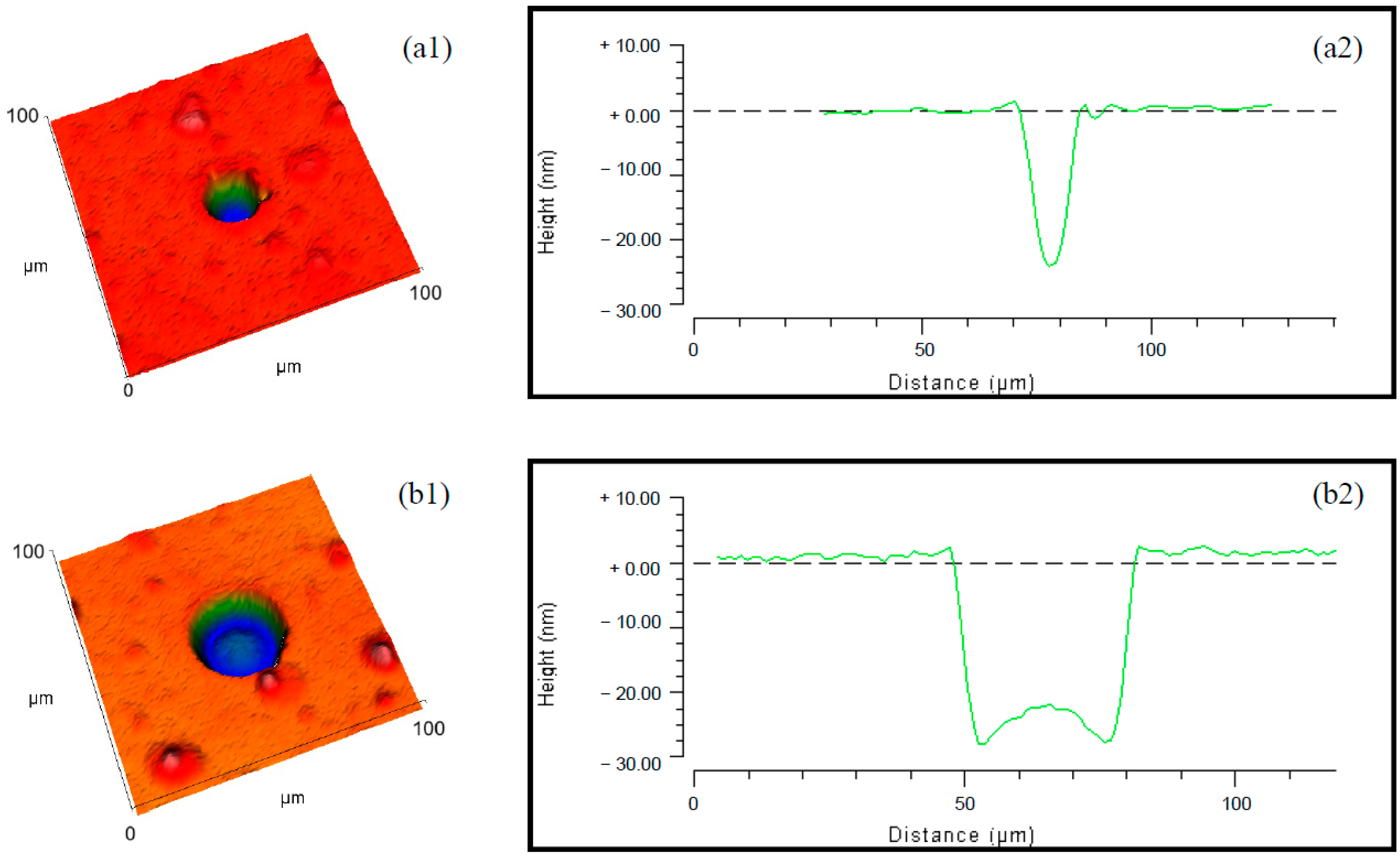

| Pulse Fluence [J/cm2] | Single-Step Ablation Minimal/Maximal Depth of the [nm] |
|---|---|
| 0.09 | 21.0 |
| 0.1 | 21.0 |
| 0.2 | 22.0/26.6 |
| 0.3 | 21.2/27.6 |
| 0.4 | 22.7/27.9 |
| Pulse fluence [J/cm2] | Two-step ablation depths [nm] |
| 0.5 | first step 23.2 second step 41.3 |
| 1.0 | >440 * |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gaković, B.; Petrović, S.; Siogka, C.; Milovanović, D.; Momčilović, M.; Tsibidis, G.D.; Stratakis, E. Selective Ablation and Laser-Induced Periodical Surface Structures (LIPSS) Produced on (Ni/Ti) Nano Layer Thin Film with Ultra-Short Laser Pulses. Photonics 2024, 11, 1054. https://doi.org/10.3390/photonics11111054
Gaković B, Petrović S, Siogka C, Milovanović D, Momčilović M, Tsibidis GD, Stratakis E. Selective Ablation and Laser-Induced Periodical Surface Structures (LIPSS) Produced on (Ni/Ti) Nano Layer Thin Film with Ultra-Short Laser Pulses. Photonics. 2024; 11(11):1054. https://doi.org/10.3390/photonics11111054
Chicago/Turabian StyleGaković, Biljana, Suzana Petrović, Christina Siogka, Dubravka Milovanović, Miloš Momčilović, George D. Tsibidis, and Emmanuel Stratakis. 2024. "Selective Ablation and Laser-Induced Periodical Surface Structures (LIPSS) Produced on (Ni/Ti) Nano Layer Thin Film with Ultra-Short Laser Pulses" Photonics 11, no. 11: 1054. https://doi.org/10.3390/photonics11111054
APA StyleGaković, B., Petrović, S., Siogka, C., Milovanović, D., Momčilović, M., Tsibidis, G. D., & Stratakis, E. (2024). Selective Ablation and Laser-Induced Periodical Surface Structures (LIPSS) Produced on (Ni/Ti) Nano Layer Thin Film with Ultra-Short Laser Pulses. Photonics, 11(11), 1054. https://doi.org/10.3390/photonics11111054









