Effects of Black Silicon Surface Morphology Induced by a Femtosecond Laser on Absorptance and Photoelectric Response Efficiency
Abstract
1. Introduction
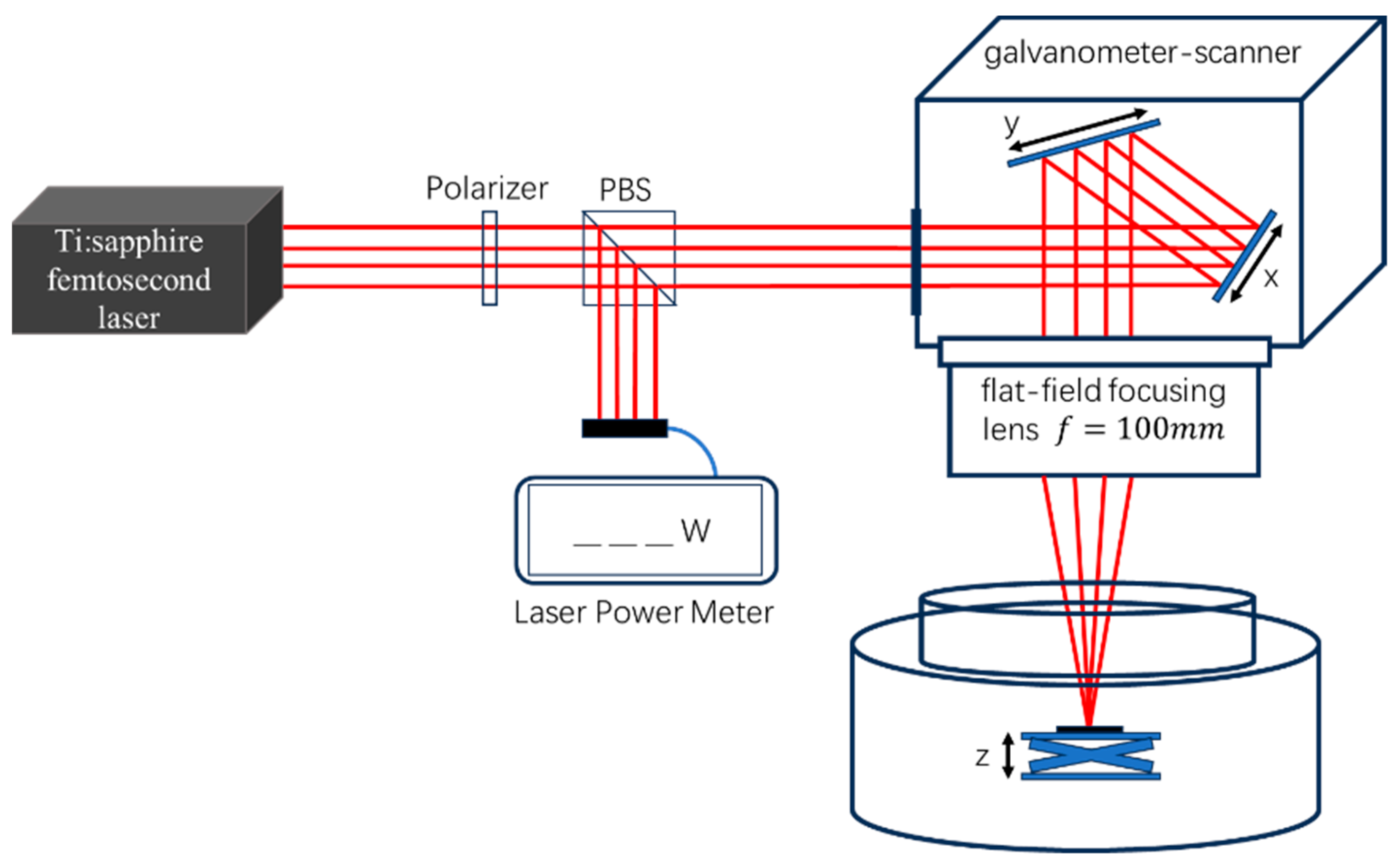
2. Experiments and Simulations
3. Results and Analysis
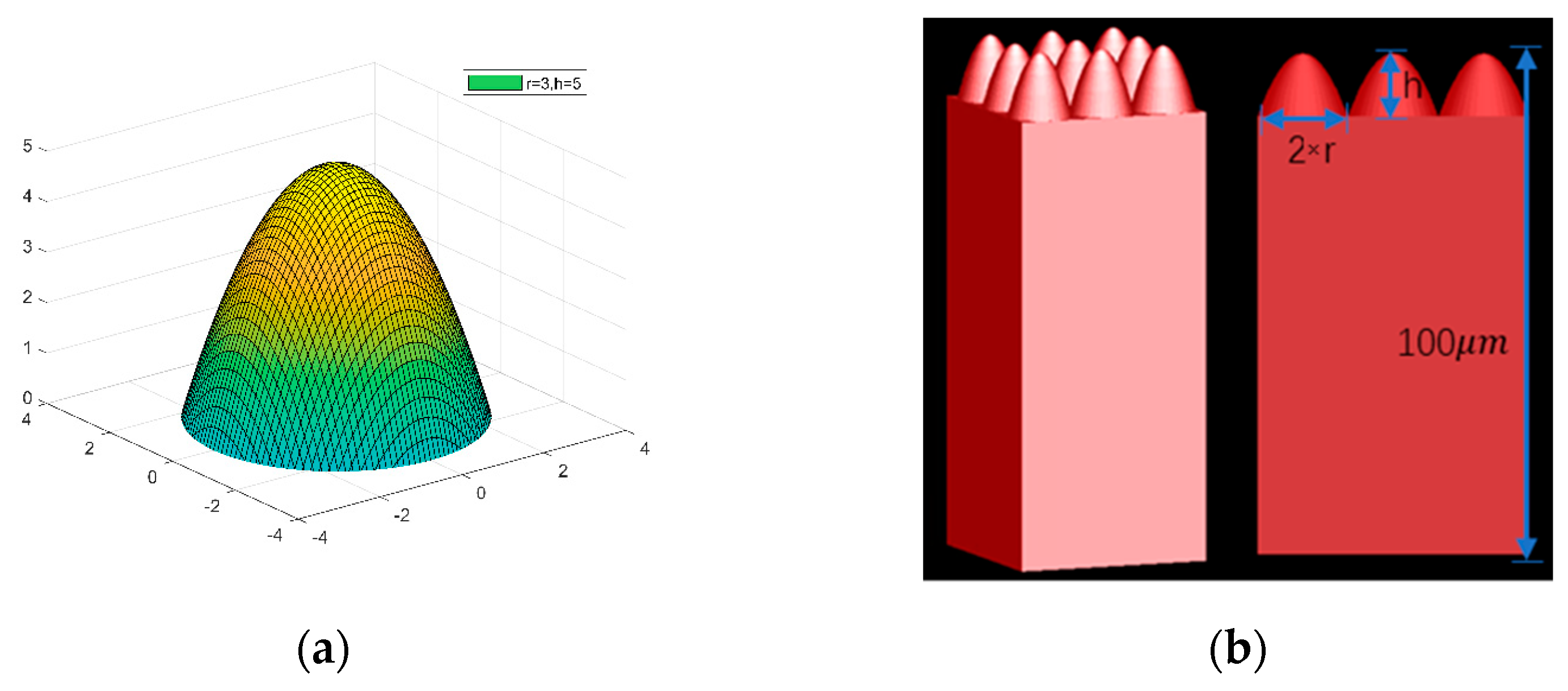
3.1. FDTD Simulation Results for Black Silicon
- 1.
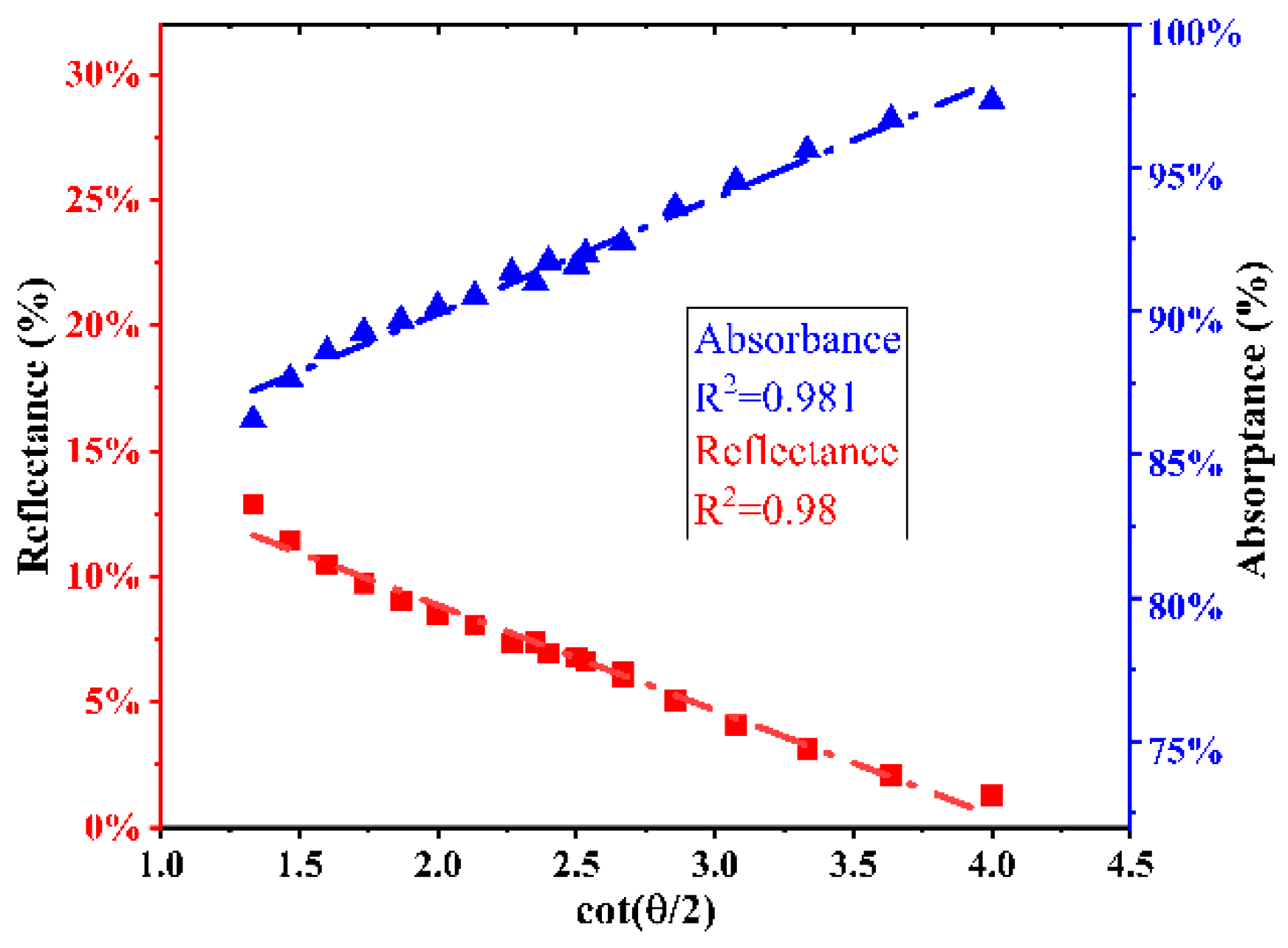
- Without considering substrate thinning, an increase in the height of black silicon surface microstructures corresponds to a decrease in reflectance and an increase in absorptance. Similarly, a reduction in the bottom radius of black silicon surface microstructures leads to a decrease in reflectance and an increase in absorptance.
- 2.
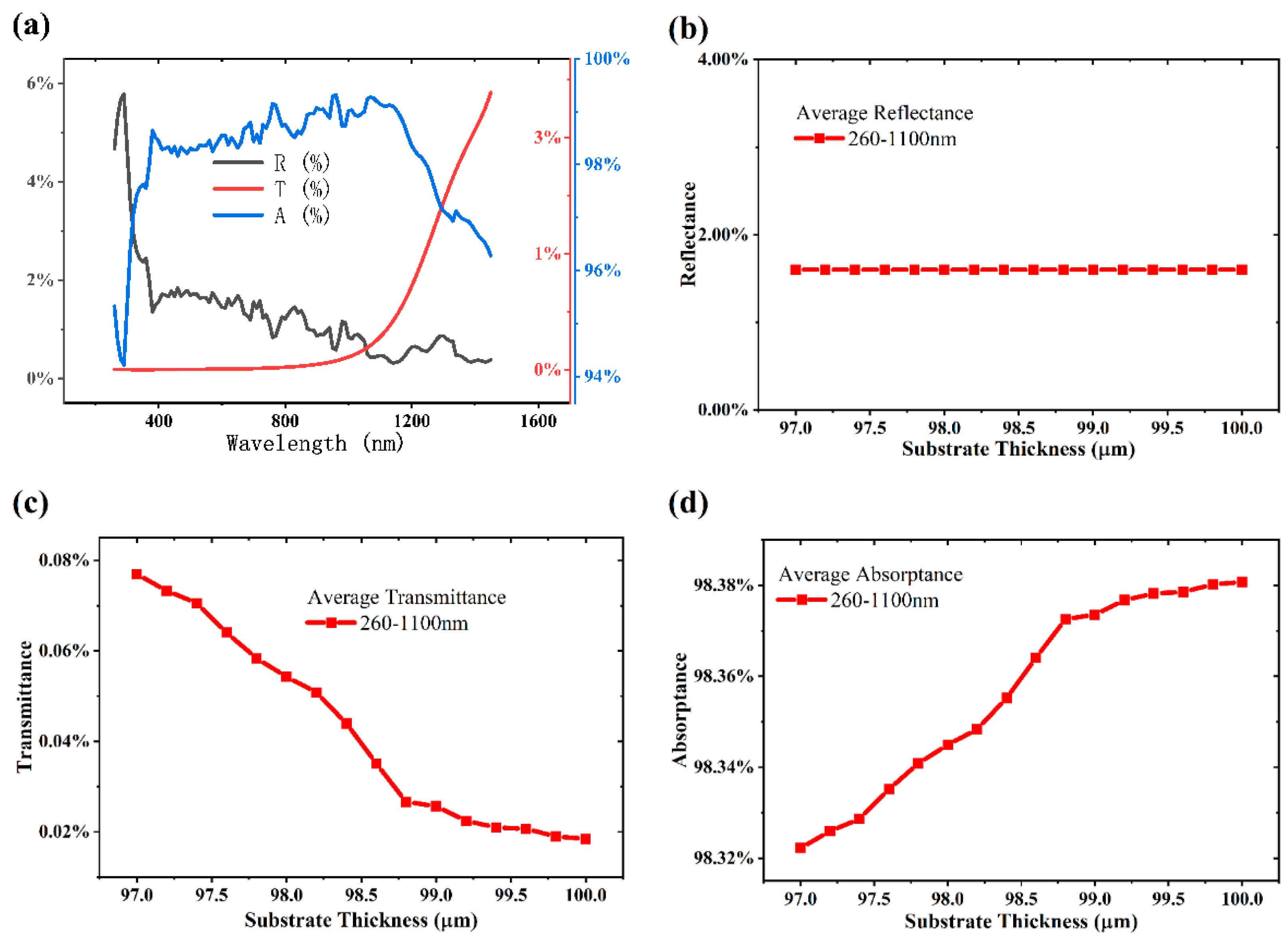
- In the wavelength range where the photon energy exceeds the bandgap width of silicon (200 < λ < 1100 ), the majority of incident light is absorbed by black silicon. The primary factor influencing the absorptance of black silicon is the trapped light energy of its surface microstructures, specifically the magnitude of light reflectance. Although there is a certain enhancement in transmittance in this wavelength range, its magnitude can be considered negligible compared with the overall absorptance.
- 3.
- After simulating black silicon with substrate thicknesses of 97 to 100 , we find that substrate thinning increases the transmittance and decreases the absorptance of black silicon, but in the 260–1100 band, the difference in absorptance between the 97 and 100 substrate thicknesses of black silicon is only 0.06%, which is a negligible effect. Meanwhile, it can be observed in Figure 6d that the thicker the substrate, the higher the absorptance, but with the increase in the thickness, the phenomenon of absorptance saturation occurs [33].
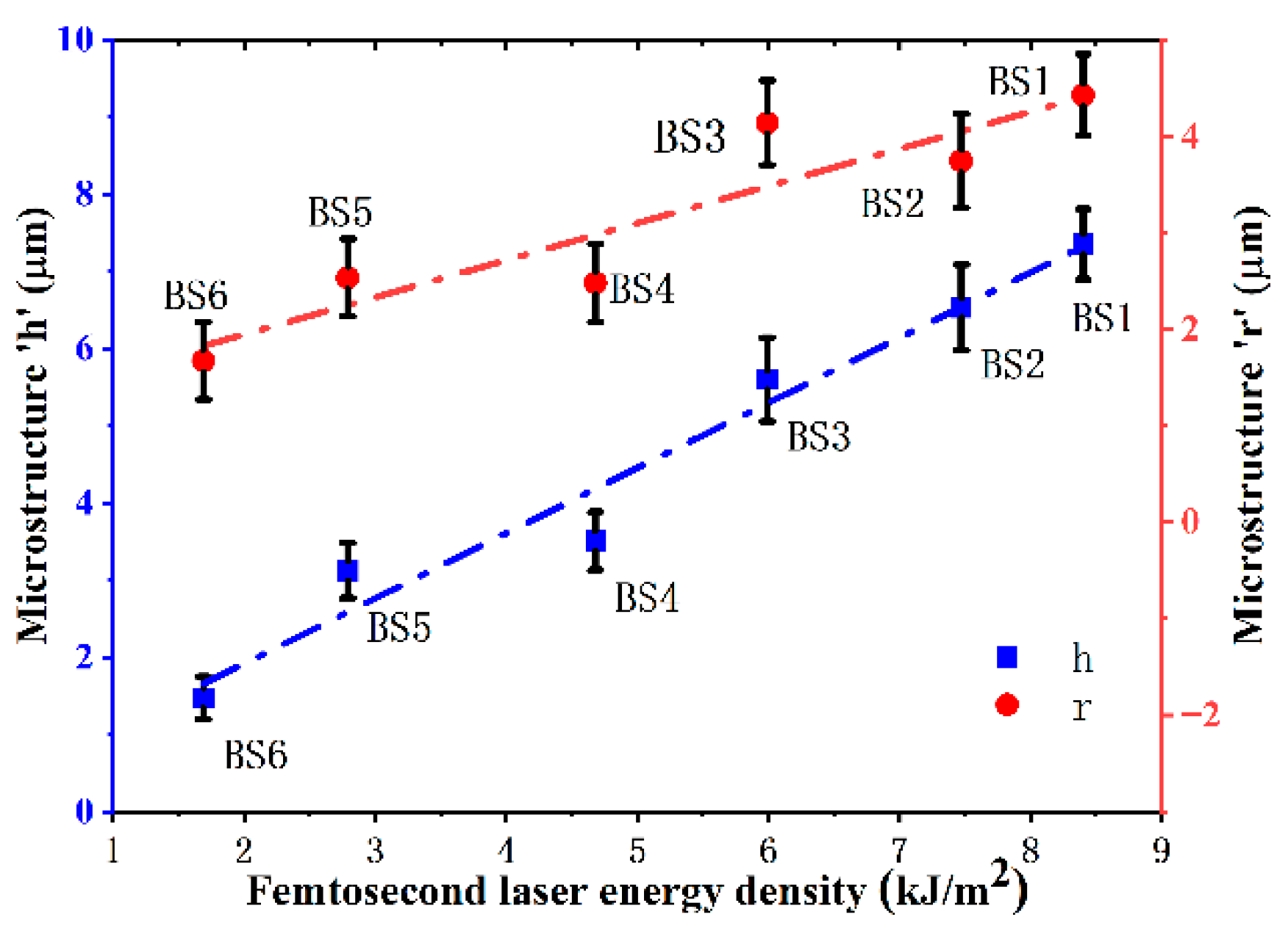
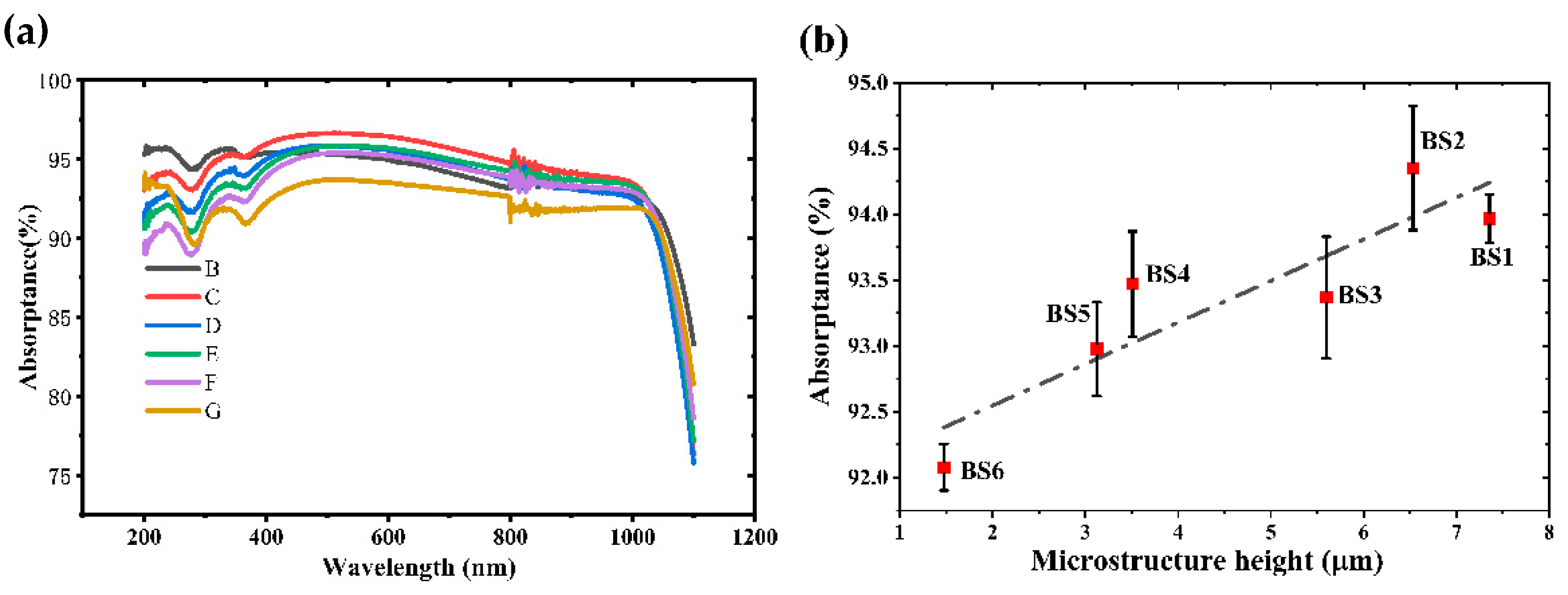
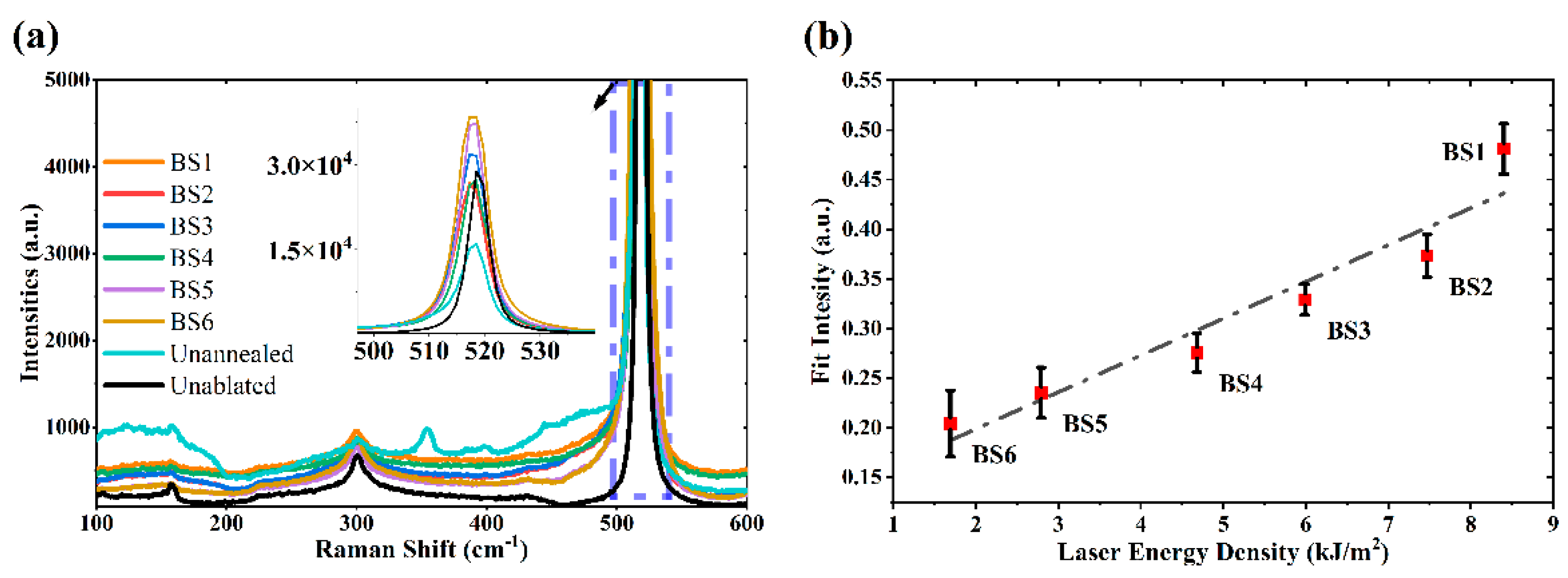
3.2. Black Silicon Surface Morphology and Its Absorptance
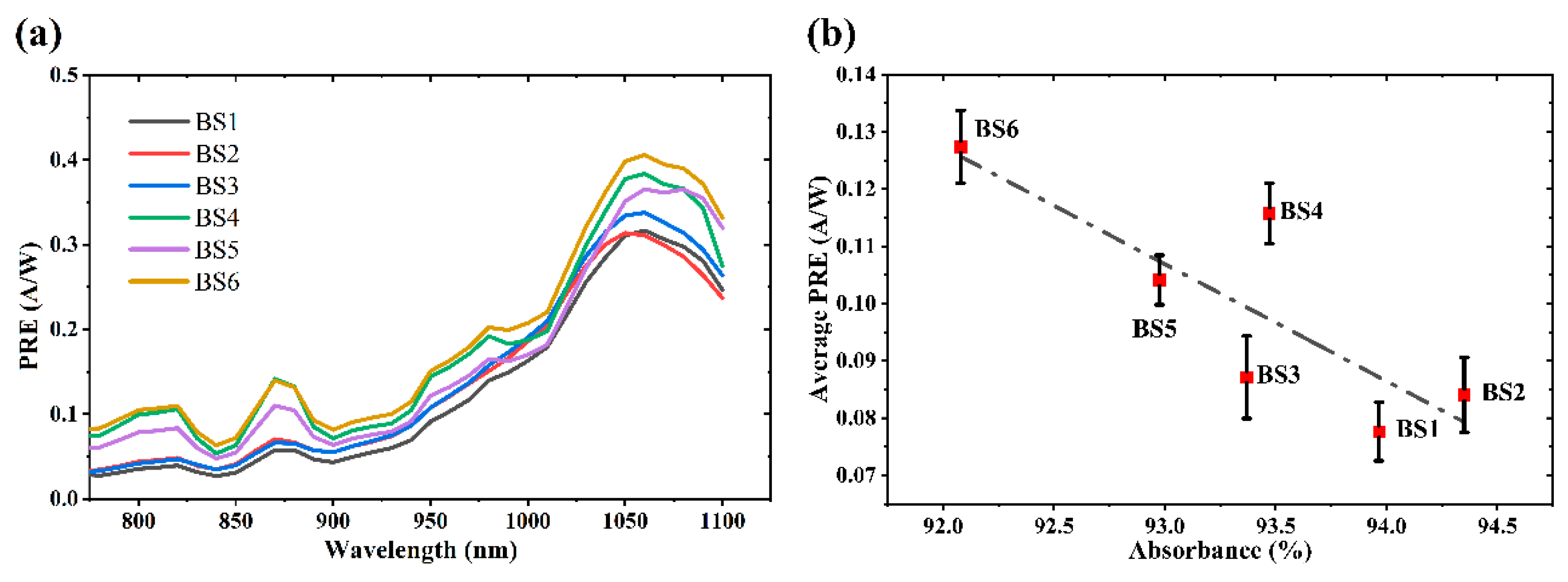
3.3. Influence of Surface Morphology on the Photoelectric Properties of Black Silicon Photodiodes
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zheng, J.P.; Jiao, K.L.; Shen, W.P.; Anderson, W.A.; Kwok, H.S. Highly sensitive photodetector using porous silicon. Appl. Phys. Lett. 1992, 61, 459–461. [Google Scholar] [CrossRef]
- Otto, M.; Algasinger, M.; Branz, H.; Gesemann, B.; Gimpel, T.; Füchsel, K.; Käsebier, T.; Kontermann, S.; Koynov, S.; Li, X. Black silicon photovoltaics. Adv. Opt. Mater. 2015, 3, 147–164. [Google Scholar] [CrossRef]
- Liu, X.; Coxon, P.R.; Peters, M.; Hoex, B.; Cole, J.M.; Fray, D.J. Black silicon: Fabrication methods, properties and solar energy applications. Energy Environ. Sci. 2014, 7, 3223–3263. [Google Scholar] [CrossRef]
- Fan, Z.; Cui, D.; Zhang, Z.; Zhao, Z.; Chen, H.; Fan, Y.; Li, P.; Zhang, Z.; Xue, C.; Yan, S. Recent progress of black silicon: From fabrications to applications. Nanomaterials 2020, 11, 41. [Google Scholar] [CrossRef]
- Ma, L.L.; Zhou, Y.C.; Jiang, N.; Lu, X.; Shao, J.; Lu, W.; Ge, J.; Ding, X.M.; Hou, X.Y. Wide-band “black silicon” based on porous silicon. Appl. Phys. Lett. 2006, 88, 171907. [Google Scholar] [CrossRef]
- Wu, J.; Jiang, Q.; Chen, S.; Tang, M.; Mazur, Y.I.; Maidaniuk, Y.; Benamara, M.; Semtsiv, M.P.; Masselink, W.T.; Sablon, K.A.; et al. Monolithically integrated InAs/GaAs quantum dot mid-infrared photodetectors on silicon substrates. Acs Photonics 2016, 3, 749–753. [Google Scholar] [CrossRef]
- Martin, T.; Dixon, P.; Gagliardi, M.A.; Masaun, N. 320×240 pixel InGaAs/InP focal plane array for short-wave infrared and visible light imaging. In Semiconductor Photodetectors II; SPIE: Bellingham, WA, USA, 2005; Volume 5726, pp. 85–91. [Google Scholar]
- Miao, Y.; Wang, G.; Kong, Z.; Xu, B.; Zhao, X.; Luo, X.; Lin, H.; Dong, Y.; Lu, B.; Dong, L. Review of Si-based GeSn CVD growth and optoelectronic applications. Nanomaterials 2021, 11, 2556. [Google Scholar] [CrossRef]
- Liu, S.J.; Chu, J.H. Theoretical study of monolithic dielectric bolometer by thermal diffusion equation. In Infrared Components and Their Applications; SPIE: Bellingham, WA, USA, 2005; Volume 5640, pp. 49–53. [Google Scholar]
- Feldman, A. Algorithm for solutions of the thermal diffusion equation in a stratified medium with a modulated heating source. High Temperatures-High Pressures 1999, 31, 293–298. [Google Scholar] [CrossRef]
- Her, T.-H.; Finlay, R.J.; Wu, C.; Deliwala, S.; Mazur, E. Microstructuring of silicon with femtosecond laser pulses. Appl. Phys. Lett. 1998, 73, 1673–1675. [Google Scholar] [CrossRef]
- Jin, X.; Sun, Y.; Wu, Q.; Jia, Z.; Huang, S.; Yao, J.; Huang, H.; Xu, J. High-performance free-standing flexible photodetectors based on sulfur-hyperdoped ultrathin silicon. ACS Appl. Mater. Interfaces 2019, 11, 42385–42391. [Google Scholar] [CrossRef]
- Dong, B.; Wang, W.; Liu, X.-L.; Li, H.; Li, Y.; Huang, Y.; Ning, X.; Zhao, L.; Zhuang, J. Light and gas dual-function detection and mutual enhancement based on hyperdoped black silicon. Optics Express 2024, 32, 13384–13395. [Google Scholar] [CrossRef] [PubMed]
- Bardalen, E.; Bouchouri, A.; Akram, M.N.; Nguyen, H.-V. Black Silicon as Anti-Reflective Structure for Infrared Imaging Applications. Nanomaterials 2023, 14, 20. [Google Scholar] [CrossRef]
- Zhang, Y.; Loh, J.Y.; Kherani, N.P. Facilely Achieved Self-Biased Black Silicon Heterojunction Photodiode with Broadband Quantum Efficiency Approaching 100%. Adv. Sci. 2022, 9, 2203234. [Google Scholar] [CrossRef] [PubMed]
- Kalem, S. Time-resolved radiative recombination in black silicon. J. Mater. Sci. Mater. Electron. 2023, 34, 724. [Google Scholar] [CrossRef]
- Wu, S.; Chen, Q.; Zhang, L.; Ren, H.; Zhou, H.; Hu, L.; Tan, C.S. Wafer-scale nanostructured black silicon with morphology engineering via advanced Sn-assisted dry etching for sensing and solar cell applications. Nanoscale 2023, 15, 4843–4851. [Google Scholar] [CrossRef]
- Kim, J.; Lee, J.; Lee, J.M.; Facchetti, A.; Marks, T.J.; Park, S.K. Recent Advances in Low-Dimensional Nanomaterials for Photodetectors. Small Methods 2024, 8, 2300246. [Google Scholar] [CrossRef]
- Zhao, J.-H.; Li, X.-B.; Chen, Q.-D.; Chen, Z.-G.; Sun, H.-B. Ultrafast laser-induced black silicon, from micro-nanostructuring, infrared absorption mechanism, to high performance detecting devices. Mater. Today Nano 2020, 11, 100078. [Google Scholar] [CrossRef]
- Holton, W.C.; Cavin, R. A perspective on CMOS technology trends. Proc. IEEE 1986, 74, 1646–1668. [Google Scholar] [CrossRef]
- Ma, S.; Liu, S.; Xu, Q.; Xu, J.; Lu, R.; Liu, Y.; Zhong, Z. A theoretical study on the optical properties of black silicon. AIP Adv. 2018, 8, 035010. [Google Scholar] [CrossRef]
- Rahman, T.; Boden, S.A. Optical modeling of black silicon for solar cells using effective index techniques. IEEE J. Photovolt. 2017, 7, 1556–1562. [Google Scholar] [CrossRef]
- Elsayed, A.A.; Sabry, Y.M.; Marty, F.; Bourouina, T.; Khalil, D. Optical modeling of black silicon using an effective medium/multi-layer approach. Opt. Express 2018, 26, 13443–13460. [Google Scholar] [CrossRef] [PubMed]
- Schäfer, S.; McKearney, P.; Paulus, S.; Kontermann, S. Analytical model for extracting optical properties from absorptance of femtosecond-laser structured hyperdoped silicon. J. Appl. Phys. 2022, 131, 243102. [Google Scholar] [CrossRef]
- Wu, C.; Crouch, C.; Zhao, L.; Carey, J.; Younkin, R.; Levinson, J.; Mazur, E.; Farrell, R.; Gothoskar, P.; Karger, A. Near-unity below-band-gap absorption by microstructured silicon. Appl. Phys. Lett. 2001, 78, 1850–1852. [Google Scholar] [CrossRef]
- Apel, C.; Meister, J.; Ioana, R.; Franzen, R.; Hering, P.; Gutknecht, N. The ablation threshold of Er: YAG and Er: YSGG laser radiation in dental enamel. Lasers Med. Sci. 2002, 17, 246–252. [Google Scholar] [CrossRef] [PubMed]
- Parker, G.; Bonar, J.; Starbuck, C. Long incubation times for selective epitaxial growth of silicon using silane only. Electron. Lett. 1991, 27, 1595–1597. [Google Scholar] [CrossRef]
- Wang, W.; Ma, S.; Liu, X.; Zhao, Y.; Li, H.; Li, Y.; Ning, X.; Zhao, L.; Zhuang, J. NO2 gas sensor with excellent performance based on thermally modified nitrogen-hyperdoped silicon. Sens. Actuators B Chem. 2022, 354, 131193. [Google Scholar] [CrossRef]
- Tabbal, M.; Kim, T.; Woolf, D.N.; Shin, B.; Aziz, M.J. Fabrication and sub-band-gap absorption of single-crystal Si supersaturated with Se by pulsed laser mixing. Appl. Phys. A 2010, 98, 589–594. [Google Scholar] [CrossRef]
- Ma, S.-X.; Liu, X.-L.; Sun, H.-B.; Zhao, Y.; Hu, Y.; Ning, X.-J.; Zhao, L.; Zhuang, J. Enhanced responsivity of co-hyperdoped silicon photodetectors fabricated by femtosecond laser irradiation in a mixed SF 6/NF 3 atmosphere. JOSA B 2020, 37, 730–735. [Google Scholar] [CrossRef]
- Crouch, C.H.; Carey, J.E.; Warrender, J.M.; Aziz, M.J.; Mazur, E.; Génin, F.Y. Comparison of structure and properties of femtosecond and nanosecond laser-structured silicon. Appl. Phys. Lett. 2004, 84, 1850–1852. [Google Scholar] [CrossRef]
- Neudeck, G.; Thompson Jr, H.; Schwartz, R. High-Frequency Noise in Germanium-Silicon n-n Heterojunctions. J. Appl. Phys. 1969, 40, 4108–4114. [Google Scholar] [CrossRef]
- Zhao, X.; Zhao, B.; Lin, K.; Nivas, J.J.; Amoruso, S.; Du, W.; Wang, X. Direct fabrication of black Si with enhanced infrared transmittance using femtosecond laser irradiation. Opt. Laser Technol. 2024, 168, 109881. [Google Scholar] [CrossRef]
- James, R.; Schweig, E.; Smith, D.; McGill, T. Experimental investigation of the infrared absorption saturation in p-type germanium and silicon. Appl. Phys. Lett. 1982, 40, 231–233. [Google Scholar] [CrossRef]
- Del Corro, E.; Taravillo, M.; Baonza, V.G. Nonlinear strain effects in double-resonance Raman bands of graphite, graphene, and related materials. Phys. Rev. B—Condens. Matter Mater. Phys. 2012, 85, 033407. [Google Scholar] [CrossRef]
- Golovkin, B. Quantitative analysis of solid-phase mixtures by Raman spectroscopy. J. Appl. Spectrosc. 2008, 75, 444–447. [Google Scholar] [CrossRef]
- Shen, W.; Lin, X.; Jiang, C.; Li, C.; Lin, H.; Huang, J.; Wang, S.; Liu, G.; Yan, X.; Zhong, Q. Reliable quantitative SERS analysis facilitated by core–shell nanoparticles with embedded internal standards. Angew. Chem. Int. Ed. 2015, 54, 7308–7312. [Google Scholar] [CrossRef]
- Carey, J.E.; Crouch, C.H.; Shen, M.; Mazur, E. Visible and near-infrared responsivity of femtosecond-laser microstructured silicon photodiodes. Opt. Lett. 2005, 30, 1773–1775. [Google Scholar] [CrossRef]
- Wang, Y.; Gao, J.; Yang, H.; Wang, X. Near-infrared absorption enhancement in microstructured silicon by Ag film deposition. J. Mater. Sci. Mater. Electron. 2016, 27, 9002–9007. [Google Scholar] [CrossRef]








Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Li, W.; Jin, C.; Cao, Y.; Liu, F.; Wei, N.; Wang, B.; Zhou, R.; Zhu, X.; Zhao, W. Effects of Black Silicon Surface Morphology Induced by a Femtosecond Laser on Absorptance and Photoelectric Response Efficiency. Photonics 2024, 11, 947. https://doi.org/10.3390/photonics11100947
Zhang X, Li W, Jin C, Cao Y, Liu F, Wei N, Wang B, Zhou R, Zhu X, Zhao W. Effects of Black Silicon Surface Morphology Induced by a Femtosecond Laser on Absorptance and Photoelectric Response Efficiency. Photonics. 2024; 11(10):947. https://doi.org/10.3390/photonics11100947
Chicago/Turabian StyleZhang, Xiaomo, Weinan Li, Chuan Jin, Yi Cao, Feng Liu, Na Wei, Bo Wang, Rundong Zhou, Xiangping Zhu, and Wei Zhao. 2024. "Effects of Black Silicon Surface Morphology Induced by a Femtosecond Laser on Absorptance and Photoelectric Response Efficiency" Photonics 11, no. 10: 947. https://doi.org/10.3390/photonics11100947
APA StyleZhang, X., Li, W., Jin, C., Cao, Y., Liu, F., Wei, N., Wang, B., Zhou, R., Zhu, X., & Zhao, W. (2024). Effects of Black Silicon Surface Morphology Induced by a Femtosecond Laser on Absorptance and Photoelectric Response Efficiency. Photonics, 11(10), 947. https://doi.org/10.3390/photonics11100947





