Diffractive Optical Encryption Systems Based on Multiple Wavelengths and Multiple Distances
Abstract
1. Introduction
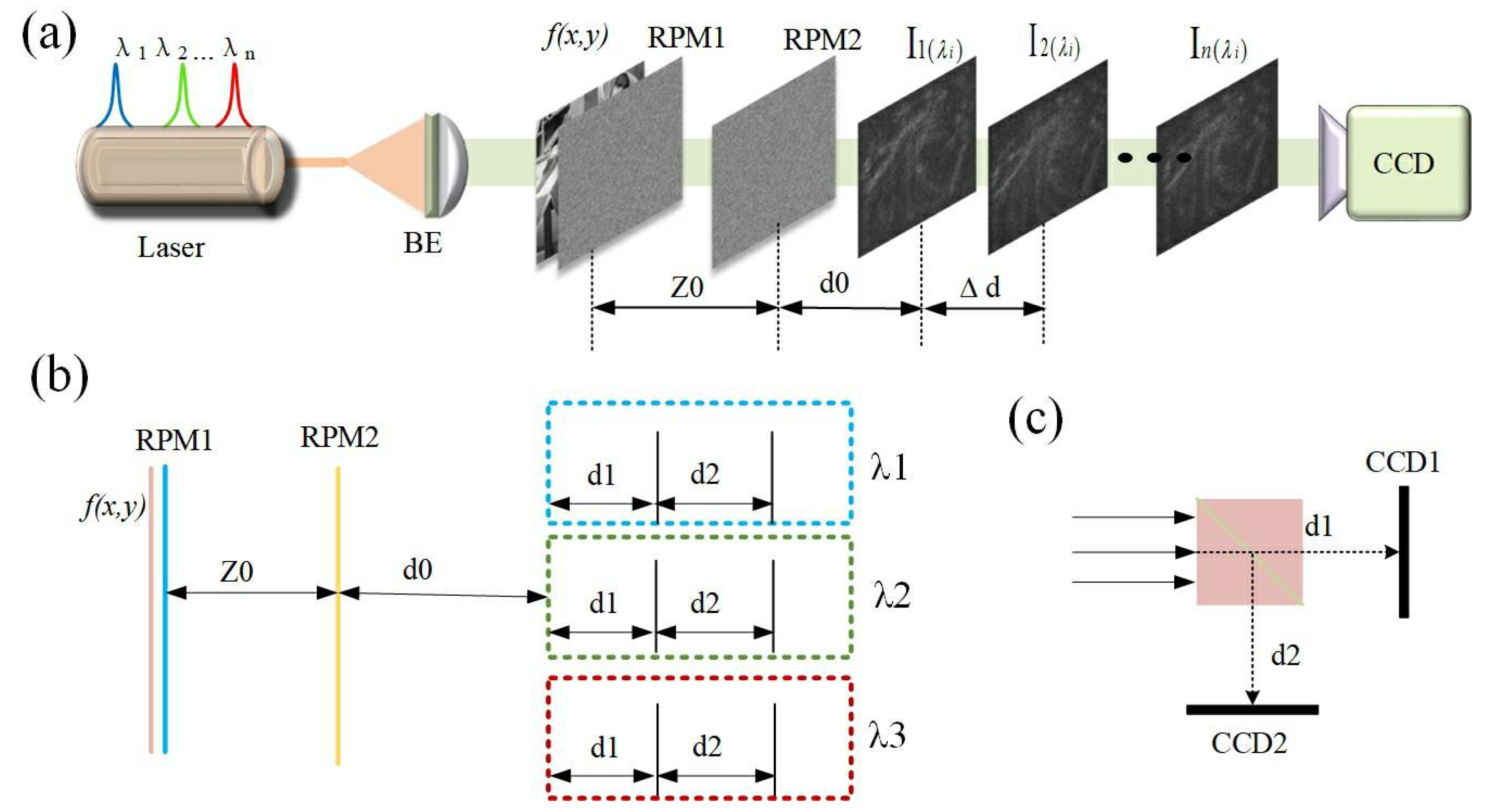
2. Basic Principle
2.1. Encryption Process
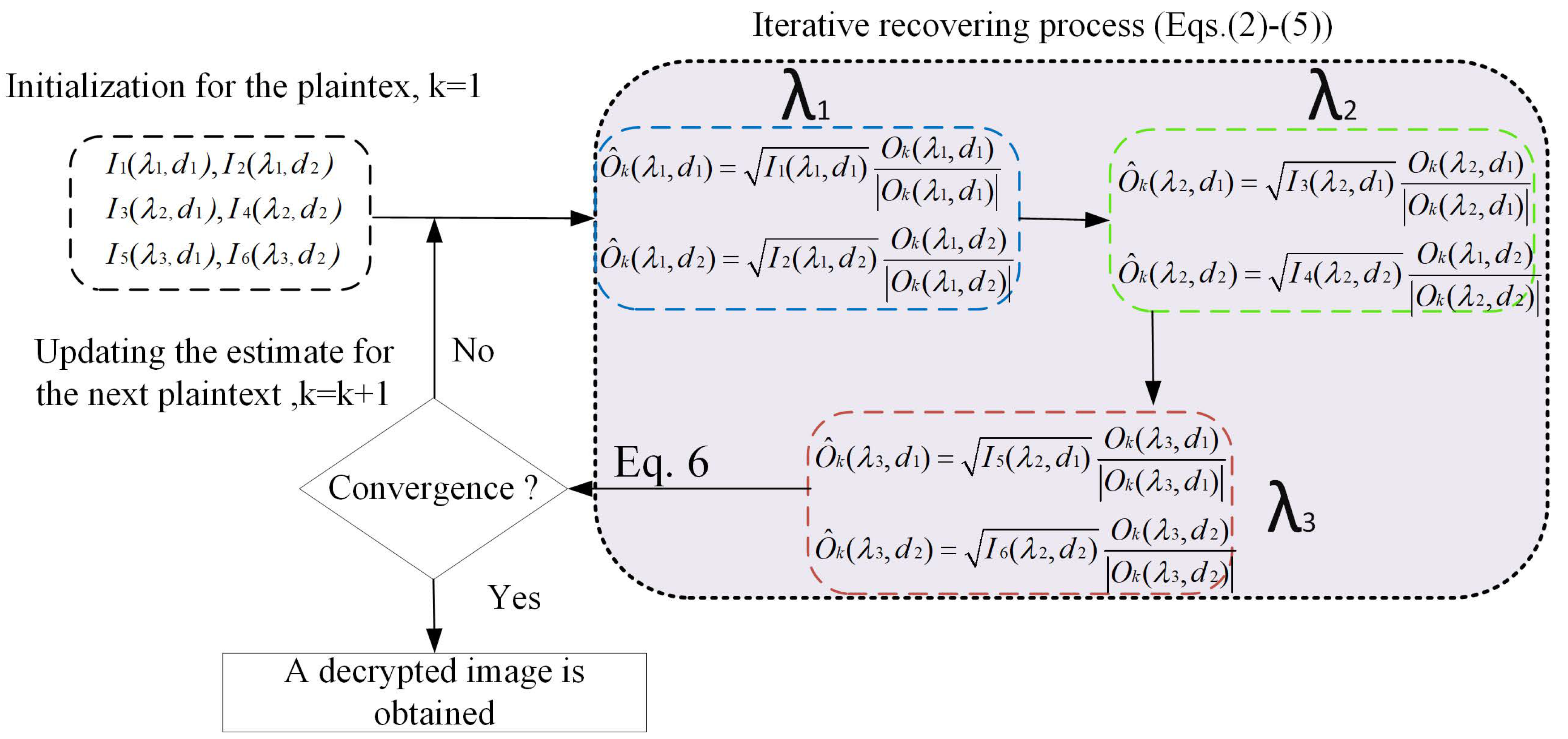
2.2. Decryption Process
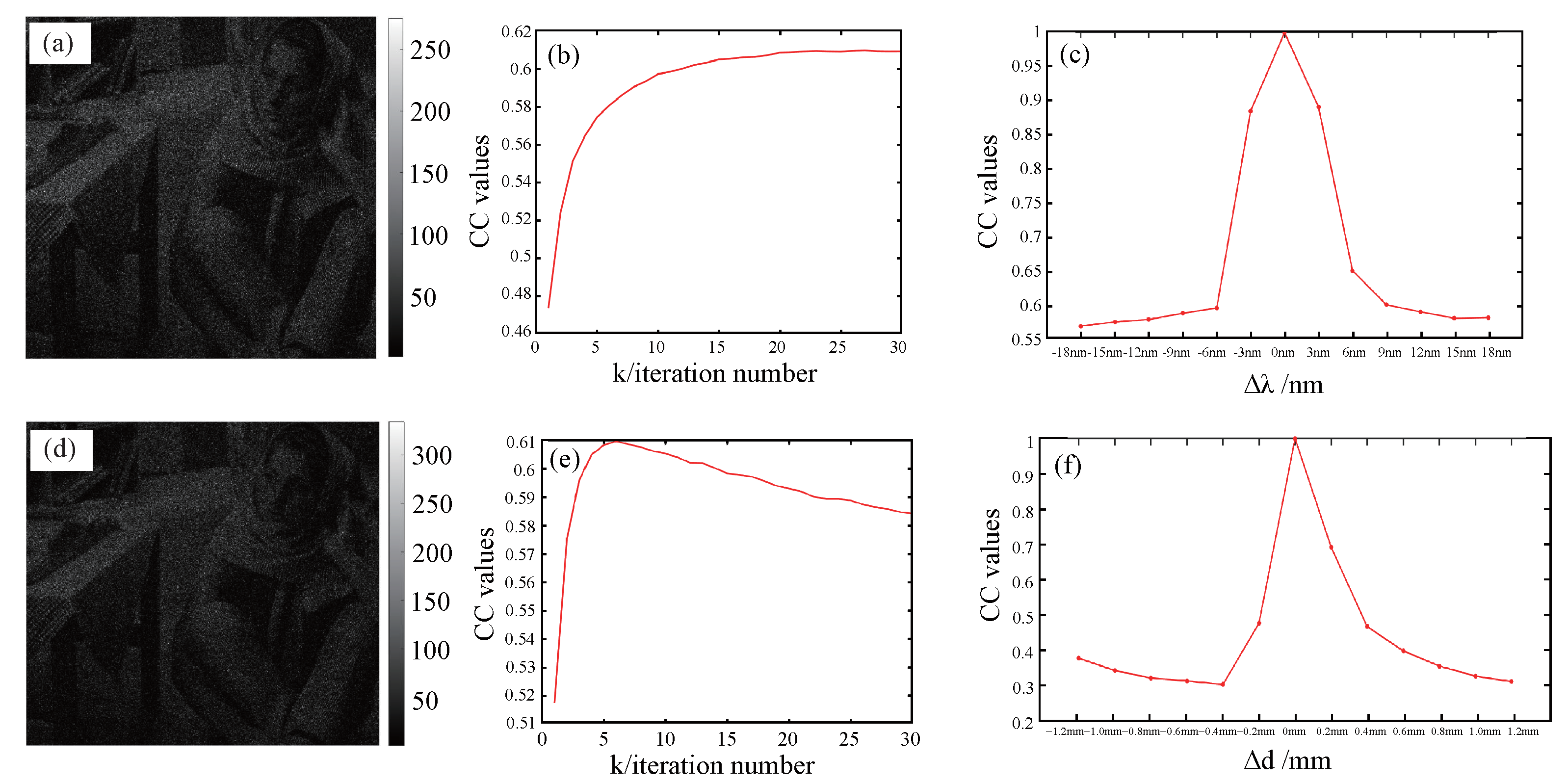
3. Simulation Verification
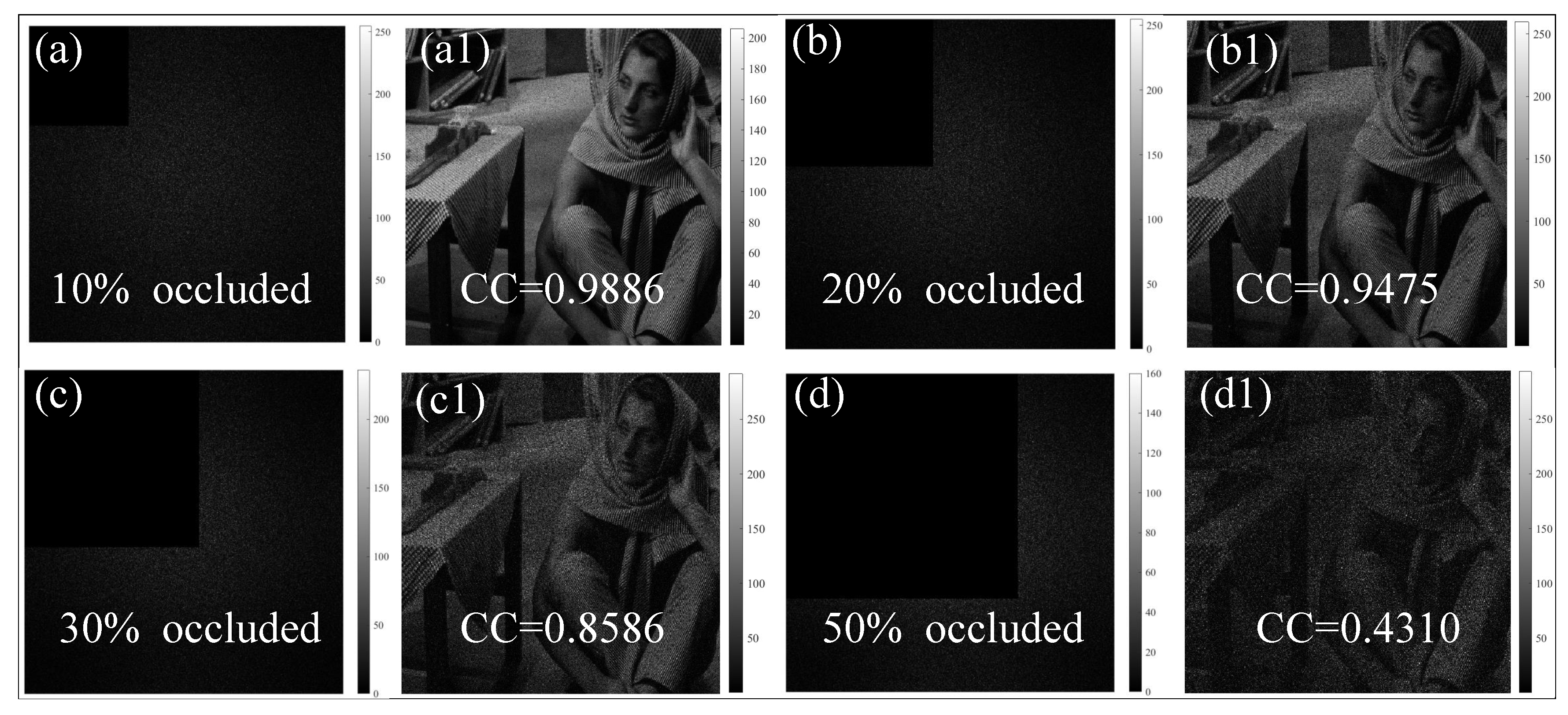
4. Analysis and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Refregier, P.; Javidi, B. Optical image encryption based on input plane and Fourier plane random encoding. Opt. Lett. 1995, 20, 767–769. [Google Scholar] [CrossRef] [PubMed]
- Unnikrishnan, G.; Joseph, J.; Singh, K. Optical encryption by double-random phase encoding in the fractional Fourier domain. Opt. Lett. 2000, 25, 887–889. [Google Scholar] [CrossRef] [PubMed]
- Sui, L.; Duan, K.; Liang, J.; Hei, X. Asymmetric double-image encryption based on cascaded discrete fractional random transform and logistic maps. Opt. Express 2014, 22, 10605–10621. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.; Xu, W.; Wang, S.; Wu, S. Asymmetric optical cryptosystem based on modulus decomposition in Fresnel domain. Opt. Commun. 2017, 402, 302–310. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, B. Asymmetric optical Optical image encryption based on interference. Opt. Lett. 2008, 33, 2443–2445. [Google Scholar] [CrossRef]
- Li, J.; Li, J.; Shen, L.; Pan, Y.; Li, R. Optical image encryption and hiding based on a modified Mach-Zehnder interferometer. Opt. Express 2014, 22, 4849–4860. [Google Scholar] [CrossRef] [PubMed]
- Tay, C.; Quan, C.; Chen, W.; Fu, Y. Color image encryption based on interference and virtual optics. Opt. Laser Tech. 2010, 42, 409–415. [Google Scholar] [CrossRef]
- Zhu, N.; Wang, Y.; Liu, J.; Xie, J.; Zhang, H. Optical image encryption based on interference of polarized light. Opt. Express 2009, 17, 13418–13424. [Google Scholar] [CrossRef]
- Yang, L.; Yang, J.; Wang, H.; Wang, Y.; Huang, T.; Zhang, W.; Di, J.; Zhong, L. Single shot interferenceless coded aperture correlation holography via a learnable Wiener deconvolution network. Opt. Lasers Eng. 2024, 178, 108227–108232. [Google Scholar] [CrossRef]
- Yang, L.; Yang, J.; Huang, T.; Rosen, J.; Wang, Y.; Wang, H.; Lu, X.; Zhang, W.; Di, J.; Zhong, L. Accelerating quad Airy beams-based point response for interferenceless coded aperture correlation holography. Opt. Lett. 2024, 49, 4429–4432. [Google Scholar] [CrossRef]
- Chen, W.; Chen, X.; Sheppard, C.J.R. Optical image encryption based on diffractive imaging. Opt. Lett. 2010, 35, 3817–3819. [Google Scholar] [CrossRef] [PubMed]
- Qin, Y.; Wang, Z.; Gong, Q. Diffractive-imaging-based optical image encryption with simplified decryption from single diffraction pattern. Appl. Opt. 2014, 53, 4094–4099. [Google Scholar] [CrossRef] [PubMed]
- Hu, J.; Mengu, D.; Tzarouchis, D.C.; Edwards, B.; Engheta, N.; Ozcan, A. Diffractive optical computing in free space. Nat. Commun. 2024, 15, 1525–1545. [Google Scholar] [CrossRef] [PubMed]
- Zhou, L.; Xiao, Y.; Chen, W. Vulnerability to machine learning attacks of optical encryption based on diffractive imaging. Opt. Lasers Eng. 2020, 125, 105858. [Google Scholar] [CrossRef]
- Situ, G.; Zhang, J. Multiple-image encryption by wavelength multiplexing. Opt. Lett. 2005, 30, 1306–1308. [Google Scholar] [CrossRef]
- Shi, Y.; Li, T.; Wang, Y.; Gao, Q.; Zhang, S.; Li, H. Optical image encryption via ptychography. Opt. Lett. 2013, 38, 1425–1427. [Google Scholar] [CrossRef]
- Clemente, P.; Durán, V.; Torres-Company, V.; Tajahuerce, E.; Lancis, J. Optical encryption based on computational ghost imaging. Opt. Lett. 2010, 35, 2391–2393. [Google Scholar] [CrossRef]
- Luan, G.; Quan, C. Optical phase-truncation-based double-image encryption using equal modulus decomposition and random masks. Sci. Rep. 2024, 14, 7155–7159. [Google Scholar] [CrossRef]
- Yi, J.; Tan, G. Binary-tree encryption strategy for optical multiple-image encryption. Appl. Opt. 2016, 55, 5280–5291. [Google Scholar] [CrossRef]
- César, C.; Araceli, V.M.; Hilario, M.; David, T.; Gina, G.; Lizbeth, M.C.; Carlos, T. Asymmetric encryption by optical Kerr nonlinearities exhibited by electrochromic NiO thin films. Opt. Express 2022, 30, 39849–39859. [Google Scholar]
- Chang, H.; Wang, Y.; Chen, C. Angle Multiplexing Optical Image Encryption in the Fresnel Transform Domain Using Phase-Only Computer-Generated Hologram. Photonics 2020, 7, 1. [Google Scholar] [CrossRef]
- Zhang, N.; Xiong, B.; Zhang, X.; Yuan, X. Holographic Encryption Applications Using Composite Orbital Angular Momentum Beams. Photonics 2022, 9, 605. [Google Scholar] [CrossRef]
- Zhang, Y.S.; Xiao, D.; Wen, W.Y.; Liu, H. Vulnerability to chosen-plaintext attack of a general optical encryption model with the architecture of scrambling-then-double random phase encoding. Opt. Lett. 2013, 38, 4506–4509. [Google Scholar] [CrossRef] [PubMed]
- Goodman, J.W. Introduction to Fourier Optics; Roberts and Company Publishers: Greenwood Village, CO, USA, 2005. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Y.; Duan, H.; He, Y.; Liu, Y. Diffractive Optical Encryption Systems Based on Multiple Wavelengths and Multiple Distances. Photonics 2024, 11, 922. https://doi.org/10.3390/photonics11100922
Wu Y, Duan H, He Y, Liu Y. Diffractive Optical Encryption Systems Based on Multiple Wavelengths and Multiple Distances. Photonics. 2024; 11(10):922. https://doi.org/10.3390/photonics11100922
Chicago/Turabian StyleWu, Yitong, Haowei Duan, Yuze He, and Yuanyuan Liu. 2024. "Diffractive Optical Encryption Systems Based on Multiple Wavelengths and Multiple Distances" Photonics 11, no. 10: 922. https://doi.org/10.3390/photonics11100922
APA StyleWu, Y., Duan, H., He, Y., & Liu, Y. (2024). Diffractive Optical Encryption Systems Based on Multiple Wavelengths and Multiple Distances. Photonics, 11(10), 922. https://doi.org/10.3390/photonics11100922




