Investigation of Multiple High Quality-Factor Fano Resonances in Asymmetric Nanopillar Arrays for Optical Sensing
Abstract
1. Introduction
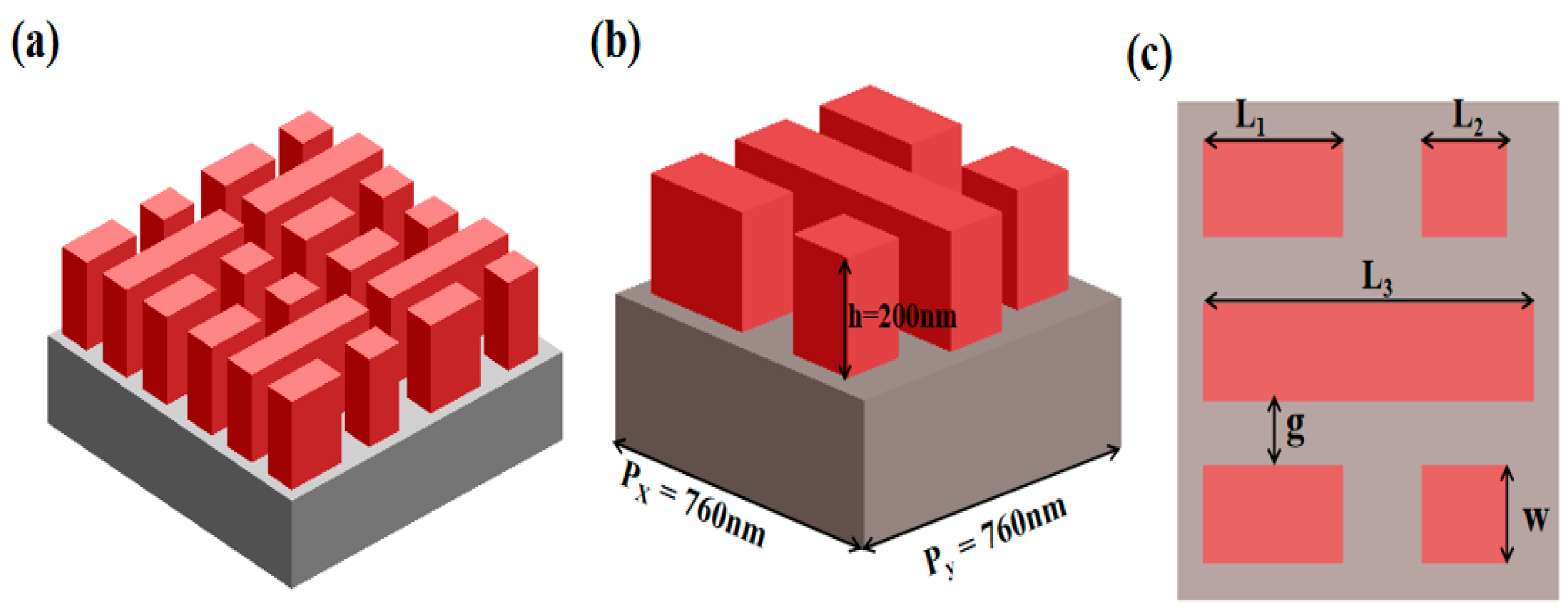
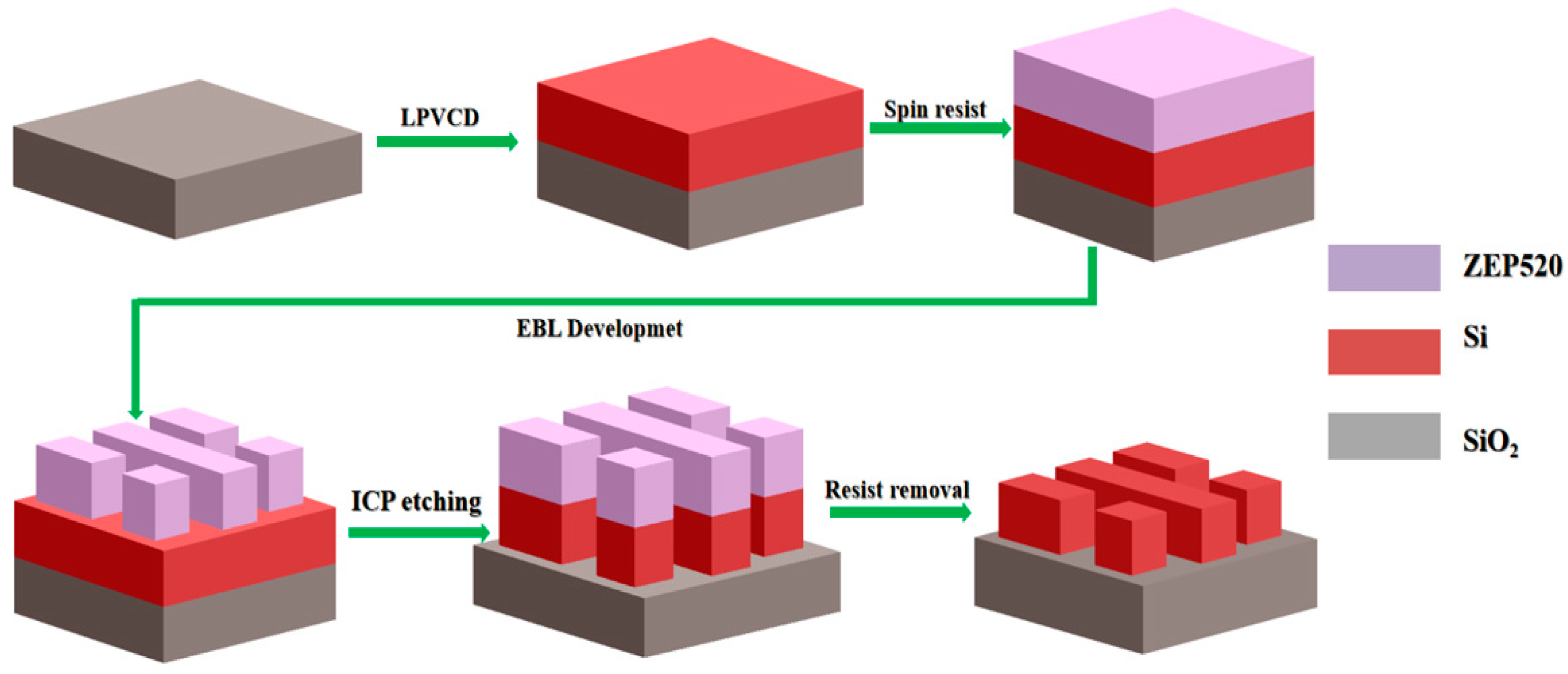
2. Structure Design
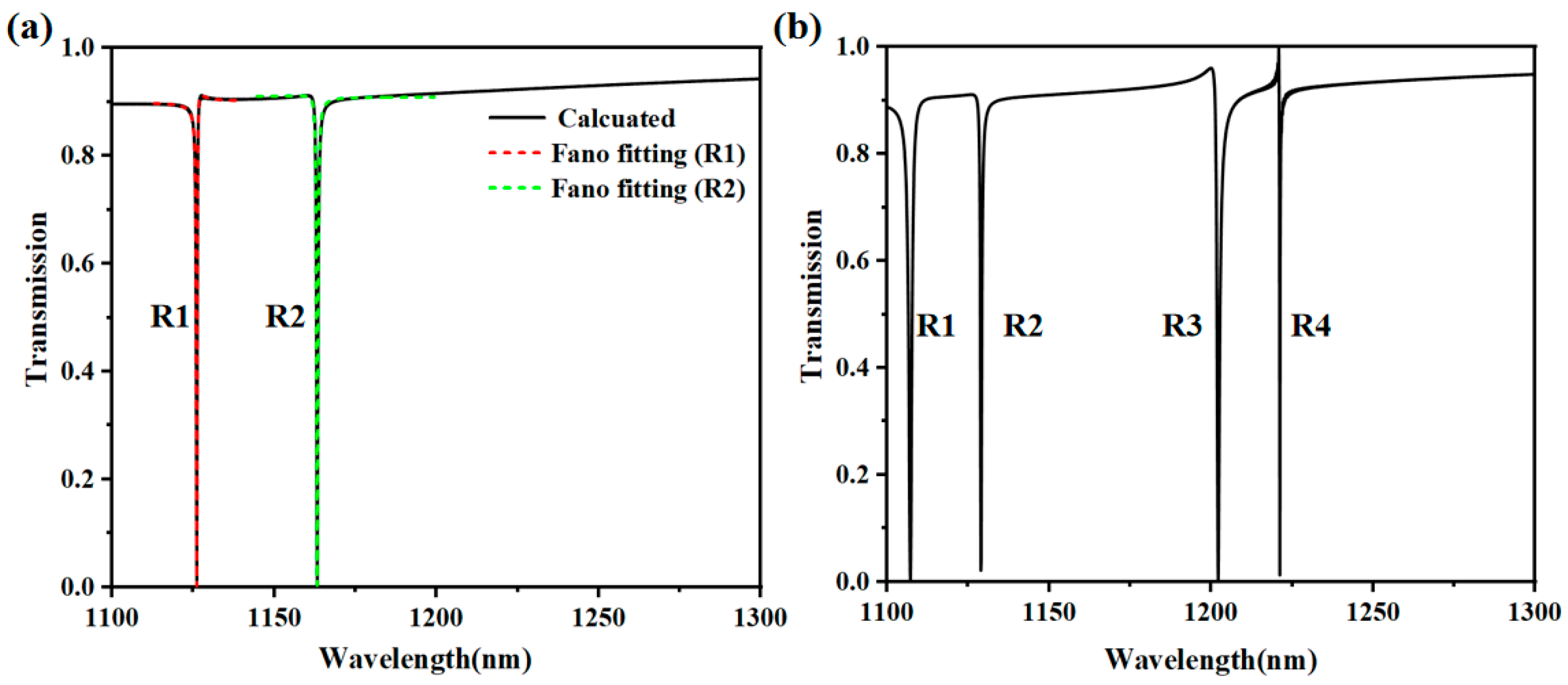
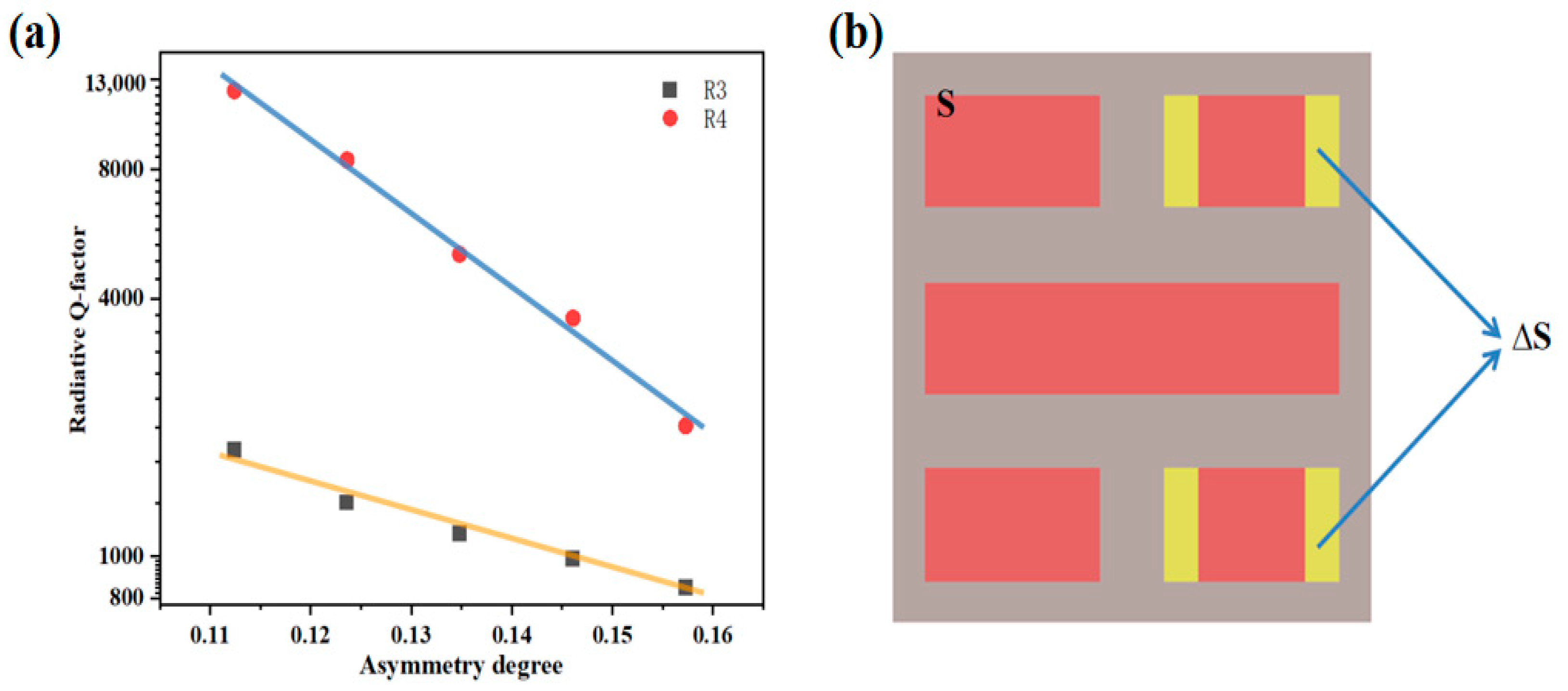
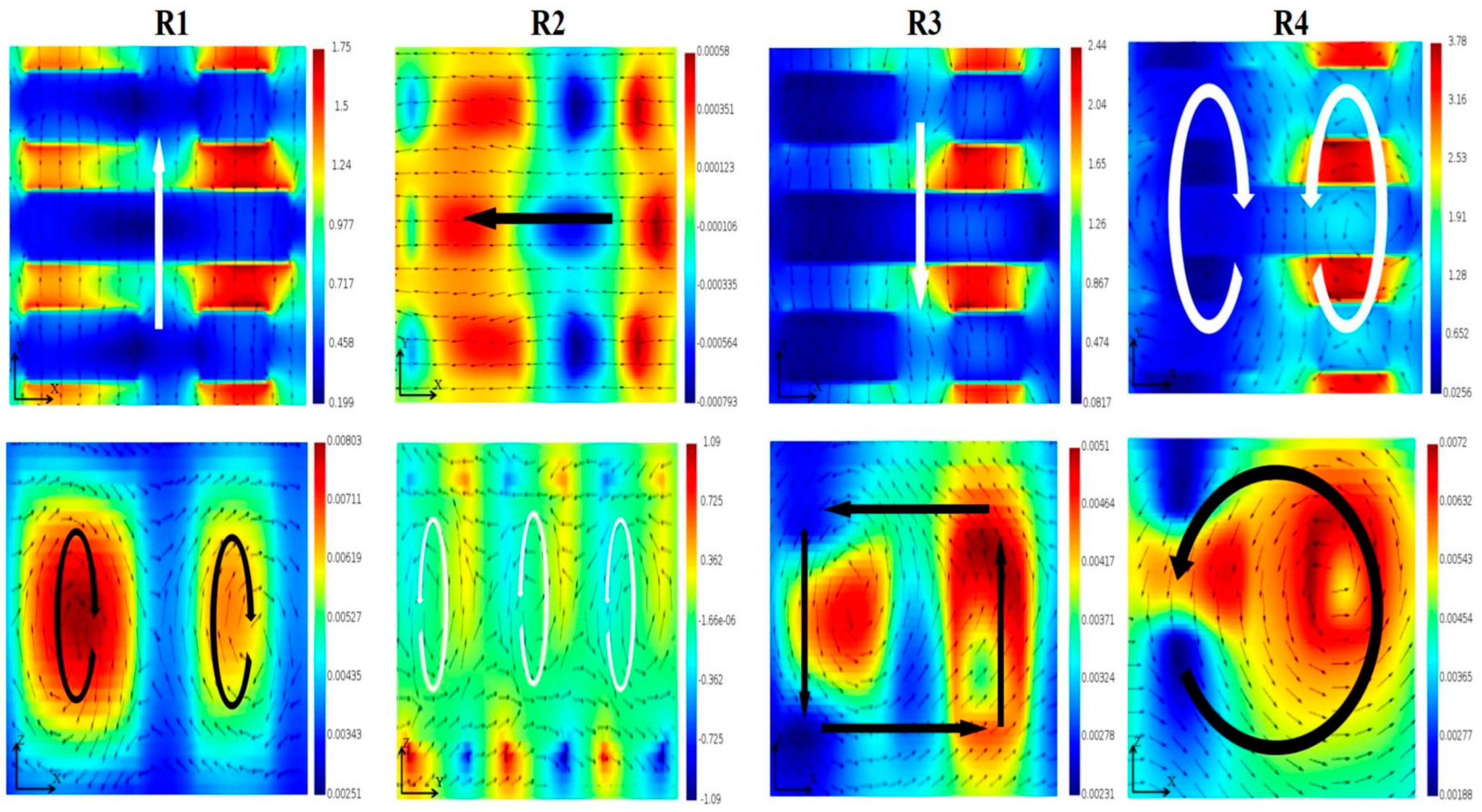
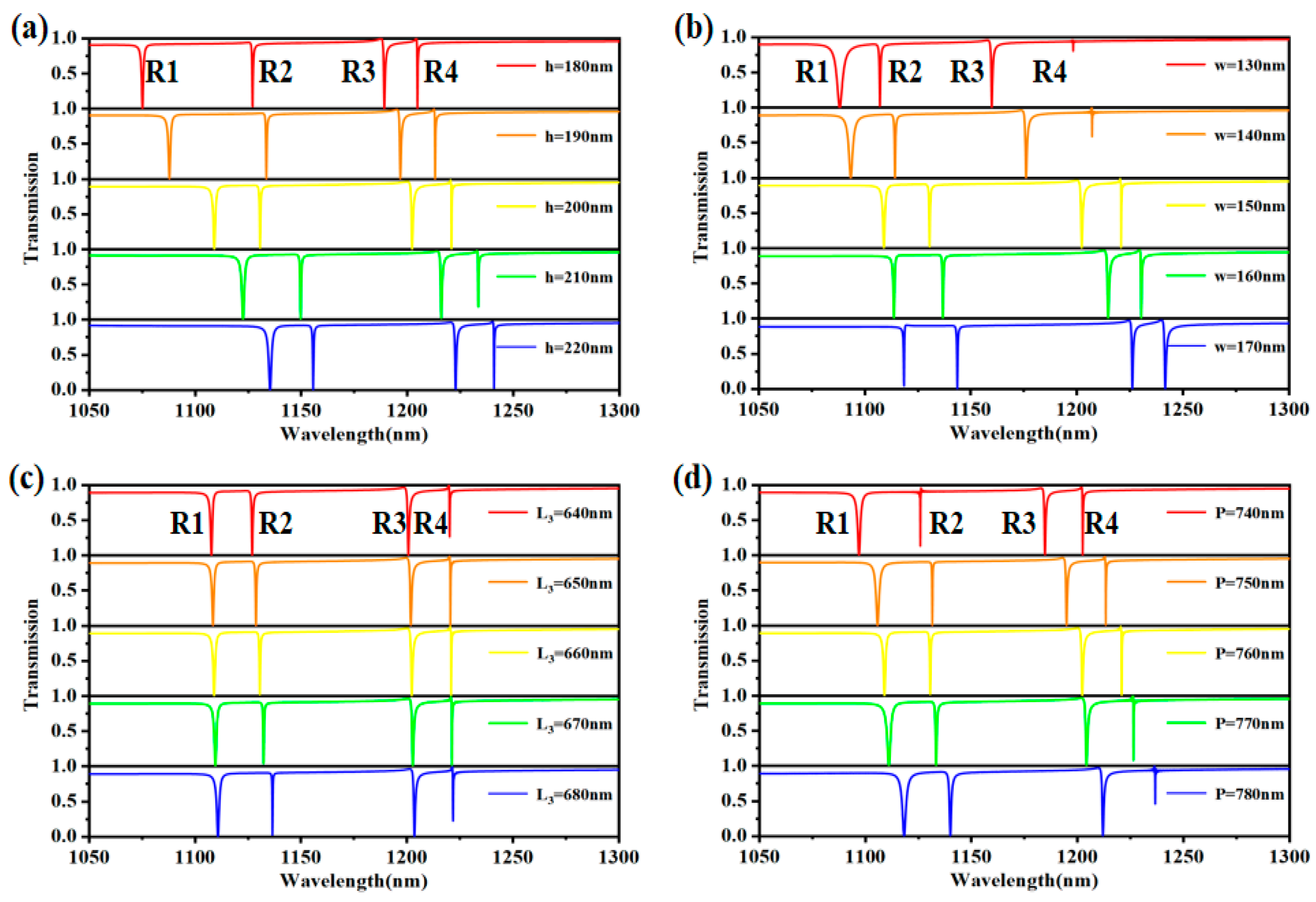
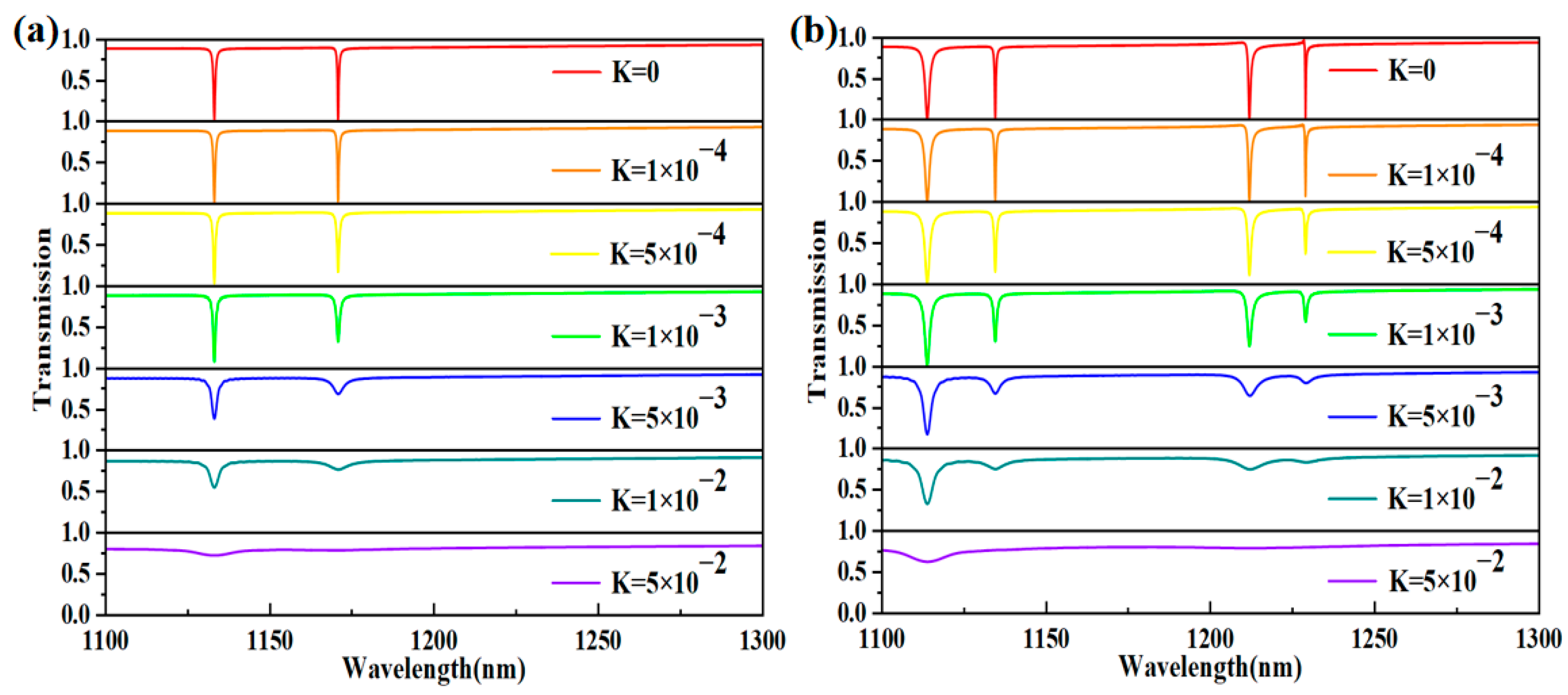
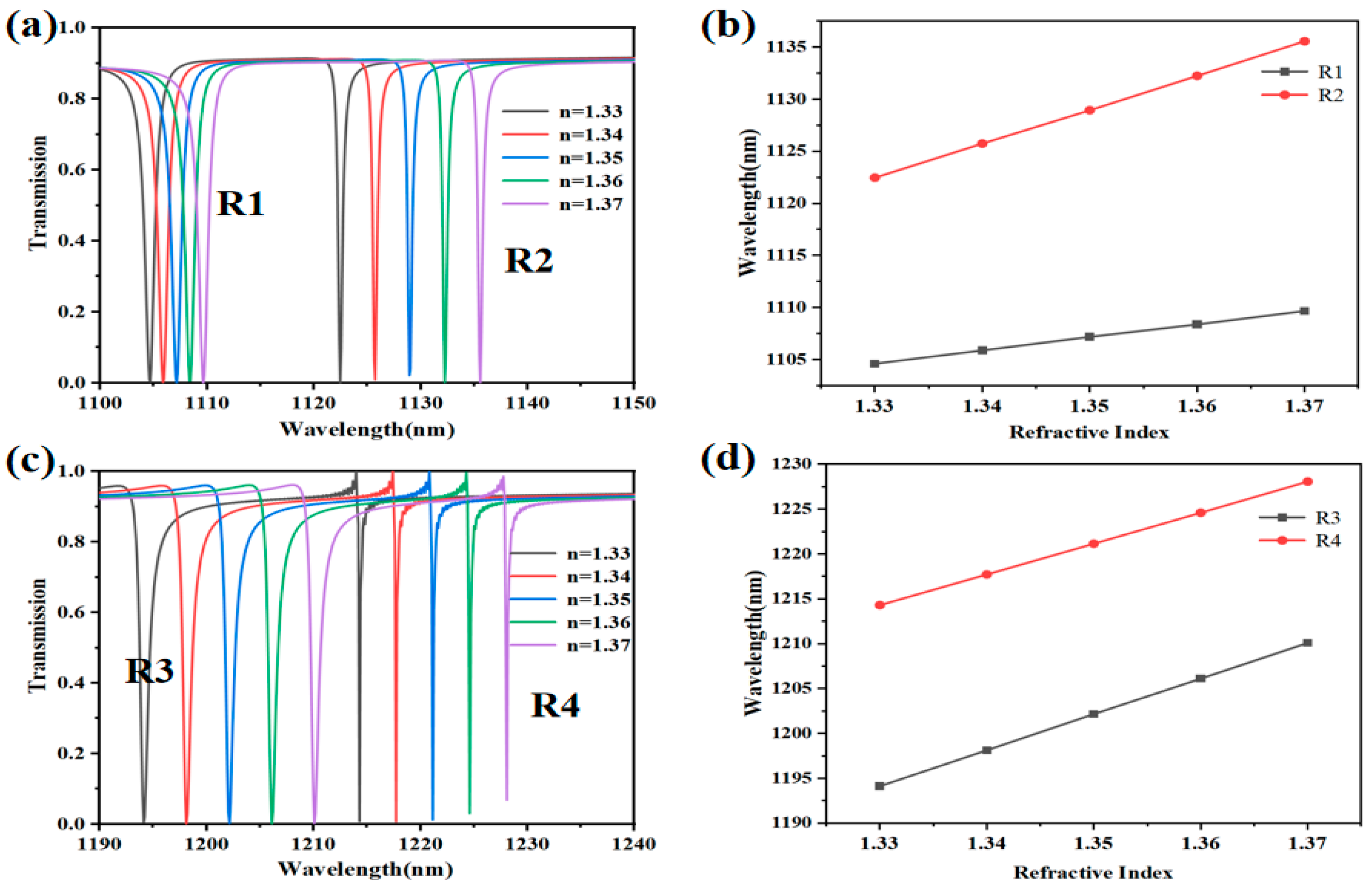
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, F.; Pu, M.; Gao, P.; Jin, J.; Li, X.; Guo, Y.; Ma, X.; Luo, J.; Hong, Y.; Luo, X. Simultaneous Full-Color Printing and Holography Enabled by Centimeter-Scale Plasmonic Metasurfaces. Adv. Sci. 2020, 7, 1903156. [Google Scholar] [CrossRef]
- Alhalaby, H.; Principe, M.; Zaraket, H.; Vaiano, P.; Aliberti, A.; Quero, G.; Crescitelli, A.; Di Meo, V.; Esposito, E.; Consales, M.; et al. Design and Optimization of All-Dielectric Fluorescence Enhancing Metasurfaces: Towards Advanced Metasurface-Assisted Optrodes. Biosensors 2022, 12, 264. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Liu, W.; Li, Z.; Li, Z.; Cheng, H.; Chen, S.; Tian, J. High-quality-factor multiple Fano resonances for refractive index sensing. Opt. Lett. 2018, 43, 1842–1845. [Google Scholar] [CrossRef] [PubMed]
- Song, S.; Yu, S.; Li, H.; Zhao, T. Ultra-high Q-factor toroidal dipole resonance and magnetic dipole quasi-bound state in the continuum in an all-dielectric hollow metasurface. Laser Phys. 2022, 32, 025403. [Google Scholar] [CrossRef]
- Gupta, M.; Srivastava, Y.K.; Singh, R. A Toroidal Metamaterial Switch. Adv. Mater. 2018, 30, 1704845. [Google Scholar] [CrossRef]
- Butet, J.; Martin, O.J. Fano resonances in the nonlinear optical response of coupled plasmonic nanostructures. Opt. Express 2014, 22, 29693–29707. [Google Scholar] [CrossRef]
- Xiao, S.; Qin, M.; Duan, J.; Liu, T. Robust enhancement of high-harmonic generation from all-dielectric metasurfaces enabled by polarization-insensitive bound states in the continuum. Opt. Express 2022, 30, 32590–32599. [Google Scholar] [CrossRef]
- Huang, L.; Li, H.; Yu, S.; Zhao, T. Analogue of electromagnetically induced transparency inspired by bound states in the continuum and toroidal dipolar response in all-dielectric metasurfaces. Photonic Nanostruct. 2022, 51, 101041. [Google Scholar] [CrossRef]
- Modi, K.S.; Kaur, J.; Singh, S.P.; Tiwari, U.; Sinha, R.K. Extremely high figure of merit in all-dielectric split asymmetric arc metasurface for refractive index sensing. Opt. Commun. 2020, 462, 125327. [Google Scholar] [CrossRef]
- Yang, H.; Chen, Y.; Liu, M.; Xiao, G.; Luo, Y.; Liu, H.; Li, J.; Yuan, L. High Q-Factor Hybrid Metamaterial Waveguide Multi-Fano Resonance Sensor in the Visible Wavelength Range. Nanomaterials 2021, 11, 1583. [Google Scholar] [CrossRef]
- Mohammadi, M.; Seifouri, M. Numerical investigation of photonic crystal ring resonators coupled bus waveguide as a highly sensitive platform. Photonic Nanostruct. 2019, 34, 11–18. [Google Scholar] [CrossRef]
- Zhou, Y.; Guo, Z.; Zhou, W.; Li, S.; Liu, Z.; Zhao, X.; Wu, X. High-Q guided mode resonance sensors based on shallow sub-wavelength grating structures. Nanotechnology 2020, 31, 325501. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Hu, C.; Jiang, J.H.; Wu, J.; Wen, W.; Hou, B. Photonic Type-III Nodal Loop and Topological Phase Transitions at Bilayer Metasurfaces. Front. Mate. 2022, 9, 909381. [Google Scholar] [CrossRef]
- Archetti, A.; Lin, R.J.; Restori, N.; Kiani, F.; Tsoulos, T.V.; Tagliabue, G. Thermally reconfigurable metalens. Nanophotonics 2022, 11, 3969–3980. [Google Scholar] [CrossRef] [PubMed]
- Fong, K.Y.; Pernice, W.H.; Tang, H.X. Frequency and phase noise of ultra-high Q silicon nitride nanomechanical resonators. Phys. Rev. B 2012, 85, 4506. [Google Scholar] [CrossRef]
- Ji, X.; Roberts, S.; Corato-Zanarella, M.; Lipson, M. Methods to achieve ultra-high quality factor silicon nitride resonators. APL Photonics 2021, 6, 071101. [Google Scholar] [CrossRef]
- YiYang, Y.; Liu, Y.; Yang, S.; Wu, Y.; Tian, H. Double-layered silicon-nitride photonic crystal slab guided-mode-resonance high-sensitivity sensor application for refractive index sensing and nanoparticle detection. J. Opt. Soc. Am. B 2021, 38, 1927–1933. [Google Scholar] [CrossRef]
- Guo, L.; Zhang, Z.; Xie, Q.; Li, W.; Xia, F.; Wang, M.; Feng, H.; You, C.; Yun, M. Toroidal dipole bound states in the continuum in all-dielectric metasurface for high-performance refractive index and temperature sensing. Appl. Surf. Sci 2023, 615, 156408. [Google Scholar] [CrossRef]
- Zhou, X.; Venkatachalam, S.; Zhou, R.; Xu, H.; Pokharel, A.; Fefferman, A.; Zaknoune, M.; Collin, E. High-Q silicon nitride drum resonators strongly coupled to gates. Nano Lett. 2021, 21, 5738–5744. [Google Scholar] [CrossRef]
- Frankis, H.C.; Kiani, K.M.; Su, D.; Mateman, R.; Leinse, A.; Bradley, J.D. High-Q tellurium-oxide-coated silicon nitride microring resonators. Opt Lett. 2019, 44, 118–121. [Google Scholar] [CrossRef]
- Spencer, D.T.; Bauters, J.F.; Heck, M.J.; Bowers, J.E. Integrated waveguide coupled Si3N4 resonators in the ultrahigh-Q regime. Optica 2014, 1, 153–157. [Google Scholar] [CrossRef]
- Ji, X.; Jang, J.K.; Dave, U.D.; Corato-Zanarella, M.; Joshi, C.; Gaeta, A.L.; Lipson, M. Exploiting Ultralow Loss Multimode Waveguides for Broadband Frequency Combs. Laser Photonics Rev. 2020, 15, 2000353. [Google Scholar] [CrossRef]
- Domeneguetti, R.R.; Zhao, Y.; Ji, X.; Martinelli, M.; Lipson, M.; Gaeta, A.L.; Nussenzveig, P. Parametric sideband generation in CMOS-compatible oscillators from visible to telecom wavelengths. Optica 2021, 8, 316–322. [Google Scholar] [CrossRef]
- Jin, W.; Yang, Q.F.; Chang, L.; Shen, B.; Wang, H.; Leal, M.A.; Wu, L.; Gao, M.; Feshali, A.; Paniccia, M.; et al. Hertz-linewidth semiconductor lasers using CMOS-ready ultra-high-Q microresonators. Nat. Photonics 2021, 15, 346–353. [Google Scholar] [CrossRef]
- Yu, F.; Chen, J.; Huang, L.; Zhao, Z.; Wang, J.; Jin, R.; Chen, J.; Wang, J.; Miroshnichenko, A.E.; Li, T.; et al. Photonic slide rule with metasurfaces. Light Sci. Appl. 2022, 11, 77. [Google Scholar] [CrossRef]
- Ye, Y.; Yu, S.; Li, H.; Gao, Z.; Yang, L.; Zhao, T. Triple Fano resonances metasurface and its extension for multi-channel ultra-narrow band absorber. Results Phys. 2022, 42, 106025. [Google Scholar] [CrossRef]
- Varasteanu, P.; Radoi, A.; Tutunaru, O.; Ficai, A.; Pascu, R.; Kusko, M.; Mihalache, I. Plasmon-Enhanced Photoresponse of Self-Powered Si Nanoholes Photodetector by Metal Nanowires. Nanomaterials 2021, 11, 2460. [Google Scholar] [CrossRef]
- Wang, W.; Zheng, L.; Xiong, L.; Qi, J.; Li, B. High Q-factor multiple Fano resonances for high-sensitivity sensing in all-dielectric metamaterials. OSA Continuum. 2019, 2, 2818. [Google Scholar] [CrossRef]
- Li, H.; Yu, S.; Yang, L.; Zhao, T. High Q-factor multi-Fano resonances in all-dielectric double square hollow metamaterials. Opt. Laser Technol. 2021, 140, 107072. [Google Scholar] [CrossRef]
- Biasco, S.; Beere, H.E.; Ritchie, D.A.; Li, L.; Davies, A.G.; Linfield, E.H.; Vitiello, M.S. Frequency-tunable continuous-wave random lasers at terahertz frequencies. Light Sci. Appl. 2019, 8, 43. [Google Scholar] [CrossRef]
- Yang, L.; Yu, S.; Li, H.; Zhao, T. Multiple Fano resonances excitation on all-dielectric nanohole arrays metasurfaces. Opt. Express 2021, 29, 14905–14916. [Google Scholar] [CrossRef]
- Fan, K.; Shadrivov, I.V.; Padilla, W.J. Dynamic bound states in the continuum. Optica 2019, 6, 169–173. [Google Scholar] [CrossRef]
- Chen, X.; Fan, W. Tunable Bound States in the Continuum in All-Dielectric Terahertz Metasurfaces. Nanomaterials 2020, 10, 623. [Google Scholar] [CrossRef]
- Mao, L.; Cheng, P.; Liu, K.; Lian, M.; Cao, T. Sieving nanometer enantiomers using bound states in the continuum from the metasurface. Nanoscale Adv. 2022, 4, 1617–1625. [Google Scholar] [CrossRef]
- Cerjan, A.; Jörg, C.; Vaidya, S.; Augustine, S.; Benalcazar, W.A.; Hsu, C.W.; von Freymann, G.; Rechtsman, M.C. Observation of bound states in the continuum embedded in symmetry bandgaps. Sci. Adv. 2021, 7, eabk1117. [Google Scholar] [CrossRef]
- Sadrieva, Z.; Frizyuk, K.; Petrov, M.; Kivshar, Y.; Bogdanov, A. Multipolar origin of bound states in the continuum. Phy. Rev. B 2019, 100, 115303. [Google Scholar] [CrossRef]
- Koshelev, K.; Lepeshov, S.; Liu, M.; Bogdanov, A.; Kivshar, Y. Asymmetric Metasurfaces with High-Q Resonances Governed by Bound States in the Continuum. Phys. Rev. Lett. 2018, 121, 193903. [Google Scholar] [CrossRef]
- Mi, Q.; Sang, T.; Pei, Y.; Yang, C.; Li, S.; Wang, Y.; Ma, B. High-quality-factor dual-band Fano resonances induced by dual bound states in the continuum using a planar nanohole slab. Nanoscale Res. Lett. 2021, 16, 150. [Google Scholar] [CrossRef]
- Leitis, A.; Tittl, A.; Liu, M.; Lee, B.H.; Gu, M.B.; Kivshar, Y.S.; Altug, H. Angle-multiplexed all-dielectric metasurfaces for broadband molecular fingerprint retrieval. Sci. Adv. 2019, 5, eaaw2817. [Google Scholar] [CrossRef]
- Reineke Matsudo, B.; Sain, B.; Carletti, L.; Zhang, X.; Gao, W.; de Angelis, C.; Huang, L.; Zentgraf, T. Efficient Frequency Conversion with Geometric Phase Control in Optical Metasurfaces. Adv. Sci. 2022, 9, e2104508. [Google Scholar] [CrossRef]
- Abbas, M.A.; Zubair, A.; Riaz, K.; Huang, W.; Teng, J.; Mehmood, M.Q.; Zubair, M. Engineering multimodal dielectric resonance of TiO2 based nanostructures for high-performance refractive index sensing applications. Opt. Express 2020, 28, 23509–23522. [Google Scholar] [CrossRef] [PubMed]
- Liu, G.D.; Zhai, X.; Wang, L.L.; Lin, Q.; Xia, S.X.; Luo, X.; Zhao, C.J. A High-Performance Refractive Index Sensor Based on Fano Resonance in Si Split-Ring Metasurface. Plasmonics 2018, 13, 15–19. [Google Scholar] [CrossRef]
- Qin, M.; Pan, C.; Chen, Y.; Ma, Q.; Liu, S.; Wu, E.; Wu, B. Electromagnetically Induced Transparency in All-Dielectric U-Shaped Silicon Metamaterials. Appl. Sci. 2018, 8, 1799. [Google Scholar] [CrossRef]
- Zhang, Q.; Wen, X.; Li, G.; Ruan, Q.; Wang, J.; Xiong, Q. Multiple magnetic mode-based Fano resonance in split-ring resonator/disk nanocavities. ACS Nano 2013, 7, 11071–11078. [Google Scholar] [CrossRef]
- Romano, S.; Zito, G.; Torino, S.; Calafiore, G.; Penzo, E.; Coppola, G.; Cabrini, S.; Rendina, I.; Mocella, V. Label-free sensing of ultralow-weight molecules with all-dielectric metasurfaces supporting bound states in the continuum. Photonics Res. 2018, 6, 726–733. [Google Scholar] [CrossRef]
| Sensor Type | Number of Resonance Peaks | Ref. | ||
|---|---|---|---|---|
| Double square hollow | 4 | 287.5 | 389 | [29] |
| U-shaped silicon cylinder | 1 | 203 | 29 | [43] |
| Split-ring disk | 2 | 282 | 4 | [44] |
| Optical sensor based on a photonic crystal metasurface | 2 | 178 | 445 | [45] |
| Bilayer Silicon Nitride Photonic Crystal Sensor | 1 | 937.64 | n.r. a | [17] |
| All-dielectric metasurface based on a silicon nitride substrate | 2 | 746 | 18650 | [18] |
| Five rectangular blocks of silicon | 4 | 403 | 2400 | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.; Fan, X.; Fang, W.; Cao, S.; Sun, Q.; Wang, D.; Niu, H.; Li, C.; Wei, X.; Bai, C.; et al. Investigation of Multiple High Quality-Factor Fano Resonances in Asymmetric Nanopillar Arrays for Optical Sensing. Photonics 2024, 11, 68. https://doi.org/10.3390/photonics11010068
Chen H, Fan X, Fang W, Cao S, Sun Q, Wang D, Niu H, Li C, Wei X, Bai C, et al. Investigation of Multiple High Quality-Factor Fano Resonances in Asymmetric Nanopillar Arrays for Optical Sensing. Photonics. 2024; 11(1):68. https://doi.org/10.3390/photonics11010068
Chicago/Turabian StyleChen, Huawei, Xinye Fan, Wenjing Fang, Shuangshuang Cao, Qinghe Sun, Dandan Wang, Huijuan Niu, Chuanchuan Li, Xin Wei, Chenglin Bai, and et al. 2024. "Investigation of Multiple High Quality-Factor Fano Resonances in Asymmetric Nanopillar Arrays for Optical Sensing" Photonics 11, no. 1: 68. https://doi.org/10.3390/photonics11010068
APA StyleChen, H., Fan, X., Fang, W., Cao, S., Sun, Q., Wang, D., Niu, H., Li, C., Wei, X., Bai, C., & Kumar, S. (2024). Investigation of Multiple High Quality-Factor Fano Resonances in Asymmetric Nanopillar Arrays for Optical Sensing. Photonics, 11(1), 68. https://doi.org/10.3390/photonics11010068







