Generation of Narrow Modes in Random Raman Fiber Laser Based on Multimode Fiber
Abstract
:1. Introduction
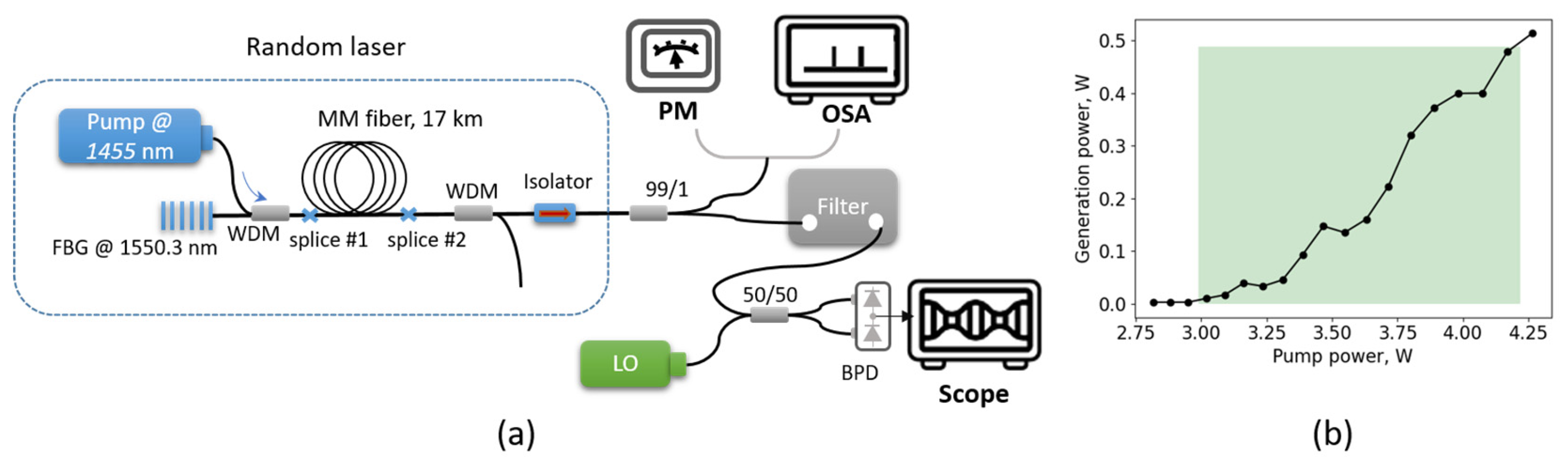
2. Materials and Methods
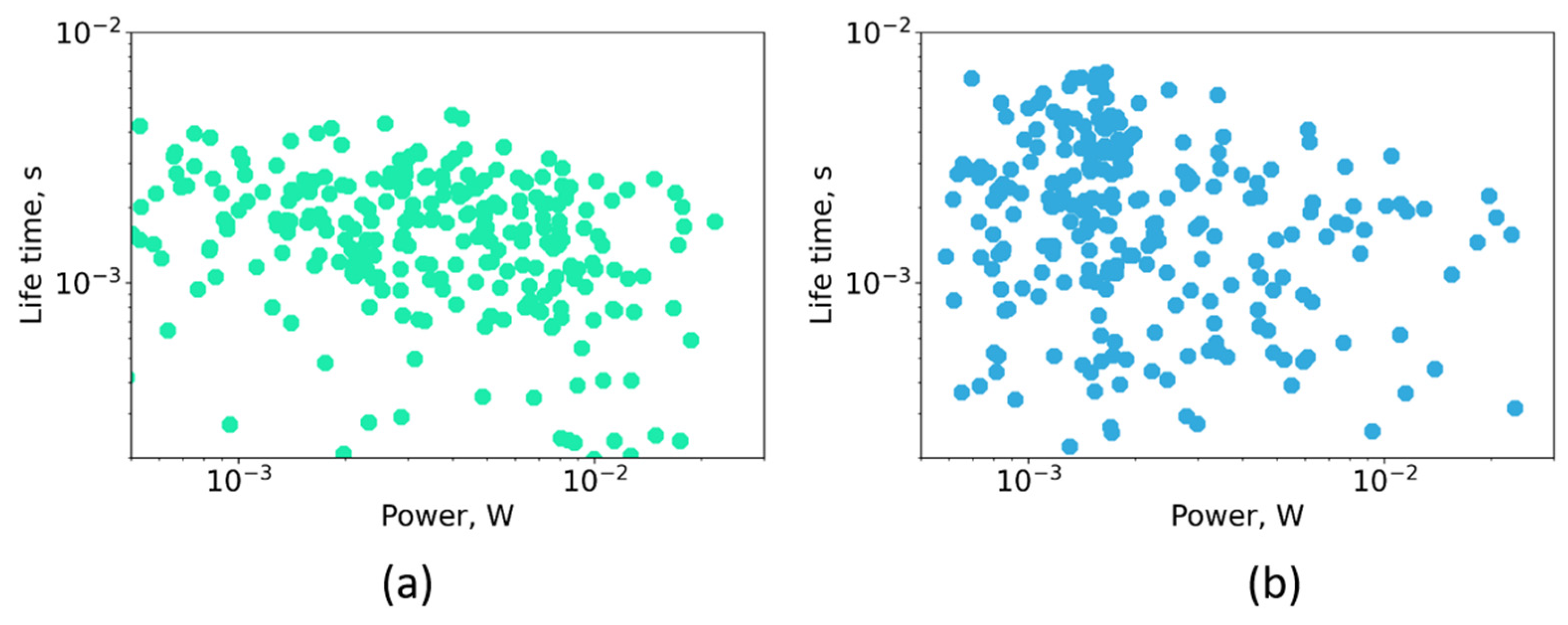
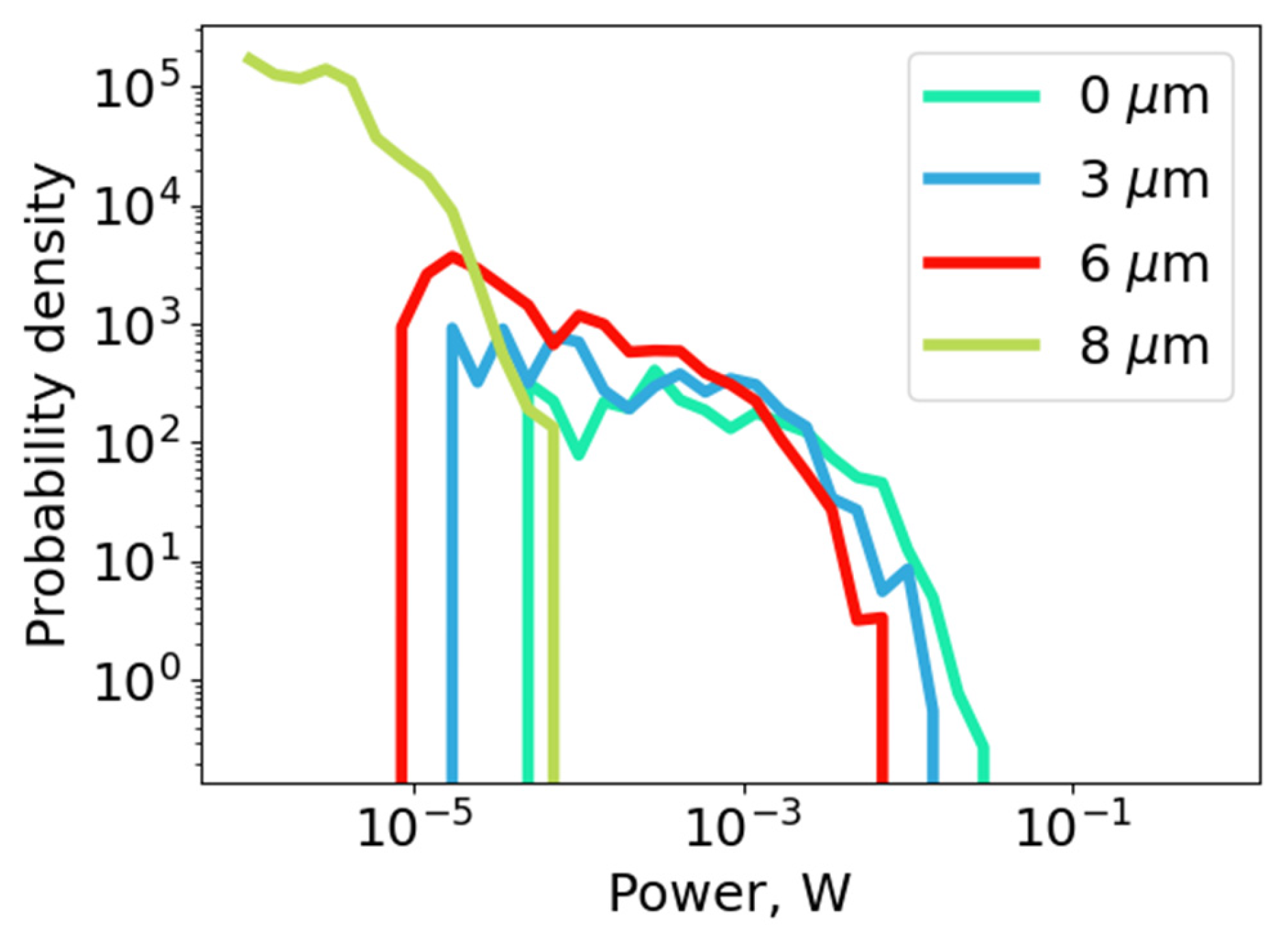
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gomes, A.S.L.; Moura, A.L.; de Araújo, C.B.; Raposo, E.P. Recent Advances and Applications of Random Lasers and Random Fiber Lasers. Prog. Quantum Electron. 2021, 78, 100343. [Google Scholar] [CrossRef]
- Lawandy, N.M.; Balachandran, R.M.; Gomes, A.S.L.; Sauvain, E. Laser Action in Strongly Scattering Media. Nature 1994, 368, 436–438. [Google Scholar] [CrossRef]
- Leong, E.S.P.; Chong, M.K.; Yu, S.F.; Pita, K. Sol–Gel ZnO–SiO2 Composite Waveguide Ultraviolet Lasers. IEEE Photonics Technol. Lett. 2004, 16, 2418–2420. [Google Scholar] [CrossRef]
- Noginov, M.A. Solid-State Random Lasers; Springer Series in Optical Sciences; Springer: New York, NY, USA, 2005; Volume 105, ISBN 0-387-23913-8. [Google Scholar]
- de Matos, C.; Menezes, L.D.S.; Brito-Silva, A.; Martinez Gámez, M.; Gomes, A.S.L.; de Araújo, C. Random Fiber Laser. Phys. Rev. Lett. 2007, 99, 153903. [Google Scholar] [CrossRef] [PubMed]
- Gagné, M.; Kashyap, R. Demonstration of a 3 MW Threshold Er-Doped Random Fiber Laser Based on a Unique Fiber Bragg Grating. Opt. Express 2009, 17, 19067. [Google Scholar] [CrossRef] [PubMed]
- Wiersma, D.S. The Physics and Applications of Random Lasers. Nat. Phys. 2008, 4, 359–367. [Google Scholar] [CrossRef]
- Bliokh, Y.; Chaikina, E.I.; Lizárraga, N.N.; Méndez, E.R.; Freilikher, V.; Nori, F. Disorder-Induced Cavities, Resonances, and Lasing in Randomly Layered Media. Phys. Rev. B 2012, 86, 054204. [Google Scholar] [CrossRef]
- Lizárraga, N.; Puente, N.P.; Chaikina, E.I.; Leskova, T.A.; Méndez, E.R. Single-Mode Er-Doped Fiber Random Laser with Distributed Bragg Grating Feedback. Opt. Express 2009, 17, 395. [Google Scholar] [CrossRef]
- Popov, S.M.; Butov, O.V.; Bazakutsa, A.P.; Vyatkin, M.Y.; Chamorovskii, Y.K.; Fotiadi, A.A. Random Lasing in a Short Er-Doped Artificial Rayleigh Fiber. Results Phys. 2020, 16, 102868. [Google Scholar] [CrossRef]
- Niu, Y.; Jia, P.; Su, J.; Wang, J.; An, G.; Ren, Q.; Xiong, J. Tunable Random Fiber Laser Based on Dual-Grating Structure. Photonics 2023, 10, 644. [Google Scholar] [CrossRef]
- Deng, J.; Li, Y.; Shen, M.; Shu, X. Single-Frequency Random Distributed Bragg Reflector Fiber Laser. J. Light. Technol. 2022, 40, 4385–4390. [Google Scholar] [CrossRef]
- Yoshino, T. Simple Theoretical Approach for Spectral Properties of Rayleigh Backscattering Feedback Fibre Lasers. Electron. Lett. 2011, 47, 1040. [Google Scholar] [CrossRef]
- Yin, G.; Saxena, B.; Bao, X. Tunable Er-Doped Fiber Ring Laser with Single Longitudinal Mode Operation Based on Rayleigh Backscattering in Single Mode Fiber. Opt. Express 2011, 19, 25981. [Google Scholar] [CrossRef] [PubMed]
- Pang, Y.; Xu, Y.; Zhao, X.; Qin, Z.; Liu, Z. Stabilized Narrow-Linewidth Brillouin Random Fiber Laser with a Double-Coupler Fiber Ring Resonator. J. Light. Technol. 2022, 40, 2988–2995. [Google Scholar] [CrossRef]
- de Matos, C.J.S.; Popov, S.V.; Taylor, J.R. Short-Pulse, All-Fiber, Raman Laser with Dispersion Compensation in a Holey Fiber. Opt. Lett. 2003, 28, 1891–1893. [Google Scholar] [CrossRef] [PubMed]
- Spirin, V.V.; Bueno Escobedo, J.L.; Korobko, D.A.; Mégret, P.; Fotiadi, A.A. Stabilizing DFB Laser Injection-Locked to an External Fiber-Optic Ring Resonator. Opt. Express 2020, 28, 478. [Google Scholar] [CrossRef]
- Skvortsov, M.I.; Abdullina, S.R.; Podivilov, E.V.; Vlasov, A.A.; Kharasov, D.R.; Fomiryakov, E.A.; Nikitin, S.P.; Treshchikov, V.N.; Babin, S.A. Extreme Narrowing of the Distributed Feedback Fiber Laser Linewidth due to the Rayleigh Backscattering in a Single-Mode Fiber: Model and Experimental Test. Photonics 2022, 9, 590. [Google Scholar] [CrossRef]
- Tovar, P.; von der Weid, J.P.; Wang, Y.; Chen, L.; Bao, X. A Random Optical Parametric Oscillator. Nat. Commun. 2023, 14, 6664. [Google Scholar] [CrossRef]
- Fan, M.; Wang, Z.; Wu, H.; Sun, W.; Zhang, L. Low-Threshold, High-Efficiency Random Fiber Laser with Linear Output. IEEE Photonics Technol. Lett. 2015, 27, 319–322. [Google Scholar] [CrossRef]
- Liu, W.; Ma, P.; Zhou, P. Unified Model for Spectral and Temporal Properties of Quasi-CW Fiber Lasers. J. Opt. Soc. Am. B 2021, 38, 3663. [Google Scholar] [CrossRef]
- Wang, P.; Lin, S.; Zhang, J.; Bao, X.; Ni, L.; Qi, Y.; Wang, Z. Efficient 1054 nm Raman Random Fiber Laser. Photonics 2023, 10, 851. [Google Scholar] [CrossRef]
- Tovar, P.; von der Weid, J.P. Dynamic Evolution of Narrow Spectral Modes in Stochastic Brillouin Random Fiber Lasers. IEEE Photonics Technol. Lett. 2021, 33, 1471–1474. [Google Scholar] [CrossRef]
- Tovar, P.; Temporão, G.; von der Weid, J.P. Longitudinal Mode Dynamics in SOA-Based Random Feedback Fiber Lasers. Opt. Express 2019, 27, 31001. [Google Scholar] [CrossRef] [PubMed]
- Lima, B.C.; Tovar, P.; Moura, A.L.; von der Weid, J.P. Replica Symmetry Breaking in a Rayleigh Backscattering-Based Random Fiber Laser. J. Opt. Soc. Am. B 2023, 40, 1680. [Google Scholar] [CrossRef]
- Kirik, A.E.; Vatnik, I.D.; Churkin, D.V. Direct Measurements of Localized Spectral Modes in Random Distributed Feedback Fiber Laser. Results Phys. 2021, 28, 104651. [Google Scholar] [CrossRef]
- Lima, B.C.; Tovar, P.; von der Weid, J.P. Generalized Extreme-Value Distribution of Maximum Intensities and Lévy-like Behavior in an SOA-Based Random Feedback Laser Emission. J. Opt. Soc. Am. B 2020, 37, 987. [Google Scholar] [CrossRef]
- Bliokh, Y.; Chaikina, E.I.I.; Vatnik, I.D.D.; Churkin, D.V. Temporal Variation of the Spectrum of a Continuously Pumped Random Fiber Laser: Phenomenological Model. J. Opt. Soc. Am. B 2019, 36, 408. [Google Scholar] [CrossRef]
- Fotiadi, A.A. Random Lasers: An Incoherent Fibre Laser. Nat. Photonics 2010, 4, 204–205. [Google Scholar] [CrossRef]
- Boschetti, A.; Taschin, A.; Bartolini, P.; Tiwari, A.K.; Pattelli, L.; Torre, R.; Wiersma, D.S. Spectral Super-Resolution Spectroscopy Using a Random Laser. Nat. Photonics 2020, 14, 177–182. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, S.; Zheng, C.; Zhang, W. Coherent Random Fiber Laser-Enabled Super-Resolution Spectroscopy. ACS Photonics 2023, 10, 2670–2678. [Google Scholar] [CrossRef]
- Babin, S.A.; Churkin, D.V.; Ismagulov, A.E.; Kablukov, S.I.; Podivilov, E.V. Spectral Broadening in Raman Fiber Lasers. Opt. Lett. 2006, 31, 3007–3009. [Google Scholar] [CrossRef] [PubMed]
- Kablukov, S.I.; Zlobina, E.A.; Podivilov, E.V.; Babin, S.A. Output Spectrum of Yb-Doped Fiber Lasers. Opt. Lett. 2012, 37, 2508. [Google Scholar] [CrossRef] [PubMed]
- Babin, S.A.; Dontsova, E.I.; Kablukov, S.I. Random Fiber Laser Directly Pumped by a High-Power Laser Diode. Opt. Lett. 2013, 38, 3301–3303. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Fan, C.; Yao, T.; Xiao, H.; Leng, J.; Zhou, P.; Nemov, I.N.; Kuznetsov, A.G.; Babin, S.A. Brightness Enhancement in Random Raman Fiber Laser Based on a Graded-Index Fiber with High-Power Multimode Pumping. Opt. Lett. 2021, 46, 1185. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.-H.; Haig, H.; Wu, Y.; Ziegler, Z.; Wise, F. Accurate Modeling of Ultrafast Nonlinear Pulse Propagation in Multimode Gain Fiber. J. Opt. Soc. Am. B 2023, 40, 2633. [Google Scholar] [CrossRef]
- Dostovalov, A.; Wolf, A.; Munkueva, Z.; Skvortsov, M.; Abdullina, S.; Kuznetsov, A.; Babin, S. Continuous and Discrete-Point Rayleigh Reflectors Inscribed by Femtosecond Pulses in Singlemode and Multimode Fibers. Opt. Laser Technol. 2023, 167, 109692. [Google Scholar] [CrossRef]
- Murray, M.J.; Davis, A.; Redding, B. Multimode Fiber Φ-OTDR with Holographic Demodulation. Opt. Express 2018, 26, 23019. [Google Scholar] [CrossRef]
- Wright, L.G.; Wu, F.O.; Christodoulides, D.N.; Wise, F.W. Physics of Highly Multimode Nonlinear Optical Systems. Nat. Phys. 2022, 18, 1018–1030. [Google Scholar] [CrossRef]
- Yablon, A.D. Optical Fiber Fusion Splicing; Springer Series in Optical Sciences; Springer: Berlin/Heidelberg, Germany, 2005; Volume 103, ISBN 978-3-540-23104-2. [Google Scholar]
- Hurand, S.; Chauny, L.-A.; El-Rabii, H.; Joshi, S.; Yalin, A.P. Mode Coupling and Output Beam Quality of 100–400 Μm Core Silica Fibers. Appl. Opt. 2011, 50, 492. [Google Scholar] [CrossRef]
- Röhrer, C.; Kleem, G.; Ahmed, M.A.; Graf, T. Analysis of Fundamental-Mode Beam Transport in Highly Multimode Fibers. J. Light. Technol. 2017, 35, 3637–3642. [Google Scholar] [CrossRef]
- Kharenko, D.S.; Gervaziev, M.D.; Kuznetsov, A.G.; Podivilov, E.V.; Wabnitz, S.; Babin, S.A. Mode-Resolved Analysis of Pump and Stokes Beams in LD-Pumped GRIN Fiber Raman Lasers. Opt. Lett. 2022, 47, 1222. [Google Scholar] [CrossRef] [PubMed]
- Sidelnikov, O.S.; Kuznetsov, A.G.; Kharenko, D.S.; Gervaziev, M.D.; Podivilov, E.V.; Fedoruk, M.P.; Wabnitz, S.; Babin, S.A. Transverse Mode Distribution in Multimode Diode-Pumped Raman Fiber Laser. J. Opt. Soc. Am. B 2023, 40, 3269. [Google Scholar] [CrossRef]
- Turitsyn, S.K.; Babin, S.A.; Churkin, D.V.; Vatnik, I.D.; Nikulin, M.; Podivilov, E.V. Random Distributed Feedback Fibre Lasers. Phys. Rep. 2014, 542, 133–193. [Google Scholar] [CrossRef]
- Kirik, A.E.; Aprelov, N.A.; Vatnik, I.D.; Churkin, D.V. Observation of Cascade Brillouin Processes in Random Distributed Feedback Raman Fiber Lasers. In Proceedings of the Quantum and Nonlinear Optics IX; He, Q., Li, C.-F., Kim, D.-S., Eds.; SPIE: Bellingham, WA, USA, 2023; p. 23. [Google Scholar]
- Mocofanescu, A.; Wang, L.; Jain, R.; Shaw, K.D.; Gavrielides, A.; Peterson, P.; Sharma, M.P. SBS Threshold for Single Mode and Multimode GRIN Fibers in an All Fiber Configuration. Opt. Express 2005, 13, 2019. [Google Scholar] [CrossRef]
- Popoff, S.M.; Gostev, P. Simple Module to Find Numerically Propagation Mode Profiles and Propagation Constants of Multimode Fibers of Arbitrary Radial Index Profiles. 2023. Available online: https://zenodo.org/records/5524192 (accessed on 1 December 2023).
- Terry, N.B.; Alley, T.G.; Russell, T.H.; Engel, K.T. An Explanation of SRS Beam Cleanup in Graded-Index Fibers and the Absence of SRS Beam Cleanup in Step-Index Fibers. Opt. Express 2007, 15, 17509. [Google Scholar] [CrossRef]
- Zlobina, E.A.; Kablukov, S.I.; Wolf, A.A.; Nemov, I.N.; Dostovalov, A.V.; Tyrtyshnyy, V.A.; Myasnikov, D.V.; Babin, S.A. Generating High-Quality Beam in a Multimode LD-Pumped All-Fiber Raman Laser. Opt. Express 2017, 25, 12581. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vatnik, I.D.; Gorbunov, O.A.; Churkin, D.V. Generation of Narrow Modes in Random Raman Fiber Laser Based on Multimode Fiber. Photonics 2024, 11, 2. https://doi.org/10.3390/photonics11010002
Vatnik ID, Gorbunov OA, Churkin DV. Generation of Narrow Modes in Random Raman Fiber Laser Based on Multimode Fiber. Photonics. 2024; 11(1):2. https://doi.org/10.3390/photonics11010002
Chicago/Turabian StyleVatnik, Ilya D., Oleg A. Gorbunov, and Dmitry V. Churkin. 2024. "Generation of Narrow Modes in Random Raman Fiber Laser Based on Multimode Fiber" Photonics 11, no. 1: 2. https://doi.org/10.3390/photonics11010002
APA StyleVatnik, I. D., Gorbunov, O. A., & Churkin, D. V. (2024). Generation of Narrow Modes in Random Raman Fiber Laser Based on Multimode Fiber. Photonics, 11(1), 2. https://doi.org/10.3390/photonics11010002




