Light Confinement in Twisted Single-Layer 2D+ Moiré Photonic Crystals and Bilayer Moiré Photonic Crystals
Abstract
:1. Introduction
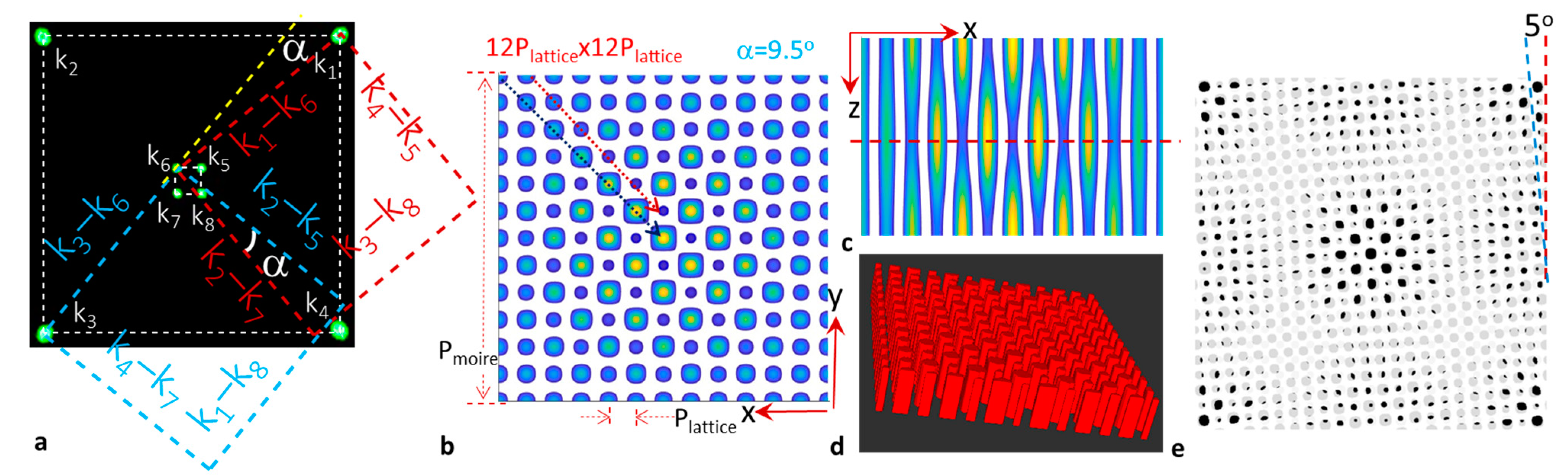
2. Concepts and Simulation Methods
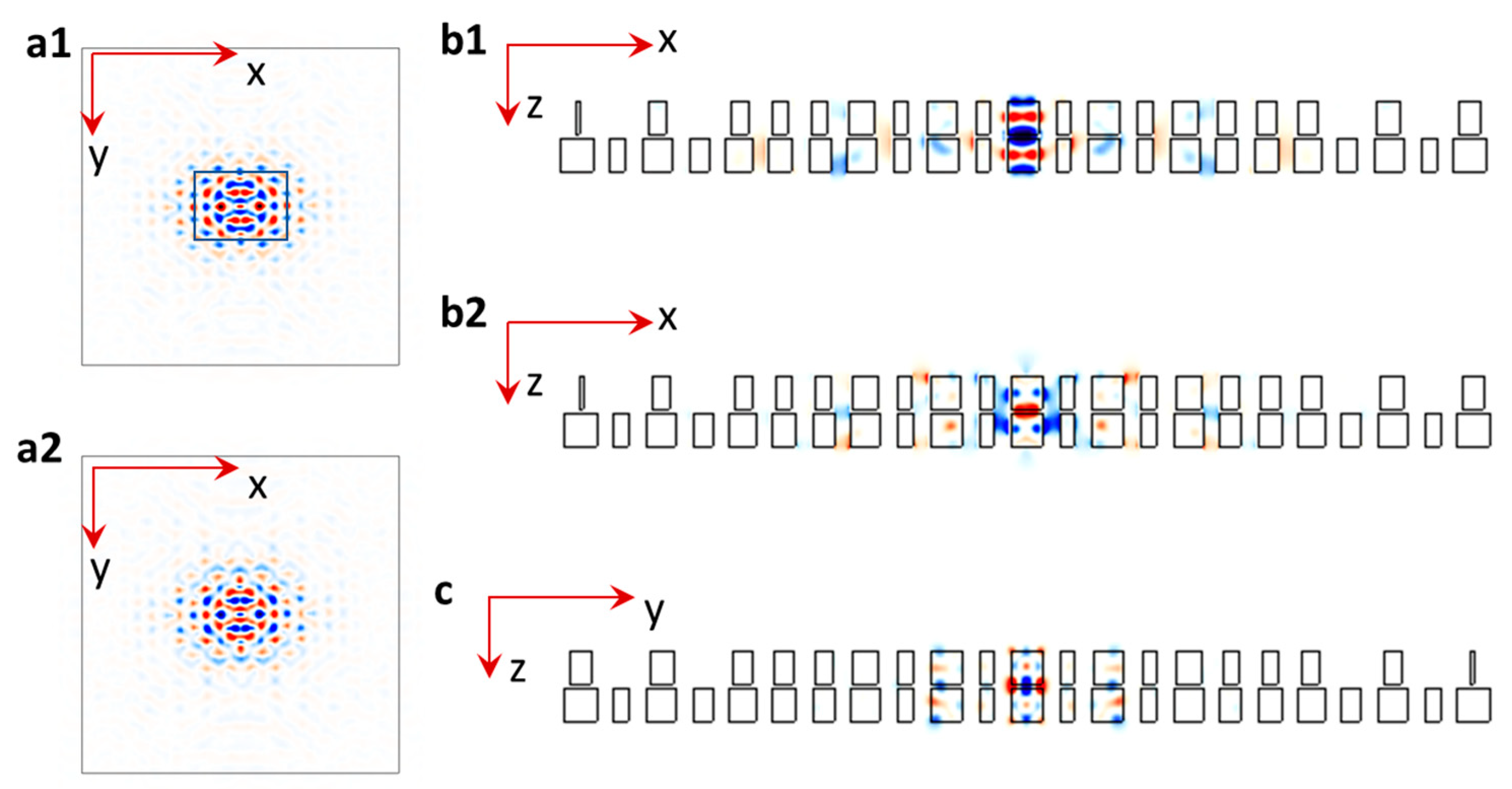
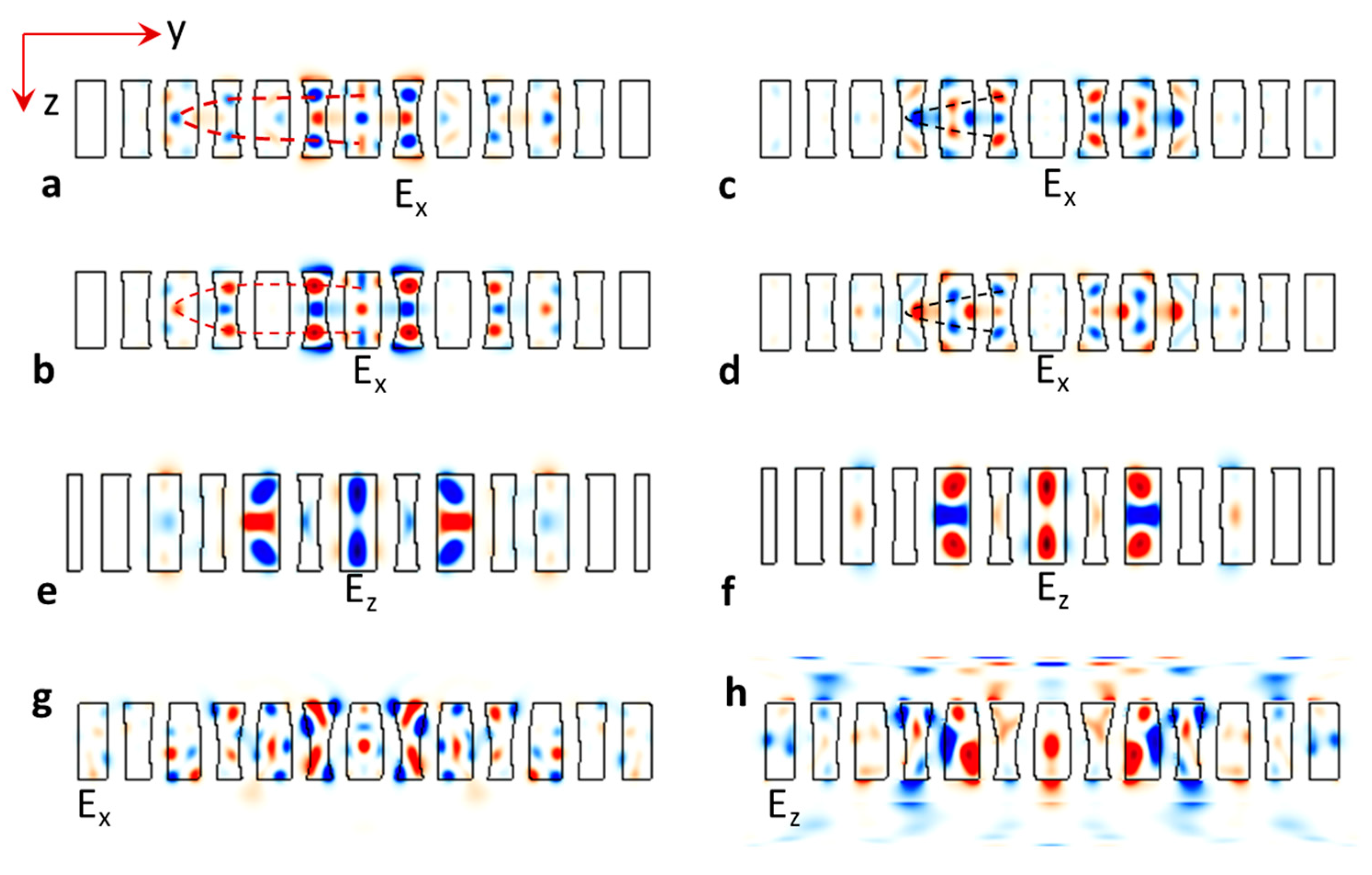
3. Results of Light Confinement in Bilayer Moiré-Over-Moiré PhCs
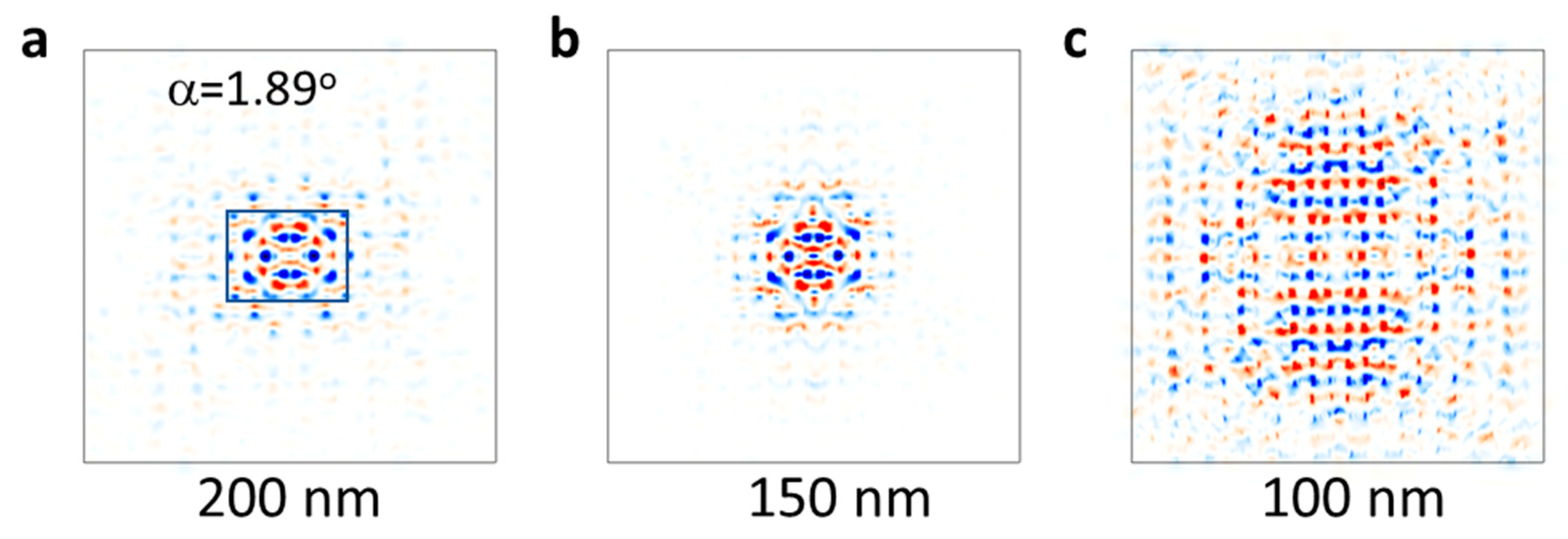
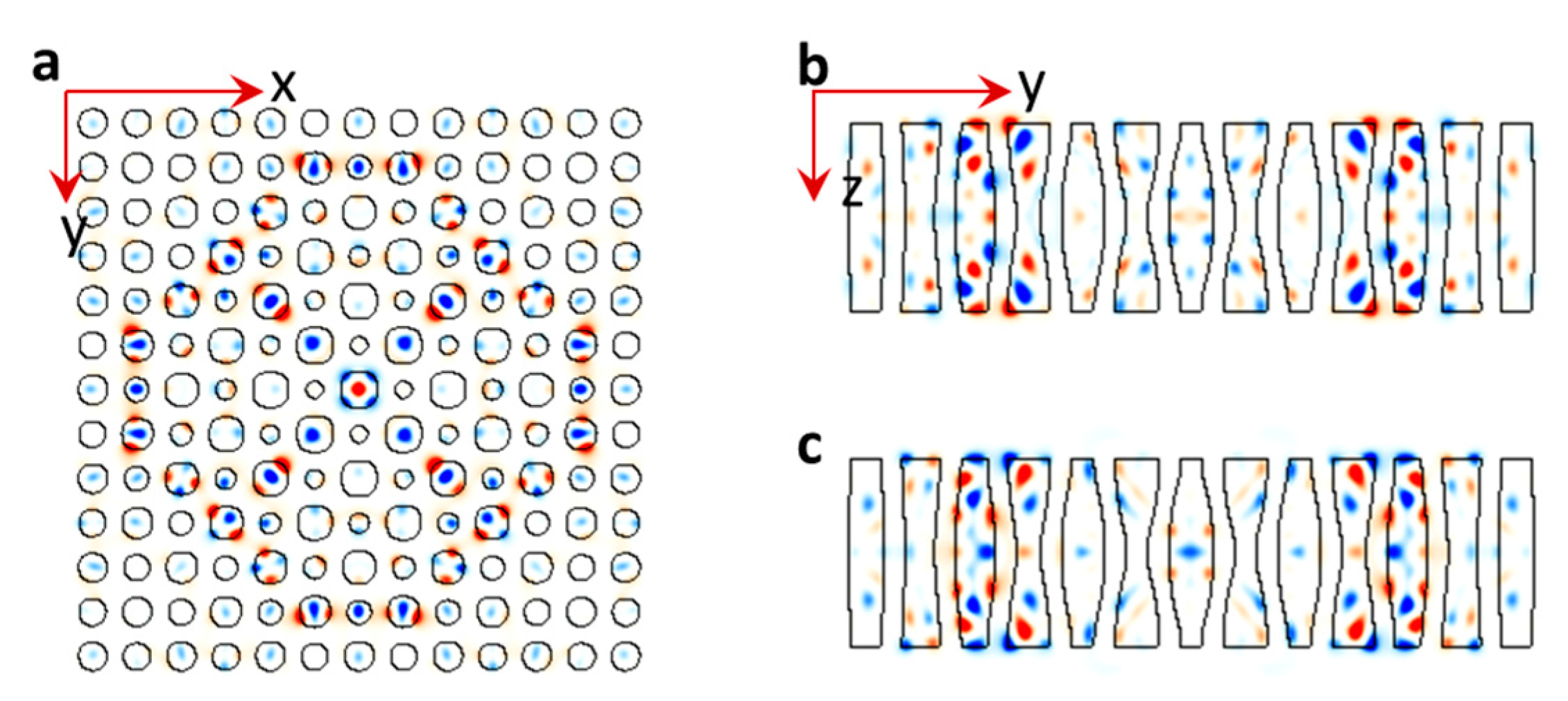
4. Results of Light Confinement in 2D+ Moiré Silicon PhCs
5. Discussion
6. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Cao, Y.; Fatemi, V.; Fang, S.; Watanabe, K.; Taniguchi, T.; Kaxiras, E.; Jarillo-Herrero, P. Unconventional Superconductivity in Magic-Angle Graphene Superlattices. Nature 2018, 556, 43–50. [Google Scholar] [CrossRef]
- Yankowitz, M.; Chen, S.; Polshyn, H.; Zhang, Y.; Watanabe, K.; Taniguchi, T.; Graf, D.; Young, A.F.; Dean, C.R. Tuning Superconductivity in Twisted Bilayer Graphene. Science 2019, 363, 1059–1064. [Google Scholar] [CrossRef] [PubMed]
- Balents, L.; Dean, C.R.; Efetov, D.K.; Young, A.F. Superconductivity and Strong Correlations in Moiré Flat Bands. Nat. Phys. 2020, 16, 725–733. [Google Scholar] [CrossRef]
- Arora, H.S.; Polski, R.; Zhang, Y.; Thomson, A.; Choi, Y.; Kim, H.; Lin, Z.; Wilson, I.Z.; Xu, X.; Chu, J.-H.; et al. Superconductivity in Metallic Twisted Bilayer Graphene Stabilized by WSe2. Nature 2020, 583, 379–384. [Google Scholar] [CrossRef] [PubMed]
- González, J.; Stauber, T. Kohn-Luttinger Superconductivity in Twisted Bilayer Graphene. Phys. Rev. Lett. 2019, 122, 026801. [Google Scholar] [CrossRef] [PubMed]
- Uri, A.; de la Barrera, S.C.; Randeria, M.T.; Rodan-Legrain, D.; Devakul, T.; Crowley, P.J.D.; Paul, N.; Watanabe, K.; Taniguchi, T.; Lifshitz, R.; et al. Superconductivity and Strong Interactions in a Tunable Moiré Quasicrystal. Nature 2023, 620, 762–767. [Google Scholar] [CrossRef]
- Uchida, K.; Furuya, S.; Iwata, J.-I.; Oshiyama, A. Atomic Corrugation and Electron Localization Due to Moiré Patterns in Twisted Bilayer Graphenes. Phys. Rev. B 2014, 90, 155451. [Google Scholar] [CrossRef]
- Sunku, S.S.; Ni, G.X.; Jiang, B.Y.; Yoo, H.; Sternbach, A.; McLeod, A.S.; Stauber, T.; Xiong, L.; Taniguchi, T.; Watanabe, K.; et al. Photonic Crystals for Nano-Light in Moiré Graphene Superlattices. Science 2018, 362, 1153–1156. [Google Scholar] [CrossRef]
- Wu, F.; Lovorn, T.; Tutuc, E.; Martin, I.; MacDonald, A.H. Topological Insulators in Twisted Transition Metal Dichalcogenide Homobilayers. Phys. Rev. Lett. 2019, 122, 086402. [Google Scholar] [CrossRef]
- Bai, Y.; Zhou, L.; Wang, J.; Wu, W.; McGilly, L.J.; Halbertal, D.; Lo, C.F.B.; Liu, F.; Ardelean, J.; Rivera, P.; et al. Excitons in Strain-Induced One-Dimensional Moiré Potentials at Transition Metal Dichalcogenide Heterojunctions. Nat. Mater. 2020, 19, 1068–1073. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, Y.; Watanabe, K.; Taniguchi, T.; Ueno, K.; Tutuc, E.; LeRoy, B.J. Flat Bands in Twisted Bilayer Transition Metal Dichalcogenides. Nat. Phys. 2020, 16, 1093–1096. [Google Scholar] [CrossRef]
- Naik, M.H.; Jain, M. Ultraflatbands and Shear Solitons in Moiré Patterns of Twisted Bilayer Transition Metal Dichalcogenides. Phys. Rev. Lett. 2018, 121, 266401. [Google Scholar] [CrossRef] [PubMed]
- Mullan, C.; Slizovskiy, S.; Yin, J.; Wang, Z.; Yang, Q.; Xu, S.; Yang, Y.; Piot, B.A.; Hu, S.; Taniguchi, T.; et al. Mixing of Moiré-Surface and Bulk States in Graphite. Nature 2023, 620, 756–761. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.; Ma, S.; Shao, R.; Zhang, L.; Yan, T.; Ma, Q.; Zhang, S.; Cui, T.J. Moiré Metasurfaces for Dynamic Beamforming. Sci. Adv. 2022, 8, eabo1511. [Google Scholar] [CrossRef] [PubMed]
- Hu, G.; Wang, M.; Mazor, Y.; Qiu, C.-W.; Alù, A. Tailoring Light with Layered and Moiré Metasurfaces. Trends Chem. 2021, 3, 342–358. [Google Scholar] [CrossRef]
- Hu, G.; Krasnok, A.; Mazor, Y.; Qiu, C.-W.; Alù, A. Moiré Hyperbolic Metasurfaces. Nano Lett. 2020, 20, 3217–3224. [Google Scholar] [CrossRef] [PubMed]
- Alnasser, K.; Kamau, S.; Hurley, N.; Cui, J.; Lin, Y. Resonance Modes in Moiré Photonic Patterns for Twistoptics. OSA Contin. 2021, 4, 1339. [Google Scholar] [CrossRef]
- Duan, J.; Capote-Robayna, N.; Taboada-Gutiérrez, J.; Álvarez-Pérez, G.; Prieto, I.; Martín-Sánchez, J.; Nikitin, A.Y.; Alonso-González, P. Twisted Nano-Optics: Manipulating Light at the Nanoscale with Twisted Phonon Polaritonic Slabs. Nano Lett. 2020, 20, 5323–5329. [Google Scholar] [CrossRef]
- Lin, H.-M.; Lu, Y.-H.; Chang, Y.-J.; Yang, Y.-Y.; Jin, X.-M. Direct Observation of a Localized Flat-Band State in a Mapped Moiré Hubbard Photonic Lattice. Phys. Rev. Appl. 2022, 18, 054012. [Google Scholar] [CrossRef]
- Dong, K.; Zhang, T.; Li, J.; Wang, Q.; Yang, F.; Rho, Y.; Wang, D.; Grigoropoulos, C.P.; Wu, J.; Yao, J. Flat Bands in Magic-Angle Bilayer Photonic Crystals at Small Twists. Phys. Rev. Lett. 2021, 126, 223601. [Google Scholar] [CrossRef]
- Yi, C.-H.; Park, H.C.; Park, M.J. Strong Interlayer Coupling and Stable Topological Flat Bands in Twisted Bilayer Photonic Moiré Superlattices. Light Sci. Appl. 2022, 11, 289. [Google Scholar] [CrossRef] [PubMed]
- Mao, X.-R.; Shao, Z.-K.; Luan, H.-Y.; Wang, S.-L.; Ma, R.-M. Magic-Angle Lasers in Nanostructured Moiré Superlattice. Nat. Nanotechnol. 2021, 16, 1099–1105. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, D.X.; Letartre, X.; Drouard, E.; Viktorovitch, P.; Nguyen, H.C.; Nguyen, H.S. Magic Configurations in Moiré Superlattice of Bilayer Photonic Crystals: Almost-Perfect Flatbands and Unconventional Localization. Phys. Rev. Res. 2022, 4, L032031. [Google Scholar] [CrossRef]
- Wang, H.; Ma, S.; Zhang, S.; Lei, D. Intrinsic Superflat Bands in General Twisted Bilayer Systems. Light Sci. Appl. 2022, 11, 159. [Google Scholar] [CrossRef] [PubMed]
- Zhou, J.; Xie, S.; Nie, C.; Xu, P.; Yi, J.; Liu, E. Optical Properties of a Moiré-Lattice Photonic Crystal Fiber with Controllable Magic Angle. Results Phys. 2023, 51, 106659. [Google Scholar] [CrossRef]
- Tang, H.; Lou, B.; Du, F.; Zhang, M.; Ni, X.; Xu, W.; Jin, R.; Fan, S.; Mazur, E. Experimental Probe of Twist Angle–Dependent Band Structure of on-Chip Optical Bilayer Photonic Crystal. Sci. Adv. 2023, 9, eadh8498. [Google Scholar] [CrossRef] [PubMed]
- Lou, B.; Wang, B.; Rodríguez, J.A.; Cappelli, M.; Fan, S. Tunable Guided Resonance in Twisted Bilayer Photonic Crystal. Sci. Adv. 2022, 8, eadd4339. [Google Scholar] [CrossRef]
- Lou, B.; Zhao, N.; Minkov, M.; Guo, C.; Orenstein, M.; Fan, S. Theory for Twisted Bilayer Photonic Crystal Slabs. Phys. Rev. Lett. 2021, 126, 136101. [Google Scholar] [CrossRef]
- Huang, L.; Zhang, W.; Zhang, X. Moiré Quasibound States in the Continuum. Phys. Rev. Lett. 2022, 128, 253901. [Google Scholar] [CrossRef]
- Hu, G.; Zheng, C.; Ni, J.; Qiu, C.-W.; Alù, A. Enhanced Light-Matter Interactions at Photonic Magic-Angle Topological Transitions. Appl. Phys. Lett. 2021, 118, 211101. [Google Scholar] [CrossRef]
- Krasnok, A.; Alù, A. Low-Symmetry Nanophotonics. ACS Photonics 2022, 9, 2–24. [Google Scholar] [CrossRef]
- Chen, J.; Lin, X.; Chen, M.; Low, T.; Chen, H.; Dai, S. A Perspective of Twisted Photonic Structures. Appl. Phys. Lett. 2021, 119, 240501. [Google Scholar] [CrossRef]
- Wu, Z.; Zheng, Y. Moiré Chiral Metamaterials. Adv. Opt. Mater. 2017, 5, 1700034. [Google Scholar] [CrossRef]
- Lou, B.; Fan, S. Tunable frequency filter based on twisted bilayer photonic crystal slabs. ACS Photonics 2022, 9, 800–805. [Google Scholar] [CrossRef]
- Zhang, T.; Dong, K.; Li, J.; Meng, F.; Li, J.; Munagavalasa, S.; Grigoropoulos, C.P.; Wu, J.; Yao, J. Twisted Moiré Photonic Crystal Enabled Optical Vortex Generation through Bound States in the Continuum. Nat. Commun. 2023, 14, 6014. [Google Scholar] [CrossRef] [PubMed]
- Meng, Z.; Wang, L.; Han, W.; Liu, F.; Wen, K.; Gao, C.; Wang, P.; Chin, C.; Zhang, J. Atomic Bose–Einstein condensate in twisted-bilayer optical lattices. Nature 2023, 615, 231–236. [Google Scholar] [CrossRef] [PubMed]
- Tang, H.; Du, F.; Carr, S.; DeVault, C.; Mello, O.; Mazur, E. Modeling the Optical Properties of Twisted Bilayer Photonic Crystals. Light Sci. Appl. 2021, 10, 157. [Google Scholar] [CrossRef] [PubMed]
- Tang, H.; Ni, X.; Du, F.; Srikrishna, V.; Mazur, E. On-Chip Light Trapping in Bilayer Moiré Photonic Crystal Slabs. Appl. Phys. Lett. 2022, 121, 231702. [Google Scholar] [CrossRef]
- Zeng, J.; Hu, Y.; Zhang, X.; Fu, S.; Yin, H.; Li, Z.; Chen, Z. Localization-to-Delocalization Transition of Light in Frequency-Tuned Photonic Moiré Lattices. Opt. Express 2021, 29, 25388. [Google Scholar] [CrossRef]
- Fu, Q.; Wang, P.; Huang, C.; Kartashov, Y.V.; Torner, L.; Konotop, V.V.; Ye, F. Optical Soliton Formation Controlled by Angle Twisting in Photonic Moiré Lattices. Nat. Photonics 2020, 14, 663–668. [Google Scholar] [CrossRef]
- Talukdar, T.H.; Hardison, A.L.; Ryckman, J.D. Moiré Effects in Silicon Photonic Nanowires. ACS Photonics 2022, 9, 1286–1294. [Google Scholar] [CrossRef]
- Zhou, X.; Lin, Z.; Lu, W.; Lai, Y.; Hou, B.; Jiang, J. Twisted Quadrupole Topological Photonic Crystals. Laser Photon Rev. 2020, 14, 2000010. [Google Scholar] [CrossRef]
- Shang, C.; Lu, C.; Tang, S.; Gao, Y.; Wen, Z. Generation of Gradient Photonic Moiré Lattice Fields. Opt. Express 2021, 29, 29116. [Google Scholar] [CrossRef] [PubMed]
- Han, J.-H.; Kim, I.; Ryu, J.-W.; Kim, J.; Cho, J.-H.; Yim, G.-S.; Park, H.-S.; Min, B.; Choi, M. Rotationally Reconfigurable Metamaterials Based on Moiré Phenomenon. Opt. Express 2015, 23, 17443. [Google Scholar] [CrossRef] [PubMed]
- Wang, P.; Zheng, Y.; Chen, X.; Huang, C.; Kartashov, Y.V.; Torner, L.; Konotop, V.V.; Ye, F. Localization and Delocalization of Light in Photonic Moiré Lattices. Nature 2020, 577, 42–46. [Google Scholar] [CrossRef] [PubMed]
- Kartashov, Y.V.; Ye, F.; Konotop, V.V.; Torner, L. Multifrequency Solitons in Commensurate-Incommensurate Photonic Moiré Lattices. Phys. Rev. Lett. 2021, 127, 163902. [Google Scholar] [CrossRef] [PubMed]
- Khurgin, J.B. Light Slowing down in Moiré Fiber Gratings and Its Implications for Nonlinear Optics. Phys. Rev. A 2000, 62, 013821. [Google Scholar] [CrossRef]
- Alnasser, K.; Li, S.; Sidhik, S.; Kamau, S.; Hou, J.; Hurley, N.; Alzaid, A.; Wang, S.; Yan, H.; Deng, J.; et al. Fabrication of Twisted Moire Photonic Crystal and Random Moire Photonic Crystal and Their Potential Applications in Light Extraction. Nanotechnology 2024, 35, 025203. [Google Scholar] [CrossRef]
- Lowell, D.; Lutkenhaus, J.; George, D.; Philipose, U.; Chen, B.; Lin, Y. Simultaneous Direct Holographic Fabrication of Photonic Cavity and Graded Photonic Lattice with Dual Periodicity, Dual Basis, and Dual Symmetry. Opt. Express 2017, 25, 14444. [Google Scholar] [CrossRef]
- Lowell, D.; Hassan, S.; Adewole, M.; Philipose, U.; Chen, B.; Lin, Y. Holographic Fabrication of Graded Photonic Super-Crystals Using an Integrated Spatial Light Modulator and Reflective Optical Element Laser Projection System. Appl. Opt. 2017, 56, 9888. [Google Scholar] [CrossRef]
- Ohlinger, K.; Lutkenhaus, J.; Arigong, B.; Zhang, H.; Lin, Y. Spatially Addressable Design of Gradient Index Structures through Spatial Light Modulator Based Holographic Lithography. J. Appl. Phys. 2013, 114, 213102. [Google Scholar] [CrossRef]
- Hurley, N.; Kamau, S.; Alnasser, K.; Philipose, U.; Cui, J.; Lin, Y. Laser Diffraction Zones and Spots from Three-Dimensional Graded Photonic Super-Crystals and Moiré Photonic Crystals. Photonics 2022, 9, 395. [Google Scholar] [CrossRef]
- Hurley, N.; Kamau, S.; Cui, J.; Lin, Y. Holographic Fabrication of 3D Moiré Photonic Crystals Using Circularly Polarized Laser Beams and a Spatial Light Modulator. Micromachines 2023, 14, 1217. [Google Scholar] [CrossRef] [PubMed]
- Gorgulu, K.; Gok, A.; Yilmaz, M.; Topalli, K.; Bıyıklı, N.; Okyay, A.K. All-Silicon Ultra-Broadband Infrared Light Absorbers. Sci. Rep. 2016, 6, 38589. [Google Scholar] [CrossRef]
- Oskooi, A.F.; Roundy, D.; Ibanescu, M.; Bermel, P.; Joannopoulos, J.D.; Johnson, S.G. MEEP: A Flexible Free-Software Package for Electromagnetic Simulations by the FDTD Method. Comput. Phys. Commun. 2010, 181, 687–702. [Google Scholar] [CrossRef]
- Luan, H.Y.; Ouyang, Y.H.; Zhao, Z.W.; Mao, W.Z.; Ma, R.M. Reconfigurable moiré nanolaser arrays with phase synchronization. Nature 2023, 624, 282–288. [Google Scholar] [CrossRef]
- Tétreault, N.; Freymann, G.V.; Deubel, M.; Hermatschweiler, M.; Willard, F.P.; John, S.; Wegener, M.; Ozin, G.A. New route to three-dimensional photonic bandgap materials: Silicon double inversion of polymer templates. Adv. Mater. 2006, 18, 457. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kamau, S.; Hurley, N.; Kaul, A.B.; Cui, J.; Lin, Y. Light Confinement in Twisted Single-Layer 2D+ Moiré Photonic Crystals and Bilayer Moiré Photonic Crystals. Photonics 2024, 11, 13. https://doi.org/10.3390/photonics11010013
Kamau S, Hurley N, Kaul AB, Cui J, Lin Y. Light Confinement in Twisted Single-Layer 2D+ Moiré Photonic Crystals and Bilayer Moiré Photonic Crystals. Photonics. 2024; 11(1):13. https://doi.org/10.3390/photonics11010013
Chicago/Turabian StyleKamau, Steve, Noah Hurley, Anupama B. Kaul, Jingbiao Cui, and Yuankun Lin. 2024. "Light Confinement in Twisted Single-Layer 2D+ Moiré Photonic Crystals and Bilayer Moiré Photonic Crystals" Photonics 11, no. 1: 13. https://doi.org/10.3390/photonics11010013
APA StyleKamau, S., Hurley, N., Kaul, A. B., Cui, J., & Lin, Y. (2024). Light Confinement in Twisted Single-Layer 2D+ Moiré Photonic Crystals and Bilayer Moiré Photonic Crystals. Photonics, 11(1), 13. https://doi.org/10.3390/photonics11010013







