Comparison of the Real Part of Dielectric Constants with Different Materials to Decrease the Emittance and a Virtual Dielectric Constant to Reproduce Reflectance
Abstract
:1. Introduction
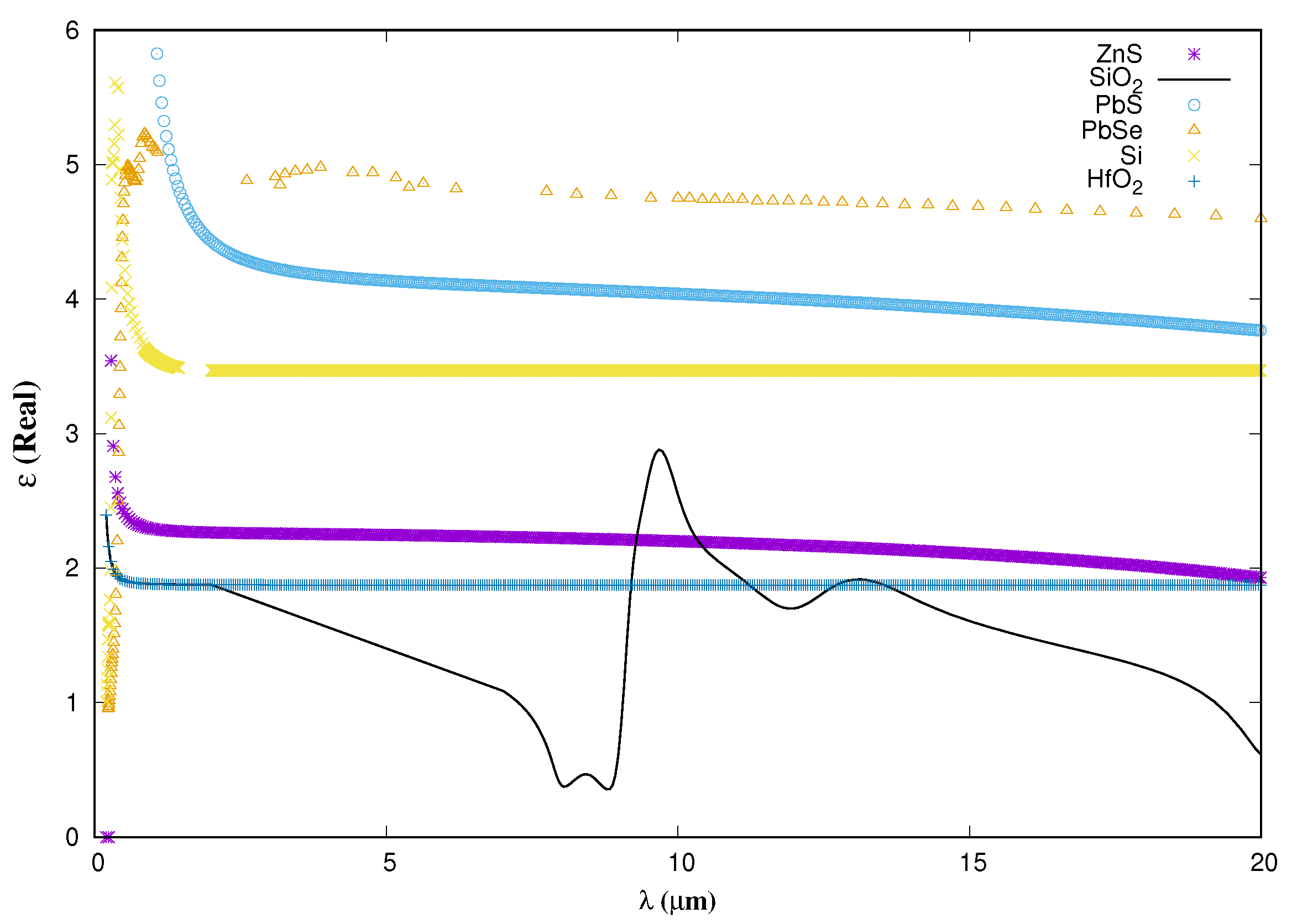
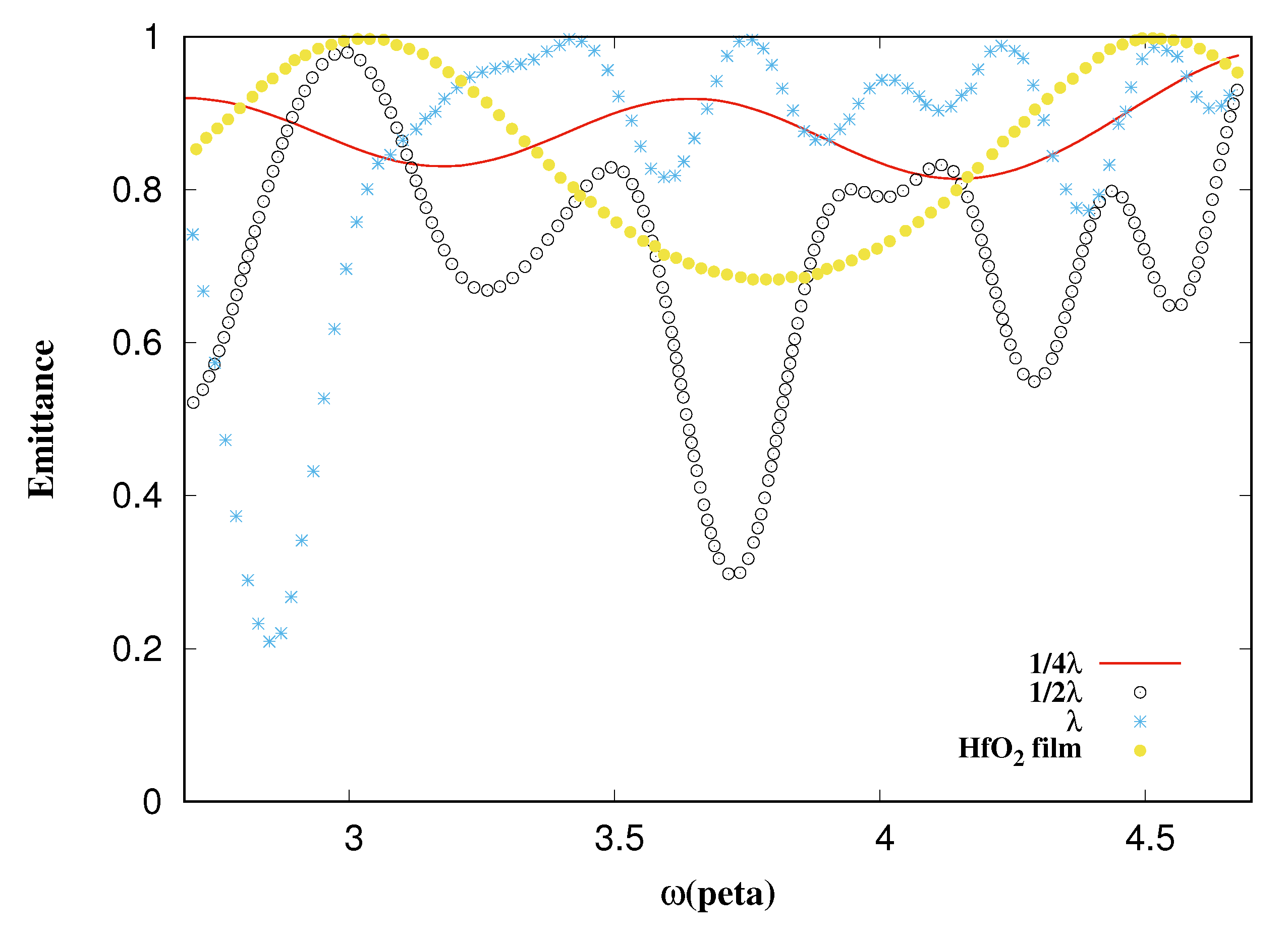
2. Emittance of Materials with Different Dielectric Constant
2.1. Theoretical Simulation of the Radiation Light
2.2. Sample Surface Temperature Experiment
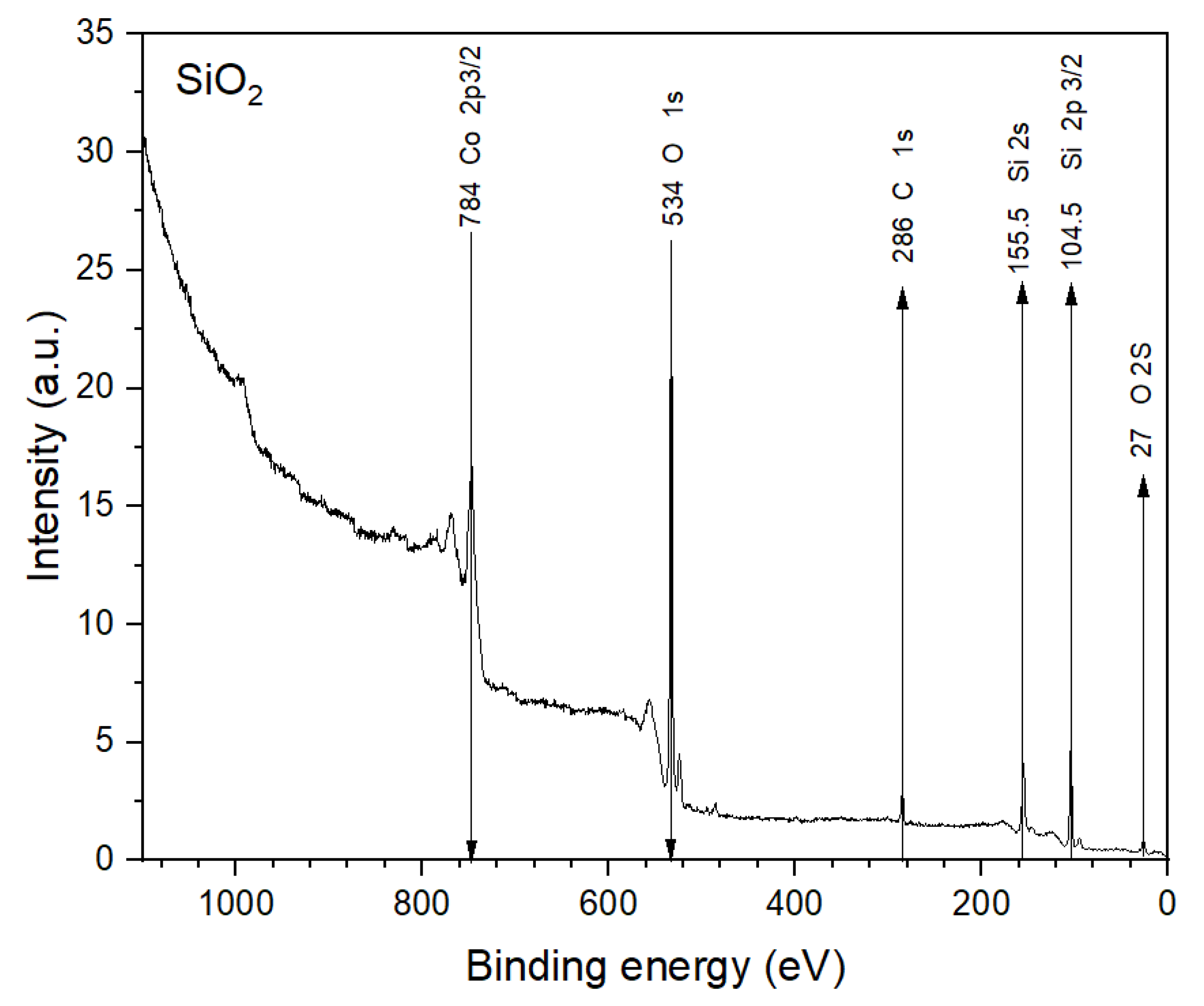
2.3. Experiment Discussion
- 1 mL of zinc acetate Zn(OCCH);
- 1 mL of Thiourea CHNS.
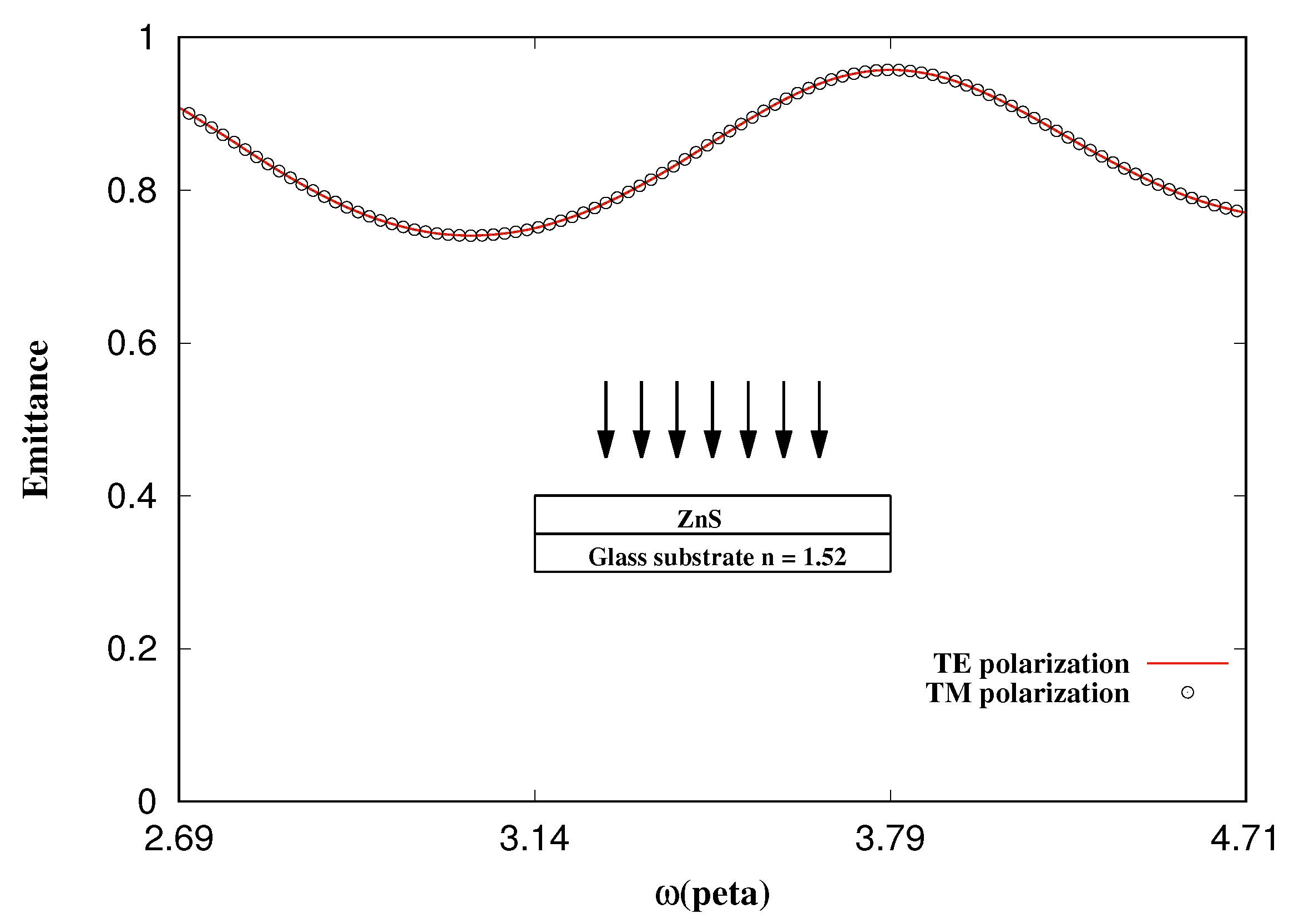
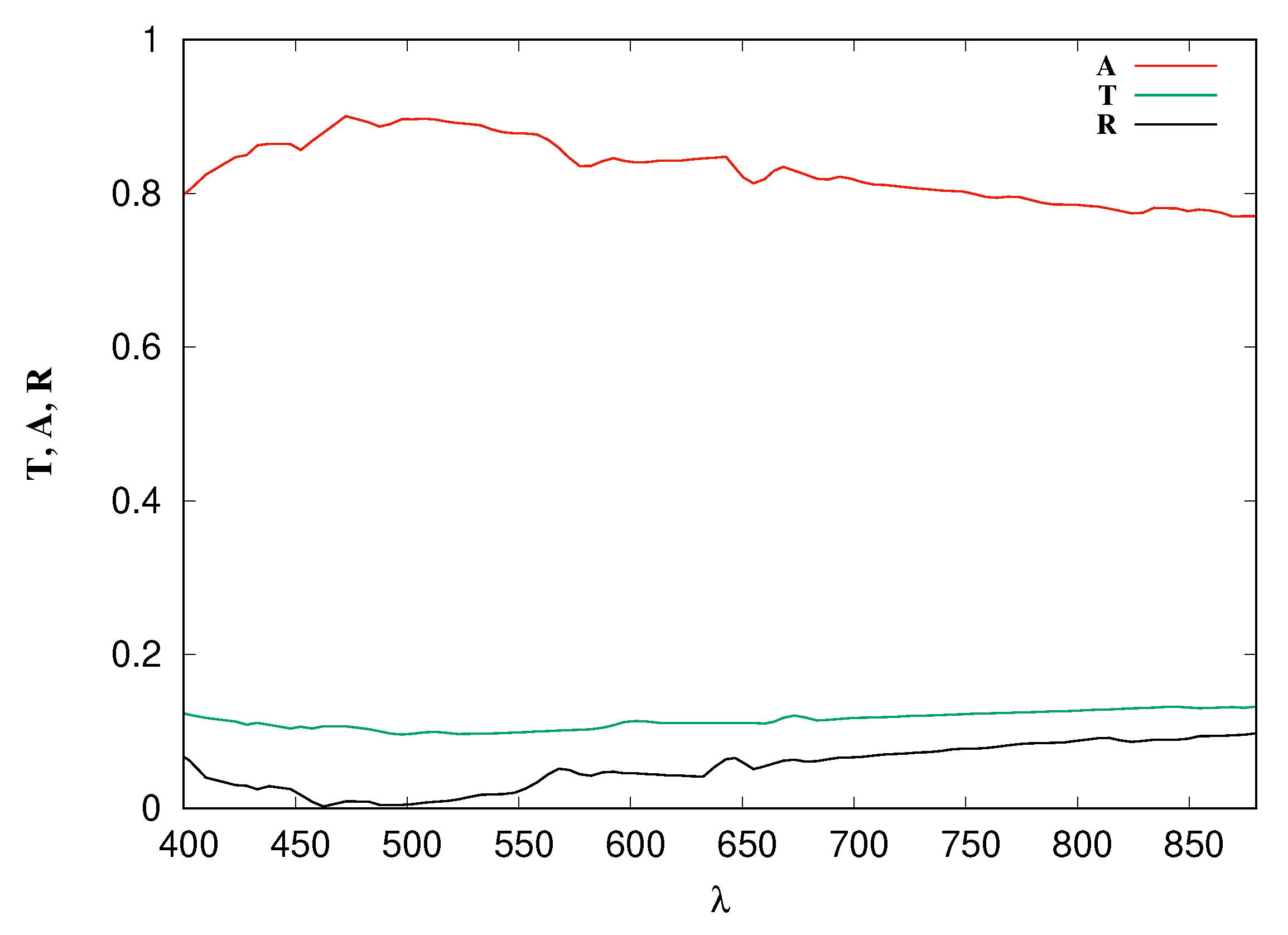
3. Reflection of ZnS in the Visible Spectrum
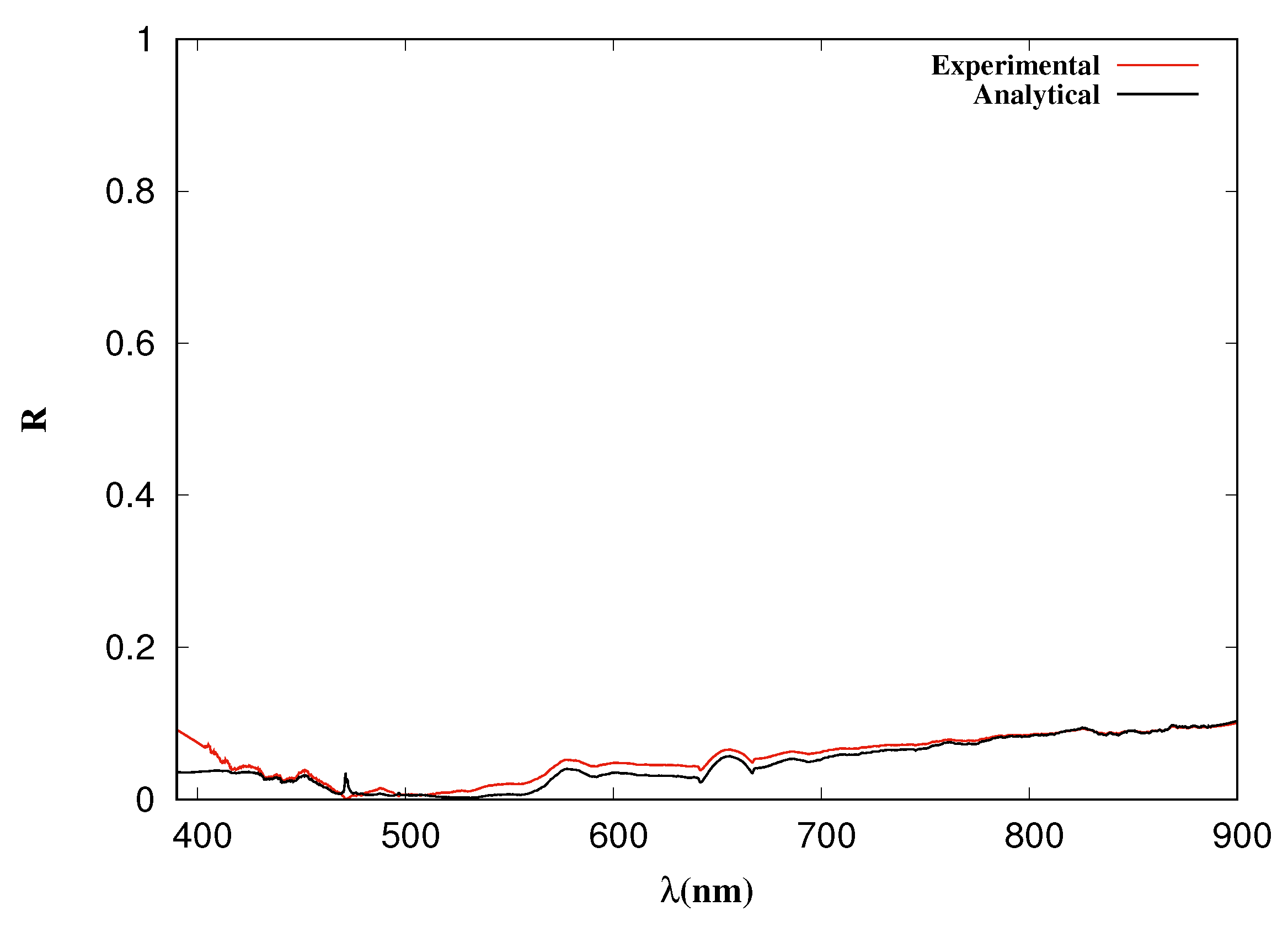
3.1. Reflectance Experiment
3.2. Theoretical Reproduction of Reflectance
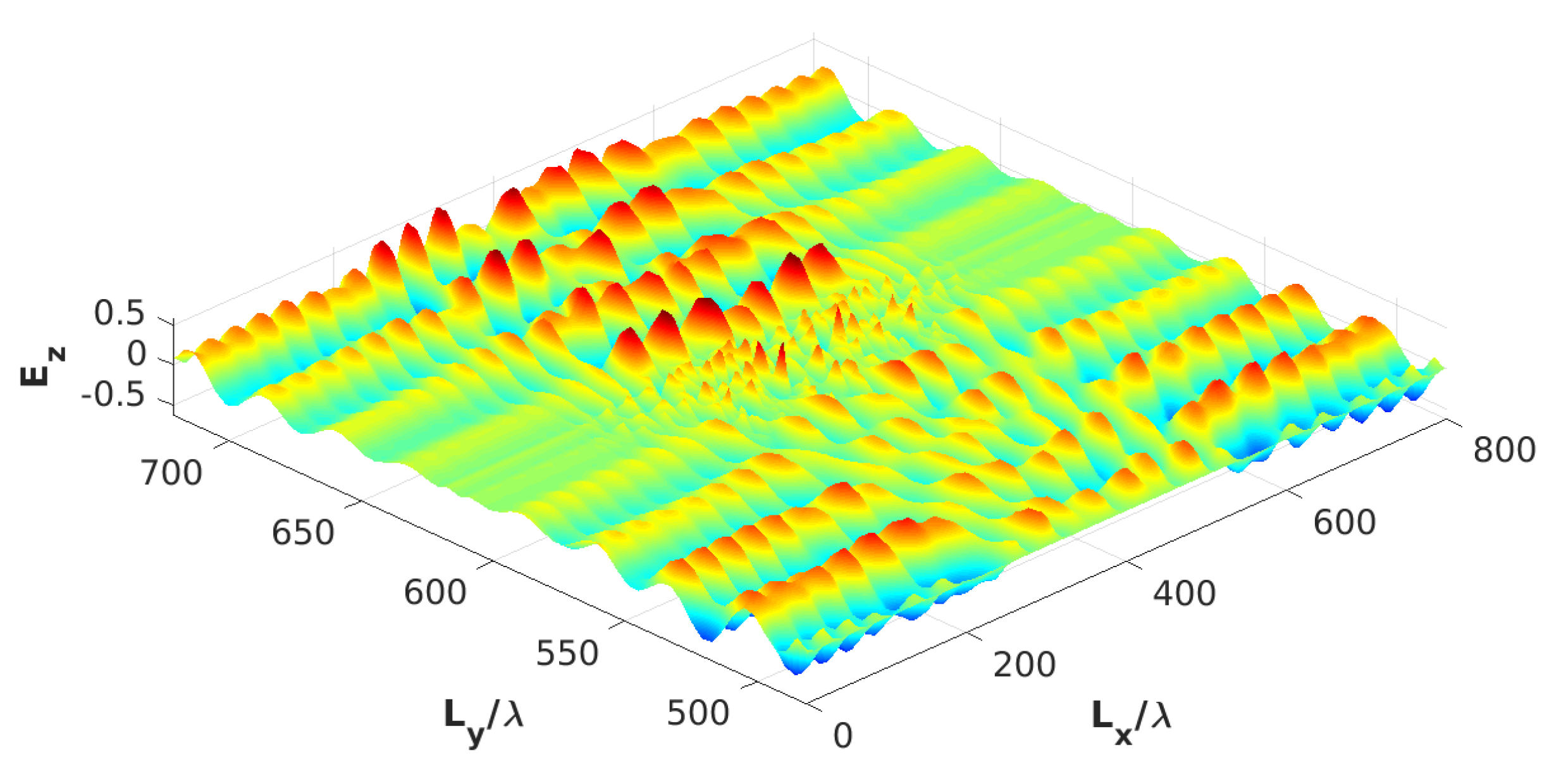
4. FDTD Emittance of a Flat Layer and Triangular Structures
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| TMM | Transfer matrix method |
| FDTD | Finite-difference time-domain |
References
- IEA. Key World Energy Statistics 2021; IEA: Paris, France, 2021; Available online: https://www.iea.org/reports/key-world-energy-statistics-2021 (accessed on 31 July 2023).
- Xu, L.; Wang, J.; Xiao, F.; EI-Badawy, S.; Awed, A. Potential strategies to mitigate the heat island impacts of highway pavement on megacities with considerations of energy uses. Appl. Energy 2021, 281, 116077. [Google Scholar] [CrossRef]
- Zhao, B.; Hu, M.; Ao, X.; Chen, N.; Pei, G. Radiative cooling: A review of fundamentals, materials, applications, and prospects. Appl. Energy 2019, 236, 489–513. [Google Scholar] [CrossRef]
- Raman, A.P.; Anoma, M.A.; Zhu, L.; Rephaeli, E.; Fan, S. Passive radiative cooling below ambient air temperature under direct sunlight. Nature 2014, 515, 540–544. [Google Scholar] [CrossRef] [PubMed]
- Abraham, M.J.; Murtola, T.; Schulz, R.; Páll, S.; Smith, J.C.; Hess, B.; Lindahl, E. GROMACS: High performance molecular simulations through multi-level parallelism from laptops to supercomputers. SoftwareX 2015, 1–2, 19–25. [Google Scholar] [CrossRef]
- Zhu, L.; Raman, A.P.; Fan, S. Radiative cooling of solar absorbers using a visibly transparent photonic crystal thermal blackbody. Proc. Natl. Acad. Sci. USA 2015, 112, 12282–12287. [Google Scholar] [CrossRef] [PubMed]
- Zhong, H.; Zhang, P.; Li, Y.; Yang, X.; Zhao, Y.; Wang, Z. Highly Solar-Reflective Structures for Daytime Radiative Cooling under High Humidity. ACS Appl. Mater. Interfaces 2020, 12, 51409–51417. [Google Scholar] [CrossRef] [PubMed]
- Castillo-Gallardo, V.; Puente-Díaz, L.E.; Ariza-Flores, D.; Pérez-Aguilar, H.; Mochán, W.L.; Agarwal, V. Optimization of wide-band quasi-omnidirectional 1-D photonic structures. Opt. Mater. 2021, 117, 111202. [Google Scholar] [CrossRef]
- Goyal, A.K.; Kumar, A. Recent advances and progresses in photonic devices for passive radiative cooling application: A review. J. Nanophotonics 2020, 14, 30901. [Google Scholar] [CrossRef]
- Ulpiani, G.; Ranzi, G.; Feng, J.; Santamouris, M. Expanding the applicability of daytime radiative cooling: Technological developments and limitations. Energy Build. 2021, 243, 110990. [Google Scholar] [CrossRef]
- Gupta, P.C.; Kim, Y.; Im, J.; Kang, G.; Urbas, A.; Kim, K. Enhancing the Efficiency of GaSb Photovoltaic Cell Using Thin-Film Multiscale Haze and Radiative Cooling. ACS Appl. Energy Mater. 2021, 4, 9304–9314. [Google Scholar] [CrossRef]
- Pirvaram, A.; Talebzadeh, N.; Leung, S.N.; O’Brien, P.G. Radiative cooling for buildings: A review of techno-enviro-economics and life-cycle assessment methods. Renew. Sustain. Energy Rev. 2022, 162, 112415. [Google Scholar] [CrossRef]
- Cazzaniga, R.; Rosa-Clot, M.; Rosa-Clot, P.; Tina, G.M. Floating tracking cooling concentrating (FTCC) systems. In Proceedings of the 2012 38th IEEE Photovoltaic Specialists Conference, Austin, TX, USA, 3–8 June 2012; Available online: https://ieeexplore.ieee.org/document/6317668 (accessed on 31 July 2023).
- Badwawi, R.A.; Abusara, M.; Mallick, T. A Review of Hybrid Solar PV and Wind Energy System. Smart Sci. 2015, 3, 127–138. [Google Scholar] [CrossRef]
- Chen, H.; Yang, J.; Zhou, N.; Chen, J.; Zhang, Y. Performance analysis of a high concentrating photovoltaic/thermal system with a water spray cooling device. IOP Conf. Ser. Mater. Sci. Eng. 2019, 556, 012034. [Google Scholar] [CrossRef]
- Yang, D.; Yin, H. Energy Conversion Efficiency of a Novel Hybrid Solar System for Photovoltaic, Thermoelectric, and Heat Utilization. IEEE Trans. Energy Convers. 2011, 26, 662–670. [Google Scholar] [CrossRef]
- Suzuki Valenzuela, M.F.; Sánchez Soto, F.; Armendáriz-Ontiveros, M.M.; Sosa-Tinoco, I.M.; Fimbres Weihs, G.A. Improving Thermal Distribution in Water-Cooled PV Modules and Its Effect on RO Permeate Recovery. Water 2021, 13, 229. [Google Scholar] [CrossRef]
- Mackay, T.G.; Lakhtakia, A. The Transfer-Matrix Method in Electromagnetics and Optics; Morgan and Claypool Publishers: San Rafael, CA, USA, 2020. [Google Scholar]
- RefractiveIndex.INFO-Refractive Index Database. Refractiveindex.info. 2015. Available online: https://refractiveindex.info/ (accessed on 31 July 2023).
- Khorrami, Y.; Fathi, D. Broadband thermophotovoltaic emitter using magnetic polaritons based on optimized one- and two-dimensional multilayer structures. J. Opt. Soc. Am. B-Opt. Phys. 2019, 36, 662. [Google Scholar] [CrossRef]
- Gutierrez, M.; Gaspar-Armenta, J. Quantification of the Field Enhancement of Surface Plasmon Under Standing Wave Conditions. Plasmonics 2021, 17, 163–172. [Google Scholar] [CrossRef]











| Material | Area above the Curve |
|---|---|
| PbSe-ZnS | 7.527 (a. u.) |
| Si-ZnS | 5.576 (a. u.) |
| ZnS | 3.457 (a. u.) |
| HfO-ZnS | 2.989 (a. u.) |
| SiO-ZnS | 2.42 (a. u.) |
| PbS-ZnS | 1.29 (a. u.) |
| Material | Area under the Curve |
|---|---|
| Si-ZnS | 20,035.96 (a. u.) |
| HfO-ZnS | 20,213.75 (a. u.) |
| SiO-ZnS | 20,738.09 (a. u.) |
| Si | 21,504.70 (a. u.) |
| HfO | 20,355.97 (a. u.) |
| SiO | 21,972.31 (a. u.) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gutiérrez-Villarreal, J.M.; Pineda-León, H.A.; Suzuki Valenzuela, M.F.; Sosa-Tinoco, I.; Castillo, S.J. Comparison of the Real Part of Dielectric Constants with Different Materials to Decrease the Emittance and a Virtual Dielectric Constant to Reproduce Reflectance. Photonics 2023, 10, 994. https://doi.org/10.3390/photonics10090994
Gutiérrez-Villarreal JM, Pineda-León HA, Suzuki Valenzuela MF, Sosa-Tinoco I, Castillo SJ. Comparison of the Real Part of Dielectric Constants with Different Materials to Decrease the Emittance and a Virtual Dielectric Constant to Reproduce Reflectance. Photonics. 2023; 10(9):994. https://doi.org/10.3390/photonics10090994
Chicago/Turabian StyleGutiérrez-Villarreal, Jesús Manuel, Horacio Antolin Pineda-León, Mario F. Suzuki Valenzuela, Ian Sosa-Tinoco, and Santos Jesús Castillo. 2023. "Comparison of the Real Part of Dielectric Constants with Different Materials to Decrease the Emittance and a Virtual Dielectric Constant to Reproduce Reflectance" Photonics 10, no. 9: 994. https://doi.org/10.3390/photonics10090994
APA StyleGutiérrez-Villarreal, J. M., Pineda-León, H. A., Suzuki Valenzuela, M. F., Sosa-Tinoco, I., & Castillo, S. J. (2023). Comparison of the Real Part of Dielectric Constants with Different Materials to Decrease the Emittance and a Virtual Dielectric Constant to Reproduce Reflectance. Photonics, 10(9), 994. https://doi.org/10.3390/photonics10090994






