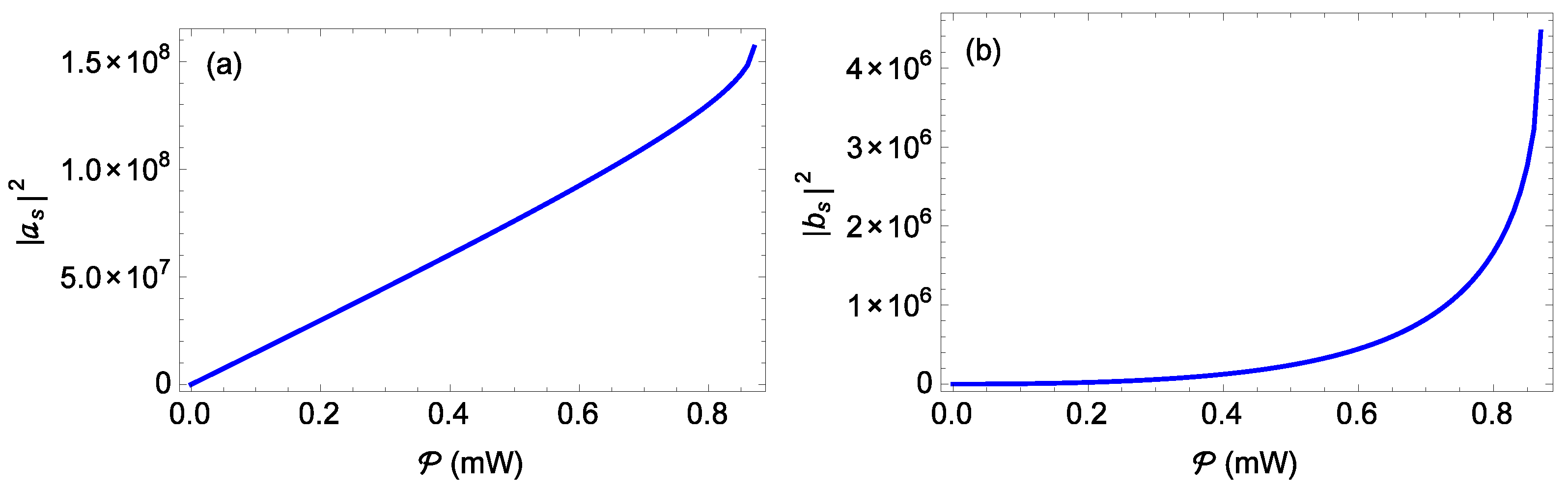Enhancing the Steady-State Entanglement between a Laguerre–Gaussian-Cavity Mode and a Rotating Mirror via Cross-Kerr Nonlinearity

Abstract

Quantum entanglement will play an important role in future quantum technologies. Here, we theoretically study the steady-state entanglement between a cavity field and a macroscopic rotating mirror in a Laguerre–Gaussian-(LG)-cavity optomechanical system with cross-Kerr nonlinearity. Logarithmic negativity is used to quantify the steady-state entanglement between the cavity and mechanical modes. We analyze the impacts of the cross-Kerr coupling strength, the cavity detuning, the input laser power, the topological charge of the LG-cavity mode, and the temperature of the environment on the steady-state optomechanical entanglement. We find that cross-Kerr nonlinearity can significantly enhance steady-state optomechanical entanglement and make steady-state optomechanical entanglement more robust against the temperature of the thermal environment.

1. Introduction

One of the most significant open problems in quantum optics is to prepare entangled states, which have potential applications in quantum sensing and networking [1]. Entangled states have been experimentally created in several microscopic systems, such as atoms [2], photons [3], and ions [4]. However, the creation of entangled states at the macroscopic level is still challenging. In the past two decades, optomechanical systems, in which a cavity field is coupled with a macroscopic mechanical oscillator via linear momentum transfer, have been proven to be a promising platform on which to prepare macroscopic entanglement [5] because the linear vibration of the mechanical oscillator can, experimentally, be cooled down close to its quantum ground state [6,7]. Meanwhile, Laguerre–Gaussian-(LG)-cavity optomechanical systems have been intensively studied [8,9,10,11,12,13,14,15,16,17,18,19,20,21]. In these, an LG-cavity mode is coupled to a rotating mirror due to the orbital angular momentum transfer from the LG-cavity mode to the rotating mirror. It has been shown that it is also possible to cool down the rotational motion of a macroscopic mirror close to its quantum ground state [8]. Thus, it is possible to observe a variety of nonlinear and quantum phenomena in such systems, including optomechanically induced transparency [9,10,11,12], optomechanically induced amplification [13], Fano resonance [14], fast and slow light [11,14], optical sum-sideband generation [15], second-order sideband effects [16], entanglement between an LG-cavity mode and a rotating mirror [17,18], stationary entanglement between two rotating mirrors [19,20], and tripartite entanglement between an LG-cavity mode, a magnon mode, and a phonon mode [21].

An LG laser beam carries an orbital angular momentum of $l\hslash$ per photon, where l is the topological charge [22]. Its phase distribution is helical and it contains a phase singularity at which the intensity is zero [23]. An LG laser beam can be produced by using spatial light modulators [24], computer-generated holograms [25], and spiral-phase elements [23]. The topological charge of the LG laser beam generated by the spiral phase element can be tuned by changing the step height and the refractive index of the spiral-phase element [23].

It is well known that the cross-Kerr effect is the change in the refractive index of a material in the presence of an applied electromagnetic field, and that the induced refractive index change is proportional to the intensity of the applied electromagnetic field [26]. It has been shown that the cross-Kerr effect can be used to realize a quantum non-demolition measurement [27], the generation of multiphoton Greenberger–Horne–Zeilinger states [28], the construction of a controlled NOT gate [29], the creation of strong micro–macro entanglement [30], and so on. In optomechanical systems, it has been theoretically proposed [31] and experimentally demonstrated [32] that a cross-Kerr type of coupling between the mechanical oscillator and the microwave cavity field can be realized by using a two-level system (qubit or artificial atom). In Ref. [31], it was shown that cross-Kerr coupling can lead to a cavity frequency shift, which is related to the phonon number of the mechanical oscillator. In the past few years, cross-Kerr coupling in optomechanical systems has been widely studied. It has been found that cross-Kerr coupling can affect the mechanical frequency shift and the optical damping rate in red and blue mechanical sidebands [33]. Cross-Kerr coupling can also make the bistable behavior of an intracavity photon number occurring at lower laser power [34,35]. Moreover, cross-Kerr coupling can lead to an improvement in optical nonreciprocity, nonreciprocal photon amplification, and optical squeezing [36]. Additionally, cross-Kerr coupling can be used to enhance the slow light effect [37]. Furthermore, cross-Kerr coupling affects photon blockade, the magnitude of single-photon mechanical displacement, and the generation of mechanical cat states [38]. Moreover, cross-Kerr coupling can improve the steady-state entanglement between optical and mechanical modes [39,40].

In this paper, we study the steady-state entanglement between an optical cavity field and a rotating mirror in an LG-cavity optomechanical system in the presence of cross-Kerr nonlinearity. We analyze how the cross-Kerr coupling strength, the cavity detuning, the input laser power, the topological charge of the LG-cavity mode, and the temperature of the environment affect the steady-state optomechanical entanglement. In the presence of cross-Kerr nonlinearity, we find that the steady-state optomechanical entanglement is greatly improved and is more robust against the thermal noise of the surrounding environment.

The article is organized as follows. In Section 2, we introduce the LG-cavity optomechanical system with cross-Kerr nonlinearity, we present the Hamiltonian of the whole system, we provide the quantum Langevin equations, and we calculate the steady-state amplitudes of the cavity and mechanical modes. In Section 3, we use logarithmic negativity as a measure of entanglement between the cavity and mechanical modes in the steady state. In Section 4, we discuss the influence of cross-Kerr coupling strength, cavity detuning, input laser power, the topological charge of an LG-cavity mode, and the temperature of the environment on steady-state optomechanical entanglement. In Section 5, we summarize our results.

2. Model

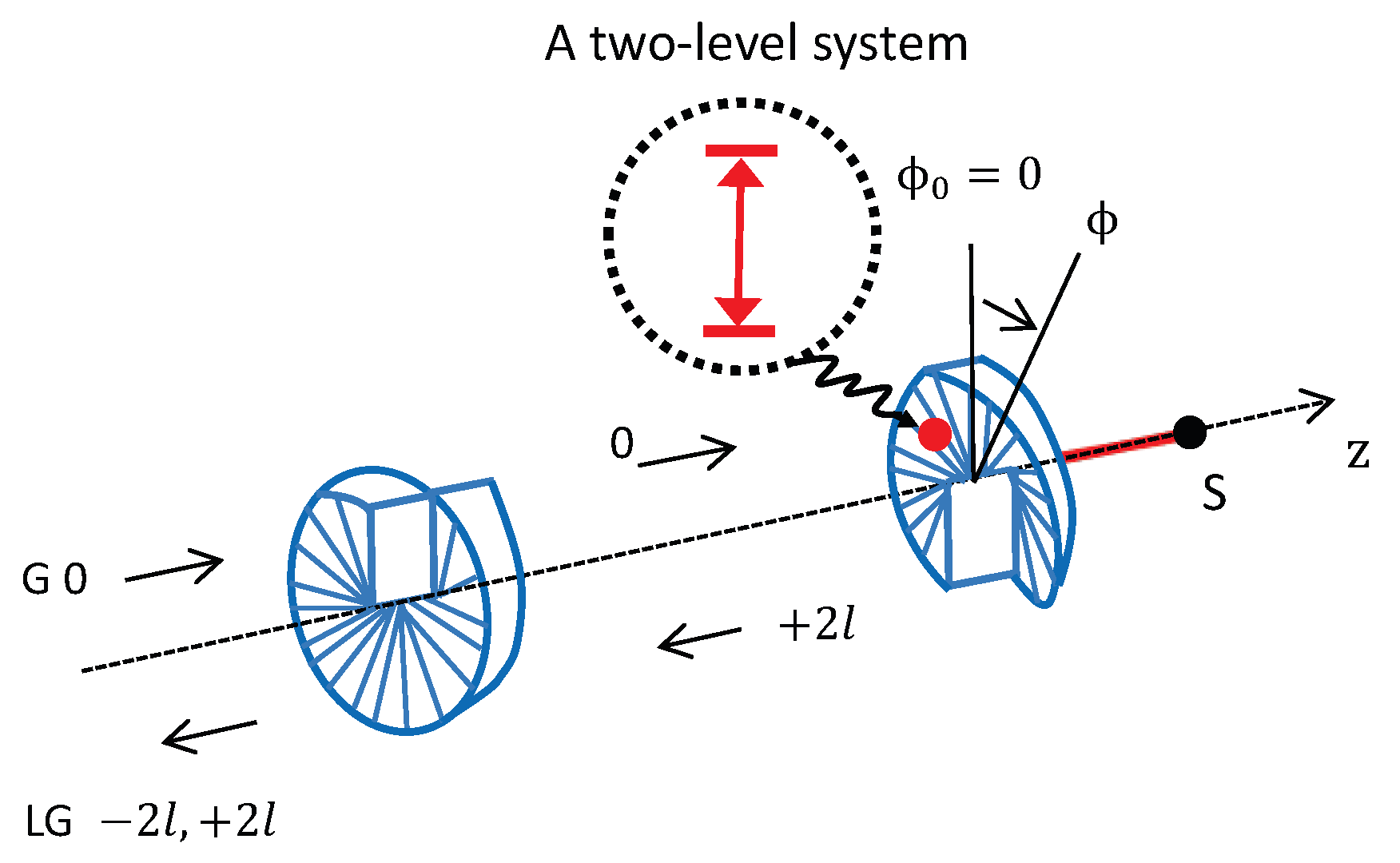
We consider an LG-cavity optomechanical system with one fixed mirror and one rotating mirror, as shown in Figure 1. The left fixed mirror transmits a small portion of the incident light, and the right rotating mirror totally reflects the incident light. The two cavity mirrors are spiral-phase elements which are designed to impart a fixed topological charge onto the incident light [8,23]. We assume that an incident Gaussian beam with charge 0 interacts with the fixed mirror, that the charge of the reflected beam is $2l$ less than that of the incident beam, and that the charge of the transmitted beam is the same as that of the input beam. Then, the beam with charge 0 interacts with the rotating mirror, and the charge of the reflected beam is $2l$ larger than that of the incident beam. In addition, the beam with charge $2l$ returns to the fixed mirror and interacts with it, the reflected component has charge 0, and the transmitted component has charge $2l$. Thus, a single LG-cavity mode is created in the optical cavity. Due to the exchange of the orbital angular momentum between the intracavity photons and the rotating mirror, the LG-cavity mode exerts a radiation torque on the rotating mirror so that the rotating mirror vibrates around the z axes. We can approximate the rotating mirror with mass m, radius R, frequency ${\omega }_m$, and damping rate ${\gamma }_m$ as a harmonic oscillator. The optomechanical coupling strength between the cavity field and the rotating mirror is $g_{\varphi }=\frac{cl}{L}$, where c is the light speed in vacuum and L is the length of the optical cavity [8]. We assume that there is a quantum two-level system on the rotating mirror which induces the cross-Kerr coupling between the cavity field and the rotating mirror [32]. The cross-Kerr coupling strength is denoted by $g_{ck}$. It has been shown that the cross-Kerr coupling strength $g_{ck}$ can be enhanced by using the optical parametric amplification [41], periodically modulating the mechanical spring constant [42], and using the Josephson capacitance of a Cooper-pair box [43]. The motion of the rotating mirror is described by the angular displacement $\varphi$ and the angular momentum $L_z$, which satisfies the commutation relation $[\varphi , L_z]=i$ and can be, respectively, expressed in terms of the annihilation operator b and the creation operator $b^{†}$ of the rotating mirror, i.e., $\varphi =\frac{1}{\sqrt{2}}(b+b^{†})$, $L_z=\frac{1}{i\sqrt{2}}(b-b^{†})$. The operators b and $b^{†}$ of the rotating mirror satisfy the commutation relation $[b, b^{†}]=i$.

The Hamiltonian of the system in the rotating frame at the frequency ${\omega }_l$ of the input Gaussian beam is given by

$$\begin{array}{ccc}\hfill H& =& \hslash ({\omega }_c-{\omega }_l)a^{†}a+\hslash {\omega }_m{b}^{†}b-\hslash g{a}^{†}a(b+b^{†})-\hslash g_{ck}{a}^{†}a{b}^{†}b+i\hslash \epsilon (a^{†}-a), \hfill \end{array}$$

(1)

where a ($a^{†}$) is the annihilation (creation) operator of the cavity mode with the resonance frequency ${\omega }_c$; ${\omega }_l$ is the frequency of the input Gaussian laser beam; g is the single-photon optomechanical coupling strength given by $g=g_{\varphi }\sqrt{\frac{\hslash }{2I{\omega }_m}}$, with $I=\frac{1}{2}m{R}^2$ being the moment of inertia of the rotating mirror; and $\epsilon$ is the amplitude of the input Gaussian beam given by $\epsilon =\sqrt{\frac{2\kappa \mathsf{\wp }}{\hslash {\omega }_l}}$, depending on the power ℘ of the input Gaussian beam and the decay rate $\kappa$ of the cavity field. In Equation (1), the first two terms are the free energies of the cavity field and the rotating mirror, respectively, the next two terms represent the optomechanical interaction and the cross-Kerr coupling between the cavity field and the rotating mirror, respectively, and the last term describes that the cavity mode is driven by the input Gaussian beam.

It is noted that the photon loss in the cavity through the left mirror is characterized with the decay rate $\kappa$, and the energy loss of the rotating mirror is characterized by the damping rate ${\gamma }_m$. Applying the Heisenberg equation of motion and adding the damping terms and the noise terms, we obtain the time evolution of the optical and mechanical modes

$$\begin{array}{ccc}\dot{a}\hfill & =\hfill & -[\kappa +i({\omega }_c-{\omega }_l)]a+iga(b+b^{†})+i{g}_{ck}a{b}^{†}b+\epsilon +\sqrt{2\kappa }{a}_{in}, \hfill \\ \dot{b}\hfill & =\hfill & -(\frac{{\gamma }_m}{2}+i{\omega }_m)b+ig{a}^{†}a+i{g}_{ck}{a}^{†}ab+\sqrt{{\gamma }_m}{b}_{in}, \hfill \end{array}$$

(2)

where $a_{in}$ is the input optical noise operator associated with the vacuum fluctuations of the continuum of modes outside the optical cavity and has zero mean value, and $b_{in}$ is the Brownian thermal noise operator of the rotating mirror due to its coupling to the thermal environment and has zero mean value. The time correlation functions for the noises $a_{in}$ and $b_{in}$ are given by

$$\begin{array}{ccc}\langle a_{in}\left(t\right)a_{in}^{†}\left(t^{\prime }\right)\rangle \hfill & =\hfill & \delta (t-t^{\prime }), \hfill \\ \langle b_{in}^{†}\left(t\right)b_{in}\left(t^{\prime }\right)\rangle \hfill & =\hfill & n_{th}\delta (t-t^{\prime }), \hfill \\ \langle b_{in}\left(t\right)b_{in}^{†}\left(t^{\prime }\right)\rangle \hfill & =\hfill & (n_{th}+1)\delta (t-t^{\prime }), \hfill \end{array}$$

(3)

where $n_{th}=1/\{exp[\hslash {\omega }_m/\left(k_{B}T\right)]-1\}$ is the mean thermal excitation number at the frequency ${\omega }_m$ of the rotating mirror, $K_B$ is the Boltzmann constant, and T is the environmental temperature. The steady-state mean values of the system operators are given by

$$\begin{array}{ccc}{a}_s\hfill & =\hfill & \frac{\epsilon }{\kappa +i[{\Delta }_0-g(b_s+b_s^{*})-g_{ck}|b_s{|}^2]}, \hfill \\ b_s\hfill & =\hfill & \frac{ig|a_s{|}^2}{\frac{{\gamma }_m}{2}+i({\omega }_m-g_{ck}|a_s{|}^2)}, \hfill \end{array}$$

(4)

where ${\Delta }_0={\omega }_c-{\omega }_l$ is the cavity detuning, $a_s$ is the steady-state amplitude of the cavity field, and $b_s$ is the steady-state amplitude of the mechanical mode. It is seen that both $a_s$ and $b_s$ depend on the optomechanical coupling strength g and the cross-Kerr coupling strength $g_{ck}$. Without the optomechanical coupling ($g=0$), the steady-state amplitude of the mechanical mode is $b_s=0$.

3. Quantum Fluctuations

Under the assumption of a strong Gaussian beam at the input, the photon number $|a_s{|}^2$ of the cavity field and the phonon number $|b_s{|}^2$ of the rotating mirror are much larger than l; thus, we can write the system operators as $a=a_s+\delta a$ and $b=b_s+\delta b$. Only keeping the first order in the fluctuations and ignoring the second and third orders in the fluctuations, we obtain the linearized quantum Langevin equations

$$\begin{array}{ccc}\delta \dot{a}\hfill & =\hfill & -(\kappa +i\Delta )\delta a+i{a}_s{G}^{*}\delta b+i{a}_{s}G\delta b^{†}+\sqrt{2\kappa }{a}_{in}, \hfill \\ \delta \dot{b}\hfill & =\hfill & -(\frac{{\gamma }_m}{2}+i{\overline{\omega }}_m)\delta b+i{a}_s^{*}G\delta a+i{a}_{s}G\delta a^{†}+\sqrt{{\gamma }_m}{b}_{in}, \hfill \end{array}$$

(5)

where $\Delta ={\Delta }_0-g(b_s+b_s^{*})-g_{ck}{\left|b_s\right|}^2$ is the effective cavity detuning, depending on the optomechancial coupling strength g and the cross-Kerr coupling strength $g_{ck}$; $G=g+g_{ck}{b}_s$ is the effective single-photon optomechanical coupling strength, modified by the cross-Kerr interaction; and ${\overline{\omega }}_m={\omega }_m-g_{ck}{\left|a_s\right|}^2$ is the effective mechanical frequency, changed by the cross-Kerr interaction.

We introduce the dimensionless angular displacement and momentum fluctuations of the mechanical mode as $\delta \varphi =\frac{1}{\sqrt{2}}(\delta b+\delta b^{†})$, $\delta L_z=\frac{1}{i\sqrt{2}}(\delta b-\delta b^{†})$; the amplitude and phase fluctuations of the cavity mode as $\delta x=\frac{1}{\sqrt{2}}(\delta a+\delta a^{†})$ and $\delta y=\frac{1}{i\sqrt{2}}(\delta a-\delta a^{†})$; and the quadrature fluctuations of the noises as ${\varphi }_{in}=\frac{1}{\sqrt{2}}(b_{in}+b_{in}^{†})$, $L_{zin}=\frac{1}{i\sqrt{2}}(b_{in}-b_{in}^{†})$, $x_{in}=\frac{1}{\sqrt{2}}(a_{in}+a_{in}^{†})$, and $y_{in}=\frac{1}{i\sqrt{2}}(a_{in}-a_{in}^{†})$. The time evolution equations for the quadrature fluctuations are given by

$$\dot{f}\left(t\right)=Af\left(t\right)+n\left(t\right),$$

(6)

where $f\left(t\right)$ is the column vector of the quadrature fluctuations, $n\left(t\right)$ is the column vector of the noise sources, their transposes are

$$\begin{array}{ccc}\hfill f{\left(t\right)}^T& =& (\delta \varphi , \delta L_z, \delta x, \delta y), \hfill \\ \hfill n{\left(t\right)}^T& =& (\sqrt{{\gamma }_m}{\varphi }_{in}, \sqrt{{\gamma }_m}{L}_{zin}, \sqrt{2\kappa }{x}_{in}, \sqrt{2\kappa }{y}_{in});\hfill \end{array}$$

(7)

and the matrix A is given by

$$A=\left(\begin{array}{cccc}-\frac{{\gamma }_m}{2}& {\overline{\omega }}_m& -u\sigma & -v\sigma \\ -{\overline{\omega }}_m& -\frac{{\gamma }_m}{2}& u\rho & v\rho \\ -v\rho & -v\sigma & -\kappa & \Delta \\ u\rho & u\sigma & -\Delta & -\kappa \end{array}\right),$$

(8)

where $u=\frac{1}{\sqrt{2}}(a_s+a_s^{*})$, $v=\frac{1}{i\sqrt{2}}(a_s-a_s^{*})$, $\rho =\frac{1}{\sqrt{2}}(G+G^{*})$, $\sigma =\frac{1}{i\sqrt{2}}(G-G^{*})$. The stability of the studied system is determined by the eigenvalues of the matrix A. When all the eigenvalues of the matrix A have negative real parts, the system is in the stable regime. We use the Routh–Hurwitz stability criterion [44] and find the stability conditions of the system

$$\begin{array}{ccc}{s}_1\hfill & =\hfill & ({\Delta }^2+{\kappa }^2)(\frac{{\gamma }^2}{4}+{\overline{\omega }}_m^2)-(u^2+v^2)({\rho }^2+{\sigma }^2)\Delta {\overline{\omega }}_m>0, \hfill \\ s_2\hfill & =\hfill & w\{{\gamma }_m[{\Delta }^2+\kappa (\kappa +\frac{{\gamma }_m}{2})]+2\kappa {\overline{\omega }}_m^2\}-{(2\kappa +{\gamma }_m)}^2{s}_1>0, \hfill \end{array}$$

(9)

where $w=2\kappa ({\kappa }^2+2\kappa {\gamma }_m+{\Delta }^2+\frac{{\gamma }_m^2}{4})+{\gamma }_m(\frac{3}{2}\kappa {\gamma }_m+\frac{{\gamma }_m^2}{4}+{\overline{\omega }}_m^2)$. It is found that the stability conditions of the system depends on the cavity detuning ${\Delta }_0$, the cross-Kerr coupling strength $g_{ck}$, the power ℘ of the input laser, and the topological charge l of the LG-cavity mode.

Since the optical vacuum noise $a_{in}$ and the mechanical thermal noise $b_{in}$ are Gaussian noises with zero mean values, and the time evolution equations for the fluctuation operators $\delta a$ and $\delta b$ are linearized, the state of the optomechanical system remains in a Gaussian state at any time, which is described by the $4\times 4$ covariance matrix V with elements

$$\begin{array}{c}\hfill V_{ij}=\frac{1}{2}[\langle f_i\left(t\right)f_j\left(t\right)\rangle +\langle f_j\left(t\right)f_i\left(t\right)\rangle ].\end{array}$$

(10)

The equations of motion for the covariance matrix V are given by

$$\begin{array}{c}\hfill \frac{dV}{dt}=AV+V{A}^T+D, \end{array}$$

(11)

where D is the diffusion matrix. The matrix D is determined by the noise correlation functions $D_{ij}=\frac{1}{2}[\langle n_i\left(t\right)n_j\left(t\right)\rangle +\langle n_j\left(t\right)n_i\left(t\right)\rangle ]$, and is found to be $D=\mathrm{Diag}[{\gamma }_m(n_{th}+\frac{1}{2}), {\gamma }_m(n_{th}+\frac{1}{2}), \kappa , \kappa ]$. The stationary covariance matrix V can be calculated by solving the Lyapunov equation [45]

$$\begin{array}{c}\hfill AV+V{A}^T=-D.\end{array}$$

(12)

The logarithmic negativity $E_N$, which is used here as a measure of bipartite entanglement, is given by [46,47]

$$\begin{array}{c}\hfill E_N=\max [0, -\ln \left(2{\eta }^{-}\right)], \end{array}$$

(13)

where ${\eta }^{-}\equiv \frac{1}{\sqrt{2}}{\{\sum \left(V\right)-{[\sum {\left(V\right)}^2-4detV]}^{1/2}\}}^{1/2}$ with $\sum \left(V\right)=det{V}_c+det{V}_m-2det{V}_{cm}$ and

$$V=\left(\begin{array}{cc}{V}_m& V_{cm}\\ V_{cm}^T& V_c\end{array}\right).$$

(14)

Here, $V_m$, $V_c$, and $V_{cm}$ are $2\times 2$ matrices, $V_m$ and $V_c$ are the variances of the mechanical and cavity modes, respectively, and $V_{cm}$ is the correlation between the cavity and mechanical modes. The necessary and sufficient condition for the bipartite Gaussian state being entangled is ${\eta }^{-}<\frac{1}{2}$. When the logarithmic negativity $E_N$ is nonzero, the cavity field and the rotating mirror are entangled. When the logarithmic negativity $E_N$ is zero, they are separable.

4. The Stationary Entanglement between the Cavity Field and the Rotating Mirror in the Presence of Cross-Kerr Nonlinearity

In this section, we discuss the effects of the cross-Kerr coupling strength $g_{ck}$, the cavity detuning ${\Delta }_0$, the input laser power ℘, the topological charge l of the LG-cavity mode, and the temperature T of the environment on the stationary entanglement between the cavity field and the rotating mirror.

The parameters we choose are similar to those in Ref. [17]: the wavelength of the input laser is $\lambda =810$ nm, the length of the optical cavity is $L=1$ mm, the photon decay rate is $\kappa =2\pi \times 1.5$ MHz, the mass of the rotating mirror is $m=100$ ng, the radius of the rotating mirror is $R=10$ $\mu$m, the resonance frequency of the rotating mirror is ${\omega }_m=2\pi \times 10$ MHz, and the damping rate of the rotating mirror is ${\gamma }_m=2\pi \times 5$ Hz. Thus, the system is working in the resolved-sideband regime ${\omega }_m\gg \kappa$. It has been demonstrated experimentally that an LG beam with a topological charge of $l=1000$ can be generated by using spiral-phase elements [48].

In Figure 2, we plot the photon number $|a_s{|}^2$ in the cavity field and the phonon number $|b_s{|}^2$ in the rotating mirror at the steady state of the system as a function of the input laser power ℘ (mW) when $l=70$, ${\Delta }_0=0.33{\omega }_m$, and $g_{ck}={10}^{-3}g$. Based on the stable conditions of the system, it is found that the system is stable only if the power ℘ of the input laser is not larger than 0.87 mW. It is seen that an input laser with a higher power can generate a larger photon number $|a_s{|}^2$ in the cavity, and it also can lead to a larger phonon number $|b_s{|}^2$ in the rotating mirror due to the optomechanical coupling and the cross-Kerr coupling. As the phonons grow, the input pump photons interact with the phonons, leading to the creation of more cavity photons via absorption of the phonons. It is noted that $|a_s{|}^2$ and $|b_s{|}^2$ are much larger than 1, so the linearization assumption we make above is valid.

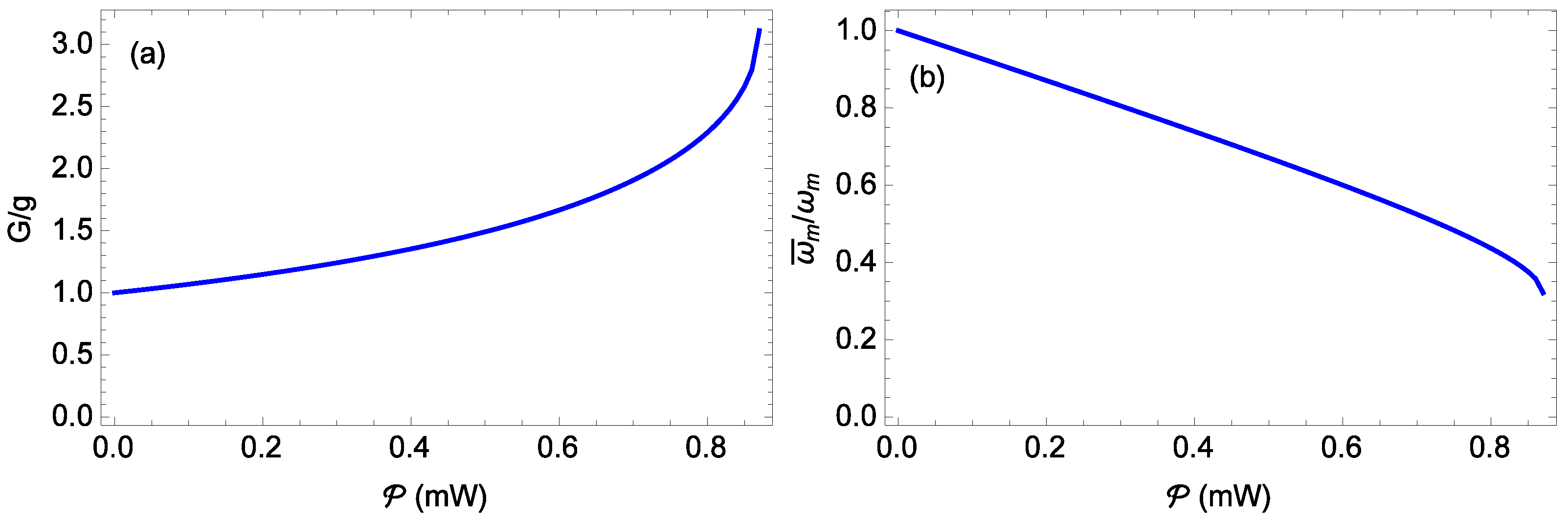
In Figure 3, we plot the normalized effective single-photon optomechanical coupling strength $G/g$ and the normalized effective mechanical frequency ${\overline{\omega }}_m/{\omega }_m$ of the rotating mirror as a function of the input laser power ℘ (mW) when $l=70$, ${\Delta }_0=0.33{\omega }_m$, and $g_{ck}={10}^{-3}g$. It is seen that the the effective single-photon optomechanical coupling strength G increases with increasing the power ℘ of the input laser, while the effective mechanical frequency ${\overline{\omega }}_m/{\omega }_m$ of the rotating mirror decreases with increasing the power of the input laser. These results are consistent with those in [39], which studies that the cross-Kerr coupling improves the optomechanical entanglement induced by the radiation pressure.

Figure 4 shows the logarithmic negativity $E_N$ as a function of the normalized cavity detuning ${\Delta }_0/{\omega }_m$ for different topological charges l of the LG-cavity mode when $\mathsf{\wp }=0.87$ mW, $T=0.1$ K, and $g_{ck}=0, 0.5\times {10}^{-3}g, {10}^{-3}g$. Without the cross-Kerr coupling ($g_{ck}=0$), for $l=30, 50, 70, 90$, and 110, the stability conditions of the system require that the cavity detuning satisfies ${\Delta }_0/{\omega }_m\ge 0.01$, 0.02, 0.03, 0.05, and 0.07, respectively. With the cross-Kerr coupling, for $l=30, 50, 70, 90$, and 110, the stability conditions of the system require that the cavity detuning satisfies ${\Delta }_0/{\omega }_m\ge 0.04$, 0.18, 0.25, 0.30, and 0.35, respectively, when $g_{ck}=0.5\times {10}^{-3}g$ and ${\Delta }_0/{\omega }_m\ge 0.17$, 0.26, 0.33, 0.39, and 0.45, respectively, when $g_{ck}={10}^{-3}g$. Thus, for a fixed value of the cross-Kerr coupling strength $g_{ck}$, as the topological charge l increases, the stable regime of the system becomes narrower. Moreover, for a fixed value of the topological charge l, increasing the cross-Kerr nonlinearity also makes the stable regime of the system smaller. Next, we first look at the case without the cross-Kerr coupling ($g_{ck}=0$). For $l=30$, as the cavity detuning ${\Delta }_0$ increases, the logarithmic negativity $E_N$ first increases and then decreases. For $l=50, 70, 90$, and 110, as the cavity detuning ${\Delta }_0$ increases, the logarithmic negativity $E_N$ first increases rapidly to the maximum value and then decreases slowly to the minimum value, again increases, and then decreases. Then, we consider the case of $g_{ck}=0.5\times {10}^{-3}g$. For $l=30$, with increasing the cavity detuning ${\Delta }_0$, the logarithmic negativity $E_N$ first increases and then decreases. For $l=50, 70, 90$, and 110, the logarithmic negativity $E_N$ decreases from the maximum value with the increase in the cavity detuning ${\Delta }_0$, and the logarithmic negativity $E_N$ takes the maximum value at a cavity detuning ${\Delta }_0$ close to the unstable regime of the system. When $g_{ck}={10}^{-3}g$, the behavior of the logarithmic negativity $E_N$ for $l=30, 50, 70, 90$, and 110 is the same as that for $l=50, 70, 90$, and 110 when $g_{ck}=0.5\times {10}^{-3}g$. Importantly, for a fixed value of the topological charge l, it is seen that the maximum entanglement between the cavity field and the rotating mirror in the presence of the cross-Kerr nonlinearity is much larger than that in the absence of the cross-Kerr nonlinearity. Without the cross-Kerr coupling ($g_{ck}=0$), for $l=30, 50, 70, 90$, and 110, the logarithmic negativity $E_N$ takes the maximum value of about 0.001, 0.003, 0.007, 0.012, and 0.018 at ${\Delta }_0/{\omega }_m=0.14, 0.18, 0.21, 0.24$, and $0.26$, respectively. In the presence of the cross-Kerr coupling, for $l=30, 50, 70, 90$, and 110, the logarithmic negativity $E_N$ takes the maximum value of about 0.031, 0.201, 0.211, 0.282, and 0.272 at ${\Delta }_0/{\omega }_m=0.10$, 0.18, 0.25, 0.30, and 0.35 when $g_{ck}=0.5\times {10}^{-3}g$, and takes the maximum value of about 0.255, 0.449, 0.459, 0.405, and 0.307 at ${\Delta }_0/{\omega }_m=0.17, 0.26, 0.33, 0.39$, and $0.45$ when $g_{ck}={10}^{-3}g$. Thus, for a given nonzero value of the cross-Kerr coupling strength $g_{ck}$, as the topological charge l increases, the maximum optomechanical entanglement occurs at a larger cavity detuning ${\Delta }_0$, but is not always increased. For a given value of the topological charge l, as the cross-Kerr coupling strength increases, the maximum optomechanical entanglement occurs at a larger cavity detuning ${\Delta }_0$, and it is always enhanced.

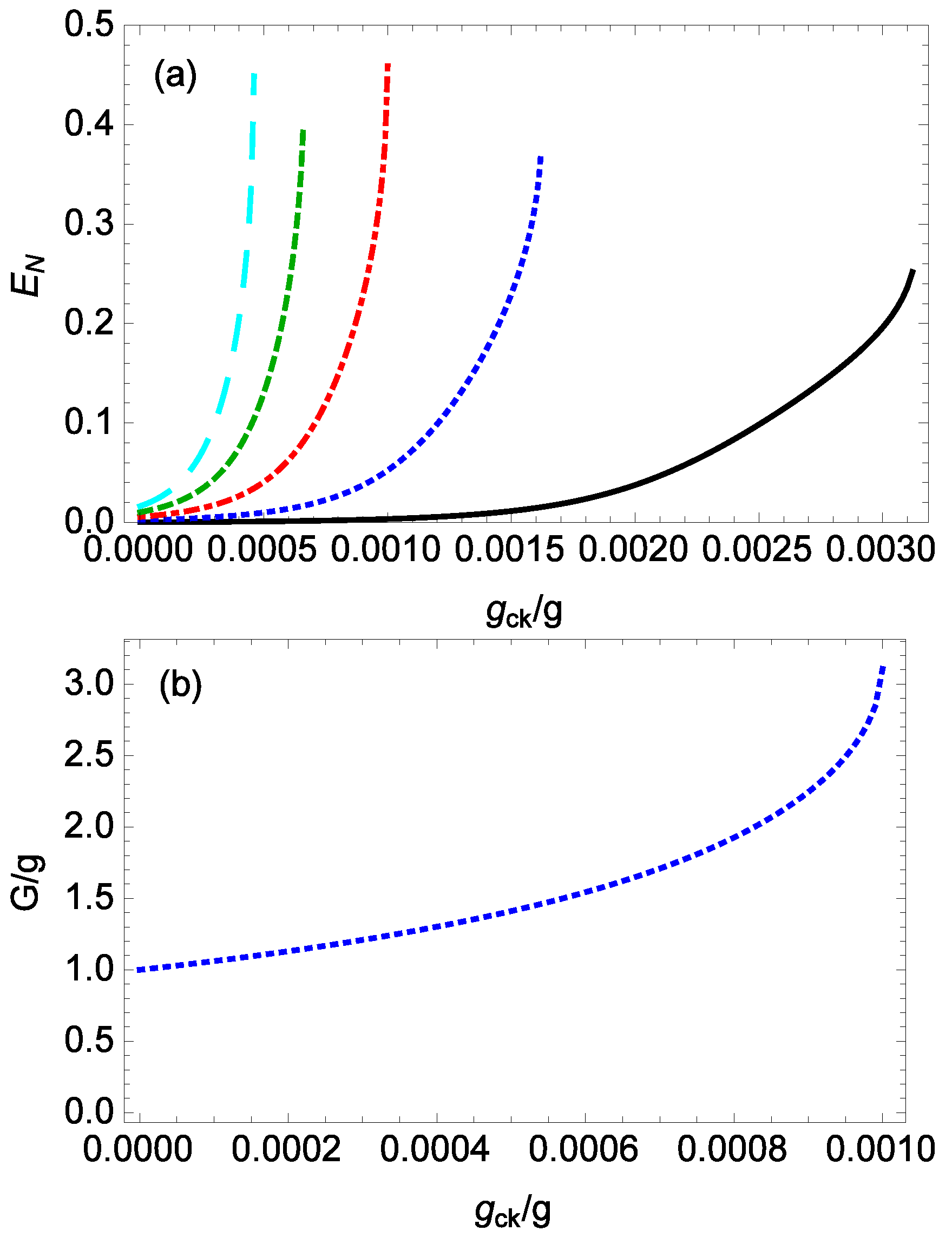
Figure 5a plots the logarithmic negativity $E_N$ as a function of the normalized cross-Kerr coupling strength $g_{ck}/g$ for different topological charges l of the LG-cavity mode when $\mathsf{\wp }=0.87$ mW, ${\Delta }_0=0.33{\omega }_m$, and $T=0.1$ K. For $l=30, 50, 70, 90$, and 110, the system is stable if the normalized cross-Kerr coupling strength $g_{ck}/g$ is not larger than $3.12\times {10}^{-3}$, $1.62\times {10}^{-3}$, ${10}^{-3}$, $0.66\times {10}^{-3}$, and $0.46\times {10}^{-3}$, respectively. Thus, the larger the topological charge l, the narrower the stable regime of the system. For a fixed value of the topological charge l, the logarithmic negativity $E_N$ increases with increasing the cross-Kerr coupling strength $g_{ck}$, and the logarithmic negativity $E_N$ takes the maximum value at a cross-Kerr coupling strength $g_{ck}$ close to the unstable regime. It is noted that the real part of the effective single-photon optomechanical coupling strength G is found to be much larger than its imaginary part by at least six orders of magnitude. For example, when $g_{ck}={10}^{-3}g$, $G=2.74+1.19\times {10}^{-6}i$, so G can be approximated as a real number. From Figure 5b, it is seen that the effective single-photon optomechanical coupling strength G is increased with the increase in the cross-Kerr coupling strength $g_{ck}$, thereby leading to the enhancement of the entanglement as shown in Figure 5a. Moreover, for a larger topological charge l, $E_N$ increases with increasing $g_{ck}$ at a faster rate. For $l=30, 50, 70, 90$, and 110, the maximum value of the logarithmic negativity $E_N$ is about 0.252, 0.373, 0.459, 0.402, and 0.449 at $g_{ck}/g=3.12\times {10}^{-3}, 1.62\times {10}^{-3}, {10}^{-3}, 0.66\times {10}^{-3}$, and $0.46\times {10}^{-3}$, respectively. Therefore, as the topological charge l increases, the maximum optomechanical entanglement occurs at a weaker cross-Kerr coupling strength $g_{ck}$, but is not always increased.

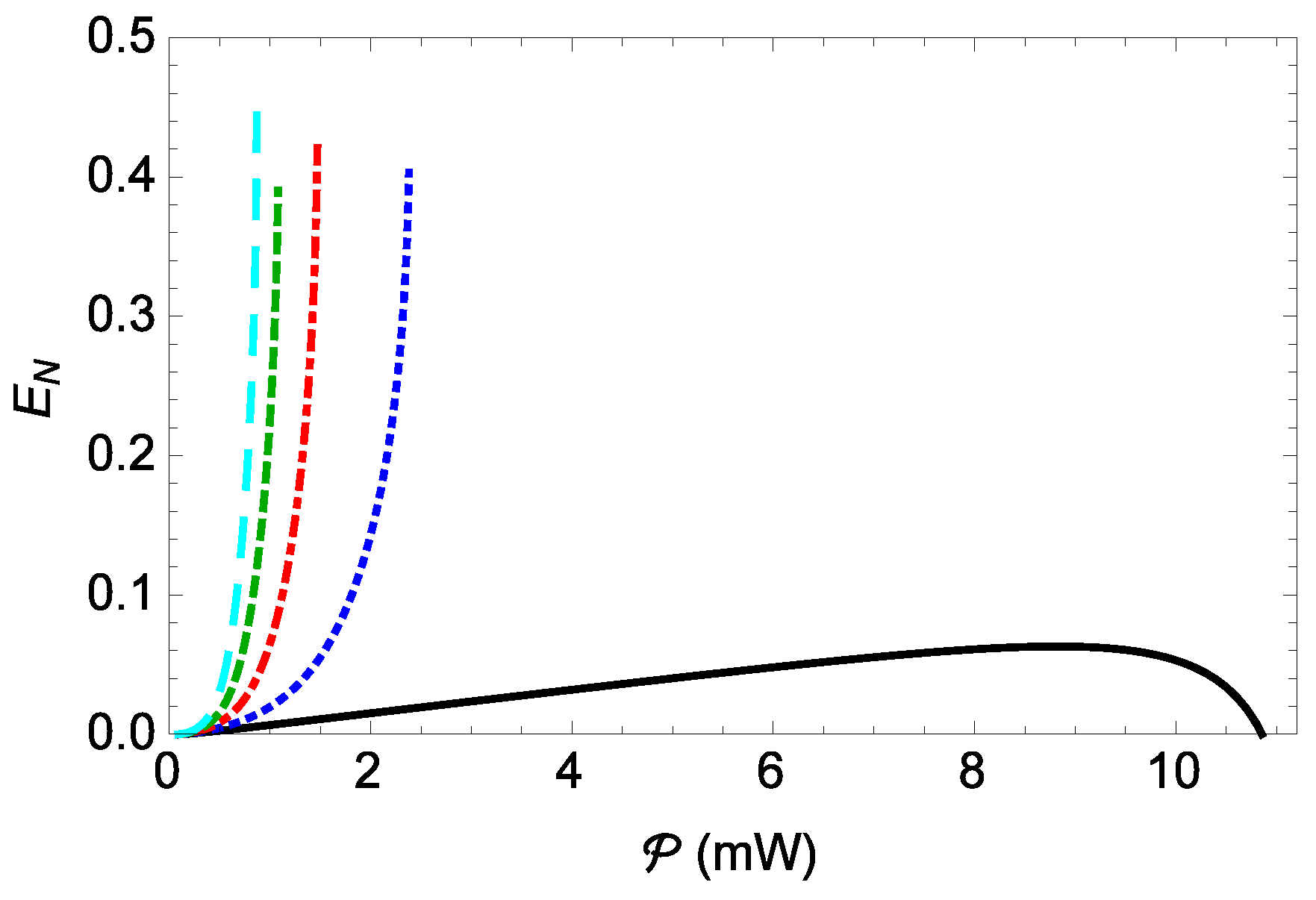
Figure 6 plots the logarithmic negativity $E_N$ against the input laser power ℘ (mW) for different cross-Kerr coupling strengths $g_{ck}$ when $l=70$, ${\Delta }_0=0.33{\omega }_m$, and $T=0.1$ K. For $g_{ck}/g=0, 0.25\times {10}^{-3}, 0.5\times {10}^{-3}, 0.75\times {10}^{-3},$ and ${10}^{-3}$, the stable conditions of the system require that the input laser power ℘ is not larger than 11.2 mW, 2.38 mW, 1.47 mW, 1.08 mW, and 0.87 mW, respectively. Thus, the stronger the cross-Kerr coupling, the smaller the stable regime of the system. Without the cross-Kerr coupling ($g_{ck}=0$), the logarithmic negativity $E_N$ first increases and then decreases with increasing the input laser power ℘. The maximum value of the logarithmic negativity $E_N$ is only about 0.063 at $\mathsf{\wp }=8.8$ mW. For a nonzero cross-Kerr coupling strength $g_{ck}$, the logarithmic negativity $E_N$ increases as the input laser power ℘ increases. The reason is that increasing the input laser power ℘ leads to a stronger optomechanical coupling and a stronger cross-Kerr coupling between the cavity and mechanical modes due to an increase in the cavity photon number and an increase in the mechanical phonon number as shown in Figure 2. It is also seen that the logarithmic negativity $E_N$ takes the maximum value at an input laser power ℘ near the unstable regime. For a larger cross-Kerr coupling strength $g_{ck}$, the logarithmic negativity $E_N$ increases faster with increasing the input laser power ℘. For $g_{ck}/g=0.25\times {10}^{-3}, 0.5\times {10}^{-3},$ and $0.75\times {10}^{-3}, {10}^{-3}$, the maximum value of the logarithmic negativity $E_N$ is about 0.403, 0.421, 0.390, and 0.459 at $\mathsf{\wp }=2.38$ mW, 1.47 mW, 1.08 mW, and 0.87 mW, respectively. Therefore, the maximum entanglement for the case with the cross-Kerr coupling is larger than that for the case without the cross-Kerr coupling. As the cross-Kerr coupling strength $g_{ck}$ increases, the maximum entanglement happens at a smaller input laser power ℘, but is not always enhanced.

Figure 7 shows the logarithmic negativity $E_N$ versus the topological charge l of the LG-cavity mode for different cross-Kerr coupling strengths $g_{ck}$ when ${\Delta }_0=0.33{\omega }_m$, $\mathsf{\wp }=0.87$ mW, and $T=0.1$ K. For $g_{ck}/g=0, 0.25\times {10}^{-3}, 0.5\times {10}^{-3}, 0.75\times {10}^{-3},$ and ${10}^{-3}$; the stable conditions of the system require that the topological charge l is not larger than 252, 144, 105, 83, and 70, respectively. Thus, when the cross-Kerr coupling strength $g_{ck}$ is larger, the stable regime of the system is narrower. We first consider the case without the cross-Kerr coupling ($g_{ck}=0$). When the cross-Kerr coupling strength $g_{ck}$ increases, the logarithmic negativity $E_N$ first increases and then decreases. The logarithmic negativity $E_N$ begins to be nonzero (the entanglement between the cavity field and the rotating mirror starts to appear) when the topological charge l is equal to 26. The maximum value of the logarithmic negativity $E_N$ is only about 0.063 at $l=222$. Next, we consider the case with the cross-Kerr coupling ($g_{ck}\ne 0$). For $g_{ck}/g=0.25\times {10}^{-3}, 0.5\times {10}^{-3}, 0.75\times {10}^{-3},$ and ${10}^{-3}$, the logarithmic negativity $E_N$ begins to be nonzero when the topological charge l is equal to 23, 22, 20, and 19, respectively. Hence, for a stronger cross-Kerr coupling, the entanglement between the cavity field and the rotating mirror appears at a smaller topological charge l, and it reaches the maximum value just before the unstable regime. For a stronger cross-Kerr coupling, the logarithmic negativity $E_N$ increases more quickly with increasing the topological charge l. For $g_{ck}/g=0.25\times {10}^{-3}, 0.5\times {10}^{-3}, 0.75\times {10}^{-3},$ and ${10}^{-3}$, the maximum value of the logarithmic negativity $E_N$ is about 0.326, 0.400, 0.369, and 0.459 at $l=144, 105, 83,$ and 70, respectively, which is much larger than that without the cross-Kerr coupling ($g_{ck}=0$). Hence, the presence of the cross-Kerr coupling makes the maximum entanglement larger than that without the cross-Kerr coupling. For a larger cross-Kerr coupling strength $g_{ck}$, the maximum entanglement happens at a smaller topological charge l. In addition, it is noted that the maximum entanglement happens when $g_{ck}/g={10}^{-3}$ and $l=70$ since the maximum value of the logarithmic negativity $E_N$ is the largest when $g_{ck}/g={10}^{-3}$ and $l=70$.

Figure 8 is a plot of the logarithmic negativity $E_N$ versus the temperature T of the environment for different cross-Kerr coupling strengths $g_{ck}$ when $\mathsf{\wp }=0.87$ mW, $l=70$, and ${\Delta }_0/{\omega }_m=0.33$. For a fixed value of the cross-Kerr coupling strength $g_{ck}$, it is seen that the logarithmic negativity $E_N$ decreases with the increase in the temperature T of the environment. For $g_{ck}/g=0, 0.5\times {10}^{-3}, 0.75\times {10}^{-3}, 0.9\times {10}^{-3},$ and ${10}^{-3}$, the logarithmic negativity $E_N$ takes the maximum value of about 0.008, 0.044, 0.122, 0.230, and 0.460 at $T=0$ K, respectively, and the logarithmic negativity $E_N$ becomes zero when $T\ge 0.7$ K, 3.2 K, 9.6 K, 23.4 K, and 70.8 K, respectively. Hence, for a stronger cross-Kerr coupling, the entanglement between the cavity field and the rotating mirror can survive at a higher temperature T of the environment.

Finally, we discuss the possibility of experimentally detecting the generated entanglement between the LG-cavity mode and the rotating mirror. In order to measure the logarithmic negativity $E_N$, we need to measure the ten independent elements of the correlation matrix V. For the cavity mode, its second moments can be directly measured by homodyne detecting the output field from the cavity. For the mechanical mode, we need to add a second cavity, adjacent to the first one, formed by the rotating mirror and a third transmissive fixed spiral phase element. There is no coupling between the two cavities. The angular displacement and the angular momentum of the rotating mirror can be measured by homodyning the output field of the second cavity via choosing the suitable parameters of the second cavity [49].

Without the cross-Kerr coupling, it is noted that the entanglement ($E_N=0.14$) between the LG-cavity mode and the rotating mirror in Ref. [17] is much larger than the entanglement ($E_N=0.018$) between the LG-cavity mode and the rotating mirror in our scheme. This is because they use a high laser power, 50 mW, and a small cavity length, 0.1 mm. In our scheme, a larger entanglement ($E_N=0.459$) between the LG-cavity mode and the rotating mirror can be achieved in the presence of the cross-Kerr coupling at a low laser power, 0.87 mW, and a large cavity length, 1 mm.

5. Conclusions

In conclusion, we have analyzed the steady-state entanglement between a cavity field and a rotating mirror in an LG-cavity optomechanical system with cross-Kerr nonlinearity. Comparing to the case without the cross-Kerr nonlinearity, the presence of the cross-Kerr nonlinearity can make the stable regime of the system narrower, and make the optomechanical entanglement significantly increase. In the presence of cross-Kerr nonlinearity, the optomechanical entanglement is increased with the increase in the input laser power or the topological charge of the LG-cavity mode. Increasing the cross-Kerr coupling strength leads to the occurrence of the maximum optomechanical entanglement at a larger cavity detuning, a lower input laser power, and a smaller topological charge of the LG-cavity mode. We have also found that the optomechanical entanglement is more robust against the temperature of the environment in the presence of cross-Kerr nonlinearity. The results we obtained are similar to those in Ref. [39], which focuses on the effect of cross-Kerr nonlinearity on the optomechanical entanglement induced by the radiation pressure. Our results may be useful for the construction of future quantum networks. It is worth mentioning that our system is assumed to be in the adiabatic limit; the rotation of the rotating mirror is very slow, so the number of photons generated by the Casimir effect [50] is negligible [51]. In the future, we plan to explore this study in the non-adiabatic limit.