Analyzing Vortex Light Beam Scattering Characteristics from a Random Rough Surface
Abstract
:1. Introduction
2. Methods
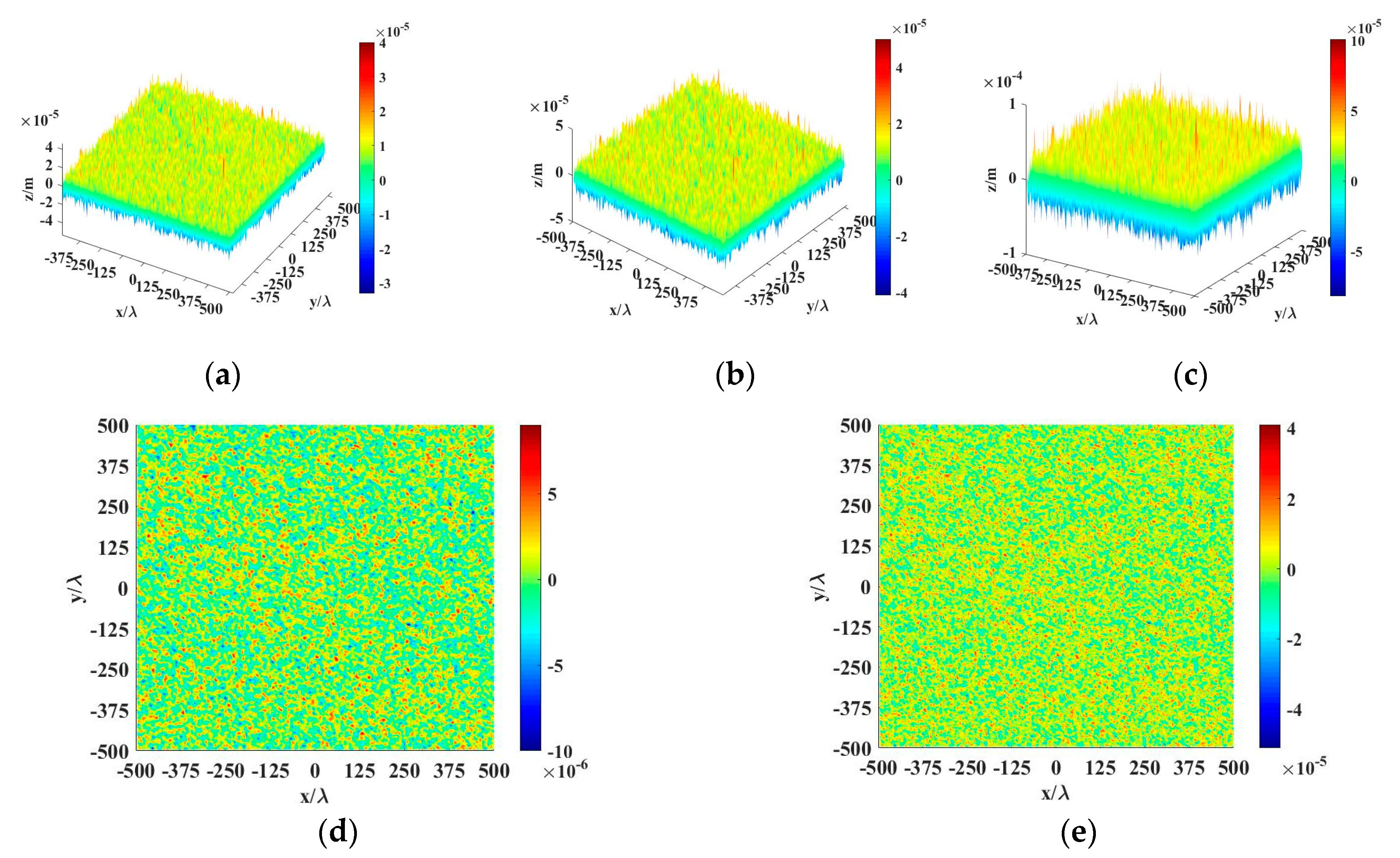
2.1. Gaussian Rough Surface Generation
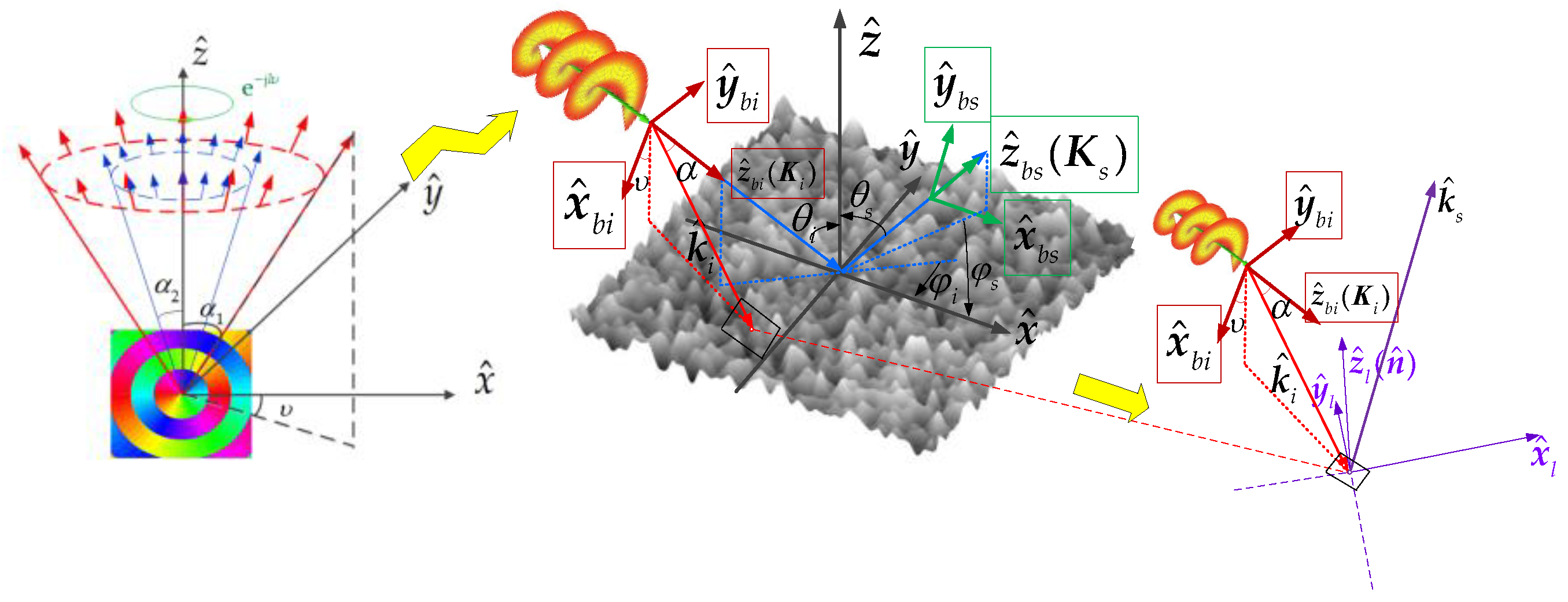
2.2. Vortex Wave Scattering Model from a Rough Surface
3. Numerical Results
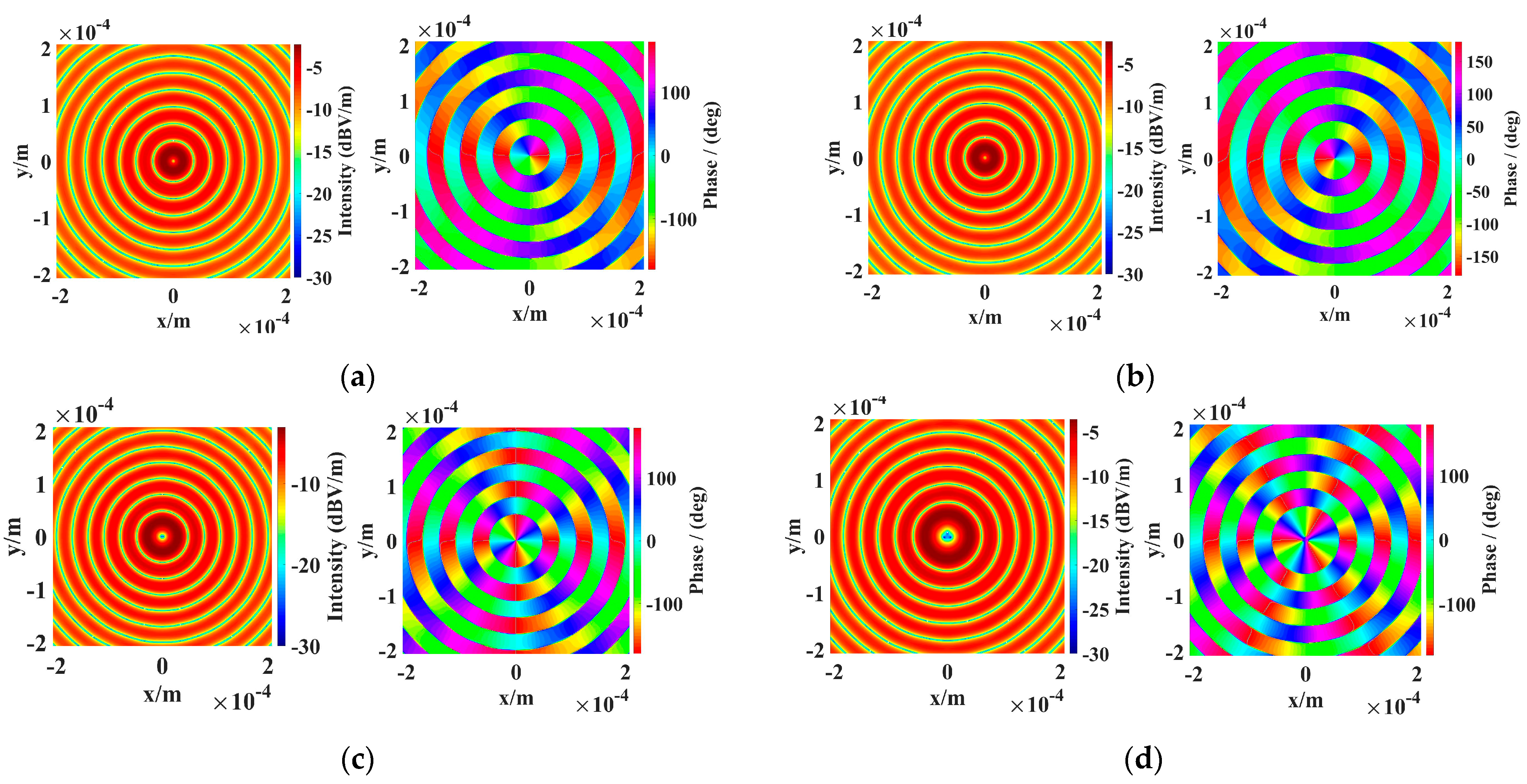
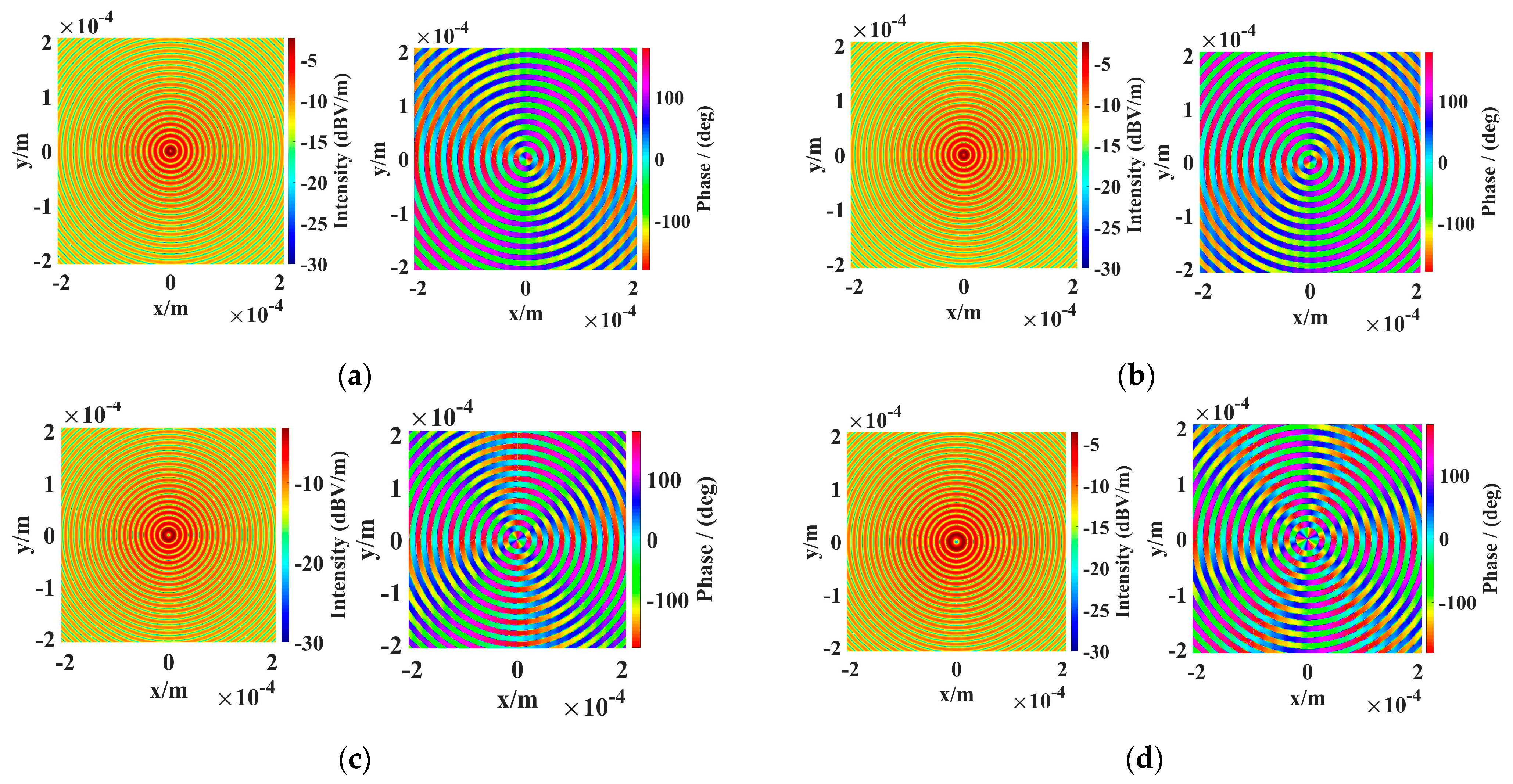
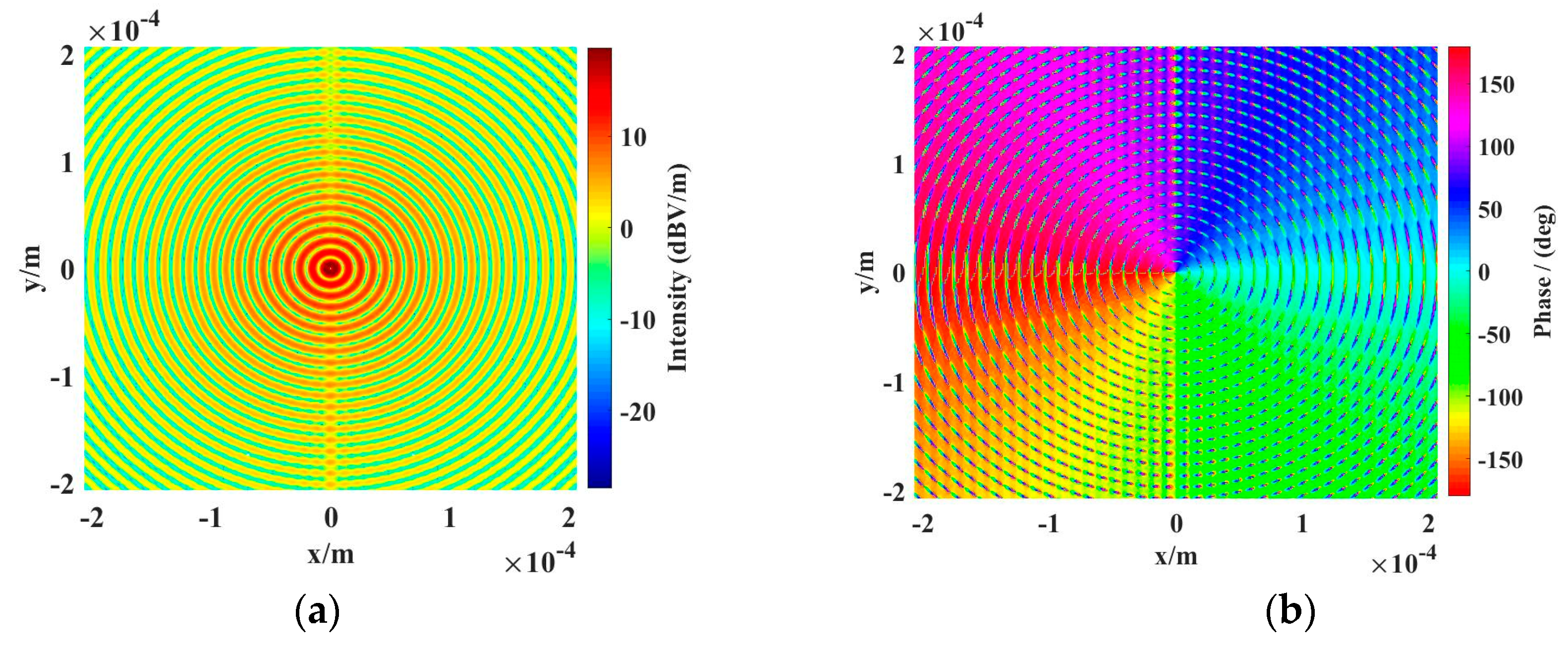
3.1. Cross Sections of OAM Waves’ Transverse Electric Fields
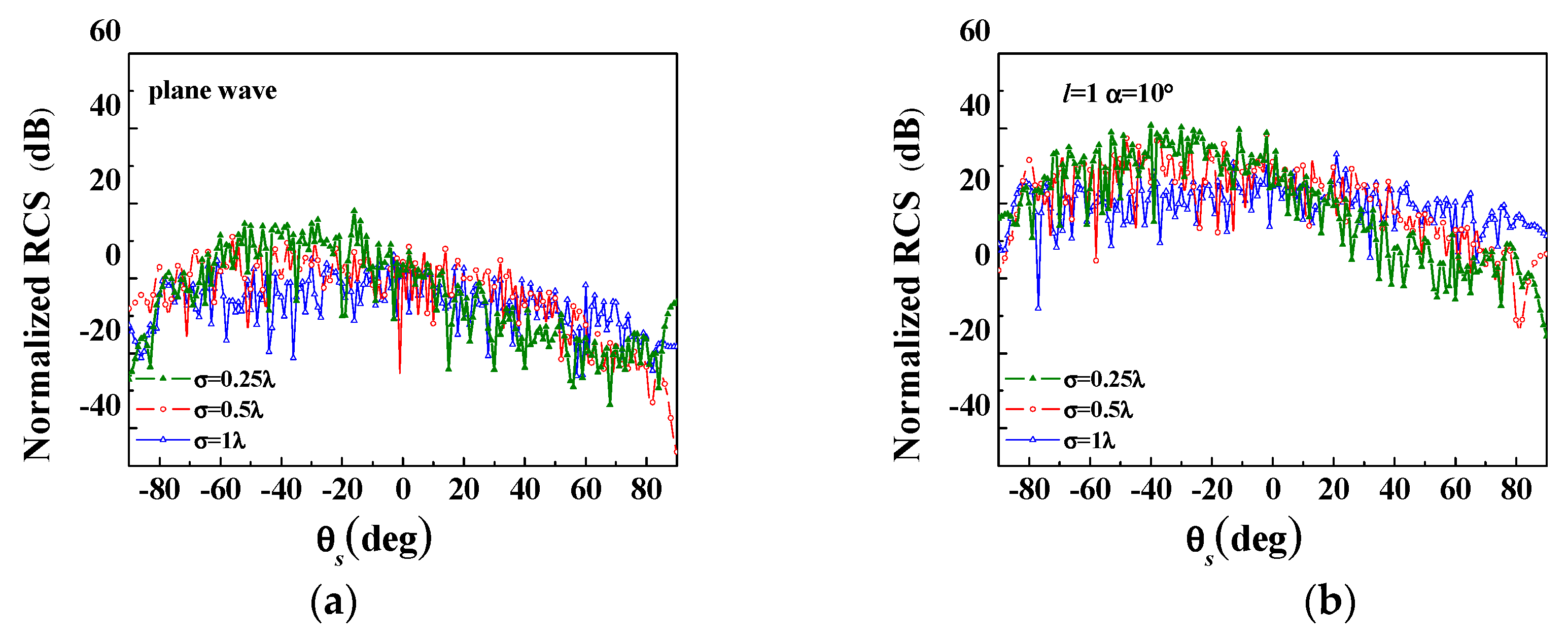
3.2. Normalized LRCS from Rough Surface
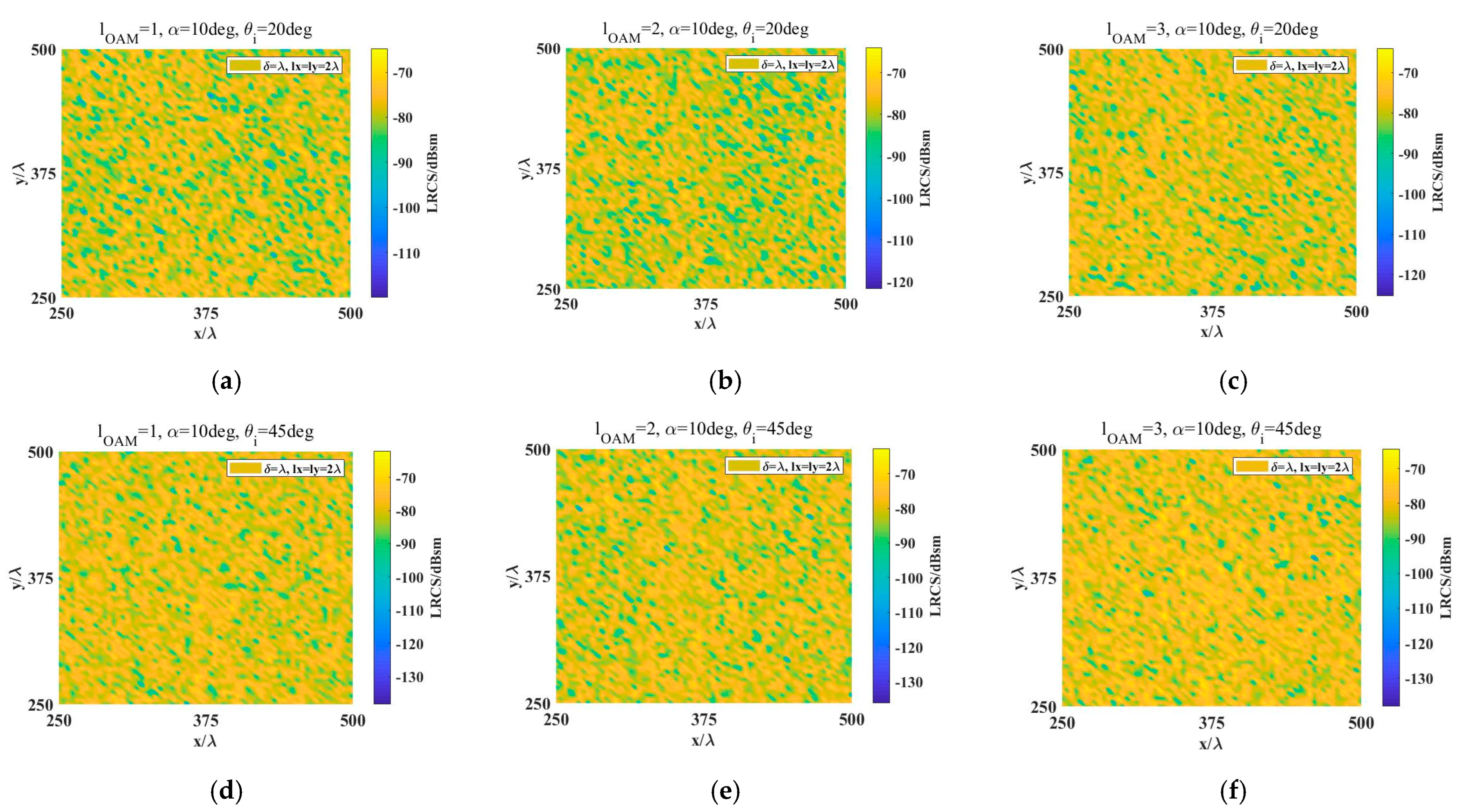
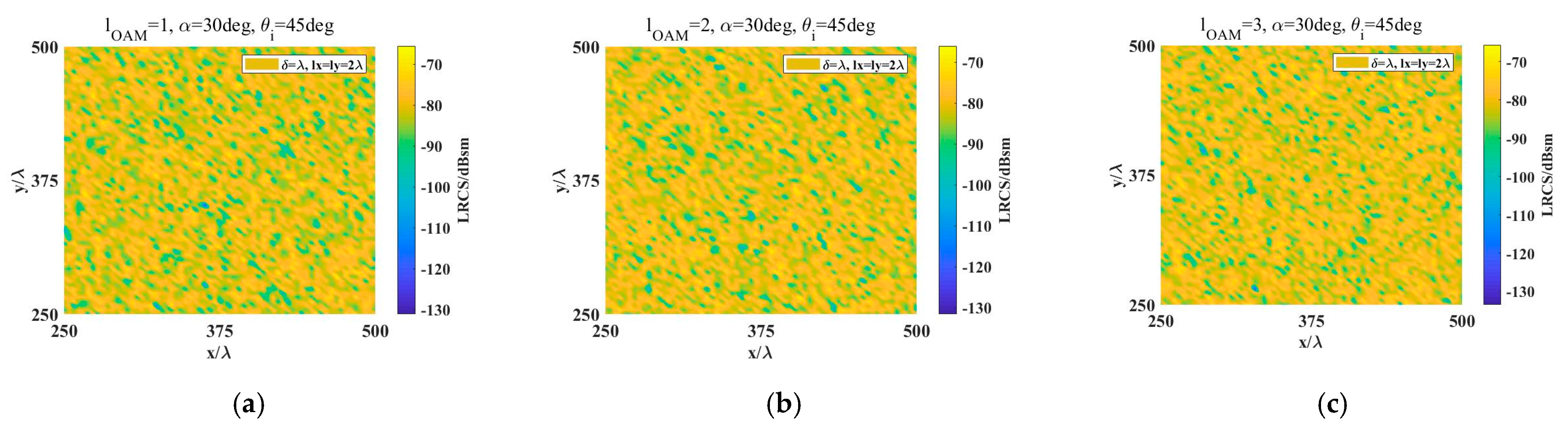
3.3. Spatial Distribution of LRCS from Rough Surface
4. Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gibson, G.; Courtial, J.; Padgett, M.J.; Vasnetsov, M.; Pas’ko, V.; Barnett, S.M.; Franke-Arnold, S. Free-space information transfer using light beams carrying orbital angular momentum. Opt. Express 2004, 12, 5448–5456. [Google Scholar] [CrossRef] [PubMed]
- Willner, A.E. OAM Light for Communications. Opt. Photonics News 2021, 32, 34–41. [Google Scholar] [CrossRef]
- Lei, Q.; Gong, H.; Tu, S.; Cai, Y.; Zhao, Q. Experimental Generation of Structured Light Beams through Highly Anisotropic Scattering Media with an Intensity Transmission Matrix Measurement. Photonics 2023, 10, 737. [Google Scholar] [CrossRef]
- Volyar, A.; Abramochkin, E.; Bretsko, M.; Khalilov, S.; Akimova, Y. General Astigmatism of Structured LG Beams: Evolution and Transformations of the OAM Super-Bursts. Photonics 2023, 10, 727. [Google Scholar] [CrossRef]
- Bai, Q.; Tennant, A.; Cano, E.; Allen, B. Experimental circular phased array for generating OAM radio beams. Electron. Lett. 2014, 50, 1414–1415. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Y.; Wang, D.; Shan, L.; Xia, M.; Zhao, Y. Statistical distribution of the OAM states of Bessel–Gaussian–Schell infrared beams in strong turbulent atmosphere. Infrared Phys. Technol. 2016, 76, 569–573. [Google Scholar] [CrossRef]
- Olivier, A.; Dimitri, M.; Mikael, K.; Brunella, C.; Valentin, C.; Denis, D.; Christian, D.; Bruno, F.C.; Pontus, F.; Julien, G.; et al. Three years of harvest with the vector vortex coronagraph in the thermal infrared. Ground-based and Airborne Instrumentation for Astronomy VI. SPIE 2016, 9908, 182–195. [Google Scholar]
- Wang, Y.; Fang, J.; Zheng, T.; Liang, Y.; Hao, Q.; Wu, E.; Yan, M.; Huang, K.; Zeng, H. Mid-Infrared Single-Photon Edge Enhanced Imaging Based on Nonlinear Vortex Filtering. Laser Photonics Rev. 2021, 15, 2100189. [Google Scholar] [CrossRef]
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.J.C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185. [Google Scholar] [CrossRef] [PubMed]
- Garces-Chavez, V.; Arlt, J.; Dholakia, K.; Volke-Sepulveda, K.; Chavez-Cerda, S. Orbital angular momentum of a high order Bessel light beam. In Summaries of Papers Presented at the Lasers and Electro-Optics. CLEO '02. Technical Diges; IEEE: Long Beach, CA, USA, 2002; Volume 1, pp. 223–224. [Google Scholar]
- Li, Y.; Wang, L.; Gong, L.; Wang, Q. Speckle characteristics of vortex beams scattered from rough targets in turbulent atmosphere. J. Quant. Spectrosc. Radiat. Transf. 2020, 257, 107342. [Google Scholar] [CrossRef]
- Ju, P.; Fan, W.; Gao, W.; Li, Z.; Gao, Q.; Jiang, X.; Zhang, T. Atmospheric Turbulence Effects on the Performance of Orbital Angular Momentum Multiplexed Free-Space Optical Links Using Coherent Beam Combining. Photonics 2023, 10, 634. [Google Scholar] [CrossRef]
- Dong, K.; Cheng, M.; Lavery, M.P.; Geng, S.; Wang, P.; Guo, L. Scattering of partially coherent vortex beam by rough surface in atmospheric turbulence. Opt. Express 2022, 30, 4165–4178. [Google Scholar] [CrossRef] [PubMed]
- Mitri, F.G. Electromagnetic Wave Scattering of a High-Order Bessel Vortex Beam by a Dielectric Sphere. IEEE Trans. Antennas Propag. 2011, 59, 4375–4379. [Google Scholar] [CrossRef]
- Tan, Q.; Wu, Z. Interactions of high-order Bessel vortex beam with a multilayered chiral sphere: Scattering and orbital angular momentum spectrum analysis. J. Quant. Spectrosc. Radiat. Transf. 2018, 217, 363–372. [Google Scholar]
- Es’kin, V.A.; Kudrin, A.V.; Popova, L.L. Scattering of an electromagnetic vortex Bessel beam by a gyrotropic cylinder perpendicular to the beam symmetry axis. In Proceedings of the 2016 Progress in Electromagnetic Research Symposium (PIERS), Shanghai, China, 8–11 August 2016; pp. 3246–3250. [Google Scholar]
- Voti, R.L.; Leahu, G.L.; Gaetani, S.; Sibilia, C.; Violante, V.; Castagna, E.; Bertolotti, M. Light scattering from a rough metal surface: Theory and experiment. J. Opt. Soc. Am. B-Opt. Physics. 2009, 26, 1585–1593. [Google Scholar] [CrossRef]
- Fuks, M. Wave diffraction by a rough boundary of an arbitrary plane-layered medium. IEEE Trans. Antennas Propag. 2001, 49, 630–639. [Google Scholar] [CrossRef]
- Zeng, J.; Chen, K.-S.; Bi, H.; Zhao, T.; Yang, X. A Comprehensive Analysis of Rough Soil Surface Scattering and Emission Predicted by AIEM With Comparison to Numerical Simulations and Experimental Measurements. IEEE Trans. Geosci. Remote Sens. 2017, 55, 1696–1708. [Google Scholar] [CrossRef]
- Jin, M.; Chen, K.-S.; Xie, D. On the Very High-Resolution Radar Image Statistics of the Exponentially Correlated Rough Surface: Experimental and Numerical Studies. Remote Sens. 2018, 10, 1369. [Google Scholar] [CrossRef]
- Zhang, X.; Su, X.; Wu, Z. Analysis of electromagnetic scattering from typical targets for orbital-angular-momentum waves: Theoretical model. IET Microw. Antennas Propag. 2022, 16, 699–708. [Google Scholar] [CrossRef]
- Ulaby, F.T.; Moore, R.K. Microwave Remote Sensing Active and Passive: Radar Remote Sensing and Surface Scattering and Emission Theory. Artech House. 1982, Chap.12, 937–941. [Google Scholar]
- Adachi, S. The Handbook on Optical Constants of Metals: In Tables and Figures; World Scientific Publishing Co. Pte. Ltd.: Singapore, 2012. [Google Scholar]

| Material | |||
|---|---|---|---|
| aluminum | 4.7784 | −2098 | 760.6 |
| 5.1765 | −2424 | 905.2 | |
| 8.8741 | −6205 | 3410 | |
| 10.3531 | −7787 | 4899 | |
| 12.4238 | −9964 | 7279 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Su, X.; Wu, Z.; Wang, S. Analyzing Vortex Light Beam Scattering Characteristics from a Random Rough Surface. Photonics 2023, 10, 955. https://doi.org/10.3390/photonics10090955
Zhang X, Su X, Wu Z, Wang S. Analyzing Vortex Light Beam Scattering Characteristics from a Random Rough Surface. Photonics. 2023; 10(9):955. https://doi.org/10.3390/photonics10090955
Chicago/Turabian StyleZhang, Xiaoxiao, Xiang Su, Zhensen Wu, and Shanzhe Wang. 2023. "Analyzing Vortex Light Beam Scattering Characteristics from a Random Rough Surface" Photonics 10, no. 9: 955. https://doi.org/10.3390/photonics10090955
APA StyleZhang, X., Su, X., Wu, Z., & Wang, S. (2023). Analyzing Vortex Light Beam Scattering Characteristics from a Random Rough Surface. Photonics, 10(9), 955. https://doi.org/10.3390/photonics10090955





