From Localized Laser Energy Absorption to Absorption Delocalization at Volumetric Glass Modification with Gaussian and Doughnut-Shaped Pulses
Abstract
1. Introduction
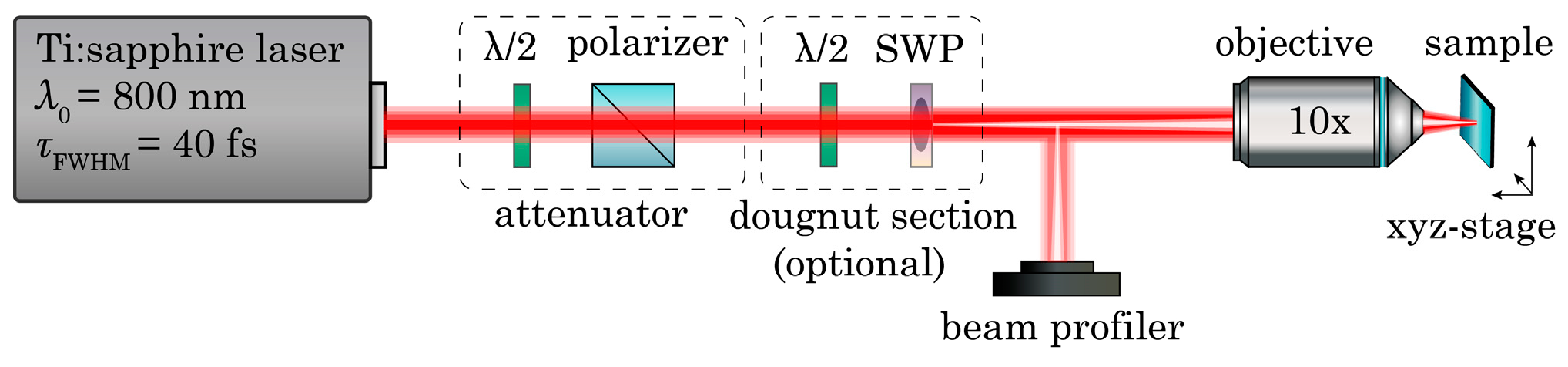
2. Experiment
3. Theoretical Model
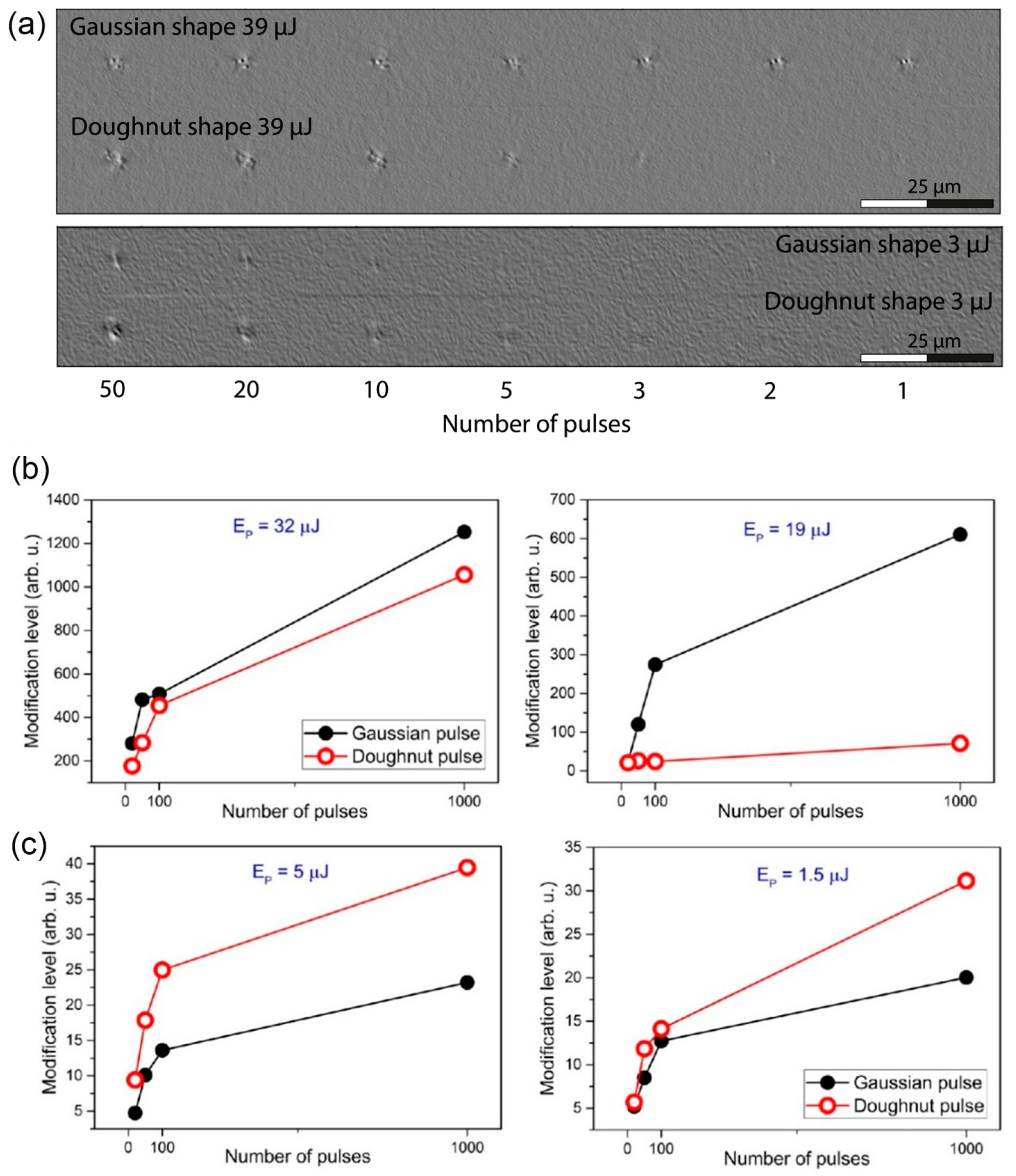
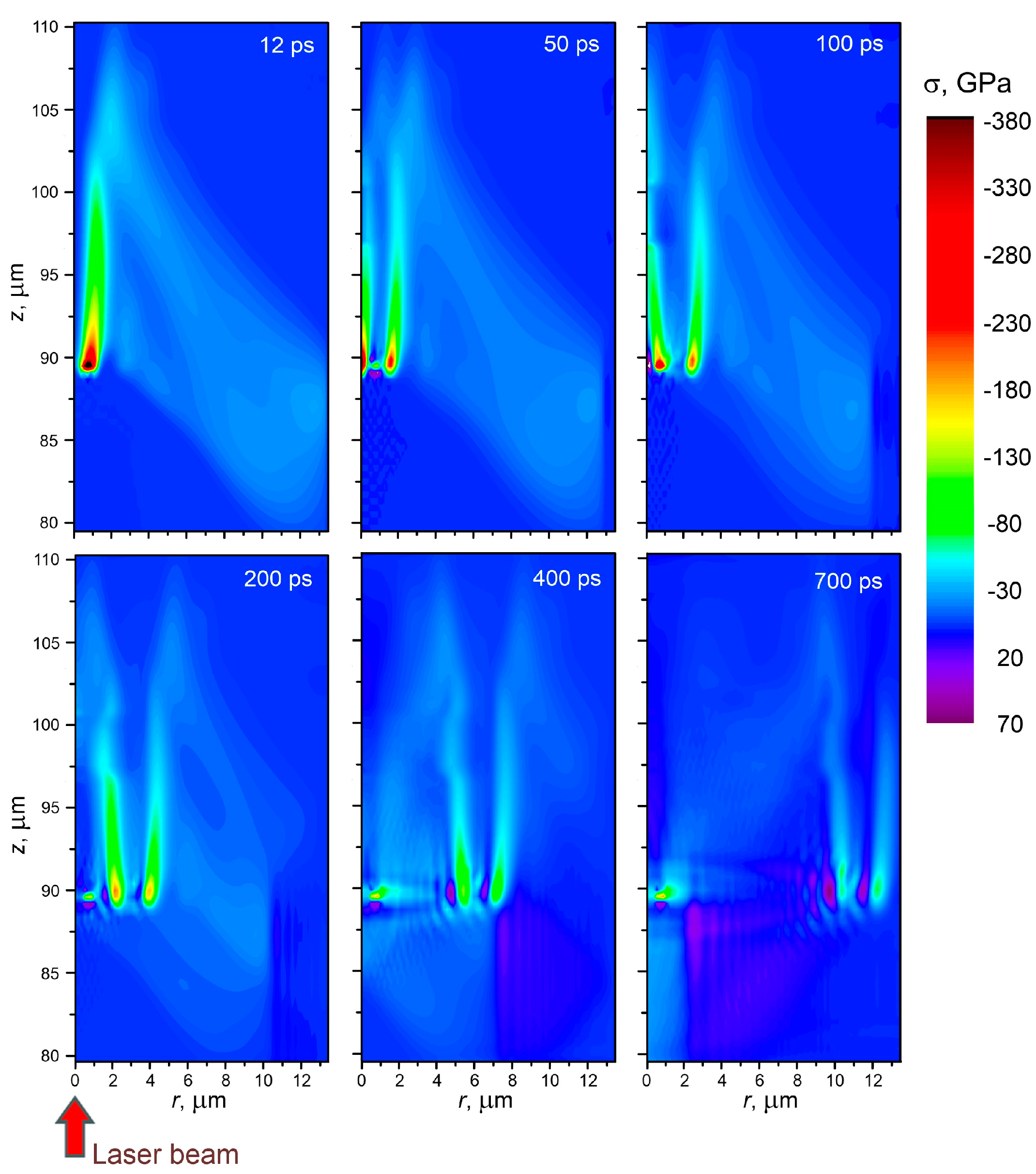
4. Results and Discussion
5. Possible Implications of Volumetric Modification of Transparent Dielectrics by Doughnut-Shaped Laser Pulses
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Dedication
References
- Davis, K.M.; Miura, K.; Sugimoto, N.; Hirao, K. Writing waveguides in glass with a femtosecond laser. Opt. Lett. 1996, 21, 1729–1731. [Google Scholar] [CrossRef]
- Homoelle, D.; Wielandy, S.; Gaeta, A.L.; Borrelli, N.F.; Smith, C. Infrared photosensitivity in silica glasses exposed to femtosecond laser pulses. Opt. Lett. 1999, 24, 1311–1313. [Google Scholar] [CrossRef]
- Burakov, I.M.; Bulgakova, N.M.; Stoian, R.; Mermillod-Blondin, A.; Audouard, E.; Rosenfeld, A.; Husakou, A.; Hertel, I.V. Spatial distribution of refractive index variations induced in bulk fused silica by single ultrashort and short laser pulses. J. Appl. Phys. 2007, 101, 043506. [Google Scholar] [CrossRef]
- Juodkazis, S.; Kohara, S.; Ohishi, Y.; Hirao, N.; Vailionis, A.; Mizeikis, V.; Saito, A.; Rode, A. Structural changes in femtosecond laser modified regions inside fused silica. J. Opt. 2010, 12, 124007. [Google Scholar] [CrossRef]
- Alimohammadian, E.; Ertorer, E.; Uzeda, E.M.; Li, J.; Herman, P.R. Inhibition and enhancement of linear and nonlinear optical effects by conical phase front shaping for femtosecond laser material processing. Sci. Rep. 2020, 10, 21528. [Google Scholar] [CrossRef]
- Kononenko, V.V.; Pashinin, V.P.; Komlenok, M.S.; Konov, V.I. Laser-induced modification of bulk fused silica by femtosecond pulses. Laser Phys. 2009, 19, 1294–1299. [Google Scholar] [CrossRef]
- Canning, J.; Lancry, M.; Cook, K.; Weickman, A.; Brisset, F.; Poumellec, B. Anatomy of a femtosecond laser processed silica waveguide. Opt. Mater. Express 2011, 1, 998–1008. [Google Scholar] [CrossRef]
- Cheng, G.H.; Lin, L.; Mishchik, K.; Stoian, R. Polarization-dependent scattering of nanogratings in femtosecond laser photowritten waveguides in fused silica. Materials 2022, 15, 5698. [Google Scholar] [CrossRef]
- Ohfuchi, T.; Sakakura, M.; Yamada, Y.; Fukuda, N.; Takiya, T.; Shimotsuma, Y.; Miura, K. Polarization imaging camera with a waveplate array fabricated with a femtosecond laser inside silica glass. Opt. Express 2017, 25, 23738–23754. [Google Scholar] [CrossRef] [PubMed]
- Tian, J.; Yao, H.; Cavillon, M.; Garcia-Caure, E.; Ossikovski, R.; Stchakovsky, M.; Eypert, C.; Poumellec, B.; Lancry, M. A comparison between nanogratings-based and stress-engineered waveplates written by femtosecond laser in silica. Micromachines 2020, 11, 131. [Google Scholar] [CrossRef] [PubMed]
- Mihailov, S.J.; Smelser, C.W.; Grobnic, D.; Walker, R.B.; Lu, P.; Ding, H.M.; Unruh, J. Bragg gratings written in all-SiO2 and Ge-doped core fibers with 800-nm femtosecond radiation and a phase mask. J. Light. Technol. 2004, 22, 94–100. [Google Scholar] [CrossRef]
- Martinez, A.; Dubov, M.; Khrushchev, I.; Bennion, I. Direct writing of fibre Bragg gratings by femtosecond laser. Electron. Lett. 2004, 40, 1170–1172. [Google Scholar] [CrossRef]
- Li, H.; Yang, B.; Wang, M.; Gao, C.; Wu, B.; Zeng, L.; Xi, X.; Chen, Z.; Wang, X.; Wang, Z.; et al. Femtosecond laser fabrication of large-core fiber Bragg gratings for high-power fiber oscillators. APL Photonics 2023, 8, 046101. [Google Scholar] [CrossRef]
- Glezer, E.N.; Milosavljevic, M.; Huang, L.; Finlay, R.J.; Her, T.-H.; Callan, J.P.; Mazur, E. Three-dimensional optical storage inside transparent materials. Opt. Lett. 1996, 21, 2023–2025. [Google Scholar] [CrossRef] [PubMed]
- Qiu, J.R.; Miura, K.; Hirao, K. Three-dimensional optical memory using glasses as a recording medium through a multi-photon absorption process. Jpn. J. Appl. Phys. 1998, 37, 2263–2266. [Google Scholar] [CrossRef]
- Shimotsuma, Y.; Sakakura, M.; Kazansky, P.G.; Beresna, M.; Qiu, J.R.; Miura, K.; Hirao, K. Ultrafast manipulation of self-assembled form birefringence in glass. Adv. Mater. 2010, 22, 4039–4043. [Google Scholar] [CrossRef]
- Cai, W.J.; Libertun, A.R.; Piestun, R. Polarization selective computer-generated holograms realized in glass by femtosecond laser induced nanogratings. Opt. Express 2006, 14, 3785–3791. [Google Scholar] [CrossRef]
- Sugioka, K.; Cheng, Y. Femtosecond laser processing for optofluidic fabrication. Lab Chip 2012, 12, 3576–3589. [Google Scholar] [CrossRef]
- Sima, F.; Sugioka, K.; Vazquez, R.M.; Osellame, R.; Kelemen, L.; Ormos, P. Three-dimensional femtosecond laser processing for lab-on-a-chip applications. Nanophotonics 2018, 7, 613–634. [Google Scholar] [CrossRef]
- Diez-Blanco, V.; Siegel, J.; Ferrer, A.; de la Cruz, A.R.; Solis, J. Deep subsurface waveguides with circular cross section produced by femtosecond laser writing. Appl. Phys. Lett. 2007, 91, 051104. [Google Scholar] [CrossRef]
- Mermillod-Blondin, A.; Burakov, I.M.; Meshcheryakov, Y.P.; Bulgakova, N.M.; Audouard, E.; Rosenfeld, A.; Husakou, A.; Hertel, I.V.; Stoian, R. Flipping the sign of refractive index changes in ultrafast-laser-irradiated borosilicate crown optical glass at high repetition rates. Phys. Rev. B 2008, 77, 104205. [Google Scholar] [CrossRef]
- Zambon, V.; McCarthy, N.; Piché, M. Laser micromachining of transparent glass using ultrafast Bessel beams. In Proceedings of the Photonics North 2009, Montréal, QC, USA, 24–27 May 2009; Volume 7386, p. 738632. [Google Scholar] [CrossRef]
- Courvoisier, F.; Stoian, R.; Couairon, A. Ultrafast laser micro- and nano-processing with nondiffracting and curved beams. Opt. Laser Technol. 2016, 80, 125–137. [Google Scholar] [CrossRef]
- Shen, T.; Tian, D.; Chen, T.; Li, P.; Hou, X. The spatiotemporal dynamics of electron plasma in femtosecond laser double pulses induced damage in fused silica. Photonics 2023, 10, 702. [Google Scholar] [CrossRef]
- Paipulas, D.; Mikutis, M.; Sirutkaitis, V.; Juodkazis, S. Volumetric modifications in fused silica using Gaussian and Bessel femtosecond laser beams. In Pacific Rim Laser Damage 2013: Optical Materials for High Power Lasers; SPIE: Paris, France, 2013; Volume 8786, pp. 66–73. [Google Scholar] [CrossRef]
- Vetter, C.; Giust, R.; Furfaro, L.; Billet, C.; Froehly, L.; Courvoisier, F. High aspect ratio structuring of glass with ultrafast Bessel beams. Materials 2021, 14, 6749. [Google Scholar] [CrossRef] [PubMed]
- Ardaneh, K.; Giust, R.; Charpin, P.-J.; Morel, B.; Courvoisier, F. Electron heating and radiation in high aspect ratio sub-micron plasma generated by an ultrafast Bessel pulse within a solid dielectric. Eur. Phys. J. Spec. Top. 2022. [Google Scholar] [CrossRef]
- Le, H.; Penchev, P.; Henrottin, A.; Bruneel, D.; Nasrollahi, V.; Ramos-de-Campos, J.A.; Dimov, S. Effects of top-hat laser beam processing and scanning strategies in laser micro-structuring. Micromachines 2020, 11, 221. [Google Scholar] [CrossRef] [PubMed]
- Möhl, A.; Kaldun, S.; Kunz, C.; Müller, F.A.; Fuchs, U.; Gräf, S. Tailored focal beam shaping and its application in laser material processing. J. Laser Appl. 2019, 31, 042019. [Google Scholar] [CrossRef]
- Zhukov, V.P.; Rubenchik, A.M.; Fedoruk, M.P.; Bulgakova, N.M. Interaction of doughnut-shaped laser pulses with glasses. J. Opt. Soc. Am. B 2017, 34, 463–471. [Google Scholar] [CrossRef]
- Couairon, A.; Mysyrowicz, A. Femtosecond filamentation in transparent media. Phys. Rep. 2007, 441, 47–189. [Google Scholar] [CrossRef]
- Gildenburg, V.B.; Pavlichenko, I.A. High contrast periodic plasma pattern formation during the laser-induced breakdown in transparent dielectric. Phys. Plasmas 2017, 24, 122306. [Google Scholar] [CrossRef]
- Bulgakova, M.M.; Zhukov, V.P.; Meshcheryakov, Y.P.; Gemini, L.; Brajer, J.; Rostohar, D.; Mocek, T. Pulsed laser modification of transparent dielectrics: What can be foreseen and predicted by numerical simulations? J. Opt. Soc. Am. B 2014, 31, C8–C14. [Google Scholar] [CrossRef]
- Itoh, C.; Tanimura, K.; Itoh, N. Optical studies of self-trapped excitons in SiO2. J. Phys. C Solid State Phys. 1988, 21, 4693–4702. [Google Scholar] [CrossRef]
- Audebert, P.; Daguzan, P.; Dos Santos, A.; Gauthier, J.C.; Geindre, J.P.; Guizard, S.; Hamoniaux, G.; Krastev, K.; Martin, P.; Petite, G.; et al. Space-time observation of an electron gas in SiO2. Phys. Rev. Lett. 1994, 73, 1990–1993. [Google Scholar] [CrossRef] [PubMed]
- Grojo, D.; Gertsvolf, M.; Lei, S.; Barillot, T.; Rayner, D.M.; Corkum, P.B. Exciton-seeded multiphoton ionization in bulk SiO2. Phys. Rev. B 2010, 81, 212301. [Google Scholar] [CrossRef]
- Kaiser, A.; Rethfeld, B.; Vicanek, M.; Simon, G. Microscopic processes in dielectrics under irradiation by subpicosecond laser pulses. Phys. Rev. B 2000, 61, 11437–11450. [Google Scholar] [CrossRef]
- Martin, P.; Guizard, S.; Daguzan, P.; Petite, G.; D’Oliveira, P.; Meynadier, P.; Perdrix, M. Subpicosecond study of carrier trapping dynamics in wide-band-gap crystals. Phys. Rev. B 1997, 55, 5799. [Google Scholar] [CrossRef]
- Bulgakova, N.M.; Zhukov, V.P.; Meshcheryakov, Y.P. Theoretical treatments of ultrashort pulse laser processing of transparent materials: Towards understanding the volume nanograting formation and “quill” writing effect. Appl. Phys. B 2013, 113, 437–449. [Google Scholar] [CrossRef]
- Zhukov, V.P.; Fedoruk, M.P. Numerically implemented impact of a femtosecond laser pulse on glass in the approximation of nonlinear Maxwell equations. Math. Models Comput. Simul. 2020, 12, 77–89. [Google Scholar] [CrossRef]
- Popov, K.I.; Bychenkov, V.Y.; Rozmus, W.; Sydora, R.D.; Bulanov, S.S. Vacuum electron acceleration by tightly focused laser pulses with nanoscale targets. Phys. Plasmas 2009, 16, 053106. [Google Scholar] [CrossRef]
- Couairon, A.; Kosareva, O.G.; Panov, N.A.; Shipilo, D.E.; Andreeva, V.A.; Jukna, V.; Nesa, F. Propagation equation for tight-focusing by a parabolic mirror. Opt. Express 2015, 23, 31240–31252. [Google Scholar] [CrossRef]
- Stratton, J.A.; Chu, L.J. Diffraction Theory of Electromagnetic Waves. Phys. Rev. 1939, 56, 99. [Google Scholar] [CrossRef]
- Zhukov, V.P.; Fedoruk, M.P. High efficient method for calculation of Stratton-Chu integral in problems of interaction of laser radiation with materials. Comput. Technol. 2021, 26, 42–60. [Google Scholar] [CrossRef]
- Bulgakova, N.M.; Zhukov, V.P.; Sonina, S.V.; Meshcheryakov, Y.P. Modification of transparent materials with ultrashort laser pulses: What is energetically and mechanically meaningful? J. Appl. Phys. 2015, 118, 233108. [Google Scholar] [CrossRef]
- Zavedeev, E.V.; Kononenko, V.V.; Gololobov, V.M.; Konov, V.I. Modeling the effect of fs light delocalization in Si bulk. Laser Phys. Lett. 2014, 11, 036002. [Google Scholar] [CrossRef]
- Liu, V.I.; Petit, S.; Becker, A.; Aközbek, N.; Bowden, C.M.; Chin, S.L. Intensity clamping of a femtosecond laser pulse in condensed matter. Opt. Commun. 2002, 202, 189–197. [Google Scholar] [CrossRef]
- Sun, Q.; Liang, F.; Vallee, R.; Chin, S.L. Nanograting formation on the surface of silica glass by scanning focused femtosecond laser pulses. Opt. Lett. 2008, 33, 2713–2715. [Google Scholar] [CrossRef] [PubMed]
- Stoian, R.; Bonse, J. (Eds.) Ultrafast Laser Nanostructuring: The Pursuit of Extreme Scales. In Springer Series in Optical Sciences; Springer: Cham, Switzerland, 2023. [Google Scholar] [CrossRef]
- Schultze, M.; Bothschafter, E.M.; Sommer, A.; Holzner, S.; Schweinberger, W.; Fiess, M.; Hofstetter, M.; Kienberger, R.; Apalkov, V.; Yakovlev, V.S.; et al. Controlling dielectrics with the electric field of light. Nature 2012, 493, 75–78. [Google Scholar] [CrossRef] [PubMed]
- Derrien, T.J.-Y.; Tancogne-Dejean, N.; Zhukov, V.P.; Appel, H.; Rubio, A.; Bulgakova, N.M. Photoionization and transient Wannier-Stark ladder in silicon: First-principles simulations versus Keldysh theory. Phys. Rev. B 2021, 104, L241201. [Google Scholar] [CrossRef]
- Borrelli, N.F.; Smith, C.; Allan, D.C.; Seward, T.P. Densification of fused silica under 193-nm excitation. J. Opt. Soc. Am. B 1997, 14, 1606–1615. [Google Scholar] [CrossRef]
- Brückner, R. Properties and structure of vitreous silica. I. J. Non-Cryst. Solids 1070, 5, 123–175. [Google Scholar] [CrossRef]
- Kazansky, P.G.; Shimotsuma, Y.; Sakakura, M.; Beresna, M.; Gecevičius, M.; Svirko, Y.; Akturk, S.; Qiu, J.; Miura, K.; Hirao, K. Photosensitivity control of an isotropic medium through polarization of light pulses with tilted intensity front. Opt. Express 2011, 19, 20657–20664. [Google Scholar] [CrossRef]
- Zhang, F.; Xie, X.; Zhao, X.; Ma, L.; Lei, L.; Qiu, J.; Nie, Z. Polarization-dependent microstructural evolution induced by a femtosecond laser in an aluminosilicate glass. Opt. Express 2021, 29, 10265–10274. [Google Scholar] [CrossRef] [PubMed]
- Sakakura, M.; Yoshimura, K.; Kurita, T.; Shimizu, M.; Shimotsuma, Y.; Fukuda, N.; Hirao, K.; Miura, K. Condensation of Si-rich region inside soda-lime glass by parallel femtosecond laser irradiation. Opt. Express 2014, 22, 16493–16503. [Google Scholar] [CrossRef] [PubMed]
- Macias-Montero, M.; Muñoz, F.; Sotillo, B.; del Hoyo, J.; Ariza, R.; Fernandez, P.; Siegel, J.; Solis, J. Femtosecond laser induced thermophoretic writing of waveguides in silicate glass. Sci. Rep. 2021, 11, 8390. [Google Scholar] [CrossRef] [PubMed]
- Meshcheryakov, Y.P.; Shugaev, M.V.; Mattle, T.; Lippert, T.; Bulgakova, N.M. Role of thermal stresses on pulsed laser irradiation of thin films under conditions of microbump formation and non-vaporization forward transfer. Appl. Phys. A 2013, 113, 521. [Google Scholar] [CrossRef]
- Gleason, A.E.; Bolme, C.A.; Lee, H.J.; Nagler, B.; Galtier, E.; Milathianaki, D.; Hawreliak, J.; Kraus, R.G.; Eggert, J.H.; Fratanduono, D.E.; et al. Ultrafast visualization of crystallization and grain growth in shock-compressed SiO2. Nat. Commun. 2015, 6, 8191. [Google Scholar] [CrossRef] [PubMed]
- Vailionis, A.; Gamaly, E.G.; Mizeikis, V.; Yang, W.; Rode, A.V.; Juodkazis, S. Evidence of superdense aluminium synthesized by ultrafast microexplosion. Nat. Commun. 2011, 2, 445. [Google Scholar] [CrossRef] [PubMed]
- McCoy, C.A.; Gregor, M.C.; Polsin, D.N.; Fratanduono, D.E.; Celliers, P.M.; Boehly, T.R.; Meyerhofer, D.D. Shock-wave equation-of-state measurements in fused silica up to 1600 GPa. J. Appl. Phys. 2016, 119, 215901. [Google Scholar] [CrossRef]
- Zukas, J.A. Shock waves in solids. In Studies in Applied Mechanics; Elsevier: Amsterdam, The Netherlands, 2004; Volume 49, Chapter 3; pp. 75–102. [Google Scholar] [CrossRef]
- Sakakura, M.; Shimotsuma, Y.; Miura, K. Observation of stress wave and thermal stress in ultrashort pulse laser bulk processing inside glass. J. Laser Micro Nanoeng. 2017, 12, 159–164. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zukerstein, M.; Zhukov, V.P.; Meshcheryakov, Y.P.; Bulgakova, N.M. From Localized Laser Energy Absorption to Absorption Delocalization at Volumetric Glass Modification with Gaussian and Doughnut-Shaped Pulses. Photonics 2023, 10, 882. https://doi.org/10.3390/photonics10080882
Zukerstein M, Zhukov VP, Meshcheryakov YP, Bulgakova NM. From Localized Laser Energy Absorption to Absorption Delocalization at Volumetric Glass Modification with Gaussian and Doughnut-Shaped Pulses. Photonics. 2023; 10(8):882. https://doi.org/10.3390/photonics10080882
Chicago/Turabian StyleZukerstein, Martin, Vladimir P. Zhukov, Yuri P. Meshcheryakov, and Nadezhda M. Bulgakova. 2023. "From Localized Laser Energy Absorption to Absorption Delocalization at Volumetric Glass Modification with Gaussian and Doughnut-Shaped Pulses" Photonics 10, no. 8: 882. https://doi.org/10.3390/photonics10080882
APA StyleZukerstein, M., Zhukov, V. P., Meshcheryakov, Y. P., & Bulgakova, N. M. (2023). From Localized Laser Energy Absorption to Absorption Delocalization at Volumetric Glass Modification with Gaussian and Doughnut-Shaped Pulses. Photonics, 10(8), 882. https://doi.org/10.3390/photonics10080882




