Extinction and Independent Scattering Criterion for Clusters of Spherical Particles Embedded in Absorbing Host Media
Abstract
1. Introduction
2. Methodology
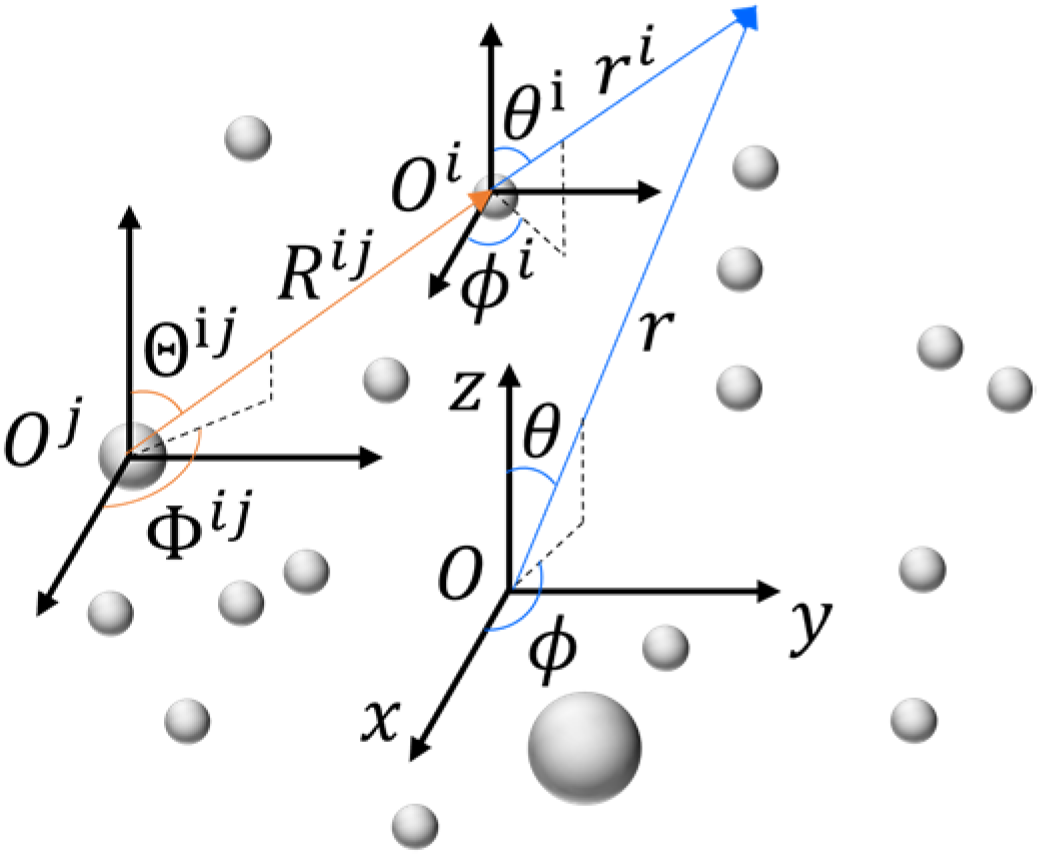
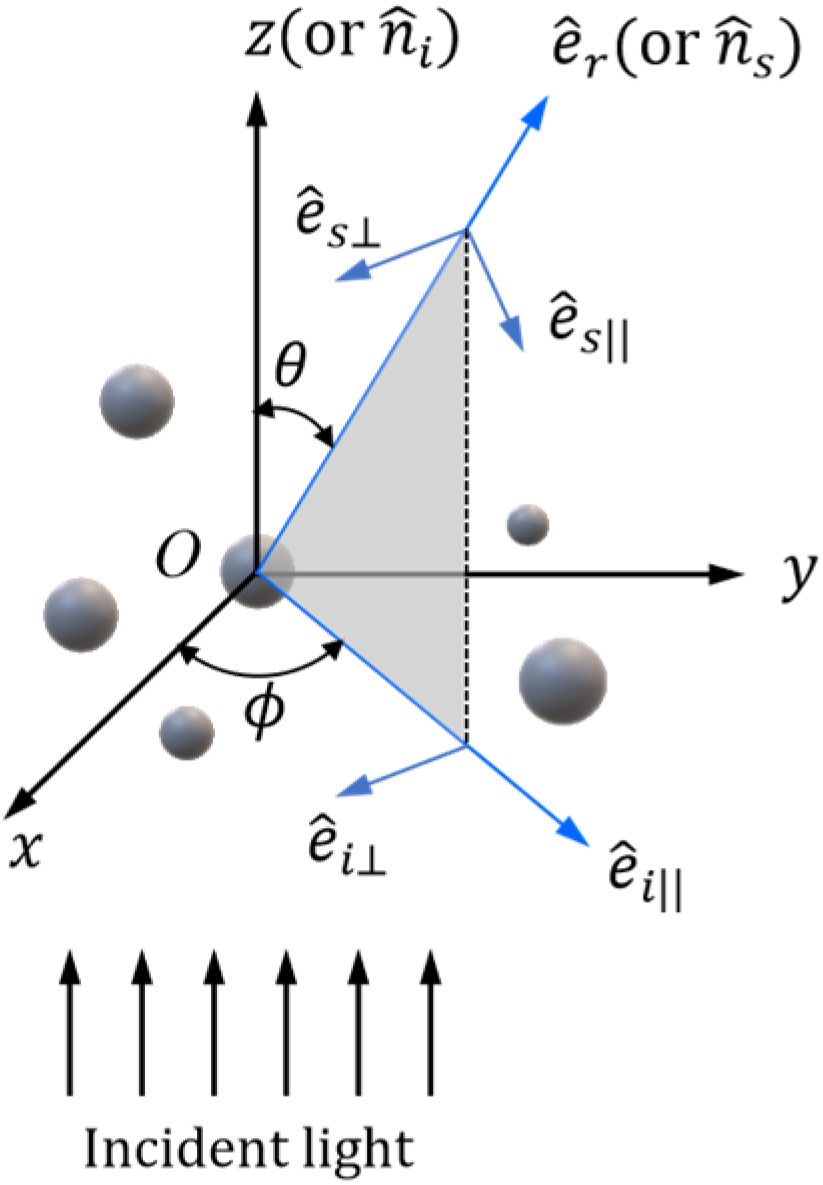
2.1. Expansion of the Scattered Field
2.2. Far-Field Extinction and Scattering of Sphere Clusters
2.3. Nanoparticle Cluster Model for Investigating the Independent Scattering Criterion
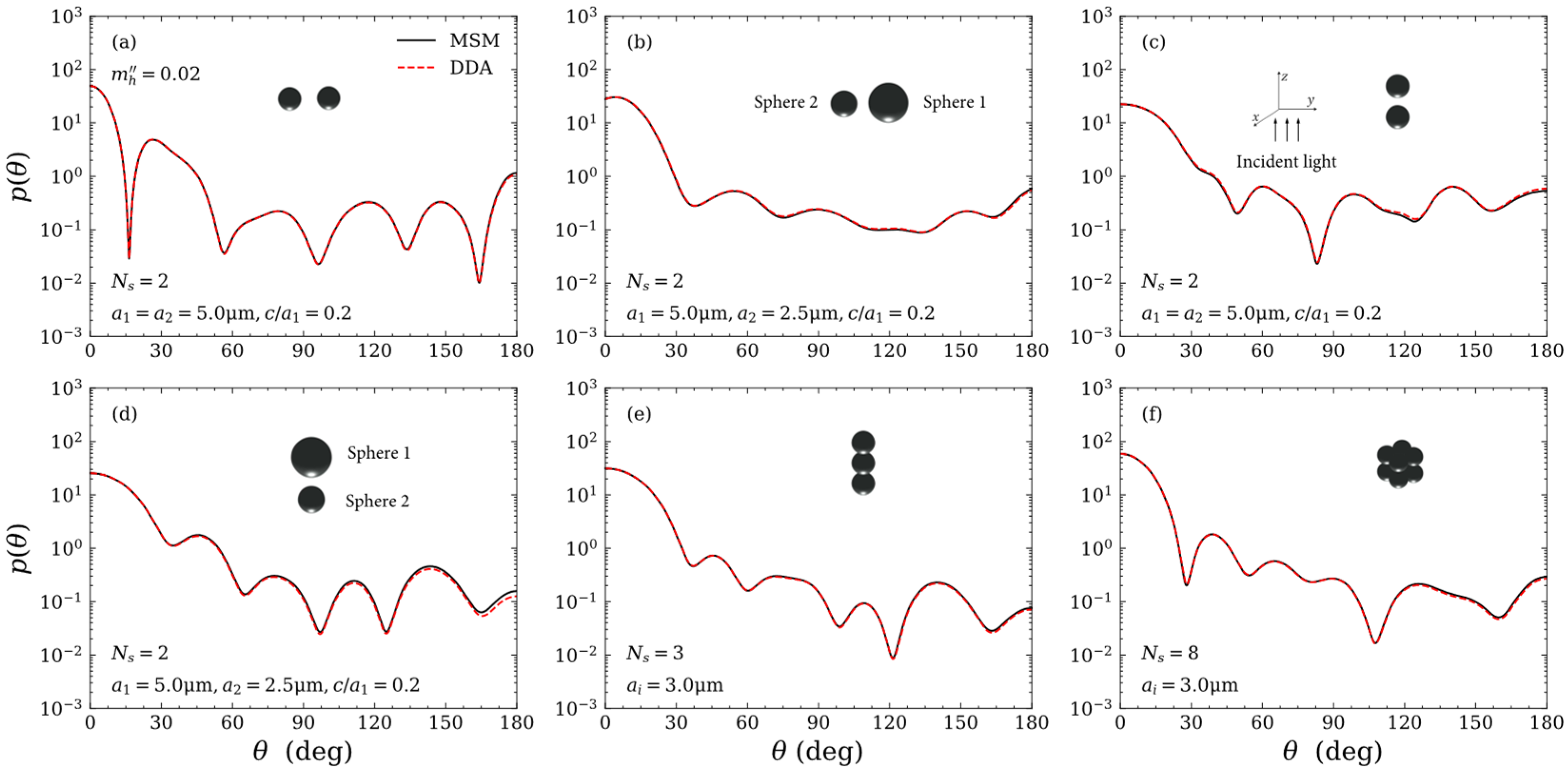
3. Validation
4. Results and Discussion
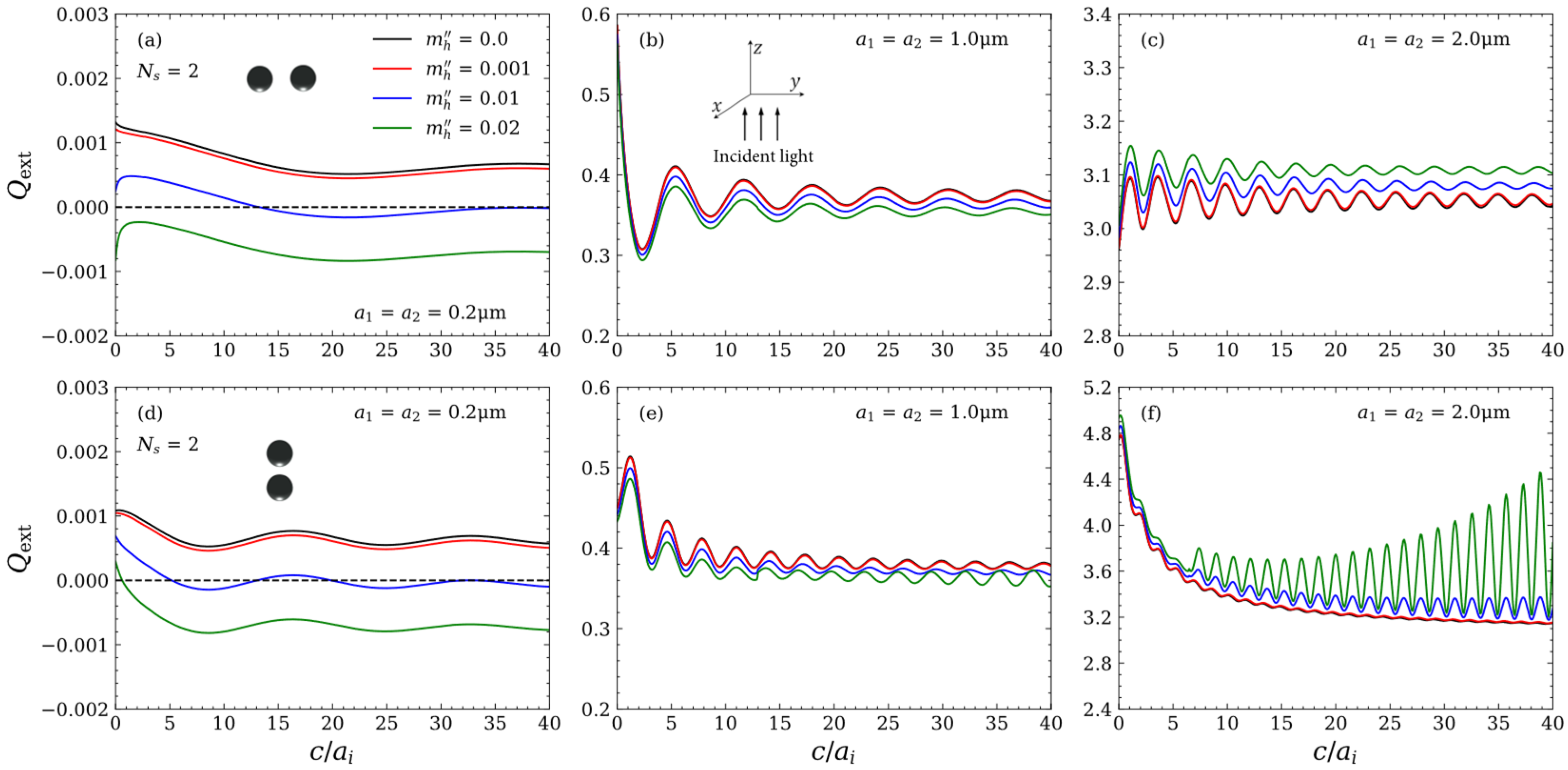
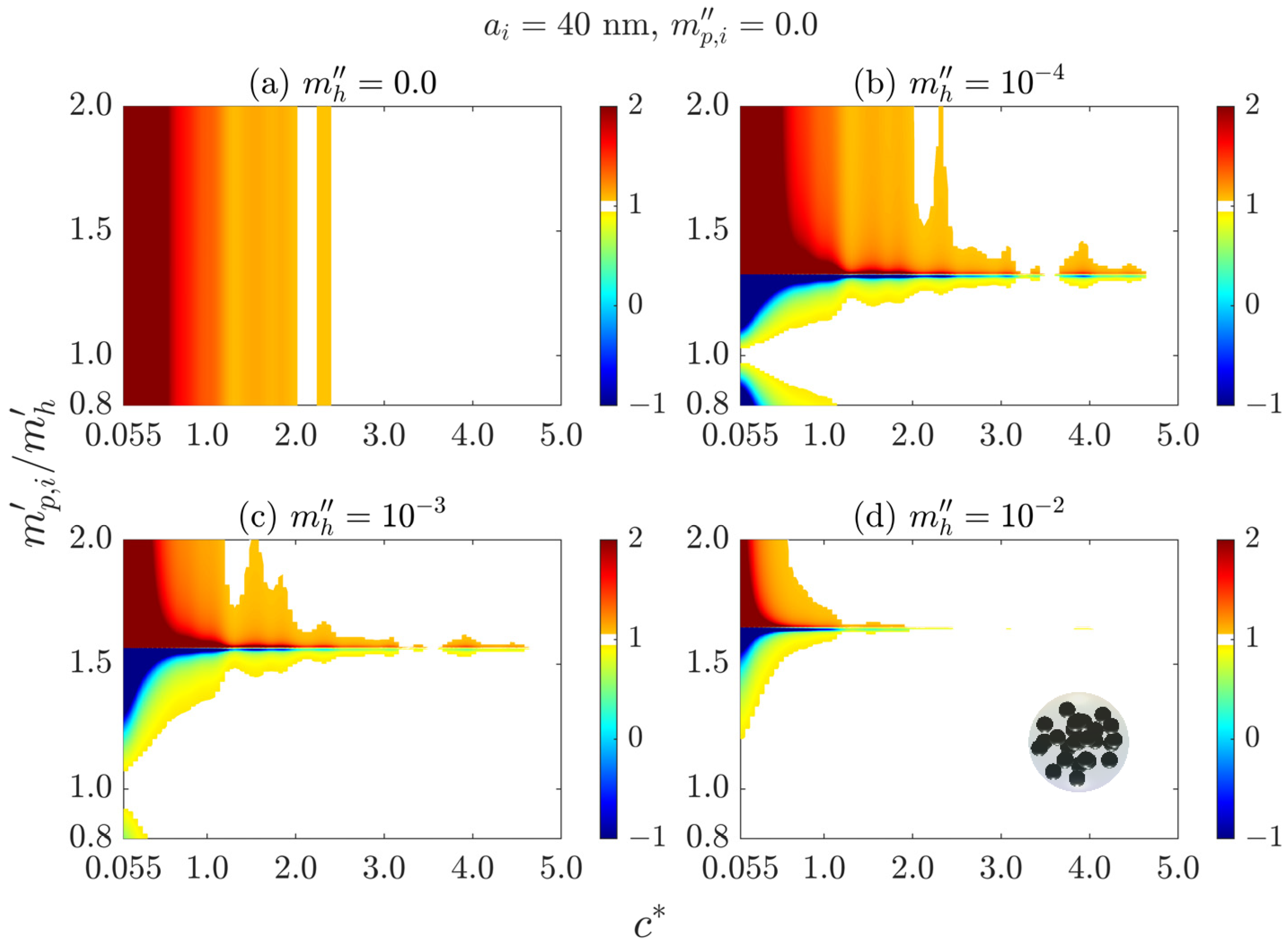
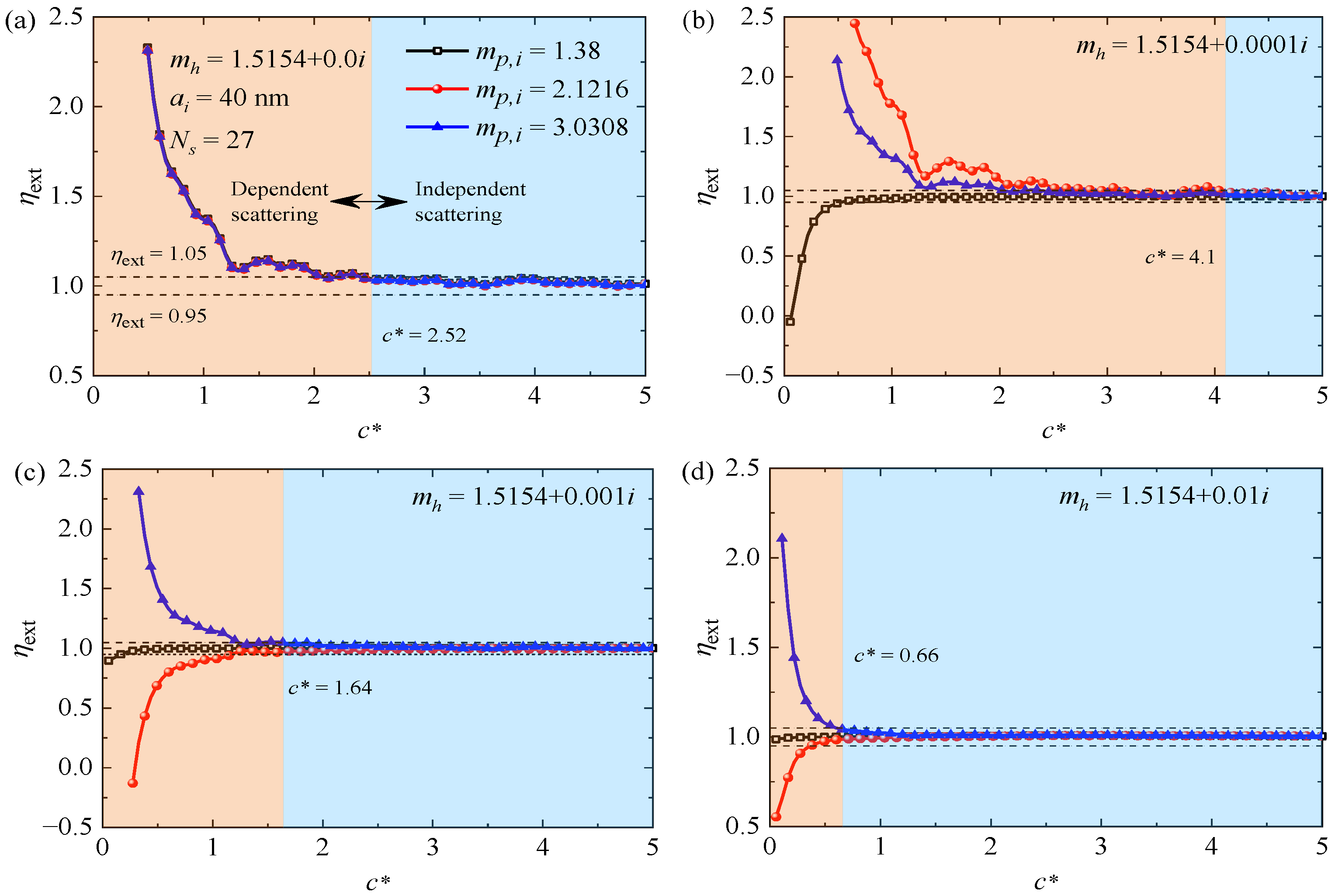
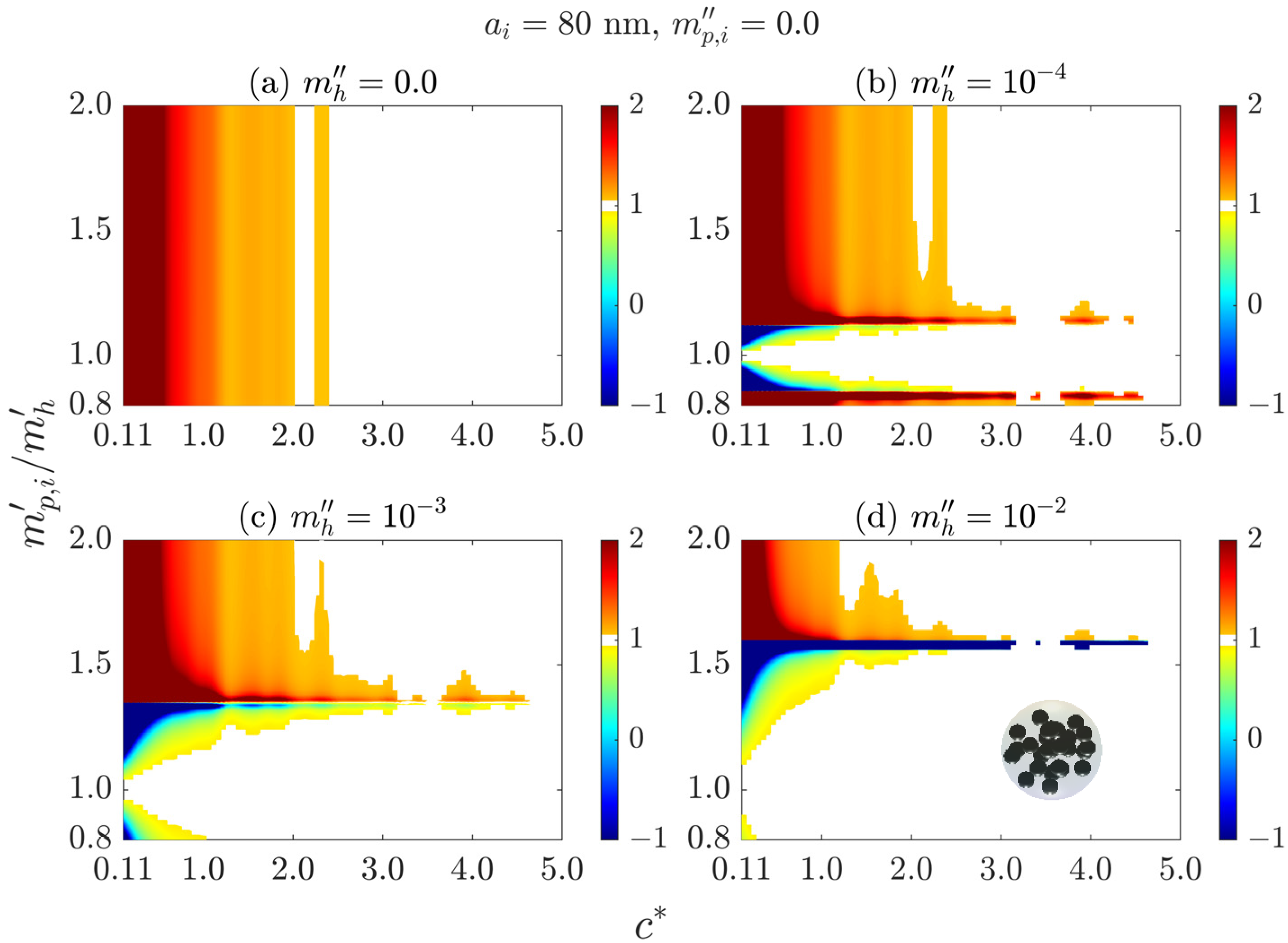
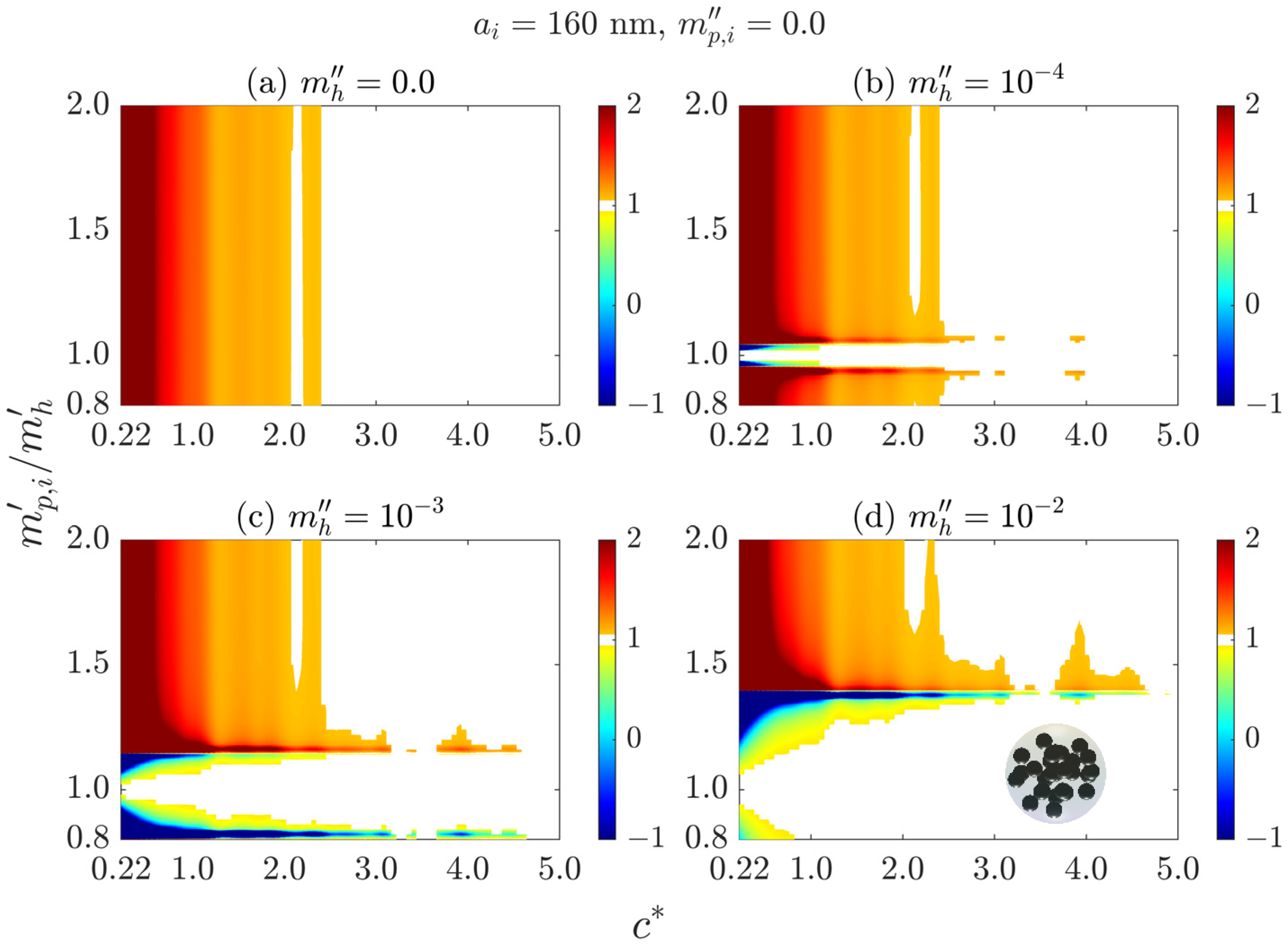
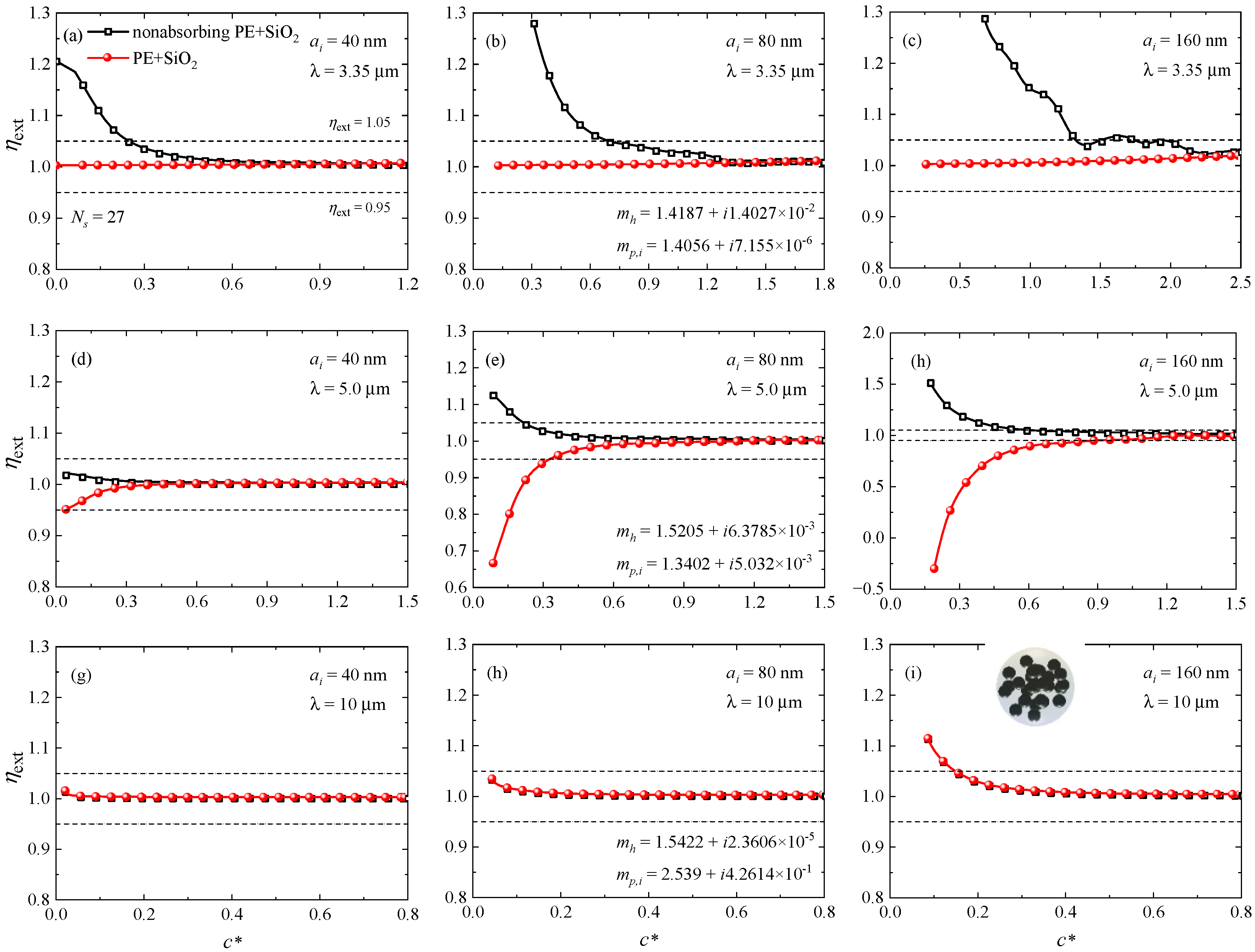
4.1. Extinction Properties of Sphere Clusters Embedded in Absorbing Host Media
4.2. Effect of Host Medium Absorption on the Dependent Scattering between Particles
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; Wiley: New York, NY, USA, 1983. [Google Scholar]
- Howell, J.R.; Mengüc, M.P.; Siegel, R. Thermal Radiation Heat Transfer; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Theobald, D.; Yu, S.; Gomard, G.; Lemmer, U. Design of Selective Reflectors Utilizing Multiple Scattering by Core–Shell Nanoparticles for Color Conversion Films. ACS Photonics 2020, 7, 1452–1460. [Google Scholar] [CrossRef]
- Gentle, A.R.; Smith, G.B. Radiative Heat Pumping from the Earth Using Surface Phonon Resonant Nanoparticles. Nano Lett. 2010, 10, 373–379. [Google Scholar] [CrossRef] [PubMed]
- Schertel, L.; Siedentop, L.; Meijer, J.-M.; Keim, P.; Aegerter, C.M.; Aubry, G.J.; Maret, G. The Structural Colors of Photonic Glasses. Adv. Opt. Mater. 2019, 7, 1900442. [Google Scholar] [CrossRef]
- Yalçın, R.A.; Blandre, E.; Joulain, K.; Drévillon, J. Colored Radiative Cooling Coatings with Nanoparticles. ACS Photonics 2020, 7, 1312–1322. [Google Scholar] [CrossRef]
- Hwang, V.; Stephenson, A.B.; Barkley, S.; Brandt, S.; Xiao, M.; Aizenberg, J.; Manoharan, V.N. Designing angle-independent structural colors using Monte Carlo simulations of multiple scattering. Proc. Natl. Acad. Sci. USA 2021, 118, e2015551118. [Google Scholar] [CrossRef]
- Boutghatin, M.; Assaf, S.; Pennec, Y.; Carette, M.; Thomy, V.; Akjouj, A.; Djafari Rouhani, B. Impact of SiO2 Particles in Polyethylene Textile Membrane for Indoor Personal Heating. Nanomaterials 2020, 10, 1968. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, J.; Wu, X.; Huang, M. Numerical analysis of light reflection and transmission in poly-disperse sea fog. Opt. Express 2020, 28, 25410–25430. [Google Scholar] [CrossRef]
- Ma, L.; Hu, L.; Jia, C.; Wang, C.; Liu, L. Quantitative Evaluation of the Phase Function Effects on Light Scattering and Radiative Transfer in Dispersed Systems. Photonics 2022, 9, 584. [Google Scholar] [CrossRef]
- Ma, L.; Hu, K.; Wang, C.; Yang, J.-Y.; Liu, L. Prediction and Inverse Design of Structural Colors of Nanoparticle Systems via Deep Neural Network. Nanomaterials 2021, 11, 3339. [Google Scholar] [CrossRef]
- Fei, T.; Lin, L.; Li, X.; Yang, J.-Y.; Zhao, J.; Liu, L. Modeling Effect of Bubbles on Time-Dependent Radiation Transfer of Microalgae in a Photobioreactor for Carbon Dioxide Fixation. Photonics 2022, 9, 864. [Google Scholar] [CrossRef]
- Mishchenko, M.I. Electromagnetic Scattering by Particles and Particle Groups: An Introduction; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar] [CrossRef]
- Penttilä, A.; Markkanen, J.; Väisänen, T.; Räbinä, J.; Yurkin, M.A.; Muinonen, K. How much is enough? The convergence of finite sample scattering properties to those of infinite media. J. Quant. Spectrosc. Radiat. Transf. 2021, 262, 107524. [Google Scholar] [CrossRef]
- Galy, T.; Huang, D.; Pilon, L. Revisiting independent versus dependent scattering regimes in suspensions or aggregates of spherical particles. J. Quant. Spectrosc. Radiat. Transf. 2020, 246, 106924. [Google Scholar] [CrossRef]
- Ma, L.; Zhai, J.; Wang, C. Investigation of the single scattering approximation through direct electromagnetic scattering simulation. OSA Contin. 2021, 4, 2496–2509. [Google Scholar] [CrossRef]
- Chen, J.; Zhao, C.Y.; Wang, B.X. Effect of nanoparticle aggregation on the thermal radiation properties of nanofluids: An experimental and theoretical study. Int. J. Heat Mass Transfer 2020, 154, 119690. [Google Scholar] [CrossRef]
- Aoyu, Z.; Fuqiang, W.; Ziming, C.; Huaxu, L.; Xuhang, S. Radiative property investigation of dispersed particulate medium with the consideration of non-uniform particle size distribution and dependent scattering effects. Int. J. Heat Mass Transfer 2022, 186, 122488. [Google Scholar] [CrossRef]
- Kokhanovsky, A. Springer Series in Light Scattering: Volume 1: Multiple Light Scattering, Radiative Transfer and Remote Sensing; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Kokhanovsky, A.A. Optics of Light Scattering Media: Problems and Solutions; Springer: London, UK, 2001. [Google Scholar]
- Cartigny, J.D.; Yamada, Y.; Tien, C.L. Radiative Transfer With Dependent Scattering by Particles: Part 1—Theoretical Investigation. J. Heat Transfer 1986, 108, 608–613. [Google Scholar] [CrossRef]
- Yamada, Y.; Cartigny, J.D.; Tien, C.L. Radiative Transfer With Dependent Scattering by Particles: Part 2—Experimental Investigation. J. Heat Transfer 1986, 108, 614–618. [Google Scholar] [CrossRef]
- Drolen, B.L.; Tien, C.L. Independent and dependent scattering in packed-sphere systems. J. Thermophys. Heat Transfer 1987, 1, 63–68. [Google Scholar] [CrossRef]
- Quirantes, A.; Arroyo, F.; Quirantes-Ros, J. Multiple Light Scattering by Spherical Particle Systems and Its Dependence on Concentration: A T-Matrix Study. J. Colloid Interface Sci. 2001, 240, 78–82. [Google Scholar] [CrossRef]
- Hohmann, A.; Voit, F.; Schäfer, J.; Kienle, A. Multiple scattering of polarized light: Influence of absorption. Phys. Med. Biol. 2014, 59, 2583. [Google Scholar] [CrossRef] [PubMed]
- Mishchenko, M.I.; Goldstein, D.H.; Chowdhary, J.; Lompado, A. Radiative transfer theory verified by controlled laboratory experiments. Opt. Lett. 2013, 38, 3522–3525. [Google Scholar] [CrossRef] [PubMed]
- Mishchenko, M.I.; Liu, L.; Videen, G. Conditions of applicability of the single-scattering approximation. Opt. Express 2007, 15, 7522–7527. [Google Scholar] [CrossRef] [PubMed]
- Yalcin, R.A.; Lee, T.; Kashanchi, G.N.; Markkanen, J.; Martinez, R.; Tolbert, S.H.; Pilon, L. Dependent Scattering in Thick and Concentrated Colloidal Suspensions. ACS Photonics 2022, 9, 3318–3332. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Dlugach, J.M. Multiple scattering of polarized light by particles in an absorbing medium. Appl. Opt. 2019, 58, 4871–4877. [Google Scholar] [CrossRef]
- Ma, L.X.; Xie, B.W.; Wang, C.C.; Liu, L.H. Radiative transfer in dispersed media: Considering the effect of host medium absorption on particle scattering. J. Quant. Spectrosc. Radiat. Transf. 2019, 230, 24–35. [Google Scholar] [CrossRef]
- Ivanenko, Y.; Gustafsson, M.; Nordebo, S. Optical theorems and physical bounds on absorption in lossy media. Opt. Express 2019, 27, 34323–34342. [Google Scholar] [CrossRef]
- Mundy, W.C.; Roux, J.A.; Smith, A.M. Mie scattering by spheres in an absorbing medium. J. Opt. Soc. Am. 1974, 64, 1593–1597. [Google Scholar] [CrossRef]
- Chýlekt, P. Light scattering by small particles in an absorbing medium. J. Opt. Soc. Am. 1977, 67, 561–563. [Google Scholar] [CrossRef]
- Quinten, M.; Rostalski, J. Lorenz-Mie Theory for Spheres Immersed in an absorbing host medium. Part. Part. Syst. Charact. 1996, 13, 89–96. [Google Scholar] [CrossRef]
- Sudiarta, I.W.; Chylek, P. Mie-scattering formalism for spherical particles embedded in an absorbing medium. J. Opt. Soc. Am. A 2001, 18, 1275–1278. [Google Scholar] [CrossRef]
- Fu, Q.; Sun, W. Mie theory for light scattering by a spherical particle in an absorbing medium. Appl. Opt. 2001, 40, 1354–1361. [Google Scholar] [CrossRef] [PubMed]
- Mishchenko, M.I. Electromagnetic scattering by a fixed finite object embedded in an absorbing medium. Opt. Express 2007, 15, 13188–13202. [Google Scholar] [CrossRef] [PubMed]
- Mishchenko, M.I. Multiple scattering by particles embedded in an absorbing medium. 1. Foldy—Lax equations, order-of-scattering expansion, and coherent field. Opt. Express 2008, 16, 2288–2301. [Google Scholar] [CrossRef]
- Mishchenko, M.I. Multiple scattering by particles embedded in an absorbing medium. 2. Radiative transfer equation. J. Quant. Spectrosc. Radiat. Transf. 2008, 109, 2386–2390. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Videen, G.; Yang, P. Extinction by a homogeneous spherical particle in an absorbing medium. Opt. Lett. 2017, 42, 4873–4876. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Dlugach, J.M. Scattering and extinction by spherical particles immersed in an absorbing host medium. J. Quant. Spectrosc. Radiat. Transf. 2018, 211, 179–187. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Dlugach, J.M. Plasmon resonances of metal nanoparticles in an absorbing medium. OSA Contin. 2019, 2, 3415–3421. [Google Scholar] [CrossRef]
- Peck, R.L.; Brolo, A.G.; Gordon, R. Absorption leads to narrower plasmonic resonances. J. Opt. Soc. Am. B 2019, 36, F117–F122. [Google Scholar] [CrossRef]
- Zhai, J.; Ma, L.; Xu, W.; Liu, L. Effect of host medium absorption on the radiative properties of dispersed media consisting of optically soft particles. J. Quant. Spectrosc. Radiat. Transf. 2020, 254, 107206. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, W.; Liu, L. Scattering by a charged sphere embedded in an absorbing medium. J. Quant. Spectrosc. Radiat. Transf. 2020, 246, 106908. [Google Scholar] [CrossRef]
- Khlebtsov, N.G. Extinction, absorption, and scattering of light by plasmonic spheres embedded in an absorbing host medium. Phys. Chem. Chem. Phys. 2021, 23, 23141–23157. [Google Scholar] [CrossRef] [PubMed]
- Peck, R.; Khademi, A.; Ren, J.; Hughes, S.; Brolo, A.G.; Gordon, R. Plasmonic linewidth narrowing by encapsulation in a dispersive absorbing material. Phys. Rev. Res. 2021, 3, 013014. [Google Scholar] [CrossRef]
- Zhang, S.; Dong, J.; Zhang, W.; Luo, M.; Liu, L. Extinction by plasmonic nanoparticles in dispersive and dissipative media. Opt. Lett. 2022, 47, 5577–5580. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.; Zhang, W.; Dong, J.; Liu, L. Optical theorem of an infinite circular cylinder in weakly absorbing media. Phys. Rev. A 2022, 105, 023516. [Google Scholar] [CrossRef]
- Dong, J.; Zhang, W.; Liu, L. Discrete dipole approximation method for electromagnetic scattering by particles in an absorbing host medium. Opt. Express 2021, 29, 7690–7705. [Google Scholar] [CrossRef] [PubMed]
- Yurkin, M.A.; Moskalensky, A.E. Open-source implementation of the discrete-dipole approximation for a scatterer in an absorbing host medium. J. Phys. Conf. Ser. 2021, 2015, 012167. [Google Scholar] [CrossRef]
- Khlebtsov, N.G. Extinction and scattering of light by nonspherical plasmonic particles in absorbing media. J. Quant. Spectrosc. Radiat. Transf. 2022, 280, 108069. [Google Scholar] [CrossRef]
- Wang, C.C.; Ma, L.X. Effect of host medium absorption on polarized radiative transfer in dispersed media. Appl. Opt. 2019, 58, 7157–7164. [Google Scholar] [CrossRef]
- Loiko, N.A.; Miskevich, A.A.; Loiko, V.A. Optical characteristics of a monolayer of identical spherical particles in an absorbing host medium. J. Opt. Soc. Am. A 2023, 40, 682–691. [Google Scholar] [CrossRef]
- Hergert, W.; Wriedt, T. The Mie Theory: Basics and Applications; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Xu, Y. Electromagnetic scattering by an aggregate of spheres. Appl. Opt. 1995, 34, 4573–4588. [Google Scholar] [CrossRef]
- Mackowski, D.W.; Mishchenko, M.I. A multiple sphere T-matrix Fortran code for use on parallel computer clusters. J. Quant. Spectrosc. Radiat. Transf. 2011, 112, 2182–2192. [Google Scholar] [CrossRef]
- Markkanen, J.; Yuffa, A.J. Fast superposition T-matrix solution for clusters with arbitrarily-shaped constituent particles. J. Quant. Spectrosc. Radiat. Transf. 2017, 189, 181–188. [Google Scholar] [CrossRef]
- Egel, A.; Pattelli, L.; Mazzamuto, G.; Wiersma, D.S.; Lemmer, U. CELES: CUDA-accelerated simulation of electromagnetic scattering by large ensembles of spheres. J. Quant. Spectrosc. Radiat. Transf. 2017, 199, 103–110. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Yang, P. Far-field Lorenz–Mie scattering in an absorbing host medium: Theoretical formalism and FORTRAN program. J. Quant. Spectrosc. Radiat. Transf. 2018, 205, 241–252. [Google Scholar] [CrossRef]
- Mackowski, D.W. Calculation of total cross sections of multiple-sphere clusters. J. Opt. Soc. Am. A 1994, 11, 2851–2861. [Google Scholar] [CrossRef]
- Mackowski, D.W. Calculation of the Scattering Properties for a Cluster of Spheres. User Guide Accompanying the SCSMFO.FOR Code. 1999. Available online: ftp://ftp.eng.auburn.edu/pub/dmckwski/scatcodes/scsmfo.ps (accessed on 5 June 2023).
- Mackowski, D.W. Analysis of radiative scattering for multiple sphere configurations. Proc. Math. Phys. Eng. Sci. 1991, 433, 599–614. [Google Scholar]
- Tsang, L.; Kong, J.A.; Ding, K.H. Scattering of Electromagnetic Waves: Theories and Applications; John Wiley & Sons: New York, NY, USA, 2000. [Google Scholar]
- Arfken, G.B.; Weber, H.J.; Harris, F.E. Mathematical Methods for Physicists; Academic Press: Boston, MA, USA, 2013. [Google Scholar]
- Bohren, C.F.; Gilra, D.P. Extinction by a spherical particle in an absorbing medium. J. Colloid Interface Sci. 1979, 72, 215–221. [Google Scholar] [CrossRef]
- Palik, E.D. Handbook of Optical Constants of Solids; Academic Press: San Diego, CA, USA, 1998. [Google Scholar]
- David, M.; Disnan, D.; Lardschneider, A.; Wacht, D.; Hoang, H.T.; Ramer, G.; Detz, H.; Lendl, B.; Schmid, U.; Strasser, G.; et al. Structure and mid-infrared optical properties of spin-coated polyethylene films developed for integrated photonics applications. Opt. Mater. Express 2022, 12, 2168–2180. [Google Scholar] [CrossRef]
- Chen, M.; Pang, D.; Chen, X.; Yan, H. Investigating the effective radiative cooling performance of random dielectric microsphere coatings. Int. J. Heat Mass Transfer 2021, 173, 121263. [Google Scholar] [CrossRef]
- Baneshi, M.; Maruyama, S.; Komiya, A. Infrared Radiative Properties of Thin Polyethylene Coating Pigmented With Titanium Dioxide Particles. J. Heat Transfer 2009, 132, 023306. [Google Scholar] [CrossRef]
- Wang, G.-H.; Zhang, Y.; Zhang, D.-H.; Fan, J.-P. Design and calculation of low infrared transmittance and low emissivity coatings for heat radiative applications. Int. J. Miner. Metall. Mater. 2012, 19, 179–184. [Google Scholar] [CrossRef]



| 0.0 | 0.001 | 0.01 | 0.02 | |
|---|---|---|---|---|
| 0.2 μm | 0.00048855 | 0.00043460 | −0.000050653 | −0.00058887 |
| 1.0 μm | 0.29701 | 0.29619 | 0.28894 | 0.28123 |
| 2.0 μm | 2.4220 | 2.4242 | 2.4444 | 2.4675 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhai, J.; Zhang, S.; Liu, L. Extinction and Independent Scattering Criterion for Clusters of Spherical Particles Embedded in Absorbing Host Media. Photonics 2023, 10, 782. https://doi.org/10.3390/photonics10070782
Zhai J, Zhang S, Liu L. Extinction and Independent Scattering Criterion for Clusters of Spherical Particles Embedded in Absorbing Host Media. Photonics. 2023; 10(7):782. https://doi.org/10.3390/photonics10070782
Chicago/Turabian StyleZhai, Jinan, Shangyu Zhang, and Linhua Liu. 2023. "Extinction and Independent Scattering Criterion for Clusters of Spherical Particles Embedded in Absorbing Host Media" Photonics 10, no. 7: 782. https://doi.org/10.3390/photonics10070782
APA StyleZhai, J., Zhang, S., & Liu, L. (2023). Extinction and Independent Scattering Criterion for Clusters of Spherical Particles Embedded in Absorbing Host Media. Photonics, 10(7), 782. https://doi.org/10.3390/photonics10070782





