Optical Helicity of Light in the Tight Focus
Abstract
1. Introduction
2. Helicity at the Focus of a Linearly Polarized Optical Vortex
3. Helicity at the Focus of a Circularly Polarized Optical Vortex
4. Helicity at the Focus of a Cylindrical Vector Beam
5. Helicity at the Focus of a Field with Hybrid Circular-Azimuthal Polarization
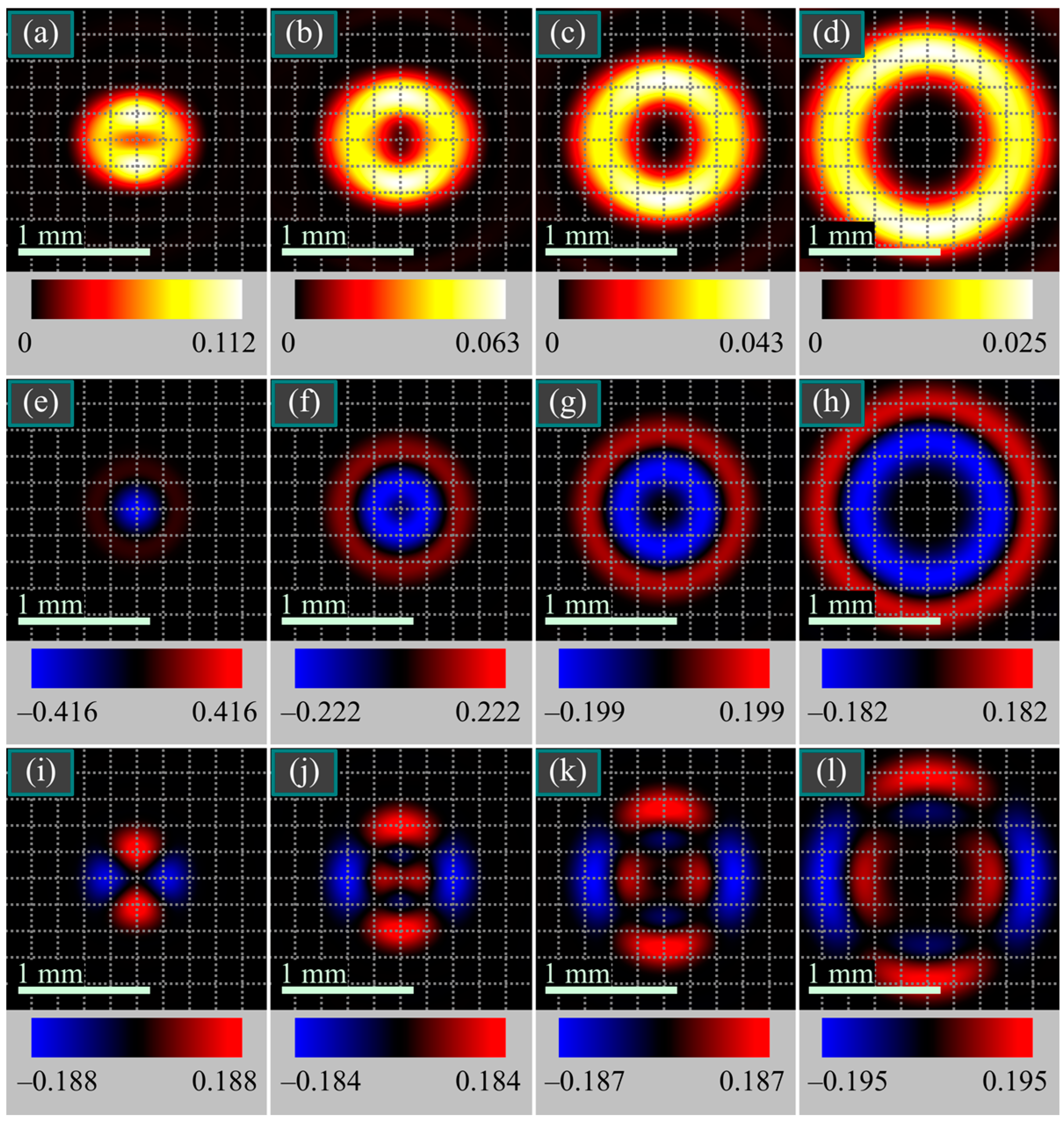
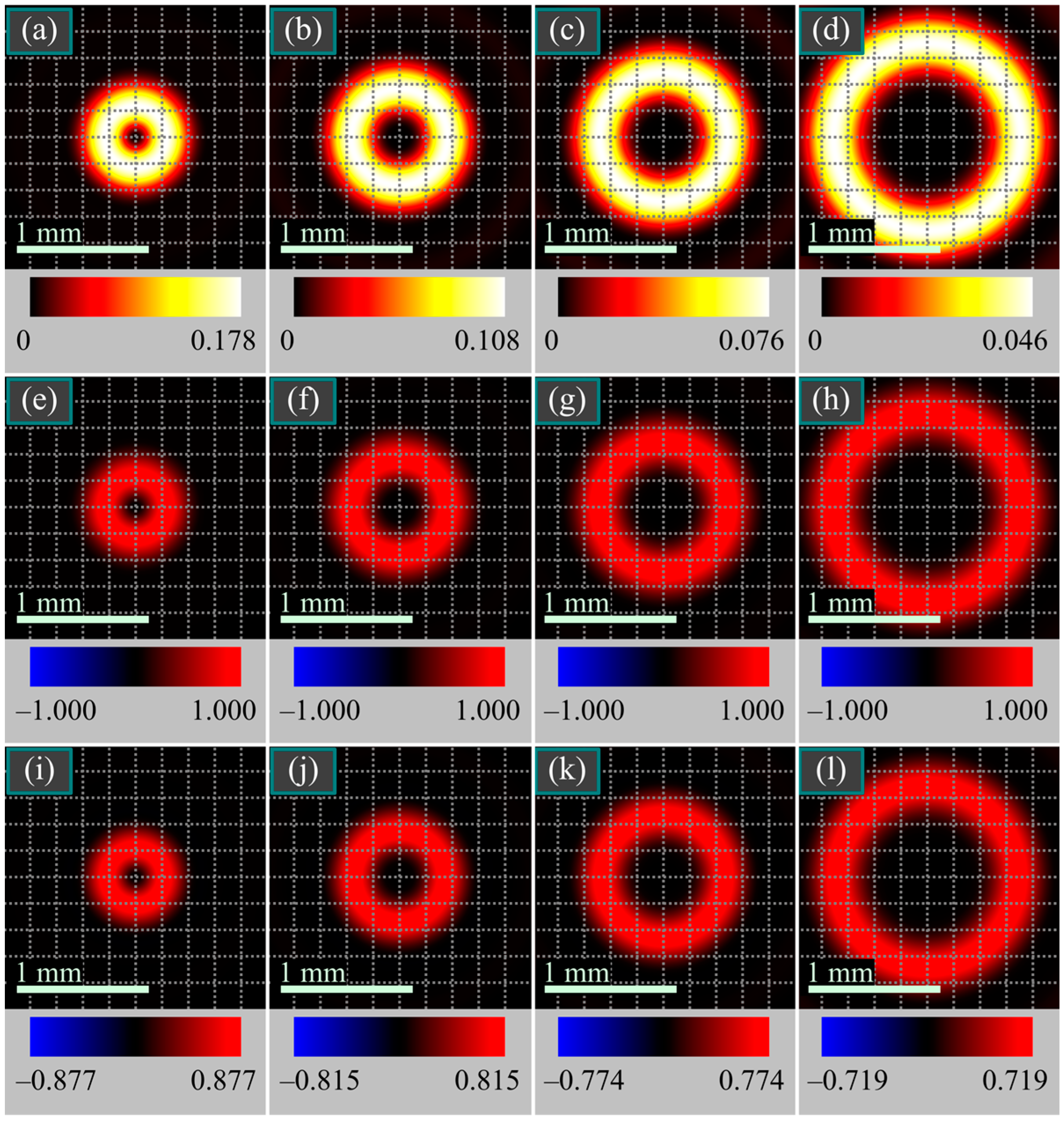
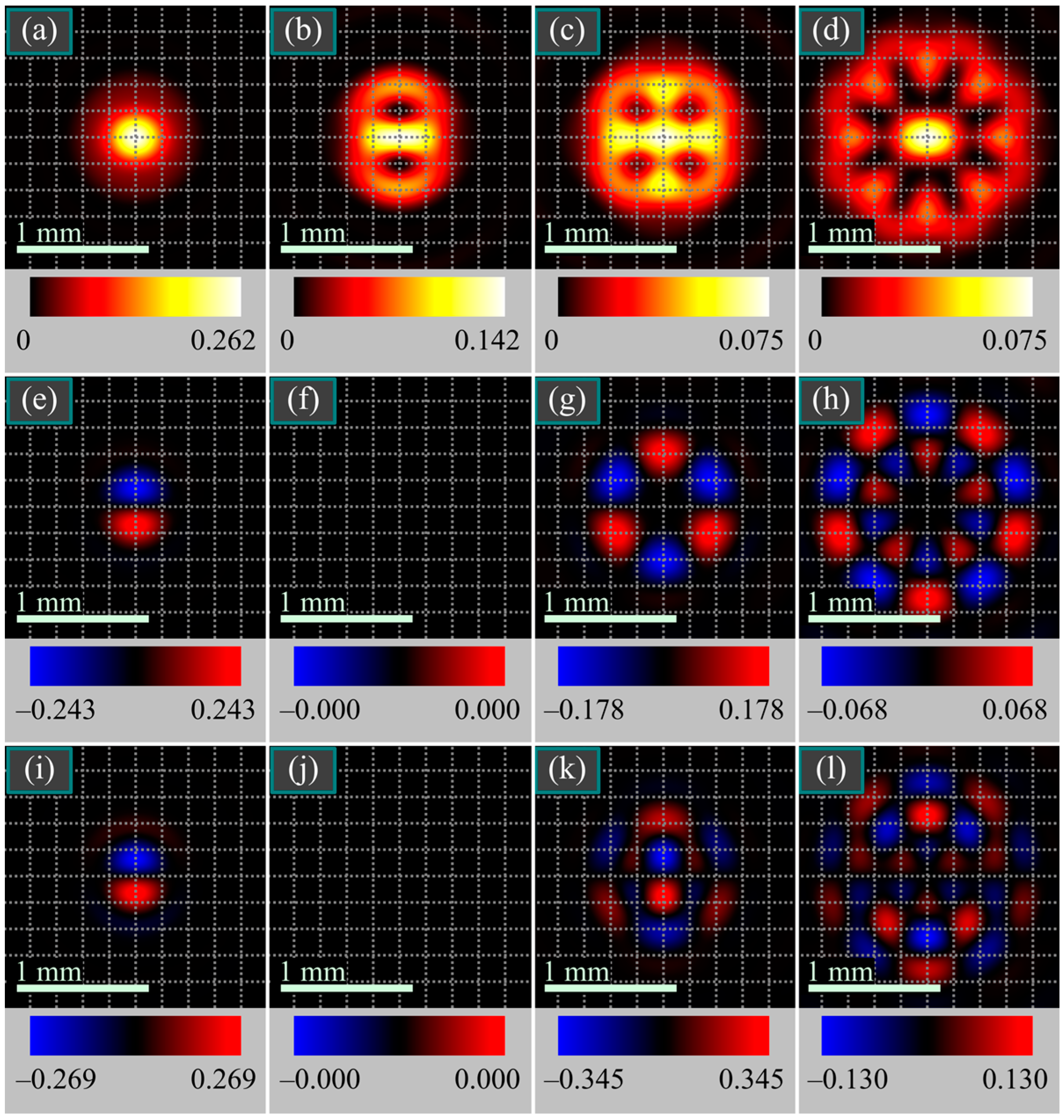
6. Numerical Simulation
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Afanasiev, G.N.; Stepanovsky, Y.P. The helicity of the free electromagnetic field and its physical meaning. Nuov. Cim. A 1996, 109, 271–279. [Google Scholar] [CrossRef]
- Trueba, J.L.; Racada, A.F. The electromagnetic helicity. Eur. J. Phys. 1996, 17, 141–144. [Google Scholar] [CrossRef]
- Cameron, R.P.; Barnett, S.M.; Yao, A.M. Optical helicity, optical spin and related quantities in electromagnetic theory. New J. Phys. 2012, 14, 053050. [Google Scholar] [CrossRef]
- Woltjer, L. A theorem on force-free magnetic fields. Proc. Natl Acad. Sci. USA 1958, 44, 489–491. [Google Scholar] [CrossRef]
- Crimin, F.; Mackinnon, N.; Gцtte, J.B.; Barnett, S.M. Optical helicity and chirality: Conservation and sources. Appl. Sci. 2019, 9, 828. [Google Scholar] [CrossRef]
- Lipkin, D.M. Existence of a new conservation law in electromagnetic theory. J. Math. Phys. 1964, 5, 696–700. [Google Scholar] [CrossRef]
- Mackinnon, N. On the differences between helicity and chirality. J. Opt. 2019, 21, 125402. [Google Scholar] [CrossRef]
- Aiello, A. Helicity, chirality, and spin of optical fields without vector potentials. Phys. Rev. A 2022, 106, 043519. [Google Scholar] [CrossRef]
- Abujetas, D.R.; Sбnchez-Gil, J.A. Spin angular momentum of guided light induced by transverse confinement and intrinsic helicity. ACS Photonics 2020, 7, 534–545. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Bekshaev, A.Y.; Nori, F. Extraordinary momentum and spin in evanescent waves. Nat. Commun. 2014, 5, 3300. [Google Scholar] [CrossRef] [PubMed]
- Richards, B.; Wolf, E. Electromagnetic diffraction in optical systems, II. Structure of the image field in an aplanatic system. Proc. R. Soc. Lond A 1959, 253, 358–379. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Kovalev, A.A.; Kozlova, E.S.; Telegin, A.M. Hall Effect at the Focus of an Optical Vortex with Linear Polarization. Micromachines 2023, 14, 788. [Google Scholar] [CrossRef]
- Kovalev, A.A.; Kotlyar, V.V. Spin Hall Effect of Double-Index Cylindrical Vector Beams in a Tight Focus. Micromachines 2023, 14, 494. [Google Scholar] [CrossRef] [PubMed]
- Kotlyar, V.V.; Nalimov, A.G.; Stafeev, S.S. Exploiting the circular polarization of light to obtain a spiral energy flow at the subwavelength focus. J. Opt. Soc. Am. B 2019, 36, 2850–2855. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Stafeev, S.S.; Kovalev, A.A.; Zaitsev, V.D. Spin Hall Effect before and after the Focus of a High-Order Cylindrical Vector Beam. Appl. Sci. 2022, 12, 12218. [Google Scholar] [CrossRef]
- Kotlyar, V.; Stafeev, S.; Zaitsev, V.; Kozlova, E. Spin-Orbital Conversion with the Tight Focus of an Axial Superposition of a High-Order Cylindrical Vector Beam and a Beam with Linear Polarization. Micromachines 2022, 13, 1112. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Stafeev, S.S.; Zaitsev, V.D.; Kovalev, A.A. Multiple optical spin-orbit Hall effect at the tight focus. Phys. Lett. A 2023, 458, 128596. [Google Scholar] [CrossRef]
- Berry, H.G.; Gabrielse, G.; Livingston, A.E. Measurement of the Stokes parameters of light. Appl. Opt. 1977, 16, 3200–3205. [Google Scholar] [CrossRef]
- D’yachkov, N.V.; Bogatov, A.P. Measuring the Stokes parameters of radiation of semiconductor lasers. Quantum Electron. 2011, 41, 869–874. [Google Scholar] [CrossRef]
- Forbes, K.A.; Jones, G.A. Measures of helicity and chirality of optical vortex beams. J. Opt. 2021, 23, 115401. [Google Scholar] [CrossRef]
- Koksal, K.; Babiker, M.; Lembessis, V.E.; Yuan, J. Chirality and helicity of linearly-polarised Laguerre-Gaussian beams of small beam waists. Opt. Commun. 2021, 490, 126907. [Google Scholar] [CrossRef]
- Litvin, I.A. The behavior of the instantaneous Poynting vector of symmetrical laser beams. J. Opt. Soc. Am. A 2012, 29, 901–907. [Google Scholar] [CrossRef] [PubMed]
- Leach, J.; Keen, S.; Padgett, M.J.; Saunter, C.; Love, G.D. Direct measurement of the skew angle of the Poynting vector in a helically phased beam. Opt. Express 2006, 14, 11919–11924. [Google Scholar] [CrossRef] [PubMed]
- Garoli, D.; Zilio, P.; De Angelis, F.; Gorodetski, Y. Helicity locking of chiral light emitted from a plasmonic nanotaper. Nanoscale 2017, 9, 6965–6969. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kovalev, A.A.; Kotlyar, V.V.; Telegin, A.M. Optical Helicity of Light in the Tight Focus. Photonics 2023, 10, 719. https://doi.org/10.3390/photonics10070719
Kovalev AA, Kotlyar VV, Telegin AM. Optical Helicity of Light in the Tight Focus. Photonics. 2023; 10(7):719. https://doi.org/10.3390/photonics10070719
Chicago/Turabian StyleKovalev, Alexey A., Victor V. Kotlyar, and Alexey M. Telegin. 2023. "Optical Helicity of Light in the Tight Focus" Photonics 10, no. 7: 719. https://doi.org/10.3390/photonics10070719
APA StyleKovalev, A. A., Kotlyar, V. V., & Telegin, A. M. (2023). Optical Helicity of Light in the Tight Focus. Photonics, 10(7), 719. https://doi.org/10.3390/photonics10070719




