High-Efficiency Second-Harmonic Generation Using Quasi-Bound State in LiNbO3 Metasurface
Abstract
1. Introduction
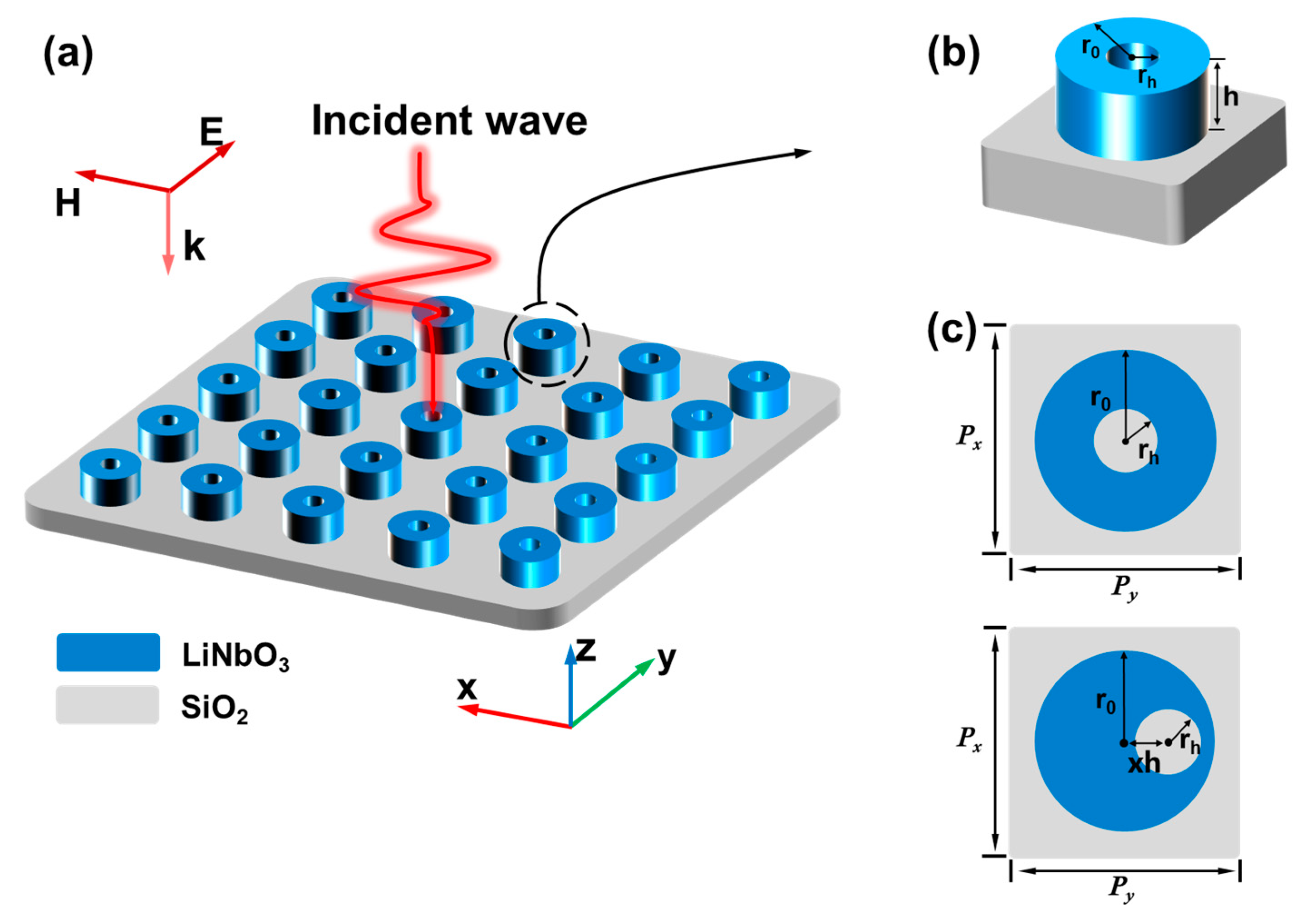
2. Materials and Methods
3. Results and Discussions
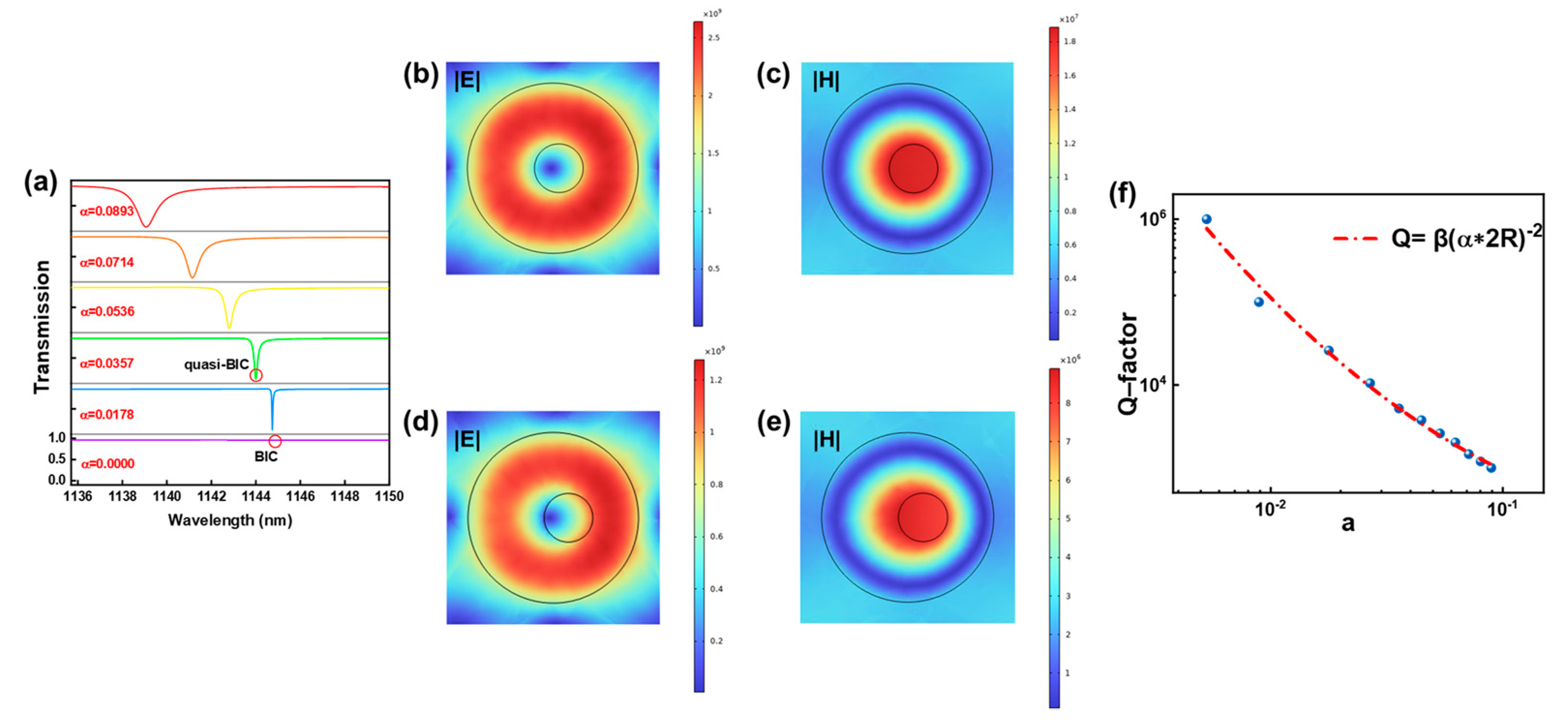
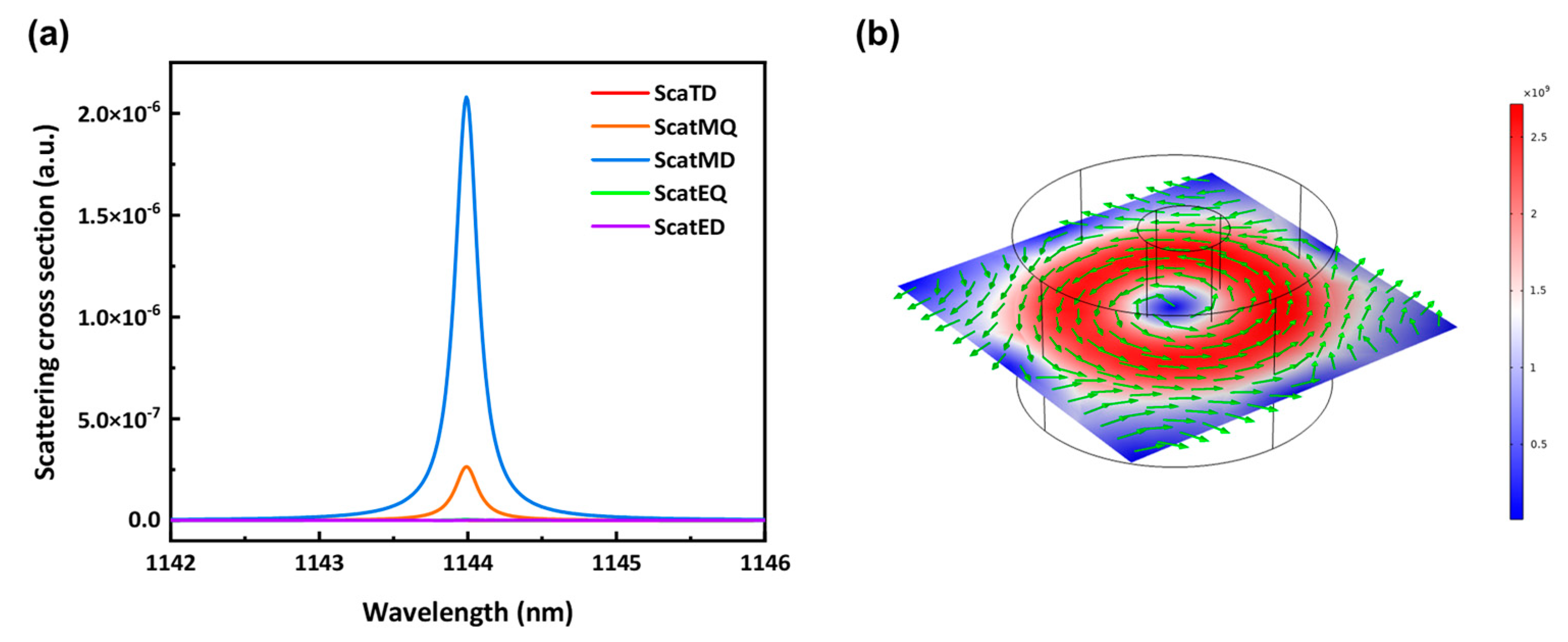
3.1. Characterization of Quasi-BIC by Changing α
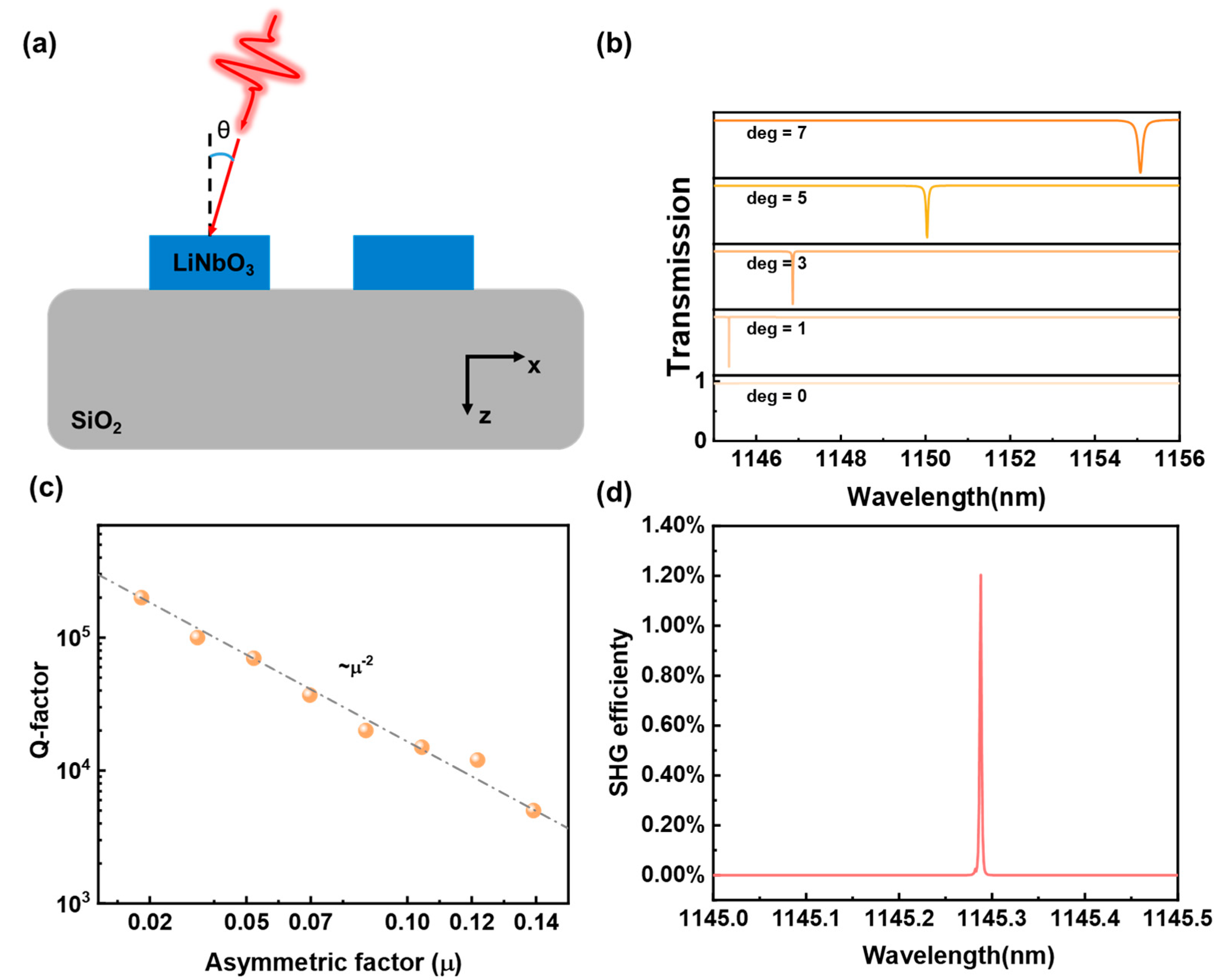
3.2. Second-Harmonic Generation
3.3. Characterization of Oblique Incidence
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kim, S.; Jin, J.; Kim, Y.J. High-harmonic generation by resonant plasmon field enhancement. Nature 2008, 453, 757–760. [Google Scholar] [CrossRef] [PubMed]
- Staedler, D.; Magouroux, T.; Hadji, R.; Joulaud, C.; Extermann, J.; Schwung, S.; Passemard, S.; Kasparian, C.; Clarke, G.; Gerrmann, M.; et al. Harmonic nanocrystals for biolabeling: A survey of optical properties and biocompatibility. ACS Nano 2012, 6, 2542–2549. [Google Scholar] [CrossRef] [PubMed]
- Timpu, F.; Hendricks, N.R.; Petrov, M.I.; Ni, S.; Renaut, C.; Wolf, H. Enhanced second-harmonic generation from sequential capillarity-assisted particle assembly of hybrid nanodimers. Nano Lett. 2017, 17, 5381–5388. [Google Scholar] [CrossRef] [PubMed]
- Cao, Q.T.; Liu, R.; Wang, H. Reconfigurable symmetry-broken laser in a symmetric microcavity. Nat. Commun. 2020, 11, 1136. [Google Scholar] [CrossRef] [PubMed]
- Jinhui, C.; Xiaoqin, S.; Shuijing, T.; Qitao, C.; Qihuang, G.; Yunfeng, X. Microcavity nonlinear optics with an organically functionalized surface. Phys. Rev. Lett. 2019, 123, 173902. [Google Scholar]
- Zhang, X.; Cao, Q.T.; Wang, Z. Symmetry-breaking-induced nonlinear optics at a microcavity surface. Nat. Photonics 2019, 13, 21–24. [Google Scholar] [CrossRef]
- Franken, P.A.; Hill, A.E.; Peters, C.W.; Weinreich, G. Generation of Second Harmonic. Phys. Rev. Lett. 1961, 7, 118. [Google Scholar] [CrossRef]
- Rouède, D.; Bellanger, J.J.; Recher, G.; Tiaho, F. Study of the effect of myofibrillar misalignment on the sarcomeric SHG intensity pattern. Opt. Express 2013, 21, 11404–11414. [Google Scholar] [CrossRef]
- Jiaqing, L.; ChangDong, C.; Xiao, L.; Jingwen, L.; Daxing, D.; Youwen, L.; Yangyang, F. Tunable dual quasi-bound states in continuum and electromagnetically induced transparency enabled by the broken material symmetry in all-dielectric compound gratings. Opt. Express. 2023, 31, 4347–4356. [Google Scholar]
- Alexander, K.; Mykhailo, T.; Andrea, A. Nonlinear metasurfaces: A paradigm shift in nonlinear optics. Mater. Today 2018, 21, 8–21. [Google Scholar]
- Giannini, V.; Fernandez-Dominguez, A.I.; Heck, S.C.; Maier, S.A. Plasmonic nanoantennas: Fundamentals and their use in controlling the radiative properties of nano emitters. Chem. Rev. 2011, 111, 3888–3912. [Google Scholar] [CrossRef]
- Thyagarajan, K.; Butet, J.; Martin, O.J. Augmenting second harmonic generation using Fano resonances in plasmonic systems. Nano Lett. 2013, 13, 1847–1851. [Google Scholar] [CrossRef]
- Grinblat, G.; Rahmani, M.; Cortes, E.; Caldarola, M.; Comedi, D.; Maier, S.A.; Bragas, A.V. High-efficiency second harmonic generation from a single hybrid ZnO nanowire/Au plasmonic nano oligomer. Nano Lett. 2014, 14, 6660–6665. [Google Scholar] [CrossRef]
- Liu, S.; Vabishchevich, P.P.; Vaskin, A.; Reno, J.L.; Keeler, G.A.; Sinclair, M.B.; Staude, I.; Brener, I. An all-dielectric metasurface as a broadband optical frequency mixer. Nat. Commun. 2018, 9, 2507. [Google Scholar] [CrossRef]
- Shcherbakov, M.R.; Neshev, D.N.; Hopkins, B.; Shorokhov, A.S.; Staude, I.; Melik-Gaykazyan, E.V.; Decker, M.; Ezhov, A.A.; Miroshnichenko, A.E.; Brener, I.; et al. Enhanced third-harmonic generation in silicon nanoparticles driven by magnetic response. Nano Lett. 2014, 14, 6488–6492. [Google Scholar] [CrossRef]
- Autere, A.; Jussila, H.; Dai, Y.; Wang, Y.; Lipsanen, H.; Sun, Z. Nonlinear optics with 2D layered materials. Adv. Mater. 2018, 30, 1705963. [Google Scholar] [CrossRef]
- Qingchen, Y.; Liang, F.; Hanlin, F.; Juntao, L.; Tao, W.; Wanqi, J.; Jianlin, Z.; Xuetao, G. Second harmonic and sum-frequency generations from a silicon metasurface integrated with a two-dimensional material. ACS Photonics 2019, 6, 2252–2259. [Google Scholar]
- Bernhardt, N.; Koshelev, K.; White, S.J.U.; Meng, K.W.C.; Fröch, J.E.; Kim, S.; Tran, T.T.; Choi, D.Y.; Kivshar, Y.; Solntsev, A.S. Quasi-BIC Resonant Enhancement of Second-Harmonic Generation in WS 2 Monolayers. Nano Lett. 2020, 2, 5309–5314. [Google Scholar] [CrossRef]
- He, X.; Lin, F.; Liu, F.; Shi, W. 3D Dirac semimetals supported tunable terahertz BIC metamaterials. Nanophotonics 2022, 11, 4705–4714. [Google Scholar] [CrossRef]
- Han, S.; Pitchappa, P.; Wang, W.; Srivastava, Y.K.; Singh, R. Extended bound states in the continuum with symmetry roken terahertz dielectric metasurfaces. Adv. Opt. Mater. 2021, 9, 2002001. [Google Scholar] [CrossRef]
- Lei, K.; Huaguang, B.; Werner, D.H. Efficient second-harmonic generation in high Q-factor asymmetric lithium niobate metasurfaces. Opt. Lett. 2021, 46, 633–636. [Google Scholar]
- Luca, C.; Cheng, L.; Sautter, J.; Staude, I.; Angelis, C.D.; Tao, L.; Neshev, D.N. Second harmonic generation in monolithic lithium niobate metasurfaces. Opt. Express 2019, 27, 33391–33398. [Google Scholar]
- Zheng, Z.; Xu, L.; Huang, L.; Smirnova, D.; Hong, P.; Ying, C.; Rahmani, M. Boosting second-harmonic generation in the LiNbO3 metasurface using high-Q guided resonances and bound states in the continuum. Phys. Rev. B 2022, 106, 125411. [Google Scholar] [CrossRef]
- Zelmon, D.E.; Small, D.L.; Jundt, D. Infrared corrected Sellmeier coefficients for congruently grown lithium niobate and 5 mol. % magnesium oxide–doped lithium niobate. J. Opt. Soc. Am. B 1997, 14, 3319–3322. [Google Scholar] [CrossRef]
- Li, J.; Shao, J.; Wang, Y.H.; Zhu, M.J.; Li, J.Q.; Dong, Z.G. Toroidal dipolar response by a dielectric microtube metamaterial in the terahertz regime. Opt. Express 2015, 23, 29138–29144. [Google Scholar] [CrossRef]
- Li, S.; Zhou, C.; Liu, T.; Xiao, S. Symmetry-protected bound states in the continuum supported by all-dielectric metasurfaces. Phys. Rev. A 2019, 100, 63803. [Google Scholar] [CrossRef]
- Sadrieva, Z.; Frizyuk, K.; Petrov, M.; Kivshar, Y.; Bogdanov, A. Multipolar origin of bound states in the continuum. Phys. Rev. B 2019, 100, 115303. [Google Scholar] [CrossRef]
- Frizyuk, K. Second-harmonic generation in dielectric nanoparticles with different symmetries. J. Opt. Soc. Am. B 2019, 36, F32–F37. [Google Scholar] [CrossRef]
- Joannopoulos, J.D.; Soljacic, M. Observation and differentiation of unique high-q optical resonances near zero wave vector in macroscopic photonic crystal slabs. Phys. Rev. Lett. 2012, 109, 67401. [Google Scholar]
- Sang, T.; Dereshgi, S.A.; Hadibrata, W.; Tanriover, I.; Aydin, K. Highly Efficient Light Absorption of Monolayer Graphene by Quasi-Bound State in the Continuum. Nanomaterials 2021, 11, 484. [Google Scholar] [CrossRef]
- Xu, T.; Wheeler, M.S.; Nair, S.V.; Ruda, H.E.; Mojahedi, M.; Aitchison, J.S. Highly confined mode above the light line in a two-dimensional photonic crystal slab. Appl. Phys. Lett. 2008, 93, 241105. [Google Scholar] [CrossRef]
- Caldarola, M.; Albella, P.; Cortes, E.; Rahmani, M.; Roschuk, T.; Grinblat, G.; Oulton, R.F.; Bragas, A.V.; Maier, S.A. Nonplasmonic nanoantennas for surface enhanced spectroscopies with ultra-low heat conversion. Nat. Commun. 2015, 6, 7915. [Google Scholar] [CrossRef]
- Kuznetsov, A.I.; Miroshnichenko, A.E.; Brongersma, M.L.; Kivshar, Y.S.; Luk’yanchuk, B. Optically resonant dielectricnanostructures. Science 2016, 354, 846. [Google Scholar] [CrossRef]
- Fan, K.; Shadrivov, I.V.; Padilla, W.J. Dynamic boundstates in the continuum. Optica 2019, 6, 446–454. [Google Scholar] [CrossRef]
- Anthur, A.P.; Zhang, H.; Paniagua-Dominguez, R.; Kalashnikov, D.; Ha, S.T.; Mass, T.W.W.; Kuznetsov, A.I.; Krivitsky, L. Continuous Wave Second Harmonic Generation Enabled by Quasi-Bound-States in the Continuum on Gallium Phosphide Metasurfaces. Nano Lett. 2020, 20, 8745–8751. [Google Scholar] [CrossRef]
- Cong, L.; Singh, R. Symmetry-protected dual bound states in the continuum in metamaterials. Adv. Opt. Mater. 2019, 7, 1900383. [Google Scholar] [CrossRef]
- He, Y.; Guo, G.; Feng, T. Toroidal dipole bound states in thecontinuum. Phys. Rev. B 2018, 98, 161112. [Google Scholar] [CrossRef]
- Carletti, L.; Rocco, D.; Locatelli, A.; De Angelis, C.; Gili, V.F.; Ravaro, M.; Favero, I.; Leo, G.; Finazzi, M.; Ghirardini, L.; et al. Controlling second-harmonic generation at the nanoscale with monolithic AlGaAs-on-AlOx antennas. Nanotechnology 2017, 28, 114005. [Google Scholar] [CrossRef]
- Hui, J.; Yangjian, C.; Zhanghua, H. Strong second-harmonic generation in dielectric optical nanoantennas resulting from the hybridization of magnetic dipoles and lattice resonances. J. Opt. Soc. Am. B 2020, 37, 3146–3151. [Google Scholar]
- Kwang-Hyon, K.; Il-Pong, K. Efficient near UV-vacuum UV sources based on second-harmonic generation enhanced by high-Q quasi-BICs in all-dielectric metasurfaces of low-index materials. Photonics Nanostruct.—Fundam. Appl. 2022, 51, 101053. [Google Scholar]
- Valencia-Caicedo, C.J.; Chaikina, E.I.; Khomenko, A.V. Second harmonic generation from GaAs-Au subwavelength relief gratings. Results Phys. 2022, 37, 105516. [Google Scholar] [CrossRef]
- Shun, W.; Chunhua, Q.; Keda, W.; Yicheng, L.; Chunying, G.; Bo, L.; Wenjia, L.; Jinhui, S. Ultra-high quality perfect absorber based on quasi bound states in the continuum. J. Appl. Phys. 2022, 131, 213104. [Google Scholar]
- Samadi, M.; Abshari, F.; Algorri, J.F.; Roldán-Varona, P.; Rodríguez-Cobo, L.; López-Higuera, J.M.; Sánchez-Pena, J.M.; Zografopoulos, D.C.; Dell’Olio, F. All-Dielectric Metasurface Based on Complementary Split-Ring Resonators for Refractive Index Sensing. Photonics 2022, 9, 130. [Google Scholar] [CrossRef]
- Feng, W.; Meibao, Q.; Shuyun, X. Quasi-bound state in the continuum supported by a compound grating waveguide structure for high-figure-of-merit refractive-index sensing. J. Appl. Phys. 2022, 132, 193101. [Google Scholar]



| Meta-Atoms | FH Wavelength (nm) | Material | Input Power | Conversion Efficiency |
|---|---|---|---|---|
| Cylinder [38] | 1200–1700 | AlGaAs | 1 GW/cm2 | 4 × 10−5 |
| Cylinder [39] | 1100–1800 | AlGaAs | 0.053 GW/cm2 | 4 × 10−4 |
| Cylinder plus slab [40] | 766–786 | LiNbO3 | 0.65 GW/cm2 | 1.4 × 10−3 |
| Grating [41] | 1280–2080 | Au | 1 GW/cm2 | 1.2 × 10−5 |
| This work | 1100–1200 | LiNbO3 | 1 MW/cm2 | 6.5×10−2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Hong, W.; Sui, X.; Hu, X. High-Efficiency Second-Harmonic Generation Using Quasi-Bound State in LiNbO3 Metasurface. Photonics 2023, 10, 661. https://doi.org/10.3390/photonics10060661
Liu S, Hong W, Sui X, Hu X. High-Efficiency Second-Harmonic Generation Using Quasi-Bound State in LiNbO3 Metasurface. Photonics. 2023; 10(6):661. https://doi.org/10.3390/photonics10060661
Chicago/Turabian StyleLiu, Siyu, Wei Hong, Xiubao Sui, and Xin Hu. 2023. "High-Efficiency Second-Harmonic Generation Using Quasi-Bound State in LiNbO3 Metasurface" Photonics 10, no. 6: 661. https://doi.org/10.3390/photonics10060661
APA StyleLiu, S., Hong, W., Sui, X., & Hu, X. (2023). High-Efficiency Second-Harmonic Generation Using Quasi-Bound State in LiNbO3 Metasurface. Photonics, 10(6), 661. https://doi.org/10.3390/photonics10060661




