An Axial Force Sensor Based on a Long-Period Fiber Grating with Dual-Peak Resonance
Abstract
1. Introduction
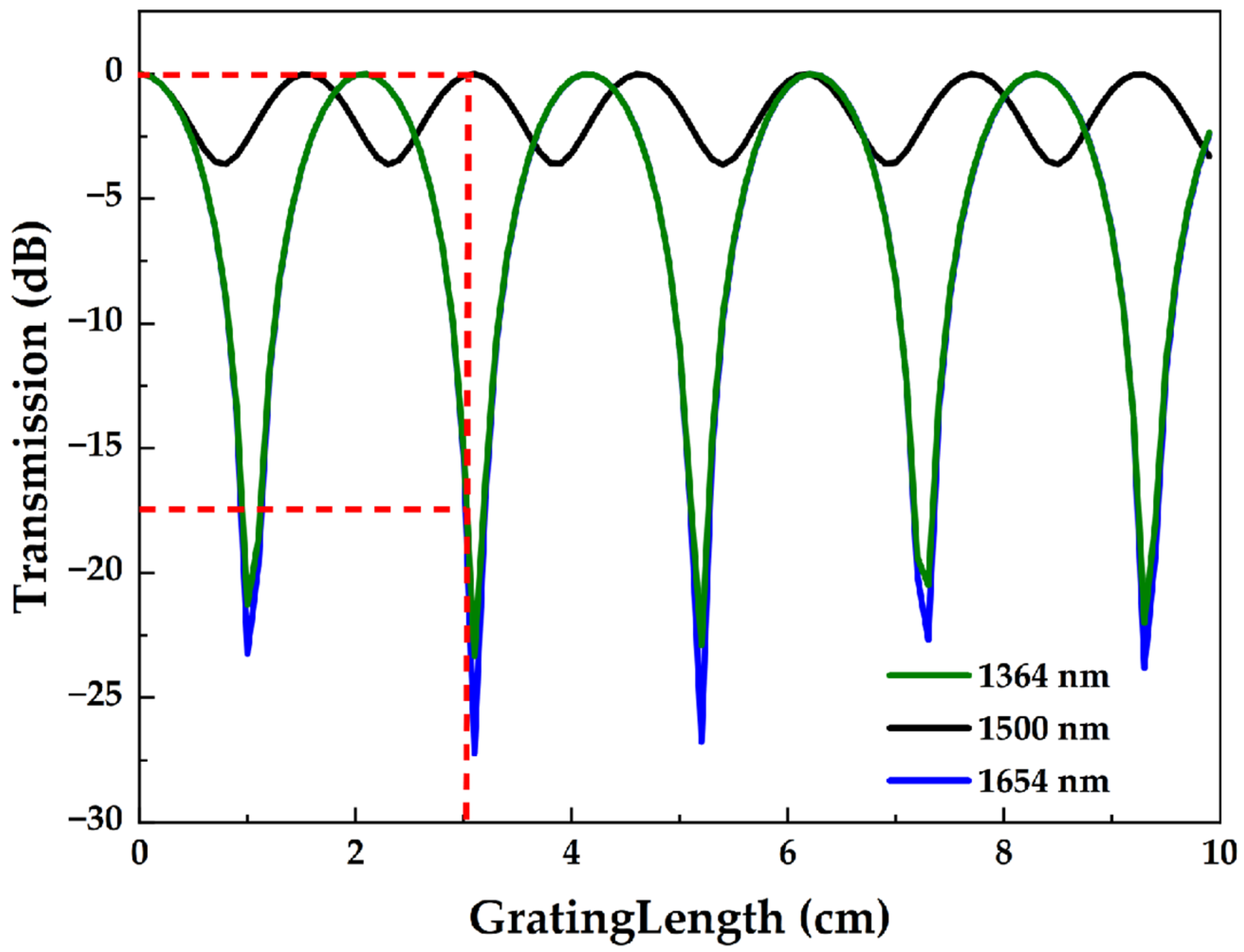
2. Working Principle
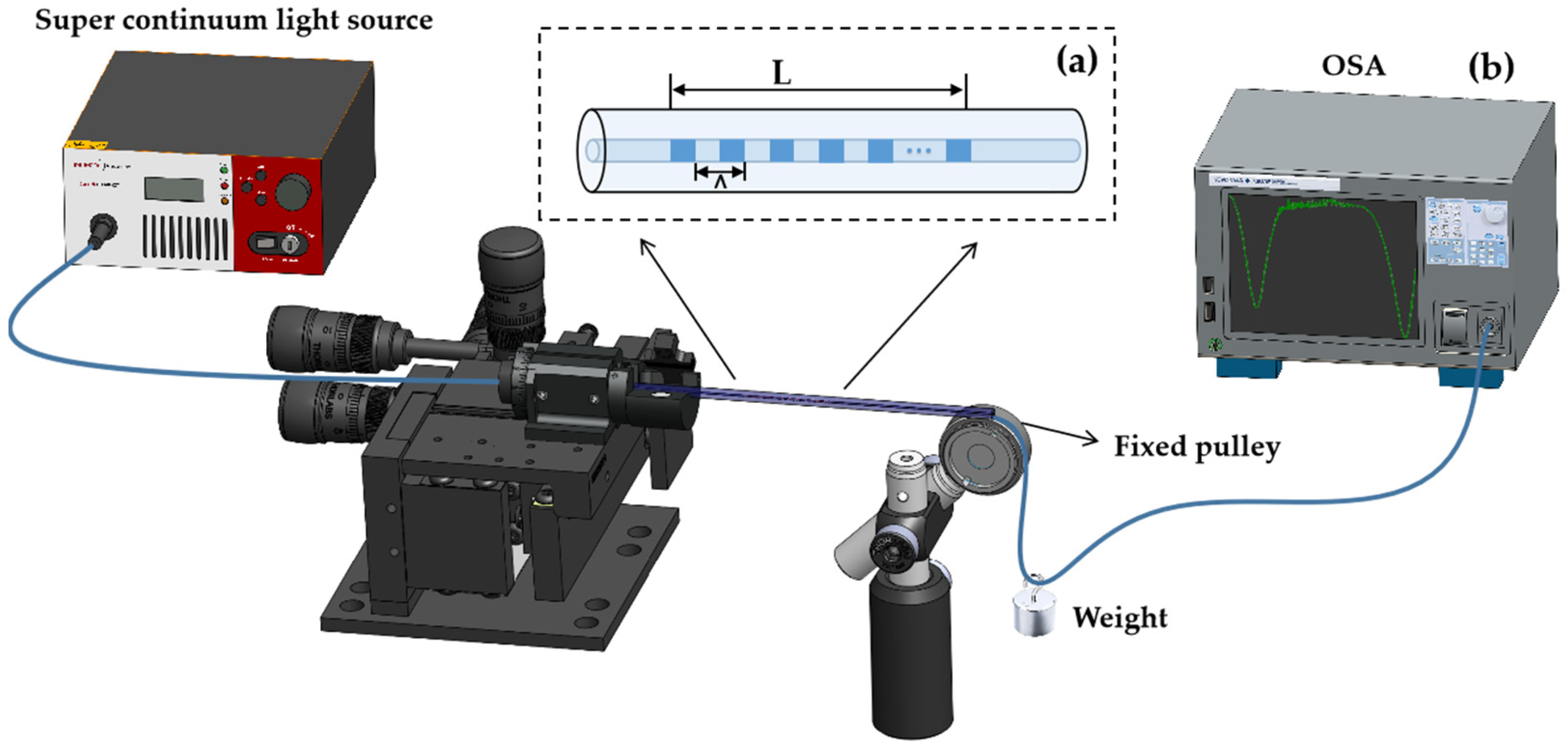
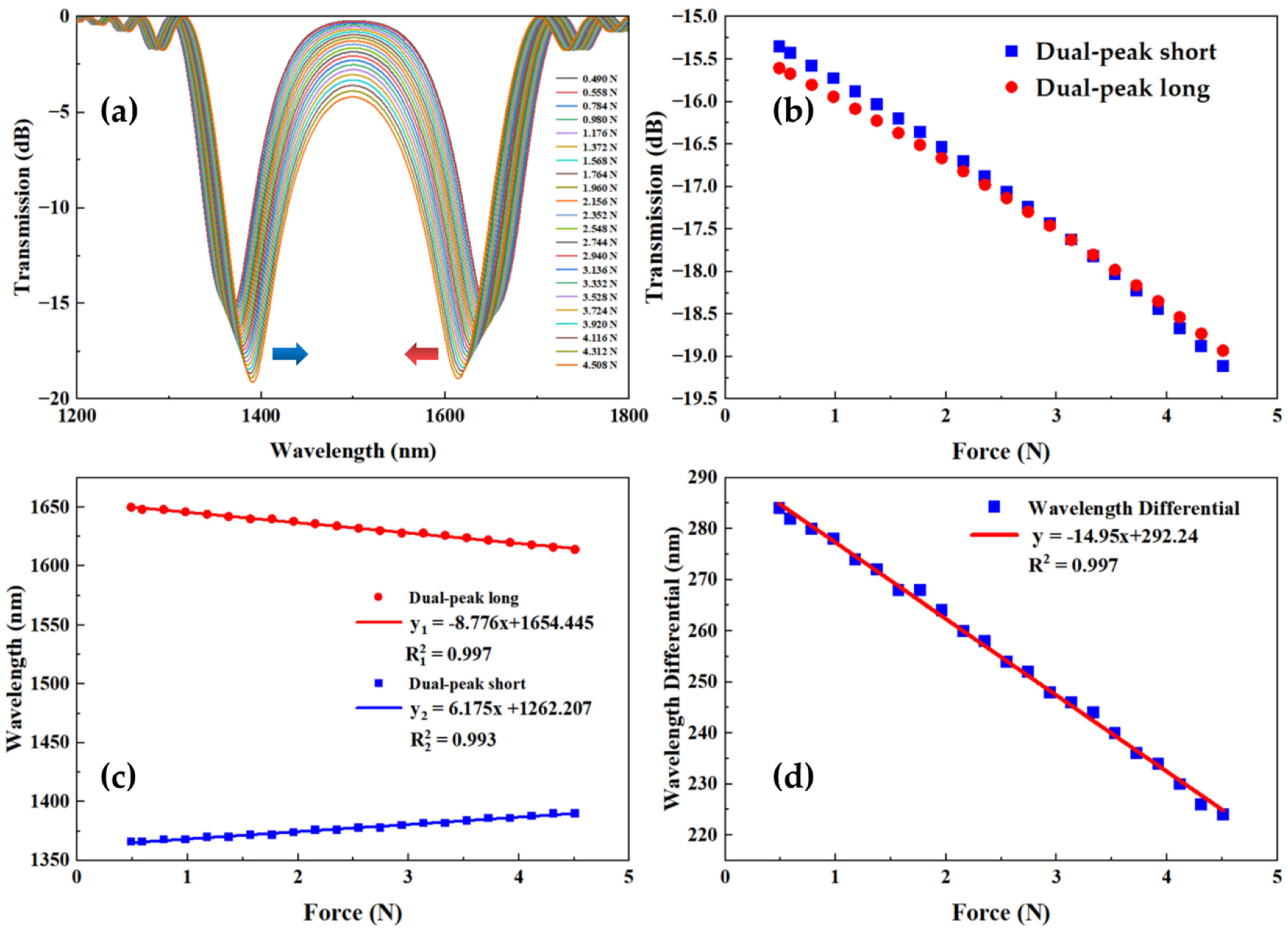
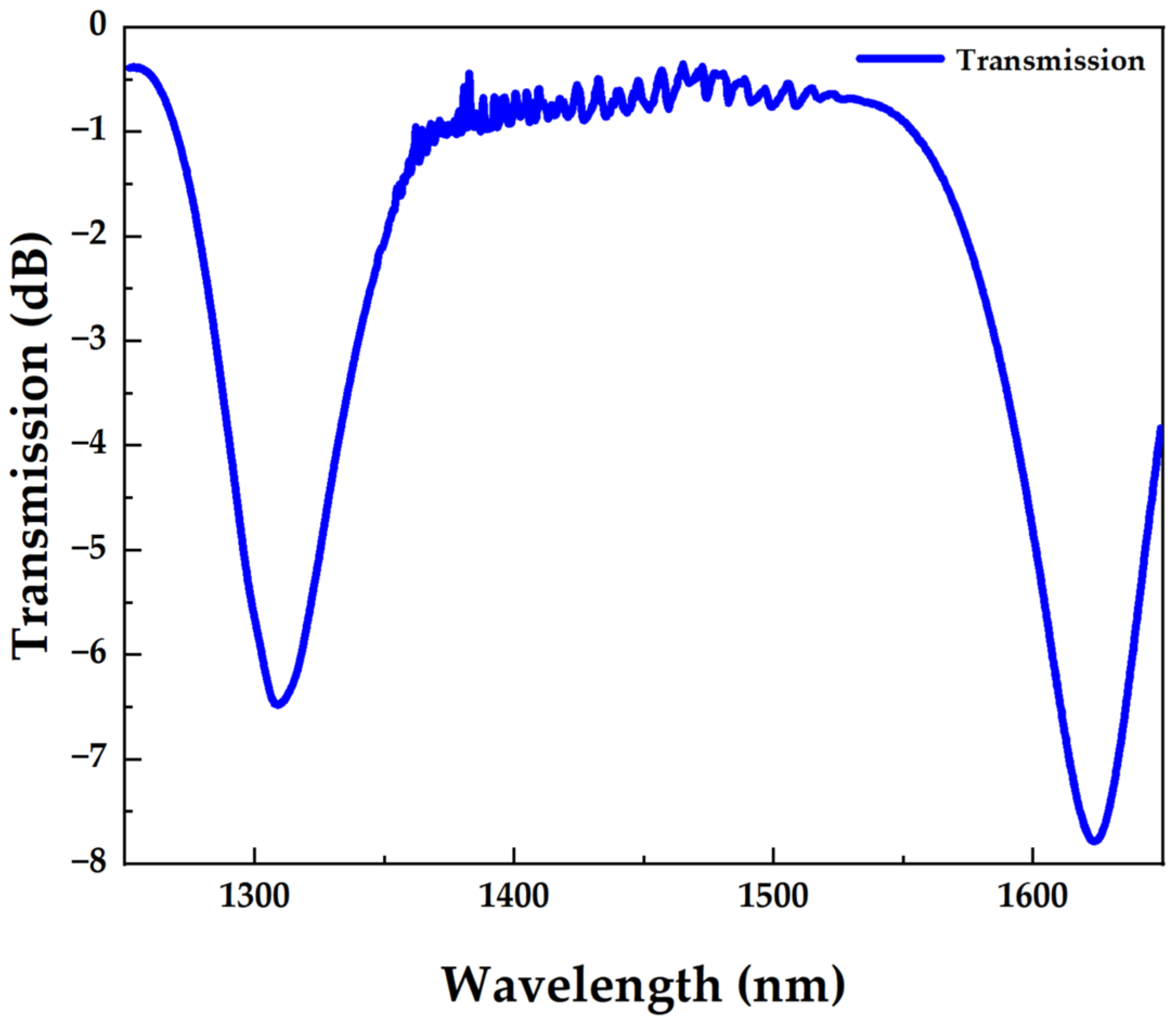
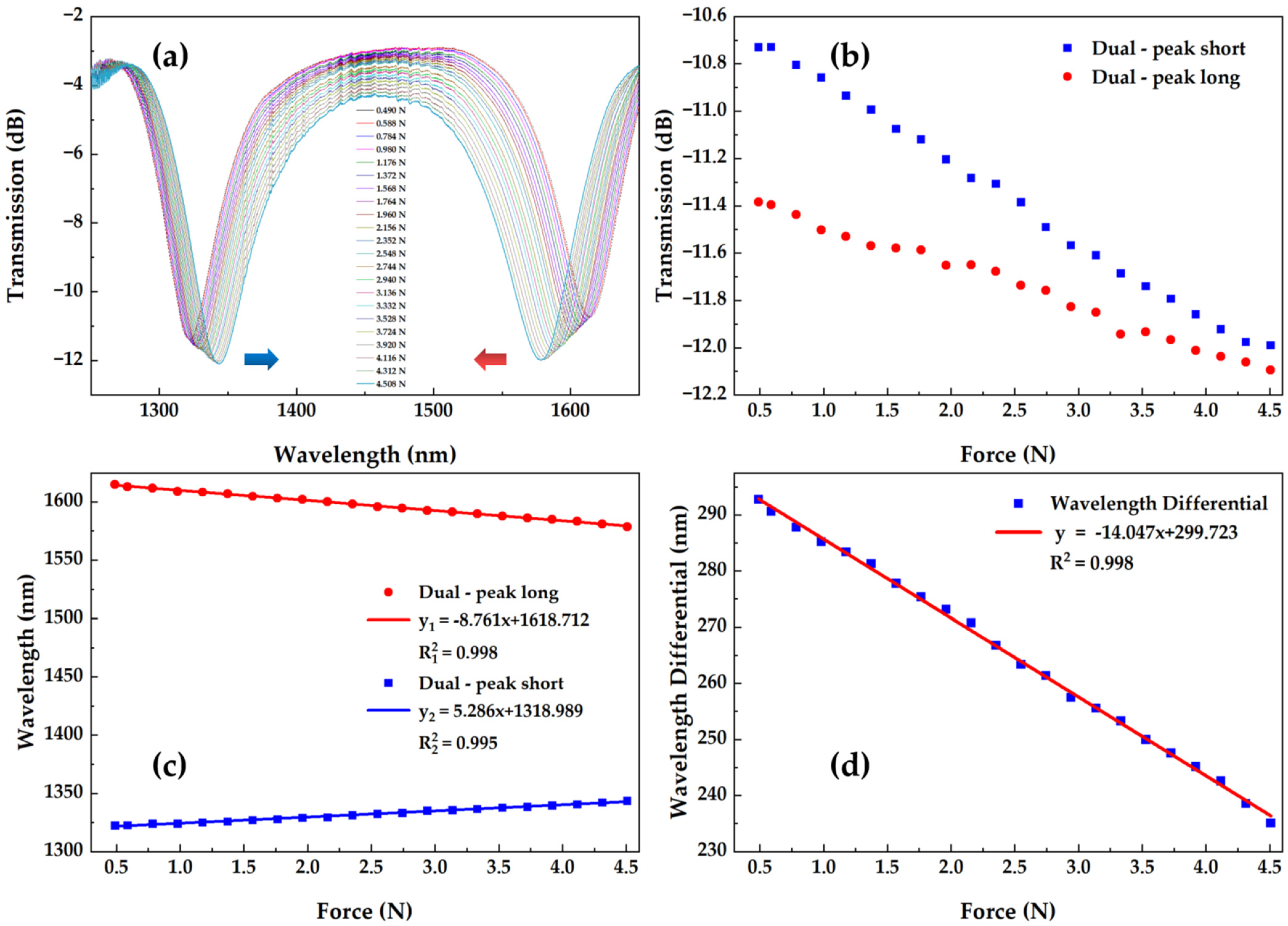
3. Experiments and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zou, M.; Liao, C.; Liu, S.; Xiong, C.; Zhao, C.; Zhao, J.; Gan, Z.; Chen, Y.; Yang, K.; Liu, D.; et al. Fiber-tip polymer clamped-beam probe for high-sensitivity nanoforce measurements. Light Sci. Appl. 2021, 10, 171. [Google Scholar] [CrossRef] [PubMed]
- Gunawardena, D.S.; Cui, J.; Cheng, X.; Vadivelu, A.N.; Mohammadi, A.; Edbert, G.; Liu, Z.; Chen, B.; Oetomo, D.; O’Leary, S.; et al. Polymeric fiber sensors for insertion forces and trajectory determination of cochlear implants in hearing preservation. Biosens. Bioelectron. 2023, 222, 114866. [Google Scholar] [CrossRef] [PubMed]
- Shen, C.; Zhong, C.; Liu, D.; Lian, X.; Zheng, J.; Wang, J.J.; Semenova, Y.; Farrell, G.; Albert, J.; Donegan, J.F. Measurements of milli-Newton surface tension forces with tilted fiber Bragg gratings. Opt. Lett. 2018, 43, 255–258. [Google Scholar] [CrossRef] [PubMed]
- Leung, C.K.; Wan, K.T.; Inaudi, D.; Bao, X.; Habel, W.; Zhou, Z.; Ghandehari, M.; Wu, H.C.; Imai, M. Optical fiber sensors for civil engineering applications. Mater. Struct. 2015, 48, 871–906. [Google Scholar] [CrossRef]
- Beiu, R.-M.; Beiu, V.; Duma, V.-F. Fiber optic mechanical deformation sensors employing perpendicular photonic crystals. Opt. Express 2017, 25, 23388–23398. [Google Scholar] [CrossRef]
- Xu, J.; Song, A. A Miniature Multiaxis Force/Torque Sensor for Acupuncture. IEEE Sens. J. 2023, 23, 6660–6671. [Google Scholar] [CrossRef]
- Kang, J.U. (Ed.) Fiber Optic Sensing and Imaging; Springer Science & Business Media: New York, NY, USA, 2013. [Google Scholar]
- Wang, Y.; Yan, Y.; Lian, Z.; Chen, D.; Lau, A.P.T.; Lu, C. Fabry–Perot interferometers for highly-sensitive multi-point relative humidity sensing based on Vernier effect and digital signal processing. Opt. Express 2022, 30, 39946–39960. [Google Scholar] [CrossRef]
- Guo, Y.; Kong, J.; Liu, H.; Xiong, H.; Li, G.; Qin, L. A three-axis force fingertip sensor based on fiber Bragg grating. Sens. Actuators A Phys. 2016, 249, 141–148. [Google Scholar] [CrossRef]
- Dey, K.; Pavan, V.; Buddu, R.; Roy, S. Axial force analysis using half-etched FBG sensor. Opt. Fiber Technol. 2021, 64, 102548. [Google Scholar] [CrossRef]
- Luo, W.; Kou, J.-L.; Chen, Y.; Xu, F.; Lu, Y.-Q. Ultra-highly sensitive surface-corrugated microfiber Bragg grating force sensor. Appl. Phys. Lett. 2012, 101, 133502. [Google Scholar] [CrossRef]
- Wang, X.; Wang, Y.; Ling, Q.; Zhang, Q.; Luo, W.; Yu, Z.; Tao, C.; Jiang, X.; Chen, H.; Guan, Z.; et al. Seven-core fiber embedded ultra-long period grating for curvature, torsion or temperature sensing. Opt. Commun. 2023, 536, 129351. [Google Scholar] [CrossRef]
- Wang, J.; Wang, A.; Yang, X.; Wang, S.; Zeng, X.; Hao, J.; Zhou, J.; Kou, Y.; Niu, C.; Geng, T.; et al. An exoskeleton type long-period fiber grating for cross-talk-resistance single-parameter measurement. Opt. Lett. 2023, 48, 1272–1275. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Zhang, Y.; Zhang, W.; Yan, T.; Zhang, Y. High-sensitive optical force sensor based on enhanced effective index modulation. Optik 2018, 168, 684–691. [Google Scholar] [CrossRef]
- Li, X.; Zhang, Y.; Zhang, W.; Yan, T. High-sensitivity temperature-independent force sensor based on PS-LPFG formed by inserting a microbend. J. Opt. 2017, 19, 035801. [Google Scholar] [CrossRef]
- Statkiewicz-Barabach, G.; Kowal, D.; Szczurowski, M.K.; Mergo, P.; Urbanczyk, W. Hydrostatic Pressure and Strain Sensitivity of Long Period Grating Fabricated in Polymer Microstructured Fiber. IEEE Photon. Technol. Lett. 2013, 25, 496–499. [Google Scholar] [CrossRef]
- Viveiros, D.; de Almeida, J.M.M.M.; Coelho, L.; Vasconcelos, H.C.A.S.G.; Maia, J.M.; Amorim, V.A.; Jorge, P.A.S.; Marques, P.V.S. Turn Around Point Long Period Fiber Gratings With Coupling to Asymmetric Cladding Modes Fabricated by a Femtosecond Laser and Coated With Titanium Dioxide. J. Light. Technol. 2021, 39, 4784–4793. [Google Scholar] [CrossRef]
- Luo, B.; Liu, Z.; Wang, X.; Shi, S.; Zhong, N.; Ma, P.; Wu, S.; Wu, D.; Zhao, M.; Liang, W. Dual-peak long period fiber grating coated with graphene oxide for label-free and specific assays of H5N1 virus. J. Biophotonics 2021, 14, e202000279. [Google Scholar] [CrossRef]
- Liu, L.; Grillo, F.; Canfarotta, F.; Whitcombe, M.; Morgan, S.P.; Piletsky, S.; Correia, R.; He, C.; Norris, A.; Korposh, S. Carboxyl-fentanyl detection using optical fibre grating-based sensors functionalised with molecularly imprinted nanoparticles. Biosens. Bioelectron. 2021, 17, 113002. [Google Scholar] [CrossRef]
- Dey, T.K.; Tombelli, S.; Biswas, P.; Giannetti, A.; Basumallick, N.; Baldini, F.; Bandyopadhyay, S.; Trono, C. Label-free immunosensing by long period fiber gratings at the lowest order cladding mode and near turn around point. Opt. Laser Technol. 2021, 142, 107194. [Google Scholar] [CrossRef]
- Ling, Q.; Gu, Z.; Jiang, X.; Gao, K. Design of long period fiber grating surrounding refractive index sensor based on mode transition near phase-matching turning point. Opt. Commun. 2019, 439, 187–192. [Google Scholar] [CrossRef]
- Erdogan, T. Fiber grating spectra. J. Light. Technol. 1997, 15, 1277–1294. [Google Scholar] [CrossRef]
- Yang, Y.; Hong, C.; Abro, Z.A.; Wang, L.; Yifan, Z. A new Fiber Bragg Grating sensor based circumferential strain sensor fabricated using 3D printing method. Sens. Actuators A Phys. 2019, 295, 663–670. [Google Scholar] [CrossRef]
- Guan, T.; Gu, Z.; Ling, Q.; Feng, W. Tilted long-period fiber grating strain sensor based on dual-peak resonance near PMTP. Opt. Laser Technol. 2019, 114, 20–27. [Google Scholar] [CrossRef]


| Structure | Axial Force Sensitivity | Range | Fabricated Method | Ref. |
|---|---|---|---|---|
| Half-etched FBG | 1.96 nm/N | 0.20–2.50 N | Chemical corrosion with hydrofluoric acid | [10] |
| Microfiber-tapered FBG | 3146 nm/N | 0–0.0062 N | Focused ion beam machining | [11] |
| Microbend LPFG | 41.24 nm/N | 0–1.90 N | Inserting a microbend at the edge | [14] |
| LPFG fabricated in a polymer microstructure fiber | 1.39 nm/N | 0–16 N | Transverse periodic loading combined with fiber heating | [16] |
| Dual-peak LPFG | 14.047 nm/N | 0.490–4.508 N | UV laser | Our work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, W.; Wang, Y.; Ling, Q.; Guan, Z.; Chen, D.; Wu, Q. An Axial Force Sensor Based on a Long-Period Fiber Grating with Dual-Peak Resonance. Photonics 2023, 10, 591. https://doi.org/10.3390/photonics10050591
Luo W, Wang Y, Ling Q, Guan Z, Chen D, Wu Q. An Axial Force Sensor Based on a Long-Period Fiber Grating with Dual-Peak Resonance. Photonics. 2023; 10(5):591. https://doi.org/10.3390/photonics10050591
Chicago/Turabian StyleLuo, Weixuan, Ying Wang, Qiang Ling, Zuguang Guan, Daru Chen, and Qiong Wu. 2023. "An Axial Force Sensor Based on a Long-Period Fiber Grating with Dual-Peak Resonance" Photonics 10, no. 5: 591. https://doi.org/10.3390/photonics10050591
APA StyleLuo, W., Wang, Y., Ling, Q., Guan, Z., Chen, D., & Wu, Q. (2023). An Axial Force Sensor Based on a Long-Period Fiber Grating with Dual-Peak Resonance. Photonics, 10(5), 591. https://doi.org/10.3390/photonics10050591







