Polarization Snapshot Imaging Spectrometer for Infrared Range
Abstract
1. Introduction
2. Principle and System Parameters
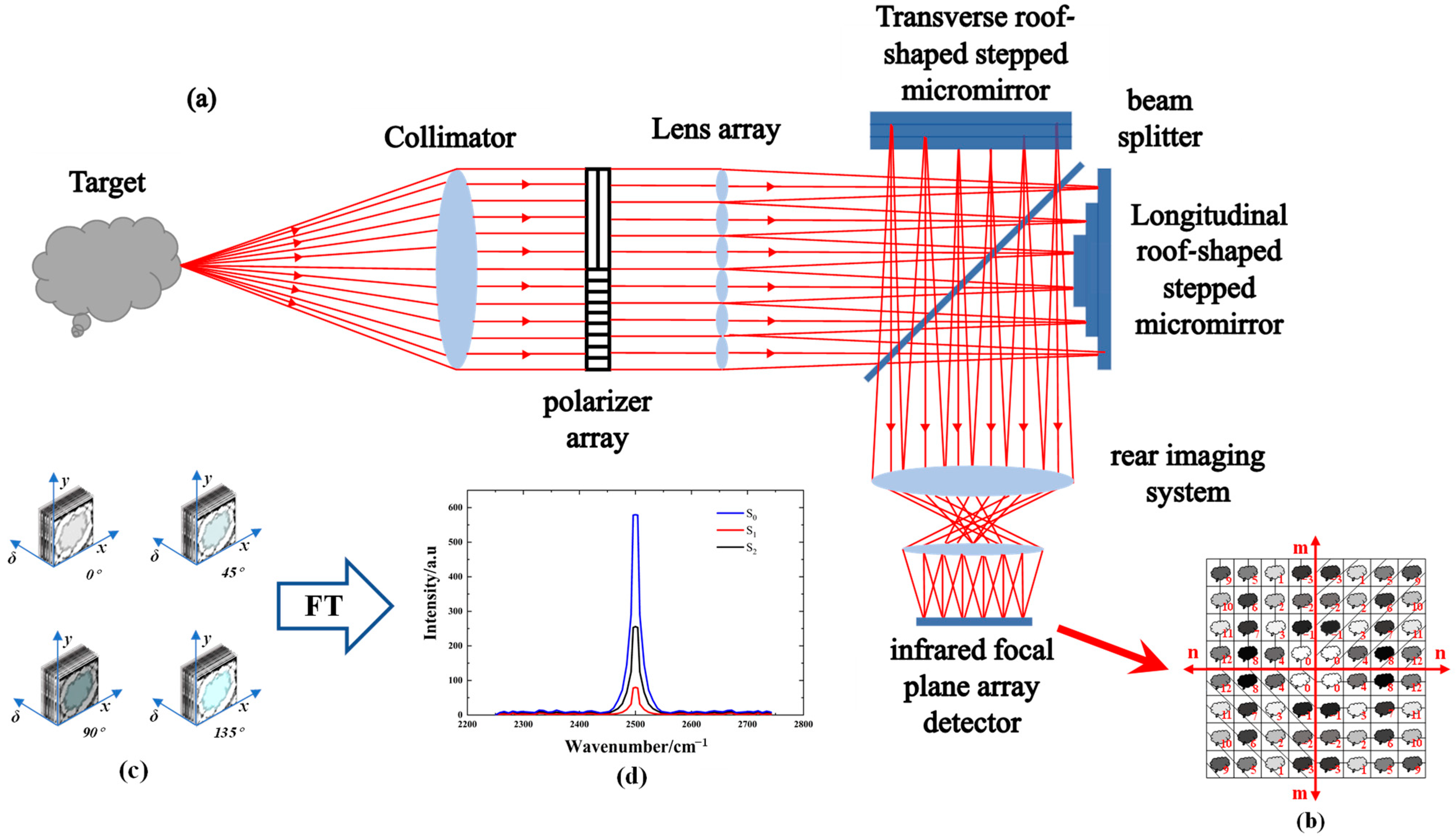
2.1. PSIFTIS Principle
2.2. System Parameters
3. System Optical Design and Evaluation
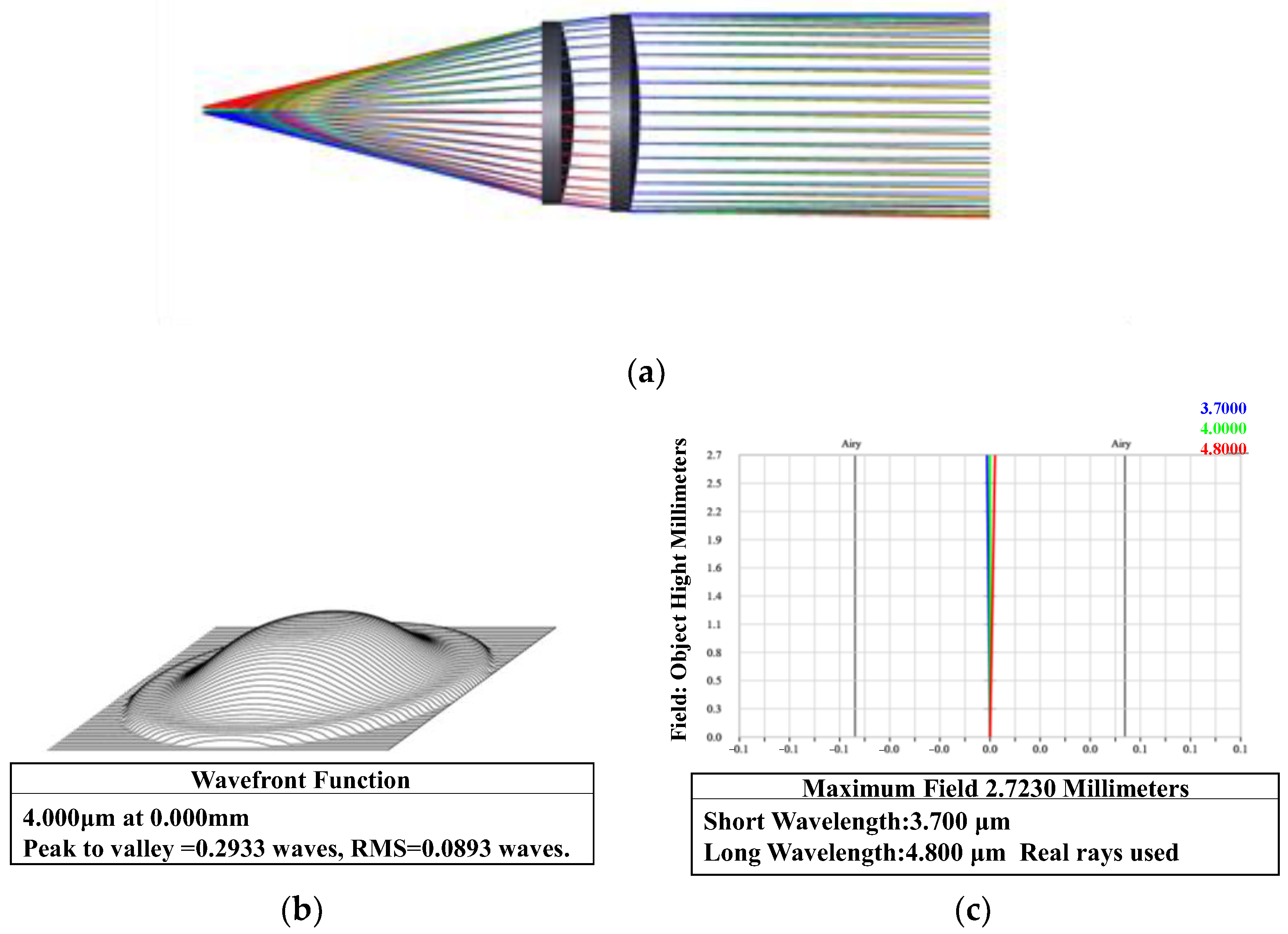
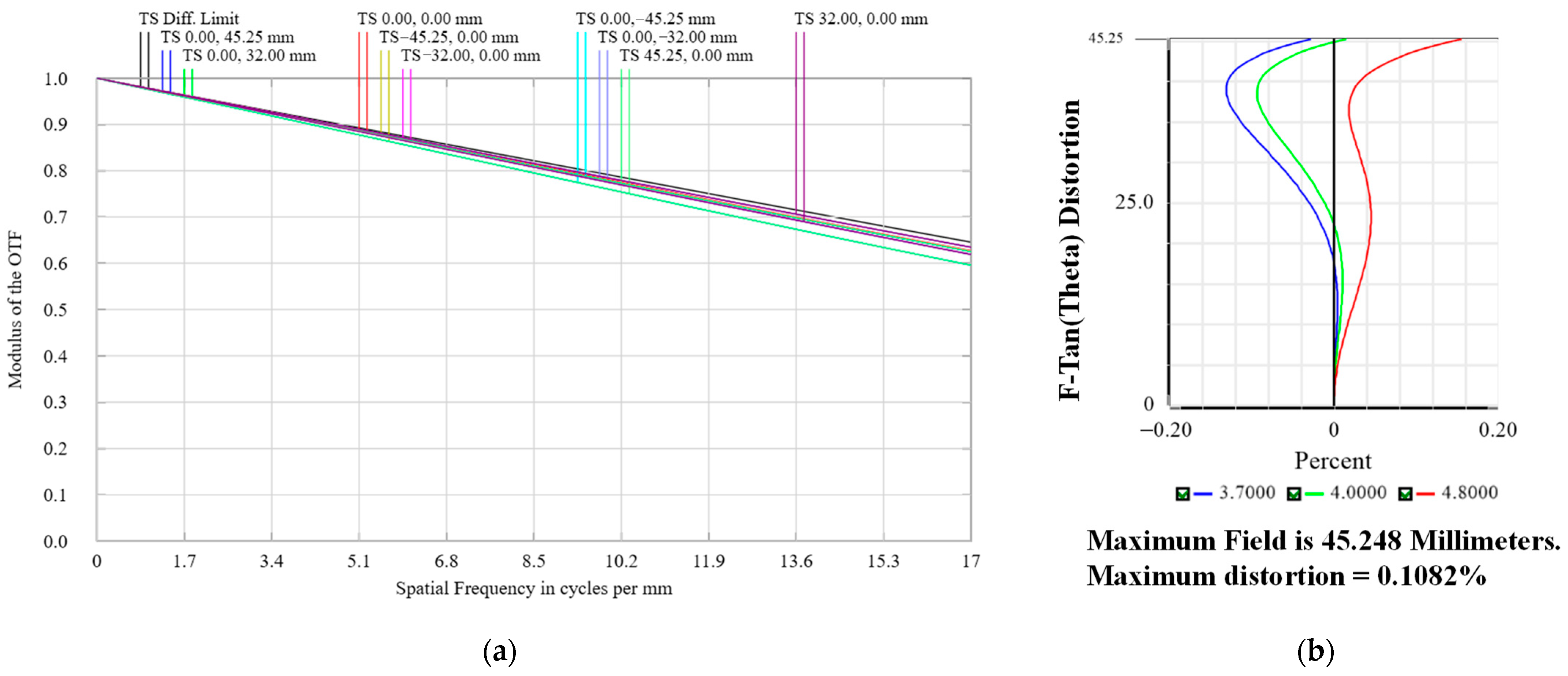
3.1. Analysis and Design of Collimation System and Lens Array
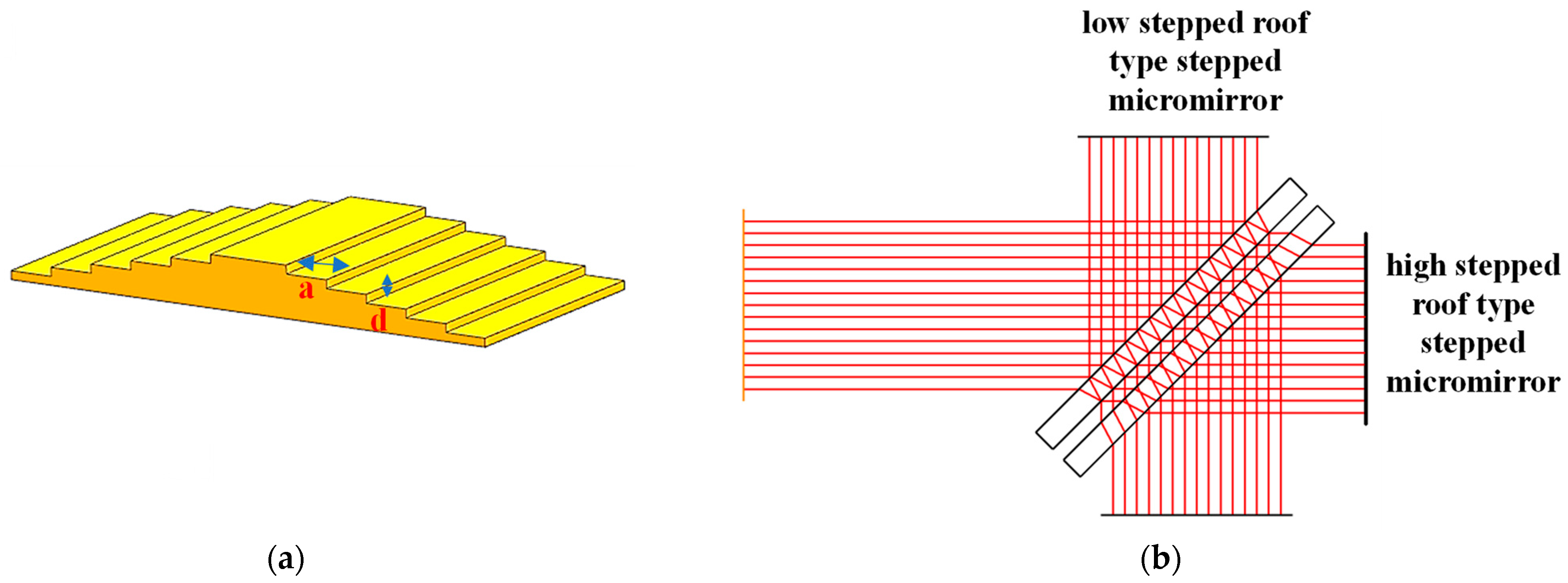
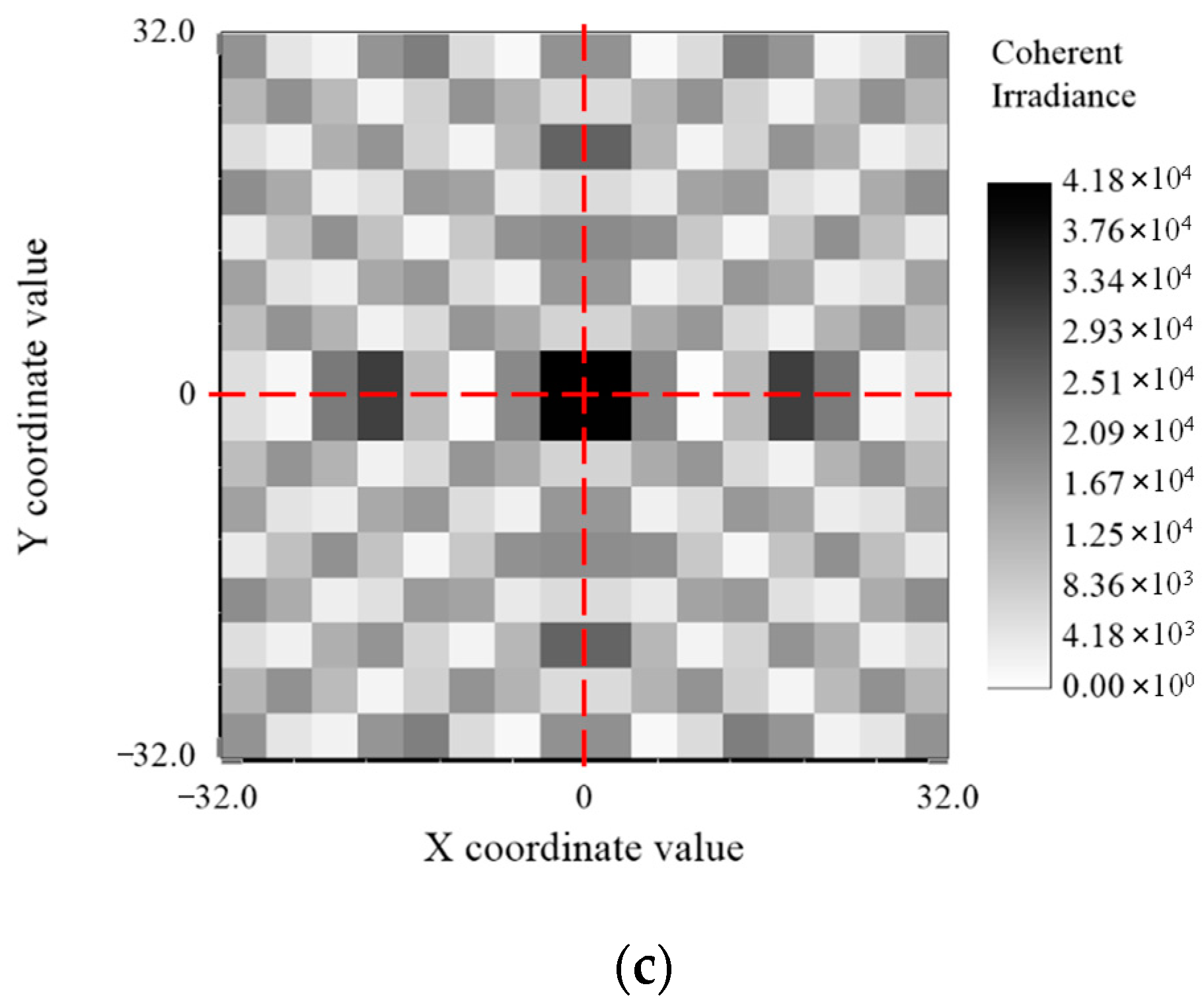
3.2. Interference System Analysis and Design
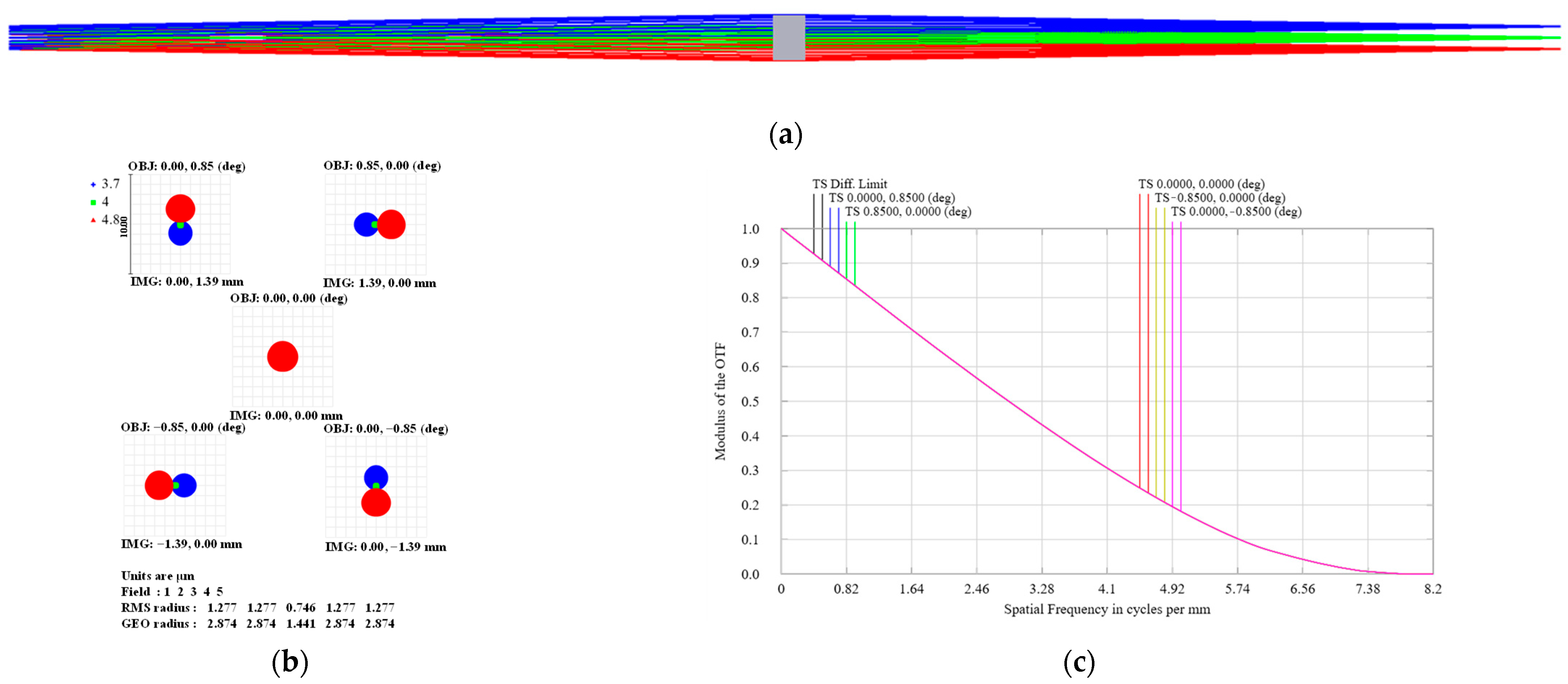
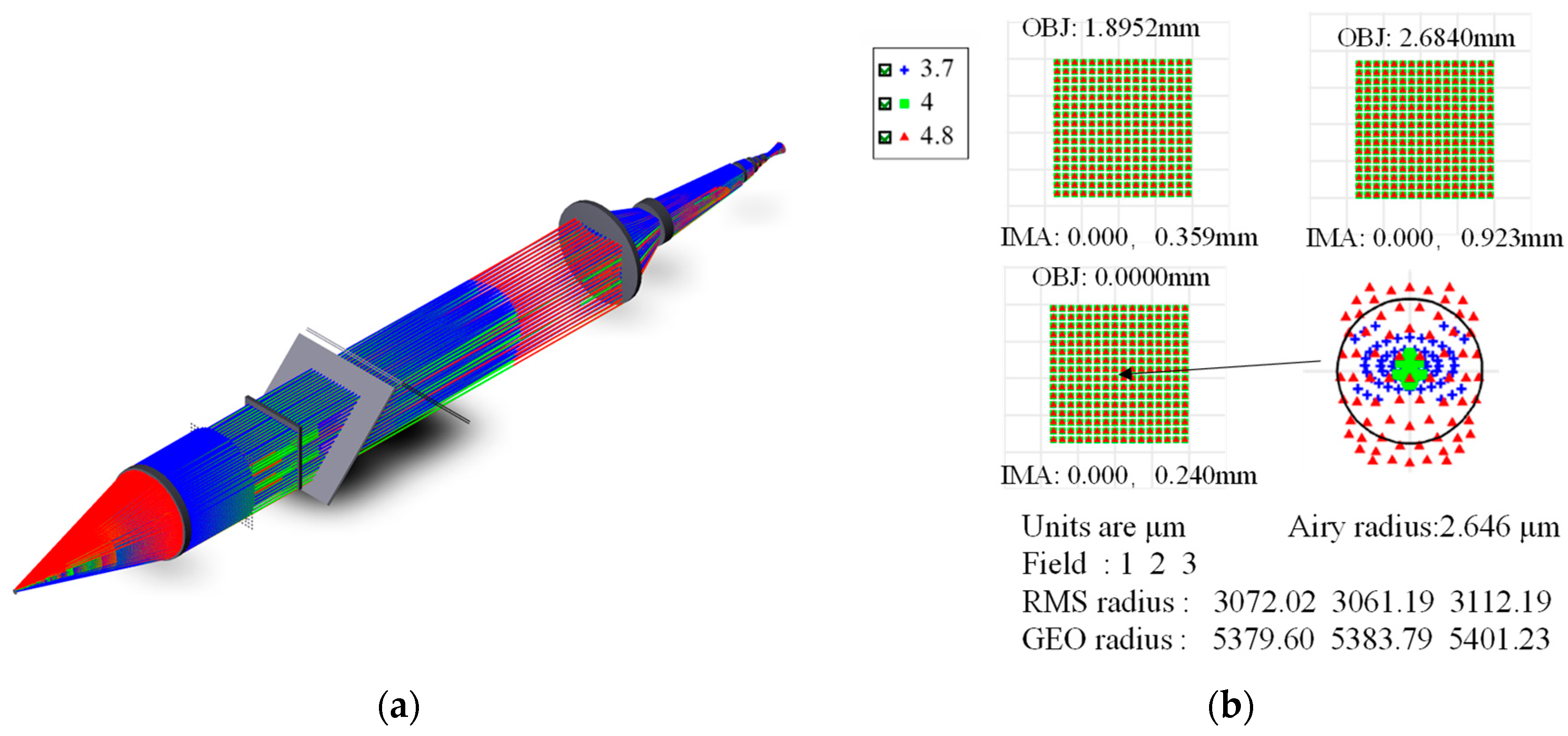
3.3. Analysis and Design of Rear Imaging System
3.4. Design and Error Analysis of Polarizer Array
4. Experiment and Discussion
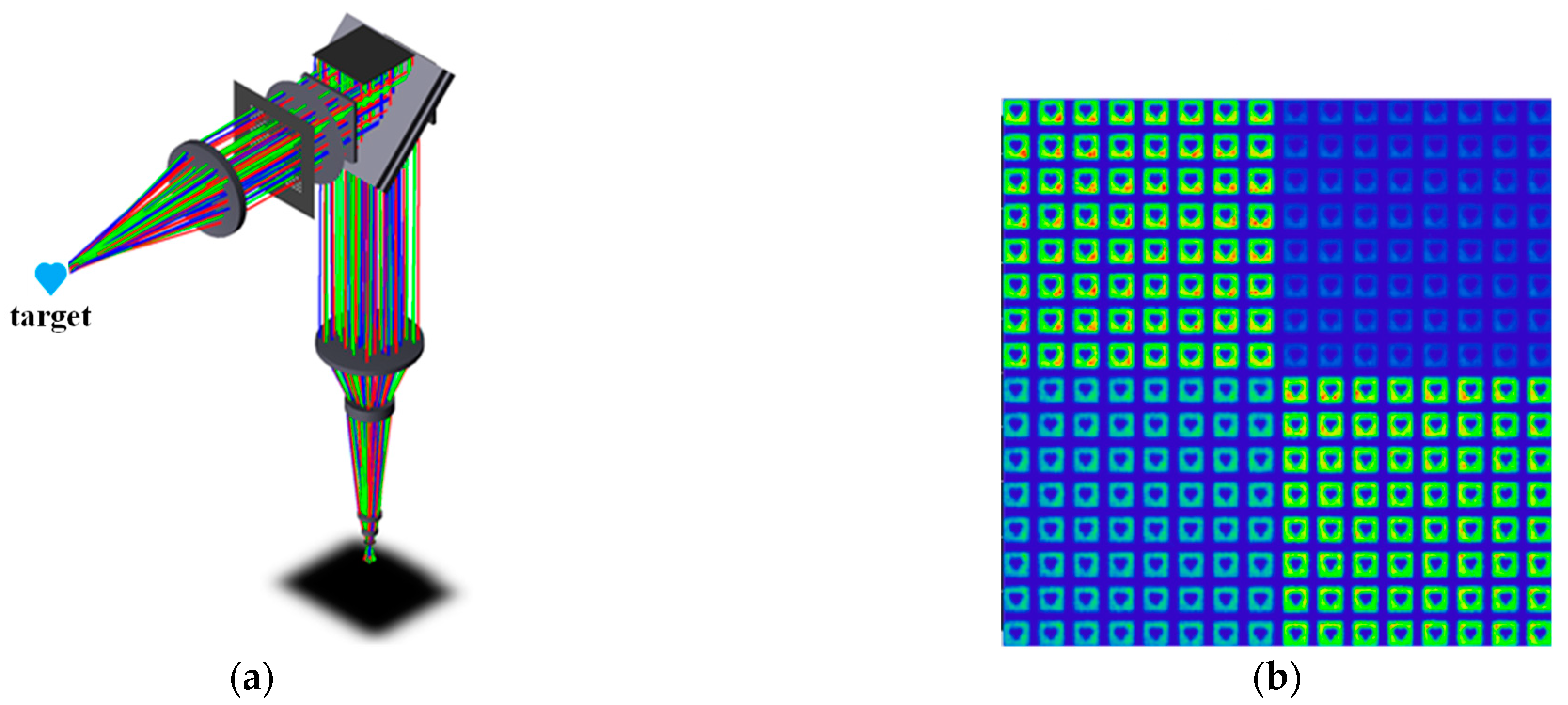
4.1. Geometric Optics Simulation Experiment
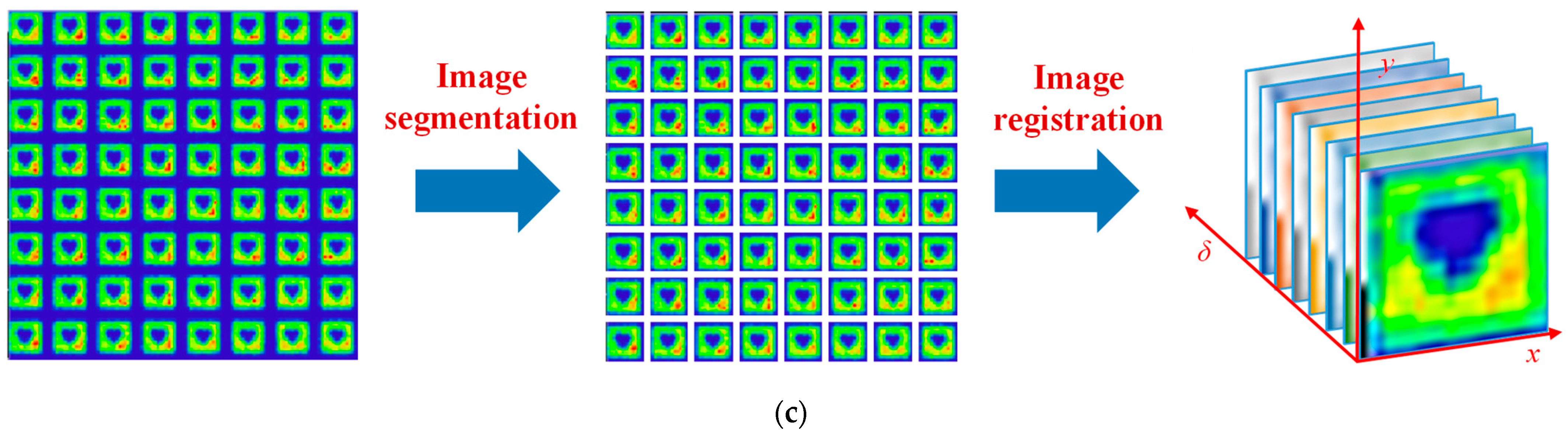
4.2. Simulation Experiment of Graph Reconstruction
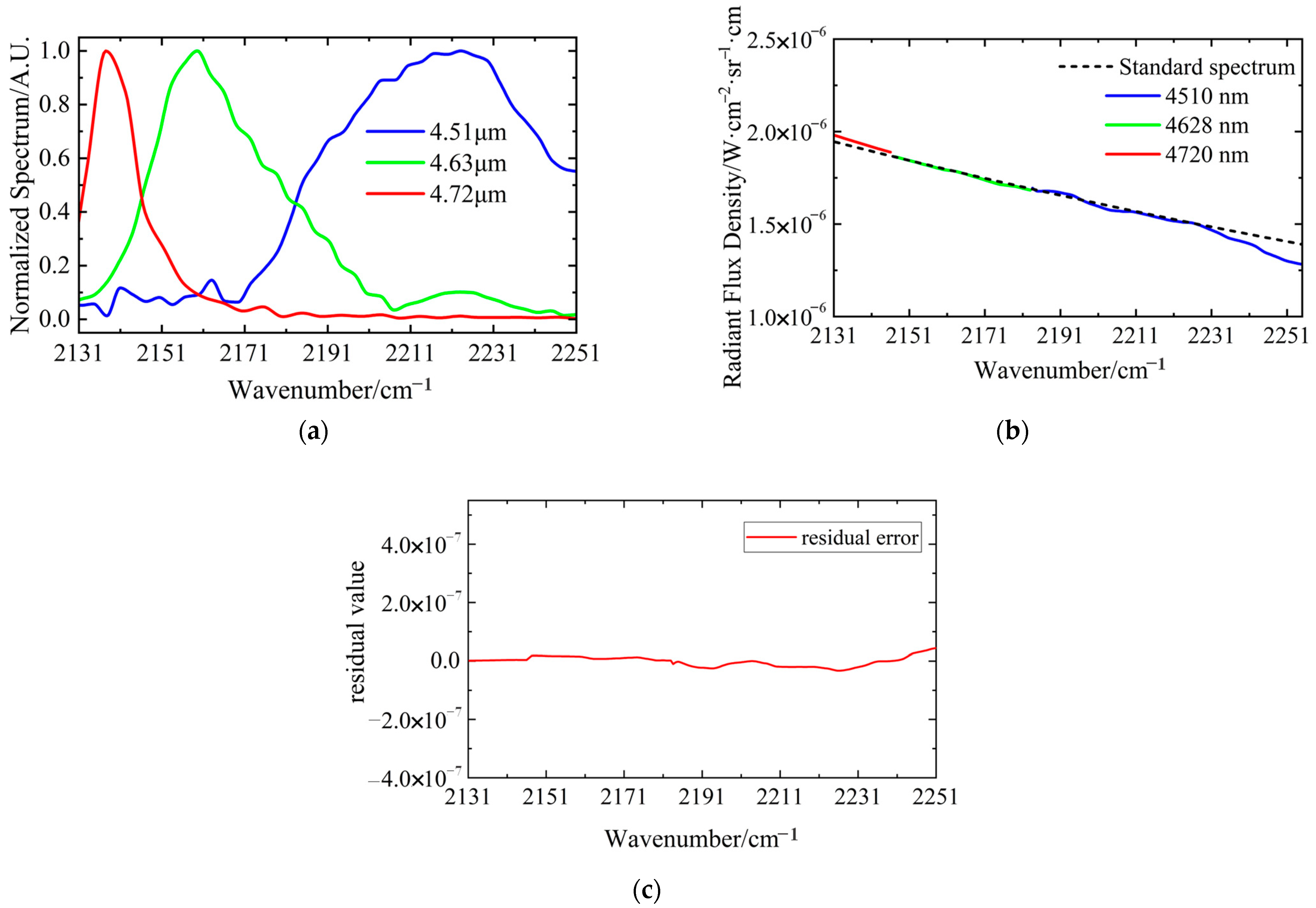
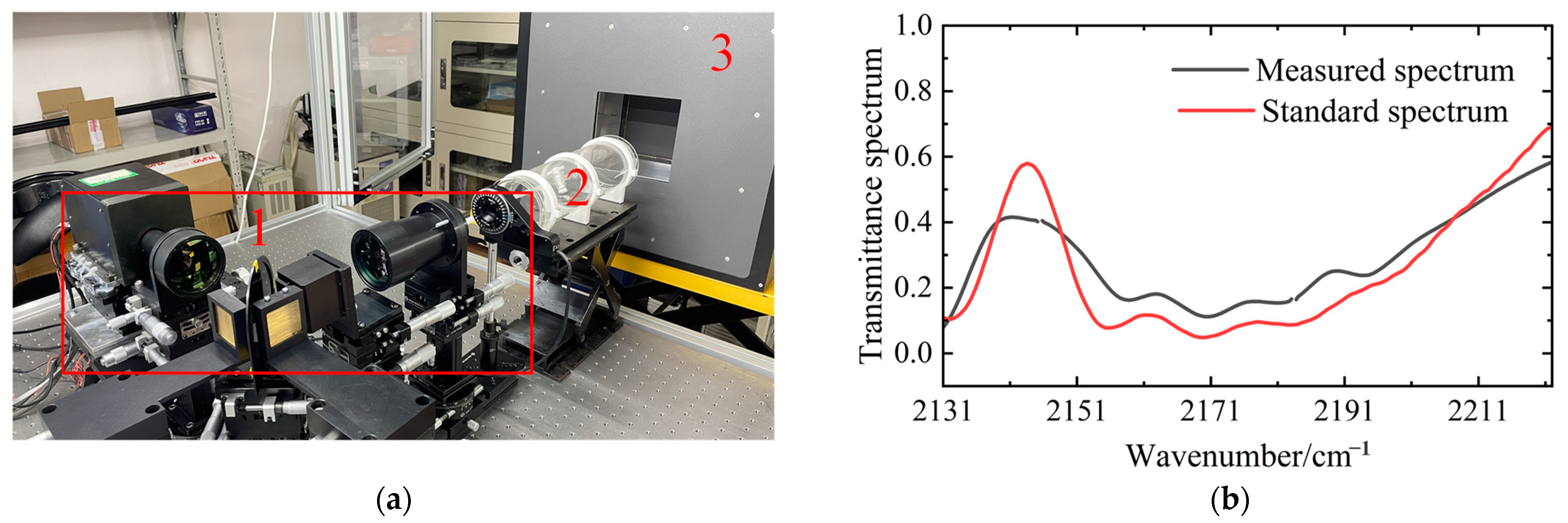
4.3. Experiment Results
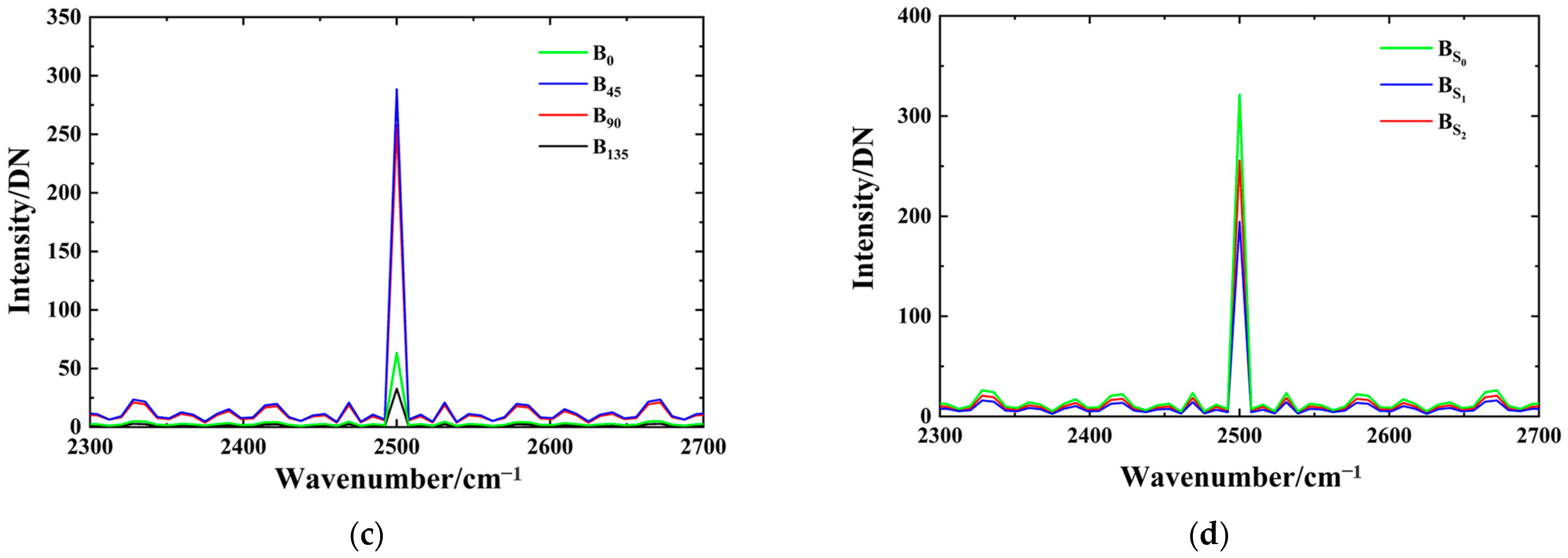
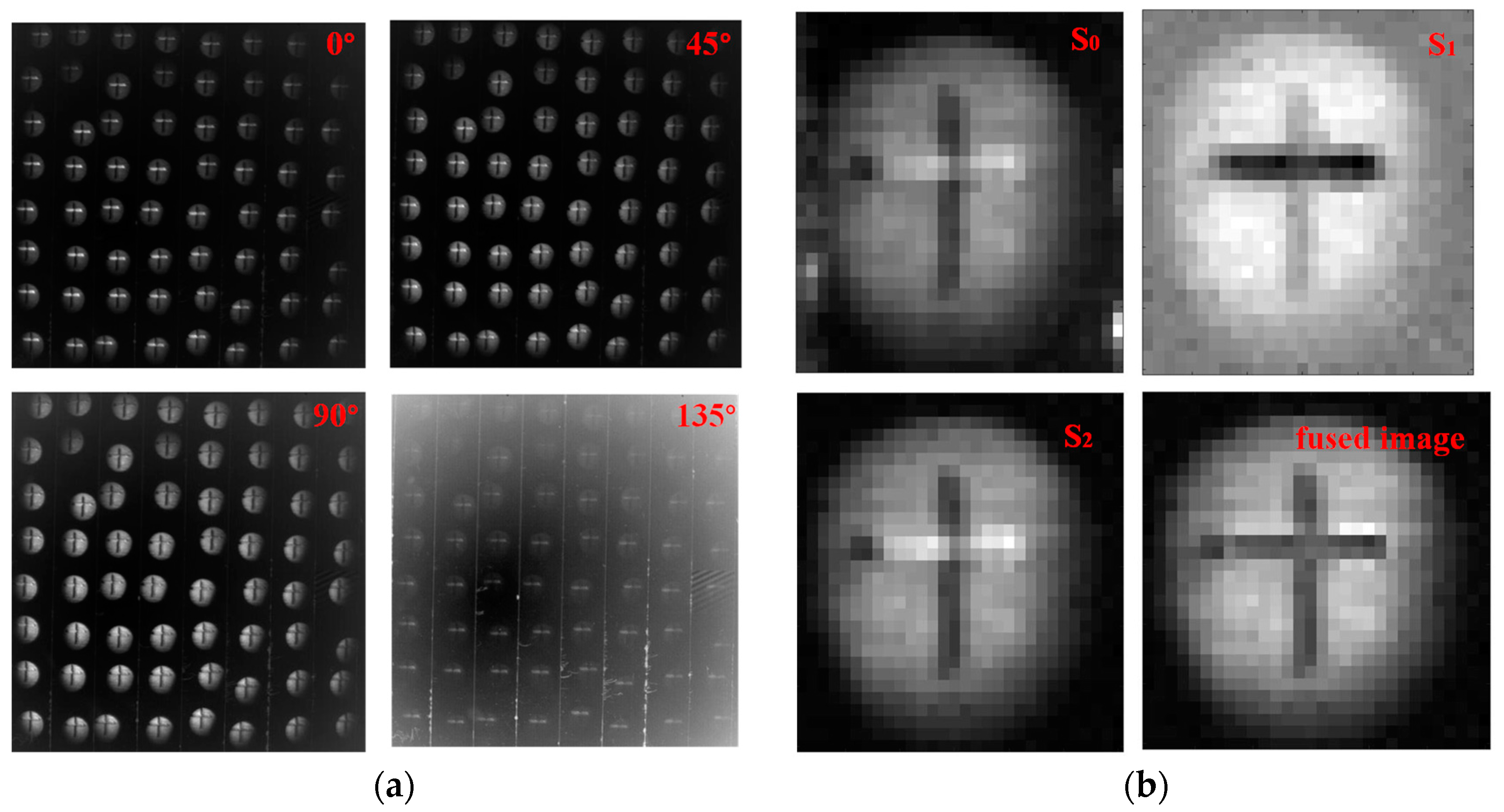
4.3.1. Polarization Diagram and Spectrum Detection Experiment
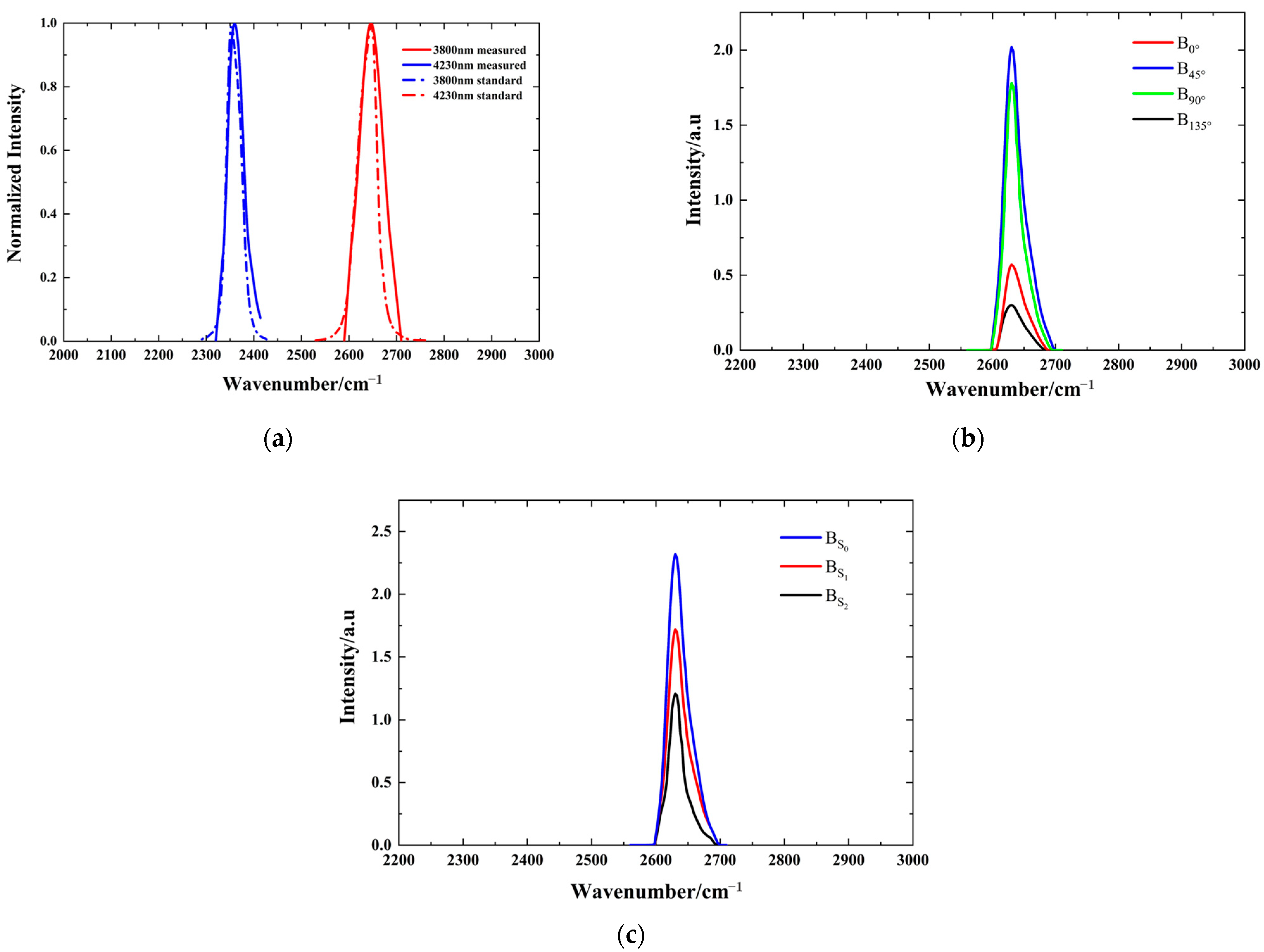
4.3.2. Wideband Detection Experiment and Gas Detection Experiment
4.4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Zhao, Y.; Zhang, L.; Pan, Q. Spectropolarimetric imaging for pathological analysis of skin. Appl. Opt. 2009, 48, 236–246. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Herten, G.V.; Boer, J.D.; Rietjens, J.H.H.; Noia, A.D.; Snik, F.; Volten, H.; Smit, J.M.; Hasekamp, O.P.; Henzing, J.S.; Keller, C.U. Atmospheric aerosol characterization with a ground-based SPEX spectropolarimetric instrument. Atmos. Meas. Tech. 2014, 12, 4341–4351. [Google Scholar] [CrossRef][Green Version]
- Jones, S.H.; Iannarilli, F.J.; Kebabian, P.L. Realization of quantitative-grade fieldable snapshot imaging spectropolarimeter. Opt. Express 2004, 12, 6559–6573. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Gu, Z.; Li, T. Method for modeling polarization characteristics of radar target. Foreign Electron. Meas. Technol. 2017, 36, 44–47. [Google Scholar]
- Snik, F.; Karalidi, T.; Keller, C.U. Spectral modulation for full linear polarimetry. Appl. Opt. 2009, 48, 1337–1346. [Google Scholar] [CrossRef] [PubMed]
- Thyparambil, A.A.; Yang, W.; Latour, R.A. Experimental characterization of adsorbed protein orientation, conformation, and bioactivity. Biointerphases 2015, 10, 019002. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Halajian, J.D.; Hallock, H.B. Computerized Terrain Mapping System. US3864513, 4 February 1975. [Google Scholar]
- Kohzo, H.; Hirokimi, S.; Hiromichi, Y.; Hirokimi, K.; Michio, S. Application of an Imaging spectropolarimeter to agro-environmental sciences. Proc. SPIE 2004, 5234, 638–647. [Google Scholar]
- Kohzo, H.; Hirokimi, S.; Hirohisa, K.; Hiromichi, Y. Outdoor experiments of LCTF spectropolarimeters for earth observation. Proc. SPIE 2004, 5234, 628–637. [Google Scholar]
- Kim, J.; Escuti, J.M. Snapshot imaging spectropolarimeter utilizing polarization gratings. Proc. SPIE 2008, 7086, 29–38. [Google Scholar]
- Zhao, Y.; Pan, Q.; Zhang, H. Material classification based on multi-band polarimetric images fusion. In Polarization: Measurement, Analysis, and Remote Sensing VII; International Society for Optics and Photonics: Washington, DC, USA, 2006. [Google Scholar]
- Zhao, Y.; Pan, Q.; Zhang, H. Fuse spectropolarimetric imagery by D-S reasoning. In Polarization: Measurement, Analysis, and Remote Sensing VII; International Society for Optics and Photonics: Washington, DC, USA, 2006. [Google Scholar]
- Zhao, Y.; Gong, P.; Pan, Q. Object detection by spectropolarimeteric imagery fusion. IEEE Trans. Geosci. Remote Sens. 2008, 46, 3337–3345. [Google Scholar] [CrossRef]
- Gao, P.; Hu, X.; Guo, G.; Kang, Y.; Ai, J.; Wang, X. Modified Sagnac imaging spectropolarimeter for full linear Stokes parameters. Europhys. Lett. 2018, 124, 54003. [Google Scholar] [CrossRef]
- Zhang, R.; Wang, Z.; Li, K.; Chen, Y.; Jing, N.; Qiao, Y.; Xie, K. Spectropolarimetric measurement based on a fast-axis-adjustable photoelastic modulator. Appl. Opt. 2019, 58, 325–332. [Google Scholar] [CrossRef] [PubMed]
- Qin, X. Research on Infrared Polarized Light Imaging. Master’s Thesis, Changchun University of Science and Technology, Changchun, China, 2020. [Google Scholar]
- Bo, J.; Gu, Y.; Xing, W.; Ju, X.; Yan, C.; Wang, X. Spatially modulated snapshot computed tomographic polarization imaging spectrometer. Appl. Opt. 2021, 60, 5861–5865. [Google Scholar] [CrossRef] [PubMed]
- Kawabata, Y.; Katsukawa, Y.; Kubo, M.; Shinoda, K.; Tsuzuki, T.; Uraguchi, F. Polarimetric calibration of a spectropolarimeter instrument with high precision: Sunrise chromospheric infrared spectropolarimeter (SCIP) for the SUNRISE III balloon telescope. Appl. Opt. 2022, 61, 9716–9736. [Google Scholar] [CrossRef] [PubMed]
- Gu, Y.; Lv, J.; Bo, J.; Zhao, B.; Chen, Y.; Tao, J.; Qin, Y.; Wang, W.; Liang, J. Joint Dense 3D Reconstruction Method for Endoscopic Images of Weak Texture Scenes. IEEE Access 2021, 9, 138254–138266. [Google Scholar] [CrossRef]
- Zhao, B.; Lv, J.; Ren, J.; Qin, Y.; Wang, W. Data processing and performance evaluation of a tempo-spatially mixed modulation imaging Fourier transform spectrometer based on stepped micro-mirror. Opt. Express 2020, 28, 6320–6335. [Google Scholar] [CrossRef] [PubMed]
- Wang, H. Mid-Wave Infrared Spectral Polarization Imaging Technology and System Research. Ph.D. Dissertation, Changchun Institute of Optics, Fine Mechanicsand Physics, Chinese Academy of Sciences, Changchun, China, 2018. [Google Scholar]
- Zheng, Y.; Liang, J.; Liang, Z. Design and fabrication of step mirrors used in space-modulated Fourier transform infrared spectrometer. Opt. Express 2013, 21, 884–892. [Google Scholar] [CrossRef] [PubMed]
- Luo, Y.; Chen, Z. Measuring Principle and Measuring Method Design of Polarized Stokes Parameters. Adv. Lasers Optoelectron. 2009, 6, 54–58. [Google Scholar]
- Azzam, R.M. Stokes-vector and Mueller-matrix polarimetry. J. Opt. Soc. Am. A Opt. Image Sci. Vis. 2016, 33, 396–1408. [Google Scholar] [CrossRef] [PubMed]




| Parameters | Value |
|---|---|
| Wavelength | 3.7–4.8 μm |
| Spectral resolution | <10 cm−1 |
| Modulation transfer function | >0.5@17 lp/mm |
| Interference channel | 16 × 16 |
| Polarization channel | 4 |
| Parameters | Value |
|---|---|
| Wavelength | 3.7–4.8 μm |
| Half field of view | 0.85° |
| Focal length | 181 mm |
| Material | Germanium |
| Clear aperture | 90.5 mm |
| Parameters | Value |
|---|---|
| Left/right steps | 8 |
| Step width | 4 mm |
| Low step height | 10 μm |
| High step height | 80 μm |
| Sampling interval | 20 μm |
| Parameters | Value |
|---|---|
| Wavelength | 3.7–4.8 μm |
| Magnification ratio | −0.12 |
| Object-space numerical aperture | 0.015 |
| Distortion | <0.15% |
| MTF@17 lp | >0.5 |
| Error Angle | S0 Error | S1 Error | P Error |
|---|---|---|---|
| 0.5° | 0.594% | 0.996% | 0.244% |
| 1° | 1.376% | 2.290% | 0.565% |
| 1.5° | 2.144% | 3.572% | 0.916% |
| 2° | 2.829% | 4.714% | 1.193% |
| 2.5° | 3.598% | 6.012% | 1.539% |
| 3° | 4.324% | 7.213% | 1.872% |
| 3.5° | 5.093% | 8.496% | 2.240% |
| 4° | 5.858% | 9.772% | 2.613% |
| 4.5° | 6.624% | 11.051% | 2.998% |
| 5° | 7.352% | 12.274% | 3.377% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tao, H.; Lv, J.; Liang, J.; Zhao, B.; Chen, Y.; Zheng, K.; Zhao, Y.; Wang, W.; Qin, Y.; Liu, G.; et al. Polarization Snapshot Imaging Spectrometer for Infrared Range. Photonics 2023, 10, 566. https://doi.org/10.3390/photonics10050566
Tao H, Lv J, Liang J, Zhao B, Chen Y, Zheng K, Zhao Y, Wang W, Qin Y, Liu G, et al. Polarization Snapshot Imaging Spectrometer for Infrared Range. Photonics. 2023; 10(5):566. https://doi.org/10.3390/photonics10050566
Chicago/Turabian StyleTao, Hongcheng, Jinguang Lv, Jingqiu Liang, Baixuan Zhao, Yupeng Chen, Kaifeng Zheng, Yingze Zhao, Weibiao Wang, Yuxin Qin, Guohao Liu, and et al. 2023. "Polarization Snapshot Imaging Spectrometer for Infrared Range" Photonics 10, no. 5: 566. https://doi.org/10.3390/photonics10050566
APA StyleTao, H., Lv, J., Liang, J., Zhao, B., Chen, Y., Zheng, K., Zhao, Y., Wang, W., Qin, Y., Liu, G., & Sheng, K. (2023). Polarization Snapshot Imaging Spectrometer for Infrared Range. Photonics, 10(5), 566. https://doi.org/10.3390/photonics10050566





