All-Glass Single-Mode Leakage Channel Microstructured Optical Fibers with Large Mode Area and Low Bending Loss
Abstract
1. Introduction
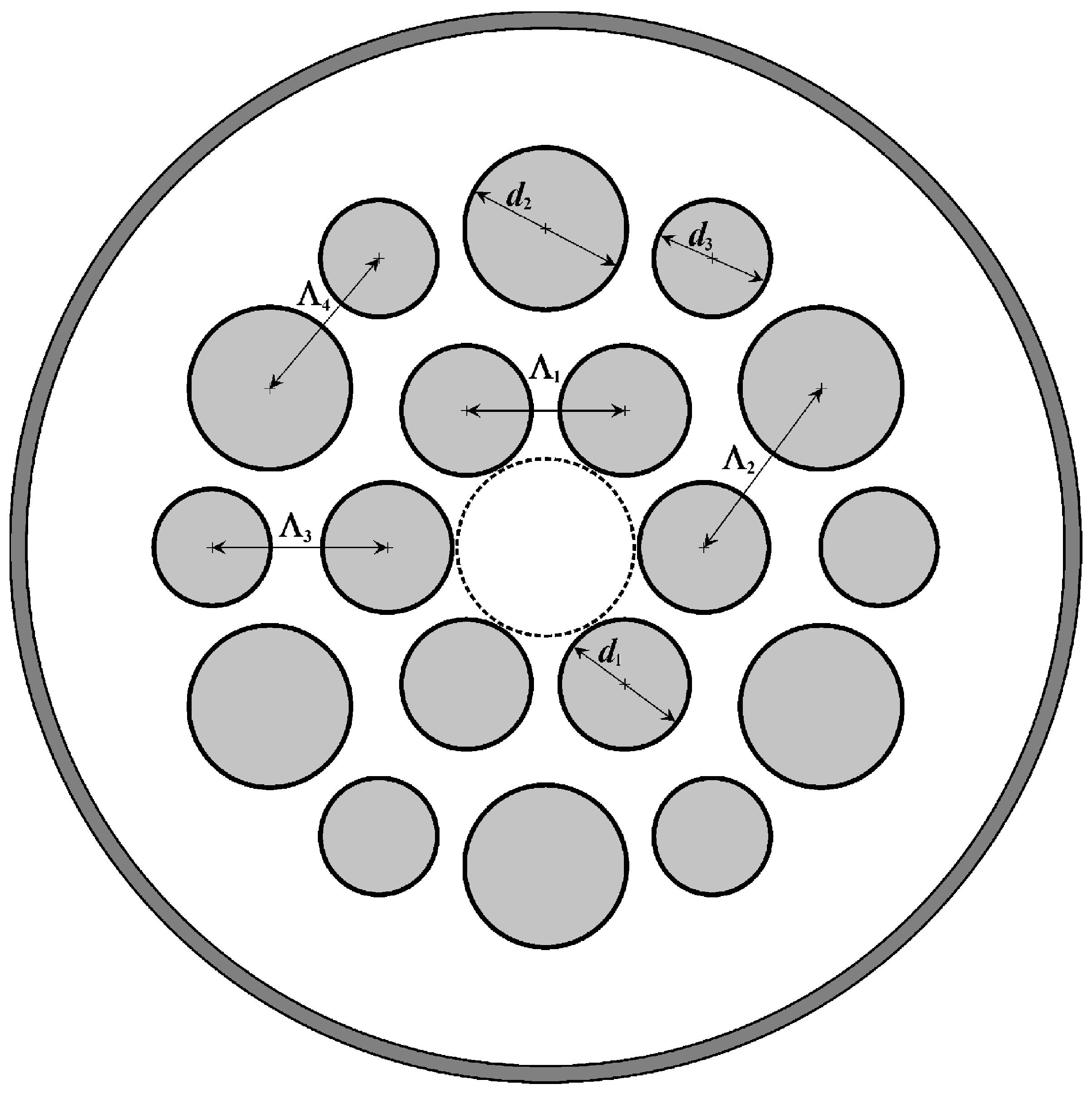
2. Materials and Methods
3. Calculation Results
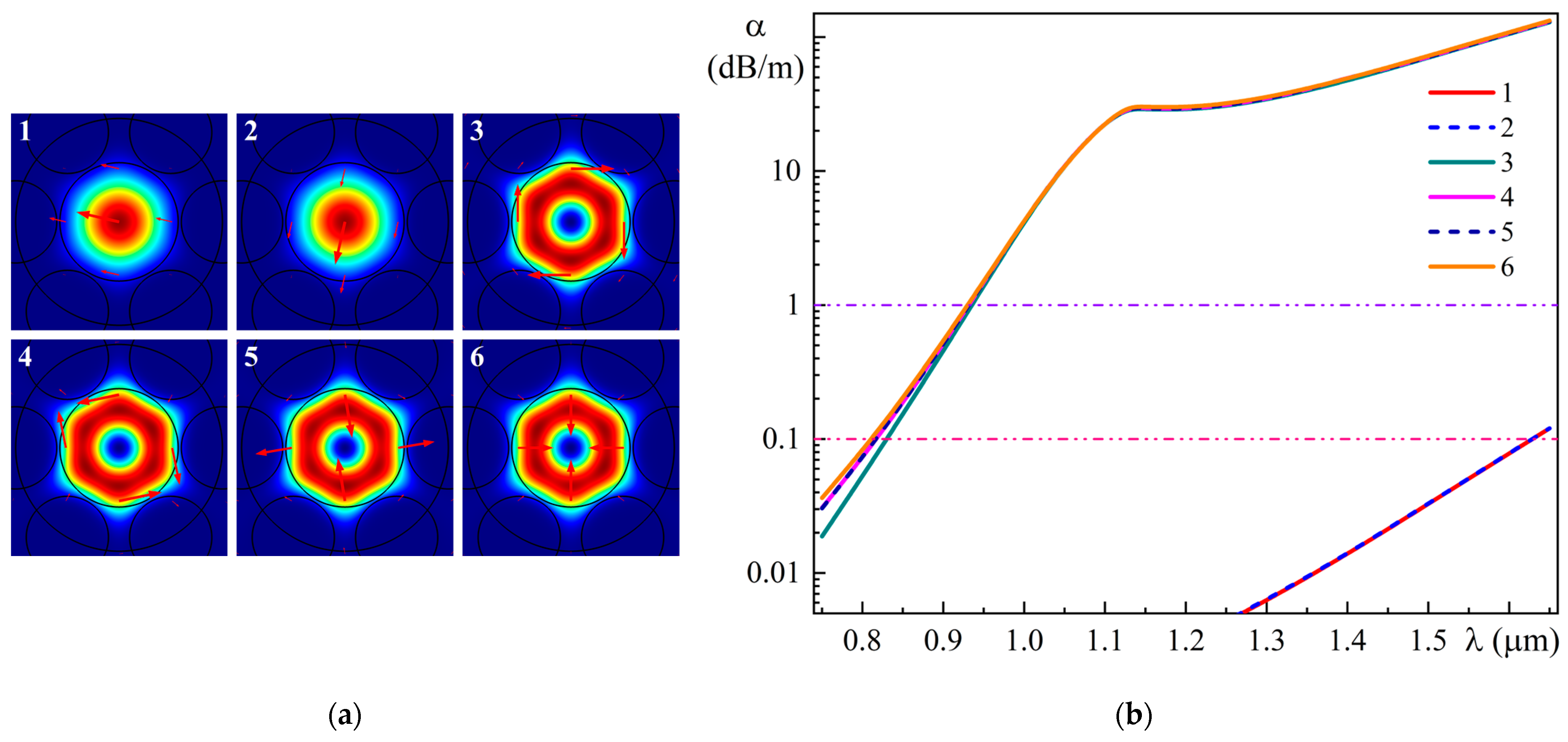
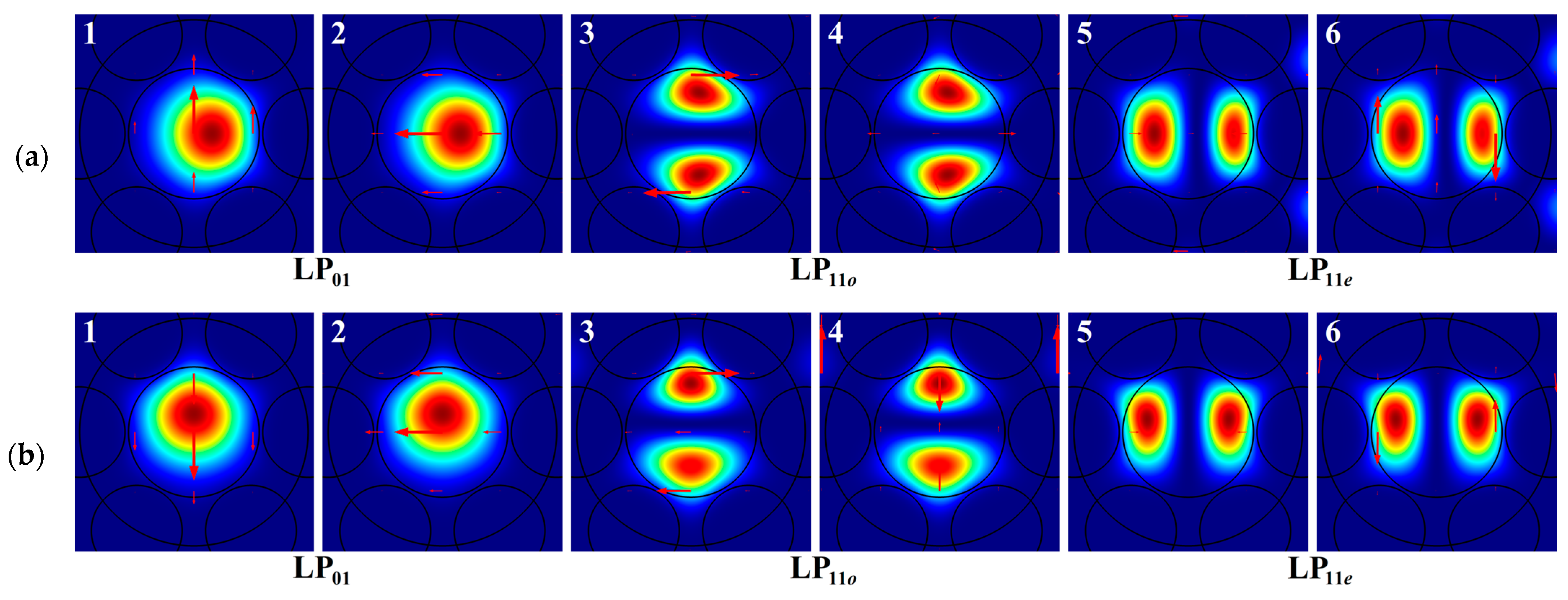
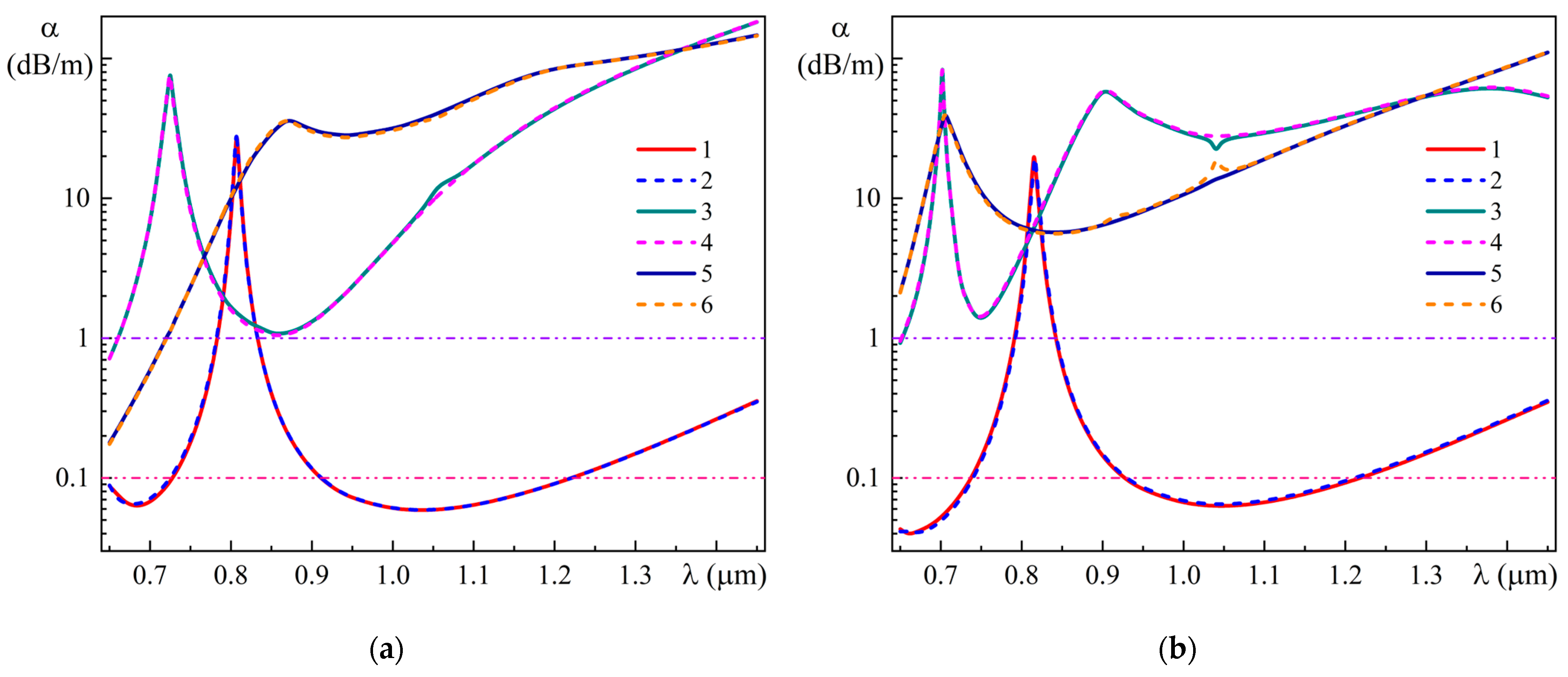
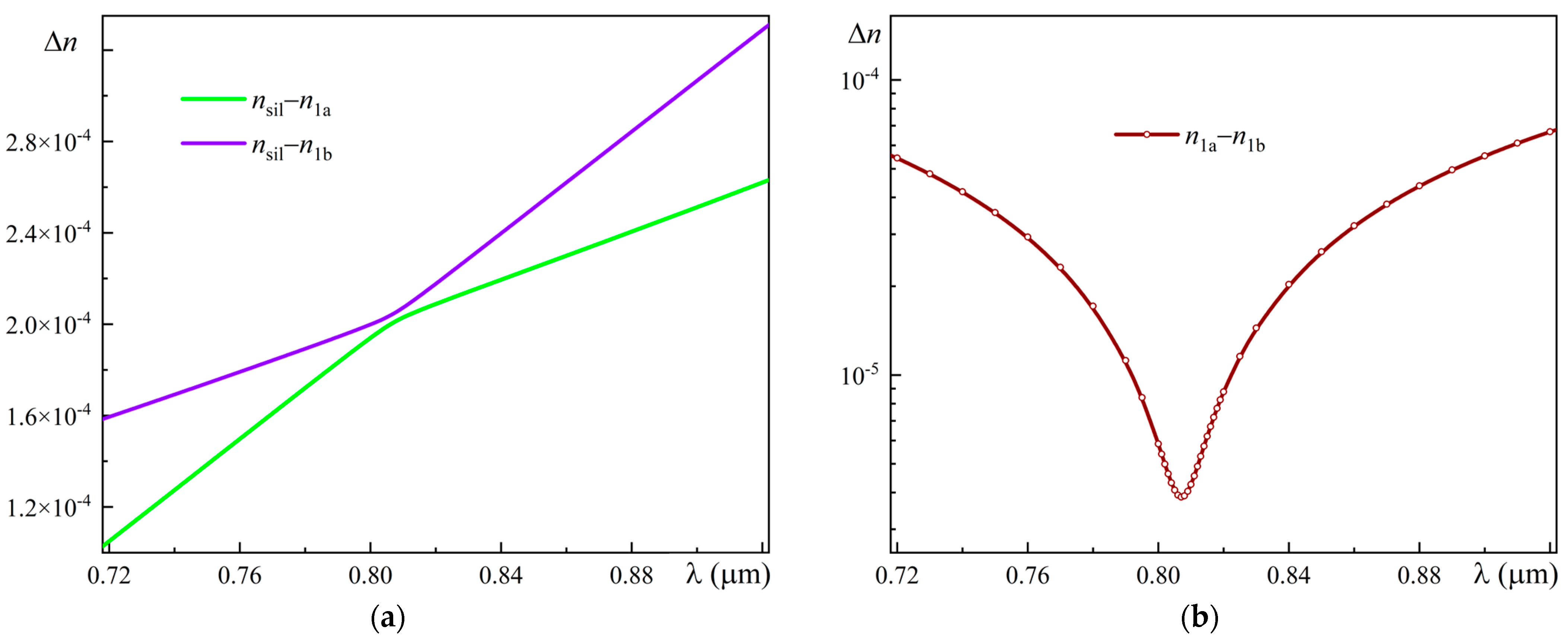
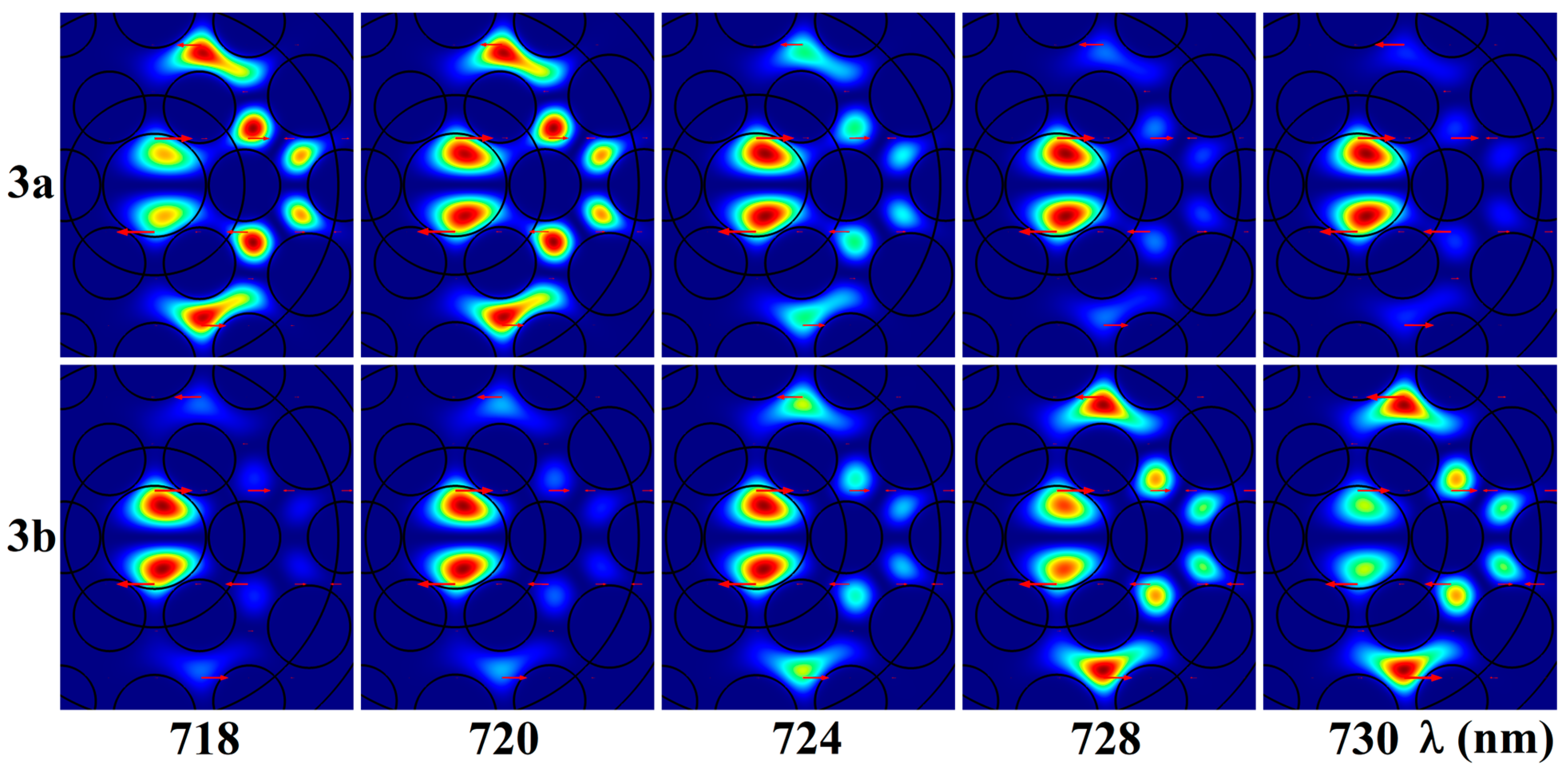
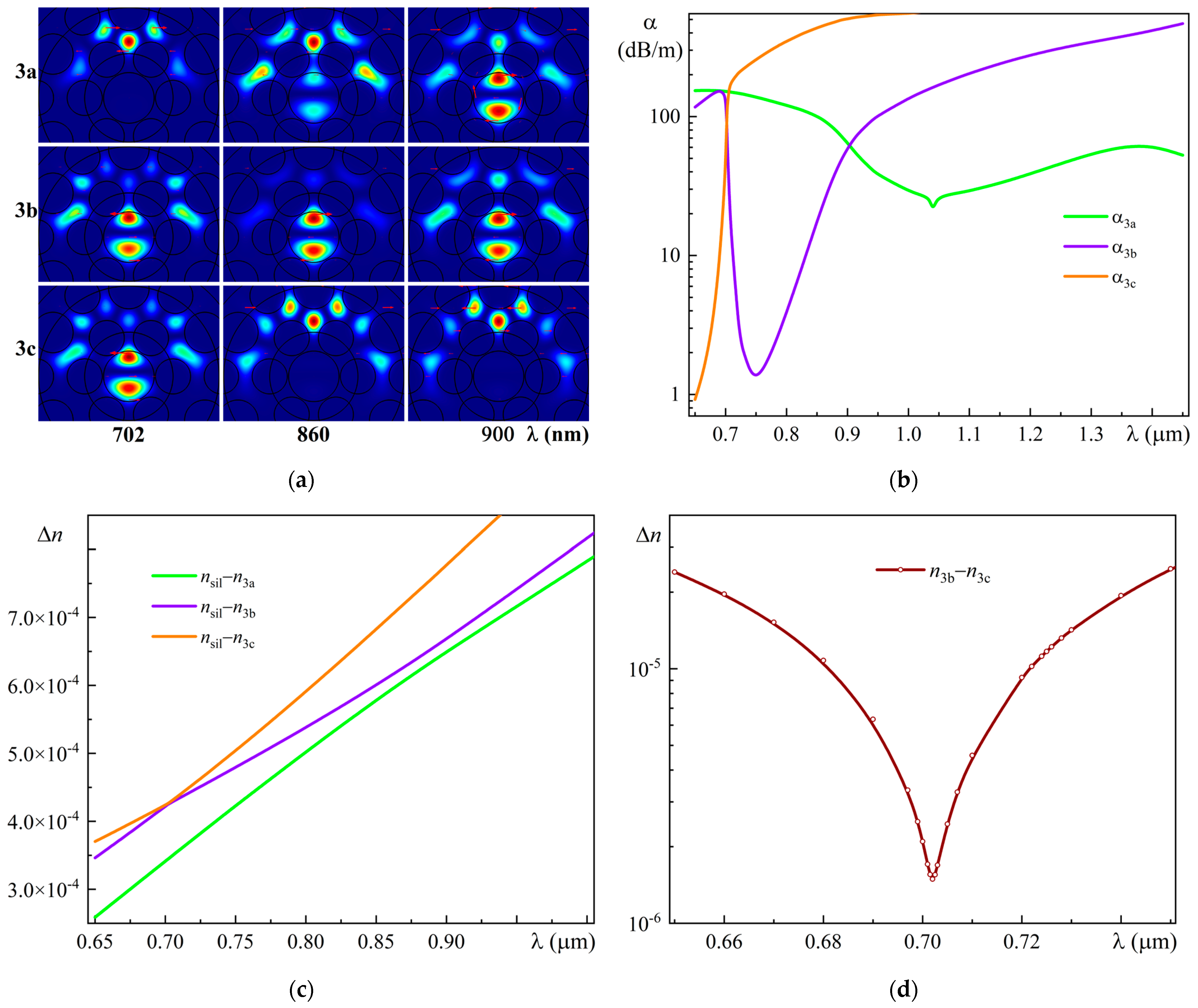
3.1. Straight MOF-18
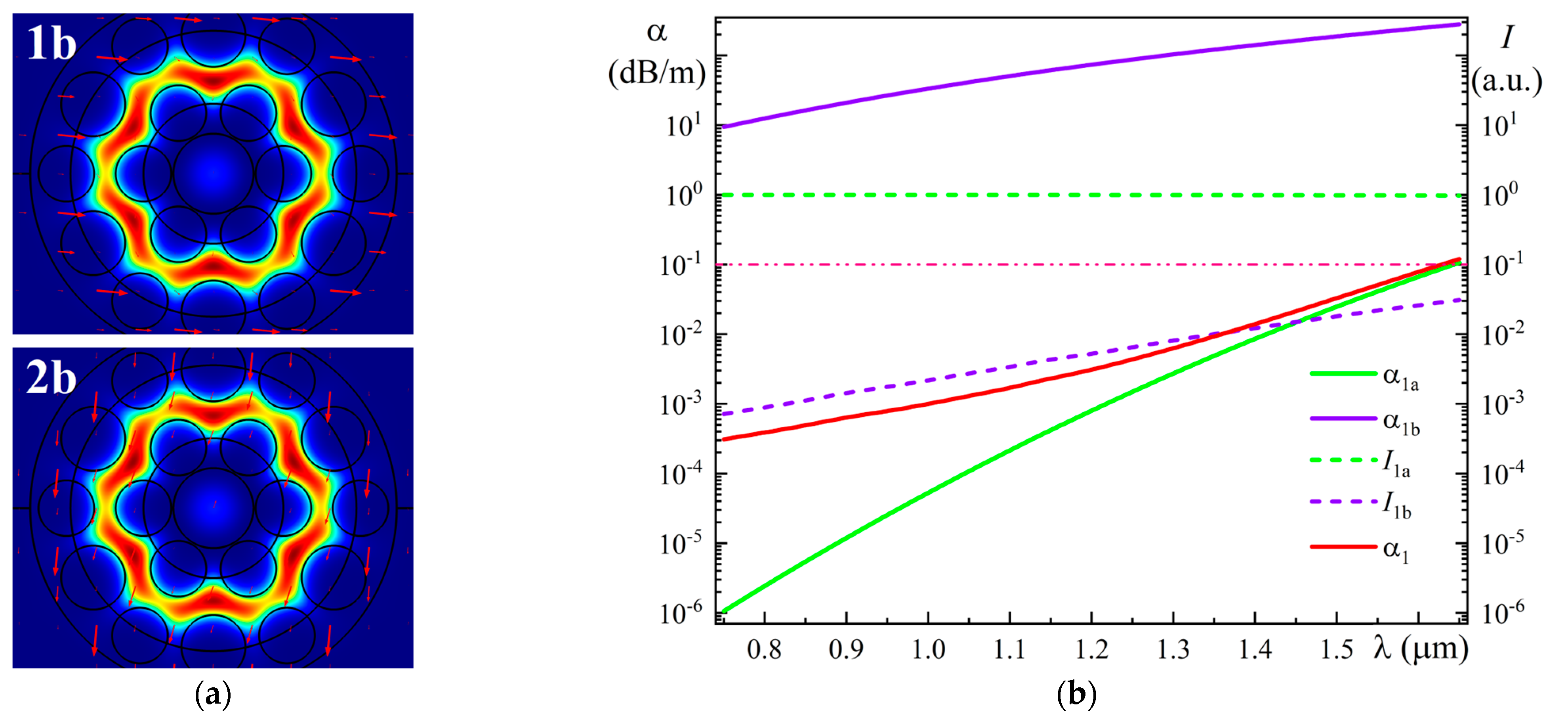
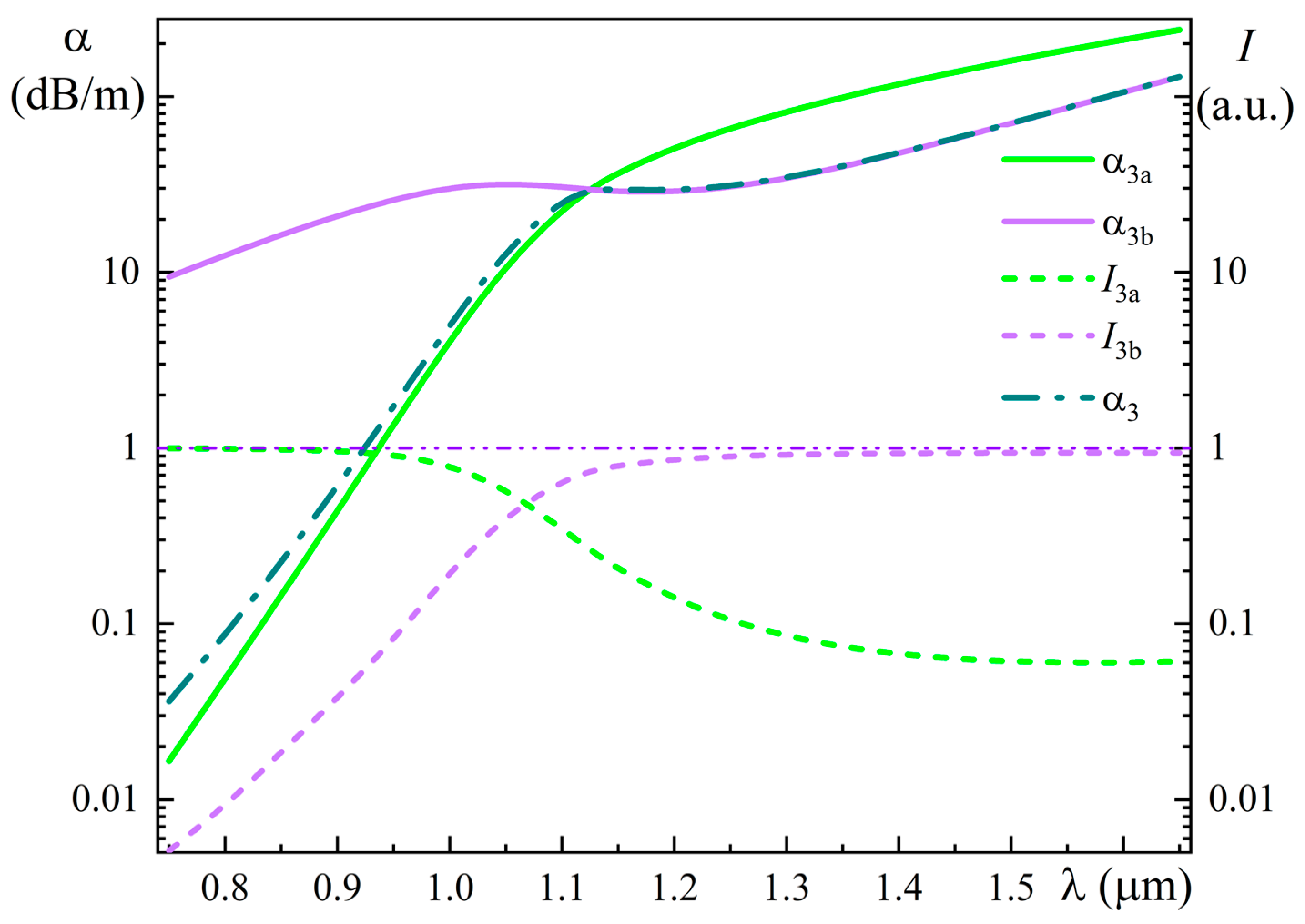
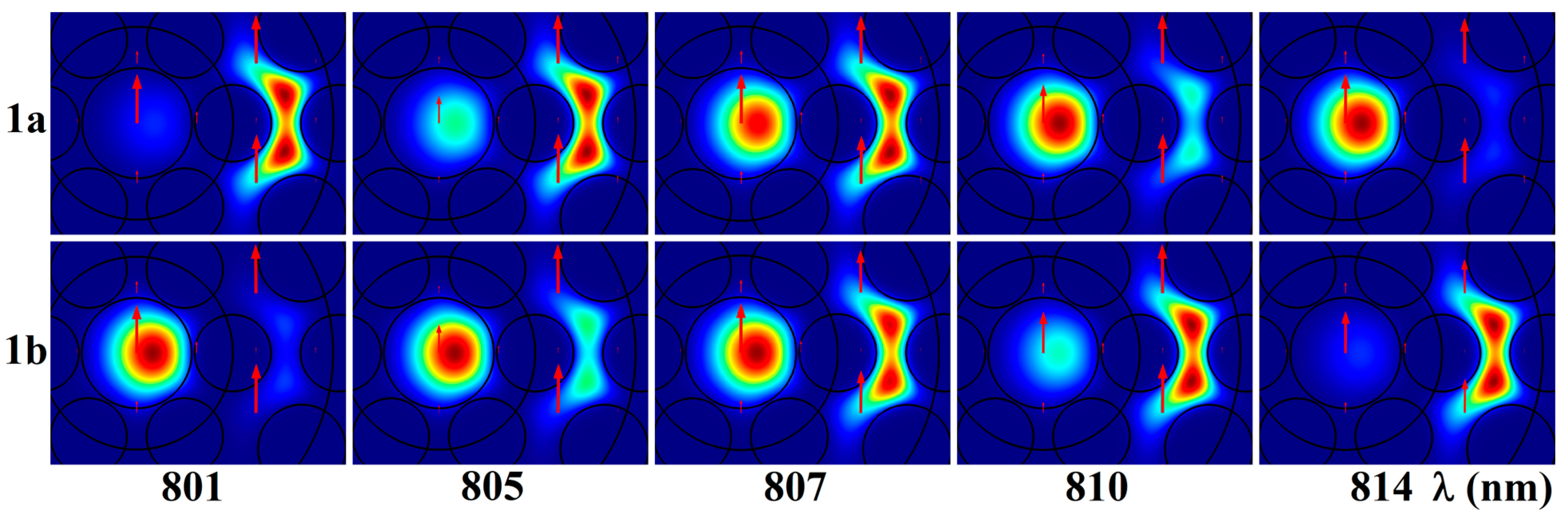
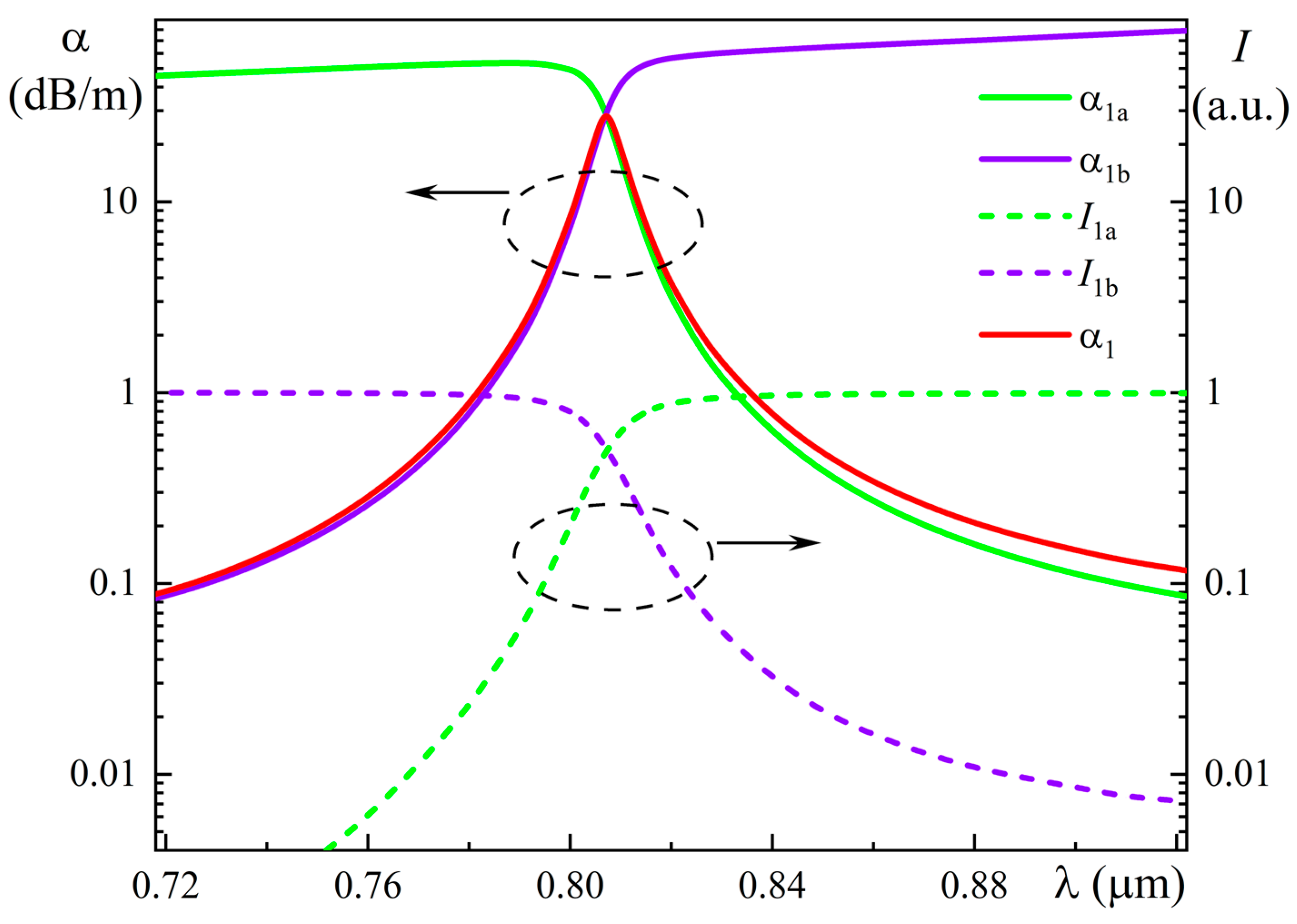
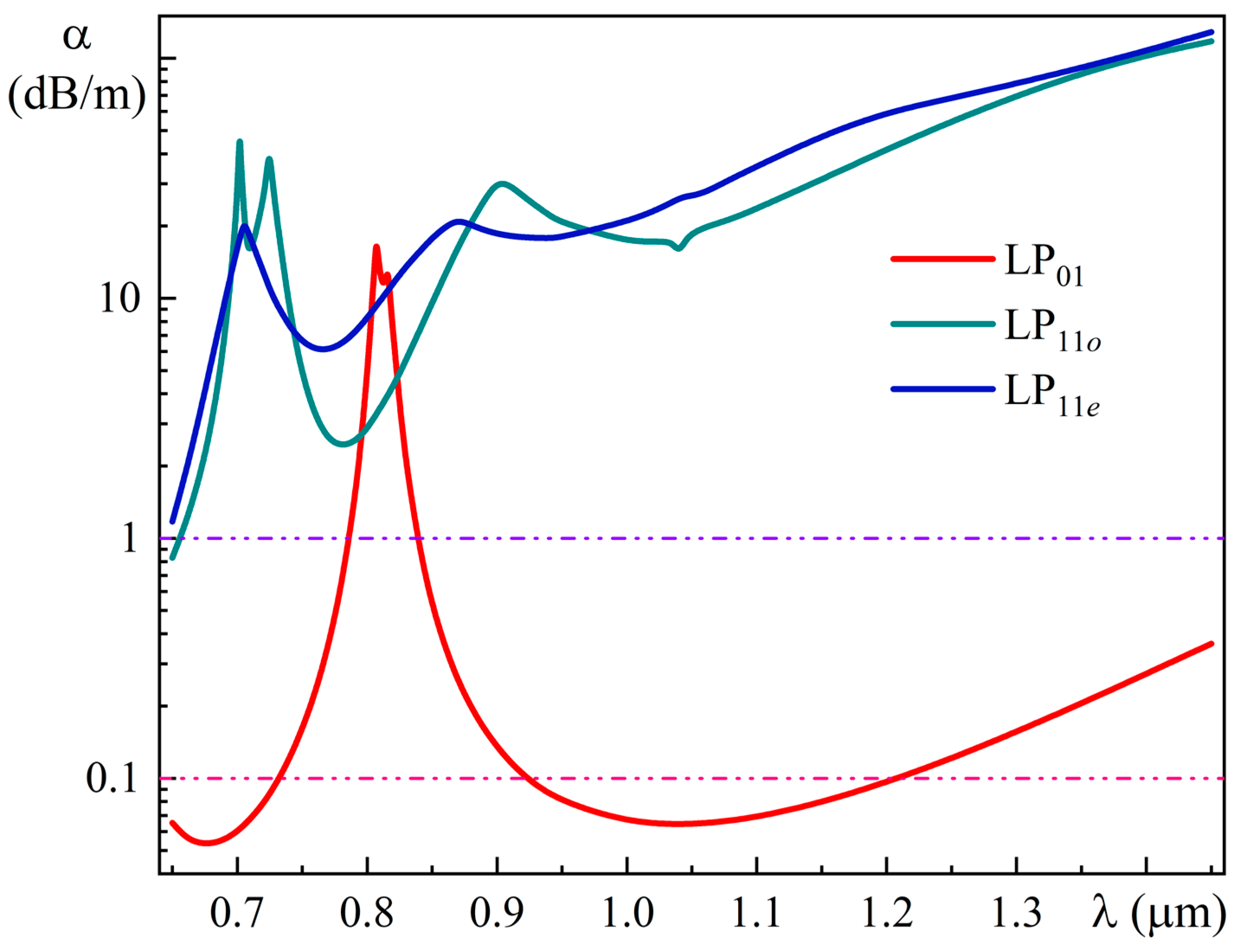
3.2. Bent MOF-18
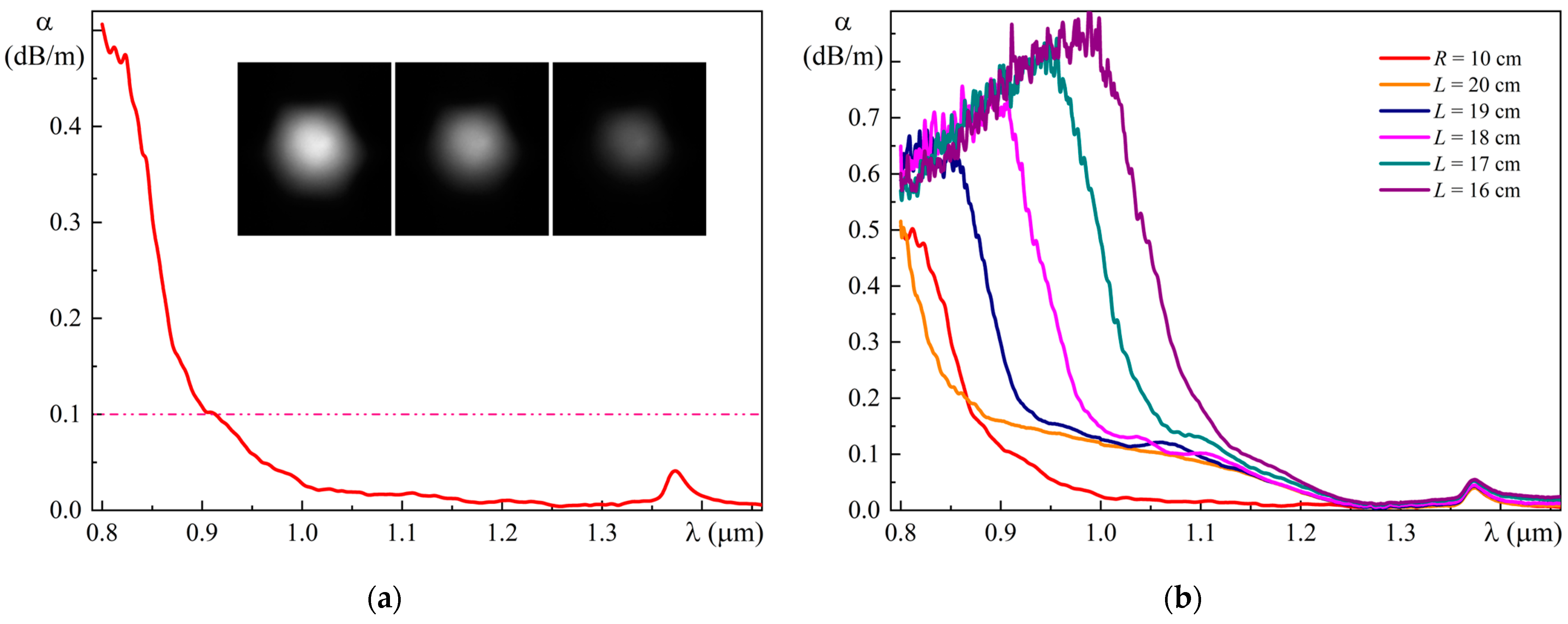
4. Experimental Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zervas, M.N.; Codemard, C.A. High Power Fiber Lasers: A Review. IEEE J. Sel. Top. Quantum Electron. 2014, 20, 219–241. [Google Scholar] [CrossRef]
- Yu, H.; Zhang, H.; Iv, H.; Wang, X.; Leng, J.; Xiao, H.; Guo, S.; Zhou, P.; Xu, X.; Chen, J. 3.15 kW direct diode-pumped near diffraction-limited all-fiber-integrated fiber laser. Appl. Opt. 2015, 54, 4556–4560. [Google Scholar] [CrossRef] [PubMed]
- Yagodkin, R.; Platonov, N.; Yusim, A.; Gapontsev, V.P. >1.5kW narrow linewidth CW diffraction-limited fiber amplifier with 40 nm bandwidth. Proc. SPIE 2016, 9728, 972807. [Google Scholar]
- Kong, F.; Gu, G.; Hawkins, T.W.; Jones, M.; Parsons, J.; Kalichevsky-Dong, M.T.; Pulford, B.; Dajani, I.; Dong, L. ~1 kilowatt Ytterbium-doped all-solid photonic bandgap fiber laser. Proc. SPIE 2017, 10083, 1008311. [Google Scholar]
- Ikoma, S.; Nguyen, H.K.; Kashiwagi, M.; Uchiyama, K.; Shima, K.; Tanaka, D. 3 kW single stage all-fiber Yb-doped single-mode fiber laser for highly reflective and highly thermal conductive materials processing. Proc. SPIE 2017, 10083, 100830Y. [Google Scholar]
- Jollivet, C.; Bradford, J.; Conroy, M.; Carter, A.; Tankala, K. Next Generation of DC fibers enabling high performance and reliability of industrial fiber lasers beyond 1.5kW levels. Proc. SPIE 2019, 11206, 1120605. [Google Scholar]
- Platonov, N.; Yagodkin, R.; Cruz, J.D.L.; Gapontsev, V. Single-mode 3kW Ytterbium fiber amplifier with diffraction-limited divergence in all-fiber format and compact modular package. Proc. SPIE 2021, 11665, 1166505. [Google Scholar]
- Nicholson, J.W.; DeSantolo, A.; Windeler, R.S.; Monberg, E.; Lukonin, V.; Pincha, J.; Hariharan, A.; Xu, X.; Williams, G.; Goldberg, Z.; et al. Next generation Yb-doped fibers for high-power, narrow-linewidth lasers. Proc. SPIE 2022, 11981, 119810R. [Google Scholar]
- Pulford, B.; Holten, R.; Matniyaz, T.; Kalichevsky-Dong, M.T.; Hawkins, T.W.; Dong, L. kW-level monolithic single-mode narrow-linewidth all-solid photonic bandgap fiber amplifier. Opt. Lett. 2021, 46, 4458–4461. [Google Scholar] [CrossRef]
- Wirth, C.; Schmidt, O.; Tsybin, I.; Schreiber, T.; Eberhardt, R.; Limpert, J.; Tünnermann, A.; Ludewigt, K.; Gowin, M.; Have, E.T.; et al. High average power spectral beam combining of four fiber amplifiers to 8.2 kW. Opt. Lett. 2011, 36, 3118–3120. [Google Scholar] [CrossRef]
- Fomin, V.; Gapontsev, V.; Shcherbakov, E.; Abramov, A.; Ferin, A.; Mochalov, D. 100 kW CW fiber laser for industrial applications. In Proceedings of the 2014 International Conference Laser Optics, Saint Petersburg, Russia, 30 June–4 July 2014; Volume 1. [Google Scholar] [CrossRef]
- Egorova, O.N.; Semjonov, S.L.; Kosolapov, A.F.; Denisov, A.N.; Pryamikov, A.D.; Gaponov, D.A.; Biriukov, A.S.; Dianov, E.M.; Salganskii, M.Y.; Khopin, V.F.; et al. Single-mode all-silica photonic bandgap fiber with 20-μm mode-field diameter. Opt. Express 2008, 16, 11735–11740. [Google Scholar] [CrossRef] [PubMed]
- Kashiwagi, M.; Saitoh, K.; Takenaga, K.; Tanigawa, S.; Matsuo, S.; Fujimaki, M. Effectively single-mode all-solid photonic bandgap fiber with large effective area and low bending loss for compact high-power all-fiber lasers. Opt. Express 2012, 20, 15061–15070. [Google Scholar] [CrossRef] [PubMed]
- Matniyaz, T.; Li, W.; Kalichevsky-Dong, M.; Hawkins, T.W.; Parsons, J.; Gu, G.; Dong, L. Highly efficient cladding-pumped single-mode three-level Yb all-solid photonic bandgap fiber lasers. Opt. Lett. 2019, 44, 807–810. [Google Scholar] [CrossRef] [PubMed]
- Février, S.; Jamier, R.; Blondy, J.-M.; Semjonov, S.L.; Likhachev, M.E.; Bubnov, M.M.; Dianov, E.M.; Khopin, V.F.; Salganskii, M.Y.; Guryanov, A.N. Low-loss singlemode large mode area all-silica photonic bandgap fiber. Opt. Express 2006, 14, 562–569. [Google Scholar] [CrossRef]
- Aleshkina, S.S.; Likhachev, M.E.; Pryamikov, A.D.; Gaponov, D.A.; Denisov, A.N.; Bubnov, M.M.; Salganskii, M.Y.; Laptev, A.Y.; Guryanov, A.N.; Uspenskii, Y.A.; et al. Very-large-mode-area photonic bandgap Bragg fiber polarizing in a wide spectral range. Opt. Lett. 2011, 36, 3566–3568. [Google Scholar] [CrossRef]
- Vanvincq, O.; Habert, R.; Cassez, A.; Baudelle, K.; Labat, D.; Delobel, S.; Quiquempois, Y.; Bouwmans, G.; Bigot, L. Polarization-maintaining and single-mode large mode area pixelated Bragg fiber. Opt. Lett. 2020, 45, 1946–1949. [Google Scholar] [CrossRef]
- Wong, W.S.; Peng, X.; McLaughlin, J.M.; Dong, L. Breaking the limit of maximum effective area for robust single-mode propagation in optical fibers. Opt. Lett. 2005, 30, 2855–2857. [Google Scholar] [CrossRef]
- Dong, L.; Peng, X.; Li, J. Leakage channel optical fibers with large effective area. J. Opt. Soc. Am. B 2007, 24, 1689–1697. [Google Scholar] [CrossRef]
- Gu, G.; Kong, F.; Hawkins, T.W.; Foy, P.; Wei, K.; Samson, B.; Dong, L. Impact of fiber outer boundaries on leaky mode losses in leakage channel fibers. Opt. Express 2013, 21, 24039–24048. [Google Scholar] [CrossRef]
- Saitoh, K.; Tsuchida, Y.; Rosa, L.; Koshiba, M.; Poli, F.; Cucinotta, A.; Selleri, S.; Pal, M.; Paul, M.; Ghosh, D.; et al. Design of all-solid leakage channel fibers with large mode area and low bending loss. Opt. Express 2009, 17, 4913–4919. [Google Scholar] [CrossRef]
- Dong, L.; Mckay, H.A.; Marcinkevicius, A.; Fu, L.; Li, J.; Thomas, B.K.; Fermann, M.E. Extending Effective Area of Fundamental Mode in Optical Fibers. J. Light. Technol. 2009, 27, 1565–1570. [Google Scholar] [CrossRef]
- Dong, L.; Li, J.; Mckay, H.A.; Fu, L.; Thomas, B.K. Large effective mode area optical fibers for high-power lasers. Proc. SPIE 2009, 7195, 71950N. [Google Scholar]
- Dong, L.; Wu, T.; Mckay, H.A.; Fu, L.; Li, J.; Winful, H.G. All-glass large-core leakage channel fibers. IEEE J. Sel. Top. Quantum Electron. 2009, 15, 47–53. [Google Scholar] [CrossRef]
- Denisov, A.N.; Semjonov, S.L. All-Glass Single-Mode Microstructured Fibers with a Large Mode Area. Dokl. Phys. 2021, 66, 64–66. [Google Scholar] [CrossRef]
- Denisov, A.N.; Semjonov, S.L. All-glass single-mode microstructured optical fibres with a large-diameter core and low bending losses. Quantum Electron. 2021, 51, 1081–1089. [Google Scholar] [CrossRef]
- Rashidi, K.; Mirjalili, S.M.; Taleb, H.; Fathi, D. Optimal Design of Large Mode Area Photonic Crystal Fibers Using a Multiobjective Gray Wolf Optimization Technique. J. Light. Technol. 2018, 36, 5626–5632. [Google Scholar] [CrossRef]
- Agraval, G. Nonlinear Fiber Optics, 4th ed.; Academic Press: New York, NY, USA, 2007. [Google Scholar]
- Guobin, R.; Zhi, W.; Shuqin, L.; Shuisheng, J. Mode classification and degeneracy in photonic crystal fibers. Opt. Express 2003, 11, 1310–1321. [Google Scholar] [CrossRef]
- Kuhlmey, B.T.; McPhedran, R.C.; de Sterke, C.M. Modal cutoff in microstructured optical fibers. Opt. Lett. 2002, 27, 1684–1686. [Google Scholar] [CrossRef]
- Tsuchida, Y.; Saitoh, K.; Koshiba, M. Design and characterization of single-mode holey fibers with low bending losses. Opt. Express 2005, 13, 4770–4779. [Google Scholar] [CrossRef]
- Schermer, R.T. Mode scalability in bent optical fibers. Opt. Express 2007, 15, 15674–15701. [Google Scholar] [CrossRef]
- Haakestad, M.W.; Skaar, J. Causality and Kramers-Kronig relations for waveguides. Opt. Express 2005, 13, 9922–9934. [Google Scholar] [CrossRef] [PubMed]
- Fini, J.M. Design of solid and microstructure fibers for suppression of higher-order modes. Opt. Express 2005, 13, 3477–3490. [Google Scholar] [CrossRef] [PubMed]
- Saitoh, K.; Florous, N.J.; Murao, T.; Koshiba, M. Design of photonic band gap fibers with suppressed higher-order modes: Towards the development of effectively single mode large hollow-core fiber platforms. Opt. Express 2006, 14, 7342–7352. [Google Scholar] [CrossRef] [PubMed]
- Tsuchida, Y.; Saitoh, K.; Koshiba, M. Design of single-moded holey fibers with large-mode-area and low bending losses: The significance of the ring-core region. Opt. Express 2007, 15, 1794–1803. [Google Scholar] [CrossRef] [PubMed]
- Denisov, A.N.; Semjonov, S.L. Microstructured optical fibres with a wide single-mode range. Quantum Electron. 2021, 51, 240–247. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Denisov, A.; Dvoyrin, V.; Kosolapov, A.; Likhachev, M.; Velmiskin, V.; Zhuravlev, S.; Semjonov, S. All-Glass Single-Mode Leakage Channel Microstructured Optical Fibers with Large Mode Area and Low Bending Loss. Photonics 2023, 10, 465. https://doi.org/10.3390/photonics10040465
Denisov A, Dvoyrin V, Kosolapov A, Likhachev M, Velmiskin V, Zhuravlev S, Semjonov S. All-Glass Single-Mode Leakage Channel Microstructured Optical Fibers with Large Mode Area and Low Bending Loss. Photonics. 2023; 10(4):465. https://doi.org/10.3390/photonics10040465
Chicago/Turabian StyleDenisov, Alexander, Vladislav Dvoyrin, Alexey Kosolapov, Mikhail Likhachev, Vladimir Velmiskin, Sergey Zhuravlev, and Sergey Semjonov. 2023. "All-Glass Single-Mode Leakage Channel Microstructured Optical Fibers with Large Mode Area and Low Bending Loss" Photonics 10, no. 4: 465. https://doi.org/10.3390/photonics10040465
APA StyleDenisov, A., Dvoyrin, V., Kosolapov, A., Likhachev, M., Velmiskin, V., Zhuravlev, S., & Semjonov, S. (2023). All-Glass Single-Mode Leakage Channel Microstructured Optical Fibers with Large Mode Area and Low Bending Loss. Photonics, 10(4), 465. https://doi.org/10.3390/photonics10040465




