Wideband Airy Beam Generation Using Reflective Metasurfaces with Both Phase and Amplitude Modulation
Abstract
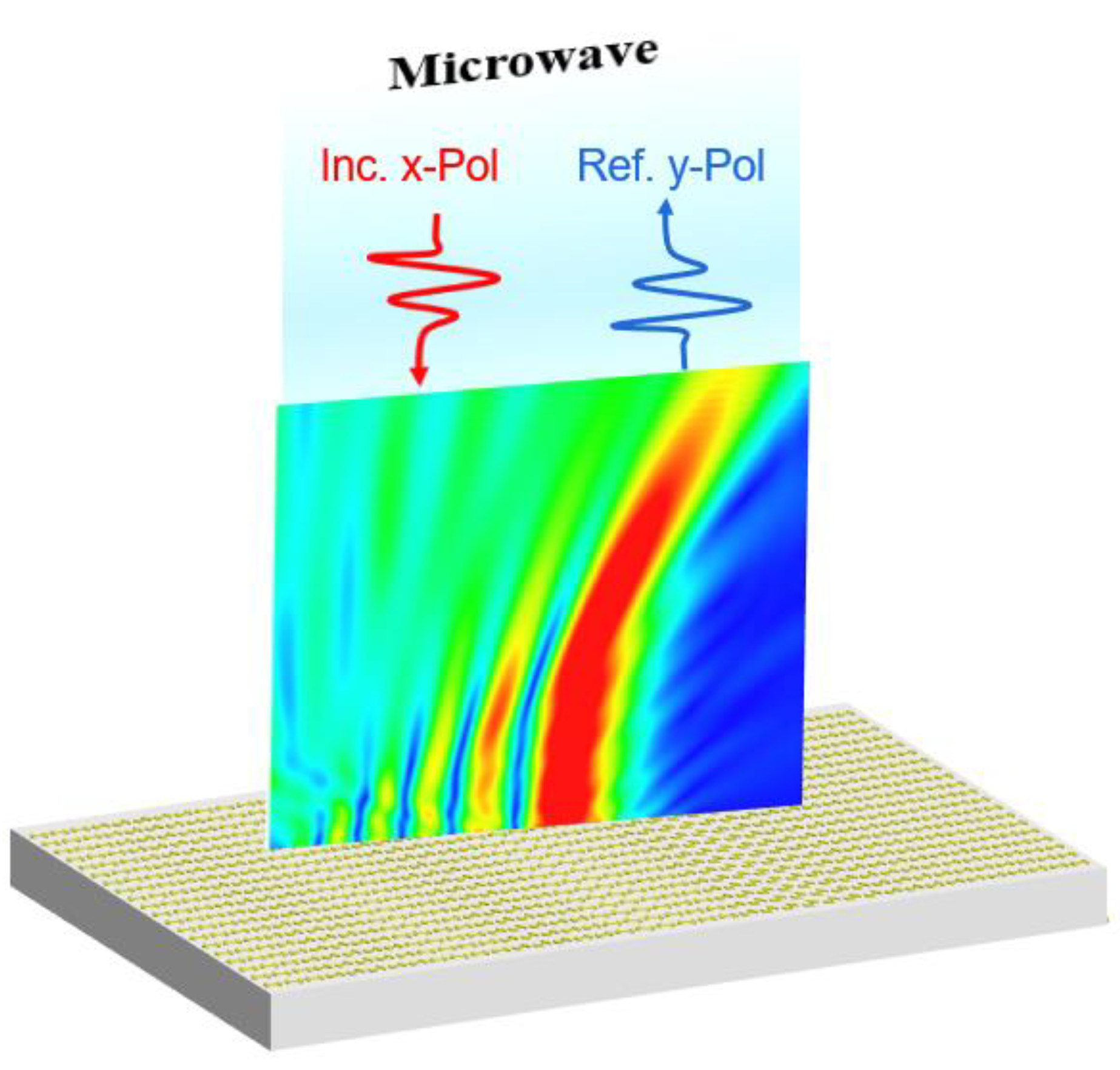
1. Introduction
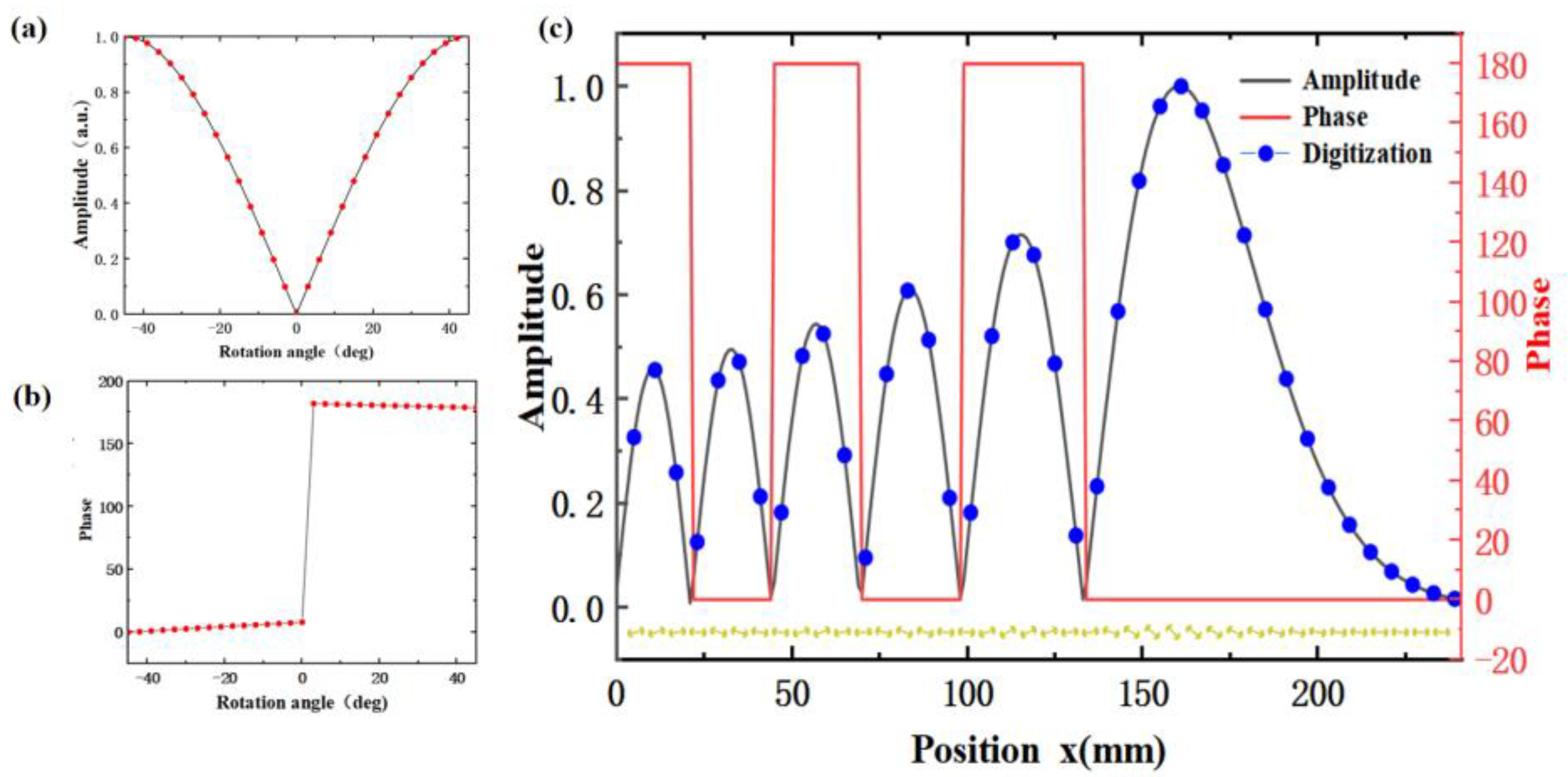
2. Materials and Methods
3. Results
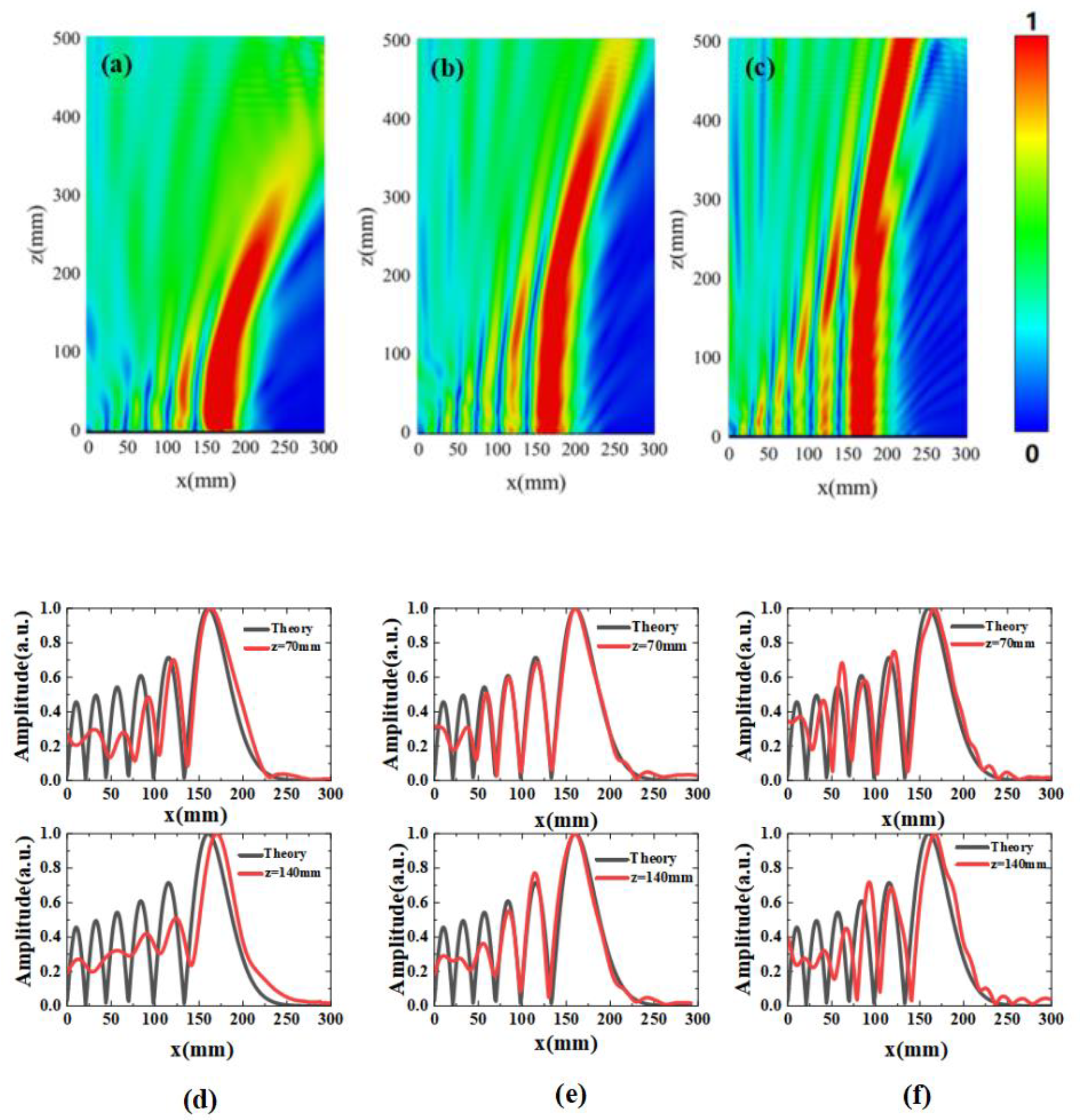
3.1. Spectrum
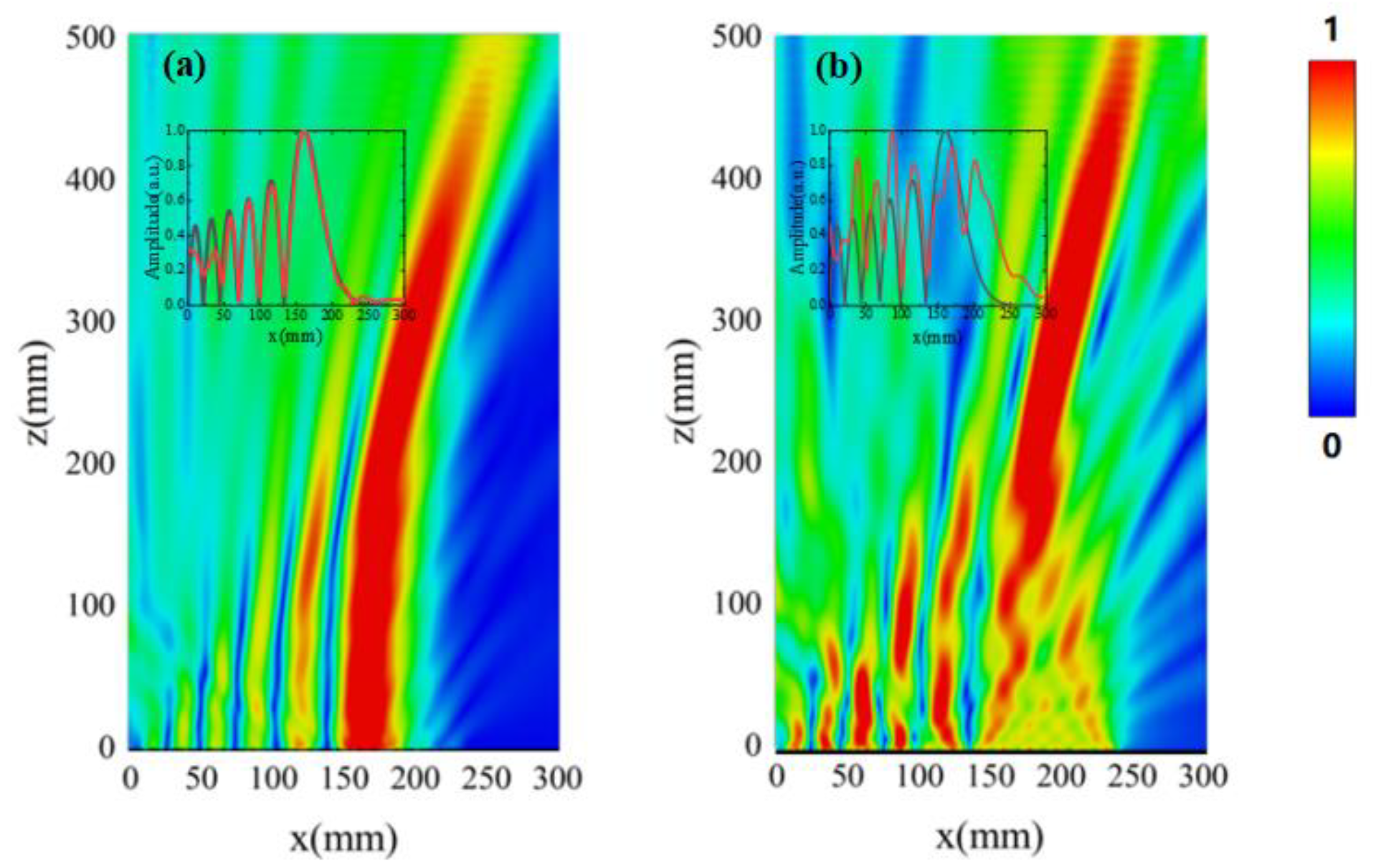
3.2. Properties
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Durnin, J.; Miceli, J., Jr.; Eberly, J.H. Diffraction-Free Beams. Appl. Phys. Lett. 1987, 58, 1499. [Google Scholar] [CrossRef]
- Durnin, J. Exact Solutions for Nondiffracting Beams. I. The Scalar Theory. J. Opt. Soc. Am. A 1987, 4, 651–654. [Google Scholar] [CrossRef]
- López-Mariscal, C.; Helmerson, K. Shaped Nondiffracting Beams. Opt. Lett. 2010, 35, 1215–1217. [Google Scholar] [CrossRef] [PubMed]
- Siviloglou, G.A.; Christodoulides, D.N. Accelerating Finite Energy Airy Beams. Opt. Lett. 2007, 32, 979–981. [Google Scholar] [CrossRef]
- Siviloglou, G.; Broky, J.; Dogariu, A.; Christodoulides, D. Observation of Accelerating Airy Beams. Appl. Phys. Lett. 2007, 99, 213901. [Google Scholar] [CrossRef] [PubMed]
- Broky, J.; Siviloglou, G.A.; Dogariu, A.; Christodoulides, D.N. Self-Healing Properties of Optical Airy Beams. Opt. Express. 2008, 16, 12880–12891. [Google Scholar] [CrossRef] [PubMed]
- Minovich, A.; Klein, A.E.; Janunts, N.; Pertsch, T.; Neshev, D.N.; Kivshar, Y.S. Generation and near-Field Imaging of Airy Surface Plasmons. Appl. Phys. Lett. 2011, 107, 116802. [Google Scholar] [CrossRef]
- Zhao, Z.; Zang, W.; Tian, J. Optical Trapping and Manipulation of Mie Particles with Airy Beam. J. Opt. 2016, 18, 025607. [Google Scholar] [CrossRef]
- Singh, B.K.; Nagar, H.; Roichman, Y.; Arie, A. Particle Manipulation Beyond the Diffraction Limit Using Structured Super-Oscillating Light Beams. Light Sci. Appl. 2017, 6, e17050. [Google Scholar] [CrossRef]
- Vettenburg, T.; Dalgarno, H.I.; Nylk, J.; Coll-Lladó, C.; Ferrier, D.E.; Čižmár, T.; Gunn-Moore, F.J.; Dholakia, K. Light-Sheet Microscopy Using an Airy Beam. Nat. Methods 2014, 11, 541–544. [Google Scholar] [CrossRef]
- He, H.; Kong, C.; Tan, X.-J.; Chan, K.Y.; Ren, Y.-X.; Tsia, K.K.; Wong, K.K. Depth-Resolved Volumetric Two-Photon Microscopy Based on Dual Airy Beam Scanning. Opt. Lett. 2019, 44, 5238–5241. [Google Scholar] [CrossRef] [PubMed]
- Tan, X.-J.; Kong, C.; Ren, Y.-X.; Lai, C.S.; Tsia, K.K.; Wong, K.K. Volumetric Two-Photon Microscopy with a Non-Diffracting Airy Beam. Opt. Lett. 2019, 44, 391–394. [Google Scholar] [CrossRef] [PubMed]
- Abdollahpour, D.; Suntsov, S.; Papazoglou, D.G.; Tzortzakis, S. Spatiotemporal Airy Light Bullets in the Linear and Nonlinear Regimes. Appl. Phys. Lett. 2010, 105, 253901. [Google Scholar] [CrossRef]
- Chong, A.; Renninger, W.H.; Christodoulides, D.N.; Wise, F.W. Airy–Bessel Wave Packets as Versatile Linear Light Bullets. Nat. Photonics 2010, 4, 103–106. [Google Scholar] [CrossRef]
- Siviloglou, G.; Broky, J.; Dogariu, A.; Christodoulides, D. Ballistic Dynamics of Airy Beams. Opt. Lett. 2008, 33, 207–209. [Google Scholar] [CrossRef]
- Dai, H.; Sun, X.; Luo, D.; Liu, Y. Airy Beams Generated by a Binary Phase Element Made of Polymer-Dispersed Liquid Crystals. Opt. Express 2009, 17, 19365–19370. [Google Scholar] [CrossRef]
- Panagiotopoulos, P.; Papazoglou, D.; Couairon, A.; Tzortzakis, S. Sharply Autofocused Ring-Airy Beams Transforming into Non-Linear Intense Light Bullets. Nat. Commun. 2013, 4, 2622. [Google Scholar] [CrossRef]
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.-P.; Capasso, F.; Gaburro, Z. Light Propagation with Phase Discontinuities, Generalized Laws of Reflection and Refraction. Science 2011, 334, 333–337. [Google Scholar] [CrossRef]
- Holloway, C.L.; Kuester, E.F.; Gordon, J.A.; O’Hara, J.; Booth, J.; Smith, D.R. An Overview of the Theory and Applications of Metasurfaces: The Two-Dimensional Equivalents of Metamaterials. IEEE Trans. Antennas Propag. 2012, 54, 10–35. [Google Scholar] [CrossRef]
- Zhao, Y.; Alù, A. Manipulating Light Polarization with Ultrathin Plasmonic Metasurfaces. Phys. Rev. B 2011, 84, 205428. [Google Scholar] [CrossRef]
- Ding, G.; Chen, K.; Luo, X.; Zhao, J.; Jiang, T.; Feng, Y. Dual-Helicity Decoupled Coding Metasurface for Independent Spin-to-Orbital Angular Momentum Conversion. Adv. Mater. Technol. 2019, 11, 044043. [Google Scholar] [CrossRef]
- Guo, W.-L.; Wang, G.-M.; Chen, K.; Li, H.-P.; Zhuang, Y.-Q.; Xu, H.-X.; Feng, Y. Broadband Polarization-Conversion Metasurface for a Cassegrain Antenna with High Polarization Purity. Phys. Rev. Appl. 2019, 12, 014009. [Google Scholar] [CrossRef]
- Aieta, F.; Kats, M.A.; Genevet, P.; Capasso, F. Multiwavelength Achromatic Metasurfaces by Dispersive Phase Compensation. Science 2015, 347, 1342–1345. [Google Scholar] [CrossRef] [PubMed]
- Chen, W.T.; Zhu, A.Y.; Sisler, J.; Huang, Y.-W.; Yousef, K.M.; Lee, E.; Qiu, C.-W.; Capasso, F. Broadband Achromatic Metasurface-Refractive Optics. Nano Lett. 2018, 18, 7801–7808. [Google Scholar] [CrossRef]
- Guo, W.-L.; Wang, G.-M.; Hou, H.-S.; Chen, K.; Feng, Y. Multi-Functional Coding Metasurface for Dual-Band Independent Electromagnetic Wave Control. Opt. Express 2019, 27, 19196–19211. [Google Scholar] [CrossRef]
- Chen, K.; Zhang, N.; Ding, G.; Zhao, J.; Jiang, T.; Feng, Y. Active Anisotropic Coding Metasurface with Independent Real-Time Reconfigurability for Dual Polarized Waves. Adv. Mater. Technol. 2020, 5, 1900930. [Google Scholar] [CrossRef]
- Wang, C.; Yang, Y.; Liu, Q.; Liang, D.; Zheng, B.; Chen, H.; Xu, Z.; Wang, H. Multi-Frequency Metasurface Carpet Cloaks. Opt. Express 2018, 26, 14123–14131. [Google Scholar] [CrossRef]
- Li, Z.; Yao, K.; Xia, F.; Shen, S.; Tian, J.; Liu, Y. Graphene Plasmonic Metasurfaces to Steer Infrared Light. Nature 2015, 5, 12423. [Google Scholar] [CrossRef]
- Li, Z.; Cheng, H.; Liu, Z.; Chen, S.; Tian, J. Plasmonic Airy Beam Generation by Both Phase and Amplitude Modulation with Metasurfaces. Adv. Opt. Mater. 2016, 4, 1230–1235. [Google Scholar] [CrossRef]
- Song, E.Y.; Lee, G.Y.; Park, H.; Lee, K.; Kim, J.; Hong, J.; Kim, H.; Lee, B. Compact Generation of Airy Beams with C-Aperture Metasurface. Adv. Opt. Mater. 2017, 5, 1601028. [Google Scholar] [CrossRef]
- Guo, W.L.; Chen, K.; Wang, G.M.; Luo, X.Y.; Cai, T.; Zhang, C.B.; Feng, Y.J.A.M.T. Airy Beam Generation, Approaching Ideal Efficiency and Ultra Wideband with Reflective and Transmissive Metasurfaces. Adv. Opt. Mater. 2020, 8, 2000860. [Google Scholar] [CrossRef]
- Fan, Q.; Zhu, W.; Liang, Y.; Huo, P.; Zhang, C.; Agrawal, A.; Huang, K.; Luo, X.; Lu, Y.; Qiu, C. Broadband Generation of Photonic Spin-Controlled Arbitrary Accelerating Light Beams in the Visible. Nano Lett. 2018, 19, 1158–1165. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Lang, T.; Qiu, Y. Wideband Airy Beam Generation Using Reflective Metasurfaces with Both Phase and Amplitude Modulation. Photonics 2023, 10, 426. https://doi.org/10.3390/photonics10040426
Wang Z, Lang T, Qiu Y. Wideband Airy Beam Generation Using Reflective Metasurfaces with Both Phase and Amplitude Modulation. Photonics. 2023; 10(4):426. https://doi.org/10.3390/photonics10040426
Chicago/Turabian StyleWang, Zhenai, Tingting Lang, and Yanqing Qiu. 2023. "Wideband Airy Beam Generation Using Reflective Metasurfaces with Both Phase and Amplitude Modulation" Photonics 10, no. 4: 426. https://doi.org/10.3390/photonics10040426
APA StyleWang, Z., Lang, T., & Qiu, Y. (2023). Wideband Airy Beam Generation Using Reflective Metasurfaces with Both Phase and Amplitude Modulation. Photonics, 10(4), 426. https://doi.org/10.3390/photonics10040426




