Changes in Wavefront Error of the Eye for Different Accommodation Targets under the Application of Phenylephrine Hydrochloride
Abstract
1. Introduction
2. Materials and Methods
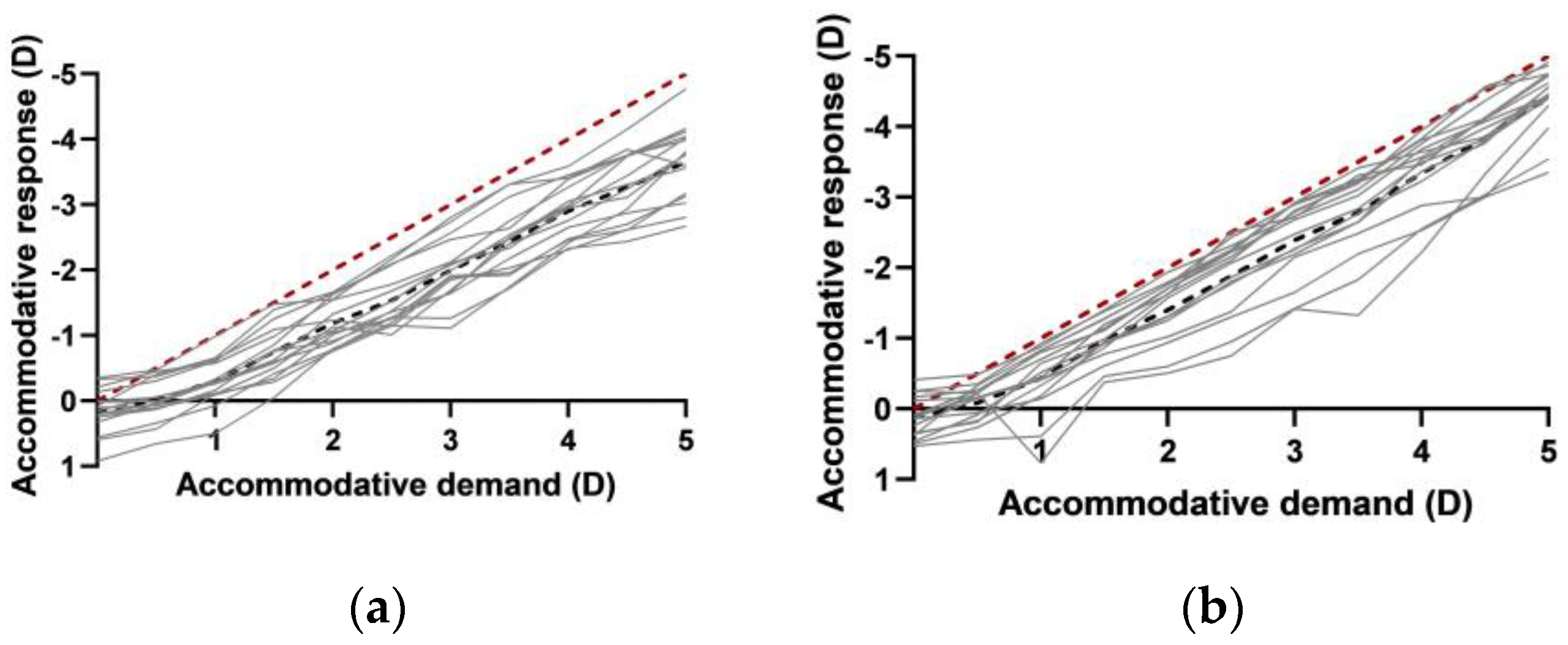
3. Results
3.1. Pupil Size
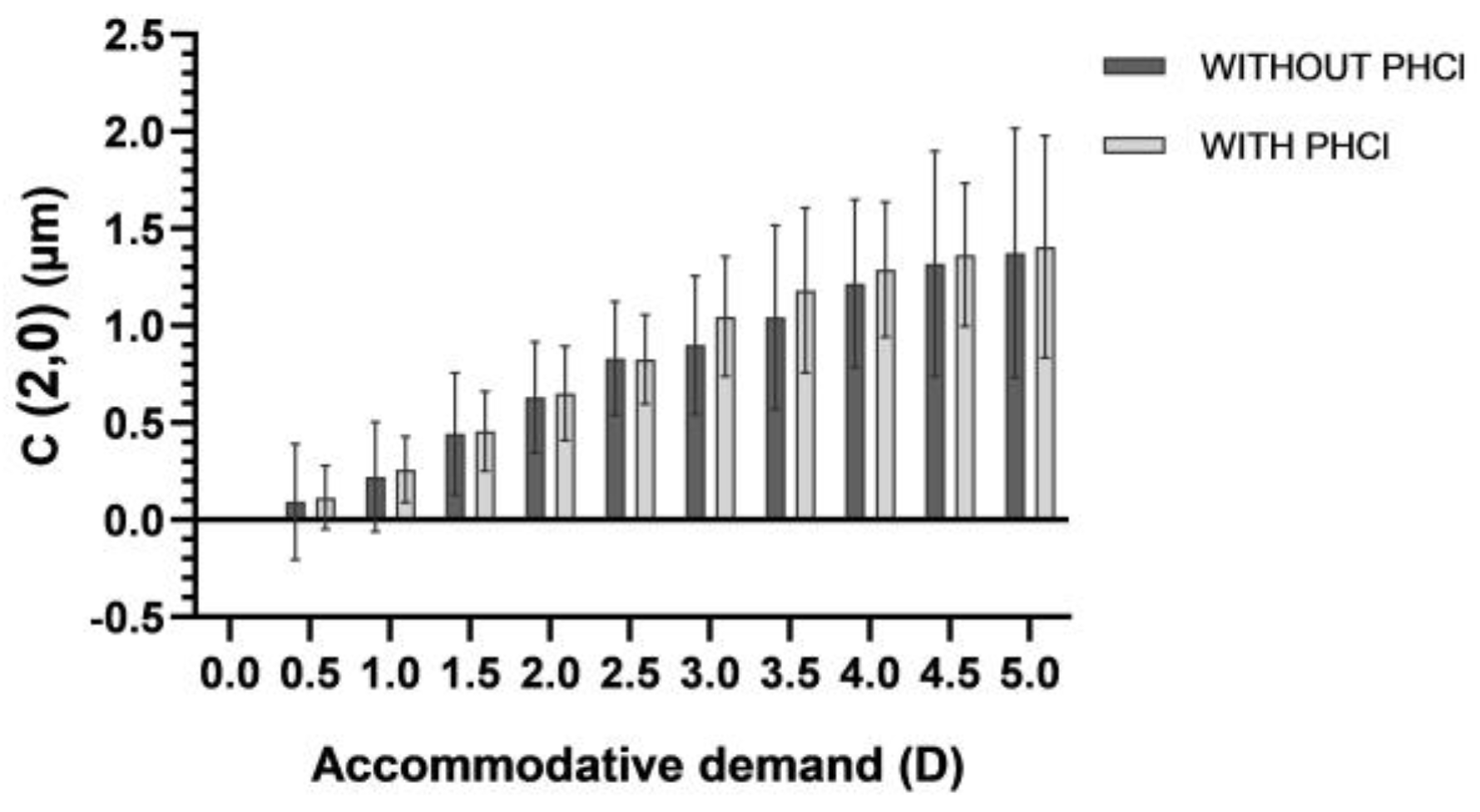
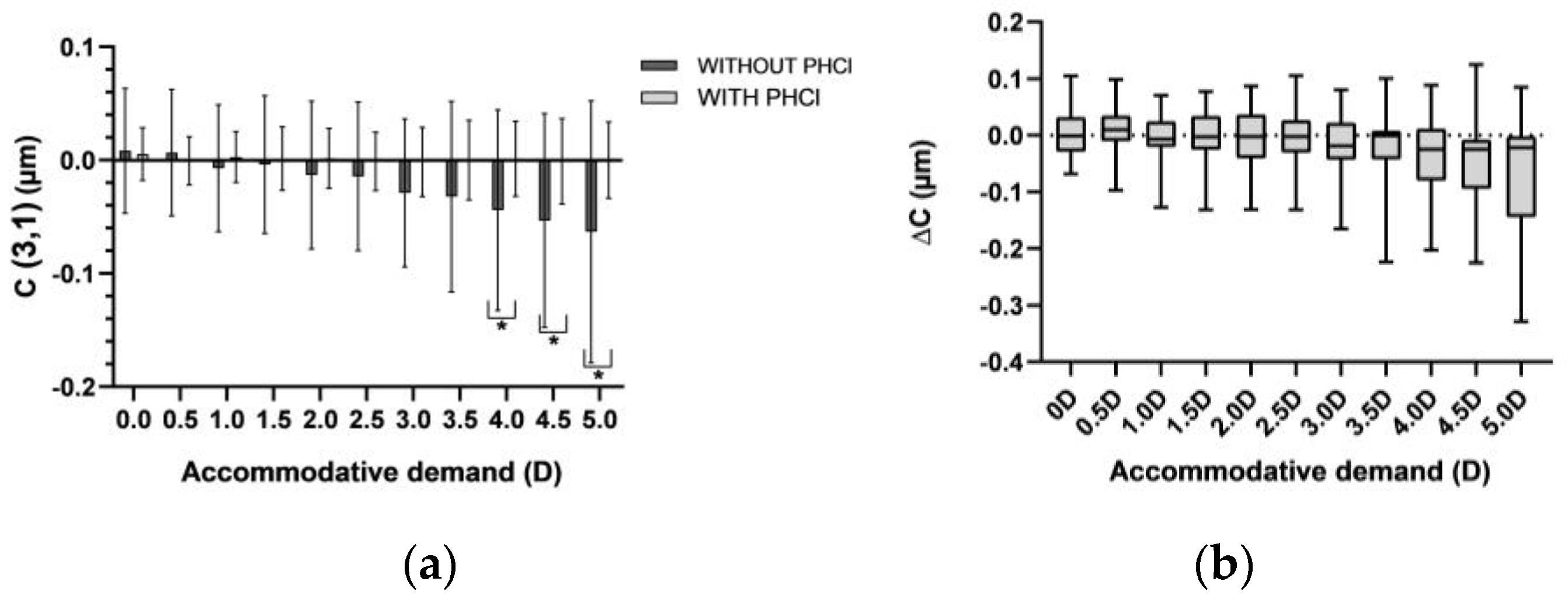
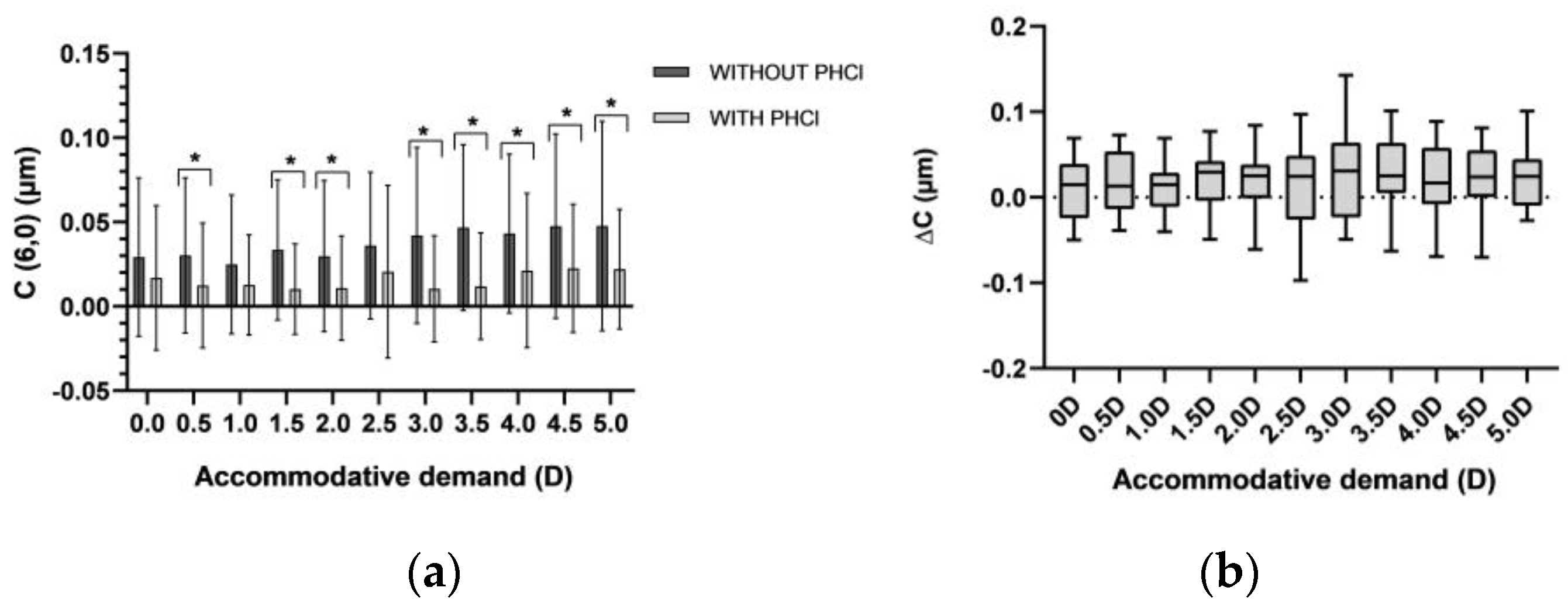
3.2. Zernike Coefficients
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fernández, J.; Rodríguez-Vallejo, M.; Burguera, N.; Rocha-de-Lossada, C.; Piñero, D.P. Spherical aberration for expanding depth of focus. J. Cataract. Refract. Surg. 2021, 47, 1587–1595. [Google Scholar] [CrossRef] [PubMed]
- Francisco Castejón-Mochón, J.; López-Gil, N.; Benito, A.; Artal, P. Ocular wave-front aberration statistics in a normal young population. Vision Res. 2002, 42, 1611–1617. [Google Scholar] [CrossRef] [PubMed]
- Porter, J.; Guirao, A.; Cox, I.G.; Williams, D.R. Monochromatic aberrations of the human eye in a large population. JOSA A 2001, 18, 1793–1803. [Google Scholar] [CrossRef]
- Thibos, L.N.; Hong, X.; Bradley, A.; Cheng, X. Statistical variation of aberration structure and image quality in a normal population of healthy eyes. JOSA A 2002, 19, 2329–2348. [Google Scholar] [CrossRef]
- Koretz, J.F.; Cook, C.A.; Kaufman, P.L. Aging of the human lens: Changes in lens shape upon accommodation and with accommodative loss. J. Opt. Soc. Am. A Opt. Image Sci. Vis. 2002, 19, 144–151. [Google Scholar] [CrossRef]
- Khan, A.; Pope, J.M.; Verkicharla, P.K.; Suheimat, M.; Atchison, D.A. Change in human lens dimensions, lens refractive index distribution and ciliary body ring diameter with accommodation. Biomed. Opt. Express 2018, 9, 1272–1282. [Google Scholar] [CrossRef]
- Charman, W.N. The eye in focus: Accommodation and presbyopia. Clin. Exp. Optom. 2008, 91, 207–225. [Google Scholar] [CrossRef]
- Richdale, K.; Bailey, M.D.; Sinnott, L.T.; Kao, C.Y.; Zadnik, K.; Bullimore, M.A. The Effect of Phenylephrine on the Ciliary Muscle and Accommodation. Optom. Vis. Sci. Off. Publ. Am. Acad. Optom. 2012, 89, 1507–1511. [Google Scholar] [CrossRef]
- Biggs, R.D.; Alpern, M.; Bennett, D.R. The effect of sympathomimetic drugs upon the amplitude of accommodation. Am. J. Ophthalmol. 1959, 48 Pt 2, 169–172. [Google Scholar] [CrossRef]
- J Mordi, J.; Tucker, J.; Charman, W.N. Effects of 0.1% cyclopentolate or 10% phenylephrine on pupil diameter and accommodation. Ophthalmic. Physiol. Opt. 1986, 6, 221–227. [Google Scholar] [CrossRef]
- Zetterström, C. The effect of phenylephrine on the accommodative process in man. Acta Ophthalmol. (Copenh.) 1984, 62, 872–878. [Google Scholar] [CrossRef]
- Gimpel, G.; Doughty, M.J.; Lyle, W.M. Large sample study of the effects of phenylephrine 2.5% eyedrops on the amplitude of accommodation in man. Ophthalmic Physiol. Opt. 1994, 14, 123–128. [Google Scholar] [CrossRef] [PubMed]
- Do, T.; Kasthurirangan, S.; Ostrin, L.; Glasser, A. The effects of phenylephrine on accommodation in humans. Optom. Vis. Sci. 2002, 79, 174. [Google Scholar] [CrossRef]
- Leibowitz, H.W.; Owens, D.A. Night myopia and the intermediate dark focus of accommodation. J. Opt. Soc. Am. 1975, 65, 1121–1128. [Google Scholar] [CrossRef]
- Eyeson-Annan, M.L.; Hirst, L.W.; Battistutta, D.; Green, A. Comparative pupil dilation using phenylephrine alone or in combination with tropicamide. Ophthalmology 1998, 105, 726–732. [Google Scholar] [CrossRef]
- Ostrin, L.A.; Glasser, A. The effects of phenylephrine on pupil diameter and accommodation in rhesus monkeys. Investig. Ophthalmol. Vis. Sci. 2004, 45, 215–221. [Google Scholar] [CrossRef] [PubMed]
- Del Águila-Carrasco, A.J.; Kruger, P.B.; Lara, F.; López-Gil, N. Aberrations and accommodation. Clin. Exp. Optom. 2020, 103, 95–103. [Google Scholar] [CrossRef]
- Liang, J.; Grimm, B.; Goelz, S.; Bille, J.F. Objective measurement of wave aberrations of the human eye with the use of a Hartmann-Shack wave-front sensor. J. Opt. Soc. Am. A Opt. Image Sci. Vis. 1994, 11, 1949–1957. [Google Scholar] [CrossRef]
- López-Gil, N.; Fernández-Sánchez, V.; Legras, R.; Montés-Micó, R.; Lara, F.; Nguyen-Khoa, J.L. Accommodation-related changes in monochromatic aberrations of the human eye as a function of age. Investig. Ophthalmol. Vis. Sci. 2008, 49, 1736–1743. [Google Scholar] [CrossRef]
- Montés-Micó, R.; Alió, J.L.; Muñoz, G.; Pérez-Santonja, J.J.; Charman, W.N. Postblink changes in total and corneal ocular aberrations. Ophthalmology 2004, 111, 758–767. [Google Scholar] [CrossRef]
- Montés-Micó, R.; Alió, J.L.; Charman, W.N. Postblink changes in the ocular modulation transfer function measured by a double-pass method. Investig. Ophthalmol. Vis. Sci. 2005, 46, 4468–4473. [Google Scholar] [CrossRef] [PubMed]
- Thibos, L.N.; Applegate, R.A.; Schwiegerling, J.T.; Webb, R. VSIA Standards Taskforce Members. Vision science and its applications. Standards for reporting the optical aberrations of eyes. J. Refract. Surg. 2002, 18, S652–S660. [Google Scholar] [CrossRef] [PubMed]
- Rozema, J.J.; Van Dyck, D.E.; Tassignon, M.J. Clinical comparison of 6 aberrometers. Part 1: Technical specifications. J. Cataract. Refract. Surg. 2005, 31, 1114–1127. [Google Scholar] [CrossRef]
- Mello, G.R.; Rocha, K.M.; Santhiago, M.R.; Smadja, D.; Krueger, R.R. Applications of wavefront technology. J. Cataract. Refract. Surg. 2012, 38, 1671–1683. [Google Scholar] [CrossRef] [PubMed]
- Thibos, L.N. Wavefront Data Reporting and Terminology. J. Refract. Surg. 2001, 17, S578–S583. [Google Scholar] [CrossRef]
- He, J.C.; Burns, S.A.; Marcos, S. Monochromatic aberrations in the accommodated human eye. Vis. Res. 2000, 40, 41–48. [Google Scholar] [CrossRef]
- Ninomiya, S.; Fujikado, T.; Kuroda, T.; Maeda, N.; Tano, Y.; Oshika, T.; Hirohara, Y.; Mihashi, T. Changes of ocular aberration with accommodation. Am. J. Ophthalmol. 2002, 134, 924–926. [Google Scholar] [CrossRef]
- Yang, Y.; Thompson, K.; Burns, S.A. Pupil Location under Mesopic, Photopic, and Pharmacologically Dilated Conditions. Investig. Ophthalmol. Vis. Sci. 2002, 43, 2508–2512. [Google Scholar]
- Lundström, L.; Unsbo, P. Transformation of Zernike coefficients: Scaled, translated, and rotated wavefronts with circular and elliptical pupils. J. Opt. Soc. Am. A Opt. Image Sci. Vis. 2007, 24, 569–577. [Google Scholar] [CrossRef]
- Sarkar, S.; Hasnat, A.M.; Bharadwaj, S.R. Revisiting the impact of phenylephrine hydrochloride on static and dynamic accommodation. Indian J. Ophthalmol. 2012, 60, 503–509. [Google Scholar]
- Del Águila-Carrasco, A.J.; Lara, F.; Bernal-Molina, P.; Riquelme-Nicolás, R.; Marín-Franch, I.; Esteve-Taboada, J.J.; Montés-Micó, R.; Kruger, P.B.; López-Gil, N. Effect of phenylephrine on static and dynamic accommodation. J. Optom. 2019, 12, 30–37. [Google Scholar] [CrossRef]
- Carkeet, A.; Velaedan, S.; Tan, Y.K.; Lee, D.Y.J.; Tan, D.T.H. Higher order ocular aberrations after cycloplegic and non-cycloplegic pupil dilation. J. Refract. Surg. 2003, 19, 316–322. [Google Scholar] [CrossRef] [PubMed]
- Mordi, J.A.; Lyle, W.M.; Mousa, G.Y. Effect of Phenylephrine on Accommodation. Optom. Vis. Sci. 1986, 63, 294–297. [Google Scholar] [CrossRef] [PubMed]
- Jankov, M.R.; Iseli, H.P.; Bueeler, M.; Schor, P.; Seiler, T.; Mrochen, M. The effect of phenylephrine and cyclopentolate on objective wavefront measurements. J. Refract. Surg. 2006, 22, 472–481. [Google Scholar] [CrossRef] [PubMed]
- He, L.; Applegate, R.A. Predicting crystalline lens fall caused by accommodation from changes in wavefront error. J. Cataract. Refract. Surg. 2011, 37, 1313–1322. [Google Scholar] [CrossRef]
- Atchison, D.A.; Collins, M.J.; Wildsoet, C.F.; Christensen, J.; Waterworth, M.D. Measurement of monochromatic ocular aberrations of human eyes as a function of accommodation by the Howland aberroscope technique. Vision Res. 1995, 35, 313–323. [Google Scholar] [CrossRef] [PubMed]
- Radhakrishnan, H.; Charman, W.N. Age-related changes in ocular aberrations with accommodation. J. Vis. 2007, 7, 1–21. [Google Scholar] [CrossRef] [PubMed]
- Plainis, S.; Ginis, H.S.; Pallikaris, A. The effect of ocular aberrations on steady-state errors of accommodative response. J. Vis. 2005, 5, 466–477. [Google Scholar] [CrossRef] [PubMed]
- Jenkins, T.C. Aberrations of the eye and their effects on vision: 1. Spherical aberration. Br. J. Physiol. Opt. 1963, 20, 59–91. [Google Scholar]
- Atchison, D.A. Accommodation and presbyopia. Ophthalmic. Physiol. Opt. 1995, 15, 255–272. [Google Scholar] [CrossRef]
- Cheng, H.; Barnett, J.K.; Vilupuru, A.S.; Marsack, J.D.; Kasthurirangan, S.; Applegate, R.A.; Roorda, A. A population study on changes in wave aberrations with accommodation. J. Vis. 2004, 4, 3. [Google Scholar] [CrossRef] [PubMed]
- López-Gil, N.; Rucker, F.J.; Stark, L.R.; Badar, M.; Borgovan, T.; Burke, S.; Kruger, P.B. Effect of third-order aberrations on dynamic accommodation. Vis. Res. 2007, 47, 755–765. [Google Scholar] [CrossRef] [PubMed]
- Vilupuru, A.S.; Roorda, A.; Glasser, A. Spatially variant changes in lens power during ocular accommodation in a rhesus monkey eye. J. Vis. 2004, 4, 299–309. [Google Scholar] [CrossRef] [PubMed]
- Roorda, A.; Glasser, A. Wave aberrations of the isolated crystalline lens. J. Vis. 2004, 4, 1. [Google Scholar] [CrossRef]
- Koomen, M.; Tousey, R.; Scolnik, R. The spherical aberration of the eye. JOSA 1949, 39, 370–376. [Google Scholar] [CrossRef]



| Accommodative Demand (D) | p-Value C (2, 0) | p-Value C (3, −1) | p-Value C (3, 1) | p-Value C (4, 0) | p-Value C (6, 0) |
|---|---|---|---|---|---|
| 0 | 1.0 | 0.13 | 0.88 | 0.22 | 0.09 |
| 0.5 | 0.35 | 0.33 | 0.41 | 0.45 | 0.04 * |
| 1.0 | 0.09 | 0.24 | 0.50 | 0.38 | 0.06 |
| 1.5 | 0.28 | 0.08 | 0.96 | 0.24 | 0.01 * |
| 2.0 | 0.15 | 0.09 | 0.35 | 0.14 | 0.02 * |
| 2.5 | 0.41 | 0.05 | 0.35 | 0.09 | 0.08 |
| 3.0 | 0.25 | 0.07 | 0.11 | 0.02 * | 0.01 * |
| 3.5 | 0.36 | 0.01 * | 0.16 | <0.001 * | <0.001 * |
| 4.0 | 0.55 | 0.02 * | 0.02§ | 0.02 * | 0.02 * |
| 4.5 | 0.77 | 0.02 * | 0.01§ | 0.02 * | 0.01 * |
| 5.0 | 0.83 | 0.01 * | 0.02§ | 0.02 * | 0.01 * |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mechó-García, M.; Blanco-Martínez, I.; Fernandes, P.; Macedo-de-Araújo, R.J.; Faria-Ribeiro, M.; González-Méijome, J.M. Changes in Wavefront Error of the Eye for Different Accommodation Targets under the Application of Phenylephrine Hydrochloride. Photonics 2023, 10, 381. https://doi.org/10.3390/photonics10040381
Mechó-García M, Blanco-Martínez I, Fernandes P, Macedo-de-Araújo RJ, Faria-Ribeiro M, González-Méijome JM. Changes in Wavefront Error of the Eye for Different Accommodation Targets under the Application of Phenylephrine Hydrochloride. Photonics. 2023; 10(4):381. https://doi.org/10.3390/photonics10040381
Chicago/Turabian StyleMechó-García, María, Iñaki Blanco-Martínez, Paulo Fernandes, Rute J. Macedo-de-Araújo, Miguel Faria-Ribeiro, and José Manuel González-Méijome. 2023. "Changes in Wavefront Error of the Eye for Different Accommodation Targets under the Application of Phenylephrine Hydrochloride" Photonics 10, no. 4: 381. https://doi.org/10.3390/photonics10040381
APA StyleMechó-García, M., Blanco-Martínez, I., Fernandes, P., Macedo-de-Araújo, R. J., Faria-Ribeiro, M., & González-Méijome, J. M. (2023). Changes in Wavefront Error of the Eye for Different Accommodation Targets under the Application of Phenylephrine Hydrochloride. Photonics, 10(4), 381. https://doi.org/10.3390/photonics10040381






