Dynamics of the Femtosecond Mid-IR Laser Pulse Impact on a Bulk Silicon
Abstract
1. Introduction
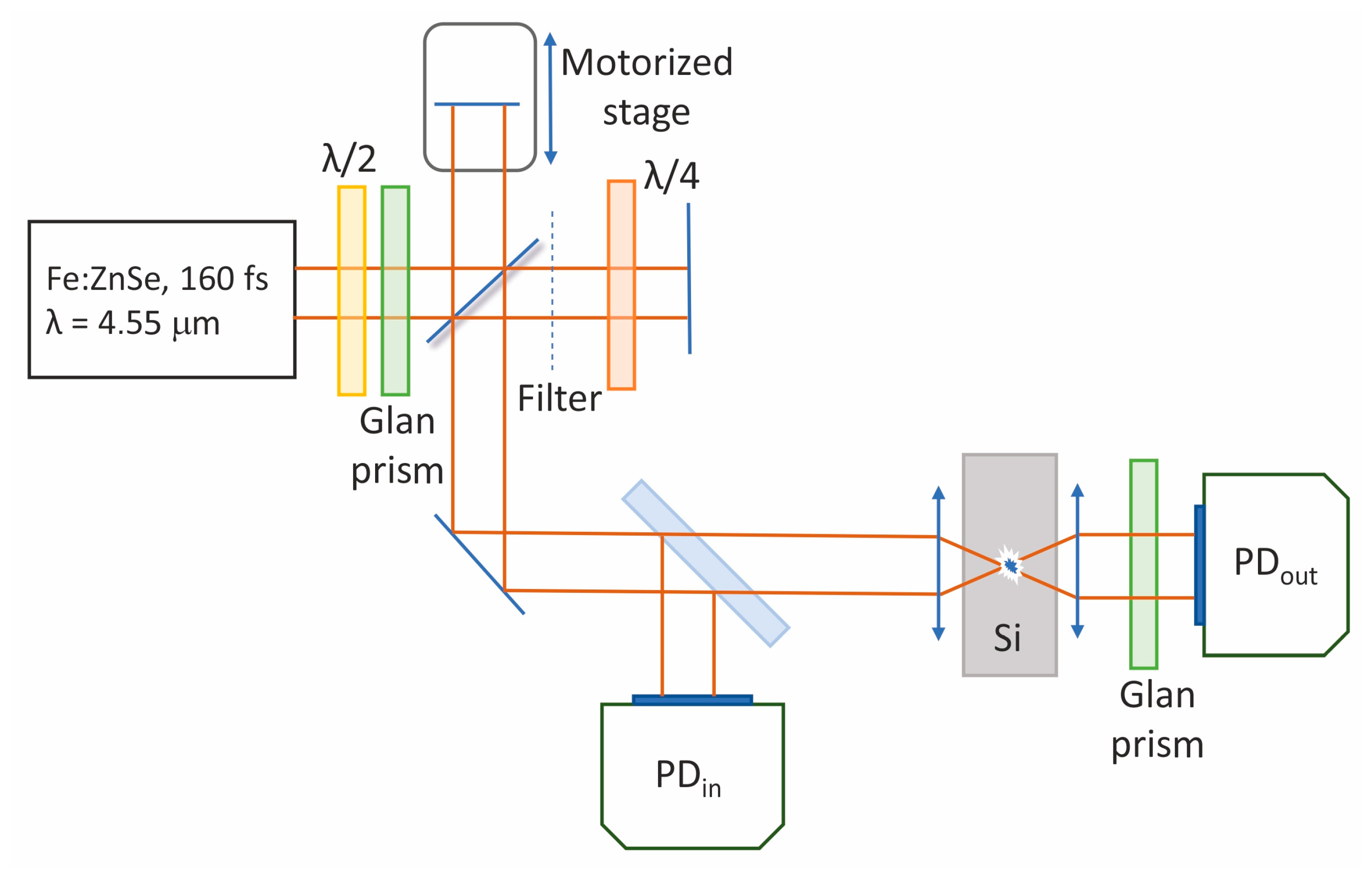
2. Materials and Methods
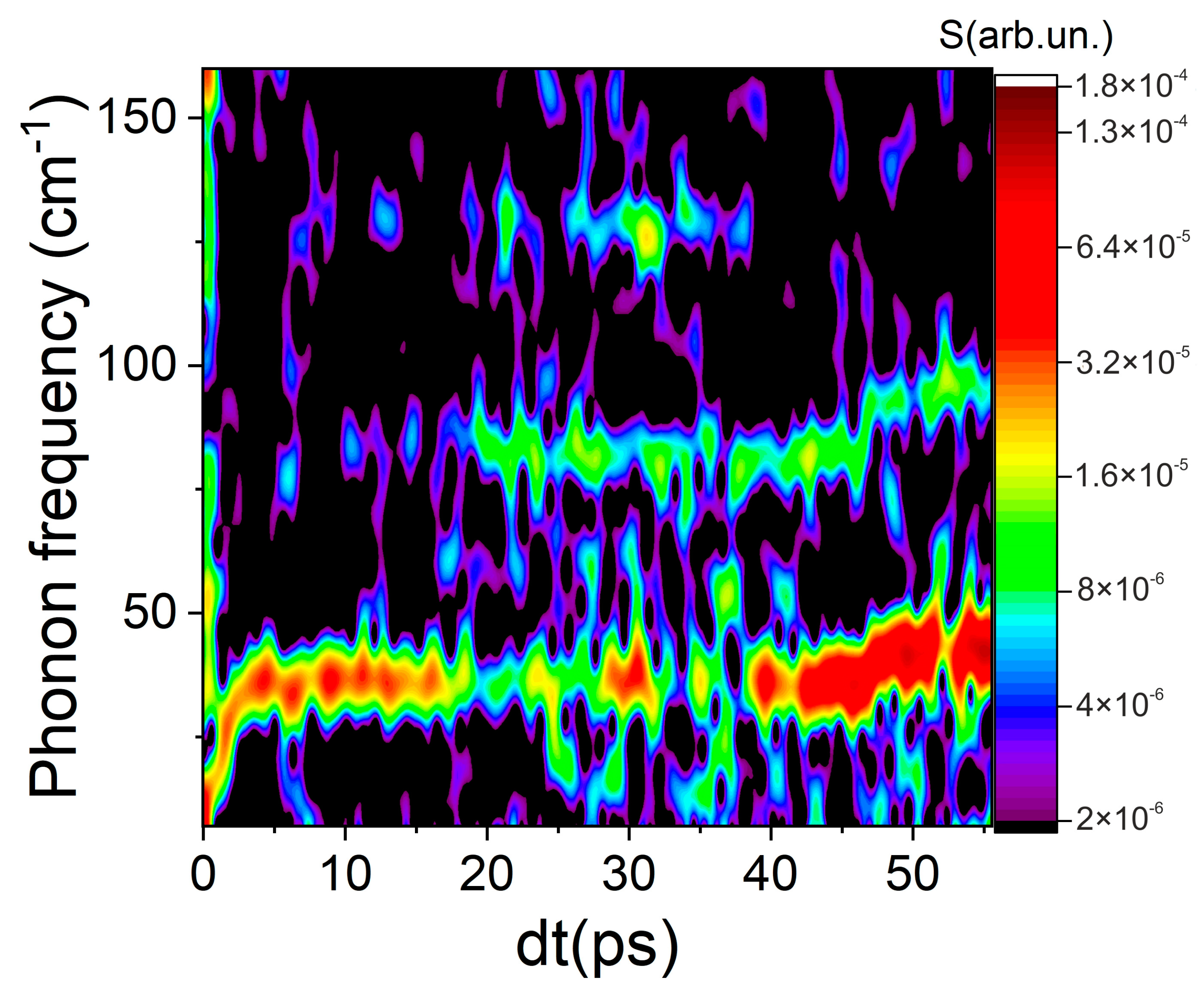
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Leuthold, J.; Koos, C.; Freude, W. Nonlinear silicon photonics. Nat. Photonics 2010, 4, 535–544. [Google Scholar] [CrossRef]
- Bogaerts, W.; Chrostowski, L. Silicon Photonics Circuit Design: Methods, Tools and Challenges. Laser Photonics Rev. 2018, 12, 1700237. [Google Scholar] [CrossRef]
- Schaffer, C.B.; Mazur, E. Laser-induced breakdown and damage in bulk transparent materials induced by tightly focused femtosecond laser pulses. Meas. Sci. Technol. 2001, 12, 1784–1794. [Google Scholar] [CrossRef]
- Fernandez, T.T.; Johnston, B.; Gross, S.; Cozic, S.; Poulain, M.; Mahmodi, H.; Kabakova, I.; Withford, M.; Fuerbach, A. Ultrafast laser inscribed waveguides in tailored fluoride glasses: An enabling technology for mid-infrared integrated photonics devices. Sci. Rep. 2022, 12, 14674. [Google Scholar] [CrossRef] [PubMed]
- Zavedeev, E.V.; Kononenko, V.V.; Konov, V.I. Delocalization of femtosecond laser radiation in crystalline Si in the mid-IR range. Laser Phys. 2016, 26, 016101. [Google Scholar] [CrossRef]
- Chanal, M.; Fedorov, V.Y.; Chambonneau, M.; Tzortzakis, S.; Grojo, D.; Clady, R. Crossing the threshold of ultrafast laser writing in bulk silicon. Nat. Commun. 2018, 8, 773. [Google Scholar] [CrossRef] [PubMed]
- Grojo, D.; Leyder, S.; Delaporte, P.; Marine, W.; Sentis, M.; Utéza, O. Long-wavelength multiphoton ionization inside band-gap solids. Phys. Rev. B-Condens. Matter Mater. Phys. 2013, 88, 195135. [Google Scholar] [CrossRef]
- Mareev, E.I.; Lvov, K.V.; Rumiantsev, B.V.; Migal, E.A.; Novikov, I.D.; Yu, S.S.; Potemkin, F.V. Effect of pulse duration on the energy delivery under nonlinear propagation of tightly focused Cr: Forsterite laser radiation in bulk silicon. Laser Phys. Lett. 2019, 17, 015402. [Google Scholar] [CrossRef]
- Chambonneau, M.; Grojo, D.; Tokel, O.; Ilday, F.Ö.; Tzortzakis, S.; Nolte, S. In-Volume Laser Direct Writing of Silicon—Challenges and Opportunities. Laser Photonics Rev. 2021, 15, 2100140. [Google Scholar] [CrossRef]
- Garcia-Lechuga, M.; Casquero, N.; Wang, A.; Grojo, D.; Siegel, J. Deep Silicon Amorphization Induced by Femtosecond Laser Pulses up to the Mid-Infrared. Adv. Opt. Mater. 2021, 9, 2100400. [Google Scholar] [CrossRef]
- Mareev, E.; Pushkin, A.; Migal, E.; Lvov, K.; Stremoukhov, S. Single-shot femtosecond bulk micromachining of silicon with mid-IR tightly focused beams. Sci. Rep. 2022, 12, 7517. [Google Scholar] [CrossRef] [PubMed]
- Chambonneau, M.; Lavoute, L.; Gaponov, D.; Fedorov, V.Y.; Hideur, A.; Février, S.; Tzortzakis, S.; Utéza, O.; Grojo, D. Competing nonlinear delocalization of light for laser inscription inside silicon with a 2-μm picosecond laser. Phys. Rev. Appl. 2019, 12, 024009. [Google Scholar] [CrossRef]
- Wang, A.; Das, A.; Grojo, D. Temporal-contrast imperfections as drivers for ultrafast laser modifications in bulk silicon. Phys. Rev. Res. 2020, 2, 033023. [Google Scholar] [CrossRef]
- Mcbride, E.E.; Krygier, A.; Ehnes, A.; Galtier, E.; Harmand, M.; Kon, Z. Phase Transition Lowering in Dynamically-Compressed Silicon. Nat. Phys. 2019, 15, 89–94. [Google Scholar] [CrossRef]
- Brown, S.B.; Gleason, A.E.; Galtier, E.; Higginbotham, A.; Arnold, B.; Fry, A.; Granados, E.; Hashim, A.; Schroer, C.G.; Schropp, A.; et al. Direct imaging of ultrafast lattice dynamics. Sci. Adv. 2019, 5, 8044. [Google Scholar] [CrossRef]
- Zeng, Z.; Zeng, Q.; Mao, W.L.; Qu, S. Phase transitions in metastable phases of silicon. J. Appl. Phys. 2014, 115, 103514. [Google Scholar] [CrossRef]
- Gogotsi, Y.; Baek, C.; Kirscht, F. Raman microspectroscopy study of processing-induced phase transformations and residual stress in silicon. Semicond. Sci. Technol. 1999, 14, 936–944. [Google Scholar] [CrossRef]
- Gorman, M.G.; Briggs, R.; McBride, E.E.; Higginbotham, A.; Arnold, B.; Eggert, J.H.; Fratanduono, D.E.; Galtier, E.; Lazicki, A.E.; Lee, H.J.; et al. Direct Observation of Melting in Shock-Compressed Bismuth with Femtosecond X-ray Diffraction. Phys. Rev. Lett. 2015, 115, 095701. [Google Scholar] [CrossRef]
- Wall, S.; Wegkamp, D.; Foglia, L.; Appavoo, K.; Nag, J.; Haglund, R.F.; StäCurrency Signhler, J.; Wolf, M. Ultrafast changes in lattice symmetry probed by coherent phonons. Nat. Commun. 2012, 3, 721. [Google Scholar] [CrossRef]
- Mareev, E.; Potemkin, F. Dynamics of ultrafast phase transitions in MgF2 triggered by laser-induced THz coherent phonons. Sci. Rep. 2022, 12, 6621. [Google Scholar] [CrossRef]
- Pawbake, A.; Bellin, C.; Paulatto, L.; Béneut, K.; Biscaras, J.; Narayana, C.; Late, D.J.; Shukla, A. Pressure-induced phase transitions in Germanium telluride: Raman signatures of anharmonicity and oxidation. Phys. Rev. Lett. 2019, 122, 145701. [Google Scholar] [CrossRef] [PubMed]
- Taylor, L.L.; Scott, R.E.; Qiao, J. Integrating two-temperature and classical heat accumulation models to predict femtosecond laser processing of silicon. Opt. Mater. Express 2018, 8, 648. [Google Scholar] [CrossRef]
- Tanimura, H.; Kanasaki, J.; Tanimura, K.; Sjakste, J.; Vast, N. Ultrafast relaxation dynamics of highly excited hot electrons in silicon. Phys. Rev. B 2019, 100, 035201. [Google Scholar] [CrossRef]
- Zijlstra, E.S.; Walkenhorst, J.; Garcia, M.E. Anharmonic noninertial lattice dynamics during ultrafast nonthermal melting of InSb. Phys. Rev. Lett. 2008, 101, 135701. [Google Scholar] [CrossRef] [PubMed]
- Tsujino, M.; Sano, T.; Ozaki, N.; Sakata, O.; Okoshi, M.; Inoue, N.; Kodama, R.; Hirose, A. Quenching of High-Pressure Phases of Silicon Using Femtosecond Laser-driven Shock Wave. Rev. Laser Eng. 2008, 36, 1218–1221. [Google Scholar] [CrossRef]
- Mareev, E.I.; Rumiantsev, B.V.; Migal, E.; Bychkov, A.S.; Karabutov, A.A.; Cherepetskaya, E.B.; Makarov, V.A.; Potemkin, F.V. A comprehensive approach for characterisation of the deposited energy density during laser-matter interaction in liquids and solids. Meas. Sci. Technol. 2020, 31, 085204. [Google Scholar] [CrossRef]
- Wang, A.; Das, A.; Hermann, J.; Grojo, D. Three-dimensional luminescence microscopy for quantitative plasma characterization in bulk semiconductors. Appl. Phys. Lett. 2021, 119, 041108. [Google Scholar] [CrossRef]
- Migal, E.; Mareev, E.; Smetanina, E.; Duchateau, G.; Potemkin, F. Role of wavelength in photocarrier absorption and plasma formation threshold under excitation of dielectrics by high-intensity laser field tunable from visible to mid-IR. Sci. Rep. 2020, 10, 14007. [Google Scholar] [CrossRef]
- Potemkin, F.V.; Mareev, E.I.; Mikheev, P.M.; Khodakovskij, N.G. Resonant laser-plasma excitation of coherent THz phonons under extreme conditions of femtosecond plasma formation in a bulk of fluorine-containing crystals. Laser Phys. Lett. 2013, 10, 076003. [Google Scholar] [CrossRef]
- Mareev, E.I.; Migal, E.A.; Potemkin, F.V. Ultrafast third harmonic generation imaging of microplasma at the threshold of laser-induced plasma formation in solids. Appl. Phys. Lett. 2019, 114, 031106. [Google Scholar] [CrossRef]
- Potemkin, F.V.; Mareev, E.I.; Mikheev, P.M.; Khodakovskij, N.G. Resonance laser-plasma excitation of coherent terahertz phonons in the bulk of fluorine-bearing crystals under high-intensity femtosecond laser irradiation. Quantum Electron. 2013, 43, 735–739. [Google Scholar] [CrossRef]
- Seres, E.; Seres, J.; Spielmann, C. Time resolved spectroscopy with femtosecond soft-x-ray pulses. Appl. Phys. A Mater. Sci. Process. 2009, 96, 43–50. [Google Scholar] [CrossRef]
- Wippermann, S.; He, Y.; Vörös, M.; Galli, G. Novel silicon phases and nanostructures for solar energy conversion. Appl. Phys. Rev. 2016, 3, 040807. [Google Scholar] [CrossRef]
- Lewis, S.P.; Cohen, M.L. Theoretical study of Raman modes in high-pressure phases of Si, Ge, and Sn. Phys. Rev. B 1993, 48, 3646–3653. [Google Scholar] [CrossRef] [PubMed]
- Mareev, E.I.; Potemkin, F.V. Dynamics of Ultrafast Phase Transitions in (001) Si on the Shock-Wave Front. Int. J. Mol. Sci. 2022, 23, 2115. [Google Scholar] [CrossRef]
- Paul, R.; Hu, S.X.; Karasiev, V.V. Crystalline phase transitions and vibrational spectra of silicon up to multiterapascal pressures. Phys. Rev. B 2019, 100, 144101. [Google Scholar] [CrossRef]
- Domnich, V.; Gogotsi, Y. Phase transformations in silicon under contact loading. Rev. Adv. Mater. Sci. 2002, 3, 1–36. [Google Scholar]
- Gaál-Nagy, K.; Strauch, D. Phonons in the β-tin, Imma, and sh phases of silicon from ab initio calculations. Phys. Rev. B-Condens. Matter Mater. Phys. 2006, 73, 014117. [Google Scholar] [CrossRef]
- Mareev, E.I.; Rumiantsev, B.V.; Potemkin, F.V. Study of the Parameters of Laser-Induced Shock Waves for Laser Shock Peening of Silicon. JETP Lett. 2020, 112, 739–744. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mareev, E.; Obydennov, N.; Potemkin, F. Dynamics of the Femtosecond Mid-IR Laser Pulse Impact on a Bulk Silicon. Photonics 2023, 10, 380. https://doi.org/10.3390/photonics10040380
Mareev E, Obydennov N, Potemkin F. Dynamics of the Femtosecond Mid-IR Laser Pulse Impact on a Bulk Silicon. Photonics. 2023; 10(4):380. https://doi.org/10.3390/photonics10040380
Chicago/Turabian StyleMareev, Evgenii, Nikolay Obydennov, and Fedor Potemkin. 2023. "Dynamics of the Femtosecond Mid-IR Laser Pulse Impact on a Bulk Silicon" Photonics 10, no. 4: 380. https://doi.org/10.3390/photonics10040380
APA StyleMareev, E., Obydennov, N., & Potemkin, F. (2023). Dynamics of the Femtosecond Mid-IR Laser Pulse Impact on a Bulk Silicon. Photonics, 10(4), 380. https://doi.org/10.3390/photonics10040380




