Key Space Enhancement of Chaos Communication Using Semiconductor Lasers with Spectrum-Programmable Optoelectronic Feedback
Abstract
1. Introduction
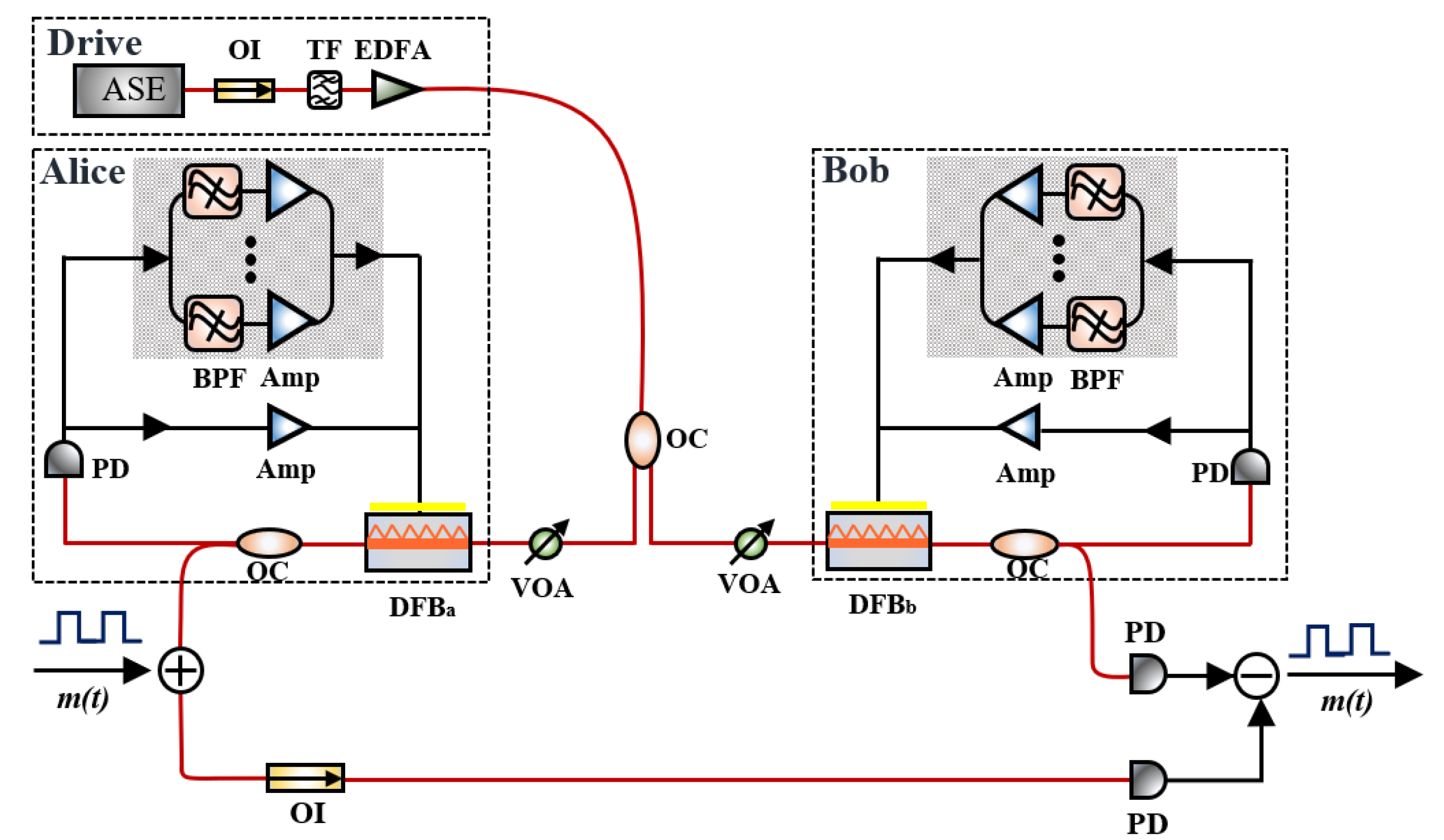
2. Theoretical Model
3. Simulation Results
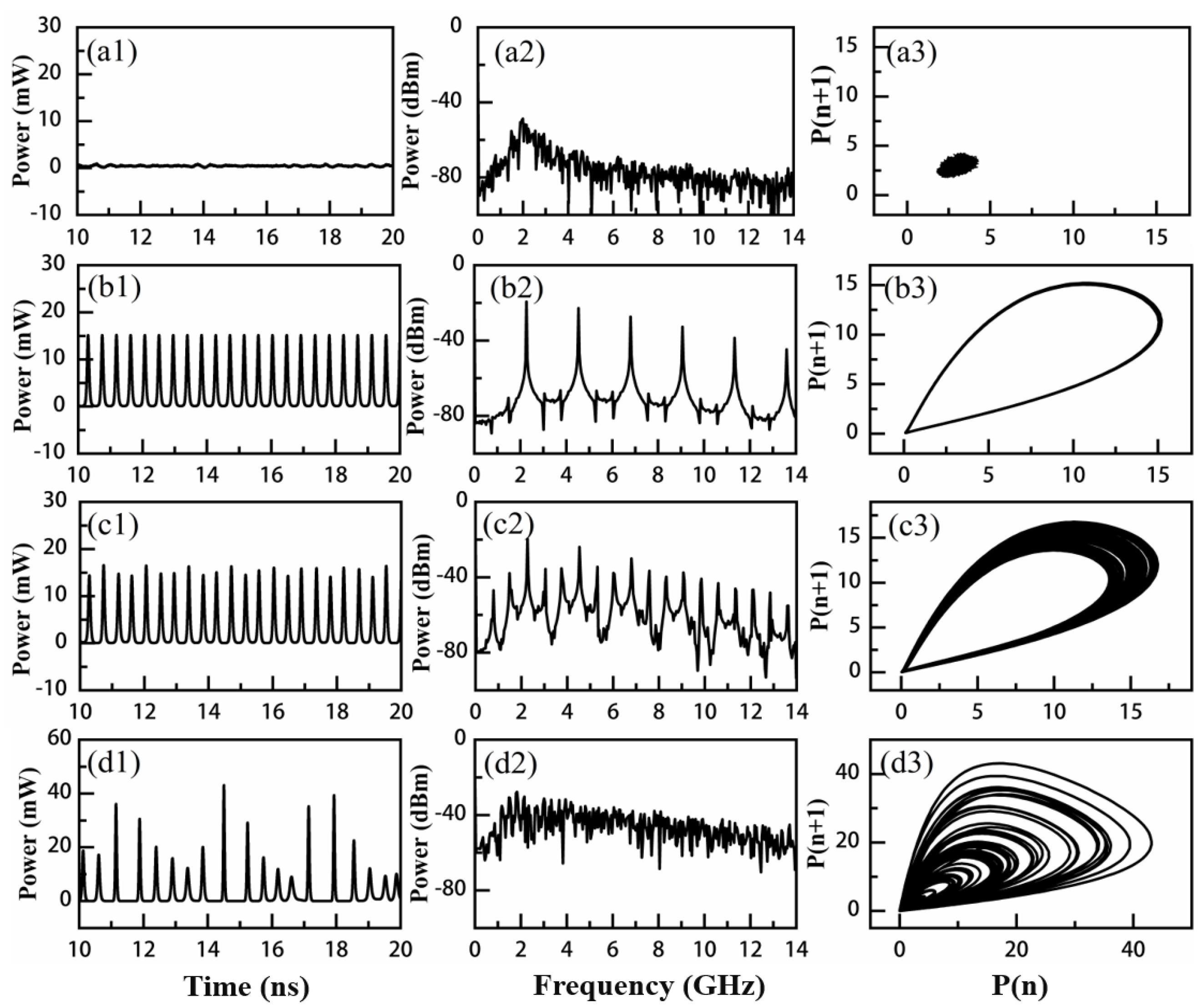
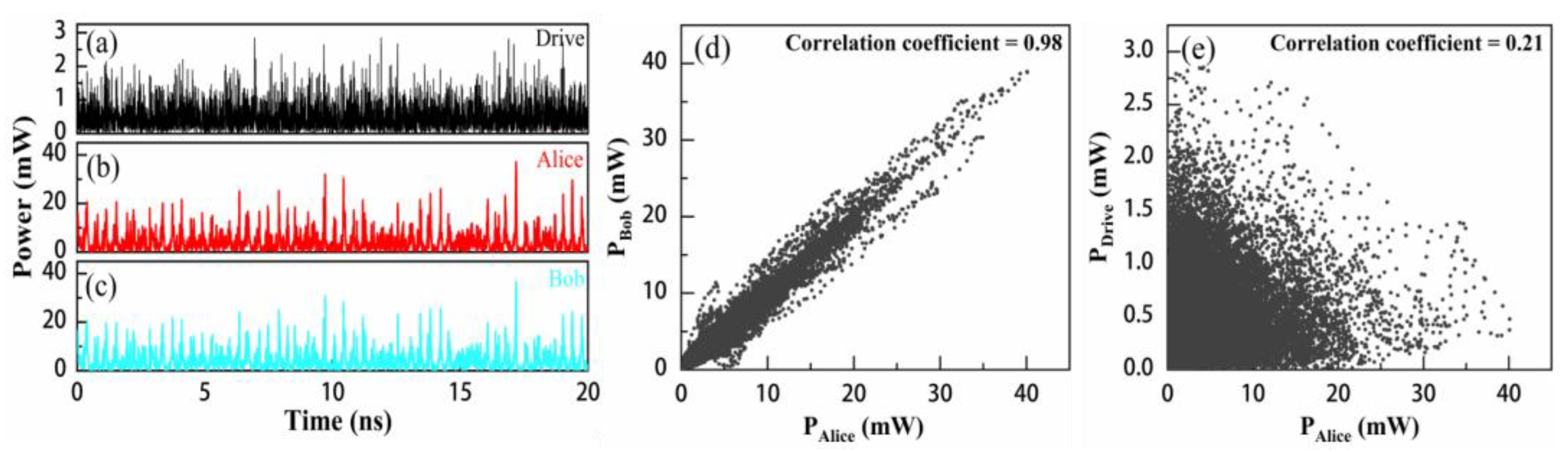
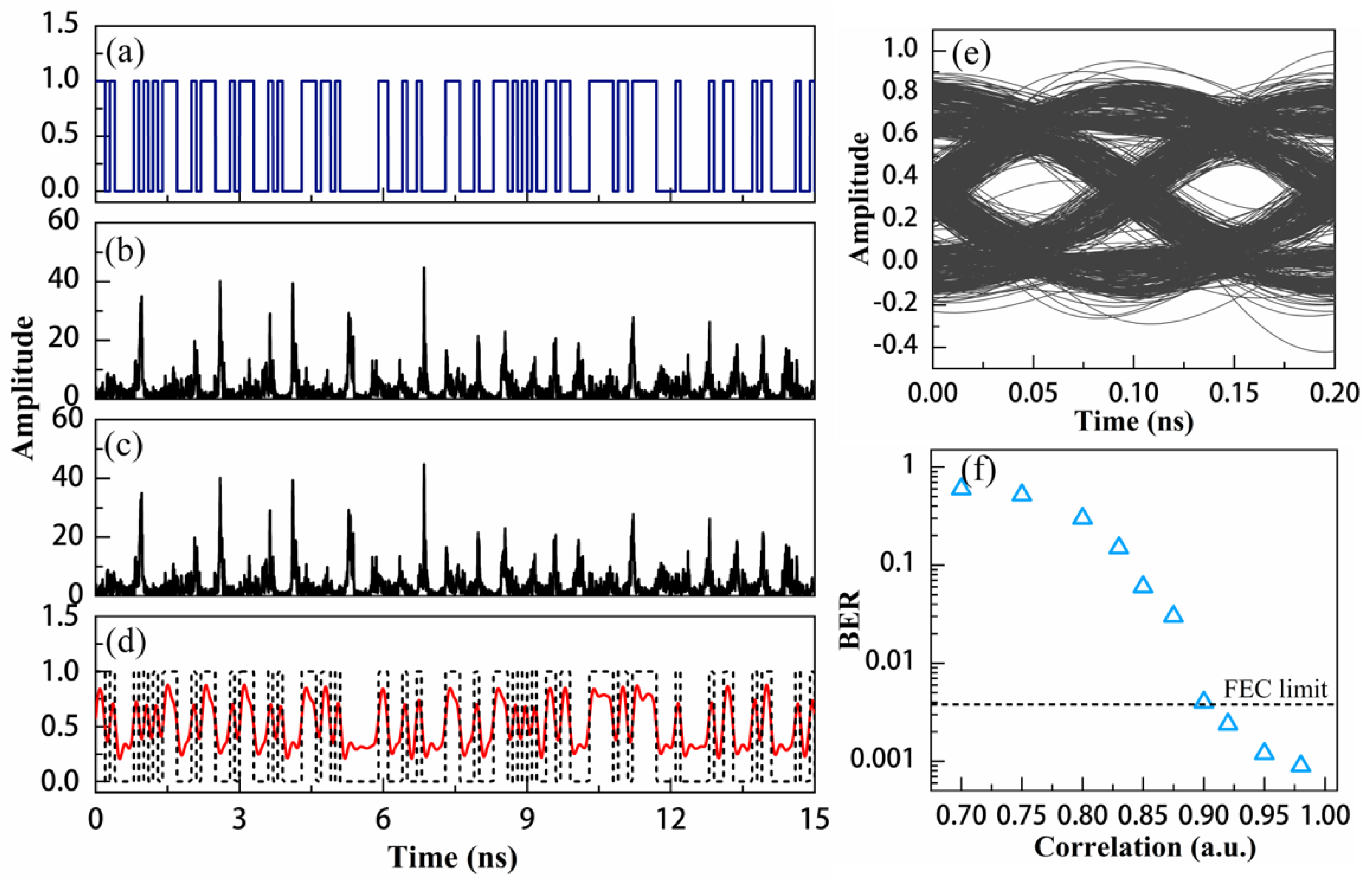
3.1. Chaos Generation and Synchronization
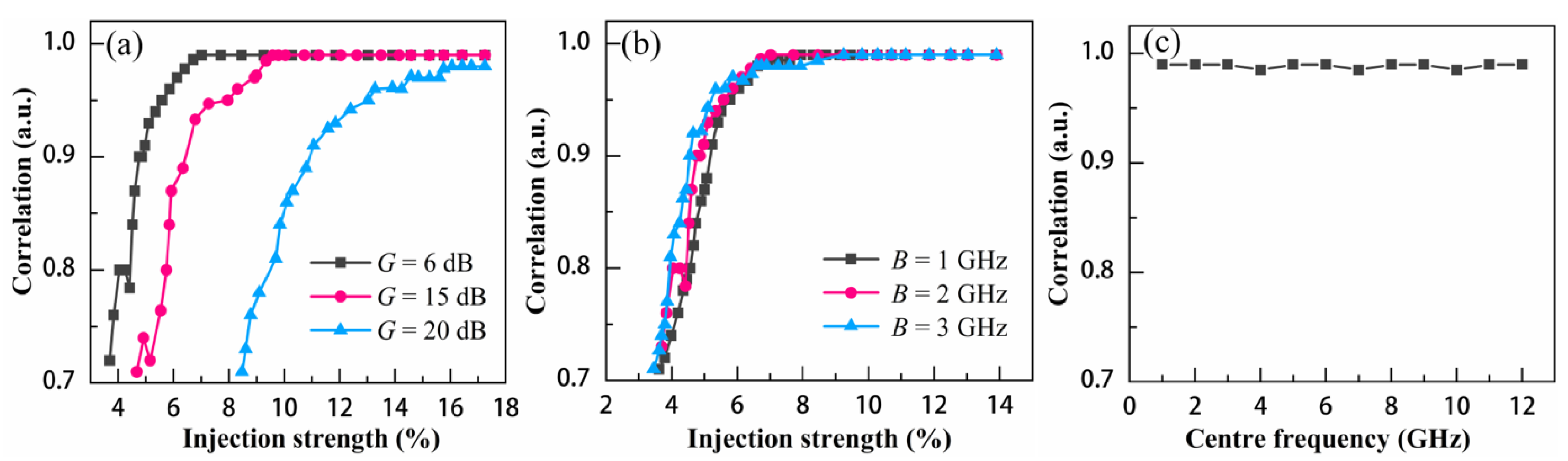
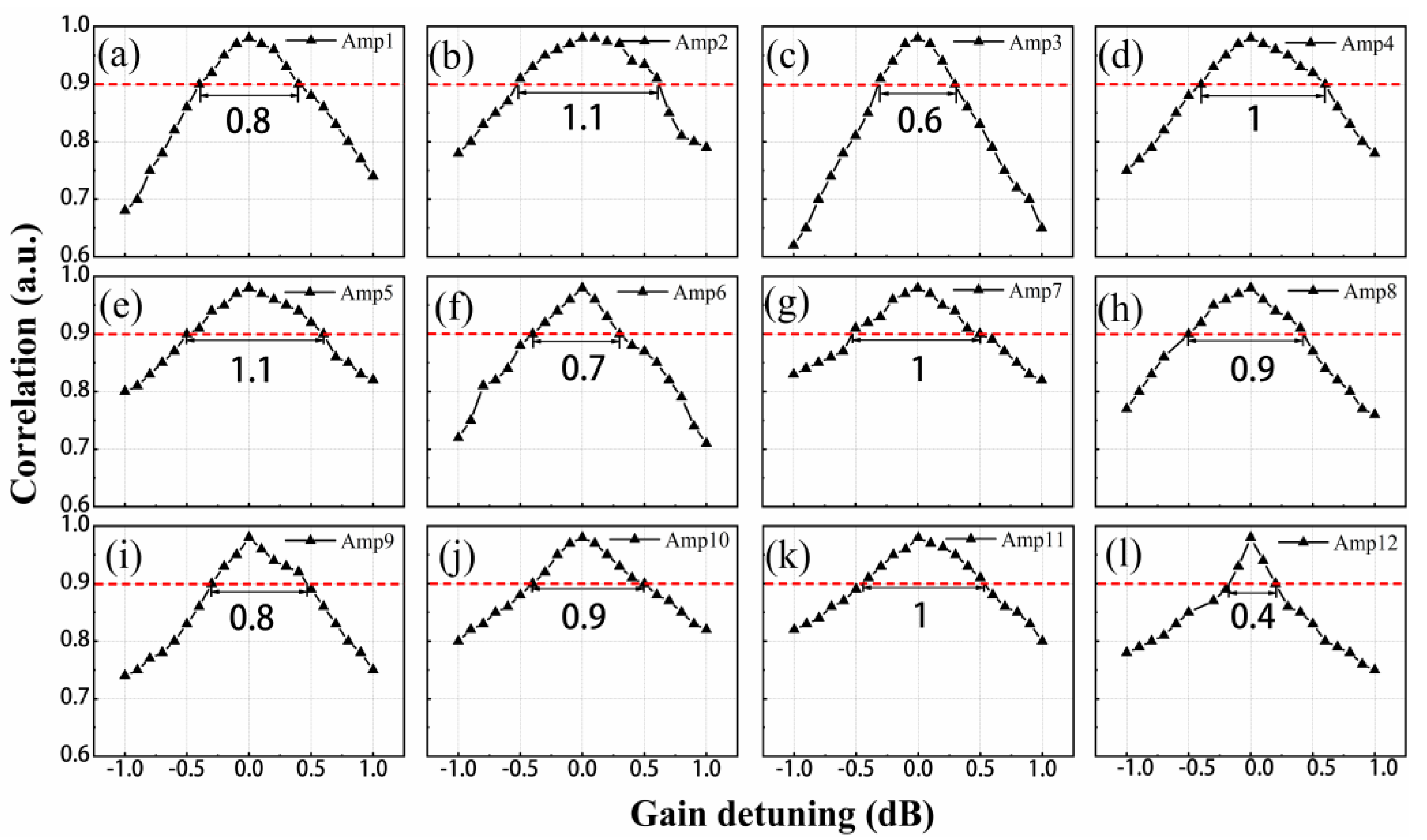
3.2. Physical Key Space Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Vanwiggeren, G.D.; Roy, R. Communication with chaotic lasers. Science 1998, 279, 1198–1200. [Google Scholar] [CrossRef] [PubMed]
- Argyris, A.; Syvridis, D.; Larger, L.; Annovazzi-Lodi, V.; Colet, P.; Fischer, I.; García-Ojalvo, J.; Mirasso, C.R.; Pesquera, L.; Shore, K.A. Chaos-based communications at high bit rates using commercial fibre-optic links. Nature 2005, 438, 343–346. [Google Scholar] [CrossRef]
- Lavrov, R.; Jacquot, M.; Larger, L. Nonlocal nonlinear electro-optic phase dynamics demonstrating 10 Gb/s chaos communications. IEEE J. Quantum Electron. 2010, 46, 1430–1435. [Google Scholar] [CrossRef]
- Argyris, A.; Grivas, E.; Hamacher, M.; Bogris, A.; Syvridis, D. Chaos-on-a-chip secures data transmission in optical fiber links. Opt. Express 2010, 18, 5188–5198. [Google Scholar] [CrossRef] [PubMed]
- Ke, J.X.; Yi, L.L.; Xia, G.Q.; Hu, W.S. Chaotic optical communications over 100-km fiber transmission at 30-Gb/s bit rate. Opt. Lett. 2018, 43, 1323–1326. [Google Scholar] [CrossRef]
- Wang, L.S.; Mao, X.X.; Wang, A.B.; Wang, Y.C.; Gao, Z.S.; Li, S.S.; Yan, L.S. Scheme of coherent optical chaos communication. Opt. Lett. 2020, 45, 4762–4765. [Google Scholar] [CrossRef]
- Yang, Z.; Ke, J.X.; Zhuge, Q.B.; Hu, W.S.; Yi, L.L. Coherent chaotic optical communication of 30 Gb/s over 340-km fiber transmission via deep leaning. Opt. Lett. 2022, 47, 2650–2653. [Google Scholar] [CrossRef]
- Jiang, L.; Feng, J.C.; Yan, L.S.; Yi, A.L.; Li, S.S.; Yang, H.; Dong, Y.X.; Wang, L.S.; Wang, A.B.; Wang, Y.C.; et al. Chaotic optical communications at 56 Gbit/s over 100-km fiber transmission based on a chaos generation model driven by long short-term memory networks. Opt. Lett. 2022, 47, 2382–2385. [Google Scholar] [CrossRef]
- Gao, Z.S.; Wu, Q.Q.; Liao, L.; Su, B.; Gao, X.L.; Fu, S.N.; Li, Z.H.; Wang, Y.C.; Qin, Y.W. Experimental demonstration of synchronous privacy enhanced chaotic temporal phase en/decryption for high speed secure optical communication. Opt. Express 2022, 30, 31209–31219. [Google Scholar] [CrossRef]
- Xiang, S.; Yang, M.; Wang, J. Chaotic optical communications of 12.5-Gbaud OOK and 10-Gbaud QPSK signals based on mutual injection of semiconductor lasers. Opt. Lett. 2022, 47, 2818–2821. [Google Scholar] [CrossRef]
- Wang, Y.; Huang, Y.; Zhou, P.; Li, N. Dual-Channel Secure Communication Based on Wideband Optical Chaos in Semiconductor Lasers Subject to Intensity Modulation Optical Injection. Electronics 2023, 12, 509. [Google Scholar] [CrossRef]
- Wang, L.; Chen, X.; Mao, X.; Jiang, L.; Li, S.; Sun, Y.; Wang, Y.; Yan, L.; Wang, A. Performance improvement of coherent optical chaos communication using probabilistic shaping. Opt. Lett. 2023, 48, 1008–1011. [Google Scholar] [CrossRef] [PubMed]
- Jiang, L.; Pan, Y.; Yi, A.L.; Feng, J.C.; Pan, W.; Yi, L.L.; Hu, W.S.; Wang, A.B.; Wang, Y.C.; Qin, Y.W.; et al. Trading off security and practicability to explore high-speed and long-haul chaotic optical communication. Opt. Express 2021, 29, 12750–12762. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.F.; Liu, J.M. Open-loop chaotic synchronization of injection-locked semiconductor lasers with gigahertz range modulation. IEEE J. Quantum Electron. 2000, 36, 27–34. [Google Scholar] [CrossRef]
- Ohtsubo, J. Chaos synchronization and chaotic signal masking in semiconductor lasers with optical feedback. IEEE J. Quantum Electron. 2002, 38, 1141–1154. [Google Scholar] [CrossRef]
- Murakami, A.; Ohtsubo, J. Synchronization of feedback-induced chaos in semiconductor lasers by optical injection. Phys. Rev. A 2002, 65, 031802. [Google Scholar] [CrossRef]
- Wang, D.M.; Wang, L.S.; Guo, Y.Y.; Wang, Y.C.; Wang, A.B. Key space enhancement of optical chaos secure communication: Chirped FBG feedback semiconductor laser. Opt. Express 2019, 27, 3065–3073. [Google Scholar] [CrossRef]
- Hou, T.T.; Yi, L.L.; Yang, X.L.; Ke, J.X.; Hu, Y.; Yang, Q.; Zhou, P.; Hu, W.S. Maximizing the security of chaotic optical communications. Opt. Express 2016, 24, 23439–23449. [Google Scholar] [CrossRef]
- Wang, D.M.; Wang, L.S.; Li, P.; Zhao, T.; Jia, Z.W.; Gao, Z.S.; Guo, Y.Y.; Wang, Y.C.; Wang, A.B. Bias current of semiconductor laser: An unsafe key for secure chaos communication. Photonics 2019, 6, 59. [Google Scholar] [CrossRef]
- Wang, H.X.; Lu, T.F.; Ji, Y.F. Key space enhancement of a chaos secure communication based on VCSELs with a common phase-modulated electro-optic feedback. Opt. Express 2020, 28, 23961–23977. [Google Scholar] [CrossRef]
- Gao, Z.S.; Li, Q.H.; Zhang, L.H.; Tang, B.; Luo, Y.; Gao, X.L.; Fu, S.N.; Li, Z.H.; Wang, Y.C.; Qin, Y.W. 32 Gb/s physical-layer secure optical communication over 200 km based on temporal dispersion and self-feedback phase encryption. Opt. Lett. 2022, 47, 913–916. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.; Wang, Y.; Sun, Y.; Xu, J.; Li, P.; Wang, A.; Qin, Y. Key Space Enhancement in Chaotic Secure Communication Utilizing Monolithically Integrated Multi-Section Semiconductor Lasers. Photonics 2023, 10, 213. [Google Scholar] [CrossRef]
- Argyris, A.; Grivas, E.; Bogris, A.; Syvridis, D. Transmission effects in wavelength division multiplexed chaotic optical communication systems. J. Light. Technol. 2010, 28, 3107–3114. [Google Scholar] [CrossRef]


| Parameters | Values | Units | |
|---|---|---|---|
| ASE noise | Noise frequency of ASE | 193.1 | THz |
| Noise bin spacing of ASE | 3.0 × 1011 | Hz | |
| Filter width of TF | 100 | GHz | |
| DFB laser | Linewidth enhancement factor | 3.0 | -- |
| Group index | 3.7 | -- | |
| Internal loss factor | 3000 | m−1 | |
| Linear gain coefficient | 3.0 × 10−20 | m2 | |
| Nonlinear gain coefficient | 1.0 × 10−23 | m3 | |
| Carrier density at transparency | 1.5 × 1024 | m−3 | |
| Initial carrier density | 1.0 × 1024 | m−3 | |
| Linear recombination coefficient | 3.0 × 108 | s−1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Y.; Wang, D.; Wang, L.; Jia, Z.; Zhao, T.; Chang, P.; Wang, Y.; Wang, A. Key Space Enhancement of Chaos Communication Using Semiconductor Lasers with Spectrum-Programmable Optoelectronic Feedback. Photonics 2023, 10, 370. https://doi.org/10.3390/photonics10040370
Guo Y, Wang D, Wang L, Jia Z, Zhao T, Chang P, Wang Y, Wang A. Key Space Enhancement of Chaos Communication Using Semiconductor Lasers with Spectrum-Programmable Optoelectronic Feedback. Photonics. 2023; 10(4):370. https://doi.org/10.3390/photonics10040370
Chicago/Turabian StyleGuo, Yuanyuan, Dongsheng Wang, Longsheng Wang, Zhiwei Jia, Tong Zhao, Pengfa Chang, Yuncai Wang, and Anbang Wang. 2023. "Key Space Enhancement of Chaos Communication Using Semiconductor Lasers with Spectrum-Programmable Optoelectronic Feedback" Photonics 10, no. 4: 370. https://doi.org/10.3390/photonics10040370
APA StyleGuo, Y., Wang, D., Wang, L., Jia, Z., Zhao, T., Chang, P., Wang, Y., & Wang, A. (2023). Key Space Enhancement of Chaos Communication Using Semiconductor Lasers with Spectrum-Programmable Optoelectronic Feedback. Photonics, 10(4), 370. https://doi.org/10.3390/photonics10040370





